Photonic Crystal Surface Modes for Trapping and Waveguiding of Ultracold Atoms
Abstract
:1. Introduction
2. Materials and Methods
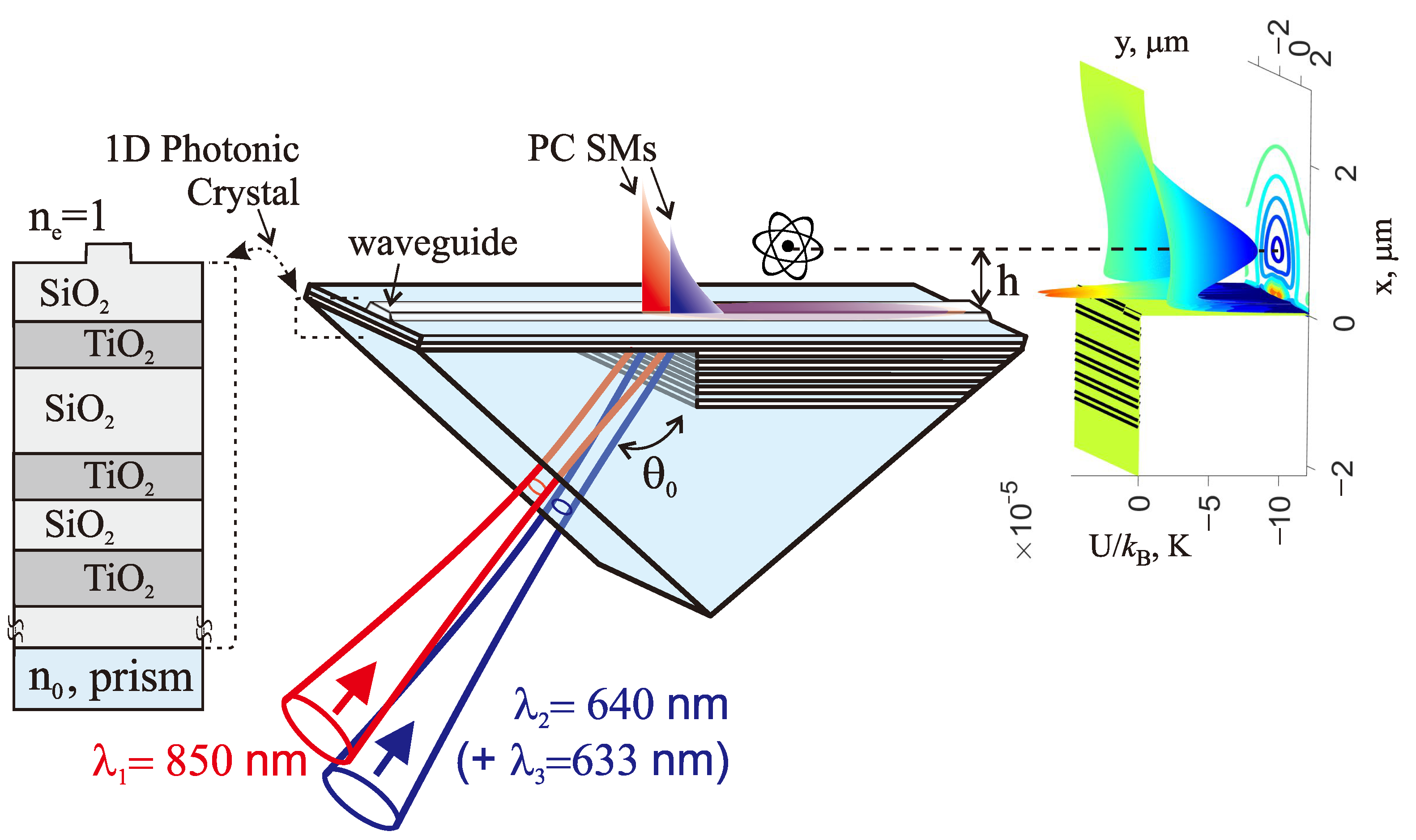
2.1. Planar Photonic Crystal Waveguides and Their Differences from Standard Waveguides
2.2. Design Methodology for a 2D Waveguide Placed on a 1D PC
2.2.1. Design of a 1D PC
2.2.2. From 1D PC Waveguide to 2D Waveguide
2.2.3. Design of a 2D Waveguide Placed on a 1D PC
2.3. Calculating the Optical Dipole Potential at Large Wavelength Detuning
3. Results
3.1. Example of Specification of a 2D Waveguide Placed on a 1D PC
3.2. Optical Trap above the Waveguide Located on the 1D PC
3.3. Techniques to Mitigate Temperature Rise Caused by Optical Absorption in Waveguides
3.4. Excitation of Waveguide Modes in a Kretschmann-like Scheme
4. Discussion
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Bongs, K.; Bennett, S.; Lohmann, A. Quantum sensors will start a revolution—if we deploy them right. Nature 2023, 617, 672–675. [Google Scholar] [CrossRef]
- Bongs, K.; Holynski, M.; Vovrosh, J.; Bouyer, P.; Condon, G.; Rasel, E.; Schubert, C.; Schleich, W.P.; Roura, A. Taking atom interferometric quantum sensors from the laboratory to real-world applications. Nat. Rev. Phys. 2019, 1, 731–739. [Google Scholar]
- Cladé, P.; Guellati-Khélifa, S.; Schwob, C.; Nez, F.; Julien, L.; Biraben, F. A promising method for the measurement of the local acceleration of gravity using Bloch oscillations of ultracold atoms in a vertical standing wave. Europhys. Lett. 2005, 71, 730. [Google Scholar] [CrossRef]
- Ferrari, G.; Poli, N.; Sorrentino, F.; Tino, G. Long-lived Bloch oscillations with bosonic Sr atoms and application to gravity measurement at the micrometer scale. Phys. Rev. Lett. 2006, 97, 060402. [Google Scholar] [CrossRef]
- Tino, G.M. Testing gravity with cold atom interferometry: Results and prospects. Quantum Sci. Technol. 2021, 6, 024014. [Google Scholar]
- Bolotin, Y.; Golovan, A.; Vyazmin, V.; Evstifeev, M.; Vershovskii, A. Advanced gravity field survey methods. In Methods and Technologies for Measuring the Earth’s Gravity Field Parameters; Springer: Berlin/Heidelberg, Germany, 2022; pp. 237–301. [Google Scholar]
- Ge, G.; Chen, X.; Li, J.; Zhang, D.; He, M.; Wang, W.; Zhou, Y.; Zhong, J.; Tang, B.; Fang, J.; et al. Accuracy improvement of a compact 85Rb atom gravimeter by suppressing laser crosstalk and light shift. Sensors 2023, 23, 6115. [Google Scholar] [CrossRef]
- Mao, D.K.; Deng, X.B.; Luo, H.Q.; Xu, Y.Y.; Zhou, M.K.; Duan, X.C.; Hu, Z.K. A dual-magneto-optical-trap atom gravity gradiometer for determining the Newtonian gravitational constant. Rev. Sci. Instrum. 2021, 92, 053202. [Google Scholar]
- Dutta, I.; Savoie, D.; Fang, B.; Venon, B.; Alzar, C.G.; Geiger, R.; Landragin, A. Continuous cold-atom inertial sensor with 1 nrad/sec rotation stability. Phys. Rev. Lett. 2016, 116, 183003. [Google Scholar] [CrossRef]
- Garrido Alzar, C.L. Compact chip-scale guided cold atom gyrometers for inertial navigation: Enabling technologies and design study. AVS Quantum Sci. 2019, 1, 014702. [Google Scholar]
- Keil, M.; Amit, O.; Zhou, S.; Groswasser, D.; Japha, Y.; Folman, R. Fifteen years of cold matter on the atom chip: Promise, realizations, and prospects. J. Mod. Opt. 2016, 63, 1840–1885. [Google Scholar]
- McGilligan, J.P.; Moore, K.; Dellis, A.; Martinez, G.; de Clercq, E.; Griffin, P.; Arnold, A.; Riis, E.; Boudot, R.; Kitching, J. Laser cooling in a chip-scale platform. Appl. Phys. Lett. 2020, 117, 054001. [Google Scholar] [CrossRef]
- Li, D.; He, W.; Shi, S.; Wu, B.; Xiao, Y.; Lin, Q.; Li, L. Review of atom chips for absolute gravity sensors. Sensors 2023, 23, 5089. [Google Scholar]
- Ol’shanii, M.; Ovchinnikov, Y.B.; Letokhov, V. Laser guiding of atoms in a hollow optical fiber. Opt. Commun. 1993, 98, 77–79. [Google Scholar] [CrossRef]
- Barnett, A.; Smith, S.; Olshanii, M.; Johnson, K.; Adams, A.; Prentiss, M. Substrate-based atom waveguide using guided two-color evanescent light fields. Phys. Rev. A 2000, 61, 023608. [Google Scholar] [CrossRef]
- Burke Jr, J.P.; Chu, S.T.; Bryant, G.W.; Williams, C.J.; Julienne, P.S. Designing neutral-atom nanotraps with integrated optical waveguides. Phys. Rev. A 2002, 65, 043411. [Google Scholar]
- Ovchinnikov, Y.B.; Shul’ga, S.; Balykin, V. An atomic trap based on evanescent light waves. J. Phys. B At. Mol. Opt. Phys. 1991, 24, 3173. [Google Scholar] [CrossRef]
- Ovchinnikov, Y.B.; Ayi-Yovo, F.E. Towards all-optical atom chips based on optical waveguides. New J. Phys. 2020, 22, 053003. [Google Scholar] [CrossRef]
- Ovchinnikov, Y.B. A perspective on integrated atomo-photonic waveguide circuits. Appl. Phys. Lett. 2022, 120, 010502. [Google Scholar] [CrossRef]
- Konopsky, V. Design of 1D photonic crystals sustaining optical surface modes. Coatings 2022, 12, 1489. [Google Scholar] [CrossRef]
- Arnaud, J.A.; Saleh, A.A.M. Guidance of surface waves by multilayer coatings. Appl. Opt. 1974, 13, 2343–2345. [Google Scholar] [CrossRef]
- Yeh, P.; Yariv, A.; Hong, C.S. Electromagnetic propagation in periodic stratified media. I. General theory. J. Opt. Soc. Am. 1977, 67, 423–438. [Google Scholar] [CrossRef]
- Yeh, P.; Yariv, A.; Cho, A.Y. Optical surface waves in periodic layered media. Appl. Phys. Lett. 1978, 32, 104–105. [Google Scholar] [CrossRef]
- Robertson, W.M.; May, M.S. Surface electromagnetic waves on one-dimensional photonic band gap arrays. Appl. Phys. Lett. 1999, 74, 1800–1802. [Google Scholar] [CrossRef]
- Shinn, A.; Robertson, W. Surface plasmon-like sensor based on surface electromagnetic waves in a photonic band-gap material. Sens. Actuator B-Chem. 2005, 105, 360–364. [Google Scholar]
- Konopsky, V.N.; Alieva, E.V. Long-range propagation of plasmon polaritons in a thin metal film on a one-dimensional photonic crystal surface. Phys. Rev. Lett. 2006, 97, 253–904. [Google Scholar] [CrossRef]
- Hamidi, S.; Ramezani, R.; Bananej, A. Hydrogen gas sensor based on long-range surface plasmons in lossy palladium film placed on photonic crystal stack. Opt. Mater. 2016, 53, 201–208. [Google Scholar] [CrossRef]
- Ignatyeva, D.O.; Knyazev, G.A.; Kapralov, P.O.; Dietler, G.; Sekatskii, S.K.; Belotelov, V.I. Magneto-optical plasmonic heterostructure with ultranarrow resonance for sensing applications. Sci. Rep. 2016, 6, 28077. [Google Scholar] [CrossRef]
- Li, J.; Tang, T.; Zhang, Y.; Luo, L.; Sun, P. Magneto-plasmonic sensor with one dimensional photonic crystal for methane detection. Optik 2018, 155, 74–80. [Google Scholar] [CrossRef]
- Ignatyeva, D.; Kapralov, P.; Golovko, P.; Shilina, P.; Khramova, A.; Sekatskii, S.; Nur-E-Alam, M.; Alameh, K.; Vasiliev, M.; Kalish, A.; et al. Sensing of surface and bulk refractive index using magnetophotonic crystal with hybrid magneto-optical response. Sensors 2021, 21, 1984. [Google Scholar] [CrossRef]
- Konopsky, V.N.; Alieva, E.V. Photonic crystal surface waves for optical biosensors. Anal. Chem. 2007, 79, 4729–4735. [Google Scholar] [CrossRef]
- Guo, Y.; Ye, J.Y.; Divin, C.; Huang, B.; Thomas, T.P.; Baker, J.R., Jr.; Norris, T.B. Real-time biomolecular binding detection using a sensitive photonic crystal biosensor. Anal. Chem. 2010, 82, 5211–5218. [Google Scholar] [CrossRef] [PubMed]
- Konopsky, V.N.; Karakouz, T.; Alieva, E.V.; Vicario, C.; Sekatskii, S.K.; Dietler, G. Photonic crystal biosensor based on optical surface waves. Sensors 2013, 13, 2566–2578. [Google Scholar] [CrossRef] [PubMed]
- Rivolo, P.; Michelotti, F.; Frascella, F.; Digregorio, G.; Mandracci, P.; Dominici, L.; Giorgis, F.; Descrovi, E. Real time secondary antibody detection by means of silicon-based multilayers sustaining Bloch surface waves. Sens. Actuators B Chem. 2012, 161, 1046–1052. [Google Scholar] [CrossRef]
- Wei, C.; Cen, M.; Chui, H.C.; Cao, T. Numerical study of biosensor based on α-MoO3/Au hyperbolic metamaterial at visible frequencies. J. Phys. D Appl. Phys. 2020, 54, 034001. [Google Scholar] [CrossRef]
- Khodami, M.; Hirbodvash, Z.; Krupin, O.; Wong, W.R.; Lisicka-Skrzek, E.; Northfield, H.; Hahn, C.; Berini, P. Fabrication of Bloch long range surface plasmon waveguides integrating counter electrodes and microfluidic channels for multimodal biosensing. J. Microelectromech. Syst. 2021, 30, 686–695. [Google Scholar] [CrossRef]
- Kalas, B.; Ferencz, K.; Saftics, A.; Czigany, Z.; Fried, M.; Petrik, P. Bloch surface waves biosensing in the ultraviolet wavelength range – Bragg structure design for investigating protein adsorption by in situ Kretschmann-Raether ellipsometry. Appl. Surf. Sci. 2021, 536, 147869. [Google Scholar] [CrossRef]
- Delfan, A.; Liscidini, M.; Sipe, J.E. Surface enhanced Raman scattering in the presence of multilayer dielectric structures. JOSA B 2012, 29, 1863–1874. [Google Scholar] [CrossRef]
- Konopsky, V.N.; Alieva, E.V.; Alyatkin, S.Y.; Melnikov, A.A.; Chekalin, S.V.; Agranovich, V.M. Phase-matched third-harmonic generation via doubly resonant optical surface modes in 1D photonic crystals. LIght Sci. Appl. 2016, 5, e16168. [Google Scholar] [CrossRef]
- Fong, N.R.; Menotti, M.; Lisicka-Skrzek, E.; Northfield, H.; Olivieri, A.; Tait, N.; Liscidini, M.; Berini, P. Bloch long-range surface plasmon polaritons on metal stripe waveguides on a multilayer substrate. ACS Photonics 2017, 4, 593–599. [Google Scholar] [CrossRef]
- Degli-Eredi, I.; Sipe, J.; Vermeulen, N. TE-polarized graphene modes sustained by photonic crystal structures. Opt. Lett. 2015, 40, 2076–2079. [Google Scholar] [CrossRef]
- Konopsky, V.; Prokhorov, V.; Lypenko, D.; Dmitriev, A.; Alieva, E.; Dietler, G.; Sekatskii, S. Electrical excitation of long-range surface plasmons in PC/OLED structure with two metal nanolayers. Nano-Micro Lett. 2020, 12, 1–8. [Google Scholar] [CrossRef] [PubMed]
- Kovalevich, T.; Belharet, D.; Robert, L.; Ulliac, G.; Kim, M.S.; Herzig, H.P.; Grosjean, T.; Bernal, M.P. Bloch surface waves at the telecommunication wavelength with lithium niobate as the top layer for integrated optics. Appl. Opt. 2019, 58, 1757–1762. [Google Scholar] [CrossRef] [PubMed]
- Konopsky, V. Version 1.1.8401.20377. 2022. Available online: https://www.pcbiosensors.com/1DPC4all.htm (accessed on 26 October 2023).
- Grimm, R.; Weidemüller, M.; Ovchinnikov, Y.B. Optical dipole traps for neutral atoms. In Advances in Atomic, Molecular, and Optical Physics; Elsevier: Amsterdam, The Netherlands, 2000; Volume 42, pp. 95–170. [Google Scholar]
- Ivanova, A.; Stoffer, R.; Hammer, M. A variational mode solver for optical waveguides based on quasi-analytical vectorial slab mode expansion. arXiv 2013, arXiv:1307.1315. [Google Scholar]
- Ivanova, O.; Hammer, M.; Stoffer, R.; Van Groesen, E. A variational mode expansion mode solver. Opt. Quantum Electron. 2007, 39, 849–864. [Google Scholar] [CrossRef]
- Zhu, Z.; Brown, T.G. Full-vectorial finite-difference analysis of microstructured optical fibers. Opt. Express 2002, 10, 853–864. [Google Scholar] [CrossRef]
- Miller, J.; Cline, R.; Heinzen, D. Far-off-resonance optical trapping of atoms. Physical Review A 1993, 47, R4567. [Google Scholar] [CrossRef]
- Thompson, J.D.; Tiecke, T.; de Leon, N.P.; Feist, J.; Akimov, A.; Gullans, M.; Zibrov, A.S.; Vuletić, V.; Lukin, M.D. Coupling a single trapped atom to a nanoscale optical cavity. Science 2013, 340, 1202–1205. [Google Scholar] [CrossRef]
- Sidqi, N.; Clark, C.; Buller, G.S.; Thalluri, G.K.V.; Mitrofanov, J.; Noblet, Y. Comparative study of dielectric coating materials for micro-cavity applications. Opt. Mater. Express 2019, 9, 3452–3468. [Google Scholar] [CrossRef]
- Wang, K.; Schonbrun, E.; Steinvurzel, P.; Crozier, K.B. Trapping and rotating nanoparticles using a plasmonic nano-tweezer with an integrated heat sink. Nat. Commun. 2011, 2, 469. [Google Scholar] [CrossRef]
- Lin, S.; Crozier, K.B. Trapping-assisted sensing of particles and proteins using on-chip optical microcavities. ACS Nano 2013, 7, 1725–1730. [Google Scholar] [CrossRef]
- Xu, Z.; Song, W.; Crozier, K.B. Optical trapping of nanoparticles using all-silicon nanoantennas. ACS Photonics 2018, 5, 4993–5001. [Google Scholar] [CrossRef]
- Brunetti, G.; Sasanelli, N.; Armenise, M.N.; Ciminelli, C. Nanoscale optical trapping by means of dielectric bowtie. Photonics 2022, 9, 425. [Google Scholar] [CrossRef]
- Sergeev, O.; Shashkov, A.; Umanskii, A. Thermophysical properties of quartz glass. J. Eng. Phys. 1982, 43, 1375–1383. [Google Scholar] [CrossRef]
- SCHOTT. Technical Information TIE-31: Mechanical and Thermal Properties of Optical Glass; SCHOTT: New York, NY, USA, 2004. [Google Scholar]
- Dobrovinskaya, E.R.; Lytvynov, L.A.; Pishchik, V. Properties of Sapphire. In Sapphire: Material, Manufacturing, Applications; Springer US: Boston, MA, USA, 2009; pp. 55–176. [Google Scholar]
- Thuau, D.; Koymen, I.; Cheung, R. A microstructure for thermal conductivity measurement of conductive thin films. Microelectron. Eng. 2011, 88, 2408–2412. [Google Scholar] [CrossRef]
- Lugo, J.; Oliva, A. Thermal properties of metallic films at room conditions by the heating slope. J. Thermophys. Heat Transf. 2016, 30, 452–460. [Google Scholar] [CrossRef]
- SCHOTT. Technical Information TIE-32: Thermal Loads on Optical Glass; SCHOTT: New York, NY, USA, 2004. [Google Scholar]
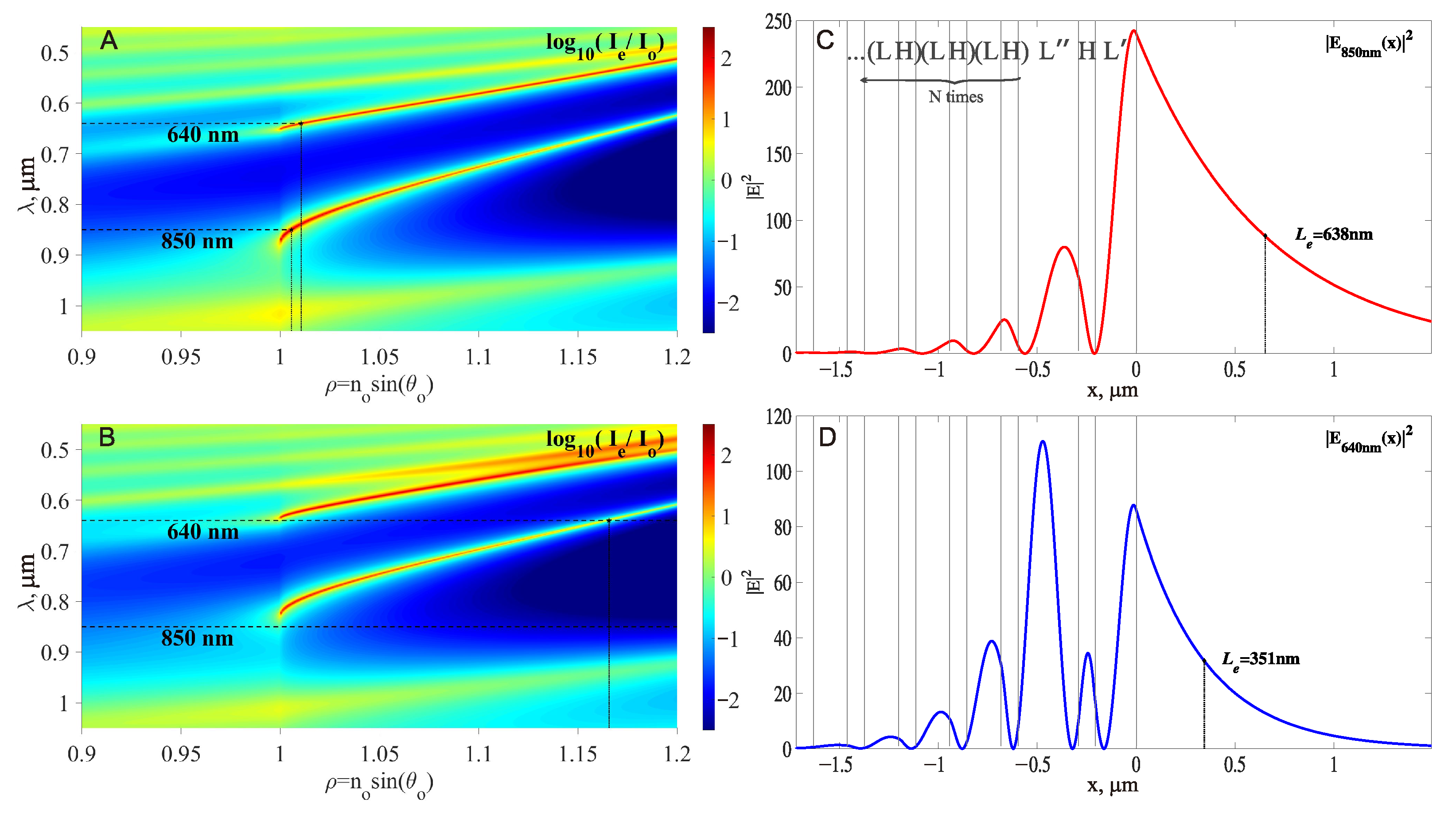
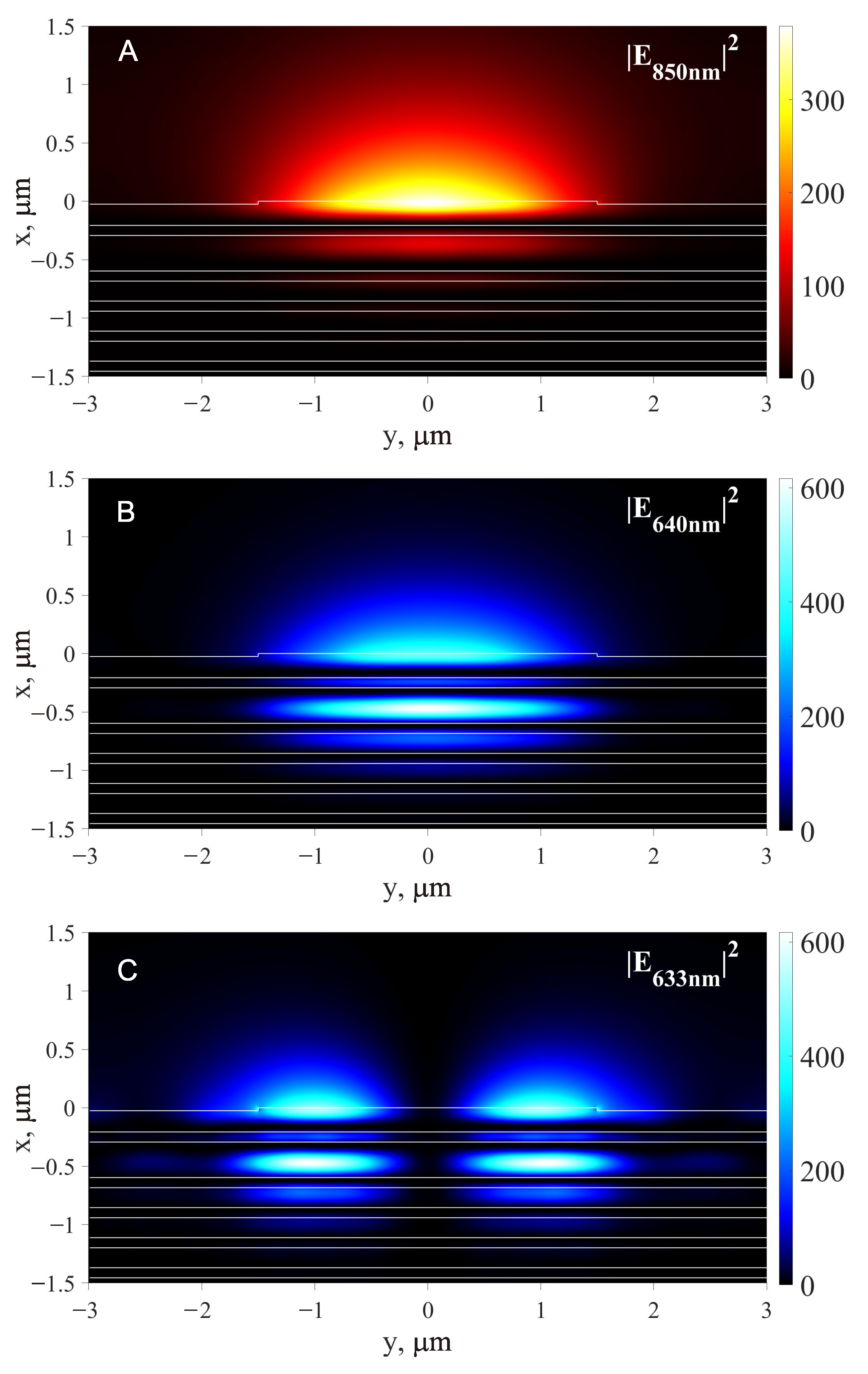

| Wavelength | , nm | |||
|---|---|---|---|---|
| 850 nm | 1.4666 | 2.3137 | 1.0056 | 638 |
| 640 nm | 1.4679 | 2.3674 | 1.0105 | 351 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the author. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Konopsky, V. Photonic Crystal Surface Modes for Trapping and Waveguiding of Ultracold Atoms. Sensors 2023, 23, 8812. https://doi.org/10.3390/s23218812
Konopsky V. Photonic Crystal Surface Modes for Trapping and Waveguiding of Ultracold Atoms. Sensors. 2023; 23(21):8812. https://doi.org/10.3390/s23218812
Chicago/Turabian StyleKonopsky, Valery. 2023. "Photonic Crystal Surface Modes for Trapping and Waveguiding of Ultracold Atoms" Sensors 23, no. 21: 8812. https://doi.org/10.3390/s23218812
APA StyleKonopsky, V. (2023). Photonic Crystal Surface Modes for Trapping and Waveguiding of Ultracold Atoms. Sensors, 23(21), 8812. https://doi.org/10.3390/s23218812





