Revealing Local Temporal Profile of Laser Pulses of Intensity above 1014 W/cm2
Abstract
1. Introduction
2. Materials and Methods
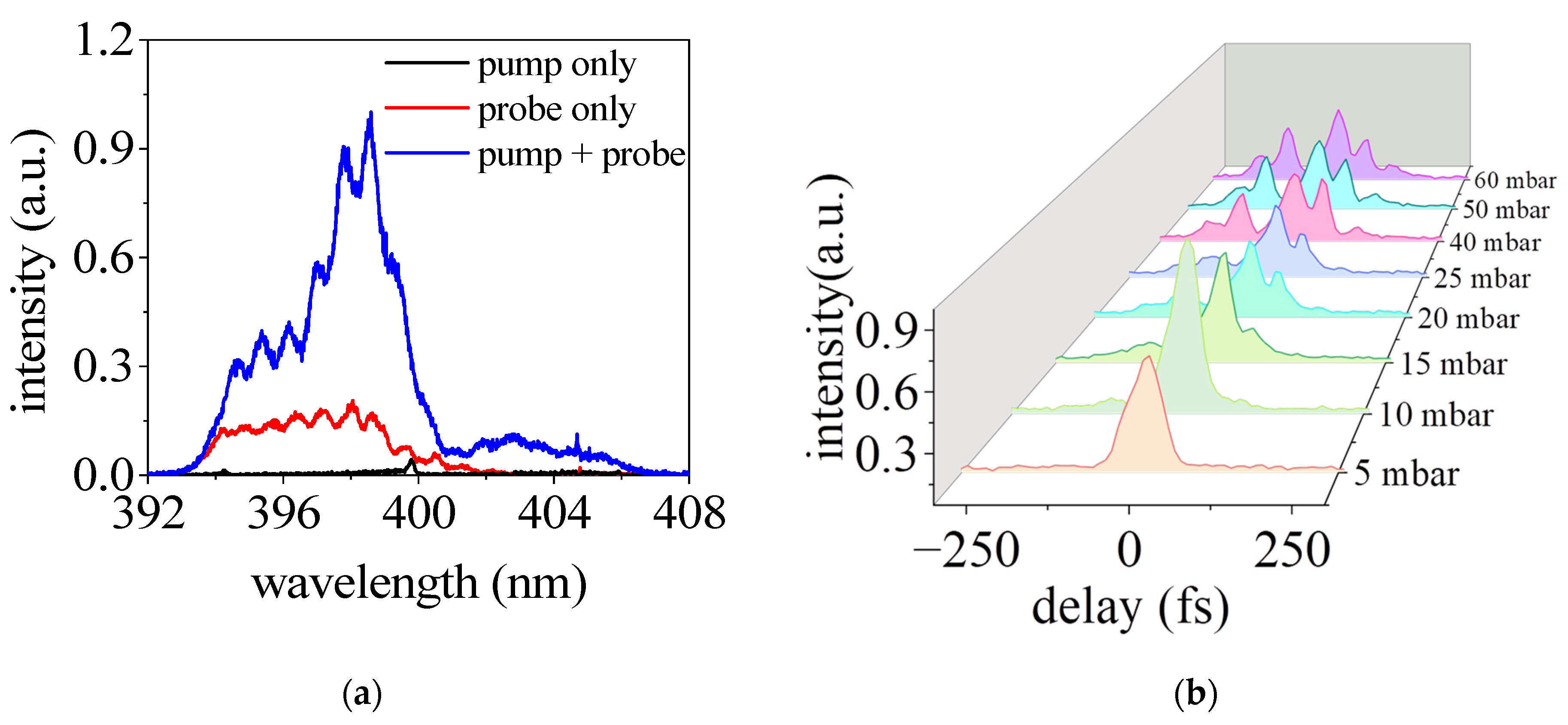
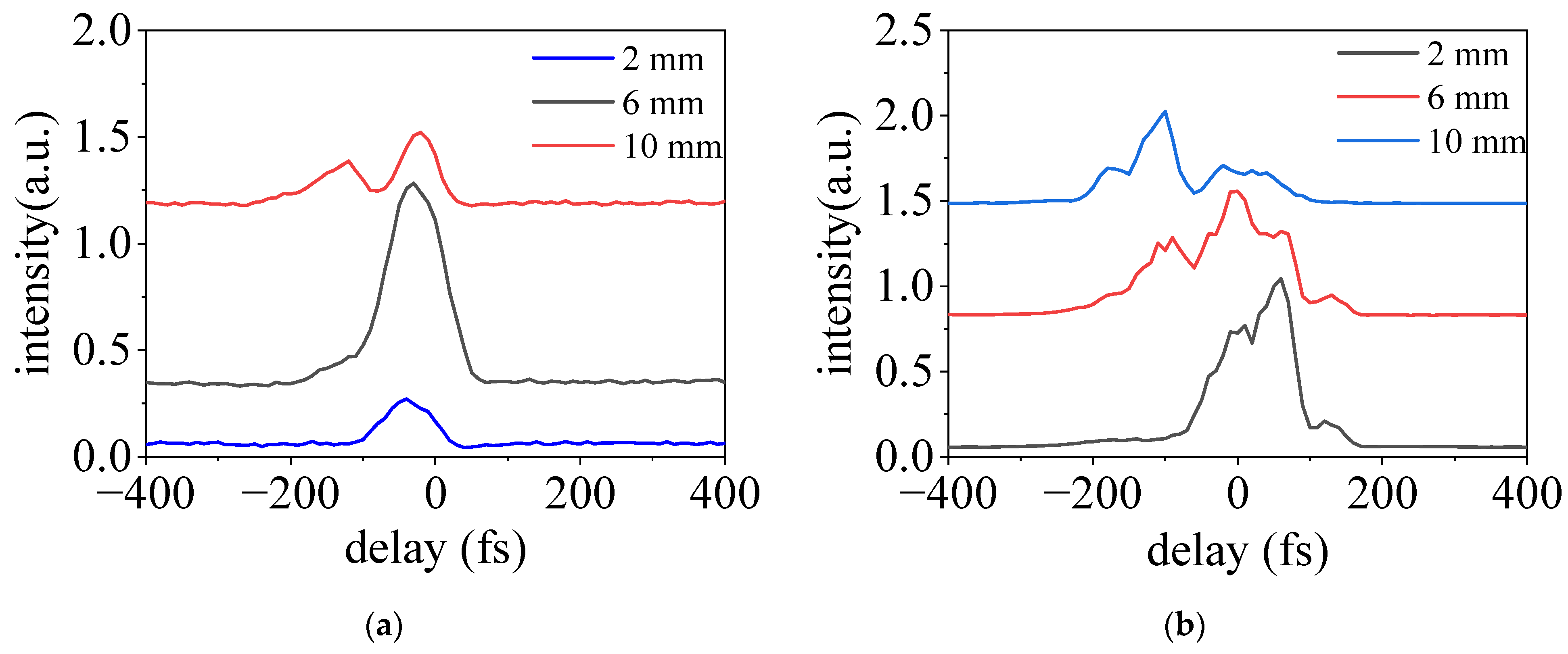
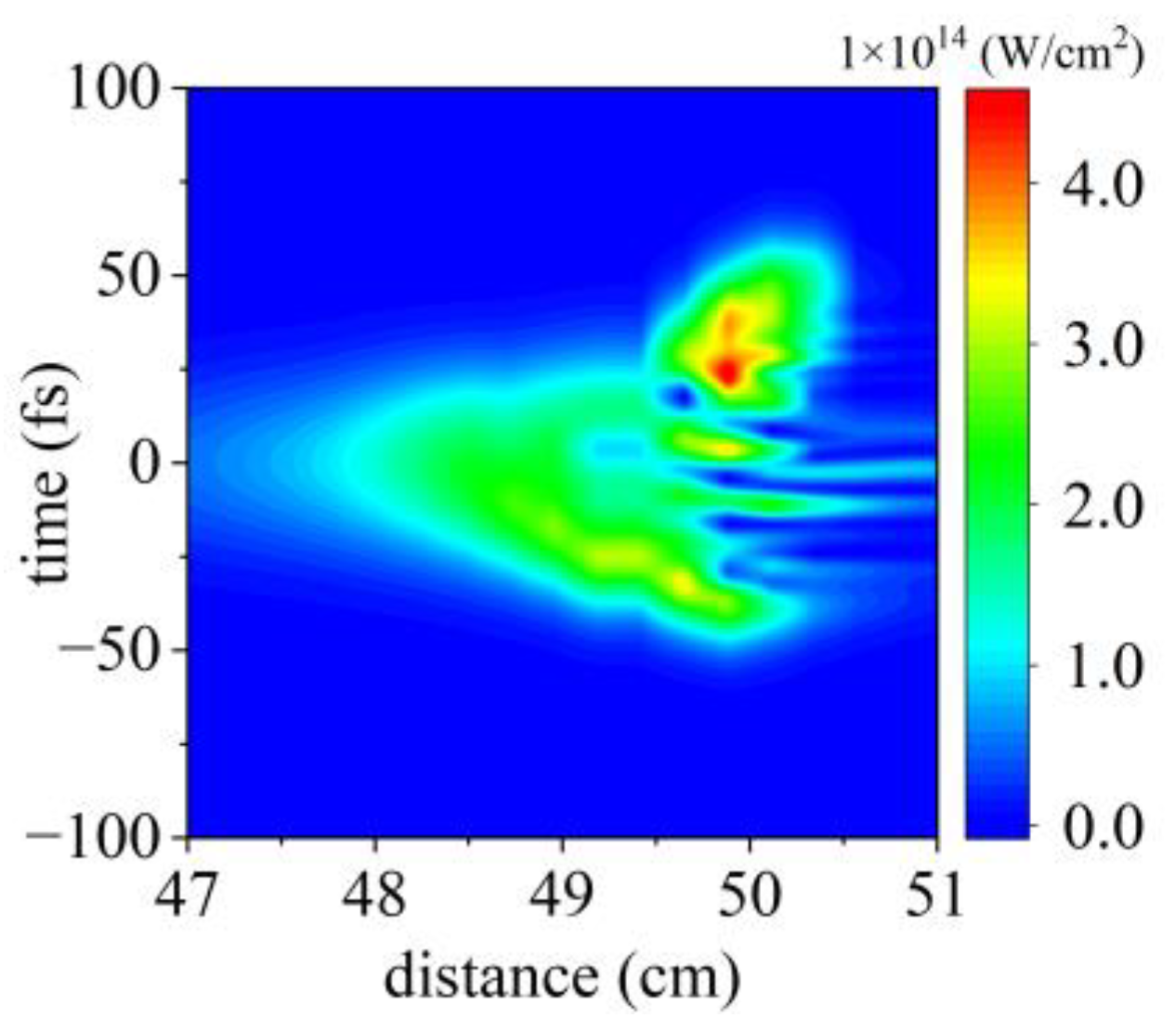
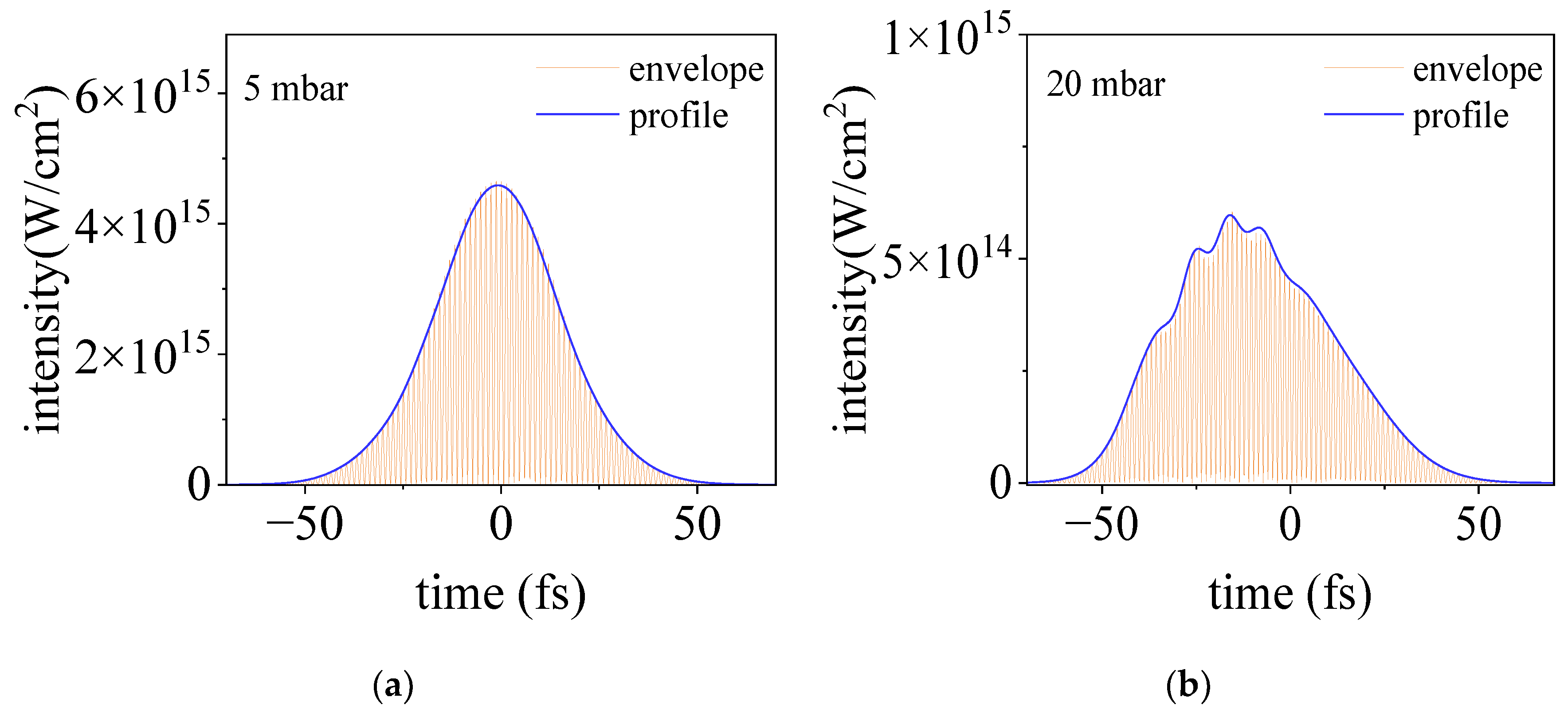
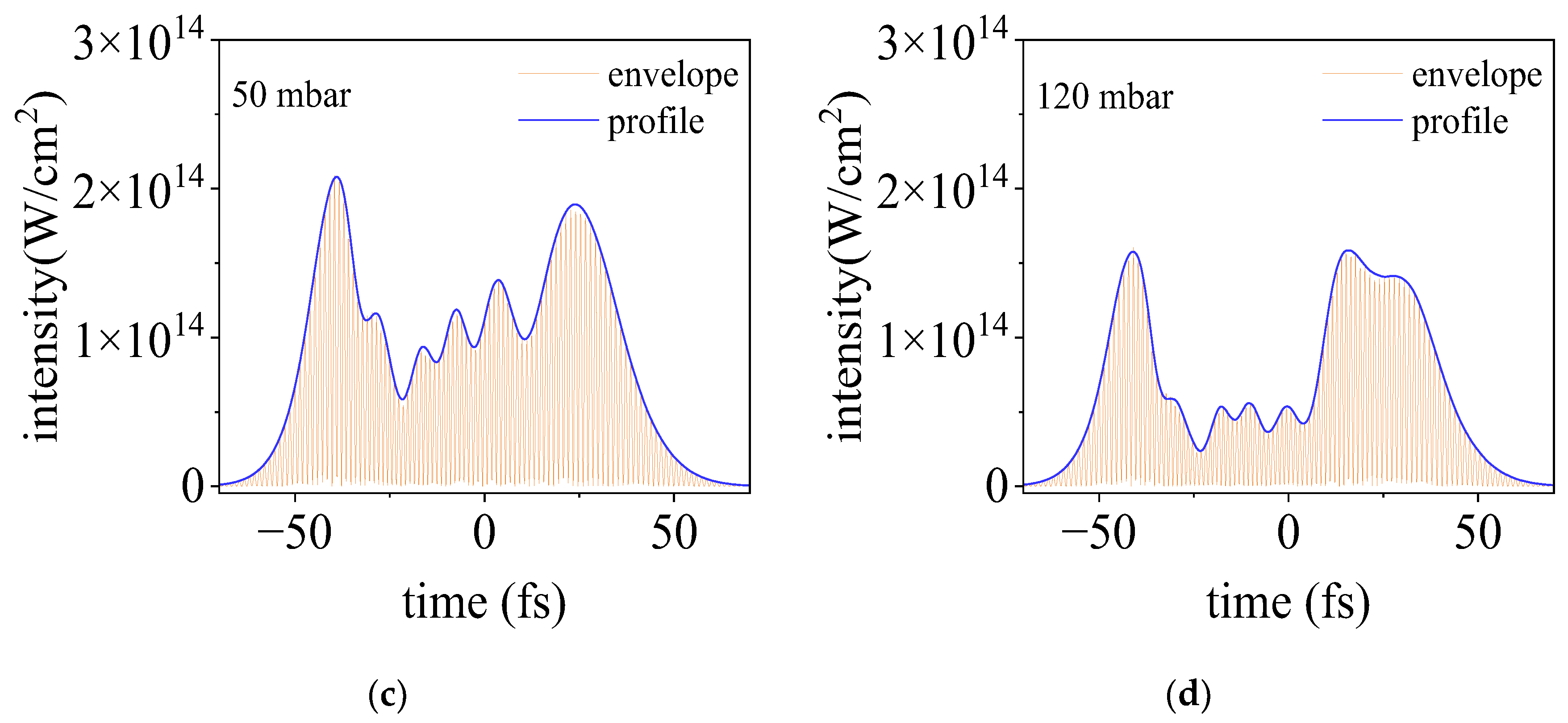
3. Results
4. Discussion
5. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Walmsley, I.; Dorrer, C. Characterization of ultrashort electromagnetic pulses. Adv. Opt. Photon. 2009, 1, 308–437. [Google Scholar] [CrossRef]
- Diels, J.C.M.; Fontaine, J.J.; McMichael, I.C.; Simoni, F. Control and measurement of ultrashort pulse shapes (in amplitude and phase) with femtosecond accuracy. Appl. Opt. 1985, 24, 1270–1282. [Google Scholar] [CrossRef]
- Gliserin, A.; Chew, S.H.; Kim, S.; Kim, D.E. Complete characterization of ultrafast optical fields by phase-preserving nonlinear autocorrelation. Light Sci. Appl. 2022, 11, 277. [Google Scholar] [CrossRef] [PubMed]
- Trebino, R.; DeLong, K.W.; Fittinghoff, D.N.; Sweetser, J.N.; Krumbügel, M.A.; Richman, B.A.; Kane, D.J. Measuring ultrashort laser pulses in the time-frequency domain using frequency-resolved optical gating. Rev. Sci. Instrum. 1997, 68, 3277–3295. [Google Scholar] [CrossRef]
- Iaconis, C.; Walmsley, I.A. Spectral phase interferometry for direct electric-field reconstruction of ultrashort optical pulses. Opt. Lett. 1998, 23, 792–794. [Google Scholar] [CrossRef] [PubMed]
- Birge, J.R.; Crespo, H.M.; Krtner, F.X. Theory and design of two-dimensional spectral shearing interferometry for few-cycle pulse measurement. J. Opt. Soc. Am. 2010, 27, 1165–1173. [Google Scholar] [CrossRef]
- Miranda, M.; Fordell, T.; Arnold, C.; L’Huillier, A.; Crespo, H. Simultaneous compression and characterization of ultrashort laser pulses using chirped mirrors and glass wedges. Opt. Express 2012, 20, 688–697. [Google Scholar] [CrossRef]
- Gomes, T.; Canhota, M.; Crespo, H. Temporal characterization of broadband femtosecond laser pulses by surface third-harmonic dispersion scan with ptychographic retrieval. Opt. Lett. 2022, 47, 3660–3663. [Google Scholar] [CrossRef]
- Sayler, A.M.; Rathje, T.; Müller, W.; Kürbis, C.; Rühle, K.; Stibenz, G.; Paulus, G.G. Real-time pulse length measurement of few-cycle laser pulses using above-threshold ionization. Opt. Express 2011, 19, 4464–4471. [Google Scholar] [CrossRef]
- Aközbek, N.; Bowden, C.M.; Talebpour, A.; Chin, S.L. Femtosecond pulse propagation in air: Variational analysis. Phys. Rev. E 2000, 61, 4540–4549. [Google Scholar] [CrossRef]
- Couairon, A.; Berge, L. Light filaments in air for ultraviolet and infrared wavelengths. Phys. Rev. Lett. 2002, 88, 135003. [Google Scholar] [CrossRef]
- Qin, Y.D.; Yang, H.; Zhu, C.J.; Gong, Q.H. Intense femtosecond laser-induced second-harmonic generation in atmospheric-pressure air. Appl. Phys. B Lasers Opt. 2000, 71, 581–584. [Google Scholar] [CrossRef]
- Beresna, M.; Kazansky, P.G.; Svirko, Y.; Barkauskas, M.; Danielius, R. High average power second harmonic generation in air. Appl. Phys. Lett. 2009, 95, 121502. [Google Scholar] [CrossRef]
- Li, G.H.; Ni, J.L.; Xie, H.Q.; Zeng, B.; Yao, J.P.; Chu, W.; Zhang, H.S.; Jing, C.R.; He, F.; Xu, H.L.; et al. Second harmonic generation in centrosymmetric gas with spatiotemporally focused intense femtosecond laser pulses. Opt. Lett. 2014, 39, 961–964. [Google Scholar] [CrossRef]
- Mitryukovskiy, S.I.; Liu, Y.; Houard, A.; Mysyrowicz, A. Re-evaluation of the peak intensity inside a femtosecond laser filament in air. J. Phys. B At. Mol. Opt. Phys. 2015, 48, 094003. [Google Scholar] [CrossRef]
- Couairon, A.; Brambilla, E.; Corti, T.; Majus, D.; de Ramírez-Góngora, O.J.; Kolesik, M. Practitioner’s guide to laser pulse propagation models and simulation. Eur. Phys. J. Spec. Top. 2011, 199, 5–76. [Google Scholar] [CrossRef]
- Kolesik, M.; Moloney, J.V. Nonlinear optical pulse propagation simulation: From Maxwell’s to unidirectional equations. Phys. Rev. E 2004, 70, 036604. [Google Scholar] [CrossRef]
- Dalgarno, A.; Kingston, A.E. The refractive indices and Verdet constants of the inert gases. Proc. R. Soc. Lond. 1960, 259, 424–431. [Google Scholar] [CrossRef]
- Raizer, Y.P. Gas Discharge Physics, 1st ed.; Springer: Berlin/Heidelberg, Germany, 1991. [Google Scholar]
- Keldysh, L.V. Ionization in the field of a strong electromagnetic wave. Sov. Phys. JETP 1965, 20, 1307–1314. [Google Scholar]
- Perelomov, A.M.; Popov, V.S.; Terentev, M.V. Ionization of atoms in an alternating electric filed. Zh. Eksp. Teor. Fiz. 1966, 50, 1393. [Google Scholar]
- Shcheblanov, N.S.; Povarnitsyn, M.E.; Terekhin, P.N.; Guizard, S.; Couairon, A. Nonlinear photoionization of transparent solids: A nonperturbative theory obeying selection rules. Phys. Rev. A 2017, 96, 063410. [Google Scholar] [CrossRef]


Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Lu, Q.; Zhang, X.; Couairon, A.; Liu, Y. Revealing Local Temporal Profile of Laser Pulses of Intensity above 1014 W/cm2. Sensors 2023, 23, 3101. https://doi.org/10.3390/s23063101
Lu Q, Zhang X, Couairon A, Liu Y. Revealing Local Temporal Profile of Laser Pulses of Intensity above 1014 W/cm2. Sensors. 2023; 23(6):3101. https://doi.org/10.3390/s23063101
Chicago/Turabian StyleLu, Qi, Xiang Zhang, Arnaud Couairon, and Yi Liu. 2023. "Revealing Local Temporal Profile of Laser Pulses of Intensity above 1014 W/cm2" Sensors 23, no. 6: 3101. https://doi.org/10.3390/s23063101
APA StyleLu, Q., Zhang, X., Couairon, A., & Liu, Y. (2023). Revealing Local Temporal Profile of Laser Pulses of Intensity above 1014 W/cm2. Sensors, 23(6), 3101. https://doi.org/10.3390/s23063101






