“Grafting-To” Covalent Binding of Plasmonic Nanoparticles onto Silica WGM Microresonators: Mechanically Robust Single-Molecule Sensors and Determination of Activation Energies from Single-Particle Events
Abstract
:1. Introduction
2. Materials and Methods
2.1. Chemicals
2.2. Colloidal Modification of Citrate-Capped Au-NR Using MPTMS
2.3. Colloidal Hybridization of Au-NR with MPTMS and Single-Strand A9 DNA
2.4. WGM Experimental Setup
2.5. WGM Data Processing and Determination of Independent Events
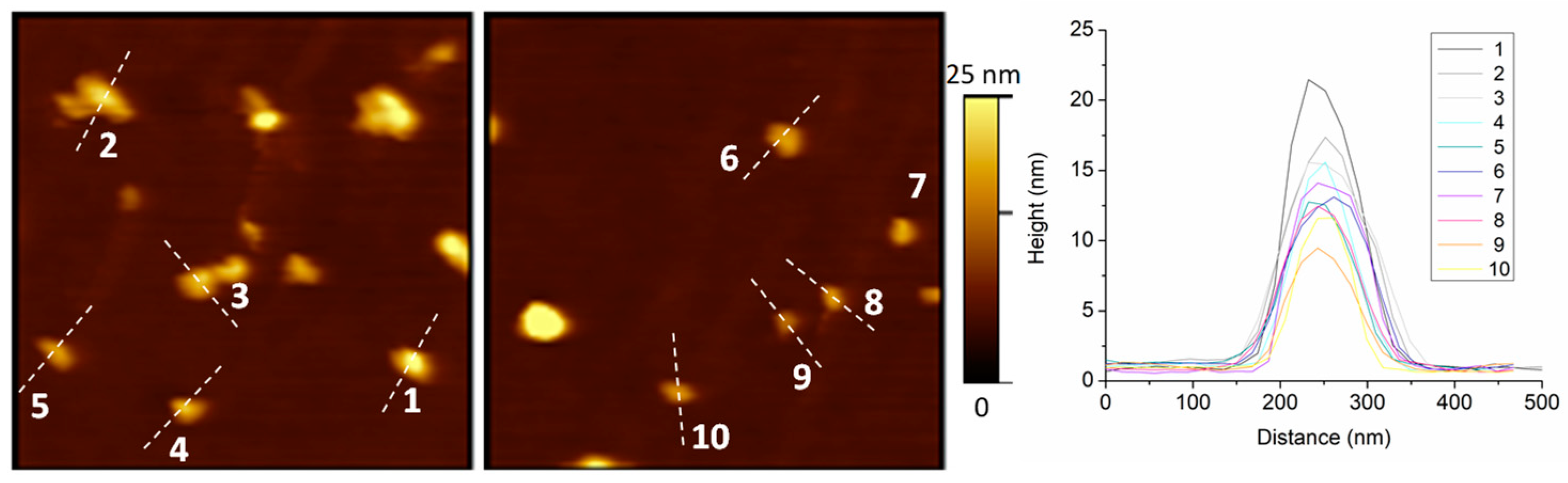
2.6. AFM Experiments and Preparation of Ex-Situ Samples
3. Results and Discussion
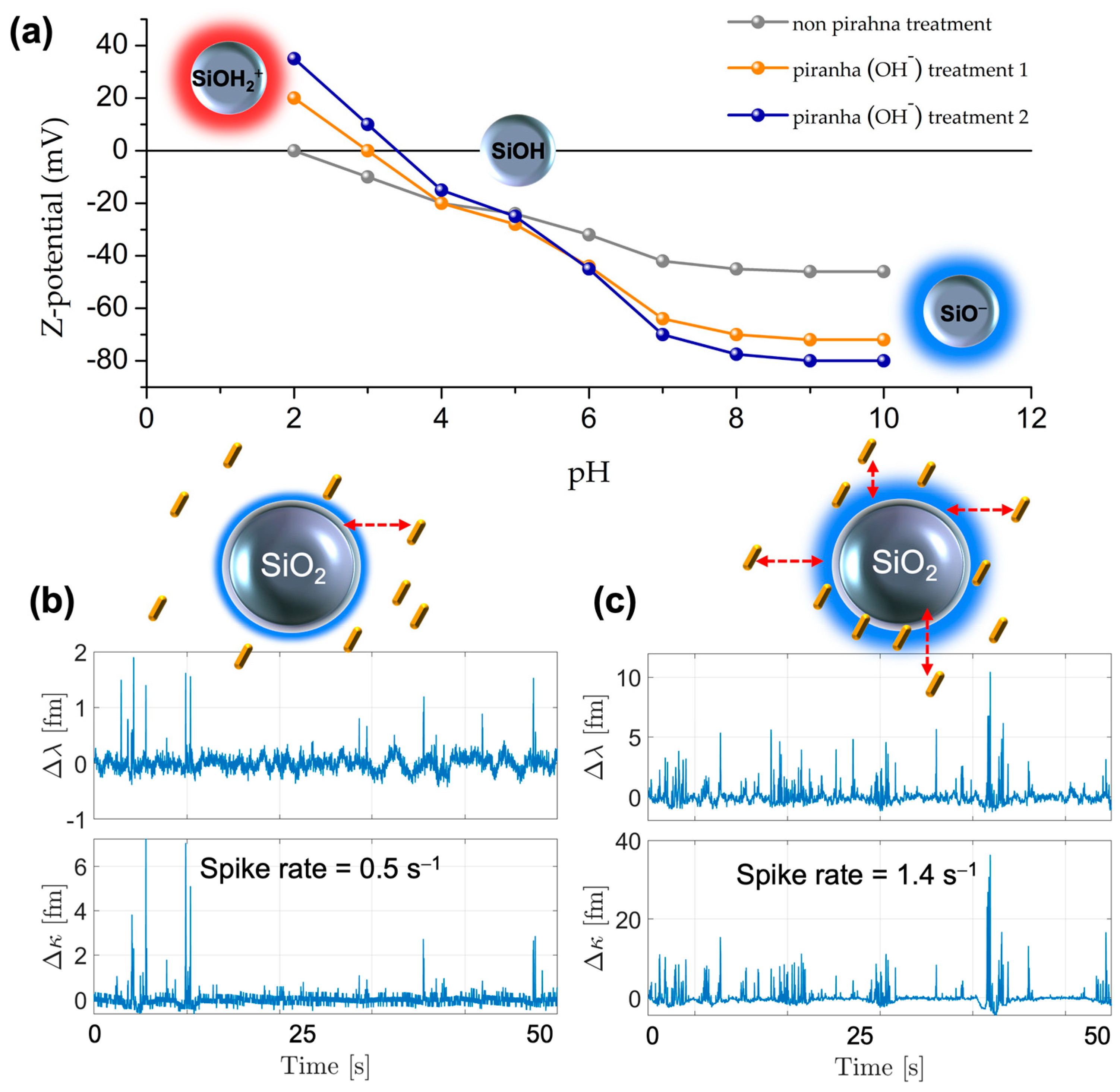
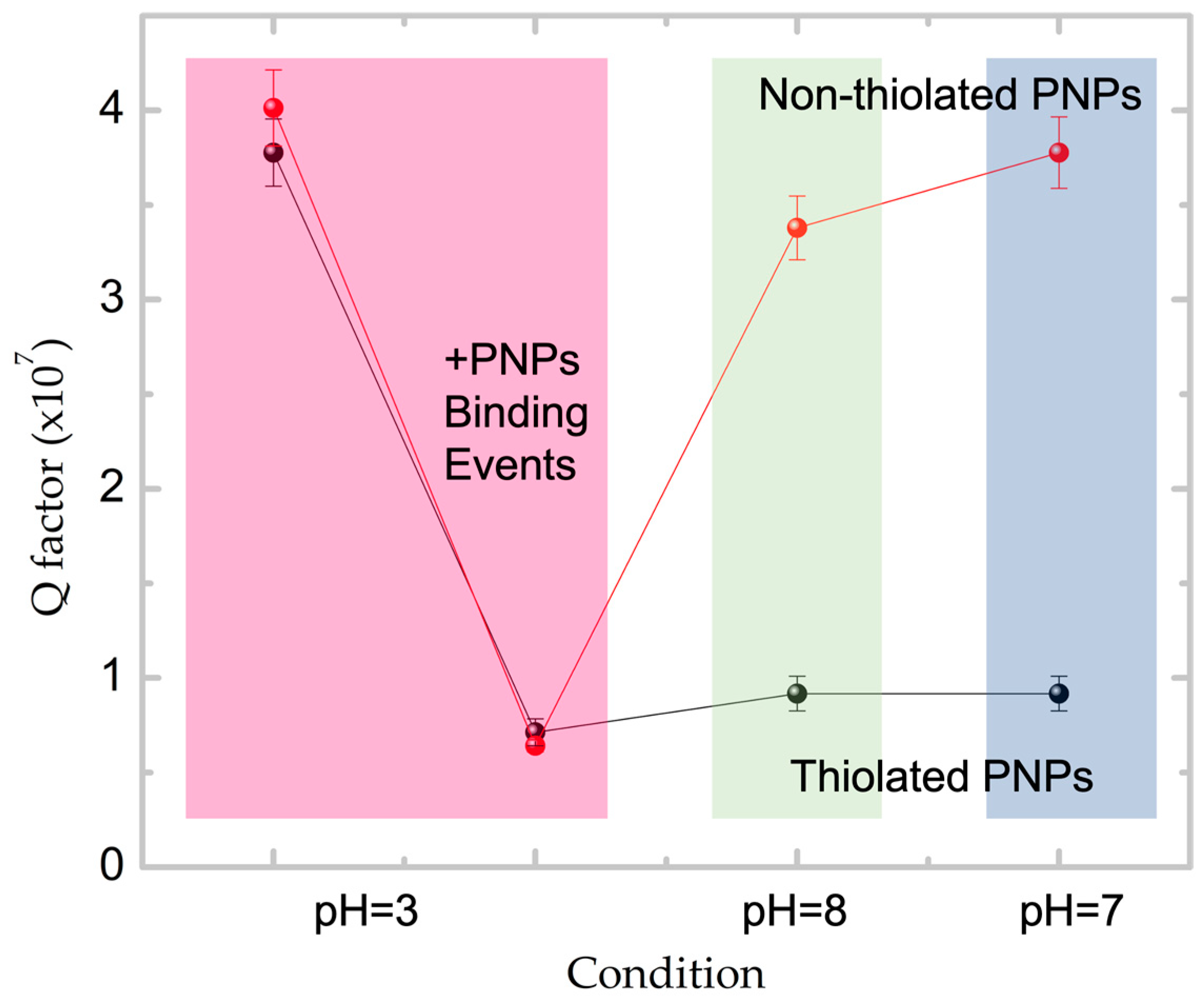
3.1. Surface Modification of Silica WGM Microspheres and the Effect of Alkaline Piranha Solution Treatment on the Interaction with PNPs
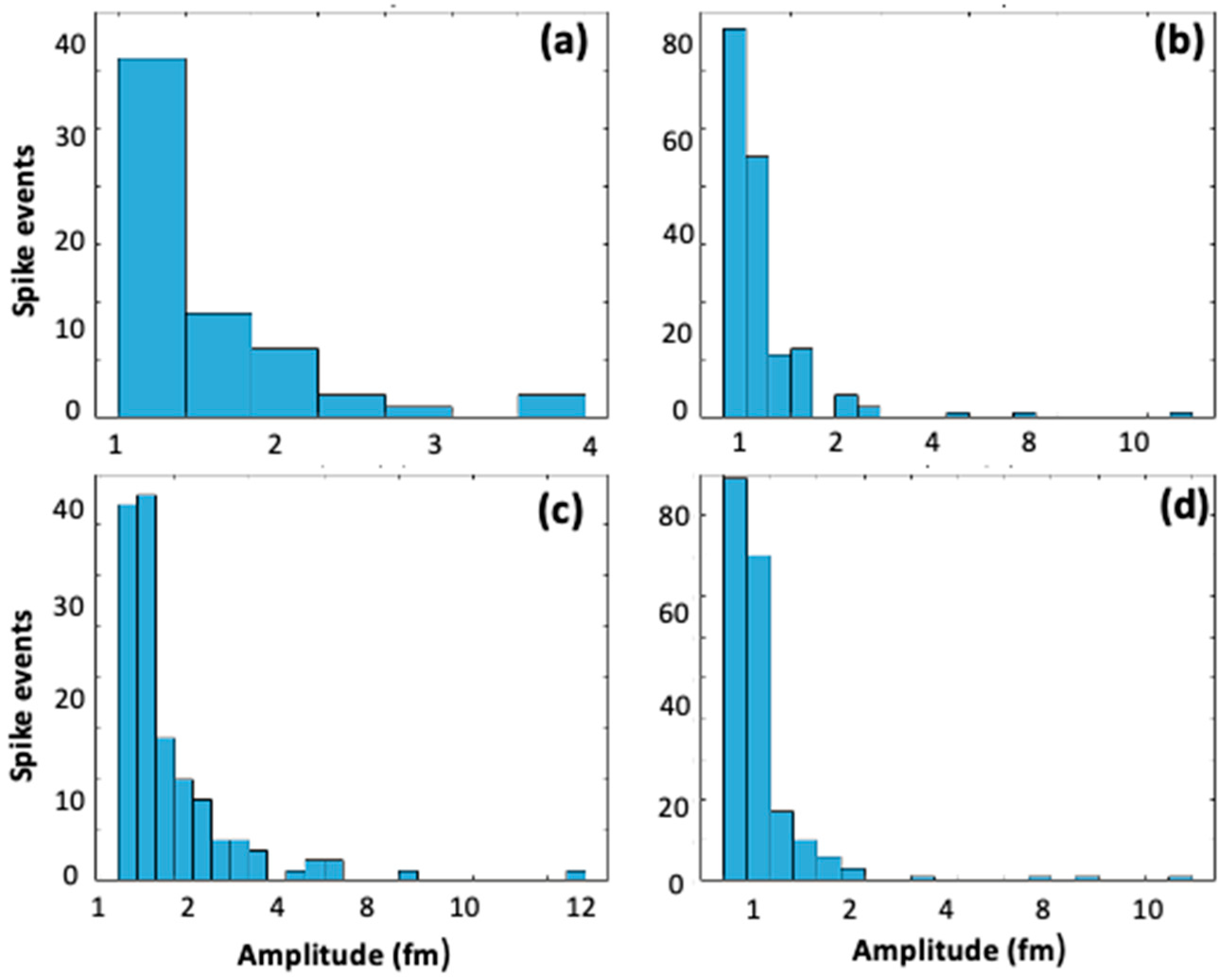
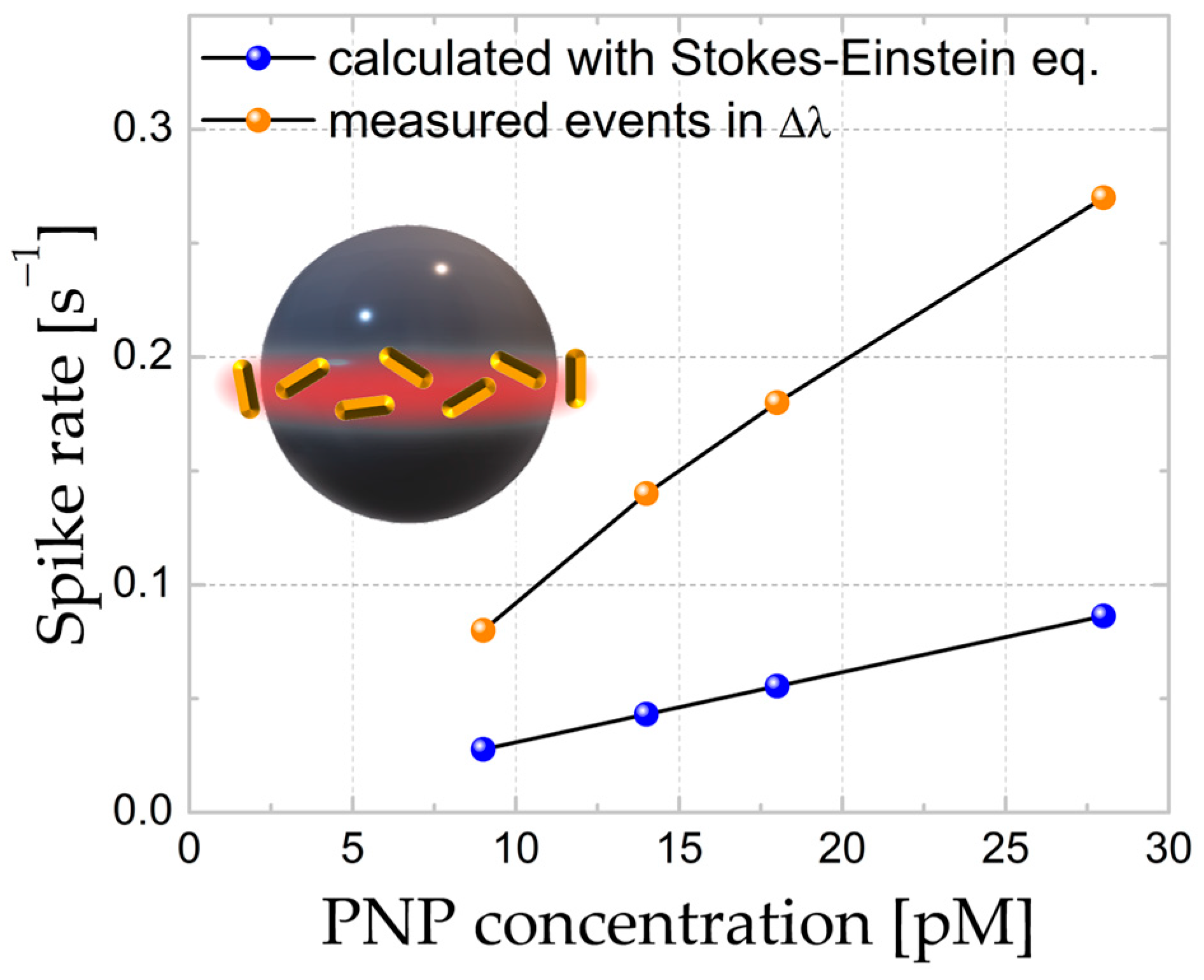
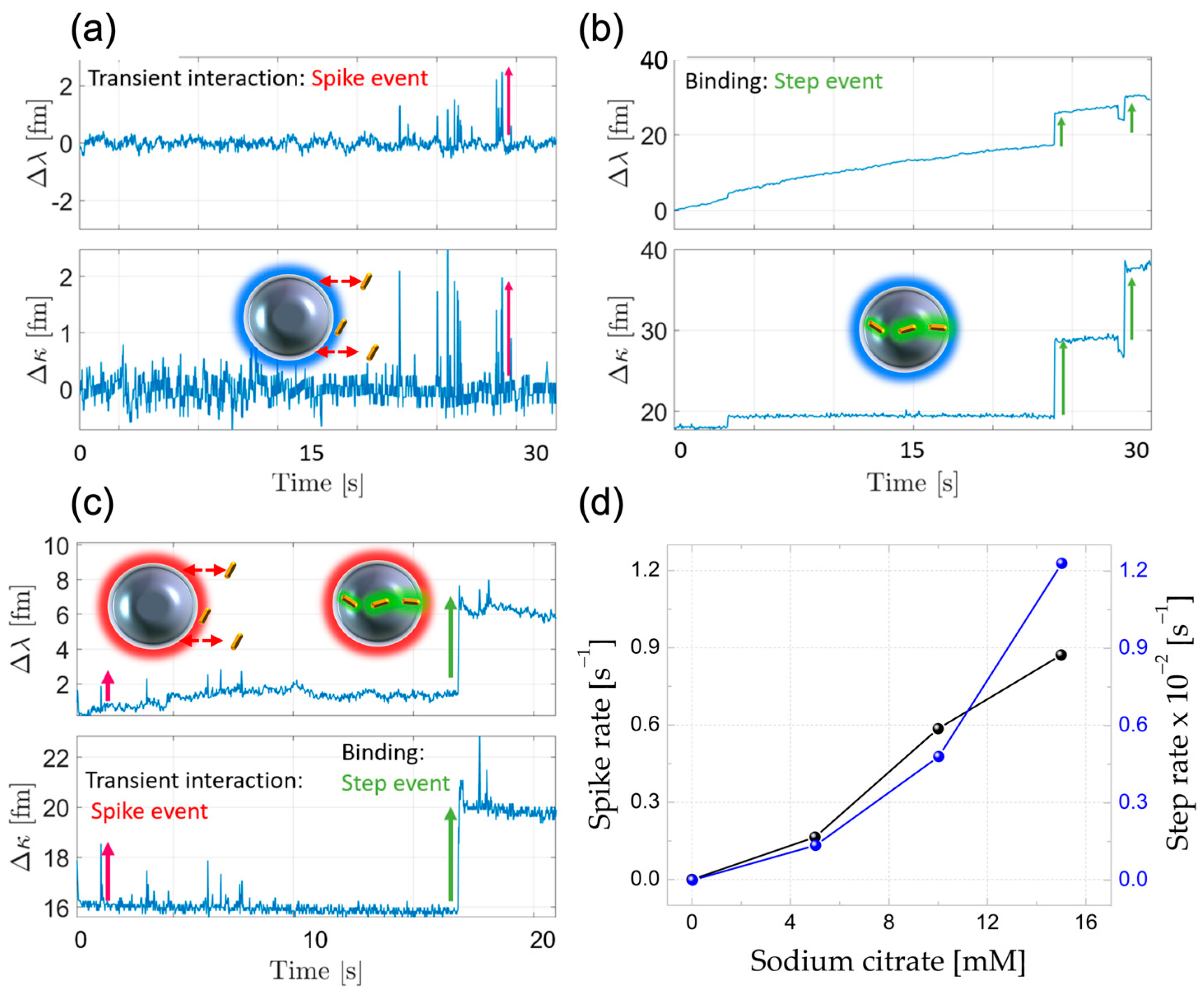
3.2. Determination of Regimes Leading to Permanent and/or Transient Interaction Events between PNPs and WGM Microresonator
3.3. Silanization Activation Energy Calculations via Arrhenius Equation
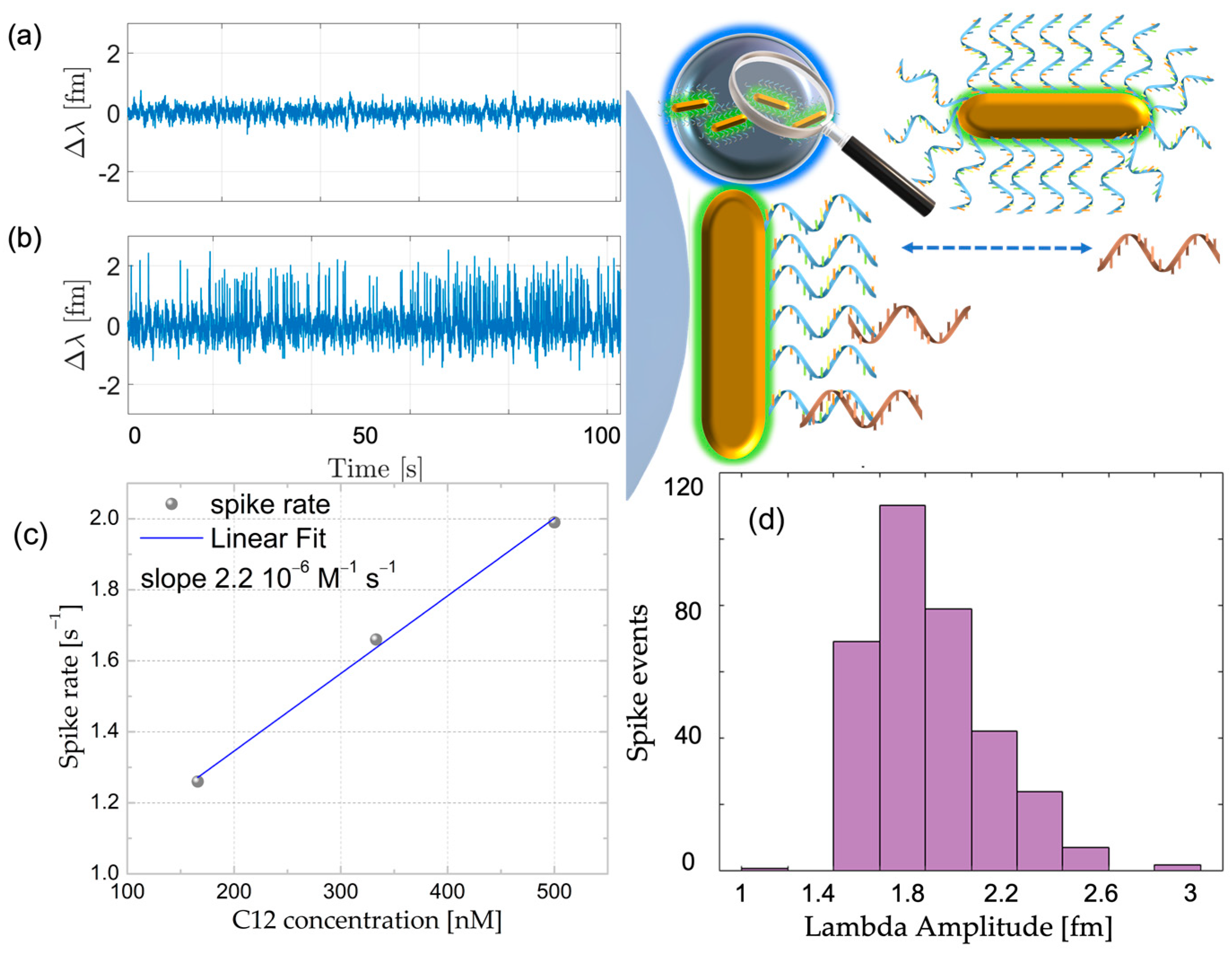
3.4. Complementary Oligonucleotide Detection Using Covalently Attached
4. Conclusions
Supplementary Materials
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Arnold, S.; Ramjit, R.; Keng, D.; Kolchenko, V.; Teraoka, I. MicroParticle Photophysics Illuminates Viral Bio-Sensing. Faraday Discuss. 2007, 137, 65–83. [Google Scholar] [CrossRef]
- Toropov, N.; Cabello, G.; Serrano, M.P.; Gutha, R.R.; Rafti, M.; Vollmer, F. Review of Biosensing with Whispering-Gallery Mode Lasers. Light Sci. Appl. 2021, 10, 42. [Google Scholar] [CrossRef] [PubMed]
- Vincent, S.; Subramanian, S.; Vollmer, F. Optoplasmonic Characterisation of Reversible Disulfide Interactions at Single Thiol Sites in the Attomolar Regime. Nat. Commun. 2020, 11, 2043. [Google Scholar] [CrossRef] [PubMed]
- Xavier, J.; Vincent, S.; Meder, F.; Vollmer, F. Advances in Optoplasmonic Sensors—Combining Optical Nano/Microcavities and Photonic Crystals with Plasmonic Nanostructures and Nanoparticles. Nanophotonics 2018, 7, 1–38. [Google Scholar] [CrossRef]
- Vollmer, F.; Braun, D.; Libchaber, A.; Khoshsima, M.; Teraoka, I.; Arnold, S. Protein Detection by Optical Shift of a Resonant Microcavity. Appl. Phys. Lett. 2002, 80, 4057–4059. [Google Scholar] [CrossRef]
- Xu, X.; Chen, W.; Zhao, G.; Li, Y.; Lu, C.; Yang, L. Wireless Whispering-Gallery-Mode Sensor for Thermal Sensing and Aerial Mapping. Light Sci. Appl. 2018, 7, 62. [Google Scholar] [CrossRef] [Green Version]
- Dong, C.; He, L.; Xiao, Y.; Gaddam, V.R.; Ozdemir, S.K.; Han, Z.; Guo, G.; Yang, L.; Dong, C.; He, L.; et al. Fabrication of High-Q Polydimethylsiloxane Optical Microspheres for Thermal Sensing. Appl. Phys. Lett. 2009, 94, 231119. [Google Scholar] [CrossRef]
- Subramanian, S.; Kalani Perera, K.M.; Pedireddy, S.; Vollmer, F. Optoplasmonic Whispering Gallery Mode Sensors for Single Molecule Characterization: A Practical Guide. In Single Molecule Sensing Beyond Fluorescence; Springer International Publishing: Berlin/Heidelberg, Germany, 2022; pp. 37–96. [Google Scholar]
- Yu, D.; Humar, M.; Meserve, K.; Bailey, R.C.; Chormaic, S.N.; Vollmer, F. Whispering-Gallery-Mode Sensors for Biological and Physical Sensing. Nat. Rev. Methods Prim. 2021, 1, 83. [Google Scholar] [CrossRef]
- Batson, P.E. Plasmonic Modes Revealed. Science 2012, 335, 47–48. [Google Scholar] [CrossRef]
- Vahala, K.J. Optical Microcavities. In Proceedings of the EQEC '05. European Quantum Electronics Conference, Munich, Germany, 12–17 June 2005; p. 352. [Google Scholar] [CrossRef]
- Foreman, M.R.; Swaim, J.D.; Vollmer, F. Whispering Gallery Mode Sensors. Adv. Opt. Photonics 2015, 7, 168. [Google Scholar] [CrossRef] [Green Version]
- Santiago-Cordoba, M.A.; Cetinkaya, M.; Boriskina, S.V.; Vollmer, F.; Demirel, M.C. Ultrasensitive Detection of a Protein by Optical Trapping in a Photonic-Plasmonic Microcavity. J. Biophotonics 2012, 5, 629–638. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Baaske, M.D.; Foreman, M.R.; Vollmer, F. Single-molecule nucleic acid interactions monitored on a label-free microcavity biosensor platform. Nat. Nanotechnol. 2014, 9, 933–939. [Google Scholar] [CrossRef] [PubMed]
- Zhang, Y.N.; Zhou, T.; Han, B.; Zhang, A.; Zhao, Y. Optical Bio-Chemical Sensors Based on Whispering Gallery Mode Resonators. Nanoscale 2018, 10, 13832–13856. [Google Scholar] [CrossRef] [PubMed]
- Arnold, S.; Khoshsima, M.; Teraoka, I.; Holler, S.; Vollmer, F. Shift of Whispering-Gallery Modes in Microspheres by Protein Adsorption. Opt. Lett. 2003, 28, 272. [Google Scholar] [CrossRef] [Green Version]
- Vollmer, F.; Arnold, S.; Keng, D. Single Virus Detection from the Reactive Shift of a Whispering-Gallery Mode. Proc. Natl. Acad. Sci. USA 2008, 105, 20701–20704. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Eerqing, N.; Subramanian, S.; Rubio, J.; Lutz, T.; Wu, H.Y.; Anders, J.; Soeller, C.; Vollmer, F. Comparing Transient Oligonucleotide Hybridization Kinetics Using DNA-PAINT and Optoplasmonic Single-Molecule Sensing on Gold Nanorods. ACS Photonics 2021, 8, 2882–2888. [Google Scholar] [CrossRef]
- Kim, E.; Baaske, M.D.; Vollmer, F. In Situ Observation of Single-Molecule Surface Reactions from Low to High Affinities. Adv. Mater. 2016, 28, 9941–9948. [Google Scholar] [CrossRef]
- Shopova, S.I.; Rajmangal, R.; Holler, S.; Arnold, S. Plasmonic Enhancement of a Whispering-Gallery-Mode Biosensor for Single Nanoparticle Detection. Appl. Phys. Lett. 2011, 98, 243104. [Google Scholar] [CrossRef] [Green Version]
- Baaske, M.D.; Vollmer, F. Optical Observation of Single Atomic Ions Interacting with Plasmonic Nanorods in Aqueous Solution. Nat. Photonics 2016, 10, 733–739. [Google Scholar] [CrossRef]
- Ament, I.; Prasad, J.; Henkel, A.; Schmachtel, S.; Sönnichsen, C. Single Unlabeled Protein Detection on Individual Plasmonic Nanoparticles. Nano Lett. 2012, 12, 1092–1095. [Google Scholar] [CrossRef] [PubMed]
- Chen, H.; Shao, L.; Li, Q.; Wang, J. Gold Nanorods and Their Plasmonic Properties. Chem. Soc. Rev. 2013, 42, 2679–2724. [Google Scholar] [CrossRef] [PubMed]
- Soria, S.; Baldini, F.; Berneschi, S.; Cosi, F.; Giannetti, A.; Conti, G.N.; Pelli, S.; Righini, G.C.; Tiribilli, B. High-Q Polymer-Coated Microspheres for Immunosensing Applications. Opt. Express 2009, 17, 14694. [Google Scholar] [CrossRef] [PubMed]
- Malikova, N.; Pastoriza-Santos, I.; Schierhorn, M.; Kotov, N.A.; Liz-Marzán, L.M. Layer-by-Layer Assembled Mixed Spherical and Planar Gold Nanoparticles: Control of Interparticle Interactions. Langmuir 2002, 18, 3694–3697. [Google Scholar] [CrossRef]
- Toren, P.; Ozgur, E.; Bayindir, M. Oligonucleotide-Based Label-Free Detection with Optical Microresonators: Strategies and Challenges. Lab Chip 2016, 16, 2572–2595. [Google Scholar] [CrossRef]
- Vashist, S.K.; Lam, E.; Hrapovic, S.; Male, K.B.; Luong, J.H.T. Immobilization of Antibodies and Enzymes on 3-Aminopropyltriethoxysilane-Functionalized Bioanalytical Platforms for Biosensors and Diagnostics. Chem. Rev. 2014, 114, 11083–11130. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Howarter, J.A.; Youngblood, J.P. Optimization of Silica Silanization by 3-Aminopropyltriethoxysilane. Langmuir 2006, 22, 11142–11147. [Google Scholar] [CrossRef]
- Panich, S.; Wilson, K.A.; Nuttall, P.; Wood, C.K.; Albrecht, T.; Edel, J.B. Label-Free Pb(II) Whispering Gallery Mode Sensing Using Self-Assembled Glutathione-Modified Gold Nanoparticles on an Optical Microcavity. Anal. Chem. 2014, 86, 6299–6306. [Google Scholar] [CrossRef]
- Pasquardini, L.; Berneschi, S.; Barucci, A.; Cosi, F.; Dallapiccola, R.; Insinna, M.; Lunelli, L.; Conti, G.N.; Pederzolli, C.; Salvadori, S.; et al. Whispering Gallery Mode Aptasensors for Detection of Blood Proteins. J. Biophotonics 2013, 6, 178–187. [Google Scholar] [CrossRef]
- Ebner, A.; Wildling, L.; Zhu, R.; Rankl, C.; Haselgrübler, T.; Hinterdorfer, P.; Gruber, H.J. Functionalization of Probe Tips and Supports for Single-Molecule Recognition Force Microscopy. Top. Curr. Chem. 2008, 285, 29–76. [Google Scholar] [CrossRef]
- Mehtala, J.G.; Zemlyanov, D.Y.; Max, J.P.; Kadasala, N.; Zhao, S.; Wei, A. Citrate-Stabilized Gold Nanorods. Langmuir 2014, 30, 13727–13730. [Google Scholar] [CrossRef] [Green Version]
- Mosquera, J.; García, I.; Henriksen-Lacey, M.; González-Rubio, G.; Liz-Marzán, L.M. Reducing Protein Corona Formation and Enhancing Colloidal Stability of Gold Nanoparticles by Capping with Silica Monolayers. Chem. Mater. 2019, 31, 57–61. [Google Scholar] [CrossRef] [Green Version]
- Shah, K.W.; Sreethawong, T.; Liu, S.H.; Zhang, S.Y.; Tan, L.S.; Han, M.Y. Aqueous Route to Facile, Efficient and Functional Silica Coating of Metal Nanoparticles at Room Temperature. Nanoscale 2014, 6, 11273–11281. [Google Scholar] [CrossRef]
- Ulman, A. Formation and Structure of Self-Assembled Monolayers. Chem. Rev. 1996, 96, 1533–1554. [Google Scholar] [CrossRef] [PubMed]
- Pavlovic, E.; Quist, A.P.; Gelius, U.; Oscarsson, S. Surface Functionalization of Silicon Oxide at Room Temperature and Atmospheric Pressure. J. Colloid Interface Sci. 2002, 254, 200–203. [Google Scholar] [CrossRef]
- Rubio, N.; Au, H.; Leese, H.S.; Hu, S.; Clancy, A.J.; Shaffer, M.S.P. Grafting from versus Grafting to Approaches for the Functionalization of Graphene Nanoplatelets with Poly(Methyl Methacrylate). Macromolecules 2017, 50, 7070–7079. [Google Scholar] [CrossRef]
- Chanana, M.; Liz-Marzán, L.M. Coating Matters: The Influence of Coating Materials on the Optical Properties of Gold Nanoparticles. Nanophotonics 2012, 1, 199–220. [Google Scholar] [CrossRef]
- Iarlori, S.; Ceresoli, D.; Bernasconi, M.; Donadio, D.; Parrinello, M. Dehydroxylation and Silanization of the Surfaces of β-Cristobalite Silica: An Ab Initio Simulation. J. Phys. Chem. B 2001, 105, 8007–8013. [Google Scholar] [CrossRef]
- Zipoli, F.; Donadio, D.; Bernasconi, M. Simulation of the Grafting of Organosilanes at the Surface of Dry Amorphous Silica. J. Phys. Condens. Matter 2008, 20, 224011. [Google Scholar] [CrossRef]
- Dalstein, L.; Potapova, E.; Tyrode, E. The Elusive Silica/Water Interface: Isolated Silanols under Water as Revealed by Vibrational Sum Frequency Spectroscopy. Phys. Chem. Chem. Phys. 2017, 19, 10343–10349. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Antonio Alves Júnior, J.; Baptista Baldo, J. The Behavior of Zeta Potential of Silica Suspensions. New J. Glas. Ceram. 2014, 4, 29–37. [Google Scholar] [CrossRef] [Green Version]
- Ertl, G. Heterogeneous Catalysis on the Atomic Scale. Chem. Rec. 2001, 1, 33–45. [Google Scholar] [CrossRef] [PubMed]
- Rafti, M.; Imbihl, R. Excitability in the H2+O2reaction on a Rh(110) Surface Induced by High Coverages of Coadsorbed Potassium. J. Chem. Phys. 2014, 141, 214707. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Petronella, F.; De Biase, D.; Zaccagnini, F.; Verrina, V.; Lim, S.-I.; Jeong, K.-U.; Miglietta, S.; Petrozza, V.; Scognamiglio, V.; Godman, N.P.; et al. Label-free and reusable antibody-functionalized gold nanorod arrays for the rapid detection of Escherichia coli cells in a water dispersion. Environ. Sci. Nano 2022, 9, 3343–3360. [Google Scholar] [CrossRef]
- Subramanian, S.; Jones, H.B.L.; Frustaci, S.; Winter, S.; van der Kamp, M.W.; Arcus, V.L.; Pudney, C.R.; Vollmer, F. Sensing Enzyme Activation Heat Capacity at the Single-Molecule Level Using Gold-Nanorod-Based Optical Whispering Gallery Modes. ACS Appl. Nano Mater. 2021, 4, 4576–4583. [Google Scholar] [CrossRef] [PubMed]


Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Serrano, M.P.; Subramanian, S.; von Bilderling, C.; Rafti, M.; Vollmer, F. “Grafting-To” Covalent Binding of Plasmonic Nanoparticles onto Silica WGM Microresonators: Mechanically Robust Single-Molecule Sensors and Determination of Activation Energies from Single-Particle Events. Sensors 2023, 23, 3455. https://doi.org/10.3390/s23073455
Serrano MP, Subramanian S, von Bilderling C, Rafti M, Vollmer F. “Grafting-To” Covalent Binding of Plasmonic Nanoparticles onto Silica WGM Microresonators: Mechanically Robust Single-Molecule Sensors and Determination of Activation Energies from Single-Particle Events. Sensors. 2023; 23(7):3455. https://doi.org/10.3390/s23073455
Chicago/Turabian StyleSerrano, Mariana P., Sivaraman Subramanian, Catalina von Bilderling, Matías Rafti, and Frank Vollmer. 2023. "“Grafting-To” Covalent Binding of Plasmonic Nanoparticles onto Silica WGM Microresonators: Mechanically Robust Single-Molecule Sensors and Determination of Activation Energies from Single-Particle Events" Sensors 23, no. 7: 3455. https://doi.org/10.3390/s23073455
APA StyleSerrano, M. P., Subramanian, S., von Bilderling, C., Rafti, M., & Vollmer, F. (2023). “Grafting-To” Covalent Binding of Plasmonic Nanoparticles onto Silica WGM Microresonators: Mechanically Robust Single-Molecule Sensors and Determination of Activation Energies from Single-Particle Events. Sensors, 23(7), 3455. https://doi.org/10.3390/s23073455





