Validation of Automated Countermovement Vertical Jump Analysis: Markerless Pose Estimation vs. 3D Marker-Based Motion Capture System
Abstract
1. Introduction
2. Materials and Methods
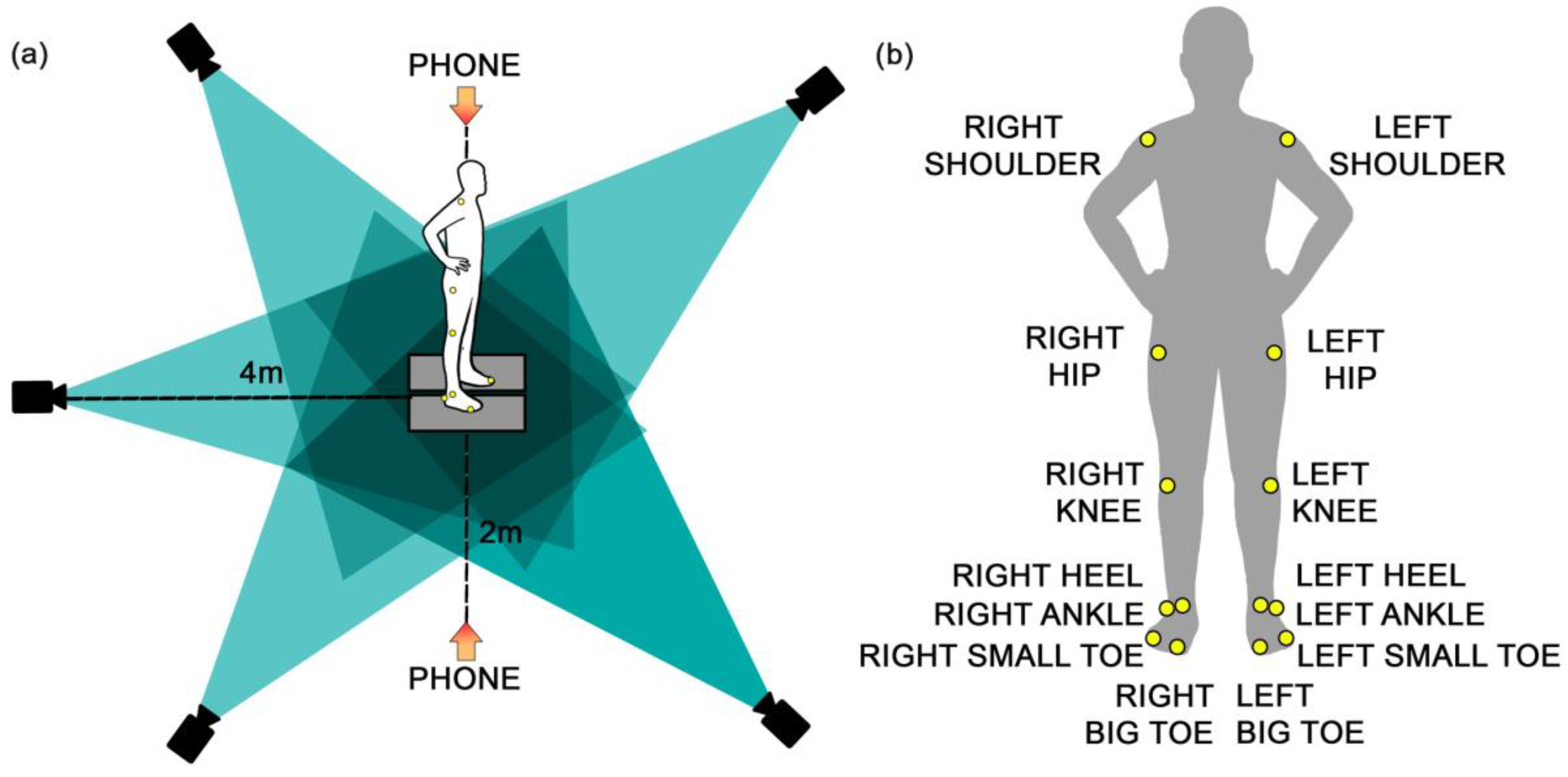
2.1. Experimental Session
2.2. Data Processing
Motion Capture Recordings
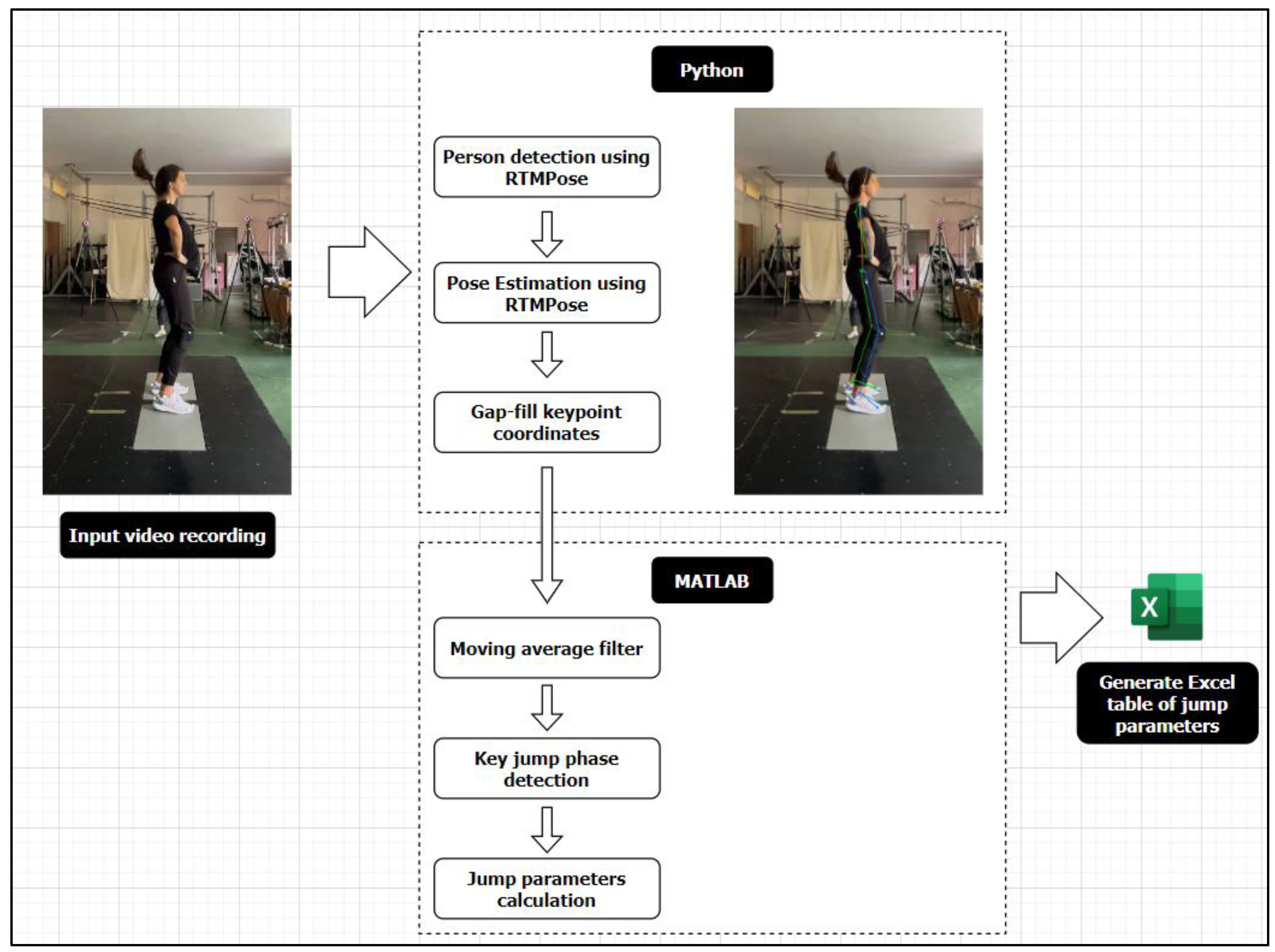
2.3. Markerless Data
2.4. Computation of CoM
2.5. Variables
2.6. Statistical Analysis
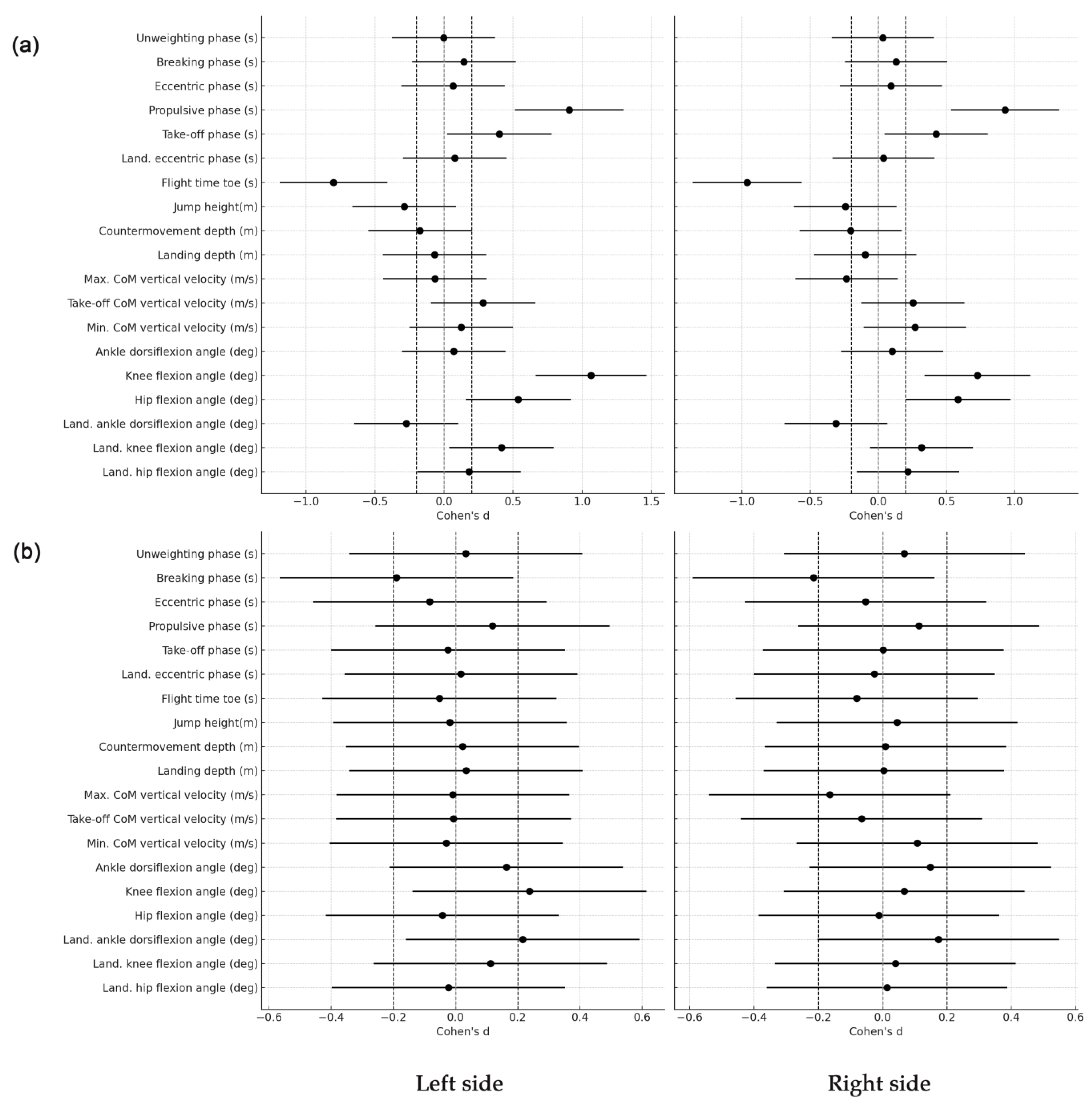
3. Results
4. Discussion
5. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Claudino, J.G.; Cronin, J.; Mezêncio, B.; McMaster, D.T.; McGuigan, M.; Tricoli, V.; Amadio, A.C.; Serrão, J.C. The countermovement jump to monitor neuromuscular status: A meta-analysis. J. Sci. Med. Sport 2017, 20, 397–402. [Google Scholar] [CrossRef]
- Pontillo, M.; Hines, S.M.; Sennett, B.J. Prediction of ACL injuries from vertical jump kinetics in Division 1 collegiate athletes. Int. J. Sports Phys. Ther. 2021, 16, 156–161. [Google Scholar] [CrossRef] [PubMed]
- Singh, B.; Kumar, A.; Ranga, M.D. Biomechanical analysis of explosive strength of legs of athletes. J. Exerc. Sci. Physiol. 2017, 13, 53–61. [Google Scholar] [CrossRef]
- Struzik, A. Biomechanical characteristics of the countermovement jump. In Measuring Leg Stiffness during Vertical Jumps; Springer: Cham, Switzerland, 2019; pp. 15–29. [Google Scholar]
- Lichtwark, G.A.; Schuster, R.W.; Kelly, L.A.; Trost, S.G.; Bialkowski, A. Markerless motion capture provides accurate predictions of ground reaction forces across a range of movement tasks. J. Biomech. 2024, 166, 112051. [Google Scholar] [CrossRef]
- Pueo, B.; Penichet-Tomás, A.; Jiménez-Olmedo, J.M. Validity, reliability and usefulness of smartphone and Kinovea motion analysis software for direct measurement of vertical jump height. Physiol. Behav. 2020, 227, 113144. [Google Scholar] [CrossRef] [PubMed]
- Anicic, Z.; Janicijevic, D.; Knezevic, O.M.; Garcia-Ramos, A.; Petrovic, M.R.; Cabarkapa, D.; Mirkov, D.M. Assessment of countermovement jump: What should we report? Life 2023, 13, 190. [Google Scholar] [CrossRef] [PubMed]
- Ceriola, L.; Mileti, I.; Donati, M.; Patanè, F. Comparison of video-based algorithms for 2D human kinematics estimation: A preliminary study. J. Phys. Conf. Ser. 2023, 2590, 012002. [Google Scholar] [CrossRef]
- Uhlrich, S.D.; Falisse, A.; Kidziński, Ł.; Muccini, J.; Ko, M.; Chaudhari, A.S.; Delp, S.L. OpenCap: Human movement dynamics from smartphone videos. PLoS Comput. Biol. 2023, 19, e1011462. [Google Scholar] [CrossRef]
- Lima, Y.; Collings, T.; Hall, M.; Bourne, M.; Diamond, L. Assessing lower-limb kinematics via OpenCap during dynamic tasks relevant to anterior cruciate ligament injury: A validity study. J. Sci. Med. Sport 2023, 26, S105. [Google Scholar] [CrossRef]
- Balsalobre-Fernández, C.; Glaister, M.; Lockey, R.A. The validity and reliability of an iPhone app for measuring vertical jump performance. J. Sports Sci. 2015, 33, 1574–1579. [Google Scholar] [CrossRef]
- Bishop, C.; Jarvis, P.; Turner, A.; Balsalobre-Fernandez, C. Validity and reliability of strategy metrics to assess countermovement jump performance using the newly developed smartphone application. J. Hum. Kinet. 2022, 83, 185–195. [Google Scholar] [CrossRef] [PubMed]
- Bridgeman, L.; Cameron, B.; Steele, J. The Validity and Reliability of the My Jump Lab Artificial Intelligence Application. SportRxiv 2024. [Google Scholar] [CrossRef]
- Gençoğlu, C.; Ulupınar, S.; Özbay, S.; Turan, M.; Savaş, B.Ç.; Asan, S.; İnce, İ. Validity and reliability of “My Jump app” to assess vertical jump performance: A meta-analytic review. Sci. Rep. 2023, 13, 20137. [Google Scholar] [CrossRef] [PubMed]
- Sharp, A.; Cronin, J.; Neville, J. Utilizing smartphones for jump diagnostics: A brief review of the validity and reliability of the My Jump app. Strength Cond. J. 2019, 41, 1. [Google Scholar] [CrossRef]
- Lombard, W.; Reid, S.; Pearson, K.; Lambert, M. Reliability of metrics associated with a counter-movement jump performed on a force plate. Meas. Phys. Educ. Exerc. Sci. 2017, 21, 235–243. [Google Scholar] [CrossRef]
- Cao, Z.; Hidalgo, G.; Simon, T.; Wei, S.E.; Sheikh, Y. OpenPose: Realtime Multi-Person 2D Pose Estimation Using Part Affinity Fields. IEEE Trans. Pattern Anal. Mach. Intell. 2021, 43, 172–186. [Google Scholar] [CrossRef]
- Bazarevsky, V.; Grishchenko, I.; Raveendran, K.; Zhu, T.; Zhang, F.; Grundmann, M. BlazePose: On-device real-time body pose tracking. arXiv 2020, arXiv:2006.10204. [Google Scholar]
- Badiola-Bengoa, A.; Mendez-Zorrilla, A. A systematic review of the application of camera-based human pose estimation in the field of sport and physical exercise. Sensors 2021, 21, 5996. [Google Scholar] [CrossRef]
- Mundt, M.; Born, Z.; Goldacre, M.; Alderson, J. Estimating ground reaction forces from two-dimensional pose data: A biomechanics-based comparison of AlphaPose, BlazePose, and OpenPose. Sensors 2023, 23, 78. [Google Scholar] [CrossRef]
- Walsh, M.; Boling, M.C.; McGrath, M.; Blackburn, J.T.; Padua, D.A. Lower extremity muscle activation and knee flexion during a jump-landing task. J. Athl. Train. 2012, 47, 406–413. [Google Scholar] [CrossRef]
- Eagles, A.N.; Sayers, M.G.L.; Bousson, M.; Lovell, D.I. Current methodologies and implications of phase identification of the vertical jump: A systematic review and meta-analysis. Sports Med. 2015, 45, 1311–1323. [Google Scholar] [CrossRef]
- Jiang, T.; Lu, P.; Zhang, L.; Ma, N.; Han, R.; Lyu, C.; Li, Y.; Chen, K. RTMPose: Real-time multi-person pose estimation based on MMPose. arXiv 2023, arXiv:2303.07399. [Google Scholar]
- D’Antonio, E.; Taborri, J.; Mileti, I.; Rossi, S.; Patanè, F. Validation of a 3D markerless system for gait analysis based on OpenPose and two RGB webcams. IEEE Sens. J. 2021, 21, 17064–17075. [Google Scholar] [CrossRef]
- Hii, C.S.T.; Gan, K.B.; Zainal, N.; Mohamed Ibrahim, N.; Azmin, S.; Mat Desa, S.H.; van de Warrenburg, B.; You, H.W. Automated gait analysis based on a marker-free pose estimation model. Sensors 2023, 23, 6489. [Google Scholar] [CrossRef] [PubMed]
- Van Hooren, B.; Pecasse, N.; Meijer, K.; Essers, J.M.N. The accuracy of markerless motion capture combined with computer vision techniques for measuring running kinematics. Scand. J. Med. Sci. Sports 2023, 33, 966–978. [Google Scholar]
- Needham, L.; Evans, M.; Cosker, D.P.; Wade, L.; McGuigan, P.M.; Bilzon, J.L.; Colyer, S.L. The accuracy of several pose estimation methods for 3D joint centre localisation. Sci. Rep. 2021, 11, 20673. [Google Scholar] [CrossRef] [PubMed]
- Serrancoli, G.; Bogatikov, P.; Huix, J.P.; Barbera, A.F.; Egea, A.J.S.; Ribe, J.T.; Kanaan-Izquierdo, S.; Susin, A. Marker-less monitoring protocol to analyze biomechanical joint metrics during pedaling. IEEE Access 2020, 8, 122782–122790. [Google Scholar] [CrossRef]
- Szucs, G.; Tamás, B. Body part extraction and pose estimation method in rowing videos. J. Comput. Inf. Technol. 2018, 26, 29–43. [Google Scholar] [CrossRef][Green Version]
- Turner, J.A.; Chaaban, C.R.; Padua, D.A. Validation of OpenCap: A low-cost markerless motion capture system for lower-extremity kinematics during return-to-sport tasks. J. Biomech. 2024, 171, 112200. [Google Scholar] [CrossRef]
- Verheul, J.; Robinson, M.A.; Burton, S. Jumping towards field-based ground reaction force estimation and assessment with OpenCap. J. Biomech. 2024, 166, 112044. [Google Scholar] [CrossRef]
- Balsalobre-Fernández, C. Real-time estimation of vertical jump height with a markerless motion capture smartphone app: A proof-of-concept case study. Proc. Inst. Mech. Eng. Part P J. Sports Eng. Technol. 2023. [Google Scholar] [CrossRef]
- Ceseracciu, E.; Sawacha, Z.; Cobelli, C. Comparison of markerless and marker-based motion capture technologies through simultaneous data collection during gait: Proof of concept. PLoS ONE 2014, 9, e87640. [Google Scholar] [CrossRef] [PubMed]
- MMPose Contributors. Openmmlab Pose Estimation Toolbox and Benchmark. Available online: https://github.com/open-mmlab/mmpose/blob/main/demo/docs/en/2d_human_pose_demo.md (accessed on 4 August 2024).
- Petronijevic, M.S.; Garcia Ramos, A.; Mirkov, D.M.; Jaric, S.; Valdevit, Z.; Knezevic, O.M. Self-Preferred Initial Position Could Be a Viable Alternative to the Standard Squat Jump Testing Procedure. J. Strength Cond. Res. 2018, 32, 3267–3275. [Google Scholar] [CrossRef] [PubMed]
- Winter, E.M.; Abt, G.; Brookes, F.B.C.; Challis, J.H.; Fowler, N.E.; Knudson, D.V.; Knuttgen, H.G.; Kraemer, W.J.; Lane, A.M.; van Mechelen, W.; et al. Misuse of “power” and other mechanical terms in sport and exercise science research. J. Strength Cond. Res. 2016, 30, 292–300. [Google Scholar] [CrossRef] [PubMed]
- Bland, J.M.; Altman, D.G. Statistical methods for assessing agreement between two methods of clinical measurement. Lancet 1986, 1, 307–310. [Google Scholar] [CrossRef]
- Hinkle, D.E.; Wiersma, W.; Jurs, S.G. Applied Statistics for the Behavioral Sciences, 5th ed.; Houghton Mifflin: Boston, MA, USA, 2003. [Google Scholar]
- Klein, R. Bland-Altman and Correlation Plot. Available online: https://www.mathworks.com/matlabcentral/fileexchange/45049-bland-altman-and-correlation-plot (accessed on 26 August 2024).
- Cohen, J. Statistical Power Analysis for the Behavioral Sciences; Routledge Academic: New York, NY, USA, 1988. [Google Scholar]
- Boldo, M.; Di Marco, R.; Martini, E.; Nardon, M.; Bertucco, M.; Bombieri, N. On the reliability of single-camera markerless systems for overground gait monitoring. Comput. Biol. Med. 2024, 171, 108101. [Google Scholar] [CrossRef] [PubMed]
- Cronin, N.J. Using deep neural networks for kinematic analysis: Challenges and opportunities. J. Biomech. 2021, 123, 110460. [Google Scholar] [CrossRef]
- Ito, N.; Sigurðsson, H.B.; Seymore, K.D.; Arhos, E.K.; Buchanan, T.S.; Snyder-Mackler, L.; Silbernagel, K.G. Markerless motion capture: What clinician-scientists need to know right now. JSAMS Plus 2022, 1, 100001. [Google Scholar] [CrossRef]
- Tang, H.; Munkasy, B.; Li, L. Differences between lower extremity joint running kinetics captured by marker-based and markerless systems were speed dependent. J. Sport Health Sci. 2024, 13, 569–578. [Google Scholar] [CrossRef]
- Aderinola, T.B.; Younesian, H.; Whelan, D.; Caulfield, B.; Ifrim, G. Quantifying jump height using markerless motion capture with a single smartphone. IEEE Open J. Eng. Med. Biol. 2023, 4, 109–115. [Google Scholar] [CrossRef]
- Strutzenberger, G.; Kanko, R.; Selbie, S.; Schwameder, H.; Deluzio, K. Assessment of kinematic CMJ data using a deep learning algorithm-based markerless motion capture system. ISBS Proc. Arch. 2021, 39, 61. [Google Scholar]
- Das, K.; de Paula Oliveira, T.; Newell, J. Comparison of markerless and marker-based motion capture systems using 95% functional limits of agreement in a linear mixed-effects modelling framework. Sci. Rep. 2023, 13, 22880. [Google Scholar] [CrossRef] [PubMed]
- Wade, L.; Needham, L.; McGuigan, P.; Bilzon, J. Applications and limitations of current markerless motion capture methods for clinical gait biomechanics. PeerJ 2022, 10, e12995. [Google Scholar] [CrossRef] [PubMed]


| Variable (Unit) | Description |
|---|---|
| Unweighting phase (s) | The time interval between point a (start of the movement) and point c (start of the braking phase) in the CoM Z-t trajectory. |
| Breaking phase (s) | The time interval between point c (start of the braking phase) and point d (end of the eccentric phase) in the CoM Z-t trajectory. |
| Eccentric phase (s) | The time interval between point a (start of the movement) and point d (end of the eccentric phase) in the CoM Z-t trajectory. |
| Propulsive phase (s) | The time interval between point d (end of the eccentric phase) and point f (take-off) in the CoM Z-t trajectory. |
| Take-off phase (s) | The time interval between point a (start of the movement) and point f (take-off) in the CoM Z-t trajectory. |
| LD eccentric phase (s) | The time interval between point h (landing) and point i (end of the landing phase) in the CoM Z-t trajectory. |
| Flight time (s) | The time interval between point f (take-off) and point h (landing) in the Toe Z-t trajectory. |
| Jump height (m) | Maximum vertical displacement of the CoM relative to the initial resting position. |
| Countermovement depth (m) | Minimum vertical displacement of the CoM relative to the initial resting position during the push-off phase. |
| LD depth (m) | Minimum vertical displacement of the CoM relative to the initial resting position during the landing phase. |
| Max CoM vertical velocity (m/s) | Maximum vertical velocity of the CoM during the movement. |
| Take-off CoM vertical velocity (m/s) | Vertical velocity of the CoM at the moment of take-off. |
| Min CoM vertical velocity (m/s) | Minimum vertical velocity of the CoM during the movement. |
| Ankle dorsiflexion angle (deg) | The ankle dorsiflexion angle during the transition from the eccentric to the concentric phase of the push-off. |
| Knee flexion angle (deg) | The knee flexion angle during the transition from the eccentric to the concentric phase of the push-off. |
| Hip flexion angle (deg) | The hip flexion angle during the transition from the eccentric to the concentric phase of the push-off. |
| LD ankle dorsiflexion angle (deg) | The ankle dorsiflexion angle during the transition from the eccentric to the concentric landing phase. |
| LD knee flexion angle (deg) | The knee flexion angle during the transition from the eccentric to the concentric landing phase. |
| LD hip flexion angle (deg) | The hip flexion angle during the transition from the eccentric to the concentric phase of landing. |
| Jump height from flight time (m) | Jump height calculated based on the flight time. |
| Jump height—CoM take-off velocity (m) | Jump height calculated based on the vertical velocity of the CoM at take-off. |
| Jump height—CoM max. velocity (m) | Jump height calculated based on the maximum vertical velocity of the CoM. |
| Bias | LoA Lower | LoA Upper | RMSE | Correlation (r) | ||
|---|---|---|---|---|---|---|
| Toe Z-t (m) | Left | −0.021 (−0.024 ÷ −0.017) | −0.073 (−0.081 ÷ −0.065) | 0.032 (0.027 ÷ 0.036) | 0.035 (0.031 ÷ 0.038) | 0.992 (0.988 ÷ 0.994) |
| Right | −0.020 (−0.024 ÷ −0.017) | −0.070 (−0.077 ÷ −0.063) | 0.030 (0.026 ÷ 0.034) | 0.034 (0.031 ÷ 0.037) | 0.993 (0.990 ÷ 0.994) | |
| CoM Z-t (m) | Left | 0.000 (−0.004 ÷ 0.003) | −0.035 (−0.041 ÷ −0.030) | 0.035 (0.025 ÷ 0.045) | 0.021 (0.016 ÷ 0.025) | 0.999 (0.998 ÷ 0.999) |
| Right | −0.001 (−0.004 ÷ 0.002) | −0.040 (−0.044 ÷ −0.035) | 0.037 (0.030 ÷ 0.045) | 0.022 (0.019 ÷ 0.025) | 0.998 (0.998 ÷ 0.999) | |
| Ankle Angle-t (deg) | Left | −0.49 (−1.45 ÷ 0.5) | −8.6 (−9.7 ÷ −7.6) | 7.7 (6.6 ÷ 8.8) | 5.4 (5.1 ÷ 5.8) | 0.984 (0.982 ÷ 0.986) |
| Right | −0.67 (−1.96 ÷ 0.62) | −9.5 (−10.9 ÷ −8.1) | 8.143 (6.775 ÷ 9.511) | 6.487 (6.019 ÷ 6.956) | 0.981 (0.978 ÷ 0.983) | |
| Knee Angle-t (deg) | Left | 2.6 (1.4 ÷ 3.8) | −6.7 (−7.9 ÷ −5.5) | 11.9 (10.2 ÷ 13.6) | 6.9 (6.3 ÷ 7.5) | 0.994 (0.993 ÷ 0.995) |
| Right | 2.01 (0.57 ÷ 3.44) | −7.3 (−8.7 ÷ −5.9) | 11.309 (9.440 ÷ 13.178) | 7.163 (6.458 ÷ 7.868) | 0.994 (0.993 ÷ 0.995) | |
| Hip Angle-t (deg) | Left | 3.9 (2.5 ÷ 5.4) | −6.1 (−8.0 ÷ −4.288) | 14.024 (12 ÷ 16) | 8.0 (6.8 ÷ 9.1) | 0.997 (0.996 ÷ 0.997) |
| Right | 5.6 (3.8 ÷ 7.4) | −3.9 (−5.9 ÷ −1.8) | 15 (12 ÷ 17) | 8.8 (7.3 ÷ 10.0) | 0.996 (0.995 ÷ 0.997) |
| Marker-Based | Markerless | System | Side | Interaction | ICC (95%CI) | ||||
|---|---|---|---|---|---|---|---|---|---|
| Variable | Left | Right | Left | Right | F(1, 110) | F(1, 110) | F(1, 110) | Left | Right |
| Unweighting phase (s) | 0.241 (0.043) | 0.239 (0.044) | 0.241 (0.047) | 0.238 (0.043) | 0.070 | 0.077 | 0.115 | 0.912 (0.851 ÷ 0.949) | 0.914 (0.853 ÷ 0.949) |
| Breaking phase (s) | 0.178 (0.032) | 0.185 (0.039) | 0.173 (0.029) | 0.180 (0.032) | 5.171 * | 1.254 | 0.003 | 0.948 (0.912 ÷ 0.970) | 0.838 (0.723 ÷ 0.905) |
| Eccentric phase (s) | 0.419 (0.065) | 0.424 (0.066) | 0.414 (0.067) | 0.418 (0.068) | 7.537 * | 0.131 | 0.236 | 0.965 (0.940 ÷ 0.979) | 0.988 (0.979 ÷ 0.993) |
| Propulsive phase (s) | 0.243 (0.038) | 0.239 (0.039) | 0.210 (0.036) | 0.206 (0.031) | 659.964 ** | 0.405 | 0.045 | 0.982 (0.969 ÷ 0.989) | 0.941 (0.900 ÷ 0.966) |
| Take-off phase (s) | 0.662 (0.092) | 0.664 (0.096) | 0.624 (0.096) | 0.625 (0.094) | 370.717 ** | 0.007 | 0.136 | 0.985 (0.974 ÷ 0.991) | 0.990 (0.983 ÷ 0.994) |
| LD eccentric phase (s) | 0.260 (0.127) | 0.258 (0.124) | 0.249 (0.135) | 0.253 (0.128) | 22.443 ** | 0.001 | 3.173 | 0.995 (0.992 ÷ 0.997) | 0.996 (0.994 ÷ 0.998) |
| Flight time toe (s) | 0.547 (0.065) | 0.553 (0.059) | 0.608 (0.086) | 0.616 (0.070) | 351.538 ** | 0.292 | 0.091 | 0.944 (0.904 ÷ 0.968) | 0.926 (0.873 ÷ 0.957) |
| Jump height (m) | 0.340 (0.076) | 0.342 (0.068) | 0.362 (0.074) | 0.359 (0.073) | 90.965 ** | 0.005 | 1.280 | 0.971 (0.950 ÷ 0.983) | 0.986 (0.975 ÷ 0.992) |
| Countermovement depth (m) | 0.292 (0.079) | 0.291 (0.065) | 0.306 (0.073) | 0.305 (0.075) | 16.156 ** | 0.007 | 0.021 | 0.923 (0.868 ÷ 0.955) | 0.949 (0.913 ÷ 0.970) |
| LD depth (m) | 0.24 (0.13) | 0.24 (0.12) | 0.25 (0.13) | 0.25 (0.14) | 27.490 ** | 0.009 | 0.804 | 0.995 (0.992 ÷ 0.997) | 0.991 (0.984 ÷ 0.995) |
| Max. CoM vertical velocity (m/s) | 2.33 (0.31) | 2.33 (0.27) | 2.35 (0.29) | 2.39 (0.27) | 20.242 ** | 0.212 | 5.519 * | 0.961 (0.934 ÷ 0.977) | 0.979 (0.964 ÷ 0.988) |
| Take-off CoM vertical velocity (m/s) | 2.33 (0.30) | 2.33 (0.26) | 2.25 (0.27) | 2.27 (0.26) | 34.395 ** | 0.057 | 0.771 | 0.945 (0.905 ÷ 0.968) | 0.950 (0.914 ÷ 0.971) |
| Min. CoM vertical velocity (m/s) | −2.23 (0.30) | −2.22 (0.28) | −2.27 (0.31) | −2.30 (0.30) | 68.549 ** | 0.047 | 8.655 * | 0.987 (0.977 ÷ 0.992) | 0.981 (0.968 ÷ 0.989) |
| Ankle dorsiflexion angle (deg) | 71 (5) | 71 (5) | 71 (7) | 70 (6) | 0.892 | 0.851 | 0.024 | 0.812 (0.680 ÷ 0.890) | 0.571 (0.269 ÷ 0.749) |
| Knee flexion angle (deg) | 105.2 (8.4) | 103.0 (10.4) | 96.8 (7.4) | 96.3 (7.8) | 170.555 ** | 0.825 | 2.245 | 0.846 (0.737 ÷ 0.909) | 0.861 (0.763 ÷ 0.918) |
| Hip flexion angle (deg) | 76 (20) | 76 (19) | 65 (18) | 66 (18) | 156.399 ** | 0.022 | 0.124 | 0.946 (0.908 ÷ 0.968) | 0.934 (0.888 ÷ 0.961) |
| LD ankle dorsiflexion angle (deg) | 71.3 (5.1) | 70.1 (5.2) | 72.7 (5.7) | 71.8 (5.3) | 8.200 * | 1.490 | 0.021 | 0.744 (0.563 ÷ 0.850) | 0.376 (−0.064 ÷ 0.634) |
| LD knee flexion angle (deg) | 109 (16) | 107 (18) | 102 (18) | 102 (18) | 128.975 ** | 0.161 | 1.111 | 0.970 (0.949 ÷ 0.983) | 0.971 (0.950 ÷ 0.983) |
| LD hip flexion angle (deg) | 96 (39) | 97 (39) | 89 (40) | 89 (40) | 118.316 ** | 0.001 | 0.997 | 0.992 (0.986 ÷ 0.995) | 0.990 (0.982 ÷ 0.994) |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Aleksic, J.; Kanevsky, D.; Mesaroš, D.; Knezevic, O.M.; Cabarkapa, D.; Bozovic, B.; Mirkov, D.M. Validation of Automated Countermovement Vertical Jump Analysis: Markerless Pose Estimation vs. 3D Marker-Based Motion Capture System. Sensors 2024, 24, 6624. https://doi.org/10.3390/s24206624
Aleksic J, Kanevsky D, Mesaroš D, Knezevic OM, Cabarkapa D, Bozovic B, Mirkov DM. Validation of Automated Countermovement Vertical Jump Analysis: Markerless Pose Estimation vs. 3D Marker-Based Motion Capture System. Sensors. 2024; 24(20):6624. https://doi.org/10.3390/s24206624
Chicago/Turabian StyleAleksic, Jelena, Dmitry Kanevsky, David Mesaroš, Olivera M. Knezevic, Dimitrije Cabarkapa, Branislav Bozovic, and Dragan M. Mirkov. 2024. "Validation of Automated Countermovement Vertical Jump Analysis: Markerless Pose Estimation vs. 3D Marker-Based Motion Capture System" Sensors 24, no. 20: 6624. https://doi.org/10.3390/s24206624
APA StyleAleksic, J., Kanevsky, D., Mesaroš, D., Knezevic, O. M., Cabarkapa, D., Bozovic, B., & Mirkov, D. M. (2024). Validation of Automated Countermovement Vertical Jump Analysis: Markerless Pose Estimation vs. 3D Marker-Based Motion Capture System. Sensors, 24(20), 6624. https://doi.org/10.3390/s24206624








