Unique Characteristics of Pulse-Echo Sensing Systems for Ultrasonic Immersion Testing in Harsh Environments
Abstract
:1. Introduction
2. Materials and Methods
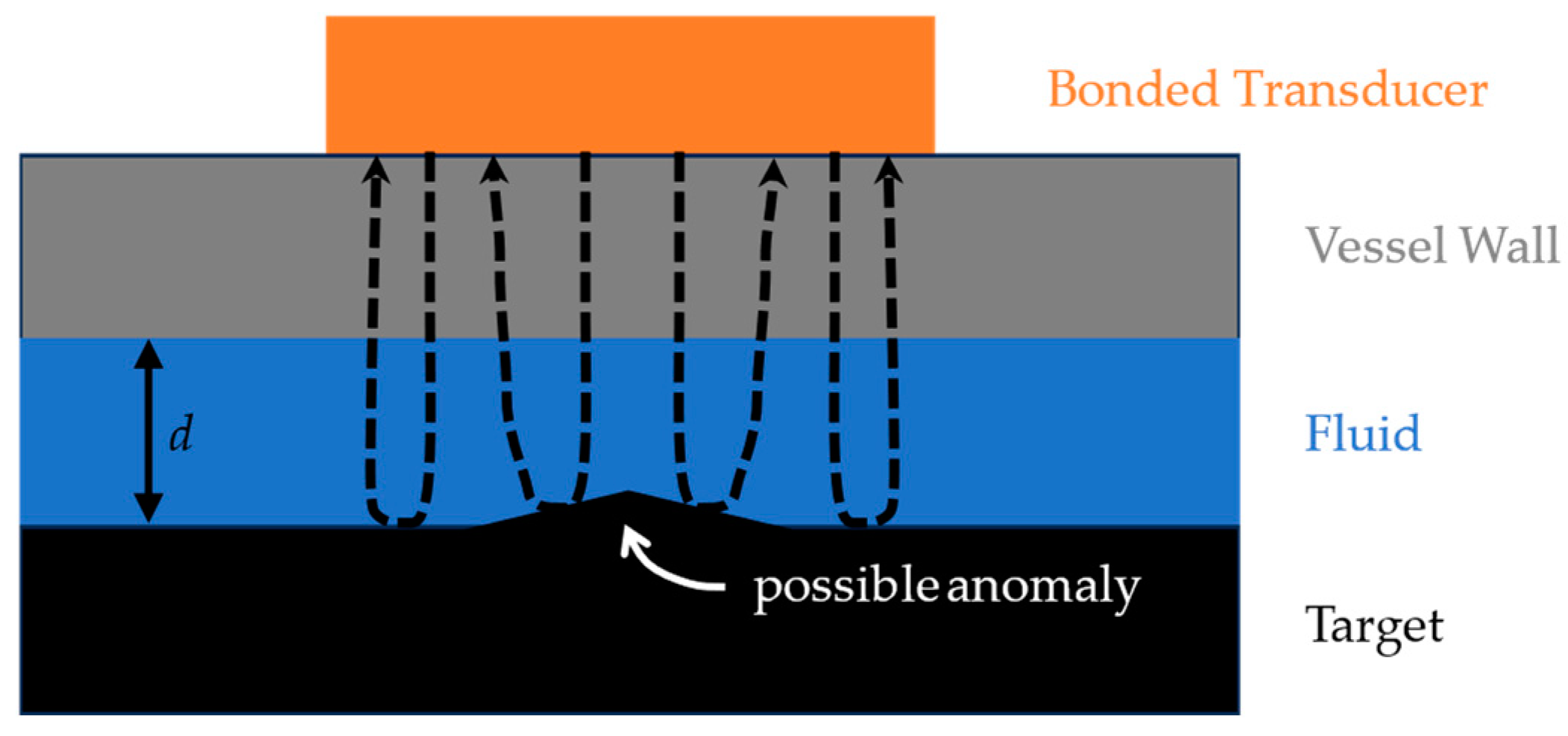
2.1. Pulse-Echo Ultrasound System
2.2. Modeling the Ultrasound System
2.2.1. Reduction to 2D Model
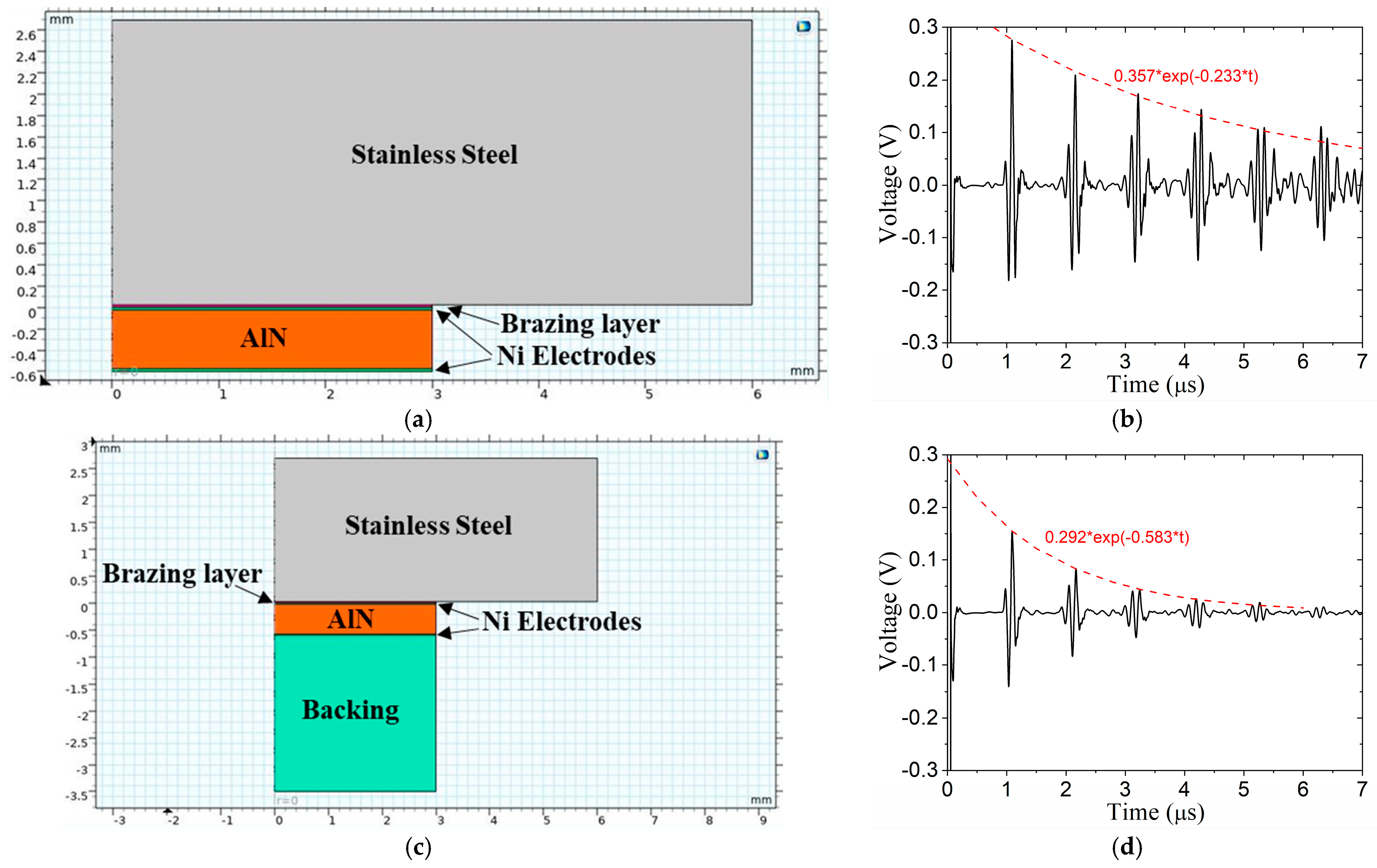
2.2.2. Effect of Backing
2.2.3. Effect of Electrode Thickness
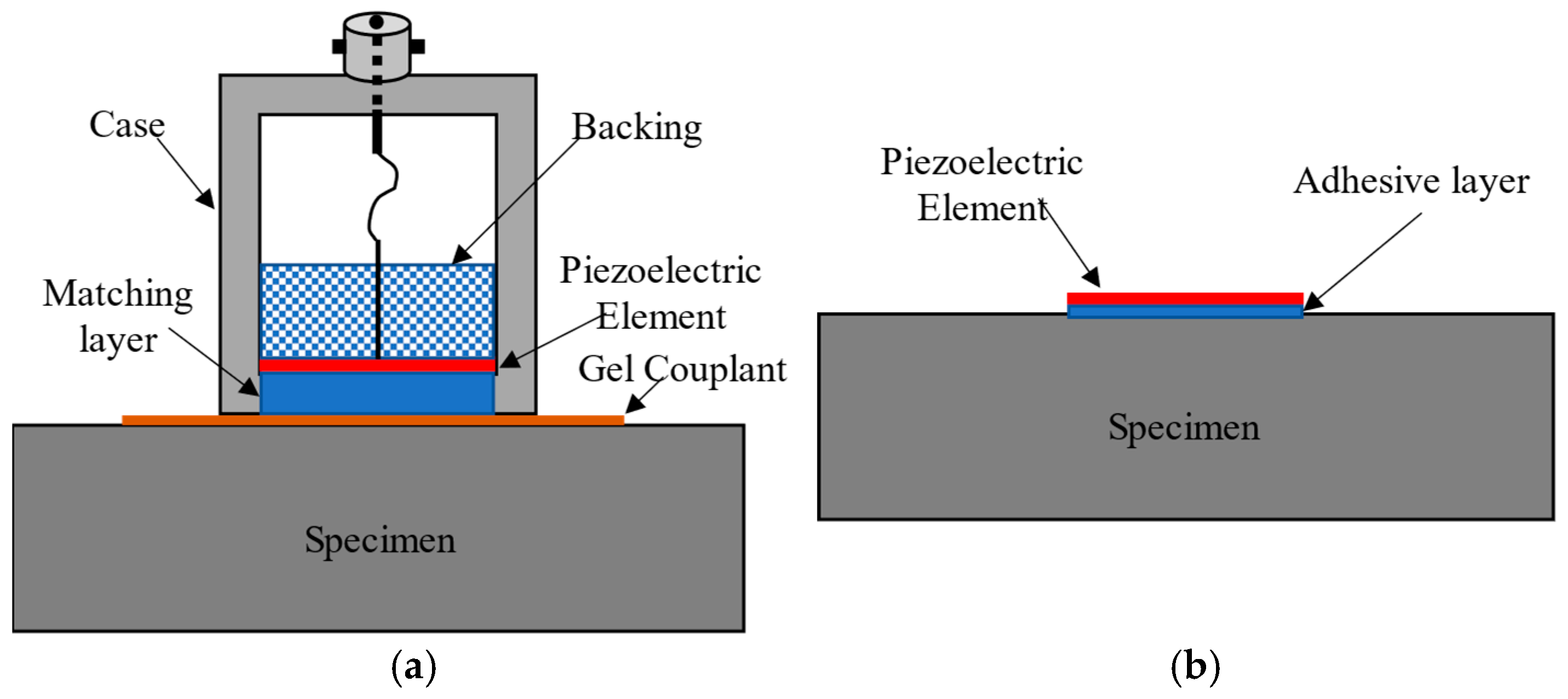
2.2.4. Effect of Coupling Material
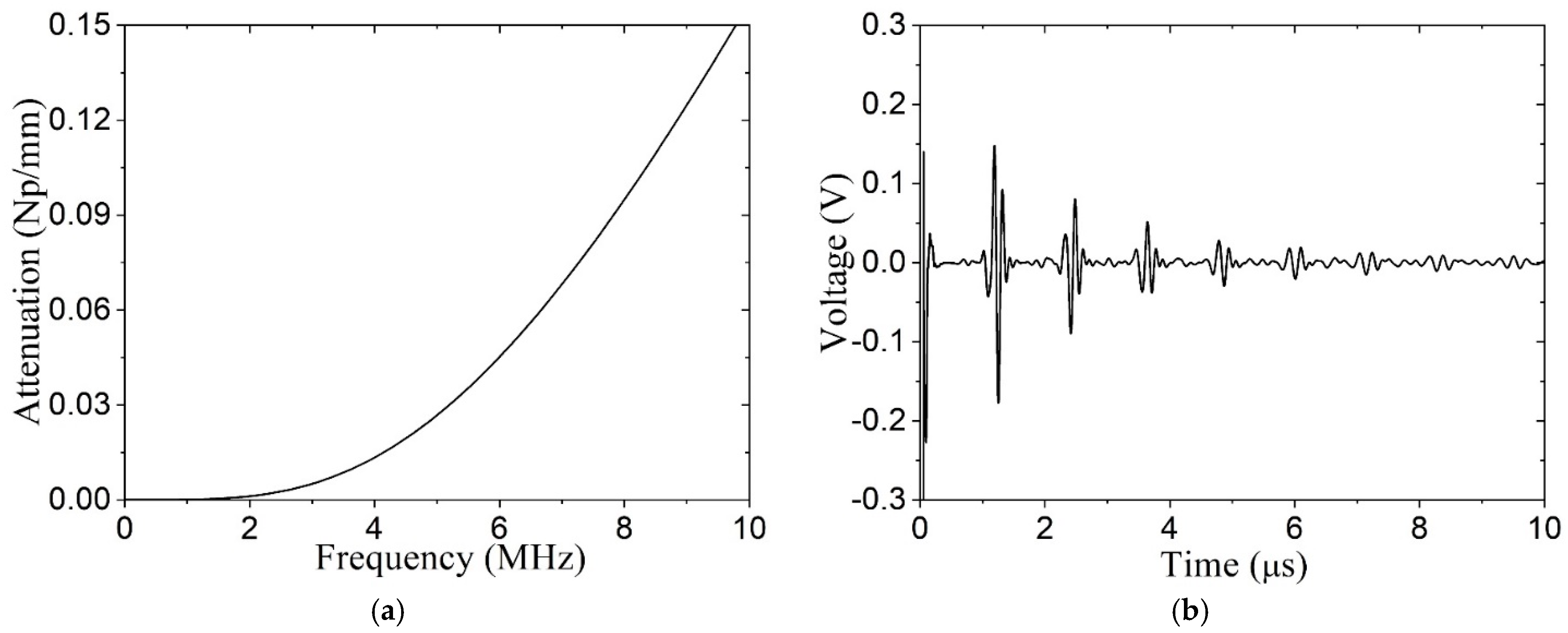
2.2.5. Effect of Grain Coarsening
2.3. Laboratory Testbed
- A surrogate for the vessel wall (henceforth known as the capsule), whose top surface is dry and has an AlN chip coupled to it with conductive silver paste. The chip dimensions are 6 × 6 × 0.55 mm, and the stainless-steel capsule thickness is 2.66 mm.
- A metal target that rests on the bottom of the water tank.
- Two blocks that set the water path between the capsule and target at one of three dimensions—2.33, 2.96, or 6.68 mm.
- Tests were conducted without and with an anomaly on the target’s surface. When the anomaly is present, it is positioned directly in line with the transducer.
3. Results
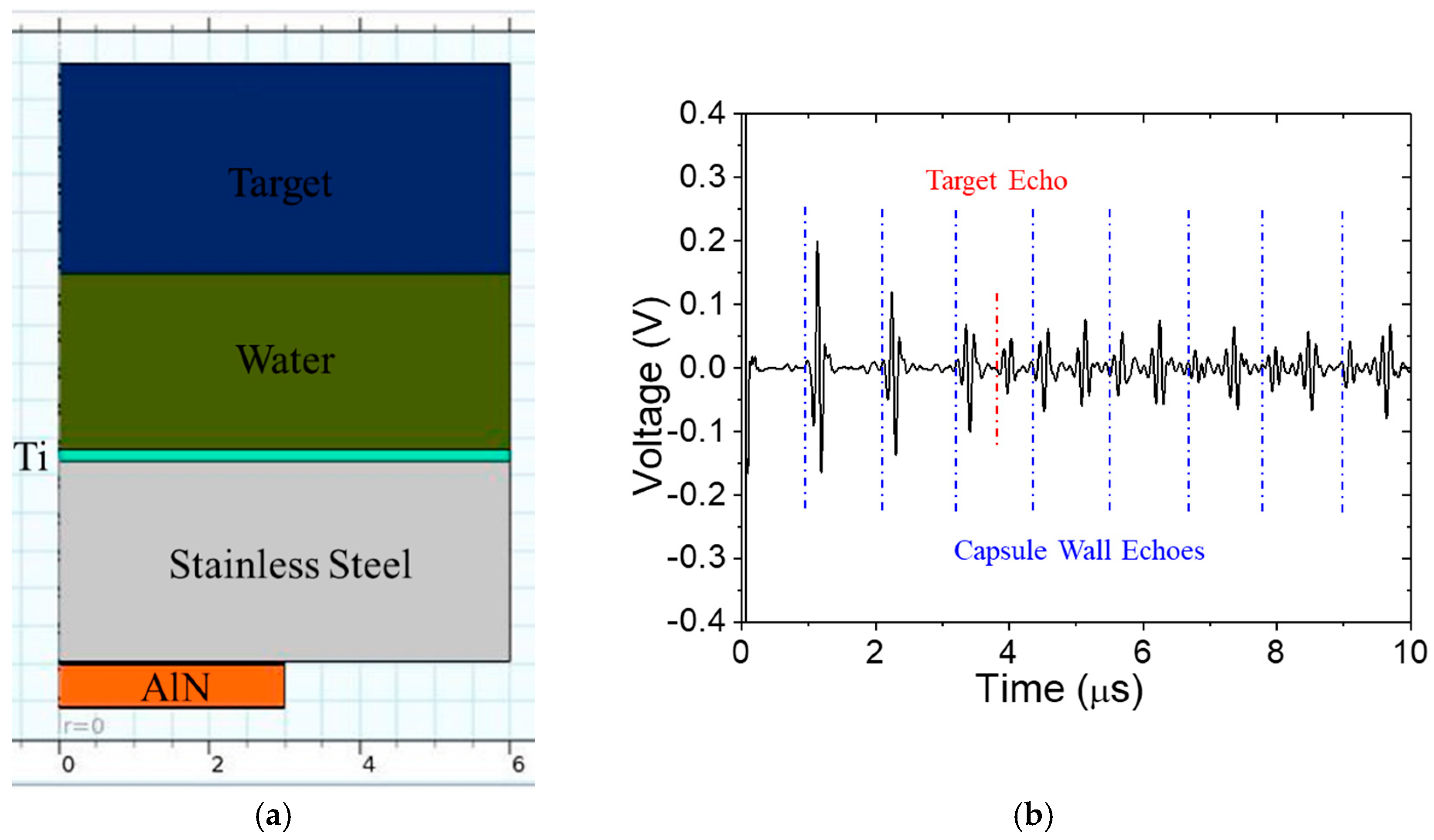
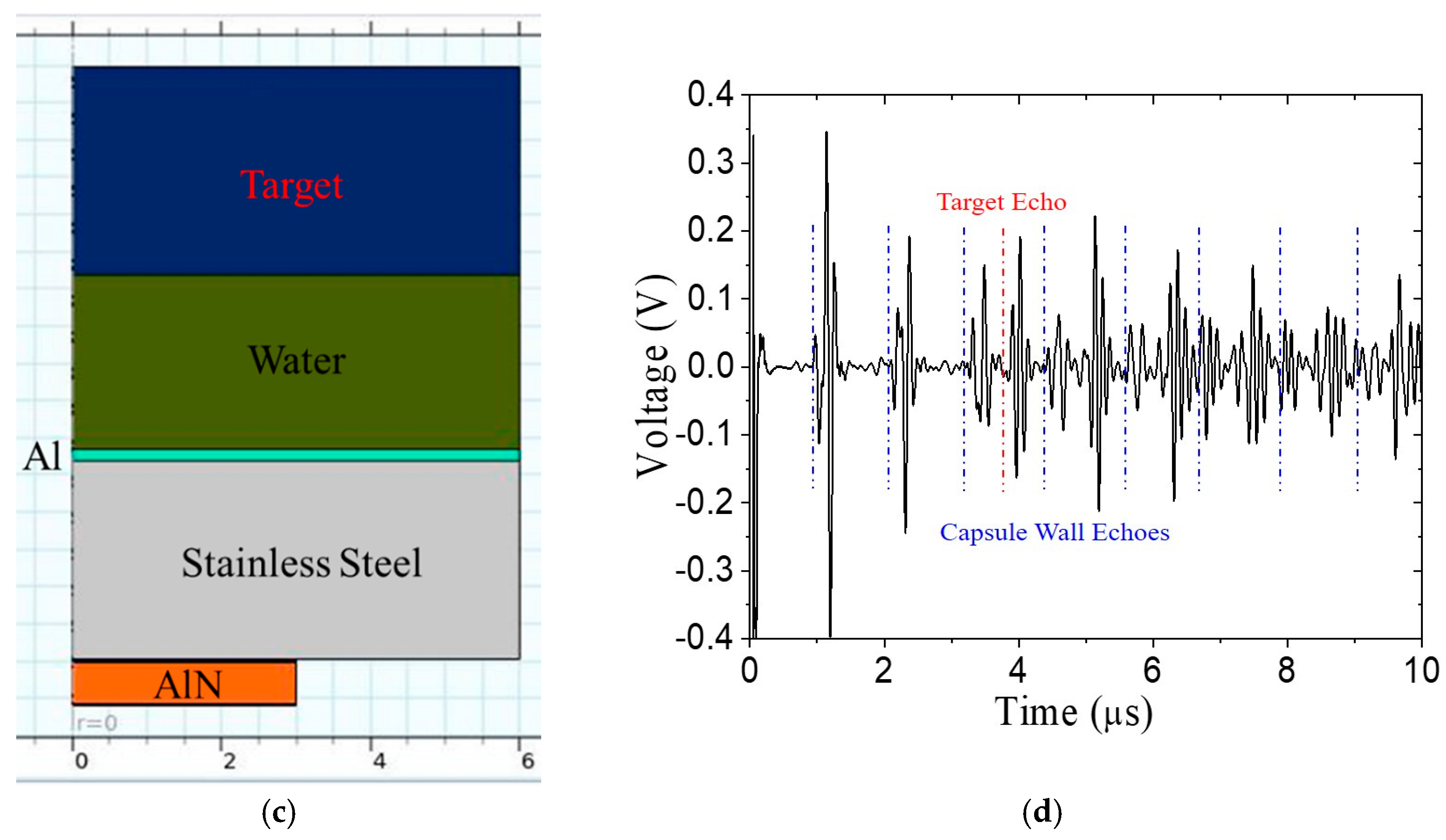
3.1. Immersion Test Model
3.2. Laboratory Testing
4. Discussion
- How effectively a viscous backing material on the piezoelectric wafer decreases transducer ringing;
- That the thickness of the free electrode on the piezoelectric wafer can eventually become large enough to change the dynamic response of the transducer, while the thickness of the sandwiched electrode mostly affects the time of arrival;
- A solid couplant can greatly influence the received signal—if it is too compliant, then ringing occurs, and if it employs thermal processes (such as brazing), then it can change the grain size of the vessel wall and affect attenuation;
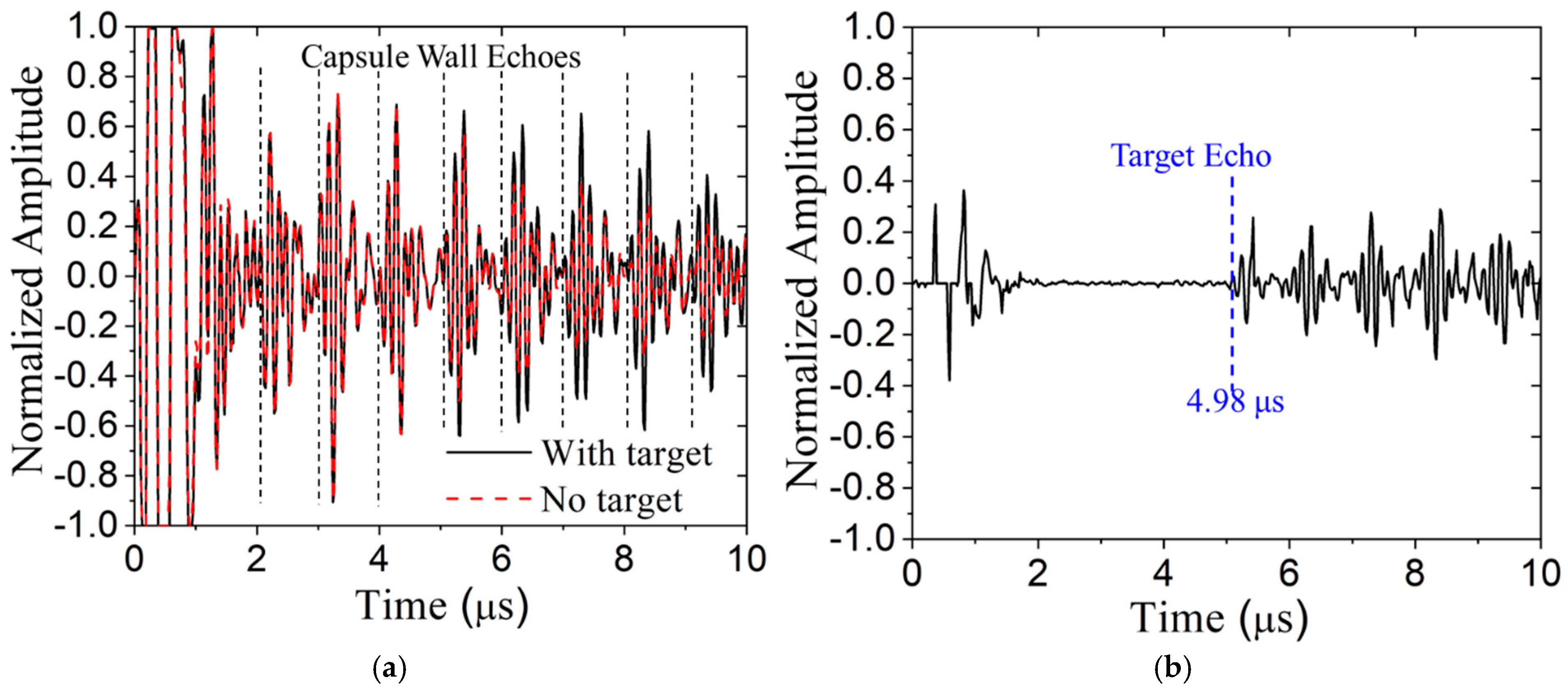
- That the target echo can be completely obscured by vessel wall reverberations, but an acoustic matching layer reduces reverberations enough that the target echo becomes visible, albeit the signal-to-noise ratio is low;
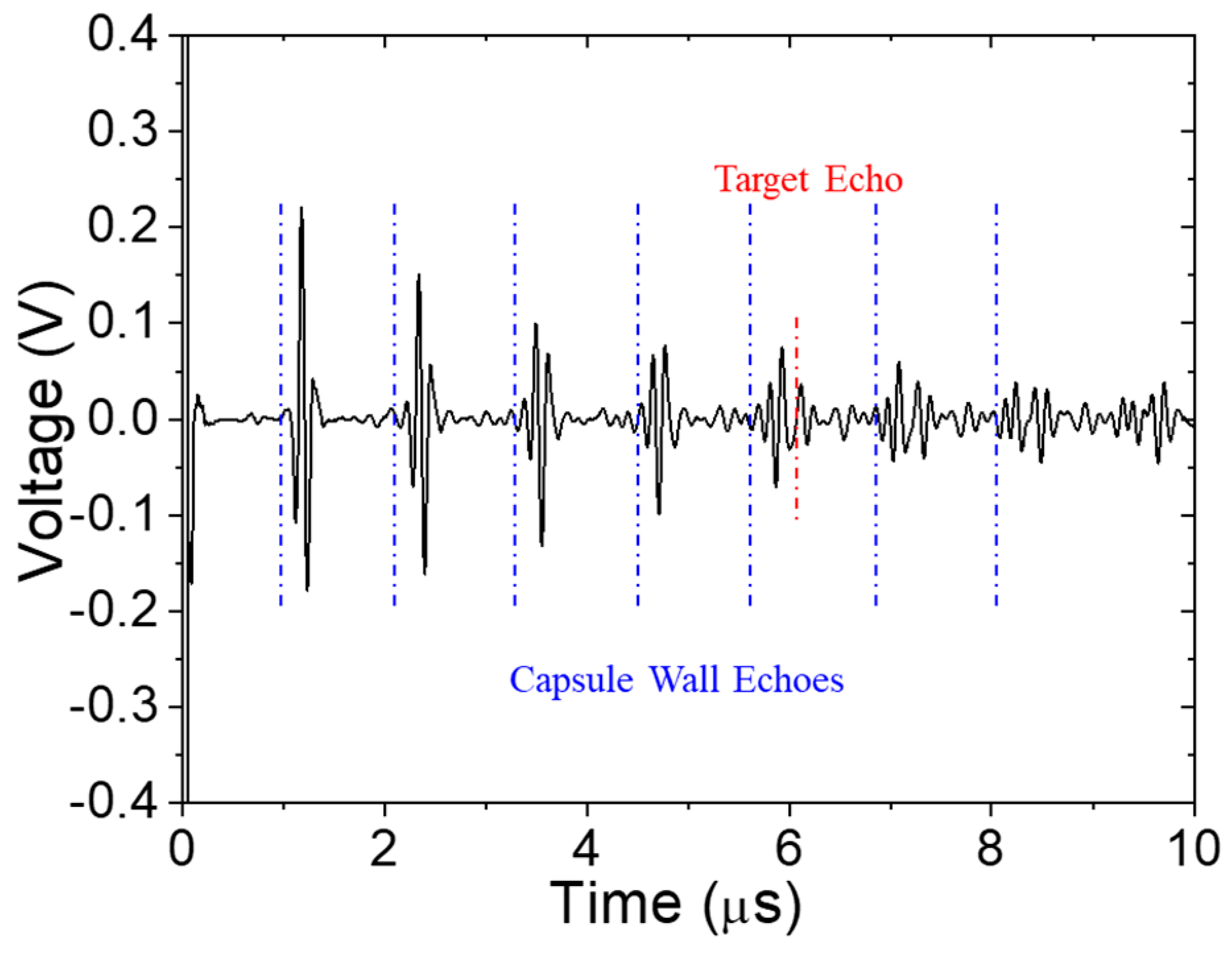
- A very simple subtractive signal processing approach can sufficiently reduce the vessel wall reverberations from the signal to provide a very reasonable signal-to-noise ratio for the target echo;
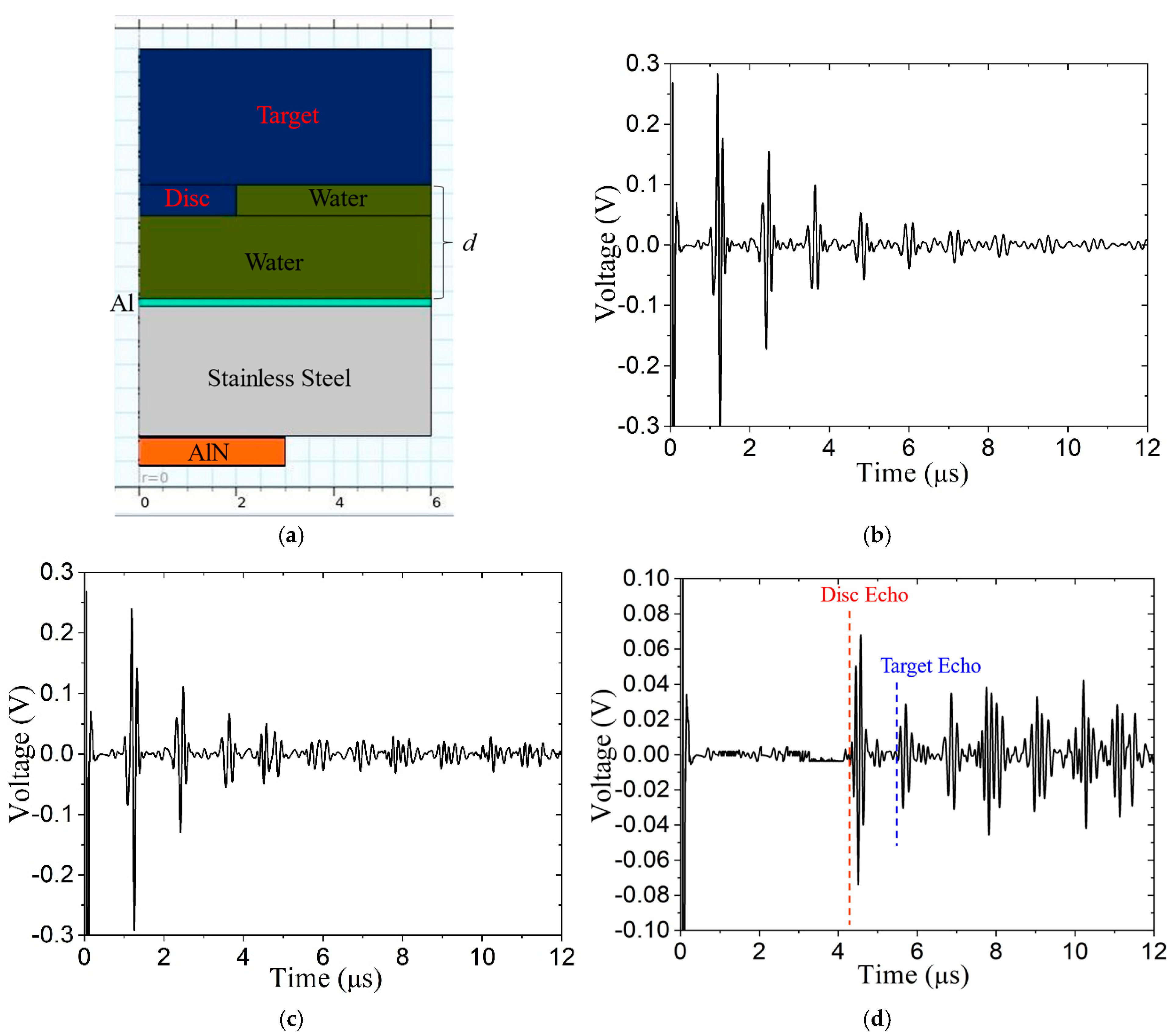
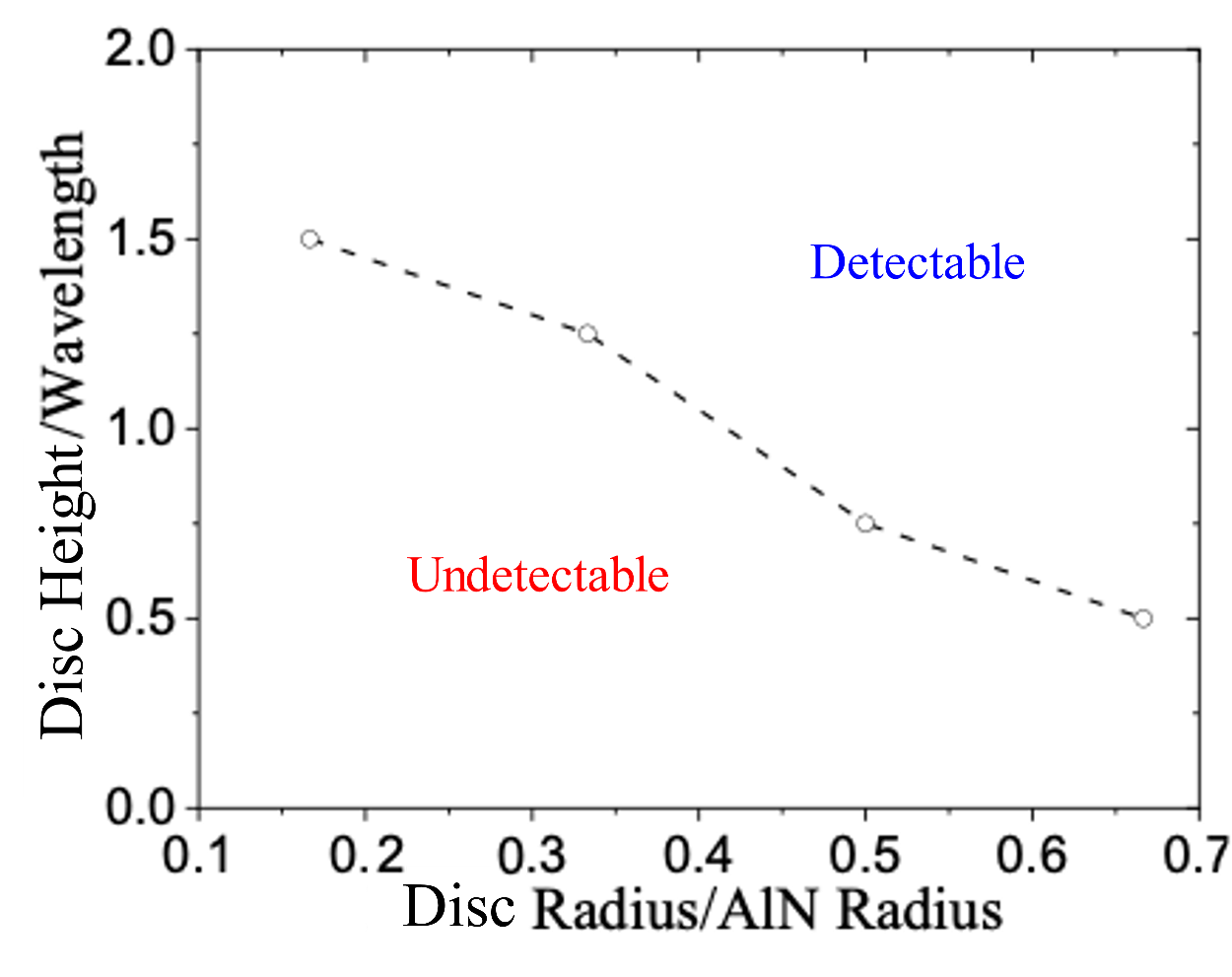
- When using the subtractive signal processing, a disc-like surface anomaly could be detected along with the target echo.
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Baba, A.; Searfass, C.T.; Tittmann, B.R. High Temperature Ultrasonic Transducer up to 1000 C Using Lithium Niobate Single Crystal. Appl. Phys. Lett. 2010, 97, 232901. [Google Scholar] [CrossRef]
- Reinhardt, B.; Daw, J.; Tittmann, B.R. Irradiation Testing of Piezoelectric (Aluminum Nitride, Zinc Oxide, and Bismuth Titanate) and Magnetostrictive Sensors (Remendur and Galfenol). IEEE Trans. Nucl. Sci. 2018, 65, 533–538. [Google Scholar] [CrossRef]
- Tittmann, B.R.; Batista, C.; Trivedi, Y.; Lissenden, C.; Reinhardt, B. State-of-the-Art and Practical Guide to Ultrasonic Transducers for Harsh Environments Including Temperatures above 2120 °F (1000 °C) and Neutron Flux above 1013 n/cm2. Sensors 2019, 19, 4755. [Google Scholar] [CrossRef] [PubMed]
- Kazys, R.; Vaskeliene, V. High Temperature Ultrasonic Transducers: A Review. Sensors 2021, 21, 3200. [Google Scholar] [CrossRef] [PubMed]
- Wang, Y.; Sha, G.; Harlow, C.; Yazbeck, M.; Khafizov, M. Impact of Nuclear Reactor Radiation on the Performance of AlN/Sapphire Surface Acoustic Wave Devices. Nucl. Instrum. Methods Phys. Res. Sect. B Beam Interact. Mater. At. 2020, 481, 35–41. [Google Scholar] [CrossRef]
- Griffin, J.W.; Peters, T.J.; Posakony, G.J.; Chien, H.-T.; Bond, L.J.; Denslow, K.M.; Sheen, S.-H.; Raptis, P. Under-Sodium Viewing: A Review of Ultrasonic Imaging Technology for Liquid Metal Fast Reactors; PNNL-18292; Pacific Northwest National Lab.: Richland, WA, USA, 2009; p. 1010482. [Google Scholar] [CrossRef]
- Korsah, K.; Ramuhalli, P.; Vlim, R.; Kisner, R.A.; Britton, C.L., Jr.; Wootan, D.W.; Anheier, N.C., Jr.; Diaz, A.A.; Hirt, E.H.; Chien, H.T.; et al. Assessment of Sensor Technologies for Advanced Reactors; ORNL/TM--2016/337; Oak Ridge National Laboratory: Oak Ridge, TN, USA, 2016; p. 1345781. [Google Scholar] [CrossRef]
- Kim, T.; Kim, J.; Dalmau, R.; Schlesser, R.; Preble, E.; Jiang, X. High-Temperature Electromechanical Characterization of AlN Single Crystals. IEEE Trans. Ultrason. Ferroelectr. Freq. Control 2015, 62, 1880–1887. [Google Scholar] [CrossRef] [PubMed]
- Giurgiutiu, V. Structural Health Monitoring with Piezoelectric Wafer Active Sensors; Academic Press: Cambridge, MA, USA, 2007. [Google Scholar]
- Trivedi, Y.; Tittmann, B.R.; Batista, C.; Xu, J.; Lissenden, C. Field Deployable Spray-on Ultrasonic Coatings for High-Temperature Applications. AIP Conf. Proc. 2019, 2102, 060008. [Google Scholar] [CrossRef]
- Moser, F.; Jacobs, L.J.; Qu, J. Modeling Elastic Wave Propagation in Waveguides with the Finite Element Method. NDT E Int. 1999, 32, 225–234. [Google Scholar] [CrossRef]
- Ren, B.; Lissenden, C.J. Modeling Guided Wave Excitation in Plates with Surface Mounted Piezoelectric Elements: Coupled Physics and Normal Mode Expansion. Smart Mater. Struct. 2018, 27, 045014. [Google Scholar] [CrossRef]
- Rafienezhad-Masouleh, M.; Honarvar, F. Investigation of the Performance of a Piezoelectric Ultrasonic Transducer by Finite Element Modeling. Russ. J. Nondestruct. Test. 2021, 57, 269–280. [Google Scholar] [CrossRef]
- Twiefel, J.; Glukhovkoy, A.; de Wall, S.; Wurz, M.C.; Sehlmeyer, M.; Hitzemann, M.; Zimmermann, S. Towards a Highly Sensitive Piezoelectric Nano-Mass Detection—A Model-Based Concept Study. Sensors 2021, 21, 2533. [Google Scholar] [CrossRef] [PubMed]
- Mustapha, S.; Ye, L. Bonding Piezoelectric Wafers for Application in Structural Health Monitoring-Adhesive Selection. Res. Nondestruct. Eval. 2015, 26, 23–42. [Google Scholar] [CrossRef]
- Weaver, R.L. Diffusivity of Ultrasound in Polycrystals. J. Mech. Phys. Solids 1990, 38, 55–86. [Google Scholar] [CrossRef]
- Rathod, V.T. A Review of Acoustic Impedance Matching Techniques for Piezoelectric Sensors and Transducers. Sensors 2020, 20, 4051. [Google Scholar] [CrossRef] [PubMed]
- Cawley, P. A Development Strategy for Structural Health Monitoring Applications. J. Nondestruct. Eval. Diagn. Progn. Eng. Syst. 2021, 4, 041012. [Google Scholar] [CrossRef]








| Module | Component | Material |
|---|---|---|
| Solid Mechanics | vessel wall, bonding layer, electrode, target | stainless steel, various, various, zircaloy |
| Piezoelectricity | transducer | aluminum nitride |
| Acoustics | fluid | water |
| Electrical | driving circuit | n.a. |
| Component | 3D Model | 2D Model |
|---|---|---|
| Transducer | 6 × 6 × 0.55 mm | Ø6 × 0.55 mm |
| Electrode | 6 × 6 × 0.025 mm | Ø6 × 0.025 mm |
| Braze | 6 × 6 × 0.025 mm | Ø6 × 0.025 mm |
| Vessel Wall | 8 × 8 × 2.66 mm | Ø12 × 2.66 mm |
| Component | Material Properties at 20 °C | Material Properties at 316 °C | ||||
|---|---|---|---|---|---|---|
| Transducer (AlN) | Ref [8] | Ref [8] | ||||
| Fluid (water) | ρ = 1000 kg/m3, cL = 1490 m/s | ρ = 750 kg/m3, cL = 1020 m/s | ||||
| Backing (Si-W) | ρ = 2500 kg/m3, E = 3.6 Gpa, α=46 dB/mm | n.a. | ||||
| ρ (kg/m3) | E (Gpa) | ν (-) | ρ (kg/m3) | E (Gpa) | ν (-) | |
| Electrode (Ni) | 8890 | 205 | 0.31 | 8700 | 189 | 0.31 |
| Braze (Bni-6) | 8200 | 160 | 0.31 | 8200 | 160 | 0.31 |
| Wall (stainless steel) | 8027 | 195 | 0.30 | 7889 | 172.4 | 0.30 |
| Stainless Steel | Water | Match | Titanium | Aluminum |
|---|---|---|---|---|
| 42.79 | 1.5 | 8.01 | 25.08 | 15.23 |
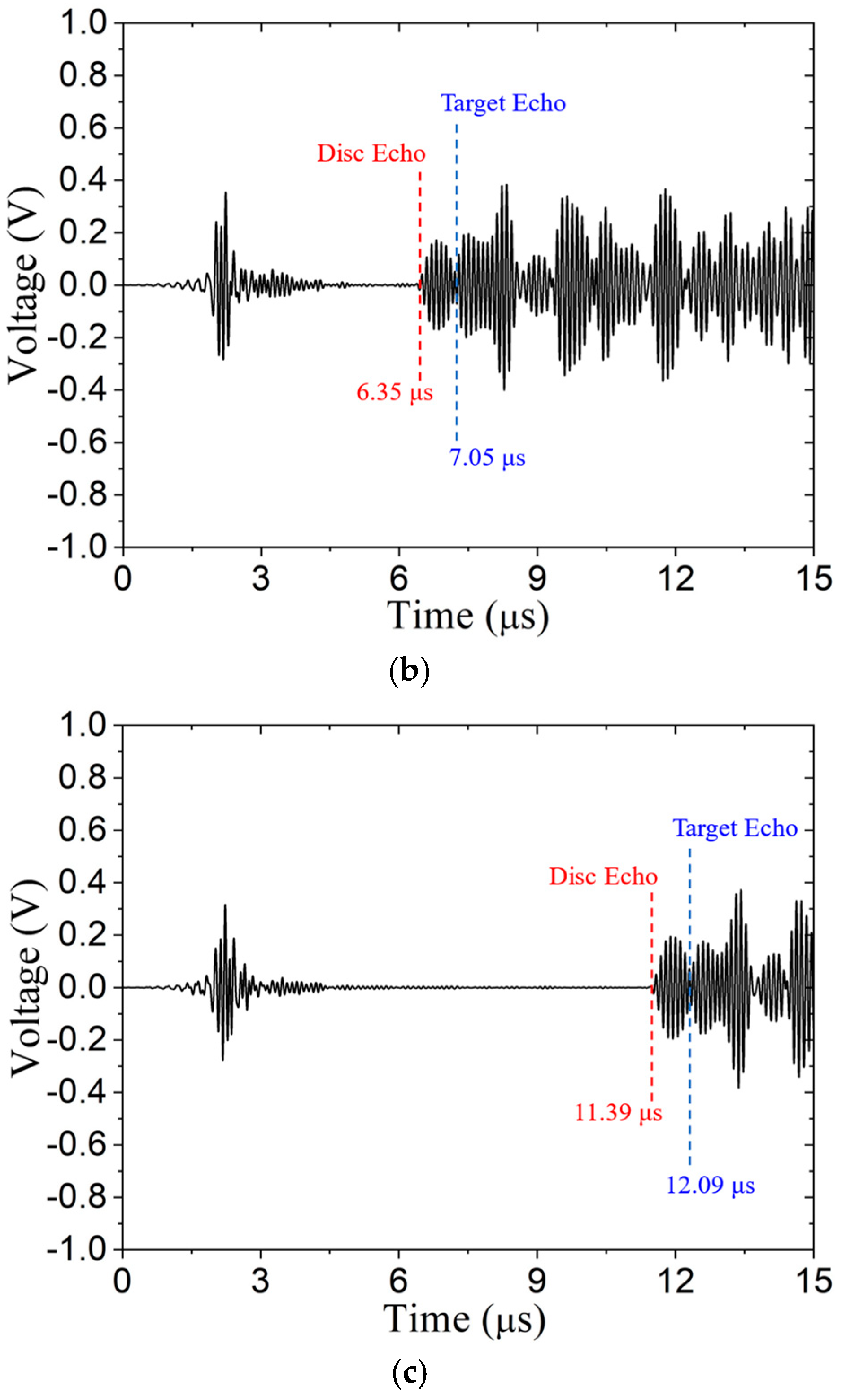
| Case | Water Path (mm) | Disc Echo TOA (μs) | Target Echo TOA (μs) | ||
|---|---|---|---|---|---|
| Theory | Experiment | Theory | Experiment | ||
| 1 | 2.33 | 3.08 | 3.12 | 3.95 | 3.79 |
| 2 | 2.96 | 4.30 | 4.35 | 5.00 | 5.05 |
| 3 | 6.68 | 9.21 | 9.39 | 9.90 | 10.09 |
| OmniScan | RAM-5000 |
|---|---|
| 9 | 20 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Sha, G.; Bozek, A.R.; Tittmann, B.R.; Lissenden, C.J. Unique Characteristics of Pulse-Echo Sensing Systems for Ultrasonic Immersion Testing in Harsh Environments. Sensors 2024, 24, 7748. https://doi.org/10.3390/s24237748
Sha G, Bozek AR, Tittmann BR, Lissenden CJ. Unique Characteristics of Pulse-Echo Sensing Systems for Ultrasonic Immersion Testing in Harsh Environments. Sensors. 2024; 24(23):7748. https://doi.org/10.3390/s24237748
Chicago/Turabian StyleSha, Gaofeng, Andrew R. Bozek, Bernhard R. Tittmann, and Cliff J. Lissenden. 2024. "Unique Characteristics of Pulse-Echo Sensing Systems for Ultrasonic Immersion Testing in Harsh Environments" Sensors 24, no. 23: 7748. https://doi.org/10.3390/s24237748
APA StyleSha, G., Bozek, A. R., Tittmann, B. R., & Lissenden, C. J. (2024). Unique Characteristics of Pulse-Echo Sensing Systems for Ultrasonic Immersion Testing in Harsh Environments. Sensors, 24(23), 7748. https://doi.org/10.3390/s24237748








