Influence of the Injection Bias on the Capacitive Sensing of the Test Mass Motion of Satellite Gravity Gradiometers
Abstract
:1. Introduction
2. Analysis of the Impact of the Carrier Wave on Capacitive Position Sensing
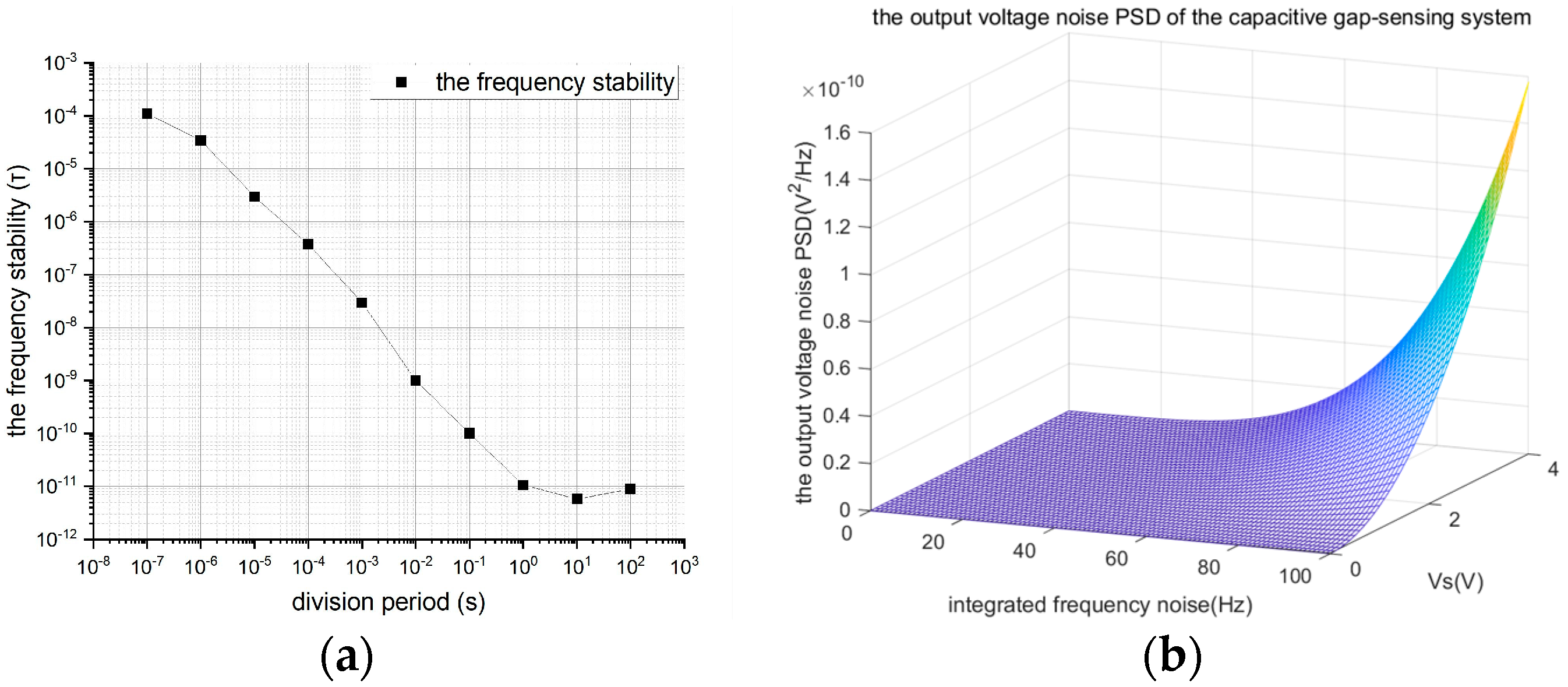
3. The Influence of Multiple Factors of the Injection Bias
3.1. Amplitude Noise
3.2. Frequency Noise and Phase Noise
3.3. Broadband Noise
3.4. Total Noise
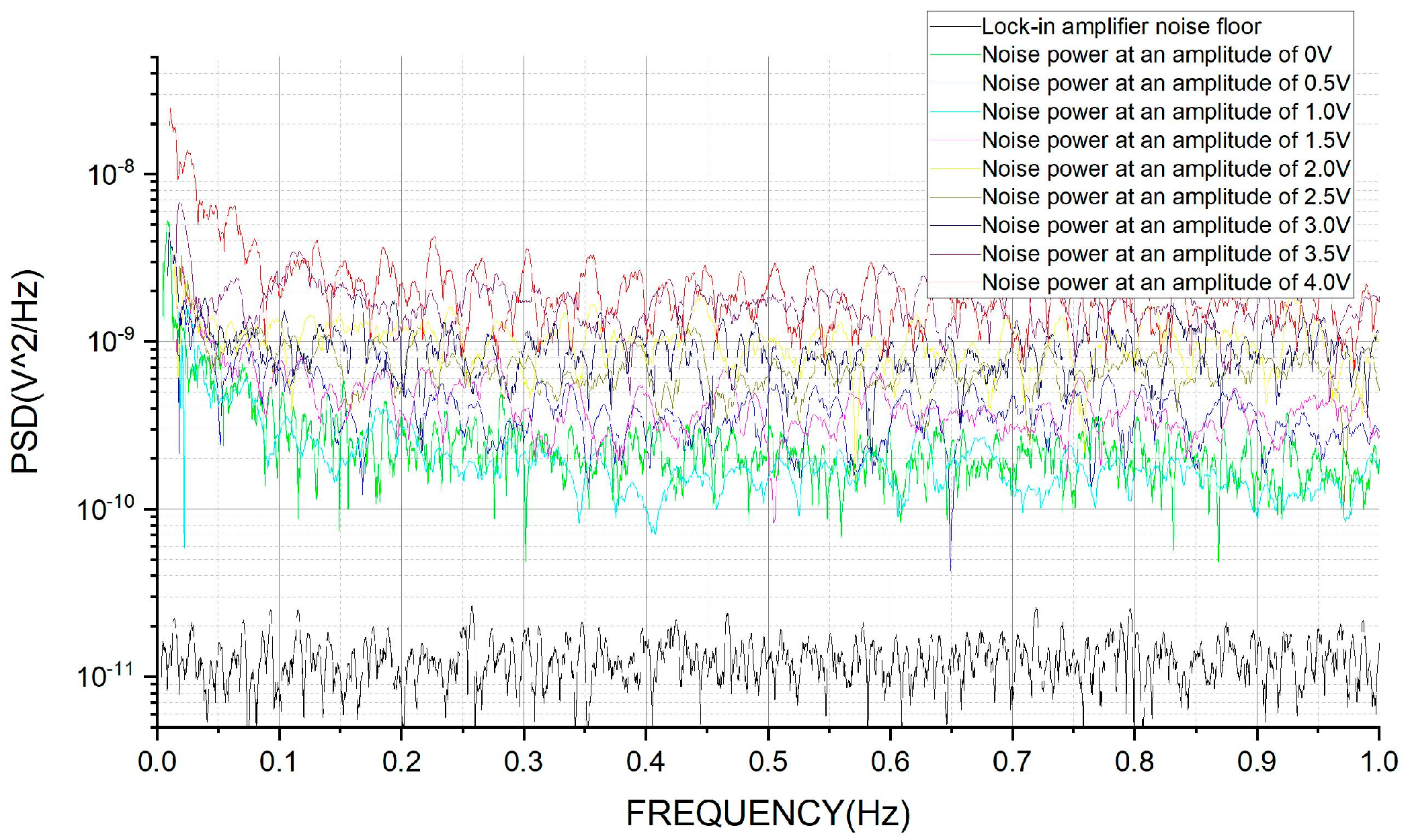
4. Experimental Results
4.1. Amplitude Noise
4.2. Frequency Noise and Phase Noise
4.3. Broadband Noise and Total Noise
4.4. Noise Analysis
5. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Drinkwater, M.; Kern, M. Calibration and Validation Plan for L1b Data Products; Technical Report EOP-SM/1363/MD-md; ESA ESTEC: Noordwijk, The Netherlands, 2006. [Google Scholar]
- Drinkwater, M.; Floberghagen, R.; Haagmans, R.; Muzi, D.; Popescu, A. GOCE: ESA’s First Earth Explorer Core Mission, Earth Gravity Field from Space-from Sensors to Earth Sciences; Beutler, G.B., Drinkwater, M., Rummel, R., von Steiger, R., Eds.; Space Sciences Series of ISSI; Kluwer Academic Publishers: Dordrecht, The Netherlands, 2003; Volume 18. [Google Scholar]
- Sünkel, H. Mathematical and Numerical Techniques in Physical Geodesy. In Lecture Notes in Earth Sciences; Springer: Berlin/Heidelberg, Germany, 1986; Volume 7. [Google Scholar]
- Rummel, R.; Yi, W.; Stummer, C. GOCE gravitational gradiometry. J. Geod. 2011, 85, 777–790. [Google Scholar] [CrossRef]
- Marque, J.; Christophe, B.; Liorzou, F.; Bodovillé, G.; Foulon, B.; Guérard, J.; Lebat, V. The ultra sensitive accelerometers of the ESA GOCE mission. In Proceedings of the 59th International Astronautical Congress (IAC-08-B1. 3.7), Glasgow, Scotland, 29 September–3 October 2008. [Google Scholar]
- Bergé, J.; Christophe, B.; Foulon, B. GOCE accelerometers data revisited: Stability and detector noise. In Proceedings of the ESA Living Planet Symposium, Edinburgh, UK, 9–13 September 2013. [Google Scholar]
- Johannessen, J.A.; Balmino, G.; Le Provost, C.; Rummel, R.; Sabadini, R.; Sünkel, H.; Tscherning, C.; Visser, P.; Woodworth, P.; Hughes, C.; et al. The European gravity field and steady-state ocean circulation explorer satellite mission its impact on geophysics. Surv. Geophys. 2003, 24, 339–386. [Google Scholar] [CrossRef]
- Josselin, V.; Touboul, P.; Kielbasa, R. Capacitive detection scheme for space accelerometers applications. Sens. Actuators A Phys. 1999, 78, 92–98. [Google Scholar] [CrossRef]
- Touboul, P.; Foulon, B.; Willemenot, E. Electrostatic space accelerometers for present and future missions. Acta Astronaut. 1999, 45, 605–617. [Google Scholar] [CrossRef]
- Weber, W.J.; Cavalleri, A.; Dolesi, R.; Fontana, G.; Hueller, M.; Vitale, S. Position sensors for LISA drag-free control. Class. Quantum Gravity 2002, 19, 1751. [Google Scholar] [CrossRef]
- Weber, W.J.; Bortoluzzi, D.; Cavalleri, A.; Carbone, L.; Da Lio, M.; Dolesi, R.; Fontana, G.; Hoyle, C.D.; Hueller, M.; Vitale, S. Position sensors for flight testing of LISA drag-free control. In Gravitational-Wave Detection; SPIE: Bellingham, WA, USA, 2003. [Google Scholar]
- Armano, M.; Audley, H.; Auger, G.; Baird, J.; Bassan, M.; Binetruy, P.; Born, M.; Bortoluzzi, D.; Brandt, N.; Caleno, M.; et al. Capacitive sensing of test mass motion with nanometer precision over millimeter-wide sensing gaps for space-borne gravitational reference sensors. Phys. Rev. D 2017, 96, 062004. [Google Scholar] [CrossRef]
- Dolesi, R.; Bortoluzzi, D.; Bosetti, P.; Carbone, L.; Cavalleri, A.; Cristofolini, I.; DaLio, M.; Fontana, G.; Fontanari, V.; Foulon, B.; et al. Gravitational sensor for LISA and its technology demonstration mission. Class. Quantum Gravity 2003, 20, S99. [Google Scholar] [CrossRef]
- Christophe, B.; Marque, J.; Foulon, B. In-orbit data verification of the accelerometers of the ESA GOCE mission. In Proceedings of the Annual Meeting of the French Society of Astronomy and Astrophysics, SF2A-2010, Marseille, France, 21–24 June 2010. [Google Scholar]
- Gan, L.; Mance, D.; Zweifel, P. Actuation to sensing crosstalk investigation in the inertial sensor front-end electronics of the laser interferometer space antenna pathfinder satellite. Sens. Actuators A Phys. 2011, 167, 574–580. [Google Scholar] [CrossRef]
- Li, K.; Bai, Y.; Hu, M.; Qu, S.; Wang, C.; Zhou, Z. Amplitude stability analysis and experimental investigation of an ac excitation signal for capacitive sensors. Sens. Actuators A Phys. 2020, 309, 112020. [Google Scholar] [CrossRef]
- Li, K.; Chen, D.; Li, D.; Wang, C.; Yang, X.; Hu, M.; Bai, Y.; Qu, S.; Zhou, Z. A novel modem model insensitive to the effect of the modulated carrier and the demodulated-signal phase adapted for capacitive sensors. Measurement 2023, 213, 112734. [Google Scholar] [CrossRef]
- Mance, D.; Zweifel, P.; Ferraioli, L.; ten Pierick, J.; Meshksar, N.; Giardini, D.; LISA Pathfinder Collaboration. GRS electronics for a space-borne gravitational wave observatory. In Journal of Physics: Conference Series; IOP Publishing: Bristol, UK, 2017; Volume 840, p. 012040. [Google Scholar]





| The Injection Bias Parameters | Unit | Typical Value |
|---|---|---|
| Amplitude value VS | V | 0~4 |
| Phase difference θ | rad | 0 |
| Angular frequency difference | rad | 0 |
| Frequency f0 | kHz | 100.0 |
| Angular frequency | rad | |
| Noise bandwidth (NBW) | Hz | 1 |
| Equivalent gain A | dB | 0 |
| Parameters | Unit | Typical Value |
|---|---|---|
| Sampling frequency | MHz | 1.024 |
| Anti-aliasing frequency | Hz | 2 |
| Window functions | - | Hanning |
| Amplitude | 1 V | 1.5 V | 2 V | 2.5 V | 3 V | 3.5 V | 4 V | ||
|---|---|---|---|---|---|---|---|---|---|
| Amplitude noise spectral density | 5 mHz | 0.04 | 0.14 | 0.24 | 0.37 | 0.43 | 0.34 | 0.49 | |
| 100 mHz | 0.01 | 0.04 | 0.03 | 0.02 | 0.15 | 0.14 | 0.14 | ||
| Amplitude noise factor | 5 mHz | ppm | 2.00 | 2.49 | 2.45 | 2.43 | 2.19 | 1.67 | 1.75 |
| 100 mHz | 1.33 | 0.87 | 0.57 | 1.29 | 1.07 | 0.94 | 1.00 | ||
| The Carrier Wave Parameters | Unit | Typical Value |
|---|---|---|
| Resolution bandwidth (RBW) | Hz | 1.8 |
| The conversion factor of the resolution bandwidth to noise bandwidth kn | - | 1.128 |
| Amplitude | V | 1 | 1.5 | 2 | 2.5 | 3 | 3.5 | 4 |
|---|---|---|---|---|---|---|---|---|
| The average PSD in the range of 5 mHz~1.005 Hz | 3.14 | 6.05 | 11.1 | 8.02 | 9.48 | 17.6 | 24.2 | |
| The average PSD in the range of 0.1 Hz~1.1 Hz | 1.77 | 4.01 | 4.84 | 6.74 | 8.87 | 16.6 | 18.8 | |
| Synthetic noise’s PSD by model@ 5 mHz | 1.91 | 2.13 | 3.47 | 4.37 | 7.84 | 14.68 | 18.63 | |
| Synthetic noise’s PSD by model@ 0.1 Hz | 1.89 | 2.09 | 3.38 | 4.23 | 7.73 | 14.60 | 18.49 | |
| The stabilized injection bias stability@ 5 mHz | 55.5 | 38.1 | 34.1 | 32.8 | 21.3 | 20.5 | 31.8 | |
| The stabilized injection bias stability@ 0.1 Hz | 14.4 | 16.5 | 17.4 | 10.5 | 7.5 | 12.5 | 10.8 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Xu, H.; Lei, J.; Li, D.; Li, Y.; Tao, W.; Zhang, W.; Chen, M. Influence of the Injection Bias on the Capacitive Sensing of the Test Mass Motion of Satellite Gravity Gradiometers. Sensors 2024, 24, 1188. https://doi.org/10.3390/s24041188
Xu H, Lei J, Li D, Li Y, Tao W, Zhang W, Chen M. Influence of the Injection Bias on the Capacitive Sensing of the Test Mass Motion of Satellite Gravity Gradiometers. Sensors. 2024; 24(4):1188. https://doi.org/10.3390/s24041188
Chicago/Turabian StyleXu, Hengtong, Jungang Lei, Detian Li, Yunpeng Li, Wenze Tao, Wenyan Zhang, and Meng Chen. 2024. "Influence of the Injection Bias on the Capacitive Sensing of the Test Mass Motion of Satellite Gravity Gradiometers" Sensors 24, no. 4: 1188. https://doi.org/10.3390/s24041188
APA StyleXu, H., Lei, J., Li, D., Li, Y., Tao, W., Zhang, W., & Chen, M. (2024). Influence of the Injection Bias on the Capacitive Sensing of the Test Mass Motion of Satellite Gravity Gradiometers. Sensors, 24(4), 1188. https://doi.org/10.3390/s24041188





