A Review: High-Precision Angle Measurement Technologies
Abstract
1. Introduction
2. High-Precision with Single Axis
2.1. Optical Methods
2.1.1. Non-Interference Methods
- (A)
- The location of the light spot
- (B)
- The energy of the light spot
- (C)
- The visual method
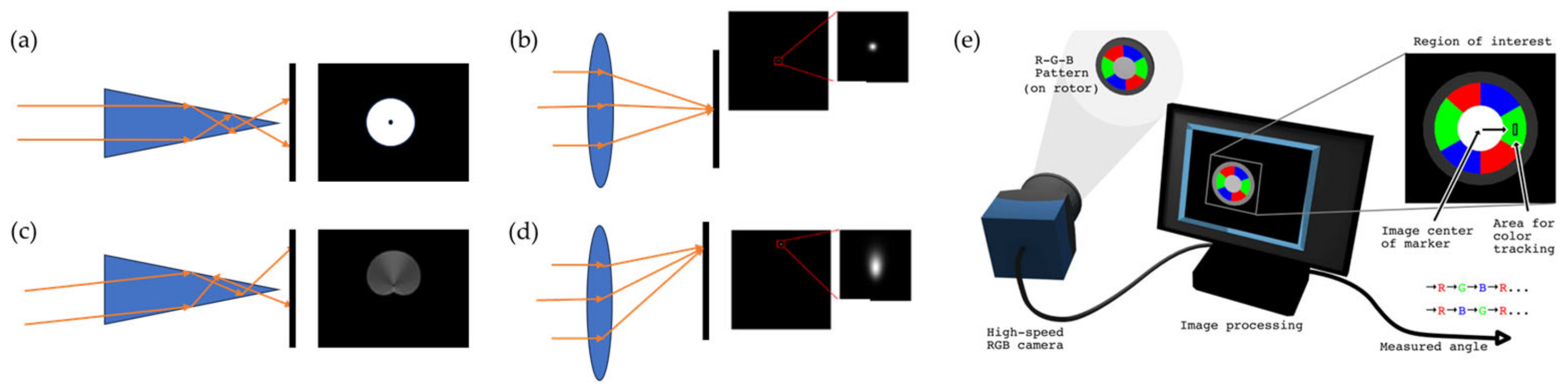
- (D)
- SHG method

- (E)
- Weak measurement
- (F)
- Quaternion
2.1.2. Interference Methods
- (A)
- Homodyne interference
- (B)
- Heterodyne interference
- (C)
- Optical Frequency Comb method
2.2. Mechanical Methods
2.3. Electromagnetic and Inertial Methods
- (A)
- Electromagnetic method: inductance, capacitance, and Hall effect
- (B)
- Inertial method
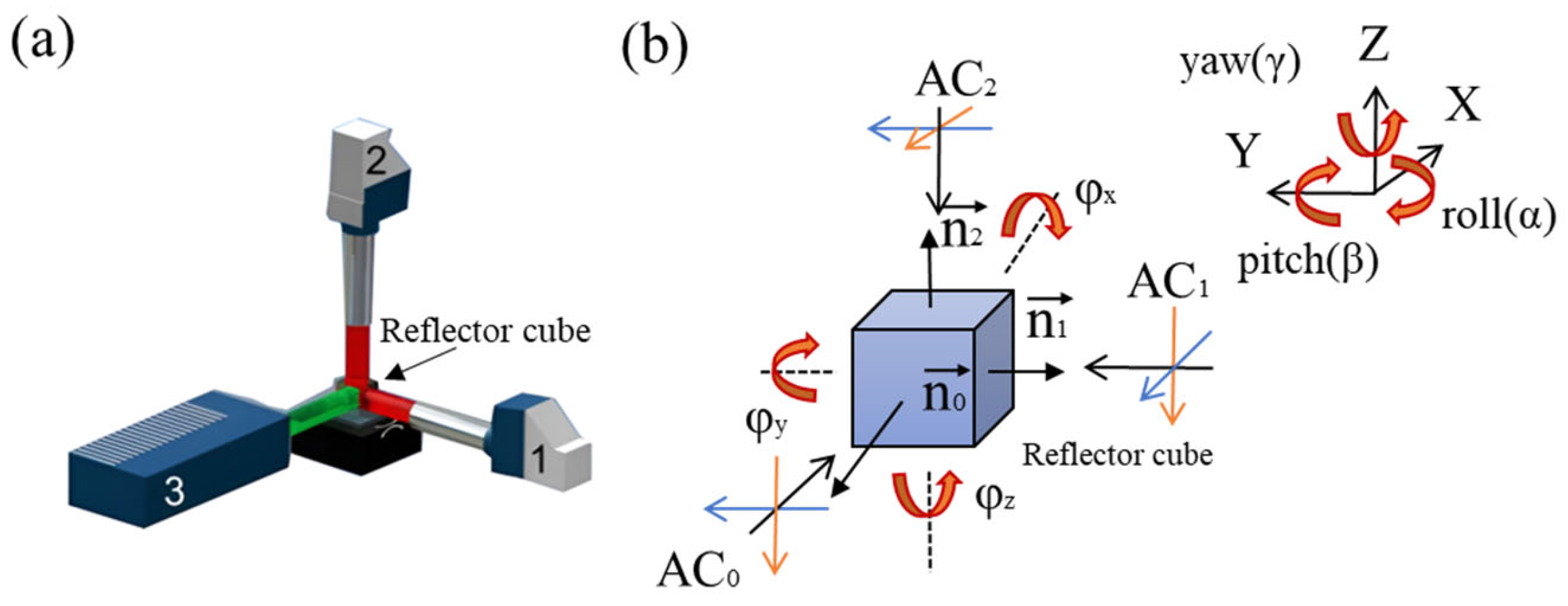
3. High Precision with Multiple Axes
3.1. Planar Mirror Target
3.2. Grating Target
3.3. Self-Designed Target
4. Conclusions and Prospects
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Kumar, A.S.A.; George, B.; Mukhopadhyay, S.C. Technologies and Applications of Angle Sensors: A Review. IEEE Sens. J. 2021, 21, 7195–7206. [Google Scholar] [CrossRef]
- Gao, W.; Kim, S.W.; Bosse, H.; Haitjema, H.; Chena, Y.L.; Lu, X.D.; Knapp, W.; Weckenmann, A.; Estler, W.T.; Kunzmann, H. Measurement technologies for precision positioning. CIRP Ann.–Manuf. Technol. 2015, 64, 773–796. [Google Scholar] [CrossRef]
- Zhang, C.; Liu, W.; Duan, F.; Fu, X.; Wang, X. Accuracy improvement method and optimal design of straightness in five-degree-of-freedom measurement of long guide. Opt. Precis. Eng. 2022, 30, 2467–2478. [Google Scholar] [CrossRef]
- Masuda, T.; Watanabe, T.; Beeks, K.; Fujimoto, H.; Hiraki, T.; Kaino, H.; Kitao, S.; Miyamoto, Y.; Okai, K.; Sasao, N.; et al. Absolute X-ray energy measurement using a high-accuracy angle encoder. J. Synchrotron Radiat. 2021, 28, 111–119. [Google Scholar] [CrossRef] [PubMed]
- Quan, L.; Shimizu, Y.; Xiong, X.; Matsukuma, H.; Gao, W. A new method for evaluation of the pitch deviation of a linear scale grating by an optical angle sensor. Precis. Eng. J. Int. Soc. Precis. Eng. Nanotechnol. 2021, 67, 1–13. [Google Scholar] [CrossRef]
- Chiu, M.-H.; Su, D.-C. Angle measurement using total-internal-reflection heterodyne interferometry. Opt. Eng. 1997, 36, 1750–1753. [Google Scholar] [CrossRef]
- Joo, K.-N.; Kim, S.-W. Absolute distance measurement by dispersive interferometry using a femtosecond pulse laser. Opt. Express 2006, 14, 5954–5960. [Google Scholar] [CrossRef] [PubMed]
- Wu, H.; Zhang, F.; Liu, T.; Li, J.; Qu, X. Absolute distance measurement with correction of air refractive index by using two-color dispersive interferometry. Opt. Express 2016, 24, 24361–24376. [Google Scholar] [CrossRef]
- Liang, X.; Lin, J.; Yang, L.; Wu, T.; Liu, Y.; Zhu, J. Simultaneous Measurement of Absolute Distance and Angle Based on Dispersive Interferometry. IEEE Photonics Technol. Lett. 2020, 32, 449–452. [Google Scholar] [CrossRef]
- Straube, G.; Fischer Calderón, J.S.; Ortlepp, I.; Füßl, R.; Manske, E. A Heterodyne Interferometer with Separated Beam Paths for High-Precision Displacement and Angular Measurements. Nanomanuf. Metrol. 2021, 4, 200–207. [Google Scholar] [CrossRef]
- Udem, T.; Holzwarth, R.; Hänsch, T.W. Optical frequency metrology. Nature 2002, 416, 233–237. [Google Scholar] [CrossRef] [PubMed]
- Zhou, S.; Shen, X.; Wu, C.; Li, D. The Error Analysis and Experimental Research of Micro-angle Measurement System Based on F-P Etalon. In Proceedings of the 10th International Symposium on Precision Mechanical Measurements, Qingdao, China, 15–17 October 2021. [Google Scholar] [CrossRef]
- Zelin, S. Calibration method for measuring angle device with CCD. In Proceedings of the 2021 International Conference of Optical Imaging and Measurement (ICOIM), Xi’an, China, 27–29 August 2021. [Google Scholar]
- Ren, Y.; Zhang, F.; Liu, F.; Hu, X.; Fu, Y. Accuracy test method of multi-instrument cooperative measurement. Proc. SPIE 2022, 12282, 1228219. [Google Scholar] [CrossRef]
- Wang, S.T.; Liao, B.Q.; Shi, N.N.; Li, X.H. A Compact and High-Precision Three-Degree-of-Freedom Grating Encoder Based on a Quadrangular Frustum Pyramid Prism. Sensors 2023, 23, 4022. [Google Scholar] [CrossRef]
- Kangning, Y.; Junhao, Z.; Weihan, Y.; Qian, Z.; Gaopeng, X.; Guanhao, W.; Xiaohao, W.; Xinghui, L. Two-channel six degrees of freedom grating-encoder for precision-positioning of sub-components in synthetic-aperture optics. Opt. Express 2021, 29, 21113–21128. [Google Scholar]
- Li, X.H.; Gao, W.; Muto, H.S.; Shimizu, Y.; Ito, S.; Dian, S. A six-degree-of-freedom surface encoder for precision positioning of a planar motion stage. Precis. Eng. J. Int. Soc. Precis. Eng. Nanotechnol. 2013, 37, 771–781. [Google Scholar] [CrossRef]
- Zhu, J.; Wang, G.; Wang, S.; Li, X. A Reflective-Type Heterodyne Grating Interferometer for Three-Degree-of-Freedom Subnanometer Measurement. IEEE Trans. Instrum. Meas. 2022, 71, 7007509. [Google Scholar] [CrossRef]
- Li, X.H.; Shimizu, Y.; Ito, T.; Cai, Y.D.; Ito, S.; Gao, W. Measurement of six-degree-of-freedom planar motions by using a multiprobe surface encoder. Opt. Eng. 2014, 53, 122405. [Google Scholar] [CrossRef]
- Li, X.H.; Shi, Y.P.; Xiao, X.; Zhou, Q.; Wu, G.H.; Lu, H.O.; Ni, K. Design and Testing of a Compact Optical Prism Module for Multi-Degree-of-Freedom Grating Interferometry Application. Appl. Sci. 2018, 8, 2495. [Google Scholar] [CrossRef]
- Wang, G.; Gao, L.; Huang, G.; Lei, X.; Cui, C.; Wang, S.; Yang, M.; Zhu, J.; Yan, S.; Li, X. A wavelength-stabilized and quasi-common-path heterodyne grating interferometer with sub-nanometer precision. IEEE Trans. Instrum. Meas. 2024, 21, 1–10. [Google Scholar] [CrossRef]
- Kim, J.-A.; Lee, J.Y.; Kang, C.-S.; Woo, J.H. Hybrid optical measurement system for evaluation of precision two-dimensional planar stages. Measurement 2022, 194, 111023. [Google Scholar] [CrossRef]
- Shimizu, Y.; Chen, L.-C.; Kim, D.W.; Chen, X.; Li, X.; Matsukuma, H. An insight into optical metrology in manufacturing. Meas. Sci. Technol. 2020, 32, 042003. [Google Scholar] [CrossRef]
- Tamir, B.; Cohen, E. Introduction to Weak Measurements and Weak Values. Quanta 2013, 2, 7–17. [Google Scholar] [CrossRef]
- Mi, M.; Malakhov, M. Measuring Tilt Angle of Gear Teeth with an Optical Dividing Head. Meas. Tech. 1970, 1, 130. [Google Scholar]
- Bručas, D.; Giniotis, V.; Augustinavičius, G.; Stepanovienė, J. Calibration of the multiangular prism (polygon). Mechanics 2010, 84, 62–66. [Google Scholar]
- Lu, X.D.; Graetz, R.; Amin-Shahidi, D.; Smeds, K. On-axis self-calibration of angle encoders. CIRP Ann. 2010, 59, 529–534. [Google Scholar] [CrossRef]
- Liu, X.; Yu, Z.; Peng, K.; Zheng, F.; Pu, H. Absolute Time-Grating Angular Displacement Sensor Based on Alternating Electric Field. The United Kingdom of Great Britain and Northern Ireland Patent GB2579311, 29 June 2022. [Google Scholar]
- Liu, X.; Yu, Z.; Peng, K.; Zheng, F.; Pu, H. Alternating Electric Field-Based Absolute Time-Grating Angular Displacement Sensor. Europe Patent EP3667229, 6 October 2021. [Google Scholar]
- Liu, X.K.; Huang, R.; Yu, Z.C.; Peng, K.; Pu, H.J. A High-Accuracy Capacitive Absolute Time-Grating Linear Displacement Sensor Based on a Multi-Stage Composite Method. IEEE Sens. J. 2021, 21, 8969–8978. [Google Scholar] [CrossRef]
- Yu, Z.; Peng, K.; Liu, X.; Chen, Z.; Huang, Y. A High-Precision Absolute Angular-Displacement Capacitive Sensor Using Three-Stage Time-Grating in Conjunction With a Remodulation Scheme. IEEE Trans. Ind. Electron. 2019, 66, 7376–7385. [Google Scholar] [CrossRef]
- Kokuyama, W.; Watanabe, T.; Nozato, H.; Ota, A. Measurement of Angle Error of Gyroscopes Using a Rotary Table Enhanced by Self-calibratable Rotary Encoder. In Proceedings of the 2015 IEEE International Symposium on Inertial Sensors and Systems (ISISS) Proceedings, Hapuna Beach, HI, USA, 23–26 March 2015. [Google Scholar] [CrossRef]
- Benammar, M.; Ben-Brahim, L.; Alhamadi, M.A. A Novel Resolver-to-360/Spl Deg/ Linearized Converter. IEEE Sens. J. 2004, 4, 96–101. [Google Scholar] [CrossRef]
- Eggenstein, F.; Schwarz, L.; Zeschke, T.; Viefhaus, J. New UHV angle encoder for high resolution monochromators, a modern spare part for the Heidenhain UHV RON 905. Nucl. Instrum. Methods Phys. Res. Sect. A Accel. Spectrometers Detect. Assoc. Equip. 2021, 1014, 165645. [Google Scholar] [CrossRef]
- Sun, S.Z.; Han, Y.; Zhang, H.; He, Z.Y.; Tang, Q.F. A Novel Inductive Angular Displacement Sensor With Multi-Probe Symmetrical Structure. IEEE Sens. J. 2022, 22, 3087–3096. [Google Scholar] [CrossRef]
- Guo, Q.; Wang, X.; Xu, C. Design of high precision angle measuring instrument based on Kalman filter. J. Phys. Conf. Ser. 2022, 2183, 012004. [Google Scholar] [CrossRef]
- Li, G.; Xue, Z.; Huang, Y.; Zhu, W.; Zou, W. Indication error analysis and compensation of circular grating angle measurement system. Chin. J. Sci. Instrum. 2021, 42, 59–65. [Google Scholar]
- Xia, Y.; Wu, Z.; Huang, M.; Liu, X.; Mi, L.; Tang, Q. An Improved Angle Calibration Method of a High-Precision Angle Comparator. Metrol. Meas. Syst. 2021, 28, 181–190. [Google Scholar] [CrossRef]
- Lu, Y.; Zhu, W.; Huang, Y.; Xue, Z. Development of Dynamic Measuring Circuit of Circular Grating Angle Based on FPGA. Instrum. Technol. Sens. 2021, 3, 35–39. [Google Scholar]
- Qu, P.; Yan, W.; Sun, S.; Zhu, T. Design of grating data acquisition system for airborne displacement and angle measurement. IET Conf. Proc. 2022, 2022, 187–191. [Google Scholar] [CrossRef]
- Li, Z.; Li, J.; Han, B.; Tang, Y.; Yanf, Y.; Ma, Y. Compensation Method of Circular Grating Angle Measurement Error. Instrum. Technol. Sens. 2021, 89, 56–59. [Google Scholar]
- Hsieh, T.-H.; Watanabe, T.; Hsu, P.-E. Calibration of Rotary Encoders Using a Shift-Angle Method. Appl. Sci. 2022, 12, 5008. [Google Scholar] [CrossRef]
- Huang, Y.; Che, S.; Zheng, G.; Zhu, W.; Zhou, Y.; Xue, Z. An Adaptive Filter for Subdivision of Circular Grating Signal of Angle Measurement. IEEE Trans. Instrum. Meas. 2022, 71, 3192068. [Google Scholar] [CrossRef]
- Zhao, G.; Ye, G.; Wu, Z.; Xing, H.; Liu, S.; Fan, X.; Li, Y.; Wei, P.; Liu, H. On-line angle self-correction strategy based on a cobweb-structured grating scale. Meas. Sci. Technol. 2021, 32, 055001. [Google Scholar] [CrossRef]
- Janson, N.; Abt, M.; Kuester, B.; Overmeyer, L. Optimization of optical rotation angle measurement by improved encodings. Tech. Mess. 2023, 90, 355–364. [Google Scholar] [CrossRef]
- Minghao, G.; Lu, W.; Fenghao, Z. Research on High Precision Angle Measurement and Compensation Technology Based on Circular Grating. J. Phys. Conf. Ser. 2021, 1838, 012075. [Google Scholar] [CrossRef]
- Das, S.; Chakraborty, B. Design and Realization of an Optical Rotary Sensor. IEEE Sens. J. 2018, 18, 2675–2681. [Google Scholar] [CrossRef]
- Ahmadi Tameh, T.; Sawan, M.; Kashyap, R. Novel Analog Ratio-Metric Optical Rotary Encoder for Avionic Applications. IEEE Sens. J. 2016, 16, 6586–6595. [Google Scholar] [CrossRef]
- Liu, B.; Wang, Y.; Liu, J.; Chen, A. Self-calibration Method of Circular Grating Error in Rotary Inertial Navigation. In Proceedings of the 2023 2nd International Symposium on Sensor Technology and Control (ISSTC), Hangzhou, China, 11–13 August 2023; pp. 139–143. [Google Scholar] [CrossRef]
- Du, Y.; Yuan, F.; Jiang, Z.; Li, K.; Yang, S.; Zhang, Q.; Zhang, Y.; Zhao, H.; Li, Z.; Wang, S. Strategy to Decrease the Angle Measurement Error Introduced by the Use of Circular Grating in Dynamic Torque Calibration. Sensors 2021, 21, 7599. [Google Scholar] [CrossRef] [PubMed]
- Chen, Z.; Liu, X.; Peng, D.; Zheng, Y.; Chen, X.; Zheng, F. Dynamic model of NC rotary table in angle measurements with time series. Trans. Inst. Meas. Control 2012, 35, 181–187. [Google Scholar] [CrossRef]
- Zhou, S.; Le, V.; Mi, Q.; Wu, G. Grating-Corner-Cube-Based Roll Angle Sensor. Sensors 2020, 20, 5524. [Google Scholar] [CrossRef] [PubMed]
- Zhou, S.; Le, V.; Xiong, S.; Yang, Y.; Ni, K.; Zhou, Q.; Wu, G. Dual-comb spectroscopy resolved three-degree-of-freedom sensing. Photonics Res. 2021, 9, 243–251. [Google Scholar] [CrossRef]
- Du, X.; Chen, Q. Dual-Laser Goniometer: A Flexible Optical Angular Sensor for Joint Angle Measurement. IEEE Trans. Ind. Electron. 2021, 68, 6328–6338. [Google Scholar] [CrossRef]
- Zhao, Y.; Zha, C.; Liu, B.; Han, F. High-precision rotation angle measurement method based on polarization self-mixing interference. Appl. Opt. 2023, 62, 7248–7253. [Google Scholar] [CrossRef]
- Zhao, Y.; Zhang, H. Angle measurement method based on speckle affected laser self-mixing interference signal. Opt. Commun. 2021, 482, 126569. [Google Scholar] [CrossRef]
- Zhao, Y.; Xiang, R.; Chen, J.; Huang, Z.; Wang, X.; Ma, Y.; Yu, B.; Lu, L. Absolute angle-measurement with a dihedral mirror of the multi-longitudinal mode laser self-mixing sensor. Precis. Eng. J. Int. Soc. Precis. Eng. Nanotechnol. 2021, 68, 256–261. [Google Scholar] [CrossRef]
- Yu, H.; Jia, X.; Wan, Q.; Liang, L.; Zhao, C. High-Resolution Angular Displacement Technology Based on Varying Moiré Figure Phase Positions. IEEE Sens. J. 2019, 19, 2126–2132. [Google Scholar] [CrossRef]
- Chen, G. Improving the angle measurement accuracy of circular grating. Rev. Sci. Instrum. 2020, 91, 065108. [Google Scholar] [CrossRef] [PubMed]
- Lee, C.H.; Huang, H.J.; Chang, J.P.; Chen, Y.C. Incremental Optical Encoder Based on a Sinusoidal Transmissive Pattern. IEEE Photonics J. 2022, 14, 6803206. [Google Scholar] [CrossRef]
- Kato, Y.; Mizutani, M. Photoelectric rotary encoder. U.S. Patent 11,506,518, 22 November 2022. [Google Scholar]
- Dong, L.; Ruan, Y.; Wang, J.; Wang, B.; Li, H.; Guo, P.; Wu, Y.; Jiang, S.; Zhang, S.; Wei, P. Progress in High Accurate Angle Measurement Technology of Long-Distance Target Based on Computational Interferometry. Laser Optoelectron. Prog. 2021, 58, 1811016. [Google Scholar] [CrossRef]
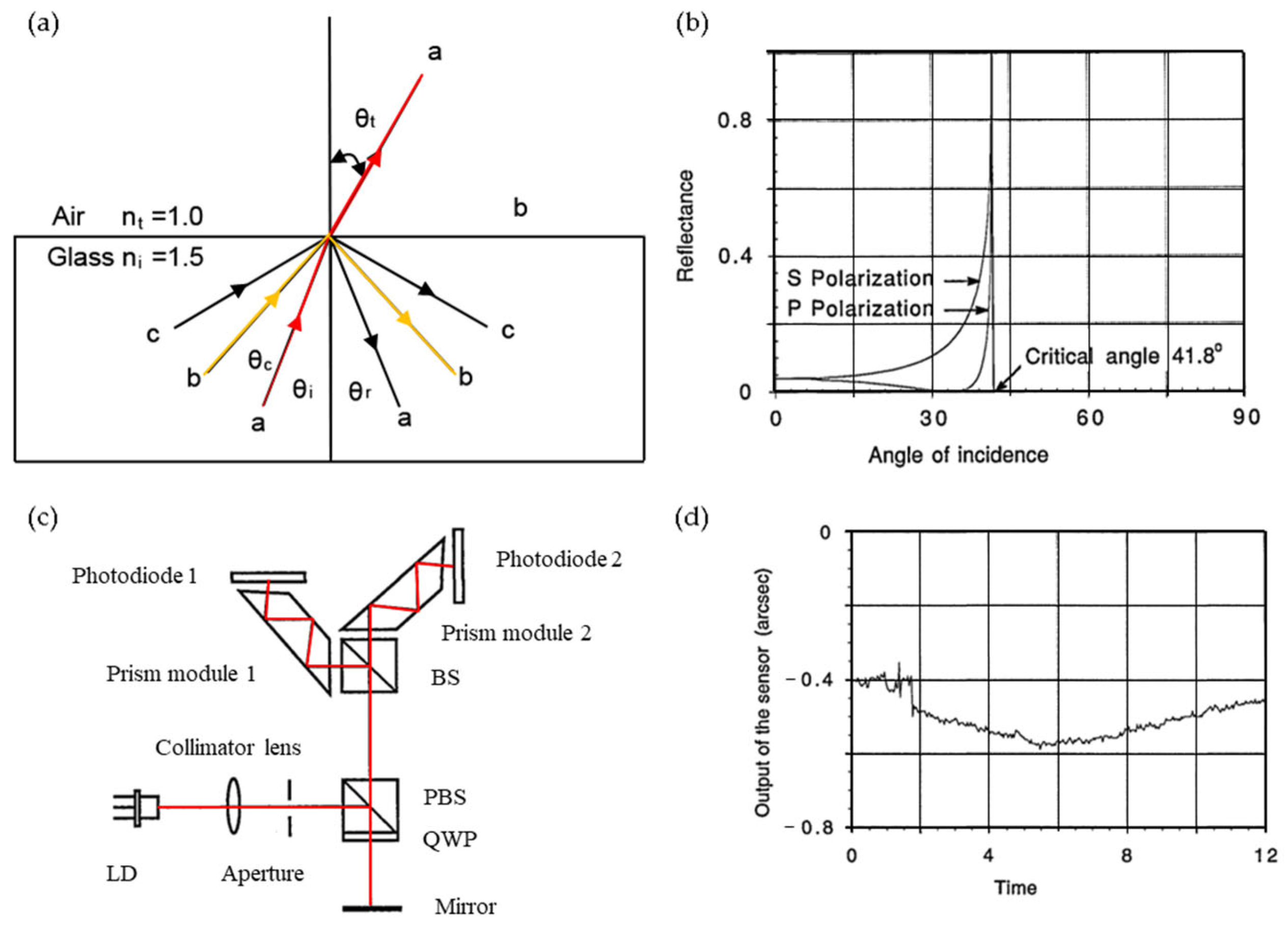
- Huang, P.S.; Kiyono, S.; Kamada, O. Angle measurement based on the internal-reflection effect: A new method. Appl. Opt. 1992, 31, 006047. [Google Scholar] [CrossRef]
- Zheng, F.; Long, F.; Zhao, Y.; Yu, C.; Jia, P.; Zhang, B.; Yuan, D.; Feng, Q. High-Precision Small-Angle Measurement of Laser-Fiber Autocollimation Using Common-Path Polarized Light Difference. IEEE Sens. J. 2023, 23, 9237–9245. [Google Scholar] [CrossRef]
- Mandal, L.; Singh, J.; Ganesan, A.R. Michelson Interferometer with mirrored right-angled prism for measurement of tilt with double sensitivity. J. Opt. 2023. [Google Scholar] [CrossRef]
- Kong, Q.; Wen, L. Fast tilt measurement based on optical block. Opt. Laser Technol. 2022, 148, 107731. [Google Scholar] [CrossRef]
- Pisani, M.; Astrua, M. Angle amplification for nanoradian measurements. Appl. Opt. 2006, 45, 1725–1729. [Google Scholar] [CrossRef]
- Liu, X.; Wang, Y.; Zhu, L.; Wang, B.; Yan, P. High-precision dynamic measurement of roll angle based on digital holography. Opt. Lasers Eng. 2023, 169, 107711. [Google Scholar] [CrossRef]
- Pang, J.; Tan, J.; Niu, Y.; Liu, Y.; Pan, W. Angular displacement measurement method based on least square fitting wave vector estimation. Opt. Technol. 2022, 48, 589–596. [Google Scholar]
- Chen, H.; Chen, Q.; Chen, H.; Yang, X.; Wang, X. Measurement of displacement and top beam attitude angle of advanced hydraulic support based on visual detection. Measurement 2023, 219, 113264. [Google Scholar] [CrossRef]
- Liu, H.; Shen, Y.; Liang, L.; Chen, P.; Yu, K.; Wang, K. Angle measurement method based on axicon lens. In Proceedings of the Conference on AOPC—Infrared Device and Infrared Technology, Beijing, China, 20–22 June 2021. [Google Scholar] [CrossRef]
- Mo, L.; Ren, J.; Ren, M. Microlens Array-Based Spatial Angle Encoding for High-Precision Visual Pose Measurement. Acta Opt. Sin. 2022, 42, 1612002. [Google Scholar] [CrossRef]
- Liu, H.; Zhao, S.; Liu, J.; Chen, P.; Zhang, L.; Wang, K. Non-imaging method for angle measurement based on an axicon. Appl. Opt. 2023, 62, 5077–5084. [Google Scholar] [CrossRef] [PubMed]
- Grubert, N.; Koenig, G.; Stollenwerk, J.; Loosen, P. Ring-Shaped Conoscopic Holography for Distance and Tilt Measurement. In Proceedings of the Conference on Lasers and Electro-Optics (CLEO), Electr Network, San Jose, CA, USA, 9–14 May 2021. [Google Scholar] [CrossRef]
- Kim, H.; Yamakawa, Y.; Senoo, T.; Ishikawa, M. Visual encoder: Robust and precise measurement method of rotation angle via high-speed RGB vision. Opt. Express 2016, 24, 13375–13386. [Google Scholar] [CrossRef] [PubMed]
- Cheng, H.; Wang, Y.; Wei, K.; Liu, Z.; Yang, M.; Cai, C. Visual encoder-based angle measurement method in low-frequency angular vibration calibration. Appl. Opt. 2022, 61, 7662–7670. [Google Scholar] [CrossRef]
- Li, Z.; Pei, Y.; Qu, C.; Yang, F. A Position and Attitude Measurement Method Based on Laser Displacement Sensor and Infrared Vision Camera. IEEE Trans. Instrum. Meas. 2022, 71, 3181902. [Google Scholar] [CrossRef]
- Wei, Z.; Feng, G.; Zhou, D.; Ma, Y.; Liu, M.; Luo, Q.; Huang, T. A Review of Position and Orientation Visual Measurement Methods and Applications. Laser Optoelectron. Prog. 2023, 60, 0312010. [Google Scholar] [CrossRef]
- Lowe, D.G. Distinctive Image Features from Scale-Invariant Keypoints. Int. J. Comput. Vis. 2004, 60, 91–110. [Google Scholar] [CrossRef]
- Xue, N.; Bai, S.; Wang, F.; Xia, G.-S.; Wu, T.; Zhang, L. Learning Attraction Field Representation for Robust Line Segment Detection. In Proceedings of the 2019 IEEE/CVF Conference on Computer Vision and Pattern Recognition (CVPR), Long Beach, CA, USA, 15–20 June 2019; pp. 1595–1603. [Google Scholar] [CrossRef]
- Von Gioi, R.G.; Jakubowicz, J.; Morel, J.M.; Randall, G. LSD: A Fast Line Segment Detector with a False Detection Control. IEEE Trans. Pattern Anal. Mach. Intell. 2010, 32, 722–732. [Google Scholar] [CrossRef] [PubMed]
- Hodan, T.; Barath, D.; Matas, J. EPOS: Estimating 6D Pose of Objects With Symmetries. In Proceedings of the 2020 IEEE/CVF Conference on Computer Vision and Pattern Recognition (CVPR), Seattle, WA, USA, 13–19 June 2020; pp. 11700–11709. [Google Scholar] [CrossRef]
- Choi, C.; Christensen, H.I. Real-time 3D Model-based Tracking Using Edge and Keypoint Features for Robotic Manipulation. In Proceedings of the 2010 IEEE International Conference on Robotics and Automation, Anchorage, AK, USA, 3–7 May 2010; pp. 4048–4055. [Google Scholar] [CrossRef]
- Choi, C.; Christensen, H.I. Robust 3D visual tracking using particle filtering on the SE(3) group. In Proceedings of the 2011 IEEE International Conference on Robotics and Automation, Shanghai, China, 9–13 May 2011; pp. 4384–4390. [Google Scholar] [CrossRef]
- Xiong, S.; Wang, Y.; Cai, Y.; Liu, J.; Liu, J.; Wu, G. Calculating the Effective Center Wavelength for Heterodyne Interferometry of an Optical Frequency Comb. Appl. Sci. 2018, 8, 2465. [Google Scholar] [CrossRef]
- Kleinman, D.A.; Ashkin, A.; Boyd, G.D. Second-harmonic generation of light by focused laser beams. Phys. Rev. 1966, 145, 338. [Google Scholar] [CrossRef]
- Dwi Astuti, W.; Matsukuma, H.; Nakao, M.; Li, K.; Shimizu, Y.; Gao, W. An Optical Frequency Domain Angle Measurement Method Based on Second Harmonic Generation. Sensors 2021, 21, 670. [Google Scholar] [CrossRef] [PubMed]
- Aharonov, Y.; Albert, D.Z.; Vaidman, L. How the Result of a Measurement of a Component of the Spin of a Spin-1/2 Particle Can Turn out to Be 100. Phys. Rev. Lett. 1988, 60, 1351–1354. [Google Scholar] [CrossRef] [PubMed]
- Dixon, P.B.; Starling, D.J.; Jordan, A.N.; Howell, J.C. Ultrasensitive Beam Deflection Measurement via Interferometric Weak Value Amplification. Phys. Rev. Lett. 2009, 102, 173601. [Google Scholar] [CrossRef]
- Dressel, J.; Malik, M.; Miatto, F.M.; Jordan, A.N.; Boyd, R.W. Understanding quantum weak values: Basics and applications. Rev. Mod. Phys. 2014, 86, 307. [Google Scholar] [CrossRef]
- Wang, Y.T.; Tang, J.S.; Hu, G.; Wang, J.; Yu, S.; Zhou, Z.Q.; Cheng, Z.D.; Xu, J.S.; Fang, S.Z.; Wu, Q.L.; et al. Experimental Demonstration of Higher Precision Weak-Value-Based Metrology Using Power Recycling. Phys. Rev. Lett. 2016, 117, 230801. [Google Scholar] [CrossRef]
- Qiu, X.D.; Xie, L.G.; Liu, X.; Luo, L.; Li, Z.X.; Zhang, Z.Y.; Du, J.L. Precision phase estimation based on weak-value amplification. Appl. Phys. Lett. 2017, 110, 4976312. [Google Scholar] [CrossRef]
- Xia, B.K.; Huang, J.Z.; Li, H.J.; Wang, H.; Zeng, G.H. Toward incompatible quantum limits on multiparameter estimation. Nat. Commun. 2023, 14, 1021. [Google Scholar] [CrossRef]
- Zhang, Y.; Liu, X.; Zhang, T.; Xu, Y.; Wang, Y.; Wang, Z. Micro-angle attitude measurement technology and research progress based on quantum weak value measurement. Proc. SPIE 2023, 12757, 81–87. [Google Scholar] [CrossRef]
- Cherepanska, I.Y.; Sazonov, A.Y.; Krushynska, N.I.; Priadko, V.A.; Lukiniuk, M.V. Quaternion Method of Calculating Angles while Measuring via Goniometric Precision Instrument System. Bull. Univ. Karaganda-Phys. 2021, 1, 46–56. [Google Scholar] [CrossRef]
- Ahmed, A.; Ju, H.H.; Yang, Y.; Xu, H. An Improved Unit Quaternion for Attitude Alignment and Inverse Kinematic Solution of the Robot Arm Wrist. Machines 2023, 11, 669. [Google Scholar] [CrossRef]
- Wang, X.; Su, J.; Yang, J.; Miao, L.; Huang, T. Investigation of heterodyne interferometer technique for dynamic angle measurement: Error analysis and performance evaluation. Meas. Sci. Technol. 2021, 32, 105016. [Google Scholar] [CrossRef]
- Mokros, J. Angle-measuring interferometer. Optoelectron. Instrum. Data Process. 1990, 3, 15–17. [Google Scholar]
- Crane, R. Angle-Scanned Interferometer. Opt. Eng. 1979, 18, 205–211. [Google Scholar] [CrossRef]
- Dai, X.; Sasaki, O.; Greivenkamp, J.E.; Suzuki, T. High accuracy, wide range, rotation angle measurement by the use of two parallel interference patterns. Appl. Opt. 1997, 36, 006190. [Google Scholar] [CrossRef] [PubMed]
- Tomlinson, W.J.; Mollenauer, L.F. Balanced Interferometers with Simply Adjustable Interference Angle. Appl. Opt. 1977, 16, 1806–1807. [Google Scholar] [CrossRef]
- Khoroshun, A.N.; Artsishevskii, D.N. Determining small angles of beam splitter rotation in optical vortex shearing interferometer. Tech. Phys. Lett. 2010, 36, 382–385. [Google Scholar] [CrossRef]
- Donati, S.; Norgia, M. Displacement and attitude angles (Tilt and Yaw) are measured by a single-channel self-mixing interferometer. In Proceedings of the CLEO: Applications and Technology 2016, San Jose, CA, USA, 5–10 June 2016. [Google Scholar]
- Serrano Galvez, P.; Butcher, M.; Masi, A. A Dual-Interferometer-Based Angular Measurement System With Absolute Angle Recovery Method. IEEE Trans. Instrum. Meas. 2019, 68, 864–873. [Google Scholar] [CrossRef]
- Liang, X.; Lin, J.; Yang, L.; Wu, T.; Liu, Y.; Zhu, J. Femtosecond pulse laser distance and angle interferometer. In Proceedings of the International Conference on Optical Instruments and Technology (OIT)—Optoelectronic Measurement Technology and Systems, Beijing, China, 26–28 October 2019. [Google Scholar] [CrossRef]
- Shestopalov, Y.N. Interference Methods and Means for Measuring Angles. Sov. J. Opt. Technol. 1990, 57, 589–593. [Google Scholar]
- Takashima, K. A Modified Angle Measuring Interferometer. Jpn. J. Appl. Phys. 1969, 8, 628. [Google Scholar] [CrossRef]
- Jiapeng, L.; Yi, W.; Zunyue, Z.; Yaojing, Z.; Hon Ki, T. Multimode Wavelength Division Demultiplexing Based on an Angled Multimode Interference Coupler. In Proceedings of the 2021 Asia Communications and Photonics Conference (ACP) 2021, Beijing, China, 24–27 October 2020; pp. 1–3. [Google Scholar]
- Wang, G.-F.; Chen, G.-L.; Chen, Y.-L. A new digital angle measurement technology by using Michelson interferometer and its application. Opt. Precis. Eng. 2001, 9, 85–88. [Google Scholar]
- Shi, P.; Stijns, E. New Optical Method for Measuring Small-Angle Rotations. Appl. Opt. 1988, 27, 4342–4344. [Google Scholar] [CrossRef] [PubMed]
- Chekirda, K.V.; Shur, V.L.; Lukin, A.Y.; Kos’mina, M.A.; Leibengardt, G.I. A Study of an Angle Examiner Based on the Fizeau Interferometer with Expanded Measurement Range. Meas. Tech. 2016, 59, 133–136. [Google Scholar] [CrossRef]
- Wang, X.; Yang, J.; Huang, T.; Shu, X. A high-frequency angular vibration calibration system based on phase modulated laser interferometer technique. In Proceedings of the Conference on AOPC—Micro-Optics and MOEMS, Beijing, China, 20–22 June 2021. [Google Scholar] [CrossRef]
- Eves, B.J.; Leroux, I.D.; Cen, A.J. Phase shifting angle interferometer. Metrologia 2023, 60, 055006. [Google Scholar] [CrossRef]
- Twu, R.-C.; Lin, B.-L. Development and investigation of birefringent Beta-Barium borate for optical angle sensor. Opt. Laser Technol. 2022, 155, 108369. [Google Scholar] [CrossRef]
- Chen, S.-H.; Chen, H.-Y.; Lai, Y.-X.; Hsieh, H.-L. Development of a double-diffraction grating interferometer for measurements of displacement and angle. In Proceedings of the Conference on Optics and Photonics for Advanced Dimensional Metrology, Electr Network, Online, 6–10 April 2020. [Google Scholar] [CrossRef]
- Hsu, Y.-T.; Hsieh, H.-L. Development of a Coplanar Grating Interferometer for Displacement and Angle Measurements. In Proceedings of the Conference on Optics and Photonics for Advanced Dimensional Metrology II Part of SPIE Photonics Europe Conference, Electr Network, Strasbourg, France, 5 April–20 May 2022. [Google Scholar] [CrossRef]
- Xu, X.; Dai, Z.R.; Wang, Y.F.; Li, M.F.; Tan, Y.D. High Sensitivity and Full-Circle Optical Rotary Sensor for Non-Cooperatively Tracing Wrist Tremor With Nanoradian Resolution. IEEE Trans. Ind. Electron. 2022, 69, 9605–9612. [Google Scholar] [CrossRef]
- Xu, X.; Dai, Z.; Tan, Y. A Dual-Beam Differential Method Based on Feedback Interferometry for Noncontact Measurement of Linear and Angular Displacement. IEEE Trans. Ind. Electron. 2023, 70, 6405–6413. [Google Scholar] [CrossRef]
- Yu, L.; Feng, X.; Hu, P.; Lin, X.; Jing, T. Development of a 3-DOF Angle Sensor Based on a Single Laser Interference Probe. Micromachines 2023, 14, 2221. [Google Scholar] [CrossRef]
- Shi, K.; Su, J.; Hou, W. Roll angle measurement system based on differential plane mirror interferometer. Opt. Express 2018, 26, 19826–19834. [Google Scholar] [CrossRef] [PubMed]
- Liang, X.; Lin, J.; Wu, T.; Yang, L.; Wang, Y.; Liu, Y.; Zhu, J. Absolute angular measurement with optical frequency comb using a dispersive interferometry. Opt. Express 2020, 28, 411546. [Google Scholar] [CrossRef] [PubMed]
- Liu, T.; Wu, J.; Suzuki, A.; Sato, R.; Matsukuma, H.; Gao, W. Improved Algorithms of Data Processing for Dispersive Interferometry using Optical Frequency Comb. Preprints 2023. [Google Scholar] [CrossRef]
- Hargrove, L.E.; Fork, R.L.; Pollack, M.A. Locking of He–Ne Laser Modes Induced by Synchronous Intracavity Modulation. Appl. Phys. Lett. 1964, 5, 4–5. [Google Scholar] [CrossRef]
- Chang, L.; Liu, S.; Bowers, J.E. Integrated optical frequency comb technologies. Nat. Photonics 2022, 16, 95–108. [Google Scholar] [CrossRef]
- Shimizu, Y.; Matsukuma, H.; Gao, W. Optical Angle Sensor Technology Based on the Optical Frequency Comb Laser. Appl. Sci. 2020, 10, 4047. [Google Scholar] [CrossRef]
- Fortier, T.; Baumann, E. 20 years of developments in optical frequency comb technology and applications. Commun. Phys. 2019, 2, 153. [Google Scholar] [CrossRef]
- Diddams, S.A. The evolving optical frequency comb. J. Opt. Soc. Am. B 2010, 27, B51–B62. [Google Scholar] [CrossRef]
- Newbury, N.R. Searching for applications with a fine-tooth comb. Nat. Photonics 2011, 5, 186–188. [Google Scholar] [CrossRef]
- Diddams, S.A.; Vahala, K.; Udem, T. Optical frequency combs: Coherently uniting the electromagnetic spectrum. Science 2020, 369, eaay3676. [Google Scholar] [CrossRef]
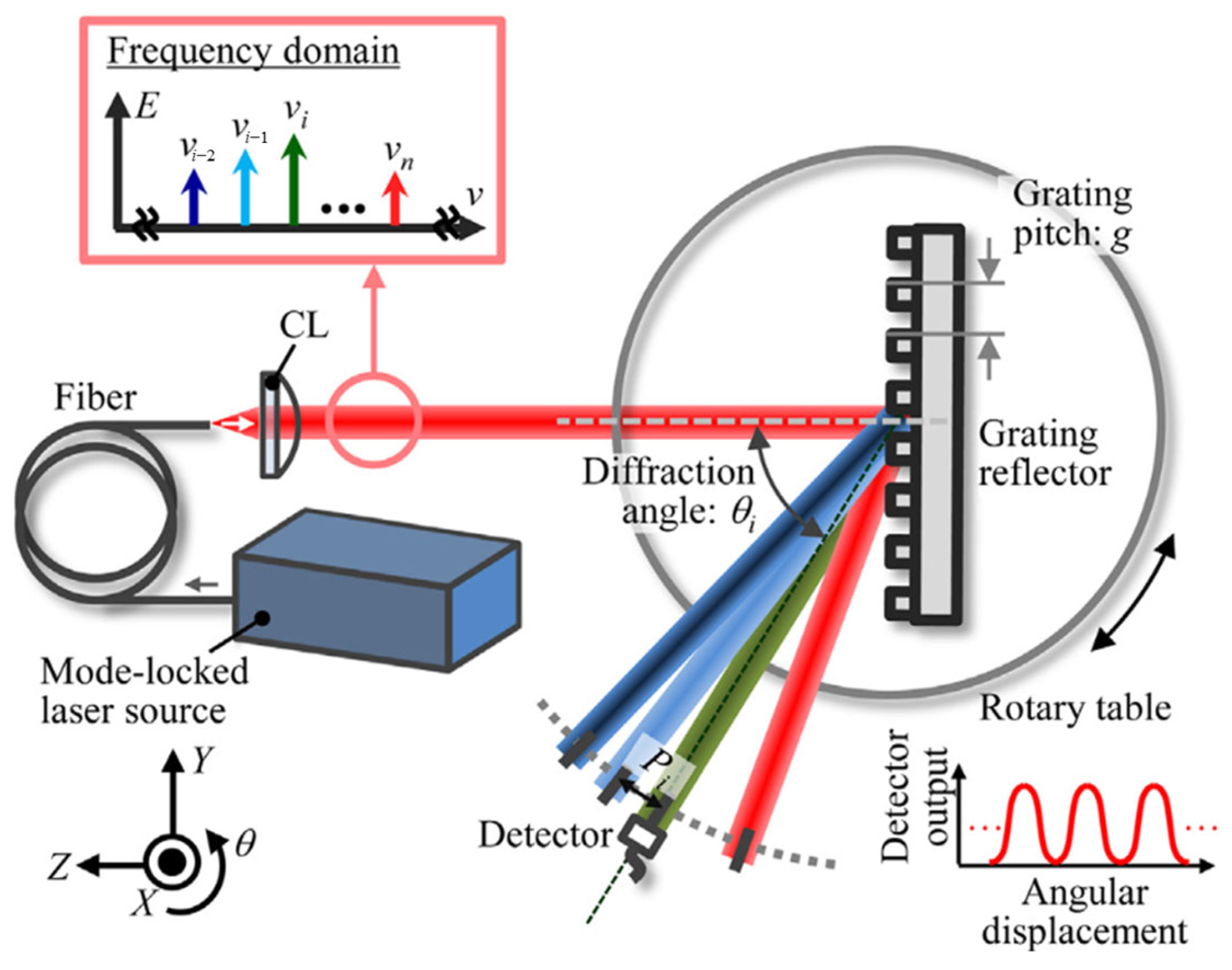
- Han, S.; Kim, Y.-J.; Kim, S.-W. Parallel determination of absolute distances to multiple targets by time-of-flight measurement using femtosecond light pulses. Opt. Express 2015, 23, 025874. [Google Scholar] [CrossRef] [PubMed]
- Dale, J.; Hughes, B.; Lancaster, A.J.; Lewis, A.J.; Reichold, A.J.H.; Warden, M.S. Multi-channel absolute distance measurement system with sub ppm-accuracy and 20 m range using frequency scanning interferometry and gas absorption cells. Opt. Express 2014, 22, 024869. [Google Scholar] [CrossRef] [PubMed]
- Cao, K.; Qiu, C.; Gong, Y.; Li, J. An accurate measurement method of gear transmission error based on a double-angle measurement device. Proc. Inst. Mech. Eng. Part C J. Mech. Eng. Sci. 2023, 238, 2521–2531. [Google Scholar] [CrossRef]
- Li, Y.; Shen, T.; Zhang, Y.; Zhao, L.; Zhou, L. The Five-axis Turn-table Angle Location Error Analysis based on Dual-frequency Laser Phase Measure System. J. Astronaut. Metrol. Meas. 2021, 41, 34–37. [Google Scholar]
- Andevari, S.A.; Olvera-Cervantes, J.-L.; Saavedra, C.E. Inclination Sensor With a Wide Angle Measurement Range Using Half-Wavelength Microstrip Resonator. IEEE Access 2023, 11, 28699–28705. [Google Scholar] [CrossRef]
- Wu, L.; Su, R.; Tong, P.; Yanchen, A.; Wu, Y. An inductive sensor for the angular displacement measurement of large and hollow rotary machinery. IEEE Sens. J. 2023, 23, 21709–21717. [Google Scholar] [CrossRef]
- Komatsuzaki, S.; Takeyama, A.; Sado, K.; Nagatsu, Y.; Hashimoto, H. Absolute Angle Calculation for Magnetic Encoder Based On Magnetic Flux Density Difference. In Proceedings of the 47th Annual Conference of the IEEE-Industrial-Electronics-Society (IECON), Electr Network, Toronto, ON, Canada, 13–16 October 2021. [Google Scholar] [CrossRef]
- Jeong, S.-H.; Rhyu, S.-H.; Kwon, B.-I.; Kim, B.-T. Design of the Rotary Magnetic Position Sensor With the Sinusoidally Magnetized Permanent Magnet. IEEE Trans. Magn. 2007, 43, 1837–1840. [Google Scholar] [CrossRef]
- Zhang, Z.; Ni, F.; Dong, Y.; Guo, C.; Jin, M.; Liu, H. A Novel Absolute Magnetic Rotary Sensor. IEEE Trans. Ind. Electron. 2015, 62, 4408–4419. [Google Scholar] [CrossRef]
- Wu, S.-T.; Chen, J.-Y.; Wu, S.-H. A Rotary Encoder With an Eccentrically Mounted Ring Magnet. IEEE Trans. Instrum. Meas. 2014, 63, 1907–1915. [Google Scholar] [CrossRef]
- Liu, C.; Xu, F. A Novel Absolute Angle-Measuring Method with Rotary Inductosyn. In Proceedings of the 2018 Eighth International Conference on Instrumentation & Measurement, Computer, Communication and Control (IMCCC), Harbin, China, 19–21 July 2018; pp. 1753–1757. [Google Scholar] [CrossRef]
- Zhang, Z.; Ni, F.; Dong, Y.; Jin, M.; Liu, H. A novel absolute angular position sensor based on electromagnetism. Sens. Actuators A Phys. 2013, 194, 196–203. [Google Scholar] [CrossRef]
- Dezhi, Z.; Shaobo, Z.; Shuai, W.; Chun, H.; Xiaomeng, Z. A Capacitive Rotary Encoder Based on Quadrature Modulation and Demodulation. IEEE Trans. Instrum. Meas. 2015, 64, 143–153. [Google Scholar] [CrossRef]
- Anandan, N.; George, B. A Wide-Range Capacitive Sensor for Linear and Angular Displacement Measurement. IEEE Trans. Ind. Electron. 2017, 64, 5728–5737. [Google Scholar] [CrossRef]
- Sun, S.Z.; Lv, Z.; Han, Y.; He, Z.Y.; Zhang, J.M. A novel inductive angular displacement sensor based on time-grating. Meas. Sci. Technol. 2022, 33, 055105. [Google Scholar] [CrossRef]
- Chen, X.; He, X.; Zhang, Y.; Gao, B. Angle Measurement Method Based on Cross-Orthogonal Hall Sensor. In Proceedings of the 2023 IEEE 16th International Conference on Electronic Measurement & Instruments (ICEMI), Harbin, China, 9–11 August 2023. [Google Scholar]
- Dorofeev, N.V.; Kuzichkin, O.R.; Tsaplev, A.V. Accelerometric Method of Measuring the Angle of Rotation of the Kinematic Mechanisms of Nodes. Appl. Mech. Mater. 2015, 770, 592–597. [Google Scholar] [CrossRef]
- Gao, Y.; Zhang, X.; Mai, G.; Hu, R. Measurement Method for Angle Acceleration of Turn-table Based on Accelerometer. J. Astronaut. Metrol. Meas. 2015, 35, 14–16. [Google Scholar]
- Lu, Y.-l.; Pan, Y.-J.; Li, L.-l.; Liu, Y.; Peng, H. Measurement method of projectile’s heading and pitching angle velocities based on biaxial accelerometer. J. Chin. Inert. Technol. 2015, 23, 160–164. [Google Scholar] [CrossRef]
- Shi, K.; Xu, G.; Qian, R.; Wang, J. Single Accelerometer Roll Angle Measurement System Error Analysis. J. Detect. Control 2016, 38, 61–65. [Google Scholar]
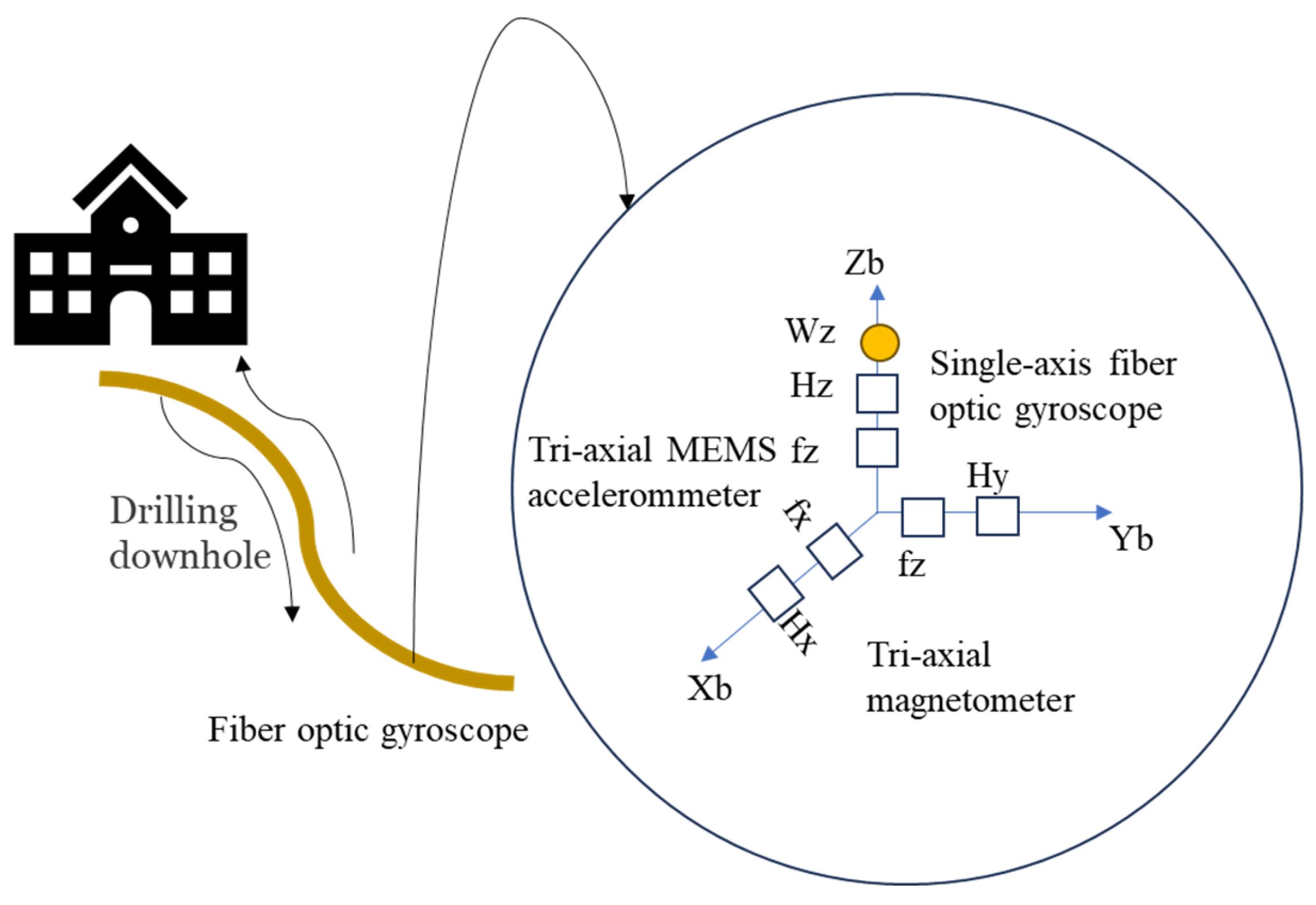
- Song, H.; Huang, H.; Zhang, J.; Qu, D. A new measurement-while-drilling system based on inertial technology. Adv. Mech. Eng. 2018, 10, 1687814018767498. [Google Scholar] [CrossRef]
- Tian, H.; Liu, Y.; Zhou, J.; Wang, Y.; Wang, J.; Zhang, W. Attitude Angle Compensation for a Synchronous Acquisition Method Based on an MEMS Sensor. Sensors 2019, 19, 483. [Google Scholar] [CrossRef]
- Wang, K.; Wu, Y. Error Compensation Technology for Inclinometer Accelerometer Bias. Instrum. Technol. Sens. 2022, 1, 45–48. [Google Scholar]
- Jabbar, K.A.; Khemri, N.A.; Brayyich, M.R.; Ftaiet, A.A. Design of a tilt angle measurement and control system. AIP Conf. Proc. 2023, 2845, 070017. [Google Scholar] [CrossRef]
- Ren, C.; Guo, D.; Zhang, L.; Wang, T. Research on Nonlinear Compensation of the MEMS Gyroscope under Tiny Angular Velocity. Sensors 2022, 22, 6577. [Google Scholar] [CrossRef] [PubMed]
- Lijia, Y.; Qiang, L.; Guochen, W.; Hualiang, S.; Qingzhi, Z. An on-orbit high-precision angular vibration measuring device based on laser gyro. Proc. SPIE 2022, 12169, 1216943. [Google Scholar] [CrossRef]
- Liu, Y.; Luo, X.; Chen, X. Improvement of angle random walk of fiber-optic gyroscope using polarization-maintaining fiber ring resonator. Opt. Express 2022, 30, 29900–29906. [Google Scholar] [CrossRef]
- Ma, R.; Yu, H.; Ni, K.; Zhou, Q.; Li, X.; Wu, G. Angular velocity measurement system based on optical frequency comb. In Proceedings of the Conference on Semiconductor Lasers and Applications IX, Hangzhou, China, 21–23 October 2019. [Google Scholar] [CrossRef]
- Yu, C.; Cai, Y.; Ren, Y.; Wang, W.; Yin, Z.; Li, L.; Han, W.; Han, W. Angular Rate Measurement Method of Magnetically Suspended Control and Sensing Gyroscope Using Component-Level Rotation Modulation. IEEE Sens. J. 2022, 22, 21575–21584. [Google Scholar] [CrossRef]
- Sugiyama, Y.; Matsui, Y.; Toyoda, H.; Mukozaka, N.; Ihori, A.; Abe, T.; Takabe, M.; Mizuno, S. A 3.2 kHz, 14-Bit Optical Absolute Rotary Encoder With a CMOS Profile Sensor. IEEE Sens. J. 2008, 8, 1430–1436. [Google Scholar] [CrossRef]
- Kim, J.-A.; Kim, J.W.; Kang, C.-S.; Jin, J.; Eom, T.B. Absolute angle measurement using a phase-encoded binary graduated disk. Measurement 2016, 80, 288–293. [Google Scholar] [CrossRef]
- Yu, H.; Jia, X.; Wan, Q.; Zhao, C.; Sun, Y. High-resolution angular measurement arithmetic based on pixel interpolations. Measurement 2020, 149, 106948. [Google Scholar] [CrossRef]
- Kim, J.-A.; Lee, J.Y.; Kang, C.-S.; Eom, S.H. Measurement of Six-Degree-of-Freedom Absolute Postures Using a Phase-Encoded Pattern Target and a Monocular Vision System. Int. J. Precis. Eng. Manuf. 2023, 24, 1191–1203. [Google Scholar] [CrossRef]
- Dong, H.; Fu, Q.; Zhao, X.; Quan, Q.; Zhang, R. Practical rotation angle measurement method by monocular vision. Appl. Opt. 2015, 54, 425. [Google Scholar] [CrossRef]
- Yu, H. Angle measurement based on in-line digital holographic reconstruction. Opt. Lasers Eng. 2021, 137, 106385. [Google Scholar] [CrossRef]
- Gao, S.; Bai, L. Monocular Camera-Based Three-Point Laser Pointer Ranging and Pose Estimation Method. Acta Opt. Sin. 2021, 41, 0915001. [Google Scholar] [CrossRef]
- Zhao, Y.; Zhang, Y.; Zhang, H.; Xue, L.; Ren, M.; Miao, Y. Rotation angle measurement method based on self-mixing interference of a fiber laser. Appl. Opt. 2022, 61, 3174–3181. [Google Scholar] [CrossRef] [PubMed]
- Vadapalli, D.R.P.; Dey, K.; Roy, S. Optical Fiber-Based Intensity-Modulated Cost-Effective Small Lean Angle Measurement Sensor. Mapan-J. Metrol. Soc. India 2021, 36, 925–930. [Google Scholar] [CrossRef]
- Zheng, L.-F.; Zhang, J.-S.; Liang, H.-J.; Wang, H.-J. Angle sensor for humidity-insensitive angle measurement based on multimode interference. Opt. Commun. 2022, 522, 128579. [Google Scholar] [CrossRef]
- Dai, M.; Zhang, C.; Pan, X.; Yang, Y.; Li, Z. A Novel Attitude Measurement While Drilling System Based on Single-Axis Fiber Optic Gyroscope. IEEE Trans. Instrum. Meas. 2022, 71, 3133328. [Google Scholar] [CrossRef]
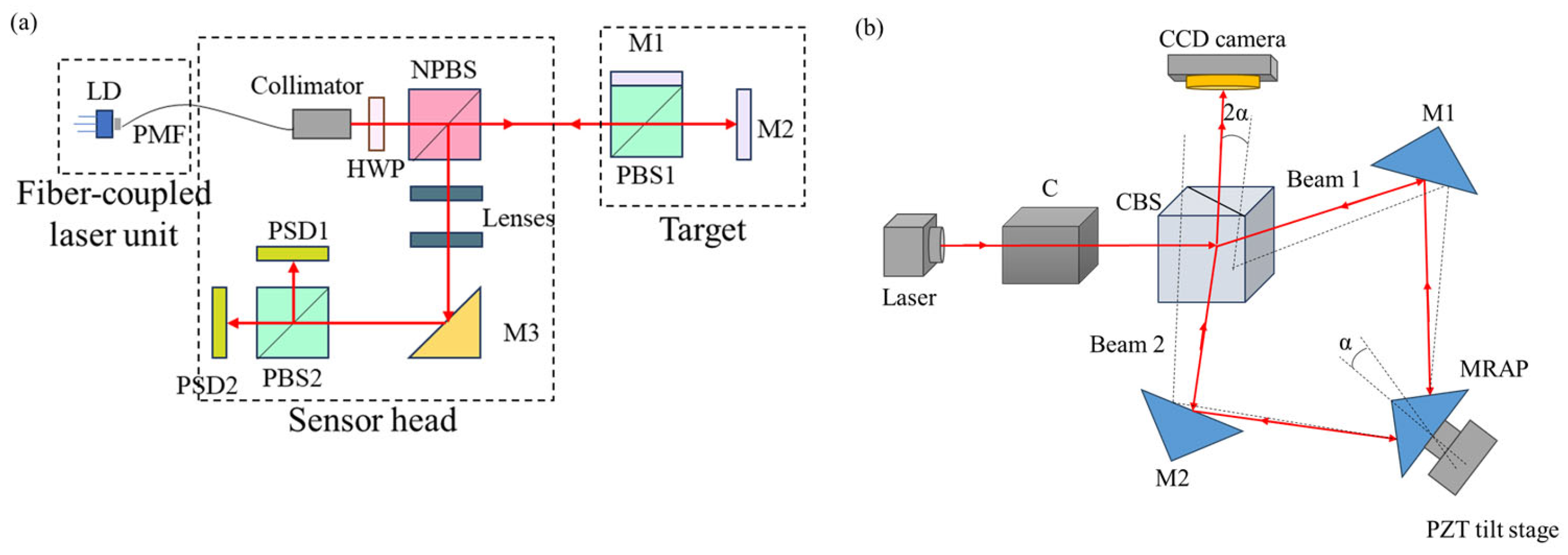
- Yusuke, S.; Yoshikazu, A.; Wei, G. Detection of three-axis angles by an optical sensor. Sens. Actuators A Phys. 2009, 150, 175–183. [Google Scholar] [CrossRef]
- Heikkinen, V.; Byman, V.; Palosuo, I.; Hemming, B.; Lassila, A. Interferometric 2D small angle generator for autocollimator calibration. Metrologia 2017, 54, 253–261. [Google Scholar] [CrossRef]
- Gao, W. Metrology; Springer: Singapore, 2019. [Google Scholar]
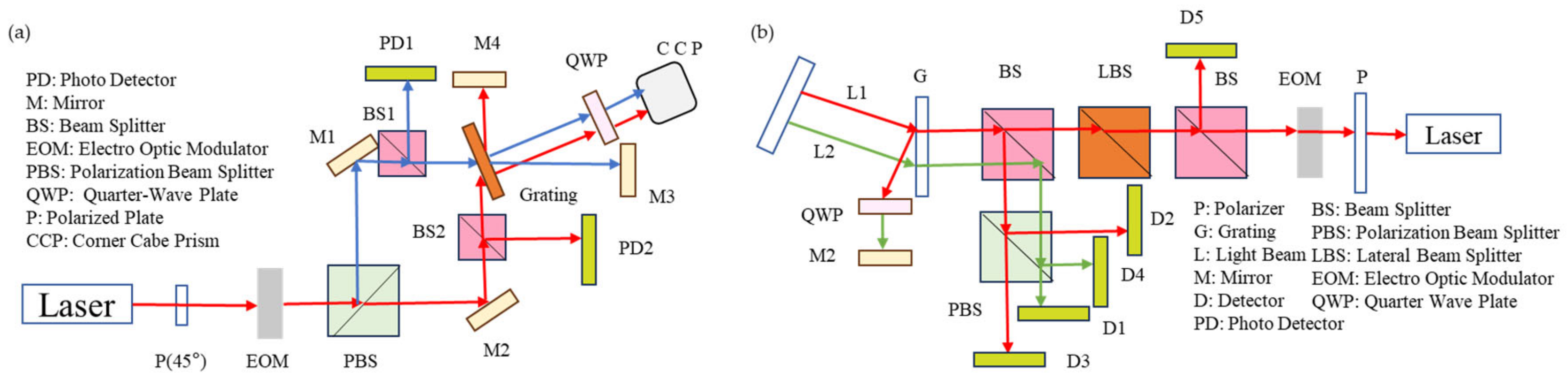
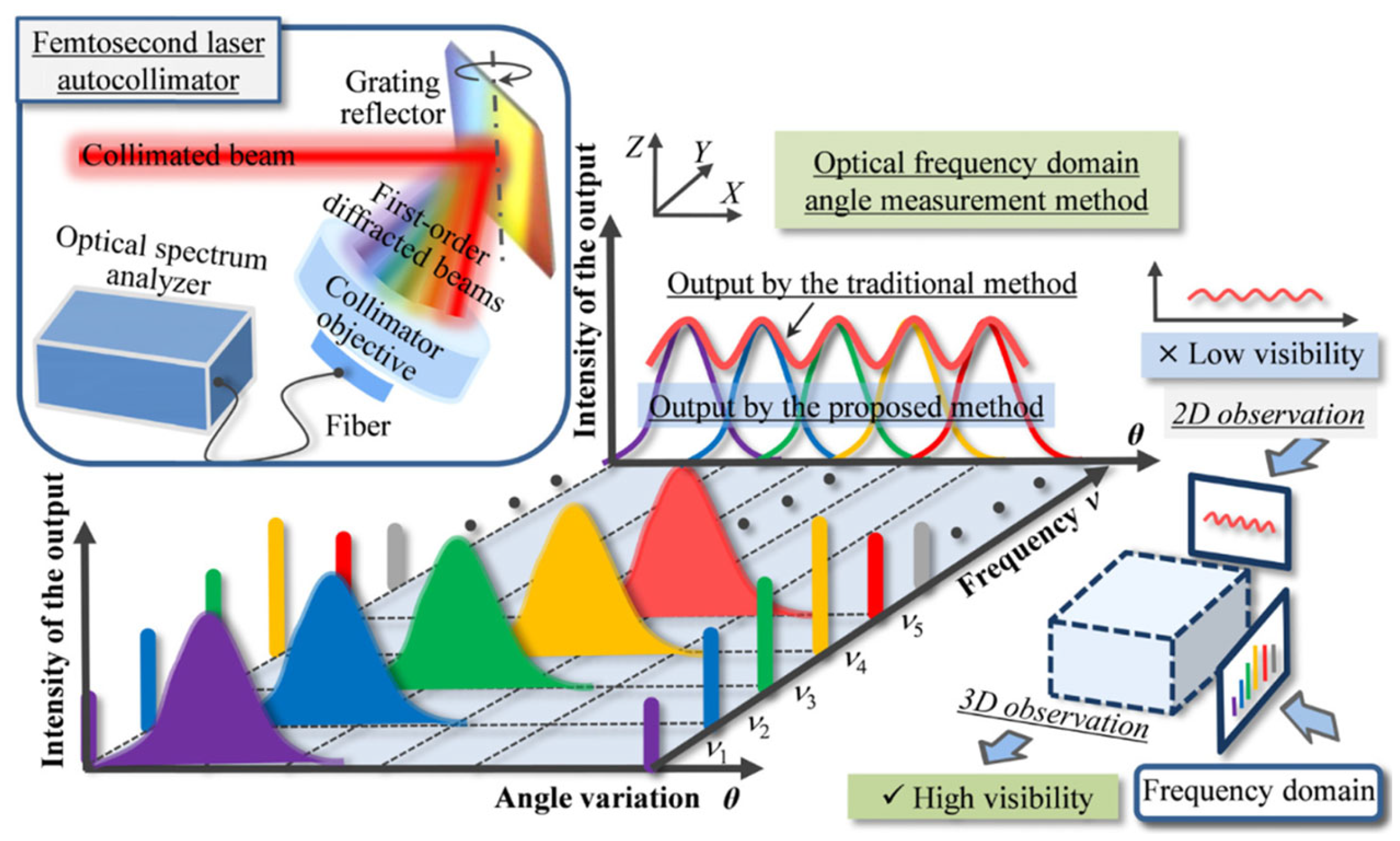
- Chen, Y.-L.; Shimizu, Y.; Tamada, J.; Kudo, Y.; Madokoro, S.; Nakamura, K.; Gao, W. Optical frequency domain angle measurement in a femtosecond laser autocollimator. Opt. Express 2017, 25, 16725–16738. [Google Scholar] [CrossRef]
- Chen, Y.-L.; Shimizu, Y.; Tamada, J.; Nakamura, K.; Matsukuma, H.; Chen, X.; Gao, W. Laser autocollimation based on an optical frequency comb for absolute angular position measurement. Precis. Eng. 2018, 54, 284–293. [Google Scholar] [CrossRef]
- Matsukuma, H.; Ikeda, K.; Sato, R.; Gao, W. Autocollimation employing optical frequency comb. Proc. SPIE 2023, 12607, 20–22. [Google Scholar] [CrossRef]
- Chen, Y.-L.; Shimizu, Y.; Kudo, Y.; Ito, S.; Gao, W. Mode-locked laser autocollimator with an expanded measurement range. Opt. Express 2016, 24, 015554. [Google Scholar] [CrossRef] [PubMed]
- Shimizu, Y.; Madokoro, S.; Matsukuma, H.; Gao, W. An optical angle sensor based on chromatic dispersion with a mode-locked laser source. J. Adv. Mech. Des. Syst. Manuf. 2018, 12, JAMDSM0096. [Google Scholar] [CrossRef]
- Gao, W.; Saito, Y.; Muto, H.; Arai, Y.; Shimizu, Y. A three-axis autocollimator for detection of angular error motions of a precision stage. CIRP Ann.-Manuf. Technol. 2011, 60, 515–518. [Google Scholar] [CrossRef]
- Wang, S.T.; Luo, L.B.; Zhu, J.H.; Shi, N.N.; Li, X.H. An Ultra-Precision Absolute-Type Multi-Degree-of-Freedom Grating Encoder. Sensors 2022, 22, 9047. [Google Scholar] [CrossRef]
- Li, X.H.; Gao, W.; Shimizu, Y.; Ito, S. A two-axis Lloyd’s mirror interferometer for fabrication of two-dimensional diffraction gratings. CIRP Ann.-Manuf. Technol. 2014, 63, 461–464. [Google Scholar] [CrossRef]
- Li, X.H.; Shimizu, Y.; Ito, S.; Gao, W. Fabrication of Scale Gratings for Surface Encoders by Using Laser Interference Lithography with 405 nm Laser Diodes. Int. J. Precis. Eng. Manuf. 2013, 14, 1979–1988. [Google Scholar] [CrossRef]
- Zhou, Q.; Pang, J.C.; Li, X.H.; Ni, K.; Tian, R. Concave grating miniature spectrometer with an expanded spectral band by using two entrance slits. Chin. Opt. Lett. 2015, 13, 110501. [Google Scholar] [CrossRef]
- Xue, G.P.; Zhai, Q.H.; Lu, H.O.; Zhou, Q.; Ni, K.; Lin, L.Y.; Wang, X.H.; Li, X.H. Polarized holographic lithography system for high-uniformity microscale patterning with periodic tunability. Microsyst. Nanoeng. 2021, 7, 31. [Google Scholar] [CrossRef]
- Li, X.H.; Ni, K.; Zhou, Q.; Wang, X.H.; Tian, R.; Pang, J.C. Fabrication of a concave grating with a large line spacing via a novel dual-beam interference lithography method. Opt. Express 2016, 24, 010759. [Google Scholar] [CrossRef]
- Zhou, Q.; Li, X.H.; Ni, K.; Tian, R.; Pang, J.C. Holographic fabrication of large-constant concave gratings for wide-range flat-field spectrometers with the addition of a concave lens. Opt. Express 2016, 24, 732–738. [Google Scholar] [CrossRef] [PubMed]
- Matsukuma, H.; Ishizuka, R.; Furuta, M.; Xinghui, L.; Shimizu, Y.; Wei, G. Reduction in Cross-Talk Errors in a Six-Degree-of-Freedom Surface Encoder. Nanomanuf. Metrol. 2019, 2, 111–123. [Google Scholar] [CrossRef]
- Xue, G.P.; Lu, H.O.; Li, X.H.; Zhou, Q.; Wu, G.H.; Wang, X.H.; Zhai, Q.H.; Ni, K. Patterning nanoscale crossed grating with high uniformity by using two-axis Lloyd’s mirrors based interference lithography. Opt. Express 2020, 28, 2179–2191. [Google Scholar] [CrossRef] [PubMed]
- Li, X.H.; Zhou, Q.; Zhu, X.W.; Lu, H.O.; Yang, L.; Ma, D.H.; Sun, J.H.; Ni, K.; Wang, X.H. Holographic fabrication of an arrayed one-axis scale grating for a two-probe optical linear encoder. Opt. Express 2017, 25, 16028–16039. [Google Scholar] [CrossRef] [PubMed]
- Li, X.H.; Lu, H.O.; Zhou, Q.; Wu, G.H.; Ni, K.; Wang, X.H. An Orthogonal Type Two-Axis Lloyd’s Mirror for Holographic Fabrication of Two-Dimensional Planar Scale Gratings with Large Area. Appl. Sci. 2018, 8, 2283. [Google Scholar] [CrossRef]
- Xu, B.; Jia, Z.; Li, X.; Chen, Y.-L.; Shimizu, Y.; Ito, S.; Gao, W. Surface form metrology of micro-optics. In Proceedings of the International Conference on Optics in Precision Engineering and Nanotechnology, icOPEN 2013, Singapore, 9–11 April 2013. [Google Scholar] [CrossRef]
- Li, X.; Zhu, X.; Zhou, Q.; Wang, H.; Ni, K. Low-cost lithography for fabrication of one-dimensional diffraction gratings by using laser diodes. In Proceedings of the 2015 International Conference on Optical Instruments and Technology: Micro/Nano Photonics and Fabrication, OIT 2015, Beijing, China, 17–19 May 2015. [Google Scholar] [CrossRef]
- Li, X.H.; Ni, K.; Zhou, Q.; Yan, P.; Pang, J.C.; Wang, X.H. Improved master-replica separation process for fabrication of a blazed concave grating by using a combination-type convex grating. Appl. Opt. 2017, 56, 298–302. [Google Scholar] [CrossRef]
- Wang, G.C.; Xue, G.P.; Zhai, Q.H.; Zhu, J.H.; Yu, K.N.; Huang, G.Y.; Wang, M.; Zhong, A.H.; Zhu, L.X.; Yan, S.H.; et al. Planar diffractive grating for magneto-optical trap application: Fabrication and testing. Appl. Opt. 2021, 60, 9358–9364. [Google Scholar] [CrossRef]
- Gao, W.; Kimura, A. A three-axis displacement sensor with nanometric resolution. CIRP Ann.-Manuf. Technol. 2007, 56, 529–532. [Google Scholar] [CrossRef]
- Liao, B.; Wang, S.; Lin, J.; Dou, Y.; Wang, X.; Li, X.; Zhu, J.; Jiang, J.; Han, S.; Zeng, L. A research on compact short-distance grating interferometer based on ridge prism. In Proceedings of the 2021 International Conference on Optical Instruments and Technology: Optoelectronic Measurement Technology and Systems, Online, 8–10 April 2022. [Google Scholar] [CrossRef]
- Wang, S.; Gao, L.; Luo, L.; Deng, F.; Wang, X.; Ma, R.; Li, X. Codes coupling optimization for absolute measurement. In Proceedings of the Optical Metrology and Inspection for Industrial Applications X, Beijing, China, 15–16 October 2023. [Google Scholar] [CrossRef]
- Wang, S.; Luo, L.; Gao, L.; Wang, X.; Ma, R.; Li, X. Long binary coding design for absolute positioning using genetic algorithm. In Proceedings of the Optical Metrology and Inspection for Industrial Applications X, Beijing, China, 15–16 October 2023. [Google Scholar] [CrossRef]
- Wang, S.; Zhu, J.; Shi, N.; Luo, L.; Wen, Y.; Li, X. Modeling and test of an absolute four-degree-of-freedom (DOF) grating encoder. In Proceedings of the Optical Metrology and Inspection for Industrial Applications IX, Electr Network, Beijing, China, 5–12 December 2022. [Google Scholar] [CrossRef]
- Shimizu, Y.; Kudo, Y.; Chen, Y.-L.; Ito, S.; Gao, W. An optical lever by using a mode-locked laser for angle measurement. Precis. Eng. 2017, 47, 72–80. [Google Scholar] [CrossRef]
- Shan, S.; Li, J.; Liu, P.; Li, Q.; Wang, X.; Li, X. A Microlens Array Grating for Miniature Multi-Channel Spectrometers. Sensors 2023, 23, 8381. [Google Scholar] [CrossRef]
- Tan, S.L.; Shimizu, Y.; Meguro, T.; Ito, S.; Gao, W. Design of a laser autocollimator-based optical sensor with a rangefinder for error correction of precision slide guideways. Int. J. Precis. Eng. Manuf. 2015, 16, 423–431. [Google Scholar] [CrossRef]
- Shimizu, Y.; Tan, S.L.; Murata, D.; Maruyama, T.; Ito, S.; Chen, Y.-L.; Gao, W. Ultra-sensitive angle sensor based on laser autocollimation for measurement of stage tilt motions. Opt. Express 2016, 24, 2788. [Google Scholar] [CrossRef] [PubMed]
- Gao, W.; Ohnuma, T.; Satoh, H.; Shimizu, H.; Kiyono, S. A Precision Angle Sensor Using a Multi-cell Photodiode Array. CIRP Ann. 2004, 53, 425–428. [Google Scholar] [CrossRef]
- Matsukuma, H.; Madokoro, S.; Astuti, W.D.; Shimizu, Y.; Gao, W. A New Optical Angle Measurement Method Based on Second Harmonic Generation with a Mode-Locked Femtosecond Laser. Nanomanuf. Metrol. 2019, 2, 187–198. [Google Scholar] [CrossRef]
- Matsukuma, H.; Asumi, Y.; Nagaoka, M.; Shimizu, Y.; Gao, W. An autocollimator with a mid-infrared laser for angular measurement of rough surfaces. Precis. Eng. 2021, 67, 89–99. [Google Scholar] [CrossRef]
- Lim, H.; Shimizu, Y. Feasible Resolution of Angular Displacement Measurement by an Optical Angle Sensor Based on Laser Autocollimation. Nanomanuf. Metrol. 2023, 6, 32. [Google Scholar] [CrossRef]
- Astrua, M.; Pisani, M. Improved performance of a refurbished photoelectric autocollimator. Meas. Sci. Technol. 2021, 32, 015010. [Google Scholar] [CrossRef]
- Luo, L.; Gao, L.; Wang, S.; Deng, F.; Wu, Y.; Li, X. An ultra-precision error estimation for a multi-axes grating encoder using quadrant photodetectors. In Proceedings of the Conference on Optical Metrology and Inspection for Industrial Applications IX Part of SPIE/COS Photonics Asia Conference, Electr Network, Online, 5–12 December 2022. [Google Scholar] [CrossRef]
- Gibson, S.J.; Charrett, T.O.H.; Tatam, R.P.; Lehmann, P.; Osten, W.; Albertazzi Gonçalves, A. Absolute angle measurement using dual-wavelength laser speckle for robotic manufacturing. In Proceedings of the Optical Measurement Systems for Industrial Inspection XI, Munich, Germany, 21 June 2019. [Google Scholar] [CrossRef]
- Yan, B.; Tan, Q.; Lv, N. Application of photoelectric autocollimator in detecting position precision of NC motorized stage. In Proceedings of the Conference on Optical Metrology and Inspection for Industrial Applications, Beijing, China, 18–20 October 2010. [Google Scholar] [CrossRef]
- Yandayan, T.; Akgoz, S.A.; Asar, M. Calibration of high-resolution electronic autocollimators with demanded low uncertainties using single reading head angle encoders. Meas. Sci. Technol. 2014, 25, 015010. [Google Scholar] [CrossRef]
- Peng, L.I.U.; Li-min, G.A.O.; Su-wen, Z. CCD in the Autocollimator Used for Moire Fringe Imaging. Acta Photonica Sin. 2010, 39, 494–498. [Google Scholar] [CrossRef]
- Sharma, B.D. Design and testing of a multipurpose autocollimator. CSIO Commun. 1975, 2, 51–55. [Google Scholar]
- Yan, B.; Tan, Q.; Lv, N. Detection of Positional Precision of NC Motorized Stage Based on Photoelectric Autocollimator. In Proceedings of the 6th International Symposium on Precision Engineering Measurements and Instrumentation, Hangzhou, China, 8–11 August 2010. [Google Scholar] [CrossRef]
- Andreeva, T.A.; Bokhman, E.D.; Venediktov, V.Y.; Gordeev, S.V.; Korolev, A.N.; Kos’mina, M.A.; Lukin, A.Y.; Shur, V.L. Estimation of metrological characteristics of a high-precision digital autocollimator using an angle encoder. J. Opt. Technol. 2018, 85, 406–409. [Google Scholar] [CrossRef]
- Shen, M.Z.; Liao, S. A high precision dynamic autocollimator. Meas. Technol. Intell. Instrum. VI 2005, 295–296, 337–342. [Google Scholar] [CrossRef]
- Heikkinen, V.; Byman, V.; Shpak, M.; Geckeler, R.; Just, A.; Krause, M.; Schumann, M.; Lassila, A. High-accuracy autocollimator calibration by interferometric 2D angle generator. In Proceedings of the Conference on Advances in Metrology for X-Ray and EUV Optics VIII, San Diego, CA, USA, 11–12 August 2019. [Google Scholar] [CrossRef]
- Bergues, G.J.; Schurrer, C.; Brambilla, N.; Canali, L. Misalignment contribution to the autocollimator’s scale distortion. In Proceedings of the IEEE International Instrumentation and Measurement Technology Conference (I2MTC), Politecnico Torino, Torino, Italy, 22–27 May 2017; pp. 231–235. [Google Scholar]
- Kranz, O.; Geckeler, R.D.; Just, A.; Krause, M. Modelling PTB’s Spatial Angle Autocollimator Calibrator. In Proceedings of the Conference on Modeling Aspects in Optical Metrology IV as part of the SPIE Optical Metrology Symposium, Munich, Germany, 13–14 May 2013. [Google Scholar] [CrossRef]
- Wei, Y.; Wu, Y.; Xiao, M.; Lu, W. Optical System Design of Dual-spectrum Autocollimator. In Proceedings of the Annual Conference of the Chinese-Society-for-Optical-Engineering on Applied Optics and Photonics, China (AOPC), Beijing, China, 5–7 May 2015. [Google Scholar] [CrossRef]
- Gao, Y.; Liao, J.; Zhao, X.; Xu, J.; Shen, X.; Zhu, H. Optimal design for three dimensional autocollimator. In Proceedings of the Annual Conference of the Chinese-Society-for-Optical-Engineering (CSOE) on Applied Optics and Photonics China (AOPC)—3D Measurement Technology for Intelligent Manufacturing, Beijing, China, 4–6 June 2017. [Google Scholar] [CrossRef]
- Filatov, Y.V.; Larichev, R.A. Precision issues in angle measurements by means of autocollimator. In Proceedings of the Conference on Optical Metrology and Inspection for Industrial Applications III held as part of SPIE/COS Photonics Asia, Beijing, China, 9–11 October 2014. [Google Scholar] [CrossRef]
- Hu, T.; Wang, D.; Li, Z. Research of the Influence of Rectangular Prism Pien Error on Angle Measurement for Photoelectric Autocollimator. In Proceedings of the 4th International Conference on Instrumentation and Measurement, Computer, Communication and Control (IMCCC), Harbin Inst Technol, Harbin, China, 18–20 September 2014; pp. 439–442. [Google Scholar] [CrossRef]
- Yang, Z.; Fan, B.; Xi, Q.; Wang, C.; Wang, R. Research on the Method of Building Space Rectangular Coordinate System of Photoelectric Autocollimator. Acta Metrol. Sin. 2018, 39, 12–14. [Google Scholar]
- Liu, Y.; Zhen, Y.; Xie, L.; Wang, W.; Yang, J.; Li, R. Roll angle of autocollimator measurement method based on hollow cube corner reflector. Optoelectron. Lett. 2022, 18, 91–96. [Google Scholar] [CrossRef]
- Konyakhin, I.A.; Turgalieva, T.V. Three-coordinate digital autocollimator. J. Opt. Technol. 2013, 80, 772–777. [Google Scholar] [CrossRef]
- Shur, V.L.; Lukin, A.Y.; Shestopalov, Y.N.; Popov, O.I. Two-coordinate digital autocollimator. Meas. Tech. 2005, 48, 901–906. [Google Scholar] [CrossRef]
- Zou, Y.; Zhang, N.; Yuan, H.; Liu, C.; Liu, D. High precision deflection angle measurement of irregular light spot. Chin. Space Sci. Technol. 2022, 42, 107–113. [Google Scholar] [CrossRef]
- Guo, Y.; Cheng, H.; Liu, G. Three-degree-of-freedom autocollimation angle measurement method based on crosshair displacement and rotation. Rev. Sci. Instrum. 2023, 94, 0126806. [Google Scholar] [CrossRef]
- Yan, L.; Yan, Y.; Chen, B.; Lou, Y. A Differential Phase-Modulated Interferometer with Rotational Error Compensation for Precision Displacement Measurement. Appl. Sci. 2022, 12, 5002. [Google Scholar] [CrossRef]
- Cai, Y.; Yang, B.; Fan, K.-C. Robust roll angular error measurement system for precision machines. Opt. Express 2019, 27, 008027. [Google Scholar] [CrossRef]
- Ren, W.; Cui, J.; Tan, J. Precision roll angle measurement system based on autocollimation. Appl. Opt. 2022, 61, 3811–3818. [Google Scholar] [CrossRef] [PubMed]
- Schwenke, H.; Neuschaefer-Rube, U.; Pfeifer, T.; Kunzmann, H. Optical methods for dimensional metrology in production engineering. CIRP Ann.-Manuf. Technol. 2002, 51, 685–699. [Google Scholar] [CrossRef]
- Zhang, C.; Liu, W.; Duan, F.; Fu, X.; Li, X.; Yan, M. A novel method for reducing the defocus error of a five-degree-of-freedom measurement system. Proc. SPIE 2022, 12282, 1228209. [Google Scholar] [CrossRef]
- Qibo, F.; Bin, Z.; Cunxing, C.; Cuifang, K.; Yusheng, Z.; Fenglin, Y. Development of a simple system for simultaneously measuring 6DOF geometric motion errors of a linear guide. Opt. Express 2013, 21, 25805–25819. [Google Scholar] [CrossRef] [PubMed]
- Cui, C.; Feng, Q.; Zhang, B.; Zhao, Y. System for simultaneously measuring 6DOF geometric motion errors using a polarization maintaining fiber-coupled dual-frequency laser. Opt. Express 2016, 24, 6735–6748. [Google Scholar] [CrossRef] [PubMed]
- Cai, Y.; Lou, Z.; Ling, S.; Liao, B.-s.; Fan, K.-c. Development of a Compact Three-Degree-of-Freedom Laser Measurement System with Self-Wavelength Correction for Displacement Feedback of a Nanopositioning Stage. Appl. Sci. 2018, 8, 2209. [Google Scholar] [CrossRef]
- Wenzheng, L.; Cong, Z.; Fajie, D.; Xiao, F.; Ruijia, B.; Xiuming, L.; Ming, Y. A high-precision calibration method of PSD in long-distance laser auto-collimation system. Proc. SPIE 2022, 12282, 122820T. [Google Scholar] [CrossRef]
- Li, B.; Dong, W.; Xiao, A.; Yu, M.; Ge, W.; He, J. High-precision non-contact two-dimensional dynamic angle measurement system based on PSD. Laser Infrared 2021, 51, 787–796. [Google Scholar]
- Du, M.-x.; Yan, Y.-f.; Zhang, R.; Cai, C.-l.; Yu, X.; Bai, S.-p.; Yu, Y. 3D position angle measurement based on a lens array. Chin. Opt. 2022, 15, 45–55. [Google Scholar] [CrossRef]
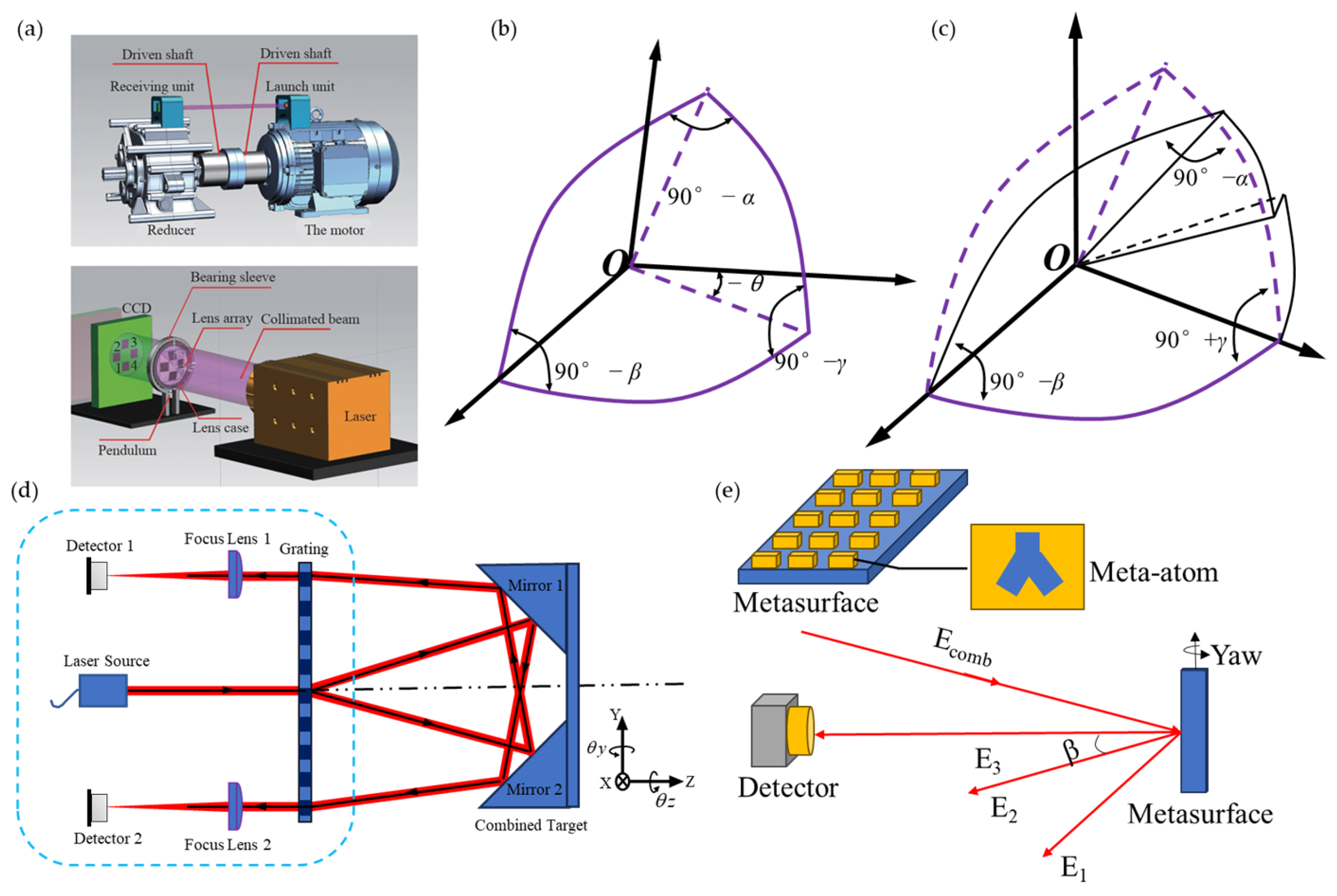
- Li, R.; Zhen, Y.; Di, K.; Wang, W.; Nikitin, M.; Tong, M.H.; Zhang, Y.; Zou, X.; Konyakhin, I. Three-degree-of-freedom autocollimator with large angle-measurement range. Meas. Sci. Technol. 2021, 32, 115005. [Google Scholar] [CrossRef]
- Gu, W.; Zhang, X.; Liu, J.; Zheng, J.; Huang, H.; Jia, L.; Qu, X.; Zhang, F. Optical Angle Barcodes Enabled by a Pixelated Metasurface for Absolute Micro-Angle Measurement. Laser Photonics Rev. 2023, 18, 2300831. [Google Scholar] [CrossRef]
- Cai, C.; Yan, Y.; Zhang, L.; Du, M.; Yu, X. A Lens-Array-Based Measurement Technique for Spatial Angle. IEEE Access 2022, 10, 8112–8120. [Google Scholar] [CrossRef]
- Barboza, R.; Babazadeh, A.; Marrucci, L.; Cardano, F.; de Lisio, C.; D’Ambrosio, V. Ultra-sensitive measurement of transverse displacements with linear photonic gears. Nat. Commun. 2022, 13, 1080. [Google Scholar] [CrossRef] [PubMed]
- Yuan, G.H.; Zheludev, N.I. Detecting nanometric displacements with optical ruler metrology. Science 2019, 364, 771. [Google Scholar] [CrossRef] [PubMed]
- Zang, H.F.; Xi, Z.; Zhang, Z.Y.; Lu, Y.H.; Wang, P. Ultrasensitive and long-range transverse displacement metrology with polarization-encoded metasurface. Sci. Adv. 2022, 8, eadd1973. [Google Scholar] [CrossRef] [PubMed]
- Li, R.; Xiao, H.; Xie, L.; Feng, T.; Ma, Y.; Guo, J.; Zhou, M.; Nikitan, M.; Konyakhin, I. Autocollimation angle-measurement method with a large range based on spot deformation. Opt. Express 2022, 30, 38727–38744. [Google Scholar] [CrossRef]
- Geckeler, R.D.; Schumann, M.; Just, A.; Krause, M.; Lassila, A.; Heikkinen, V. A comparison of traceable spatial angle autocollimator calibrations performed by PTB and VTT MIKES. Metrologia 2022, 59, 024002. [Google Scholar] [CrossRef]
- Kranz, O.; Geckeler, R.D.; Just, A.; Krause, M.; Osten, W. From plane to spatial angles: PTB’s spatial angle autocollimator calibrator. Adv. Opt. Technol. 2015, 4, 397–411. [Google Scholar] [CrossRef]
- Watanabe, T.; Fujimoto, H.; Masuda, T. Self-calibratable rotary encoder. J. Phys. Conf. Ser. 2005, 13, 240–245. [Google Scholar] [CrossRef]
- Stone, J.A.; Amer, M.; Faust, B.; Zimmerman, J. Angle metrology using AAMACS and two small-angle measurement systems. Dev. Traceable Dimens. Meas. II 2003, 5190, 146–155. [Google Scholar] [CrossRef]
- Burnashev, M.N.; Pavlov, P.A.; Filatov, Y.V. Development of precision laser goniometer systems. Quantum Electron. 2013, 43, 130–138. [Google Scholar] [CrossRef]
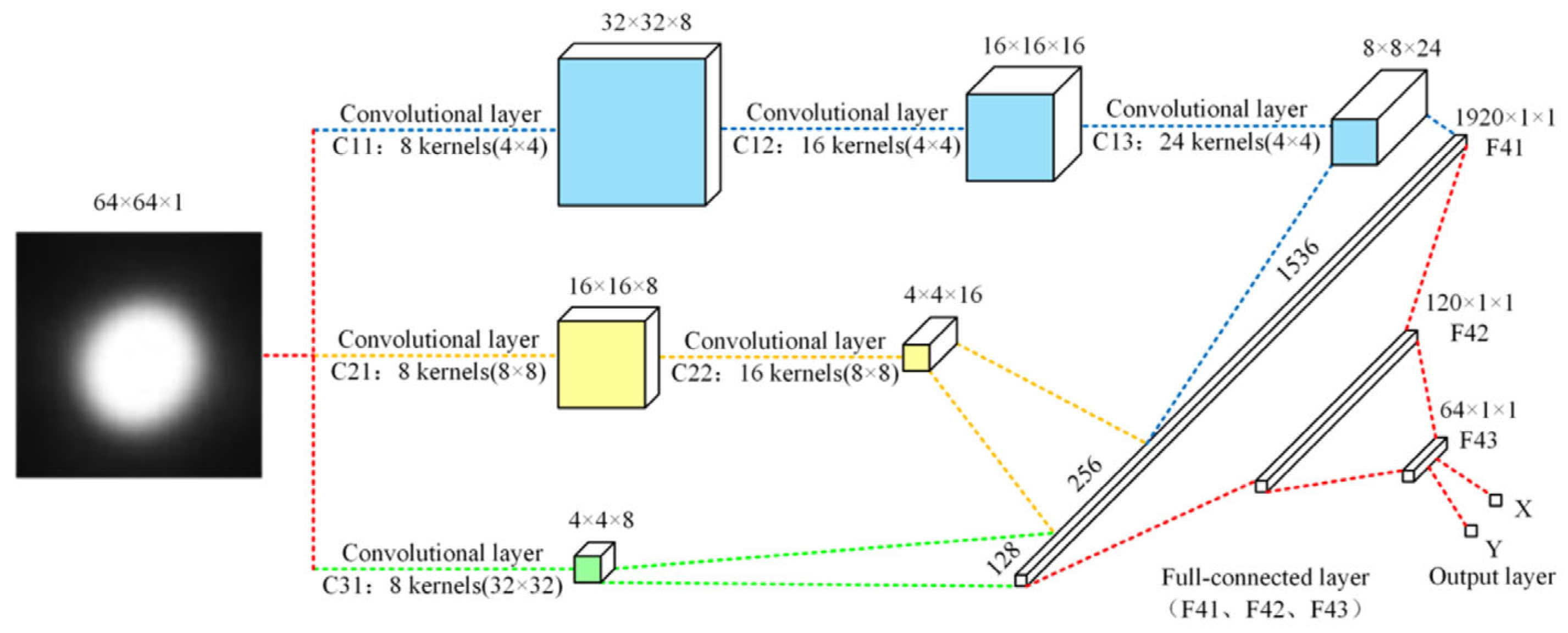
- Shi, J.; Li, Y.C.; Tao, Z.X.; Zhang, D.X.; Xing, H.Y.; Tan, J.B. High-precision autocollimation method based on a multiscale convolution neural network for angle measurement. Opt. Express 2022, 30, 29821–29832. [Google Scholar] [CrossRef] [PubMed]




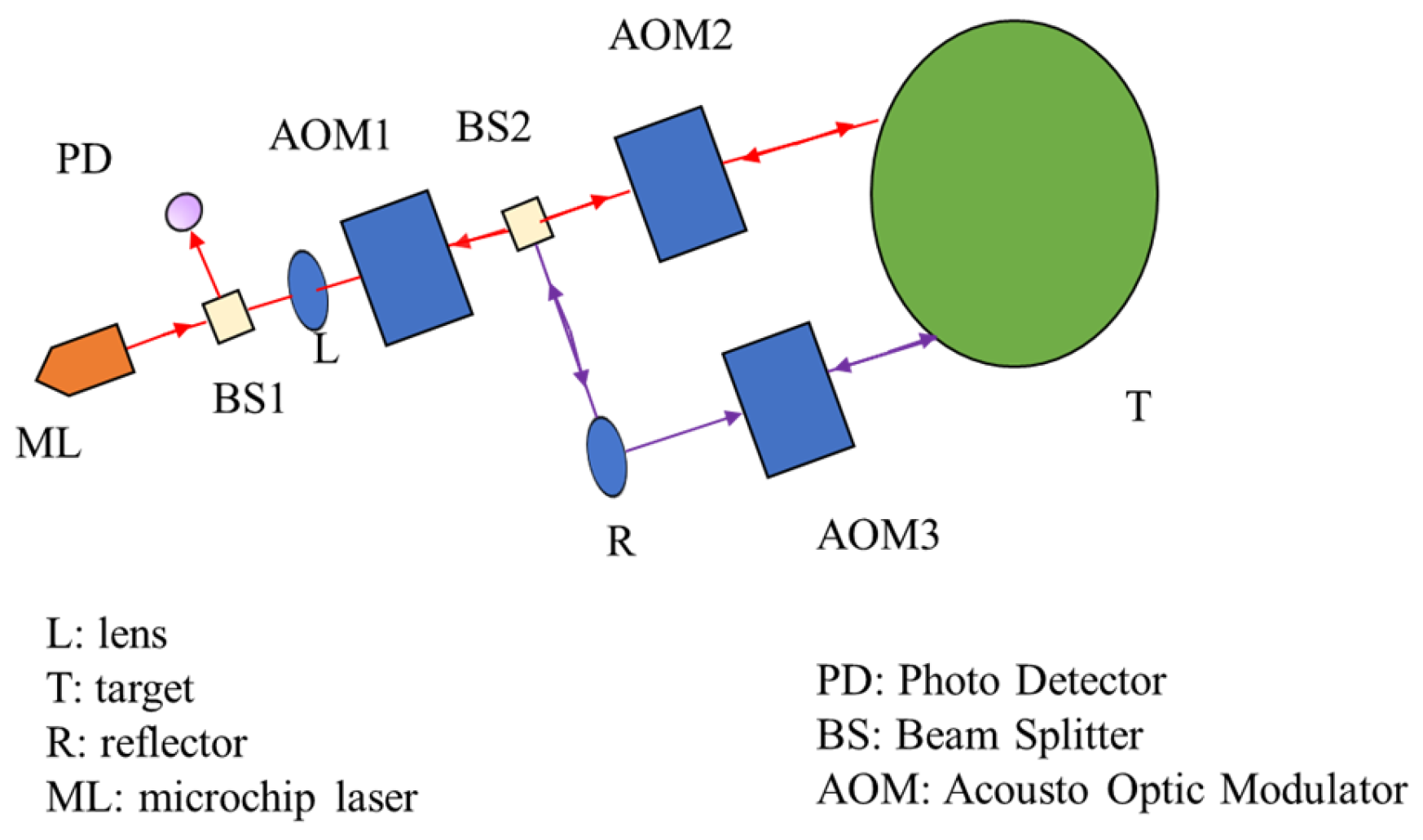


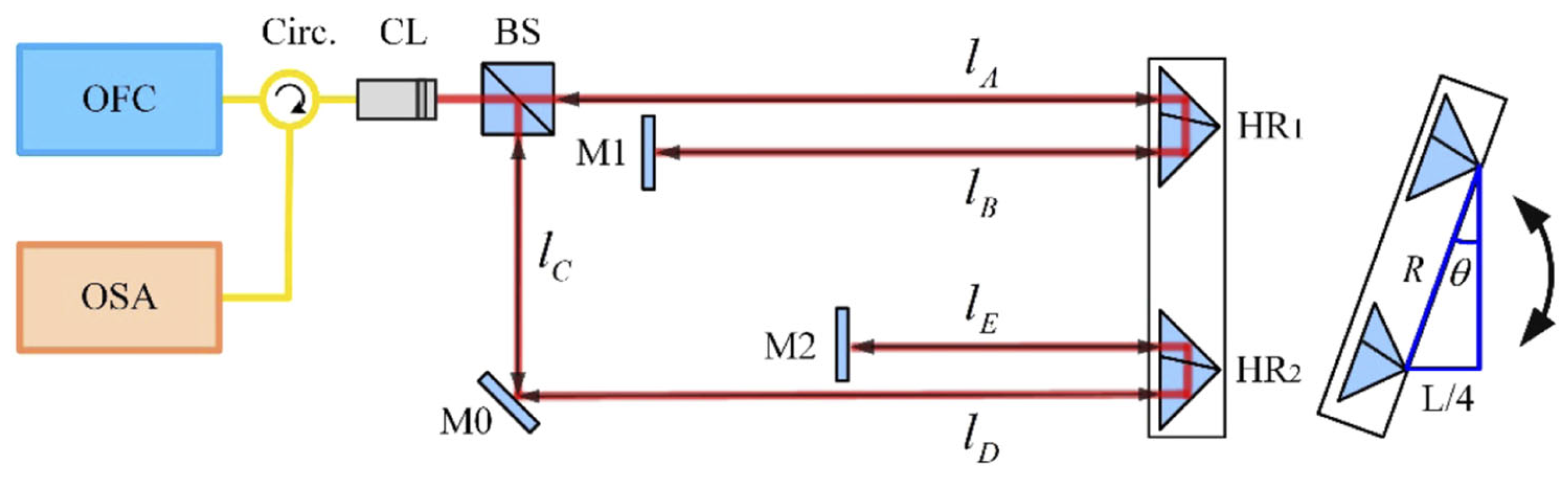


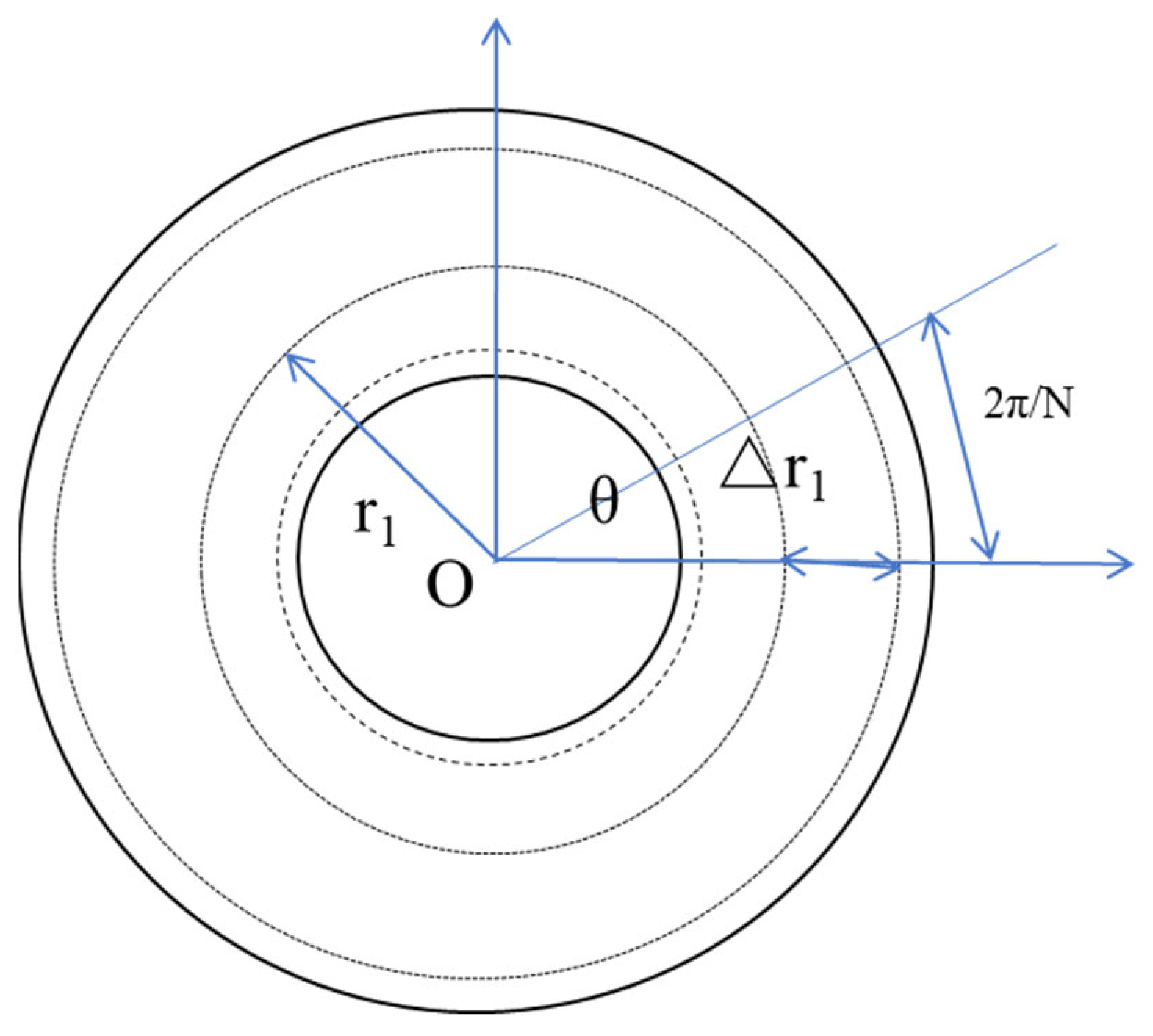
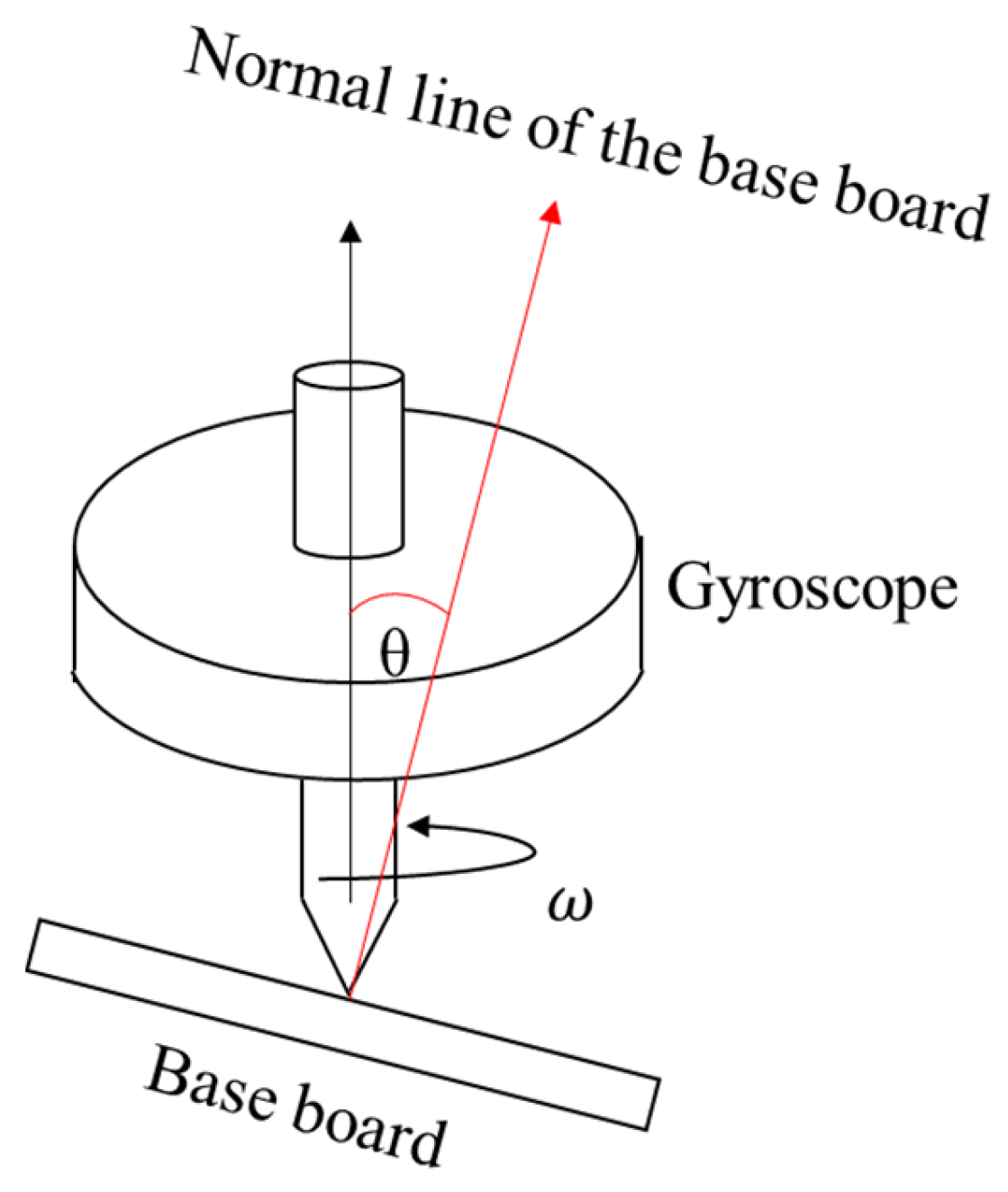








| Parameters | Measurement Range | Resolution/Arcsec | Cost |
|---|---|---|---|
| Dispersive Characteristics of Grating [173] | >6° | 0.03 | middle |
| Absolute Distance Measurement [130] | >300 arcsec | 0.073 | Middle~high |
| The other OFC method [204] | >3.3° | 0.23 | Middle~high |
| Parameters | Measurement Range | Resolution/Arcsec | Accuracy/Arcsec | Cost |
|---|---|---|---|---|
| Optical internal reflection | 3 arcmin/−5.6–5° | 0.02/0.288 | 0.02 | low |
| Autocollimator | <30° | 0.001 [227] | 0.004 [227] | Middle~high |
| Visual method | 5760 arcsec | 1 | 3.6 | middle |
| Phase method | Sub-degree | 0.0007 [116]. | 0.695 [116]. | high |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Wang, S.; Ma, R.; Cao, F.; Luo, L.; Li, X. A Review: High-Precision Angle Measurement Technologies. Sensors 2024, 24, 1755. https://doi.org/10.3390/s24061755
Wang S, Ma R, Cao F, Luo L, Li X. A Review: High-Precision Angle Measurement Technologies. Sensors. 2024; 24(6):1755. https://doi.org/10.3390/s24061755
Chicago/Turabian StyleWang, Shengtong, Rui Ma, Feifan Cao, Linbin Luo, and Xinghui Li. 2024. "A Review: High-Precision Angle Measurement Technologies" Sensors 24, no. 6: 1755. https://doi.org/10.3390/s24061755
APA StyleWang, S., Ma, R., Cao, F., Luo, L., & Li, X. (2024). A Review: High-Precision Angle Measurement Technologies. Sensors, 24(6), 1755. https://doi.org/10.3390/s24061755








