Analytical Analysis of Factors Affecting the Accuracy of a Dual-Heat Flux Core Body Temperature Sensor
Abstract
:1. Introduction
2. Materials and Methods
2.1. CBT Measurement Uncertainty Analysis
2.1.1. Measurement Setup
2.1.2. Monte Carlo Analysis
2.1.3. First-Order Tylor Series Expansion
2.2. Tools
3. Results
3.1. Experimental Setup Validation
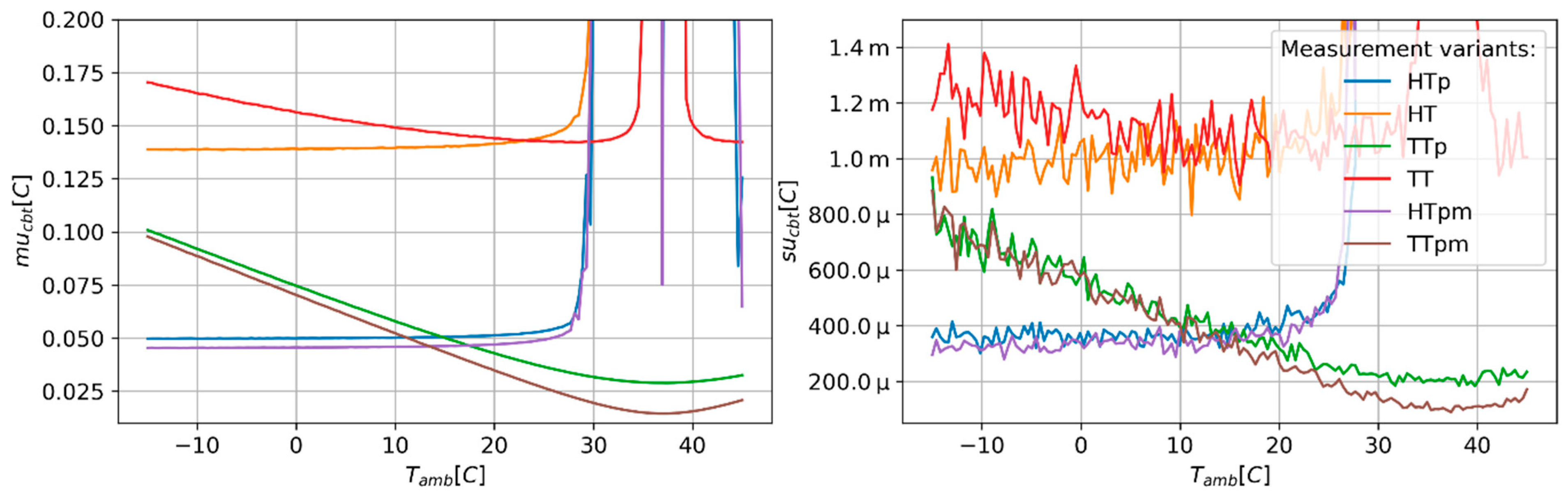
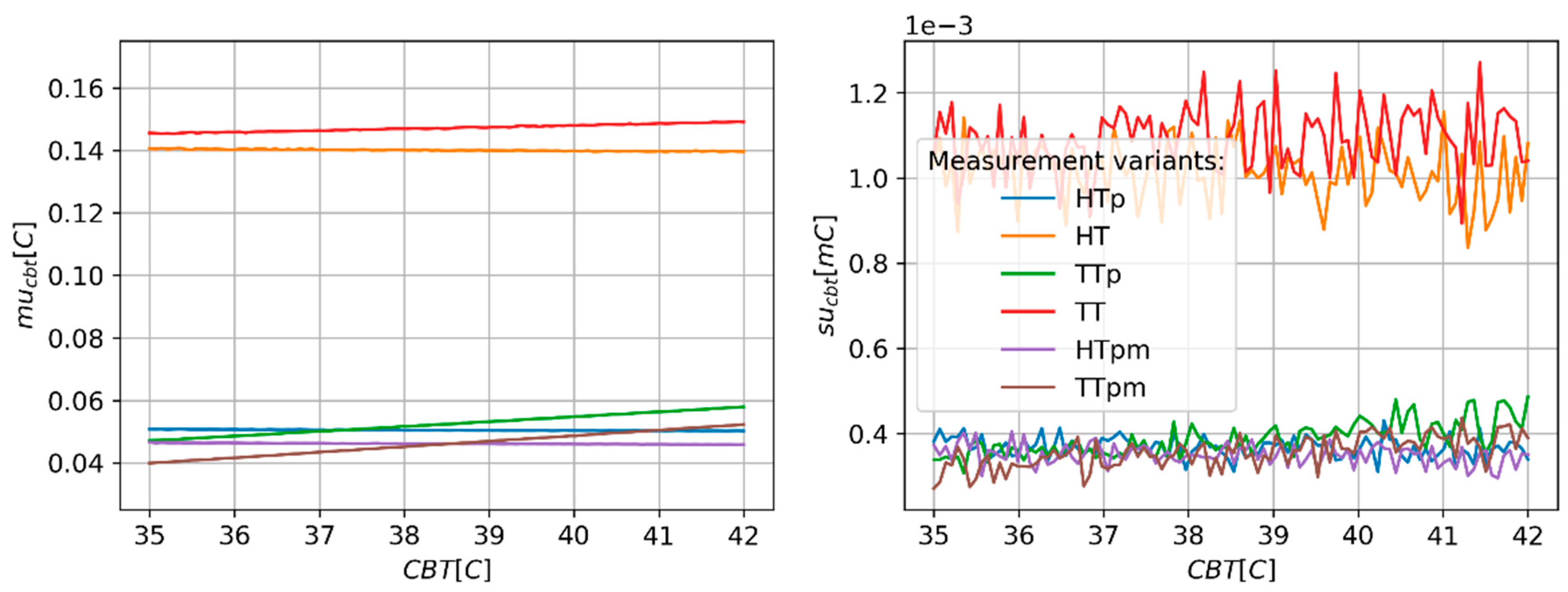
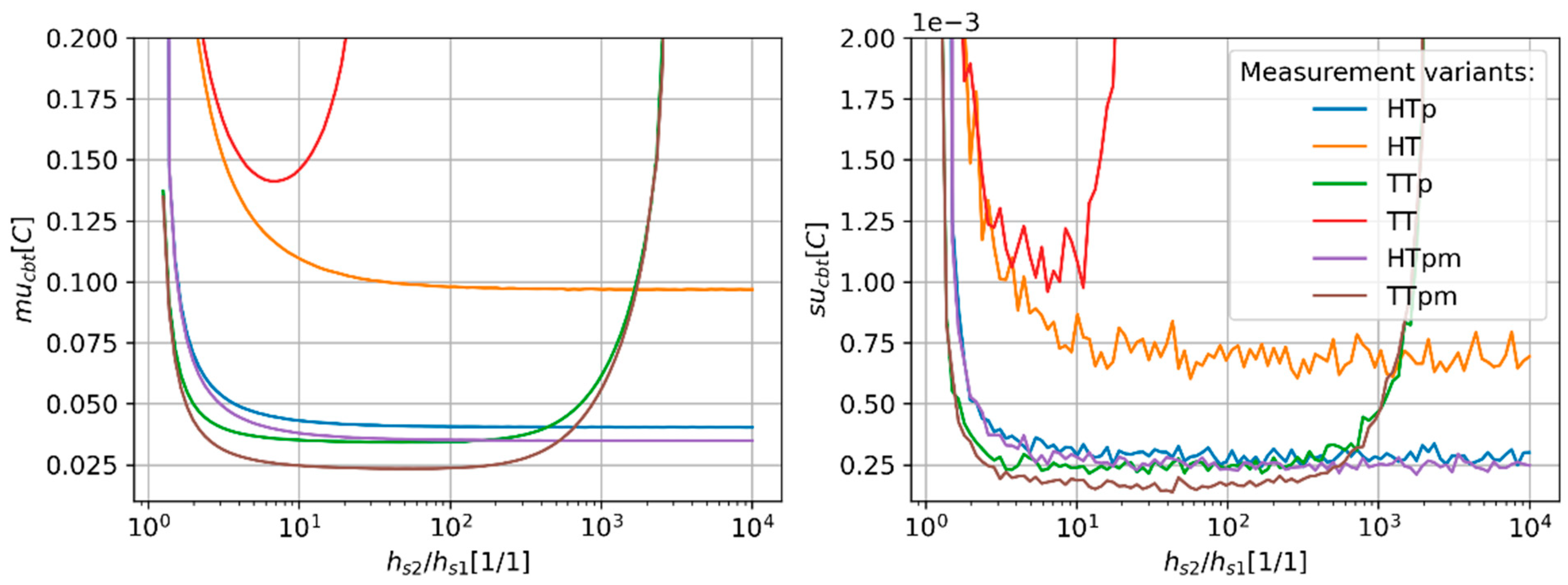
3.2. Monte Carlo Simulations
3.3. Linearization
4. Discussion
5. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
Abbreviations
| CBT | Core body temperature |
| CBTe | Estimated core body temperature |
| CBTeHT | Estimated core body temperature using sensor in HT configuration |
| CBTeHTp | Estimated core body temperature using sensor in HT configuration with paired temperature sensors |
| CBTeHTpm | Estimated core body temperature for HT configuration with paired temperature sensors with averaged value |
| CBTeTT | Estimated core body temperature using sensor in TT configuration |
| CBTeTTp | Estimated core body temperature using sensor in TT configuration with paired temperature sensors |
| CBTeTTpm | Estimated core body temperature for TT configuration with paired temperature sensors with averaged value |
| ds, ds1, ds2 | The thickness of the PDMS layer, and the thickness of the PDMS layer in sensors 1 and 2, respectively |
| has, has1, has2, | The heat transfer coefficients of the interference between the sensor and air, and the heat transfer coefficient between air and sensors 1 and 2, respectively |
| hs, hs1, hs2 | The heat transfer coefficient of the sensor, and the heat transfer coefficient of sensors 1 and 2, respectively |
| HT | The CBT sensor configuration, comprising combinations of flux sensor and temperature sensor |
| ht | Skin heat transfer coefficient |
| HTp | CBT sensor with heat flux sensors and paired temperature sensors |
| HTpm | CBT sensor with heat flux sensors and paired temperature sensors. The value of the final estimate is the average value of estimates derived from measurements using Equations (8) and (13). |
| k | The ratio of the heat transfer coefficient of the DHF sensor equals hs2/hs1 |
| ks, ks1, ks2 | Thermal conductivity of sensor, and thermal conductivity of sensors 1 and 2, respectively |
| kt | Thermal conductivity of skin |
| MC | Monte Carlo method |
| mucbt | Measurement uncertainty |
| PSI | Physiological Strain Index |
| q, q1, q2 | Heat flux, and heat flux flowing through sensors 1 and 2, respectively |
| SBT | Surface Body Temperature |
| sucbt | Standard deviations of mucbt |
| Tamb | Ambient (air) temperature |
| Tsa, Tsa1, Tsa2 | The temperature of the air-sensor surface, and the temperature measured between air and sensors 1 and 2, respectively |
| Tss, Tss1, Tss2 | The temperature of the skin-sensor surface, and the temperature measured between the skin and sensors 1 and 2, respectively |
| TT | The CBT sensor configuration comprising four temperature sensors |
| TTp | CBT sensor comprising four temperature sensors with temperature sensors paired |
| TTpm | CBT sensor comprising four temperature sensors with temperature sensors paired. The value of the final estimate is the averaged value of estimates derived from measurements using Equations (9), (14), (15) and (16). |
| uCBTeHT | combined uncertainty for the dual-flux sensor in the HT variant with non-paired temperature sensor configuration |
| uh | The standard uncertainty of the measurement of the heat transfer coefficients hs1 and hs2 |
| uq | The standard uncertainty of heat-flux measurement |
| uT | The standard uncertainty temperature measurement using a non-paired sensor |
| uΔT | The standard uncertainty of the differential temperature measurement using paired sensors |
References
- Dolson, C.M.; Harlow, E.R.; Phelan, D.M.; Gabbett, T.J.; Gaal, B.; McMellen, C.; Geletka, B.J.; Calcei, J.G.; Voos, J.E.; Seshadri, D.R. Wearable Sensor Technology to Predict Core Body Temperature: A Systematic Review. Sensors 2022, 22, 7639. [Google Scholar] [CrossRef]
- Moran, D.S.; Shitzer, A.; Pandolf, K.B. A Physiological Strain Index to Evaluate Heat Stress. Am. J. Physiol. Regul. Integr. Comp. Physiol. 1998, 275, R129–R134. [Google Scholar] [CrossRef]
- Moran, D.S.; Montain, S.J.; Pandolf, K.B. Evaluation of Different Levels of Hydration Using a New Physiological Strain Index. Am. J. Physiol. Regul. Integr. Comp. Physiol. 1998, 275, R854–R860. [Google Scholar] [CrossRef] [PubMed]
- Buller, M.J.; Welles, A.P.; Friedl, K.E. Wearable Physiological Monitoring for Human Thermal-Work Strain Optimization. J. Appl. Physiol. 2017, 124, 432–441. [Google Scholar] [CrossRef] [PubMed]
- Huggins, R.; Glaviano, N.; Negishi, N.; Casa, D.J.; Hertel, J. Comparison of Rectal and Aural Core Body Temperature Thermometry in Hyperthermic, Exercising Individuals: A Meta-Analysis. J. Athl. Train. 2012, 47, 329–338. [Google Scholar] [CrossRef] [PubMed]
- Coiffard, B.; Diallo, A.B.; Culver, A.; Antonini, F.; Hammad, E.; Leone, M.; Mege, J.-L. Exacerbation of Circadian Rhythms of Core Body Temperature and Sepsis in Trauma Patients. J. Crit. Care 2020, 60, 23–26. [Google Scholar] [CrossRef] [PubMed]
- Baron, K.G.; Reid, K.J. Circadian Misalignment and Health. Int. Rev. Psychiatry 2014, 26, 139–154. [Google Scholar] [CrossRef]
- Videnovic, A.; Willis, G.L. Circadian System—A Novel Diagnostic and Therapeutic Target in Parkinson’s Disease? Mov. Disord. 2016, 31, 260–269. [Google Scholar] [CrossRef]
- Taillard, J.; Sagaspe, P.; Philip, P.; Bioulac, S. Sleep Timing, Chronotype and Social Jetlag: Impact on Cognitive Abilities and Psychiatric Disorders. Biochem. Pharmacol. 2021, 191, 114438. [Google Scholar] [CrossRef]
- Molzof, H.E.; Prapanjaroensin, A.; Patel, V.H.; Mokashi, M.V.; Gamble, K.L.; Patrician, P.A. Misaligned Core Body Temperature Rhythms Impact Cognitive Performance of Hospital Shift Work Nurses. Neurobiol. Learn. Mem. 2019, 160, 151–159. [Google Scholar] [CrossRef]
- Crouse, J.J.; Carpenter, J.S.; Song, Y.J.C.; Hockey, S.J.; Naismith, S.L.; Grunstein, R.R.; Scott, E.M.; Merikangas, K.R.; Scott, J.; Hickie, I.B. Circadian Rhythm Sleep–Wake Disturbances and Depression in Young People: Implications for Prevention and Early Intervention. Lancet Psychiatry 2021, 8, 813–823. [Google Scholar] [CrossRef]
- Vinales, K.L.; Begaye, B.; Thearle, M.S.; Krakoff, J.; Piaggi, P. Core Body Temperature, Energy Expenditure, and Epinephrine during Fasting, Eucaloric Feeding, and Overfeeding in Healthy Adult Men: Evidence for a Ceiling Effect for Human Thermogenic Response to Diet. Metabolism 2019, 94, 59–68. [Google Scholar] [CrossRef] [PubMed]
- Webster, W.W.; Smarr, B. Using Circadian Rhythm Patterns of Continuous Core Body Temperature to Improve Fertility and Pregnancy Planning. J. Circadian Rhythm. 2020, 18, 5. [Google Scholar] [CrossRef]
- Wang, X.-J.; Li, X.-T.; Chen, N.; Huang, L.; Huang, S.-X.; Chen, T.-T.; Liu, G.-H.; Hu, R.-F. Mental Health, Sleep Quality, and Hormonal Circadian Rhythms in Pregnant Women with Threatened Preterm Labor: A Prospective Observational Study. BMC Pregnancy Childbirth 2023, 23, 501. [Google Scholar] [CrossRef]
- Sund-Levander, M.; Grodzinsky, E. Time for a Change to Assess and Evaluate Body Temperature in Clinical Practice. Int. J. Nurs. Pract. 2009, 15, 241–249. [Google Scholar] [CrossRef] [PubMed]
- Chen, W. Thermometry and Interpretation of Body Temperature. Biomed. Eng. Lett. 2019, 9, 3–17. [Google Scholar] [CrossRef]
- Huang, M.; Tamura, T.; Tang, Z.; Chen, W.; Kanaya, S. A Wearable Thermometry for Core Body Temperature Measurement and Its Experimental Verification. IEEE J. Biomed. Health Inform. 2017, 21, 708–714. [Google Scholar] [CrossRef]
- Niedermann, R.; Wyss, E.; Annaheim, S.; Psikuta, A.; Davey, S.; Rossi, R.M. Prediction of Human Core Body Temperature Using Non-Invasive Measurement Methods. Int. J. Biometeorol. 2014, 58, 7–15. [Google Scholar] [CrossRef] [PubMed]
- Tamura, T.; Huang, M.; Togawa, T. Current Developments in Wearable Thermometers. Adv. Biomed. Eng. 2018, 7, 88–99. [Google Scholar] [CrossRef]
- Uth, M.-F.; Koch, J.; Sattler, F. Body Core Temperature Sensing: Challenges and New Sensor Technologies. Procedia Eng. 2016, 168, 89–92. [Google Scholar] [CrossRef]
- Fang, J.; Zhou, C.; Ye, X. Optimization of a Wearable Device for Core Body Temperature Monitoring Based on the Dual-Heat-Flux Model. IOP Conf. Ser. Mater. Sci. Eng. 2019, 677, 032006. [Google Scholar] [CrossRef]
- Huang, M.; Tamura, T.; Chen, W.; Kanaya, S. Evaluation of Structural and Thermophysical Effects on the Measurement Accuracy of Deep Body Thermometers Based on Dual-Heat-Flux Method. J. Therm. Biol. 2015, 47, 26–31. [Google Scholar] [CrossRef]
- Xu, X.; Wu, G.; Lian, Z.; Xu, H. Feasibility Analysis of Applying Non-Invasive Core Body Temperature Measurement in Sleep Research. Energy Build. 2024, 303, 113827. [Google Scholar] [CrossRef]
- Panunzio, N.; Diamanti, A.; Marrocco, G. Flexible Multi-Layer Sensor for the Wireless Implementation of Dual-Heat-Flux Monitoring of Body Temperature. In Proceedings of the 2022 IEEE International Conference on Flexible and Printable Sensors and Systems (FLEPS), Vienna, Austria, 10–13 July 2022; pp. 1–4. [Google Scholar]
- Zhang, Y.; Chad Webb, R.; Luo, H.; Xue, Y.; Kurniawan, J.; Cho, N.H.; Krishnan, S.; Li, Y.; Huang, Y.; Rogers, J.A. Theoretical and Experimental Studies of Epidermal Heat Flux Sensors for Measurements of Core Body Temperature. Adv. Healthc. Mater. 2016, 5, 119–127. [Google Scholar] [CrossRef]
- Matsunaga, D.; Tanaka, Y.; Tajima, T.; Seyama, M. Technology for Visualizing the Circadian Rhythm: Wearable Core-Body-Temperature Sensor. NTT Tech. Rev. 2021, 19, 34–39. [Google Scholar] [CrossRef]
- Tamura, T.; Huang, M.; Yoshimura, T.; Umezu, S.; Ogata, T. An Advanced Internet of Things System for Heatstroke Prevention with a Noninvasive Dual-Heat-Flux Thermometer. Sensors 2022, 22, 9985. [Google Scholar] [CrossRef]
- Janke, D.; Kagelmann, N.; Storm, C.; Maggioni, M.A.; Kienast, C.; Gunga, H.-C.; Opatz, O. Measuring Core Body Temperature Using a Non-Invasive, Disposable Double-Sensor During Targeted Temperature Management in Post-Cardiac Arrest Patients. Front. Med. 2021, 8, 666908. [Google Scholar] [CrossRef]
- Lauronen, S.-L.; Kalliomäki, M.-L.; Kalliovalkama, J.; Aho, A.; Huhtala, H.; Yli-Hankala, A.M.; Mäkinen, M.-T. Comparison of Zero Heat Flux and Double Sensor Thermometers during Spinal Anaesthesia: A Prospective Observational Study. J. Clin. Monit. Comput. 2022, 36, 1547–1555. [Google Scholar] [CrossRef] [PubMed]
- Daanen, H.A.M.; Kohlen, V.; Teunissen, L.P.J. Heat Flux Systems for Body Core Temperature Assessment during Exercise. J. Therm. Biol. 2023, 112, 103480. [Google Scholar] [CrossRef] [PubMed]
- Etienne, S.; Oliveras, R.; Schiboni, G.; Durrer, L.; Rochat, F.; Eib, P.; Zahner, M.; Osthoff, M.; Bassetti, S.; Eckstein, J. Free-Living Core Body Temperature Monitoring Using a Wrist-Worn Sensor after COVID-19 Booster Vaccination: A Pilot Study. BioMedical Eng. OnLine 2023, 22, 25. [Google Scholar] [CrossRef]
- Smits, E.C.P.; van Heck, G.T.; Kingma, B.R.M.; van den Brand, J. Core Body Temperature Sensor and Method for the Manufacturing Thereof. US20220128413A1, 28 April 2022. [Google Scholar]
- Saggin, B.; Tarabini, M.; Lanfranchi, G. A Device for the Skin–Contact Thermal Resistance Measurement. IEEE Trans. Instrum. Meas. 2012, 61, 489–495. [Google Scholar] [CrossRef]
- Gunga, H.-C.; Sandsund, M.; Reinertsen, R.E.; Sattler, F.; Koch, J. A Non-Invasive Device to Continuously Determine Heat Strain in Humans. J. Therm. Biol. 2008, 33, 297–307. [Google Scholar] [CrossRef]
- Matsunaga, D.; Tanaka, Y.; Seyama, M.; Nagashima, K. Non-Invasive and Wearable Thermometer for Continuous Monitoring of Core Body Temperature under Various Convective Conditions. In Proceedings of the 2020 42nd Annual International Conference of the IEEE Engineering in Medicine Biology Society (EMBC), Montreal, QC, Canada, 27 August 2020; pp. 4377–4380. [Google Scholar]
- Tanaka, Y.; Matsunaga, D.; Tajima, T.; Seyama, M. Skin-Attachable Sensor for Core Body Temperature Monitoring. IEEE Sens. J. 2021, 21, 16118–16123. [Google Scholar] [CrossRef]
- de Rivera, P.J.R.; de Rivera, M.R.; Socorro, F.; de Rivera, M.R. Measurement of Human Body Surface Heat Flux Using a Calorimetric Sensor. J. Therm. Biol. 2019, 81, 178–184. [Google Scholar] [CrossRef] [PubMed]
- Mark, J.E. (Ed.) Polymer Data Handbook; Oxford University Press: Oxford, UK, 1999. [Google Scholar]
- Hasgall, P.; Di Gennaro, F.; Baumgartner, C.; Neufeld, E.; Lloyd, B.; Gosselin, M.; Payne, D.; Klingenböck, A.; Kuster, N. IT’IS Database for Thermal and Electromagnetic Parameters of Biological Tissues. 2022. Available online: https://itis.swiss/virtual-population/tissue-properties/overview/ (accessed on 12 March 2024).
- Arens, E.A.; Zhang, H. The Skin’s Role in Human Thermoregulation and Comfort. In Thermal and Moisture Transport in Fibrous Materials; Woodhead Publishing Ltd.: Sawston, UK, 2006; pp. 560–602. [Google Scholar]
- Lienhard, J.H. A Heat Transfer Textbook; Phlogiston Press: Cambridge, MA, USA, 2020. [Google Scholar]
- Natural Convection Coefficient Calculator. QuickField FEA Software. Available online: https://quickfield.com/natural_convection.htm (accessed on 25 March 2022).
- THB. New Transient Hot Bridge Instrument. LINSEIS. Available online: https://www.linseis.com/en/products/thermal-conductivity-instruments/thb-basic-advance-ultimate/ (accessed on 25 March 2022).
- Convection Wizard. Available online: https://thermal.mayahtt.com/tmwiz/convect/forced/fp-isot/fp-isot.htm (accessed on 31 March 2022).
- Taylor, N.A.S.; Tipton, M.J.; Kenny, G.P. Considerations for the Measurement of Core, Skin and Mean Body Temperatures. J. Therm. Biol. 2014, 46, 72–101. [Google Scholar] [CrossRef] [PubMed]
- E1112-00; Standard Specification for Electronic Thermometer for Intermittent Determination of Patient Temperature. ASTM International: West Conshohocken, PA, USA, 2011.
- Huang, M.; Kanaya, S.; Tamura, T.; Tang, Z.; Chen, W. Structural Optimization of a Wearable Deep Body Thermometer: From Theoretical Simulation to Experimental Verification. J. Sens. 2016, 2016, 4828093. [Google Scholar] [CrossRef]
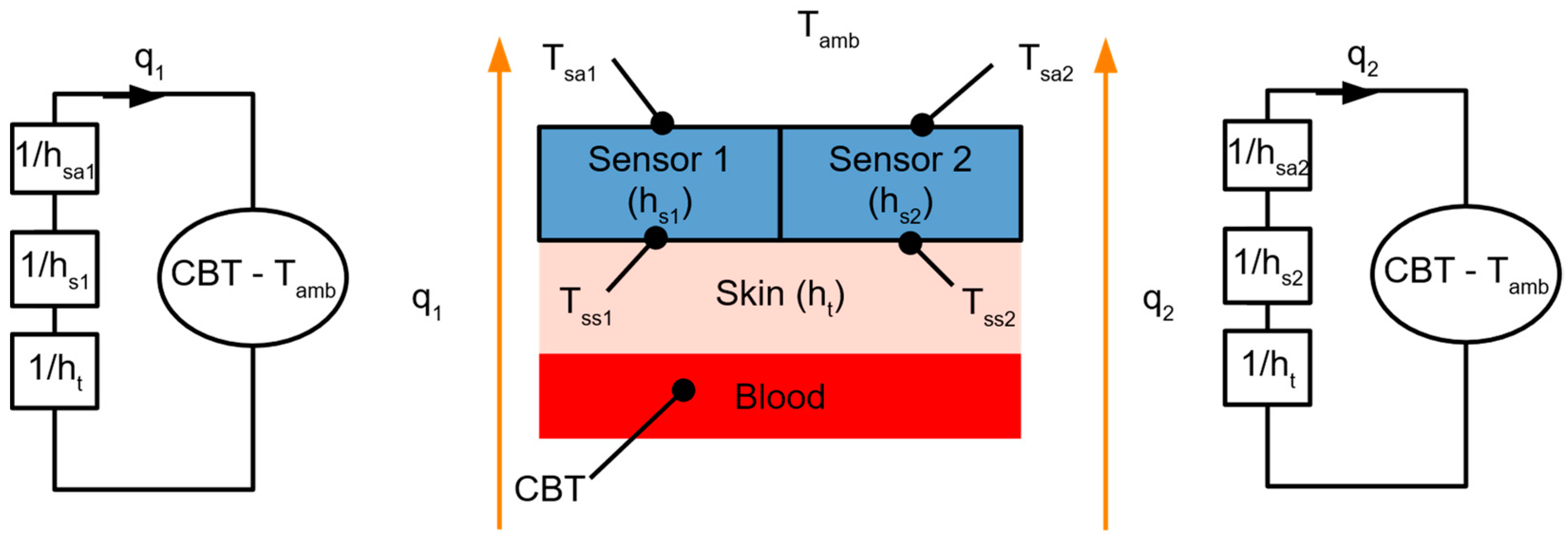




| Method * | Designation of Independent Measurement Uncertainties (See Section 2.1.2. Monte Carlo Analysis) | Description |
|---|---|---|
| HT | uT, uq | The temperature sensors are not paired. |
| HTp | uT, uq, uΔT | The temperature sensors are paired. |
| HTpm | uT, uq, uΔT | The temperature sensors are paired. The value of the final estimate is the average value of estimates derived from measurements using Equations (8) and (13). |
| TT | uT | The temperature sensors are not paired. |
| TTp | uT, uΔT | The temperature sensors are paired. |
| TTpm | uT, uΔT, | The temperature sensors are paired. The value of the final estimate is the averaged value of estimates derived from measurements using Equations (9), (14), (15) and (16). |
| Parameter | Unit | Default Value | Description |
|---|---|---|---|
| CBT | [°C] | 37 | Core body temperature. |
| Tamb | [°C] | 25 | Ambient (air) temperature. |
| ks1, ks2 | 0.15 | Thermal conductivity of sensors in channels 1 and 2 as typical material Poly(dimethylsiloxane)(PDMS) was chosen [38]. | |
| ds1, ds2 | [mm] | 15, 30 | The thickness of the PDMS layer of sensors in channels 1 and 2, respectively. |
| kt | 0.37 | Thermal conductivity of the skin [39] | |
| dt | [mm] | 2.5 | Thickness of the skin [40] |
| hsa | 6.1 | Natural convection coefficient at the surface between sensors and air. Its value was estimated for an upward-oriented circular plate with a radius of 1.5 cm [41,42]. The radius of the plate was chosen based on the description of sensors presented in publications [17,21,35,36,37]. | |
| hs1, hs2 | 10, 5 | Heat transfer coefficient of sensors 1 and 2, respectively. These values were calculated according to the general equation h = k/d. |
| Parameter | Unit | Default Value | Description |
|---|---|---|---|
| uT | [°C] | The standard uncertainty of non-paired sensor temperature measurement. The value was estimated based on the datasheet of the LMT70 (Texas Instruments, Dallas, TX, USA) sensor. | |
| uΔT | [°C] | The standard uncertainty of paired sensor differential temperature measurement. The value was estimated based on spectral output noise distribution presented in the datasheet of the LMT70 (Texas Instruments, USA) sensor. | |
| uq | ±1.3 | The standard uncertainty of heat flux measurement. The value was estimated based on the PHFS-01 sensor (Fluxteq, Blacksburg, VA, USA) sensor and AD7713 (Analog Devices, Wilmington, NC, USA) analog-digital converter (ADC) datasheet. | |
| uh | ±0.12 | The standard uncertainty of measurement of the heat transfer coefficients hs1 and hs2. This value was estimated assuming that the standard uncertainty of sensor thickness equals ±0.1 mm and the relative uncertainty of material conductivity measurement equals ±1% [43]. |
| Changed Parameter | Unit | Range of Values | Description |
|---|---|---|---|
| Tamb | [°C] | −15–+45 and 36.95–37.05 | The influence of ambient temperature on CBT uncertainty. This test was restated with a narrowed temperature range for TTp and TTpm measurement variants. |
| CBT | [°C] | 35–45 | The influence of CBT value on CBT uncertainty. |
| hs1, hs2 | 0.7–6667 | The influence of the heat transfer coefficient values of the DHF probe channels on CBT uncertainty. The ratio of the hs1 to hs2 was constant and equal to 2. The range of the hs1 value was selected by changing the thermal conductivity k1 and k2 in the range from 0.01 W/(m·K) to 100 W/(m·K). | |
| hs2/hs1 | [1/1] | 1.25–10,000 | The influence of the ratio between heat transfer coefficients of the DHF probe channels on CBT uncertainty. The hs1 value was constant as hs2 was reduced by increasing the thickness (d2) of the second channel. |
| hsa | 6–104 | The influence of heat transfer coefficient between air and sensor for air flow changing from 0 m/s to 14 m/s on CBT uncertainty. The maximum value of hsa for airflow equal to 14 m/s was estimated using an online [44] calculator assuming that the probe has a size of 2 cm by 2 cm and a temperature equal to 36 °C, and the surrounding air temperature was 25 °C. |
| Measurement Variant | ru [%] | ||||||||||
|---|---|---|---|---|---|---|---|---|---|---|---|
| Tss1 | Tss2 | Tsa1 | Tsa2 | q1 | q2 | ΔTss | ΔTs1 | ΔTs2 | hs1 | hs2 | |
| HT | 31.832 | 59.694 | - | - | 2.947 | 5.527 | - | - | - | - | - |
| HTp | 33.857 | - | - | - | 22.969 | 43.074 | 0.1 | - | - | - | - |
| TT | 35.306 | 62.085 | 0.141 | 0.066 | - | - | - | - | - | 0.48 | 1.921 |
| TTp | 90.048 | - | - | - | - | - | 0.367 | 0.001 | 0.001 | 1.917 | 7.667 |
| Reference | Accuracy [°C] | Device | Type of Experiment |
|---|---|---|---|
| [21] | 0.09 | DHF | Physical model |
| [22] | 0.3 | DHF | Numerical simulation (FEM) |
| [20] | 0.15–0.29 | SHF | Clinical experiment |
| [47] | 0.095–0.019 | Modified SHF | Numerical (FEM) and limited trials with humans |
| [31] | 0.34 | Calera® (greenTEG, Rümlang, Switzerland) | Clinical experiment |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Żmigrodzki, J.; Cygan, S.; Łusakowski, J.; Lamprecht, P. Analytical Analysis of Factors Affecting the Accuracy of a Dual-Heat Flux Core Body Temperature Sensor. Sensors 2024, 24, 1887. https://doi.org/10.3390/s24061887
Żmigrodzki J, Cygan S, Łusakowski J, Lamprecht P. Analytical Analysis of Factors Affecting the Accuracy of a Dual-Heat Flux Core Body Temperature Sensor. Sensors. 2024; 24(6):1887. https://doi.org/10.3390/s24061887
Chicago/Turabian StyleŻmigrodzki, Jakub, Szymon Cygan, Jan Łusakowski, and Patryk Lamprecht. 2024. "Analytical Analysis of Factors Affecting the Accuracy of a Dual-Heat Flux Core Body Temperature Sensor" Sensors 24, no. 6: 1887. https://doi.org/10.3390/s24061887
APA StyleŻmigrodzki, J., Cygan, S., Łusakowski, J., & Lamprecht, P. (2024). Analytical Analysis of Factors Affecting the Accuracy of a Dual-Heat Flux Core Body Temperature Sensor. Sensors, 24(6), 1887. https://doi.org/10.3390/s24061887






