Study on the Improvement of Droplet Penetration Effect by Nozzle Tilt Angle under the Influence of Orthogonal Side Wind
Abstract
:1. Introduction
2. Materials and Methods
2.1. Test Materials
2.2. Experimental Design and Methods
2.2.1. Determination of Test Parameters
2.2.2. Spray Test Design
- As illustrated in Figure 7C, the canopy is divided into three equal layers from front to back along the spray direction. On each side of the layer, three horizontal beams support fine lines threaded through the canopy in a straight line, with nine measurement points (Ai, Bi, Ci, …, Ii) evenly arranged on the plane formed by the three lines. Water-sensitive papers are attached at each point with paper clips positioned perpendicular to the spray direction.
- In order to prevent obstruction between front and back water-sensitive papers, slight adjustments are made to their positions. If foliage obstructs the lines, they are adjusted to ensure a straight path through the canopy, maintaining all three lines in the same vertical plane.
- At each wind speed and nozzle inclination angle, a one-second spray operation is conducted. After the water-sensitive papers dry, they are collected in sequence into a storage box and labeled according to the order of the experiment. Each experimental condition is repeated three times, with results averaged.
- Wind speed and nozzle inclination angles are varied, repeating step 3 until droplet sampling for all conditions is completed.
- DepositScan software is used to scan and analyze the deposition on water-sensitive papers.
- The average of the parameters from all sampling points on the same layer represents the average parameter on that vertical cross-section of the canopy. Equations (1) and (3) are used to calculate the droplet penetration ratio for each layer.
2.2.3. Field Trial Design
3. Results
3.1. Spray Test Results and Analysis
3.1.1. Distribution of Droplet Penetration Deposits
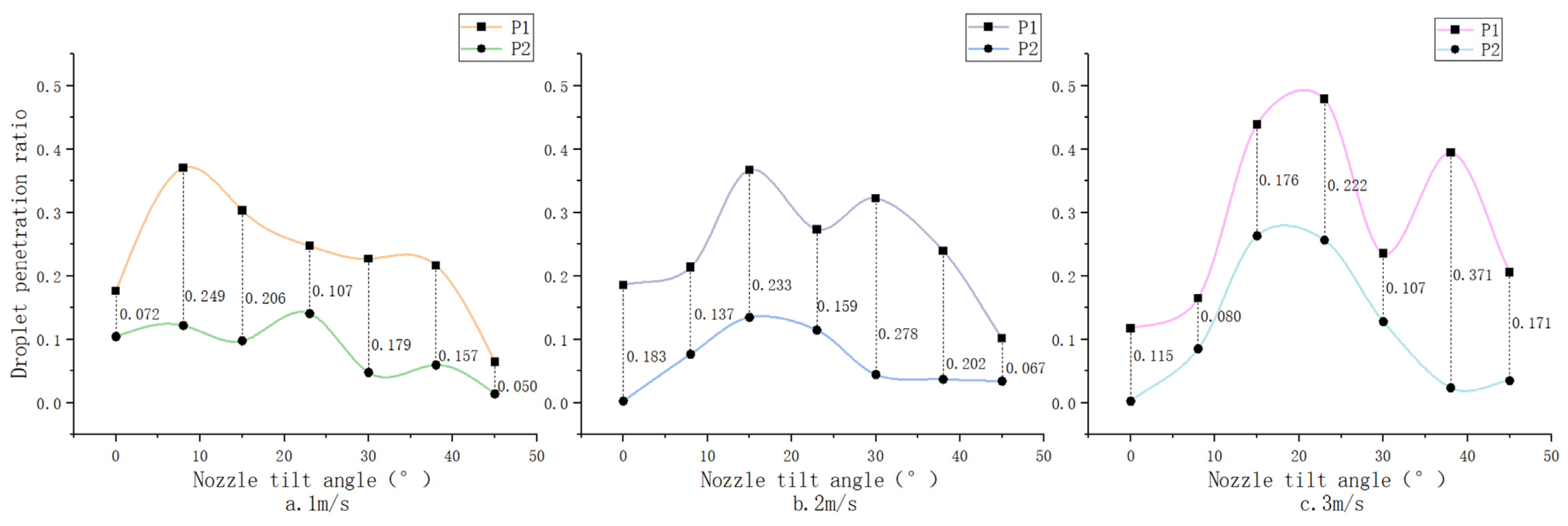
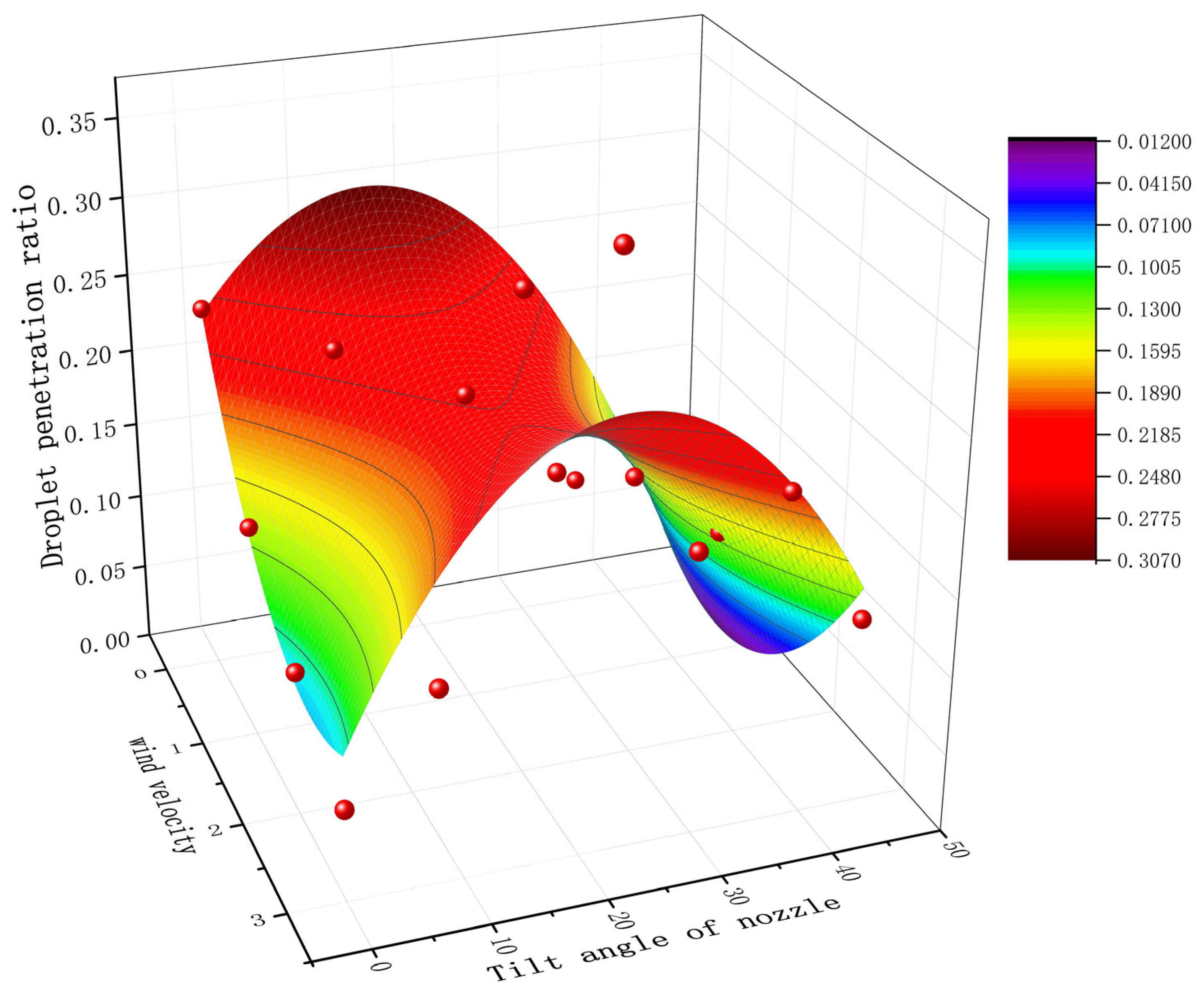
3.1.2. Analysis of Droplet Penetration Ratio
3.2. Field Trial Results and Analysis
4. Discussion
- The deposition of droplets on and within the canopy is influenced by lateral crosswinds. Adjusting the nozzle tilt angle against the wind direction effectively enhances the average deposition of droplets across the canopy layers, although this improvement lessens with deeper canopy penetration. Laboratory tests across seven nozzle tilt angles showed the most significant effect on penetrative deposition quantity at a 23° tilt, with an average change in deposition amount of +16.705 μL/cm2. The most considerable overall change in deposition amount, 28.284 μL/cm2, was observed at this angle under a 3 m/s wind speed;
- The droplet penetration ratio is affected by both wind speed and nozzle tilt angle, impacting the attenuation effect during penetration within the canopy and demonstrating a certain level of correlation. Wind speed exerts a more substantial influence than the tilt angle. Increased wind speeds coupled with augmented nozzle tilt angles can improve the droplet penetration ratio. Optimal laboratory conditions were identified as a 23° tilt angle and a 3 m/s wind speed, resulting in a 12.6% increase in penetration ratio compared to the control. The data suggested that the most effective nozzle tilt angles for enhancing droplet penetration ratios at wind speeds of 1, 2, and 3 m/s are 8°, 17°, and 25°, respectively;
- The synergistic effects of side wind and nozzle tilt angle can significantly mitigate the attenuation of droplets during canopy penetration. Influenced by dynamic shifts in canopy porosity and the angle of droplet incidence, the minimal attenuation effect was noted at a 3 m/s wind speed.
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Liu, X.M.; Liu, X.H.; Cui, H.Y.; Yuan, J. Progress and Prospect of Research on Crop Canopy Droplet Deposition. J. Agric. Mach. 2021, 52, 1–20. [Google Scholar]
- Lv, X.L.; Fu, X.M.; Wu, P.; Ding, S.M.; Zhou, L.F.; Yan, H.J. Experimental Study on the Influence of Spray Technology Parameters on Droplet Deposition Distribution. J. Agric. Mach. 2011, 42, 70–75. [Google Scholar]
- Liu, X.H. Study on the Penetration Law of Wind-Blown Droplets in Closed Canopies Based on Layered Porosity. Ph.D. Thesis, Shandong Agricultural University, Taian, China, 2021. [Google Scholar]
- Sun, C.D.; Liu, C.D. Construction and Application of a Model for Wind-Blown Spray Droplet Penetration in Canopies. J. Agric. Eng. 2019, 35, 25–32. [Google Scholar]
- Sharpe, S.M.; Boyd, N.S.; Dittmar, P.J.; MacDonald, G.E.; Darnell, R.L.; Ferrell, J.A. Spray Penetration into a Strawberry Canopy as Affected by Canopy Structure, Nozzle Type, and Application Volume. Weed Technol. 2018, 32, 80–84. [Google Scholar] [CrossRef]
- Emanuele, C.; Giuseppe, M.; Rita, P.; Domenico, L. Modelling Spray Pressure Effects on Droplet Size Distribution from Agricultural Nozzles. Appl. Sci. 2021, 11, 9283. [Google Scholar] [CrossRef]
- Uk, S.; Courshee, R.J. Distribution and Likely Effectiveness of Spray Deposits within a Cotton Canopy from Fine Ultralow-Volume Spray Applied by Aircraft. Pestic. Sci. 1982, 13, 529–536. [Google Scholar] [CrossRef]
- Zhu, H.; Rowland, D.L.; Dorner, J.W.; Derksen, R.C.; Sorensen, R.B. Influence of Plant Structure, Orifice Size, and Nozzle Inclination on Spray Penetration into Peanut Canopy. Trans. ASAE 2002, 45, 1295. [Google Scholar]
- Duga, A.T.; Ruysen, K.; Dekeyser, D.; Nuyttens, D.; Bylemans, D.; Nicolai, B.M.; Verboven, P. Spray Deposition Profiles in Pome Fruit Trees: Effects of Sprayer Design, Training System and Tree Canopy Characteristics. Crop Prot. 2015, 67, 200–213. [Google Scholar] [CrossRef]
- Foqué, D.; Nuyttens, D. Influence of Nozzle Type and Spray Angle on Spray Deposition in Ivy Pot Plants. Pest Manag. Sci. 2011, 67, 199–208. [Google Scholar] [CrossRef]
- Ru, Y.; Hu, C.; Chen, X.; Yang, F.; Zhang, C.; Li, J.; Fang, S. A Droplet Penetration Model for Spray Applications Based on Canopy Porosity. Agriculture 2023, 13, 339. [Google Scholar] [CrossRef]
- Wei, Z.; Li, R.; Xue, X.; Sun, Y.; Zhang, S.; Li, Q.; Chang, C.; Zhang, Z.; Sun, Y.; Dou, Q. Research Status, Methods, and Prospects of Air-Assisted Spray Technology. Agronomy 2023, 13, 1407. [Google Scholar] [CrossRef]
- Zhai, C.Y.; Zhao, C.J.; Ning, W.; John, L.; Wang, X.; Paul, W.; Zhang, H.H. Research Progress on Precision Control Methods of Orchard Air-Assisted Spraying. J. Agric. Eng. 2018, 34, 1–15. [Google Scholar]
- Zhang, Y.; Huang, X.; Lan, Y.; Wang, L.; Lu, X.; Yan, K.; Deng, J.; Zeng, W. Development and Prospect of UAV-Based Aerial Electrostatic Spray Technology in China. Appl. Sci. 2021, 11, 4071. [Google Scholar] [CrossRef]
- Law, S.E. Agricultural Electrostatic Spray Application: A Review of Significant Research and Development During the 20th Century. J. Electrost. 2001, 51, 25–42. [Google Scholar]
- Miller, P.C.H.; Ellis, M.C.B. Effects of Formulation on Spray Nozzle Performance for Applications from Ground-Based Boom Sprayers. Crop Prot. 2000, 19, 609–615. [Google Scholar] [CrossRef]
- Zheng, J. Development and Prospect in Environment-Friendly Pesticide Sprayers. J. Agric. Mach./Trans. Chin. Soc. Agric. Mach. 2021, 52, 1–16. [Google Scholar]
- Sun, D.Z.; Zhan, X.R.; Liu, W.K.; Xue, X.Y.; Xie, J.X.; Li, Z.; Song, S.R.; Wang, W.X. Compensation for Droplet Drift under Crosswind by Adjusting Spray Head Angle. J. Agric. Eng. 2021, 37, 80–89. [Google Scholar]
- Ferguson, J.C.; Chechetto, R.G.; Hewitt, A.J.; Chauhan, B.S.; Adkins, S.W.; Kruger, G.R.; O’Donnell, C.C. Assessing the Deposition and Canopy Penetration of Nozzles with Different Spray Qualities in an Oat Canopy. Crop Prot. 2016, 81, 14–19. [Google Scholar] [CrossRef]
- Legleiter, T.R.; Johnson, W.G. Herbicide Coverage in Narrow Row Soybean as Influenced by Spray Nozzle Design and Carrier Volume. Crop Prot. 2016, 83, 1–8. [Google Scholar] [CrossRef]
- Zhu, H.; Dorner, J.W.; Rowland, D.L.; Derksen, R.C.; Ozkan, H.E. Spray Penetration into Peanut Canopies with Hydraulic Nozzle Tips. Biosyst. Eng. 2004, 87, 275–283. [Google Scholar] [CrossRef]
- Salyani, M.; Zhu, H.; Sweeb, R.D.; Pai, N. Assessment of Spray Distribution with Water-Sensitive Paper. Agric. Eng. Int. CIGR J. 2013, 15, 101–111. [Google Scholar]
- Ovidiu, R.; Ovidiu, M.; Valentin, M.M.; Adrian, M.; Bogdan, G.A.; Valentin, C.; Sorin, S.; Tibor, R. Quality Analysis of Some Spray Parameters When Performing Treatments in Vineyards in Order to Reduce Environment Pollution. Sustainability 2021, 13, 7780. [Google Scholar] [CrossRef]
- Liu, X.D.; Xu, Y.; Hu, C.X.; Gu, Y.W.; Zhou, J.P.; Feng, Z.J. Study on Droplet Deposition Characteristics of Heavy-Duty Plant Protection UAV Based on Fluent. Agric. Mech. Res. 2024, 46, 1–8. [Google Scholar]
- Wang, L.; Lan, Y.B.; Hoffmann, W.C.; Fritz, B.K.; Chen, D.; Wang, S.M. Design and Droplet Deposition Pattern Study of a Micro Unmanned Aerial Vehicle (UAV) Low-Altitude Variable Spraying System. J. Agric. Mach. 2016, 47, 15–22. [Google Scholar]
- Zhang, H.C.; Zheng, J.Q.; Zhou, H.P.; Dorr, G.J. Study on Droplet Deposition Distribution and Off-Target Drift During Pesticide Application. J. Agric. Mach. 2017, 48, 114–122. [Google Scholar]
- Shen, L.; Fang, G.; Wang, S.; Xing, F.; Chan, S. Numerical Study of the Secondary Atomization Characteristics and Droplet Distribution of Pressure Swirl Atomizers. Fuel 2022, 324, 124643. [Google Scholar] [CrossRef]
- Liu, X.M.; Song, L.Q.; Cui, H.Y.; Liu, Y.C.; Liu, X.H.; Wu, M.Q. Decoupling Study on the Impact of Airflow Droplet Stress and Canopy Porosity Changes on Deposition Performance. J. Agric. Mach. 2021, 52, 117–126+137. [Google Scholar] [CrossRef]
- Shang, Q.Q.; Zhang, Y.Q.; Sun, Z.W.; Zheng, J.D.; Zhao, B.G. Study on Droplet Deposition and Penetration in Tree Canopies. J. Nanjing For. Univ. (Nat. Sci. Ed.) 2004, 4705, 45–48. [Google Scholar] [CrossRef]
- Zhou, R.Q.; Zhang, H.C.; Zheng, J.Q.; Zhou, H.P.; Tang, Y.S.; Wang, D. Analysis of the Impact of Mobile Spraying Parameters on Droplet Penetration for Forest Pest and Disease Control. J. Cent. South Univ. For. Technol. 2019, 39, 114–122. [Google Scholar]

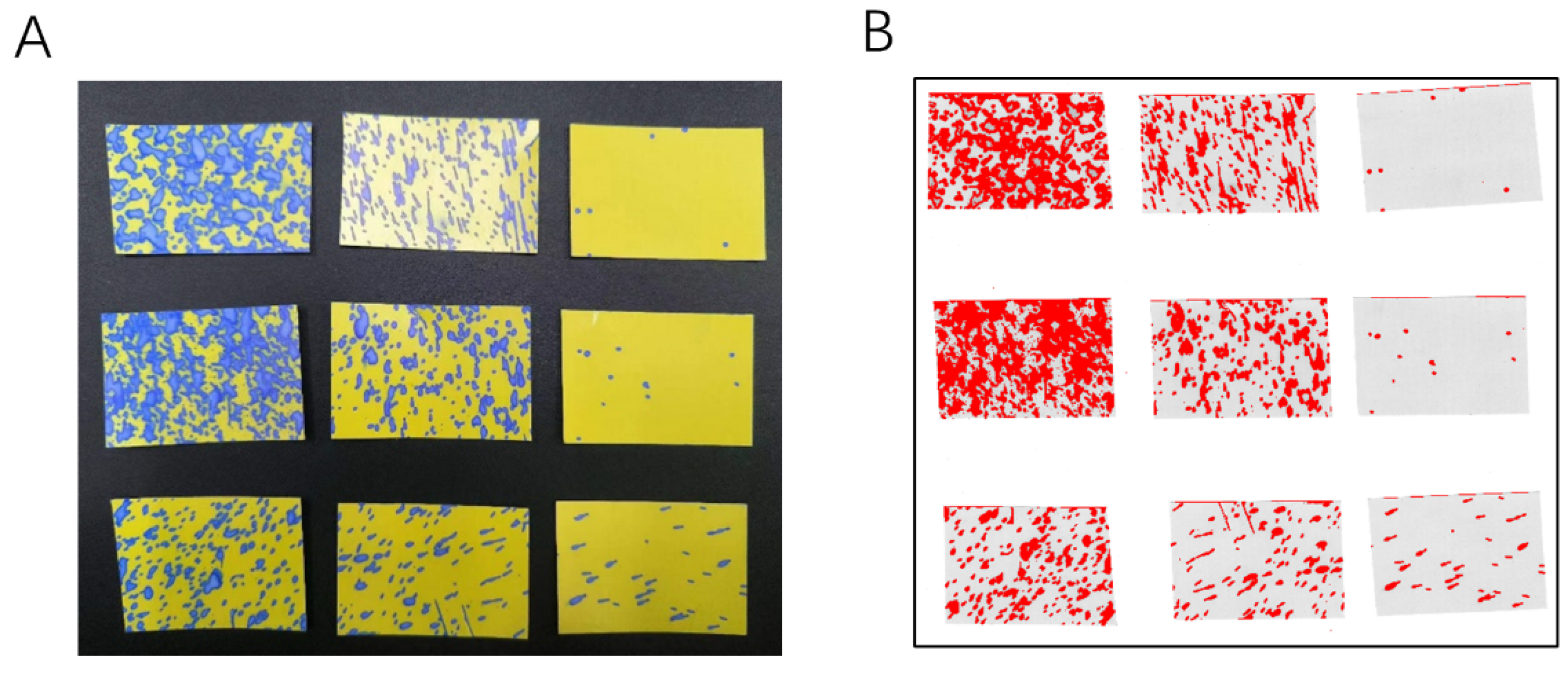









| Conditions | P1 | P2 | P |
|---|---|---|---|
| control group | 0.375 | 0.213 | 0.294 |
| experimental group | 0.390 | 0.180 | 0.285 |
| Nozzle Tilt Angle (°) | Side Wind Speed (m/s) | Value of Change in Deposition D0 (μL/cm2) | Value of Change in Deposition D1 (μL/cm2) | Value of Change in Deposition D2 (μL/cm2) |
|---|---|---|---|---|
| 8 | 1 | 3.418 | 3.652 | 0.632 |
| 2 | 5.100 | 1.337 | 1.041 | |
| 3 | 6.555 | 1.256 | 0.857 | |
| 15 | 1 | 3.089 | 2.499 | 0.220 |
| 2 | 7.116 | 4.214 | 2.121 | |
| 3 | 12.985 | 6.869 | 4.365 | |
| 23 | 1 | 3.967 | 1.853 | 1.007 |
| 2 | 9.539 | 3.385 | 2.080 | |
| 3 | 15.011 | 8.502 | 4.771 | |
| 30 | 1 | 3.355 | 1.384 | −0.536 |
| 2 | 11.671 | 4.969 | 0.884 | |
| 3 | 18.322 | 2.740 | 0.409 | |
| 38 | 1 | 1.308 | 0.776 | −0.474 |
| 2 | 10.932 | 3.089 | 0.703 | |
| 3 | 12.901 | 6.100 | 0.378 | |
| 45 | 1 | 2.955 | −1.194 | −1.072 |
| 2 | 12.733 | 0.545 | 0.708 | |
| 3 | 12.074 | 2.806 | 0.537 |
| Parameters | Numerical Value |
|---|---|
| a | 0.03047 ± 0.00116 |
| b | −2.75182 × 10−4 ± 5.13473 × 10−6 |
| c | 8.69316 × 10−4 ± 6.87885 × 10−5 |
| d | −0.10403 ± 0.00391 |
| e | 0.00827 ± 2.60412 × 10−4 |
| f | 0.21782 ± 0.00394 |
| R2 | 0.8522 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Sun, D.; Hu, J.; Huang, X.; Luo, W.; Song, S.; Xue, X. Study on the Improvement of Droplet Penetration Effect by Nozzle Tilt Angle under the Influence of Orthogonal Side Wind. Sensors 2024, 24, 2685. https://doi.org/10.3390/s24092685
Sun D, Hu J, Huang X, Luo W, Song S, Xue X. Study on the Improvement of Droplet Penetration Effect by Nozzle Tilt Angle under the Influence of Orthogonal Side Wind. Sensors. 2024; 24(9):2685. https://doi.org/10.3390/s24092685
Chicago/Turabian StyleSun, Daozong, Junyutai Hu, Xinghan Huang, Wenhao Luo, Shuran Song, and Xiuyun Xue. 2024. "Study on the Improvement of Droplet Penetration Effect by Nozzle Tilt Angle under the Influence of Orthogonal Side Wind" Sensors 24, no. 9: 2685. https://doi.org/10.3390/s24092685
APA StyleSun, D., Hu, J., Huang, X., Luo, W., Song, S., & Xue, X. (2024). Study on the Improvement of Droplet Penetration Effect by Nozzle Tilt Angle under the Influence of Orthogonal Side Wind. Sensors, 24(9), 2685. https://doi.org/10.3390/s24092685






