Fully Canonical Triple-Mode Filter with Source-Load Coupling for 5G Systems
Abstract
:1. Introduction
- Enhanced mobile broadband (eMBB) includes use cases where pedestrian experience is improved in terms of capacity, user density or coverage, among other factors.
- Ultra-reliable and low-latency communications (URLLCs) encompass real-time applications where latency, reliability and availability are critical, such as autonomous vehicles or telemedicine.
- Massive machine-type communications (mMTCs) cover applications with a high number of devices and low data transmission rates, like the Internet of Things (IoT).
2. Theoretical Design of the Triple-Mode Filter
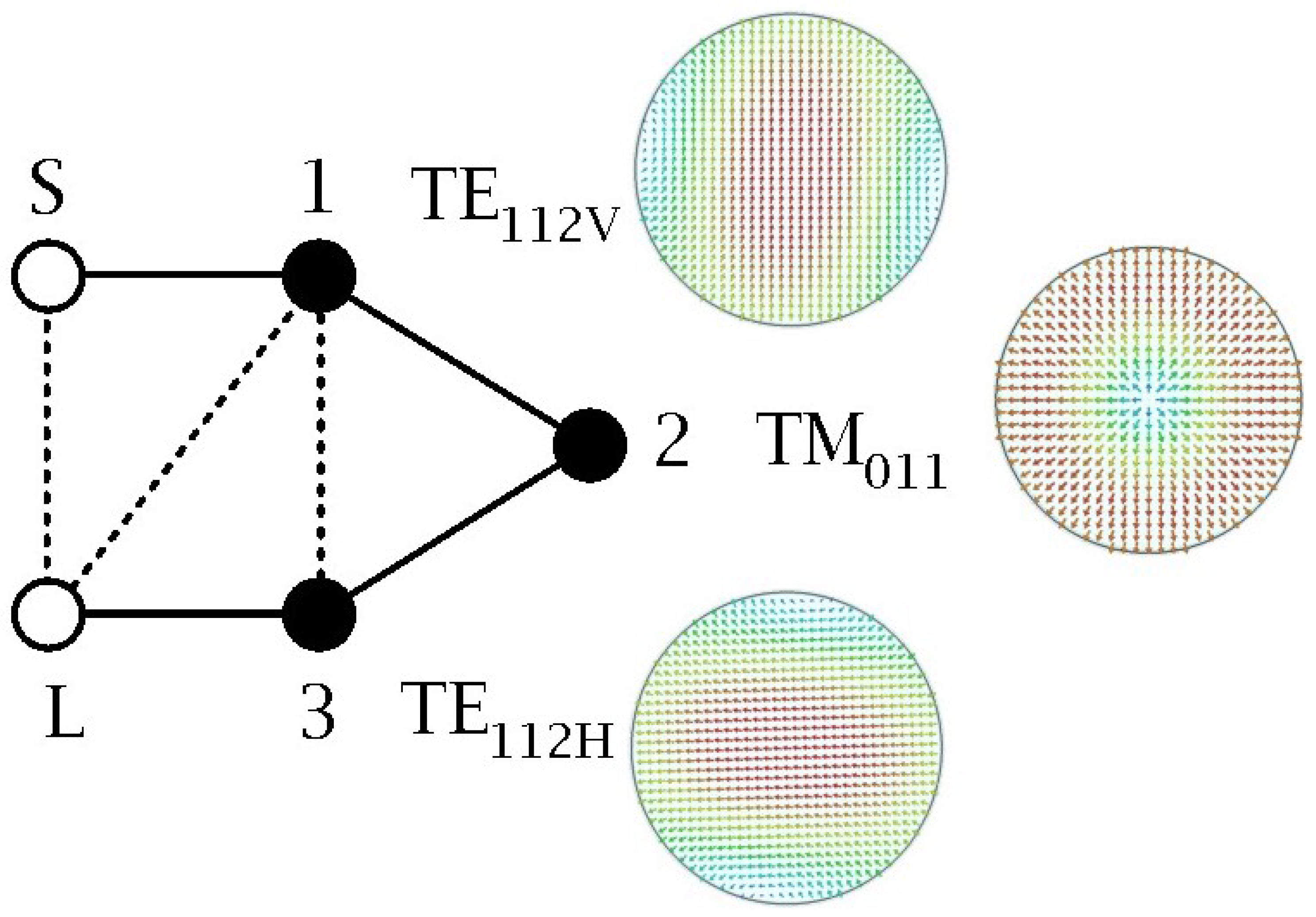
2.1. Synthesis of the Transfer Function by Means of the Coupling Matrix
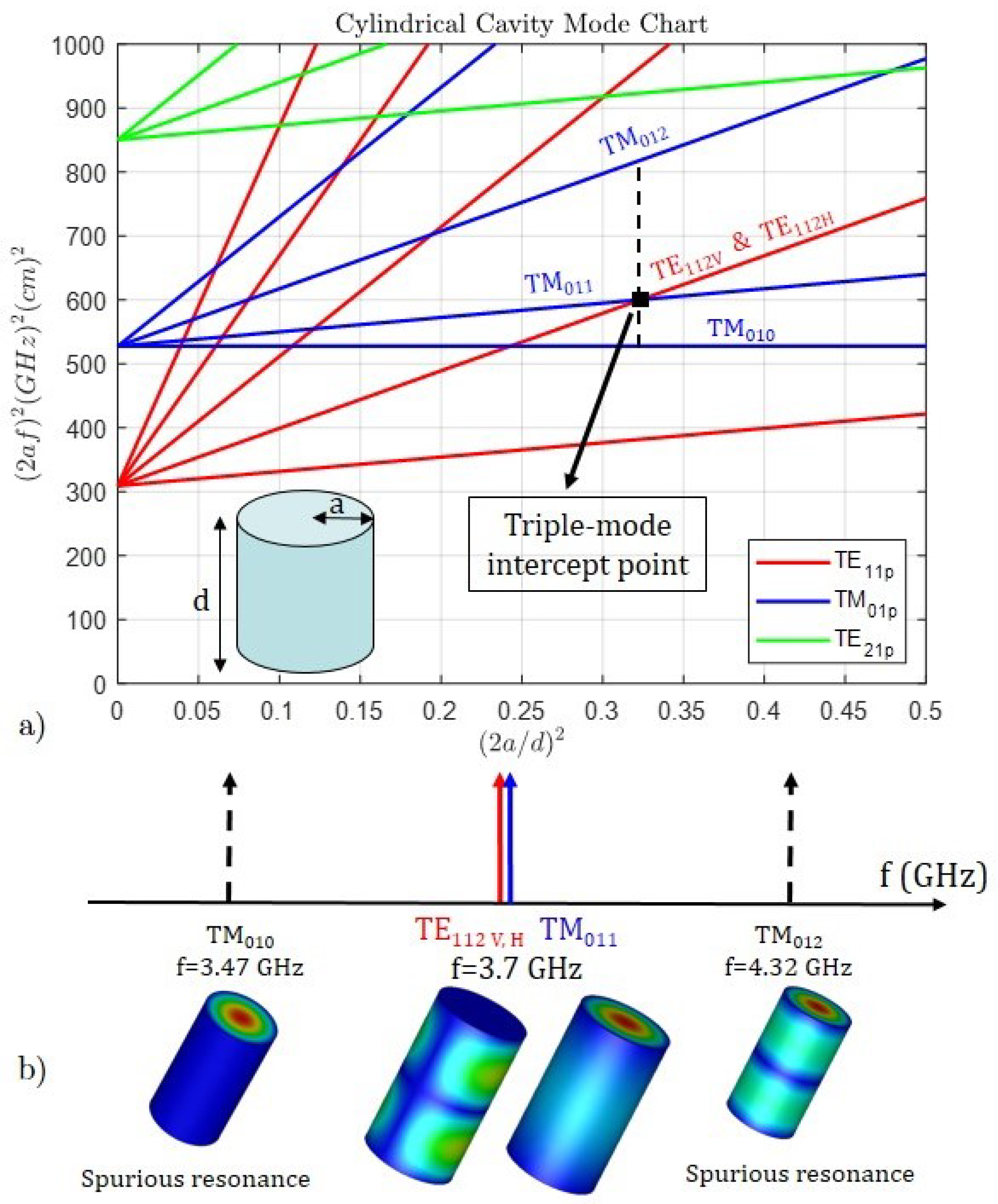
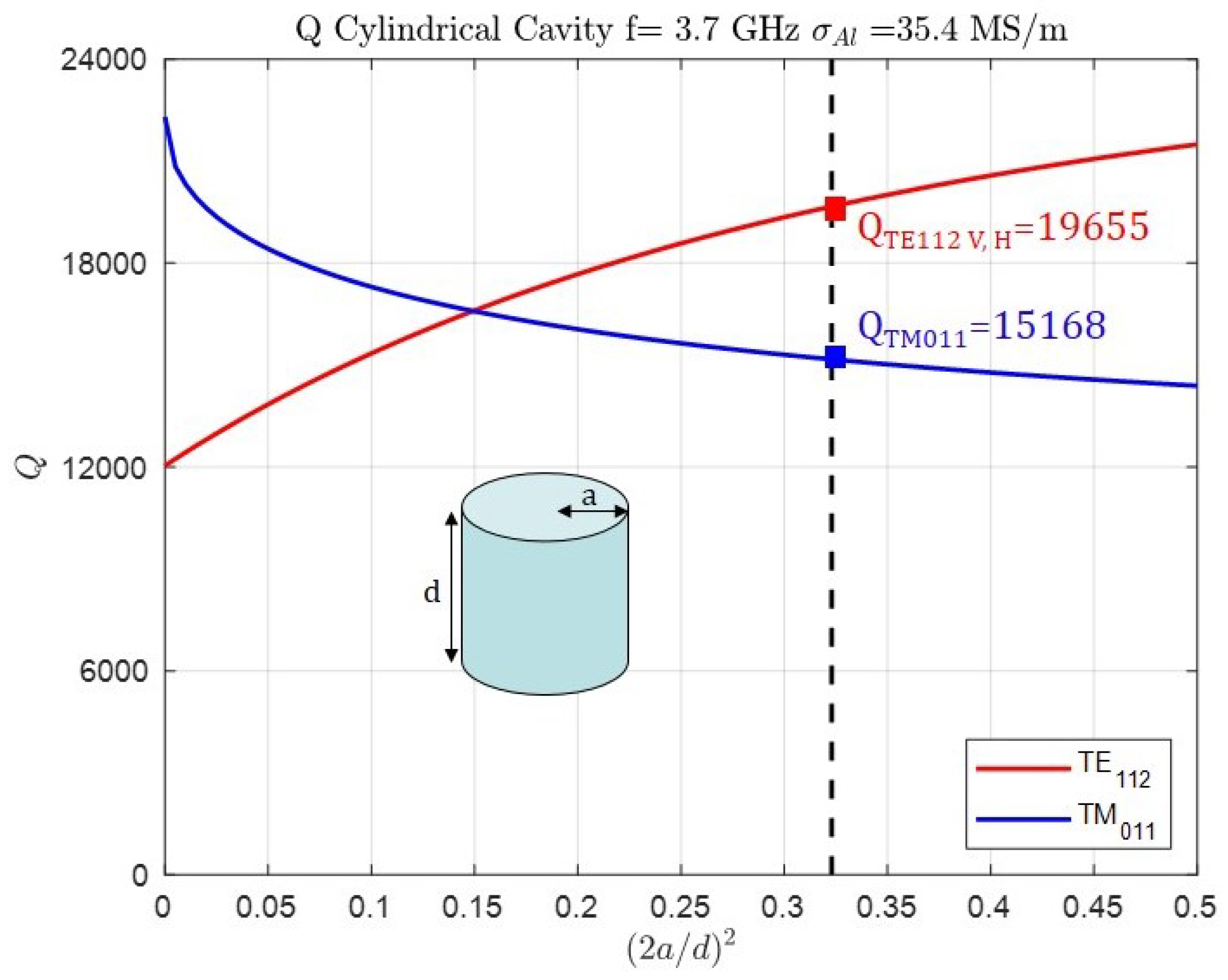
2.2. Cylindrical Cavity Design: Radius, Length, Q Factor and Spurious-Free Window
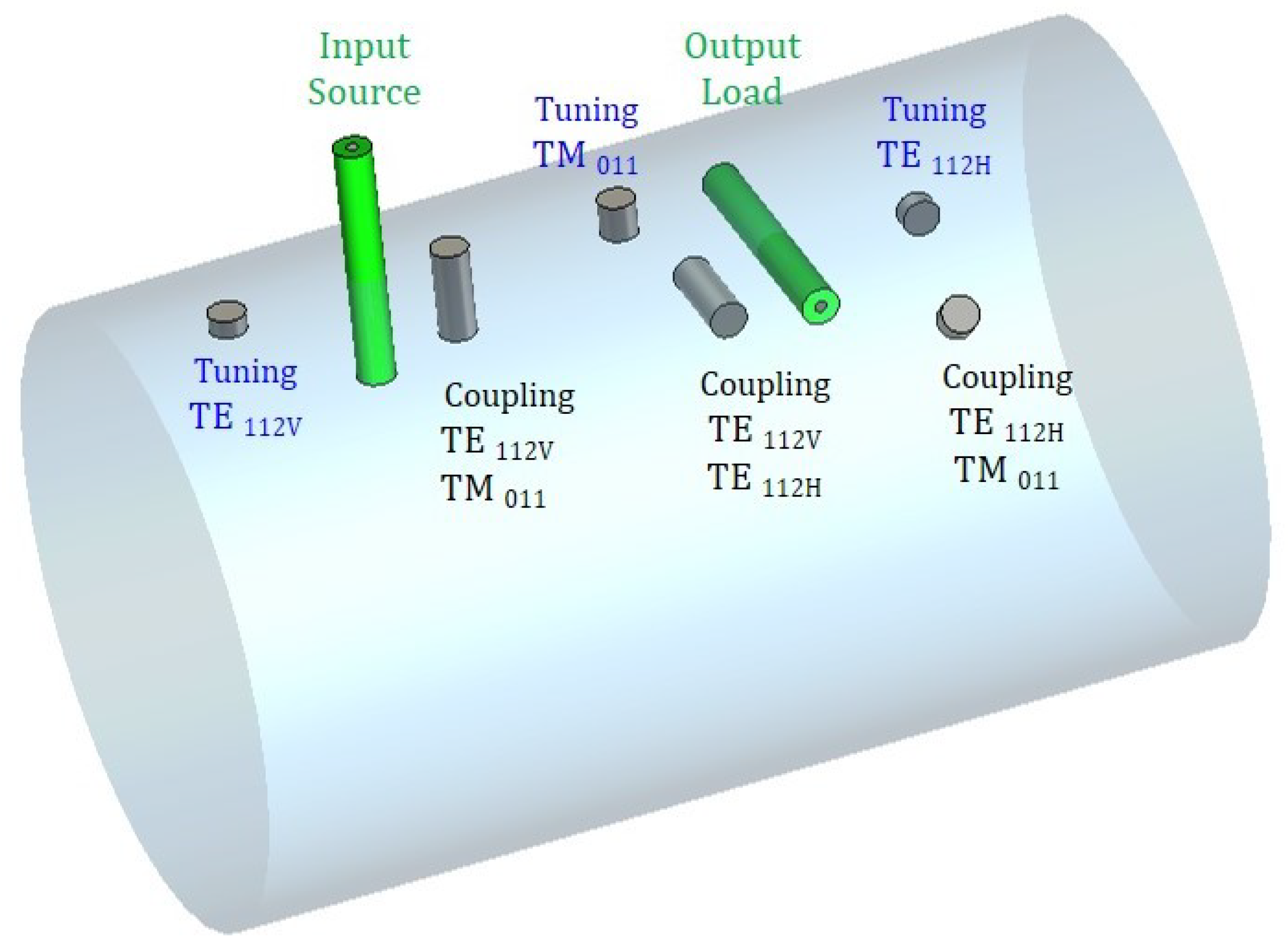
3. Electromagnetic Pre-Design of the Coupling and Tuning Elements
4. Final Design of the Triple-Mode Filter and Experimental Results
4.1. Full-Wave-Optimization of the Final Design
4.2. Manufacturing, Tuning and Measurement of the Triple-Mode Filter
5. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Shafi, M.; Molisch, A.F.; Smith, P.J.; Haustein, T.; Zhu, P.; Silva, P.D.; Tufvesson, F.; Benjebbour, A.; Wunder, G. 5G: A Tutorial Overview of Standards, Trials, Challenges, Deployment, and Practice. IEEE J. Sel. Areas Commun. 2017, 35, 1201–1221. [Google Scholar] [CrossRef]
- Watanabe, A.O.; Ali, M.; Sayyed, S.Y.B.; Tummala, R.R.; Pulugurtha, M.R. A Review of 5G Front-End Systems Package Integration. IEEE Trans. Compon. Packag. Manuf. Technol. 2021, 11, 118–133. [Google Scholar] [CrossRef]
- Wang, H.; Hu, S.; Chi, T.; Wang, F.; Li, S.; Huang, M.Y.; Park, J.S. Towards Energy-Efficient 5G Mm-Wave links: Exploiting broadband Mm-Wave doherty power amplifier and multi-feed antenna with direct on-antenna power combining. In Proceedings of the 2017 IEEE Bipolar/BiCMOS Circuits and Technology Meeting (BCTM), Miami, FL, USA, 19–21 October 2017; pp. 30–37. [Google Scholar] [CrossRef]
- Maiwald, T.; Li, T.; Hotopan, G.-R.; Kolb, K.; Disch, K.; Potschka, J.; Haag, A.; Dietz, M.; Debaillie, B.; Zwick, T.; et al. A Review of Integrated Systems and Components for 6G Wireless Communication in the D-Band. Proc. IEEE 2023, 111, 220–256. [Google Scholar] [CrossRef]
- Matthaei, G.L.; Young, L.; Jones, E.M.T. Microwave Filters, Impedance-Matching Networks, and Coupling Strucures; Artech House: London, UK, 1980. [Google Scholar]
- Pozar, D.M. Microwave Engineering, 4th ed.; John Wiley & Sons Inc.: Hoboken, NJ, USA, 2011. [Google Scholar]
- Collin, R.E. Foundations for Microwave Engineering, 2nd ed.; McGraw-Hill: New York, NY, USA, 1992. [Google Scholar]
- Southworth, G. Principles and Applications of Waveguide Transmission; Nokia Bell Labs: Murray Hill, NJ, USA, 1956. [Google Scholar]
- Sandhu, M.Y.; Afridi, S.; Lamecki, A.; Gómez-García, R.; Mrozowski, M. Miniaturized Inline Bandpass Filters Based on Triple-Mode Integrated Coaxial-Waveguide Resonators. IEEE Access 2023, 11, 81196–81204. [Google Scholar] [CrossRef]
- Salehi, H. Analysis, design and applications of the triple-mode conductor-loaded cavity filter. IET Microwaves, Antennas Propag. 2011, 5, 1136–1142. Available online: https://digital-library.theiet.org/content/journals/10.1049/iet-map.2010.0020 (accessed on 23 December 2024). [CrossRef]
- Wang, Z.; Wu, Y.; Xu, Z.; Yao, Y.; Wang, W. Adjustable Triple-Band Triple-Mode Dielectric Waveguide Filter With Multiple Transmission Zeros. IEEE Microw. Wirel. Technol. Lett. 2024, 34, 619–622. [Google Scholar] [CrossRef]
- Feng, S.-F.; Wong, S.-W.; Deng, F.; Zhu, L.; Chu, Q.-X. Triple-mode wideband bandpass filter using single rectangular waveguide cavity. In Proceedings of the 2016 IEEE International Conference on Computational Electromagnetics (ICCEM), Guangzhou, China, 23–25 February 2016; pp. 287–289. [Google Scholar] [CrossRef]
- Boe, P.; Miek, D.; Brouczek, D.; Höft, M. Bandpass Filter Based on 3D-Printed Ceramic Triple-Mode Resonator with Branches Combining TM and TE Mode Operation. In Proceedings of the 2023 53rd European Microwave Conference (EuMC), Berlin, Germany, 19–21 September 2023; pp. 396–399. [Google Scholar] [CrossRef]
- Ren, F.; Hong, W.; Wu, K. Design and implementation of an SIW triple-mode filter. In Proceedings of the 2014 3rd Asia-Pacific Conference on Antennas and Propagation, Harbin, China, 26–29 July 2014; pp. 1128–11230. [Google Scholar] [CrossRef]
- Lee, K.C.; Su, H.T.; Haldar, M.K. A novel compact triple-mode resonator for microstrip bandpass filter design. In Proceedings of the 2010 Asia-Pacific Microwave Conference, Yokohama, Japan, 7–10 December 2010; pp. 1871–1874. Available online: https://ieeexplore.ieee.org/document/5728332 (accessed on 24 December 2024).
- Gowrish, B.; Koul, S.K.; Mansour, R.R. Transversal Coupled Triple-Mode Spherical Resonator-Based Bandpass Filters. IEEE Microw. Wirel. Compon. Lett. 2021, 31, 369–372. [Google Scholar] [CrossRef]
- Montejo-Garai, J.R.; Zapata, J. Full-wave CAD of in-line symmetric and asymmetric triple-mode circular waveguide filters. Microw. Opt. Technol. Lett. 1999, 20, 135–140. [Google Scholar] [CrossRef]
- Liang, G.-Z.; Chen, F.-C.; Xiang, K.-R. A Triple-Mode Cylindrical Cavity-Backed Slot Filtering Antenna with High Selectivity. In Proceedings of the 2021 51st European Microwave Conference (EuMC), London, UK, 4–6 April 2021; pp. 664–667. [Google Scholar] [CrossRef]
- Rosenberg, U.; Wolk, D. Filter design using in-line triple-mode cavities and novel iris couplings. IEEE Trans. Microw. Theory Tech. 1989, 37, 2011–2019. [Google Scholar] [CrossRef]
- Zhang, Z.-C.; Yu, X.-Z.; Wong, S.-W.; Zhao, B.-X.; Lin, J.-Y.; Zhang, X. Miniaturization of Triple-Mode Wideband Bandpass Filters. IEEE Trans. Compon. Packag. Manuf. Technol. 2022, 12, 1368–1374. [Google Scholar] [CrossRef]
- Wong, S.-W.; Feng, S.-F.; Zhu, L.; Chu, Q.-X. Triple- and Quadruple-Mode Wideband Bandpass Filter Using Simple Perturbation in Single Metal Cavity. IEEE Trans. Microw. Theory Tech. 2015, 63, 3416–3424. [Google Scholar] [CrossRef]
- Bakr, M.S.; Gentili, F.; Bosch, W. Triple mode dielectric-loaded cavity band pass filter. In Proceedings of the 2016 16th Mediterranean Microwave Symposium (MMS), Abu Dhabi, United Arab Emirates, 14–16 November 2016; pp. 1–3. [Google Scholar] [CrossRef]
- Bakr, M.S. Triple-Mode Microwave Filters With Arbitrary Prescribed Transmission Zeros. IEEE Access 2021, 9, 22045–22052. [Google Scholar] [CrossRef]
- Yakuno, S.; Ishizaki, T. Novel cavity-type multi-mode filter using TEM-mode and TE-mode. In Proceedings of the 2012 Asia Pacific Microwave Conference Proceedings, Kaohsiung, Taiwan, 4–7 December 2012; pp. 376–378. [Google Scholar] [CrossRef]
- Amari, S.; Rosenberg, U. A Circular Triple-Mode Cavity Filter With Two Independently Controlled Transmission Zeros. In Proceedings of the 2006 European Microwave Conference, Manchester, UK, 10–15 September 2006; pp. 1091–1094. [Google Scholar] [CrossRef]
- Bell, H.C. The Coupling Matrix in Low-Pass Prototype Filters. IEEE Microw. Mag. 2007, 8, 70–76. [Google Scholar] [CrossRef]
- Cameron, R.J.; Kudsia, C.M.; Mansour, R.R. Microwave Filters for Communication Systems: Fundamentals, Design and Applications; Wiley-Interscience: Hoboken, NJ, USA, 2007. [Google Scholar]
- Ragan, G.L. Microwave Transmission Circuits, Radiation Lab Series MIT; McGraw-Hill: New York, NY, USA, 1948; pp. 673–677. [Google Scholar]
- CST Studio. Available online: https://www.3ds.com/products/simulia/cst-studio-suite (accessed on 4 April 2024).
- Hong, J.-S. Microstrip Filters for RF/Microwave Applications, 2nd ed.; Jon Wiley & Sons: New York, NY, USA, 2011. [Google Scholar]




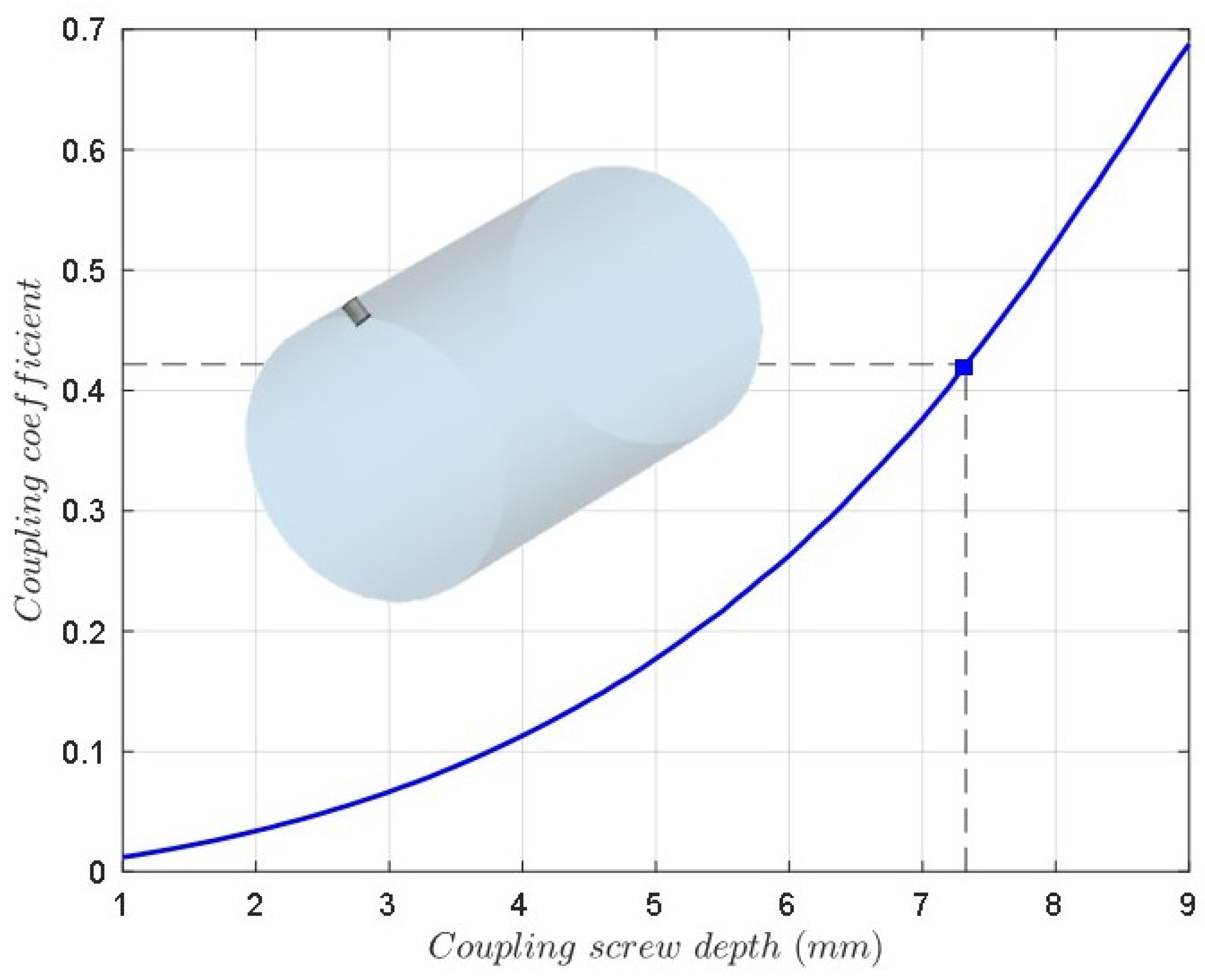

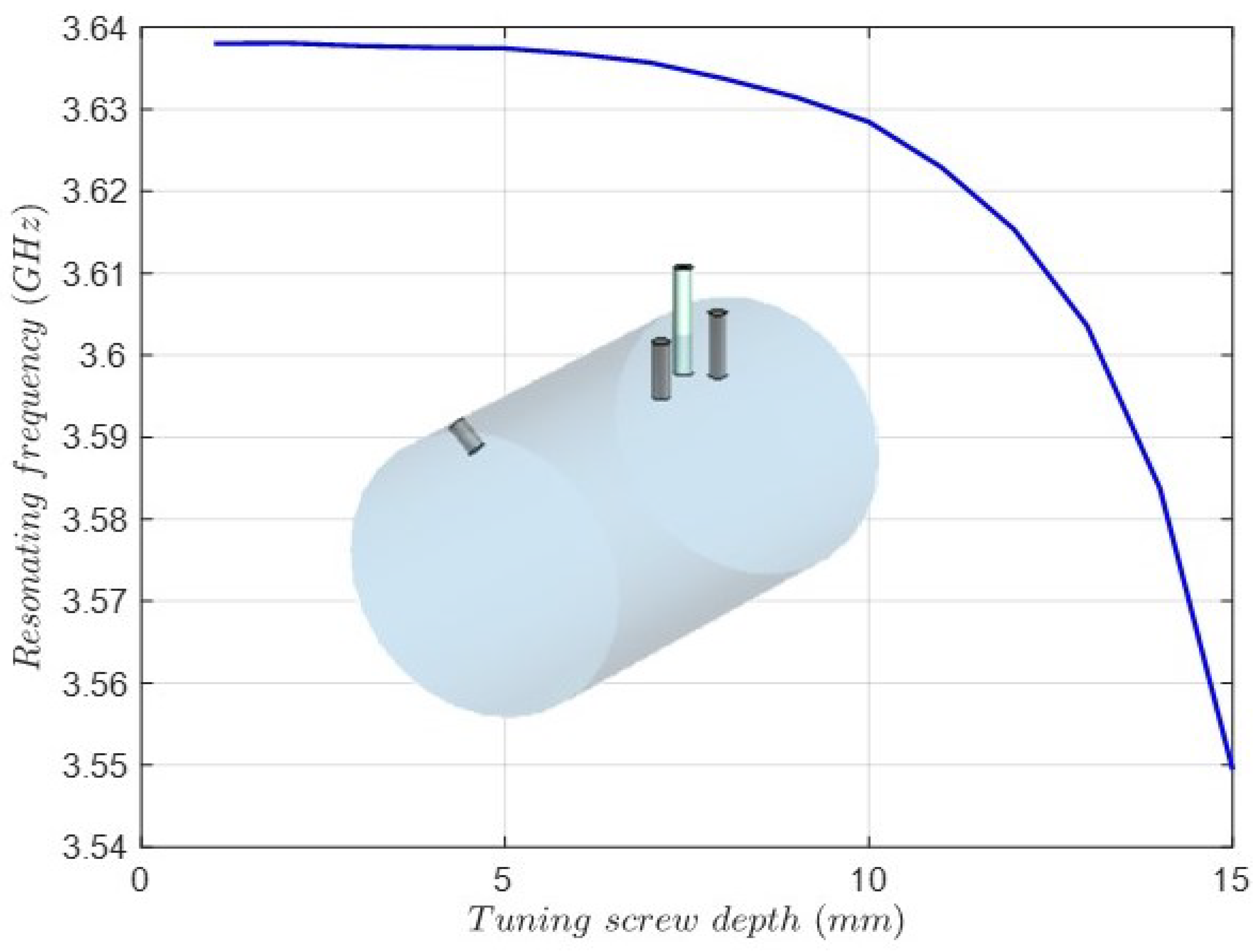


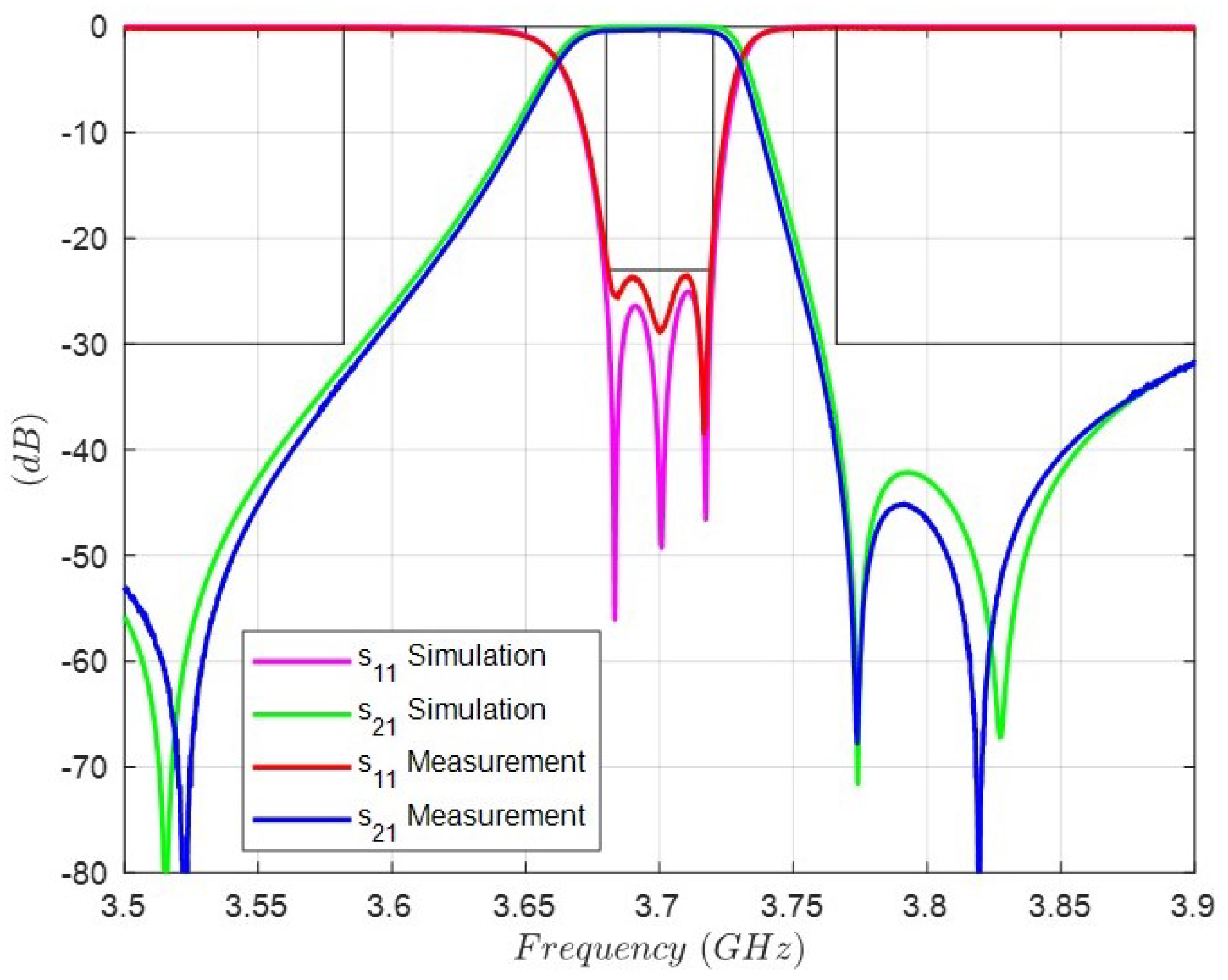

| Order N | 3 |
| Center frequency | 3.7 GHz |
| Bandwidth BW | 40 MHz |
| Transmission zeros | |
| Return loss | 23 dB |
| Insertion loss | <0.5 dB |
| Input–output ports | SMA 50 Ohms coaxial |
| Matrix 1 | Matrix 2 | Matrix 3 | |
|---|---|---|---|
| Parameter | Value (mm) |
|---|---|
| Cavity radius | 33.09 |
| Cavity length | 113 |
| Coaxial probe length | 10 |
| Screw radius | 2 |
| - coupling screw depth | 13.11 |
| - coupling screw depth | 13.11 |
| - coupling screw depth | 7.32 |
| tuning screw depth | 12.59 |
| tuning screw depth | 0 |
| Parameter | Value (mm) |
|---|---|
| Cavity radius | 33.37 |
| Cavity length | 110.51 |
| Coaxial probe length | 12.54 |
| Screw radius | 2 |
| - coupling screw depth | 0.32 |
| - coupling screw depth | 0.28 |
| - coupling screw depth | 2.49 |
| tuning screw depth | 0.12 |
| tuning screw depth | 0 |
| Characteristic | [17] | [20] | [21] | [23] | This Work |
|---|---|---|---|---|---|
| Number of resonant modes | 3 | 3 | 3 | 3 | 3 |
| Number of Tzs | 1 | 3 | 2 | 2 | 3 |
| Controllable Tzs | Yes | No | No | Yes | Yes |
| Type of intermode coupling | Coupling screw (totally tunable) | Metal disk and post (no tunable) | Metal cylinder (no tunable) | Metal disk and post (no tunable) | Coupling screw (totally tunable) |
| Type of external coupling | Rectangular waveguide | Coaxial SMA | Coaxial SMA | Coaxial SMA | Coaxial SMA |
| Center frequency CF | 12 GHz | 2.5 GHz | 3.3 GHz | 2.45 GHz | 3.7 GHz |
| Fractional bandwidth | 0.75% | 40% | 30% | 3.4% | 1.1% |
| Return losses | 23 dB | 17.5 dB | 20 dB | 20 dB | 23 dB |
| Insertion losses at CF | 0.43 dB | 0.28 dB | 0.5 dB | 0.18 dB | 0.28 dB |
| Unloaded Q (resonant modes) | N/A | — 2039 —1284 —2236 | —4828 —4672 —4941 | N/A | —19,655 —15,168 |
| Size Wavelength at CF | Diameter: 0.89 Length: 1.92 | 0.46 × 0.19 × 0.12 | 0.5 × 0.5 × 0.56 | 0.03 × 0.03 × 0.05 | Diameter: 0.82 Length: 1.36 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
López-Montes, C.; Montejo-Garai, J.R. Fully Canonical Triple-Mode Filter with Source-Load Coupling for 5G Systems. Sensors 2025, 25, 90. https://doi.org/10.3390/s25010090
López-Montes C, Montejo-Garai JR. Fully Canonical Triple-Mode Filter with Source-Load Coupling for 5G Systems. Sensors. 2025; 25(1):90. https://doi.org/10.3390/s25010090
Chicago/Turabian StyleLópez-Montes, Cristóbal, and José R. Montejo-Garai. 2025. "Fully Canonical Triple-Mode Filter with Source-Load Coupling for 5G Systems" Sensors 25, no. 1: 90. https://doi.org/10.3390/s25010090
APA StyleLópez-Montes, C., & Montejo-Garai, J. R. (2025). Fully Canonical Triple-Mode Filter with Source-Load Coupling for 5G Systems. Sensors, 25(1), 90. https://doi.org/10.3390/s25010090






