Application Research on High-Precision Tiltmeter with Rapid Deployment Capability
Abstract
:1. Introduction
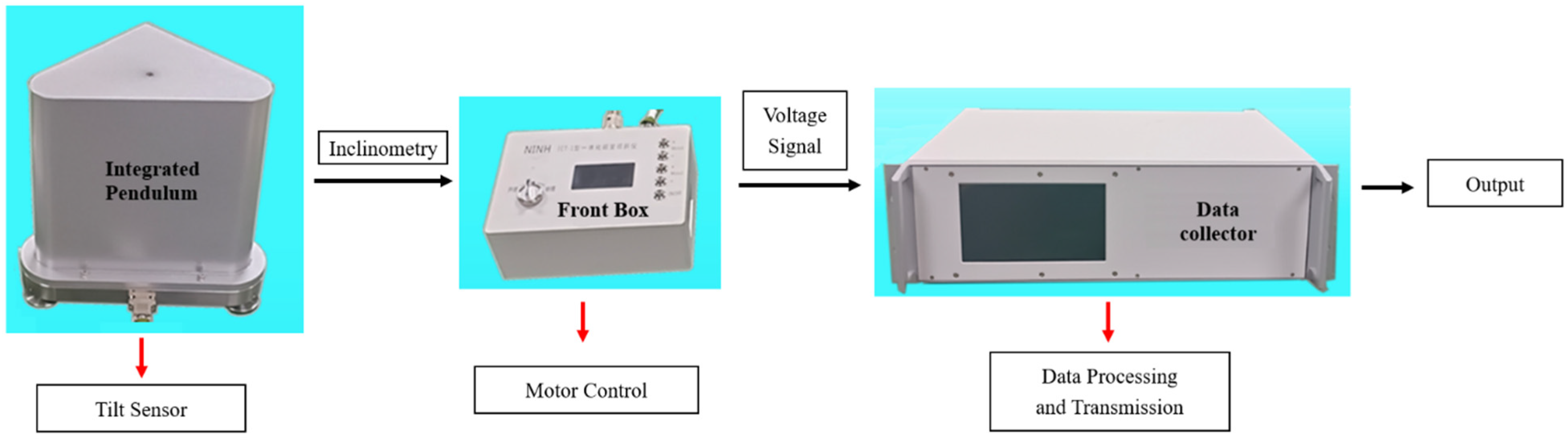
2. ICT-1 Integrated Chamber Inclinometer
2.1. Integrated Pendulum and Triangular Platform
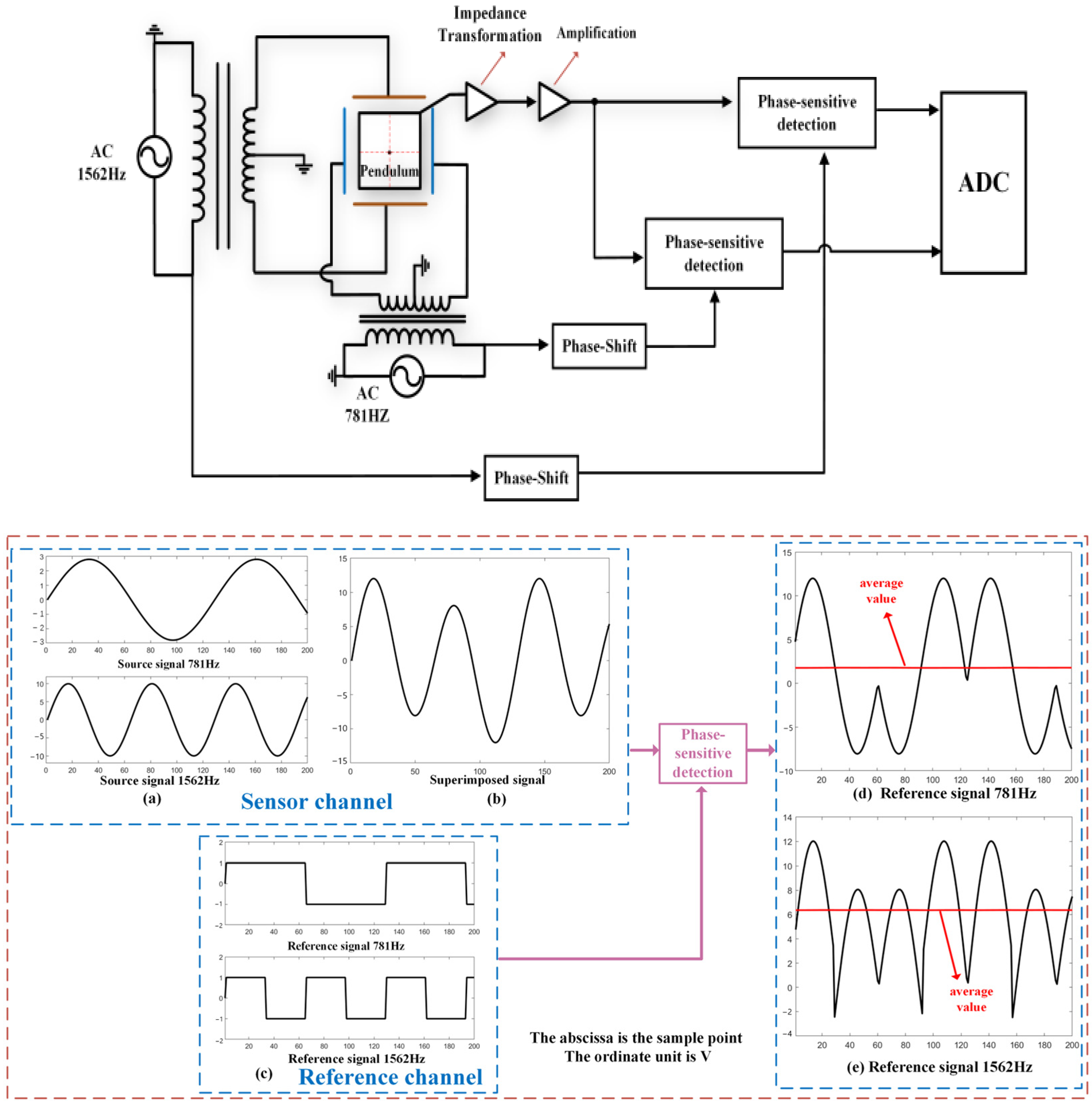
2.2. Tiltmeter Measurement and Control Circuit
2.3. Rapid Deployment Capability of Integrated Chamber Inclinometer
3. Application of the Rapid Deployment Capability of Integrated Chamber Inclinometers in Geophysical Networks
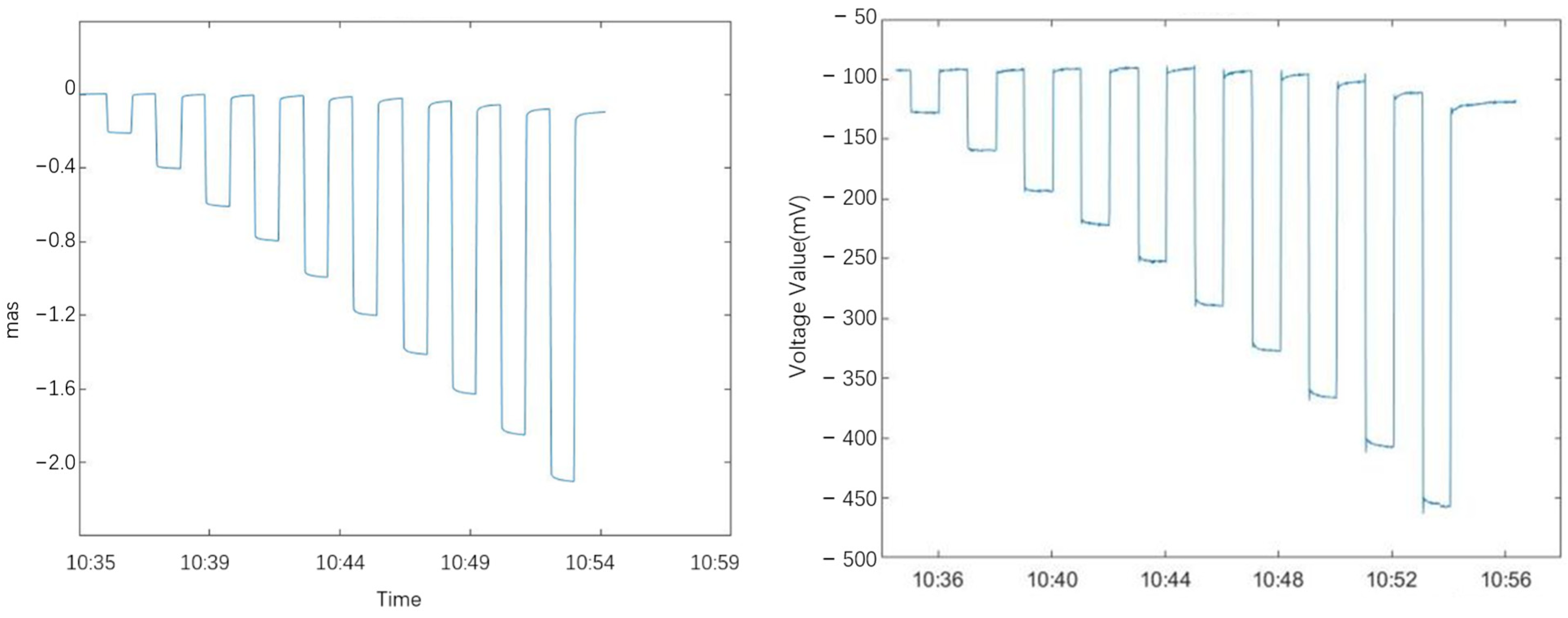
3.1. Laboratory Calibration
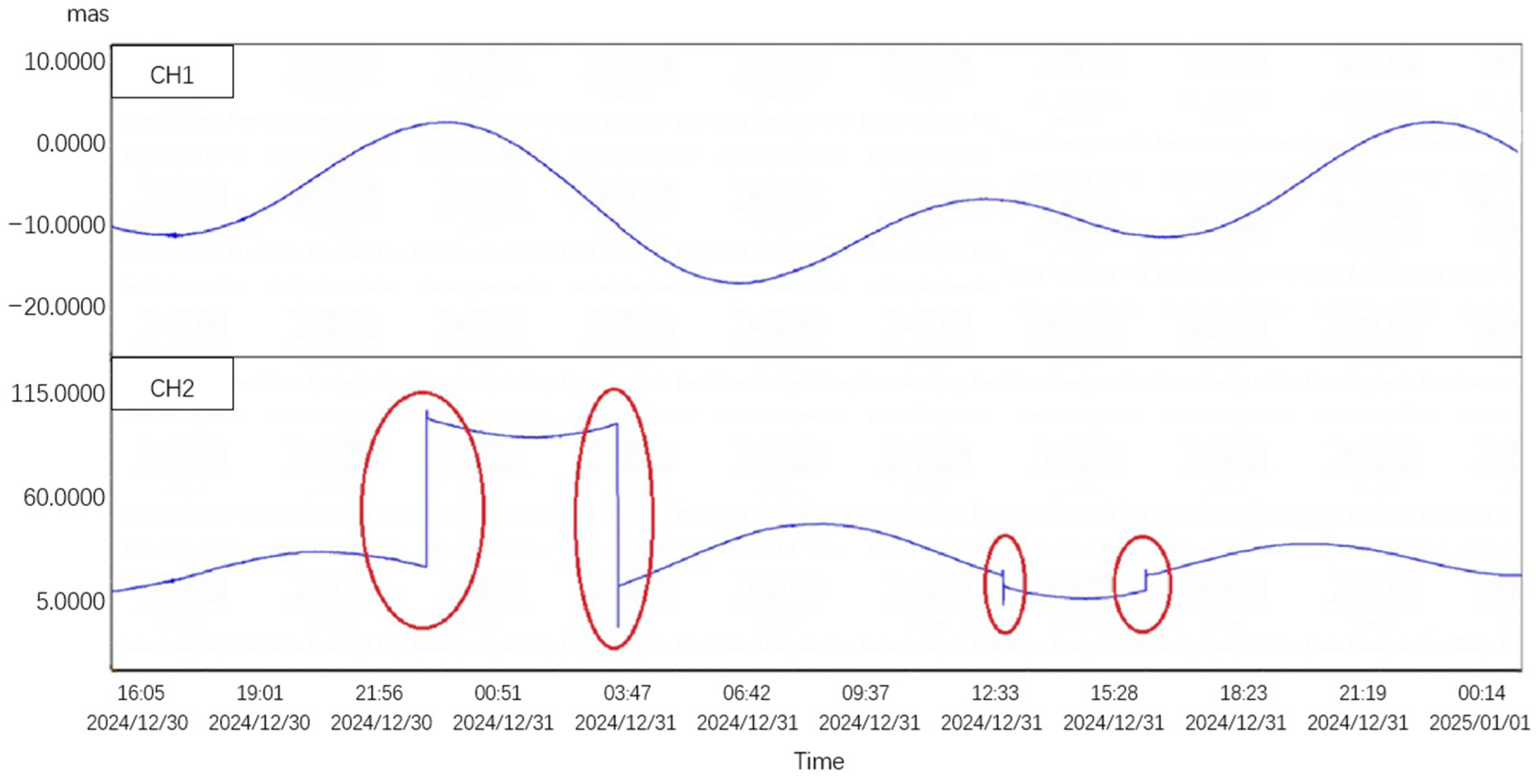
3.2. Ground Tilt Observation
3.3. Solid Tide Calculation
4. Discussion
5. Conclusions
- The ICT-1 integrated chamber inclinometer uses a cross-spring vertical pendulum, which enhances the strength of the pendulum body. After installing a self-locking mechanism and a fully enclosed outer cover, it achieves impact resistance during ordinary transportation. The use of a short baseline triangle platform greatly reduces the external dimensions of the equipment. The built-in zero adjustment motor has a compact structure, is easy to carry, does not require on-site assembly, is easy to use, and can be installed without the need for professional technicians. It has the ability to be deployed quickly.
- The observation resolution of the ICT-1 integrated chamber inclinometer reaches 0.0002”, which is equivalent to the observation resolution of existing chamber pendulum inclinometers and meets the requirements of the seismic observation instrument grid access technical standard DB/T31.2-2008. Like existing chamber tilt inclinometers, the ICT-1 integrated chamber tilt inclinometer is also affected by temperature. During the initial installation of the equipment, there may be significant drift in the observation data. However, due to its small size and fast thermal balance, solid tides can be observed within 24 h of equipment installation and stabilized within a week.
- The ICT-1 integrated chamber inclinometer has the characteristics of rapid deployment, fast stability, and small size, making it suitable for rapid detection, flow monitoring, emergency response, and other fields on platforms. It is of great significance for the observation of geophysical field deformation.
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Zadro, M.; Braitenberg, C. Measurements and interpretations of tilt–strain gauges in seismically active areas. Earth-Sci. Rev. 1999, 47, 151–187. [Google Scholar] [CrossRef]
- Tang, L.; Fan, J.; Liu, G.; Qiu, Z. The Reliability Analysis of Strain Seismic Waves Recorded by High Sampling Four-component Borehole Strainmeter. Earthq. Res. China 2023, 39, 78–87. [Google Scholar]
- Allen, R.V. A borehole tiltmeter for measurements at tidal sensitivity. Bull. Seismol. Soc. Am. 1972, 62, 815–821. [Google Scholar] [CrossRef]
- Saleh, B. Study of Earth tides using quartz tiltmeter. J. Surv. Eng. 2003, 129, 51–55. [Google Scholar] [CrossRef]
- Hou, C.; Ouyang, Z. A Review on the Observation Technique of Ground Tilt. Bull. Inst. Crustal Dyn. 2006, 18, 149–157. [Google Scholar]
- Wu, L.H.; Chen, Z.; Li, T.; Ouyang, Z. Application of capacitive tilt sensor in crustal deformation measurement. Transducer Microsyst. Technol. 2010, 29, 129–131. [Google Scholar]
- Li, T.; Wu, L.; Chen, Z.; Li, H. A Remote Calibration System for Cbt-Type Borehole-Tiltmeter Based on Stepping Motor. J. Geod. Geodyn. 2014, 34, 173–176. [Google Scholar]
- Jiang, F.; Gao, S.; Xue, B.; Li, J. Research on transfer function test method of quartz horizontal pendulum tilter with force balance feedback. Earthquake 2022, 42, 52–62. (In Chinese) [Google Scholar]
- Gao, S.; Jiang, F.; Li, J.; Xue, B.; Wang, H. Electrostatic force feedback technique for quartz horizontal pendulum inclinometer. Earthquake 2020, 40, 151–158. [Google Scholar]
- Nie, L.; Wen, X.; Yi, Z. Development of DSQ short base water pipe inclinometer and calibration device. Earth Crust Deform. Earthq. 2001, 74–81. [Google Scholar] [CrossRef]
- Liu, J. State Intellectual Property Office granted VS vertical pendulum Tiltmeter “Invention Patent Certificate”. Geod. Geodyn. 2004, 45. [Google Scholar]
- Lv, P.; Li, Z.; Geng, L.; Li, N.; Xu, C. VP type vertical pendulum tiltmeter observed frequency analysis and improvement. J. Geod. Geodyn. 2020, 40, 540–545. [Google Scholar] [CrossRef]
- Huang, Y.; Wu, L. Research Development of Tiltmeter with High Precision. Sens. World 2008, 5, 10–15. [Google Scholar]
- Wu, L.; Li, T.; Chen, Z. Experiment Study on Vibrating Stress Relief Preaging System of the CBT Borehole Tilt Sensor. Technol. Earthq. Disaster Prev. 2020, 15, 216–223. [Google Scholar]
- Huang, Y.; Wu, L. Crustal tilt measurement with two-dimension vertical pendulum tiltmeter. Chin. J. Sci. Instrum. 2007, 28, 1514. [Google Scholar]
- Wu, L.; Li, T.; Chen, Z.; Li, H. A new capacitive borehole tiltmeter for crustal deformation measurement and its performance analysis. Int. J. Min. Sci. Technol. 2015, 25, 285–290. [Google Scholar] [CrossRef]
- Xu, X.; Chen, Z.; Li, H.; Ma, S.; Wu, L.; Wang, W.; Dong, Y.; Zhan, W. Design of A High-Precision Component-Type Vertical Pendulum Tiltmeter Based on FPGA. Sensors 2023, 23, 7998. [Google Scholar] [CrossRef] [PubMed]
- Xu, X.; Chen, Z.; Li, H.; Zhan, W.; Wang, W.; Dong, Y.; Wu, L.; Li, X. A Multi-Channel Borehole Strain Measurement and Acquisition System Based on FPGA. Sensors 2023, 23, 6981. [Google Scholar] [CrossRef] [PubMed]
- Tang, L.; Qiu, Z.; Fan, J.; Yin, Z. The apparent focal depth, emergence angle, and take-off angle of seismic wave measured by YRY-4-type borehole strainmeter as one kind of strain seismograph. Front. Earth Sci. 2023, 11, 1036797. [Google Scholar] [CrossRef]
- Tang, L.; Qiu, Z.; Li, Y.; Li, Y.; Fan, J. Determination and analysis of near-surface stress-strain state of 4-component borehole strainmeter. Geomat. Inf. Sci. Wuhan Univ. 2025, 50, 63–73. [Google Scholar] [CrossRef]
- Tang, L.; Fan, J.; Miao, M.; Chen, Z. Two Different Layouts of Sensors of Four-gauge Borehole Strainmeter and Their Influence on Field Measurement. Sens. Mater. 2022, 34, 4425–4434. [Google Scholar] [CrossRef]
- DB/T 31.1-2008; Technical Requirements of Instruments in Network for Earthquake Monitoring. The Instrument for Crustal Deformation Observation. Part 1: Tiltmeter. Industry Standards—Earthquake. Institute of Crustal Dynamics, China Earthquake Administration: Beijing, China, 2008; 25p.
- Chen, Z.; Li, H.; Dong, Y.; Wang, W.; Wu, L.; Zhan, W. Research on the RZB-Type Three-Dimensional Drilling Strain Measurement System. Sensors 2024, 24, 3954. [Google Scholar] [CrossRef] [PubMed]
- Tang, L.; Qiu, Z.; Fan, J.; Luo, Z. Characteristic Analysis of Coseismic Variation of 4-Component Borehole Strain Observation with Different Sampling Rates. J. Geod. Geodyn. 2022, 42, 1196–1201. [Google Scholar]





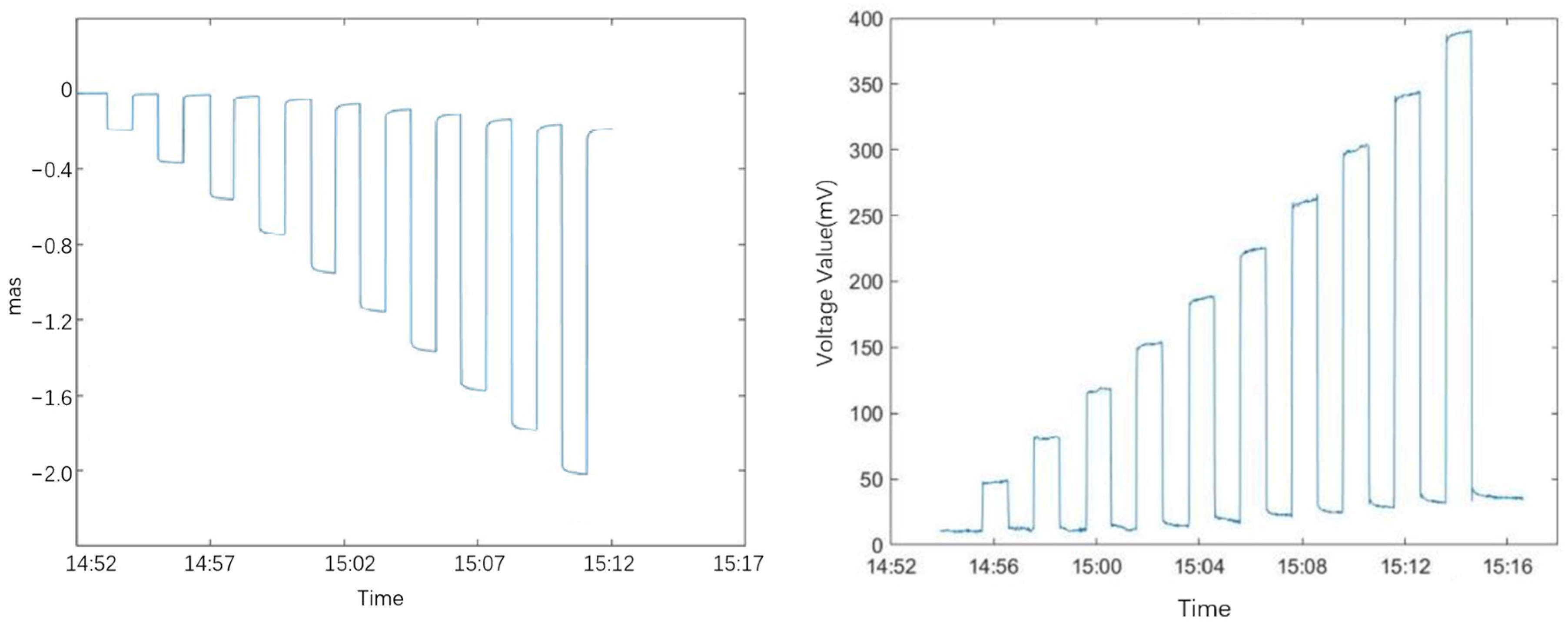
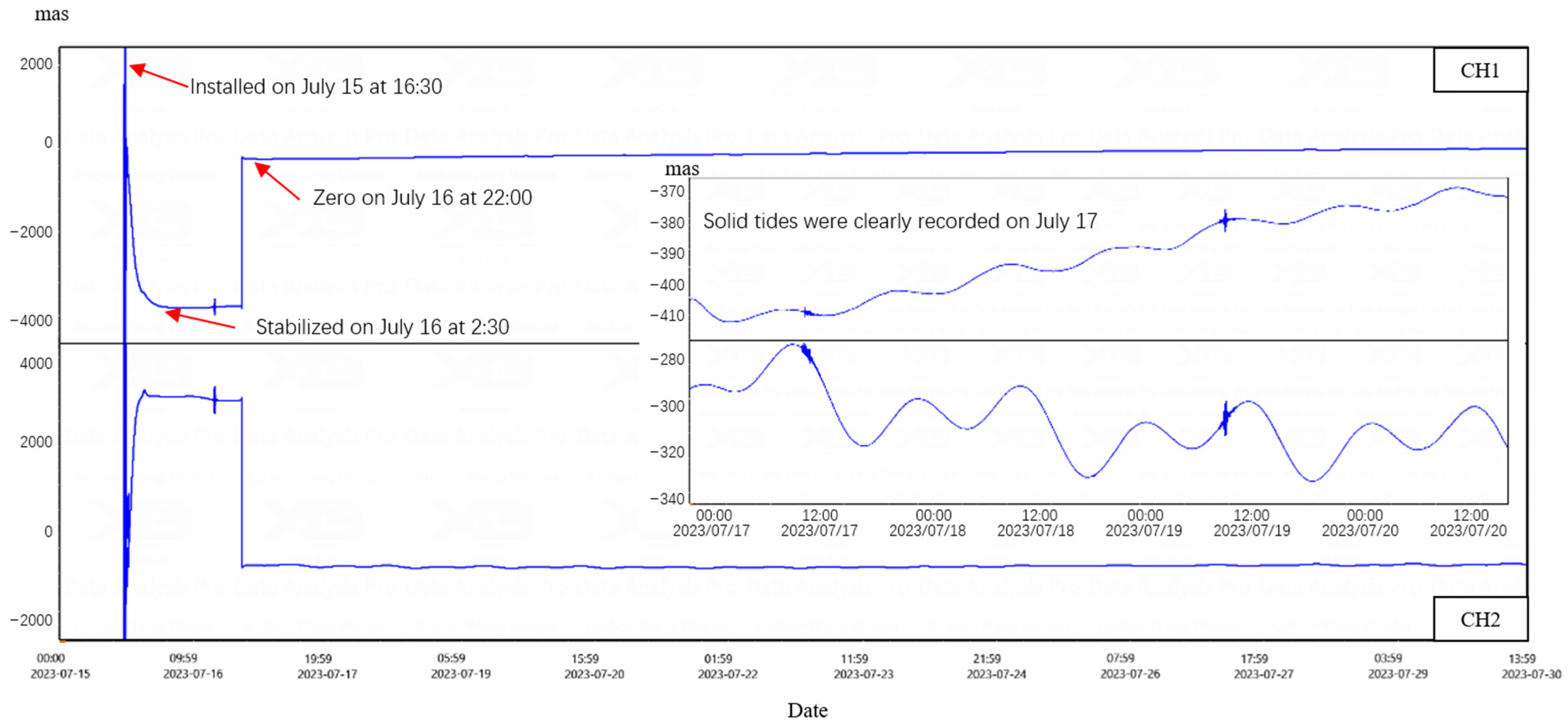


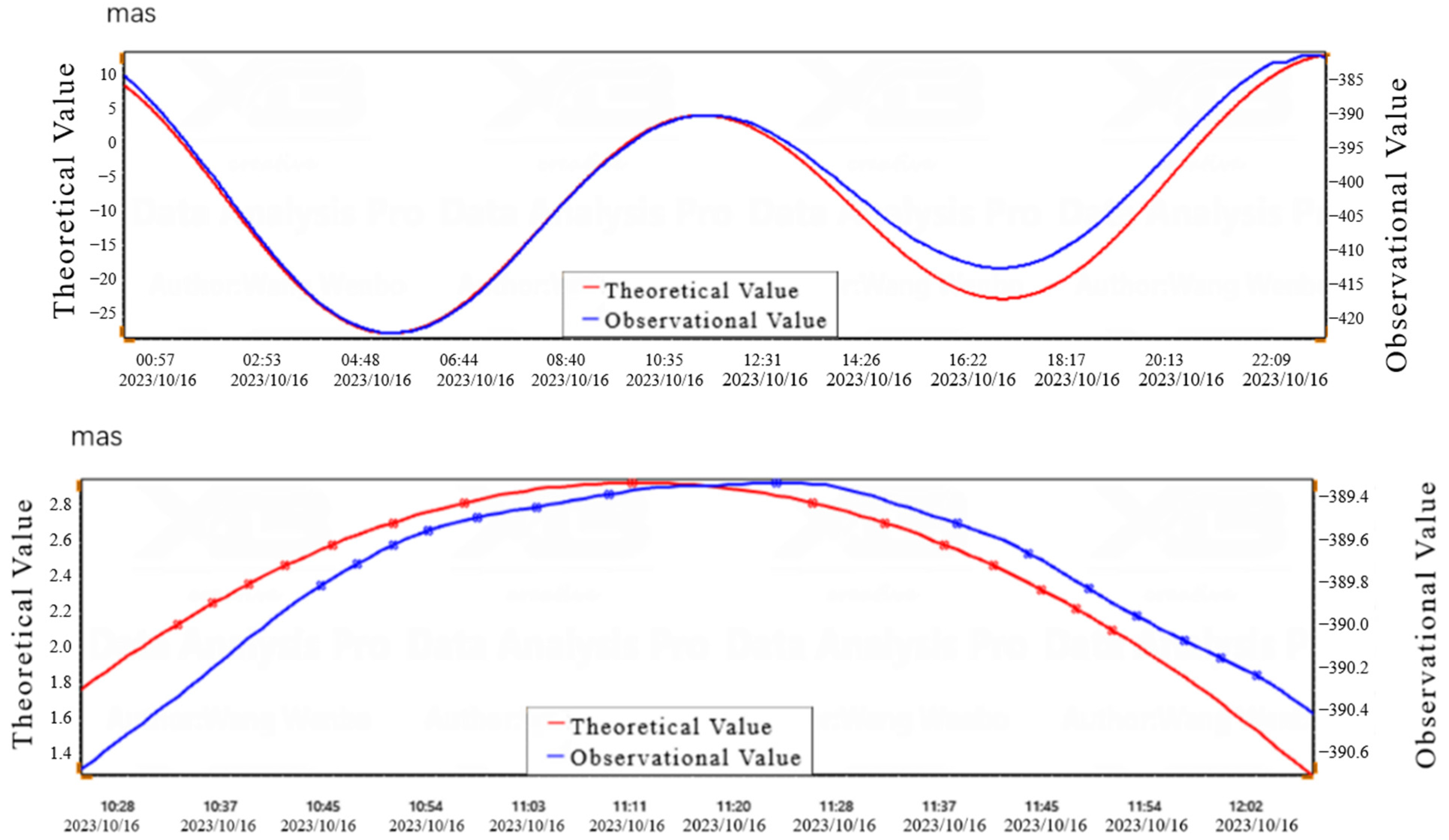
| No. | Tilt Amount (″) | Sensor Output (mV) | Fitted Value (mV) | Linear Deviation |
|---|---|---|---|---|
| 1 | 0.21859 | 35.555 | 33.075 | 2.4799 |
| 2 | 0.4169 | 67.731 | 65.97 | 1.761 |
| 3 | 0.62531 | 100.89 | 100.54 | 0.35175 |
| 4 | 0.81379 | 130.11 | 131.81 | 1.6982 |
| 5 | 1.0145 | 161.47 | 165.1 | 3.6355 |
| 6 | 1.2229 | 197.48 | 199.66 | 2.1857 |
| 7 | 1.4317 | 233.2 | 234.3 | 1.0987 |
| 8 | 1.6388 | 269.32 | 268.67 | 0.6571 |
| 9 | 1.8474 | 304.44 | 303.26 | 1.1824 |
| 10 | 2.0854 | 344.93 | 342.74 | 2.1859 |
| = 344.93 | d = 0.34% |
| No. | Tilt Amount (″) | Sensor Output (mV) | Fitted Value (mV) | Linear Deviation |
|---|---|---|---|---|
| 1 | 0.2202 | 37.2903 | 36.6965 | 0.5938 |
| 2 | 0.4116 | 68.638 | 69.0464 | 0.4084 |
| 3 | 0.6209 | 107.6002 | 104.4043 | 3.196 |
| 4 | 0.826 | 141.083 | 139.063 | 2.02 |
| 5 | 1.0348 | 173.3161 | 174.3381 | 1.022 |
| 6 | 1.2417 | 205.6679 | 209.2865 | 3.6186 |
| 7 | 1.4433 | 238.1662 | 243.3571 | 5.1909 |
| 8 | 1.6491 | 277.2836 | 278.1268 | 0.8433 |
| 9 | 1.852 | 313.1923 | 312.4131 | 0.7792 |
| 10 | 2.086 | 356.4319 | 351.9378 | 4.4942 |
| = 356.4319 | d = 0.47% |
| Strain Channel | Tidal Factor | Tidal Factor Mean Squared Error | Tidal Phase Lag | Tidal Phase Lag Mean Squared Error |
|---|---|---|---|---|
| CH1 (NS) | 0.3105 | 0.0023 | −1.5667 | −0.2473 |
| CH2 (EW) | 0.3918 | 0.0056 | −4.8036 | 0.2587 |
| N | Date | Theory Value | Time Interval | Obs Value | Norm Value | Fit Value | Difference |
|---|---|---|---|---|---|---|---|
| 16 October 2023 | dᵢ | Ƭᵢ | d′ᵢ | d″ᵢ | Δd″ᵢ | ||
| Time | 0.001″ | min | 0.001″ | 0.001″ | 0.001″ | 0.001″ | |
| −7 | 08:31 | −0.6423 | 41 | 396.0720 | 508.4365 | 508.5004 | 0.0639 |
| −6 | 08:34 | −0.5322 | 38 | 396.1450 | 508.5302 | 508.6201 | 0.0899 |
| −5 | 08:38 | −0.3981 | 34 | 396.2140 | 508.6187 | 508.7398 | 0.1210 |
| −4 | 08:42 | −0.2788 | 30 | 396.2920 | 508.7189 | 508.8595 | 0.1406 |
| −3 | 08:46 | −0.1742 | 26 | 396.3910 | 508.8460 | 508.9792 | 0.1332 |
| −2 | 08:51 | −0.0645 | 21 | 396.5200 | 509.0116 | 509.0989 | 0.0873 |
| −1 | 08:57 | 0.0362 | 15 | 396.6240 | 509.1451 | 509.2186 | 0.0735 |
| 0 | 09:12 | 0.1391 | 0 | 396.7730 | 509.3363 | 509.3383 | 0.0020 |
| 1 | 09:27 | 0.0291 | 15 | 396.6470 | 509.1746 | 509.2186 | 0.0440 |
| 2 | 09:33 | −0.0740 | 21 | 396.5930 | 509.1053 | 509.0989 | 0.0064 |
| 3 | 09:38 | −0.1853 | 26 | 396.5440 | 509.0424 | 508.9792 | 0.0632 |
| 4 | 09:42 | −0.2908 | 30 | 396.4830 | 508.9641 | 508.8595 | 0.1046 |
| 5 | 09:46 | −0.4109 | 34 | 396.4120 | 508.8729 | 508.7398 | 0.1331 |
| 6 | 09:50 | −0.5453 | 38 | 396.3310 | 508.7689 | 508.6201 | 0.1489 |
| 7 | 09:53 | −0.6553 | 41 | 396.2620 | 508.6804 | 508.5004 | 0.1800 |
| K | 1.2837 | Δd″max | 0.0883 | ||||
| N | Date | Theory Value | Time Interval | Obs Value | Norm Value | Fit Value | Difference |
|---|---|---|---|---|---|---|---|
| 16 October 2023 | dᵢ | Ƭᵢ | d′ᵢ | d″ᵢ | Δd″ᵢ | ||
| Time | 0.001″ | min | 0.001″ | 0.001″ | 0.001″ | 0.001″ | |
| −7 | 10:33 | 2.1379 | 38 | −389.8900 | −399.4775 | −399.7685 | 0.2910 |
| −6 | 10:36 | 2.2571 | 35 | −389.7870 | −399.3719 | −399.6451 | 0.2731 |
| −5 | 10:39 | 2.3668 | 32 | −389.6970 | −399.2797 | −399.5217 | 0.2419 |
| −4 | 10:42 | 2.4670 | 29 | −389.6250 | −399.2059 | −399.3983 | 0.1923 |
| −3 | 10:46 | 2.5856 | 25 | −389.5580 | −399.1373 | −399.2749 | 0.1376 |
| −2 | 10:51 | 2.7097 | 20 | −389.5100 | −399.0881 | −399.1515 | 0.0633 |
| −1 | 10:57 | 2.8229 | 14 | −389.4490 | −399.0256 | −399.0281 | 0.0024 |
| 0 | 11:11 | 2.9354 | 0 | −389.3910 | −398.9662 | −398.9047 | 0.0615 |
| 1 | 11:26 | 2.8212 | 15 | −389.5900 | −399.1701 | −399.0281 | 0.1420 |
| 2 | 11:32 | 2.7084 | 21 | −389.7340 | −399.3176 | −399.1515 | 0.1662 |
| 3 | 11:37 | 2.5856 | 26 | −389.9030 | −399.4908 | −399.2749 | 0.2159 |
| 4 | 11:41 | 2.4687 | 30 | −390.0410 | −399.6322 | −399.3983 | 0.2339 |
| 5 | 11:45 | 2.3355 | 34 | −390.1560 | −399.7500 | −399.5217 | 0.2283 |
| 6 | 11:48 | 2.2249 | 37 | −390.2440 | −399.8402 | −399.6451 | 0.1951 |
| 7 | 11:51 | 2.1054 | 40 | −390.3300 | −399.9283 | −399.7685 | 0.1598 |
| K | 1.0246 | Δd”max | 0.1657 | ||||
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2025 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Yang, F.; Guan, D.; Li, X.; Dou, C. Application Research on High-Precision Tiltmeter with Rapid Deployment Capability. Sensors 2025, 25, 1559. https://doi.org/10.3390/s25051559
Yang F, Guan D, Li X, Dou C. Application Research on High-Precision Tiltmeter with Rapid Deployment Capability. Sensors. 2025; 25(5):1559. https://doi.org/10.3390/s25051559
Chicago/Turabian StyleYang, Fuxi, Dongxiao Guan, Xiaodong Li, and Chen Dou. 2025. "Application Research on High-Precision Tiltmeter with Rapid Deployment Capability" Sensors 25, no. 5: 1559. https://doi.org/10.3390/s25051559
APA StyleYang, F., Guan, D., Li, X., & Dou, C. (2025). Application Research on High-Precision Tiltmeter with Rapid Deployment Capability. Sensors, 25(5), 1559. https://doi.org/10.3390/s25051559






