Hierarchical Multi-Scale Coupled Periodical Photonic and Plasmonic Nanopatterns Inscribed by Femtosecond Laser Pulses in Lithium Niobate
Abstract
:1. Introduction
2. Materials and Methods
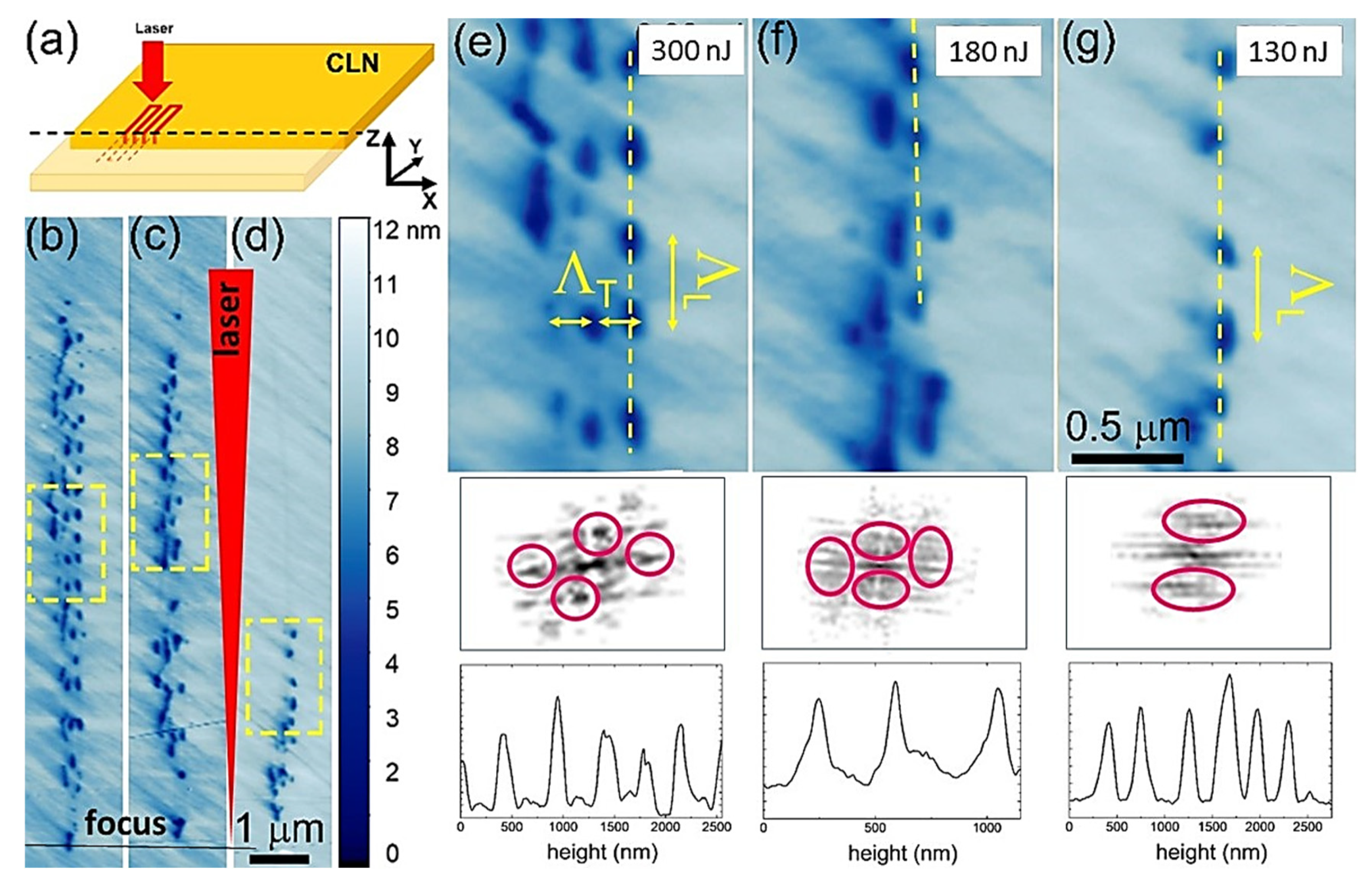
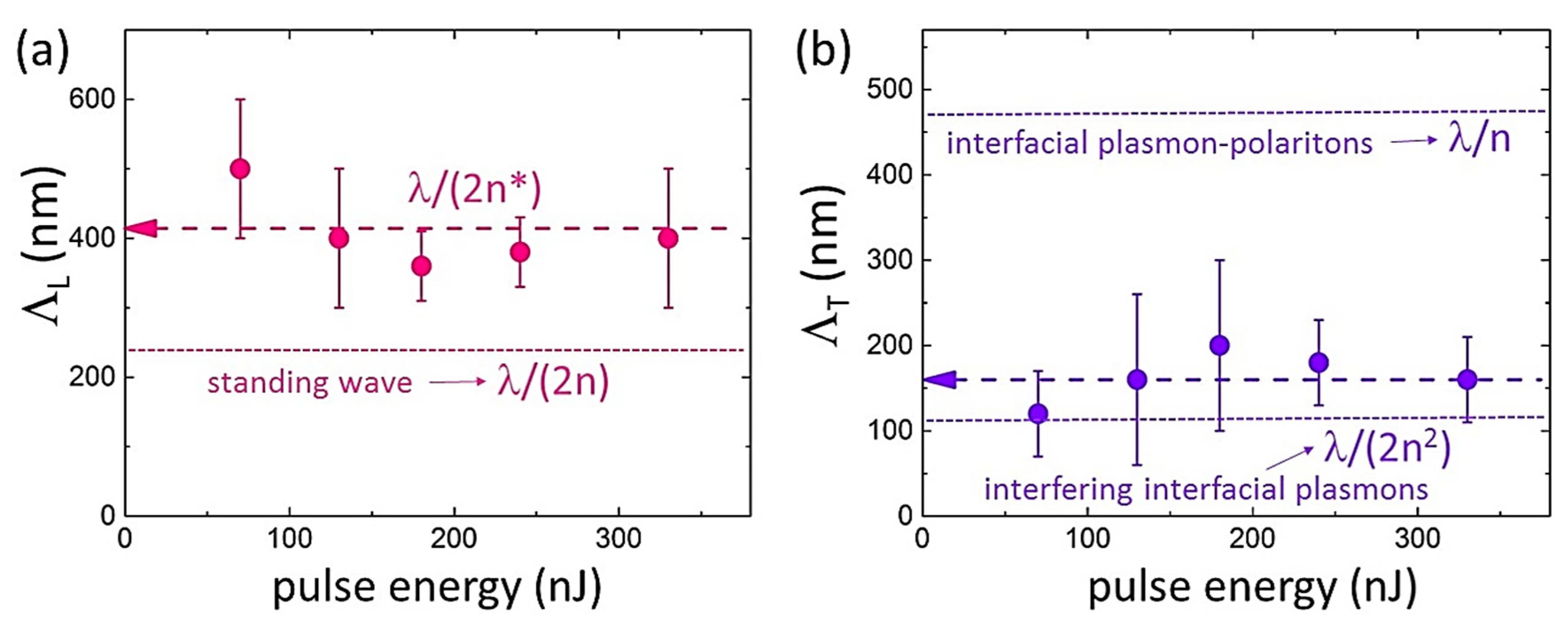
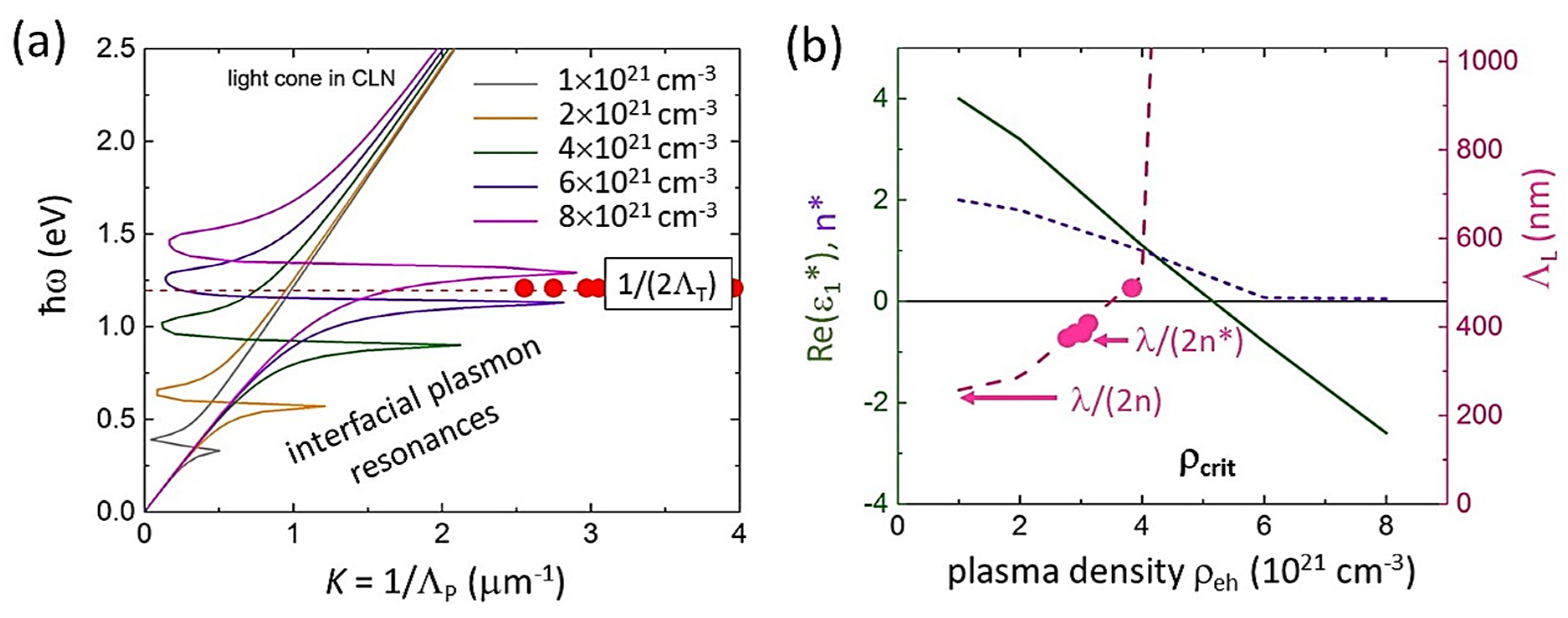
3. Experimental Results and Discussion
4. Conclusions
Supplementary Materials
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Canning, J.; Lancry, M.; Cook, K.; Weickman, A.; Brisset, F.; Poumellec, B. Anatomy of a femtosecond laser processed silica waveguide. Opt. Mater. Express 2011, 1, 998–1008. [Google Scholar] [CrossRef]
- Lin, Z.; Xu, J.; Song, Y.; Li, X.; Wang, P.; Chu, W.; Wang, Z.; Cheng, Y. Freeform Microfluidic Networks Encapsulated in Laser-Printed 3D Macroscale Glass Objects. Adv. Mater. Technol. 2019, 5, 1900989. [Google Scholar] [CrossRef]
- Ostendorf, A.; Chichkov, B.N. Two-photon polymerization: A new approach to micromachining. Photonics Spectra 2006, 40, 72. [Google Scholar]
- Hong, M.H.; Luk’yanchuk, B.; Huang, S.M.; Ong, T.S.; Van, L.H.; Chong, T.C. Femtosecond laser application for high capacity optical data storage. Appl. Phys. A 2004, 79, 791–794. [Google Scholar] [CrossRef]
- Juodkazis, S.; Nishimura, K.; Tanaka, S.; Misawa, H.; Gamaly, E.G.; Luther-Davies, B.; Hallo, L.; Nicolai, P.; Tikhonchuk, V.T. Laser-Induced Microexplosion Confined in the Bulk of a Sapphire Crystal: Evidence of Multimegabar Pressures. Phys. Rev. Lett. 2006, 96, 166101. [Google Scholar] [CrossRef] [Green Version]
- Shimotsuma, Y.; Kazansky, P.G.; Qiu, J.; Hirao, K. Self-organized nanogratings in glass irradiated by ultrashort light pulses. Phys. Rev. Lett. 2003, 91, 247405. [Google Scholar] [CrossRef] [Green Version]
- Desmarchelier, R.; Poumellec, B.; Brisset, F.; Mazerat, S.; Lancry, M. In the heart of femtosecond laser induced nanogratings: From porous nanoplanes to form birefringence. World J. Nano Sci. Eng. 2015, 5, 115–125. [Google Scholar] [CrossRef] [Green Version]
- Zhang, B.; Liu, X.; Qiu, J. Single femtosecond laser beam induced nanogratings in transparent media—Mechanisms and applications. J. Mater. 2019, 5, 1–14. [Google Scholar] [CrossRef]
- Davis, K.M.; Miura, K.; Sugimoto, N.; Hirao, K. Writing waveguides in glass with a femtosecond laser. Opt. Lett. 1996, 21, 1729–1731. [Google Scholar] [CrossRef]
- Hwang, D.J.; Choi, T.Y.; Grigoropoulos, C.P. Liquid-assisted femtosecond laser drilling of straight and three-dimensional microchannels in glass. Appl. Phys. A 2004, 79, 605–612. [Google Scholar] [CrossRef]
- Sakakura, M.; Lei, Y.; Wang, L.; Yu, Y.H.; Kazansky, P.G. Ultralow-loss geometric phase and polarization shaping by ultrafast laser writing in silica glass. Light Sci. Appl. 2020, 9, 15. [Google Scholar] [CrossRef] [PubMed]
- Xu, S.; Fan, H.; Li, Z.-Z.; Hua, J.-G.; Yu, Y.-H.; Wang, L.; Chen, Q.-D.; Sun, H.-B. Ultrafast laser-inscribed nanogratings in sapphire for geometric phase elements. Opt. Lett. 2021, 46, 536–539. [Google Scholar] [CrossRef] [PubMed]
- Kudryashov, S.I.; Danilov, P.A.; Rupasov, A.E.; Smayev, M.P.; Smirnov, N.A.; Kesaev, V.V.; Putilin, A.N.; Kovalev, M.S.; Zakoldaev, R.A.; Gonchukov, S.A. Direct laser writing regimes for bulk inscription of polarization-based spectral microfilters and fabrication of microfluidic bio/chemosensor in bulk fused silica. Laser Phys. Lett. 2022, 19, 065602. [Google Scholar] [CrossRef]
- Kudryashov, S.I.; Danilov, P.A.; Smaev, M.P.; Rupasov, A.E.; Zolot’ko, A.S.; Ionin, A.A.; Zakoldaev, R.A. Generation of an Array of Birefringent Nanogratings in the Bulk of Fluorite Irradiated by Ultrashort Laser Pulses with Different Durations. JETP Lett. 2021, 113, 493–497. [Google Scholar] [CrossRef]
- Kudryashov, S.I.; Danilov, P.A.; Rupasov, A.E.; Smayev, M.P.; Kirichenko, A.N.; Smirnov, N.A.; Ionin, A.A.; Zolot’ko, A.S.; Zakoldaev, R.A. Birefringent microstructures in bulk fluorite produced by ultrafast pulsewidth-dependent laser inscription. Appl. Surf. Sci. 2021, 568, 150877. [Google Scholar] [CrossRef]
- Kudryashov, S.; Rupasov, A.; Zakoldaev, R.; Smaev, M.; Kuchmizhak, A.; Zolot’Ko, A.; Kosobokov, M.; Akhmatkhanov, A.; Shur, V. Nanohydrodynamic Local Compaction and Nanoplasmonic Form-Birefringence Inscription by Ultrashort Laser Pulses in Nanoporous Fused Silica. Nanomaterials 2022, 12, 3613. [Google Scholar] [CrossRef] [PubMed]
- Kanehira, S.; Si, J.; Qiu, J.; Fujita, K.; Hirao, K. Periodic nanovoid structures via femtosecond laser irradiation. Nano Lett. 2005, 5, 1591–1595. [Google Scholar] [CrossRef]
- Song, J.; Wang, X.; Hu, X.; Dai, Y.; Qiu, J.; Cheng, Y.; Xu, Z. Formation mechanism of self-organized voids in dielectrics induced by tightly focused femtosecond laser pulses. Appl. Phys. Lett. 2008, 92, 092904. [Google Scholar] [CrossRef]
- Kudryashov, S.I.; Nastulyavichus, A.A.; Saraeva, I.N.; Rudenko, A.A.; Zayarny, D.A.; Ionin, A.A. Deeply sub-wavelength laser nanopatterning of Si surface in dielectric fluids: Manipulation by surface plasmon resonance. Appl. Surf. Sci. 2020, 519, 146204. [Google Scholar] [CrossRef]
- Déziel, J.L.; Dubé, L.J.; Messaddeq, S.H.; Messaddeq, Y.; Varin, C. Femtosecond self-reconfiguration of laser-induced plasma patterns in dielectrics. Phys. Rev. B 2018, 97, 205116. [Google Scholar] [CrossRef]
- Danilov, P.A.; Ionin, A.A.; Kudryashov, S.I.; Makarov, S.V.; Rudenko, A.A.; Saltuganov, P.N.; Seleznev, L.V.; Yurovskikh, V.I.; Zayarny, D.A.; Apostolova, T. Silicon as a virtual plasmonic material: Acquisition of its transient optical constants and the ultrafast surface plasmon-polariton excitation. J. Exp. Theor. Phys. 2015, 120, 946–959. [Google Scholar] [CrossRef]
- Li, X.; Xu, J.; Lin, Z.; Qi, J.; Wang, P.; Chu, W.; Fang, Z.; Wang, Z.; Chai, Z.; Cheng, Y. Polarization-insensitive space-selective etching in fused silica induced by picosecond laser irradiation. Appl. Surf. Sci. 2019, 485, 188–193. [Google Scholar] [CrossRef] [Green Version]
- Kudryashov, S.; Krasin, G.; Rupasov, A.; Danilov, P.; Kosobokov, M.; Akhmatkhanov, A.; Lisjikh, B.; Turygin, A.; Greshnyakov, E.; Kovalev, M.; et al. Ferroelectric nanodomain engineering in bulk lithium niobate crystals in ultrashort-pulse laser nanopatterning regime. Nanomaterials 2022, 12, 4147. [Google Scholar] [CrossRef]
- White, T.J.; Natarajan, L.V.; Tondiglia, V.P.; Lloyd, P.F.; Bunning, T.J.C.; Guymon, A. Holographic polymer dispersed liquid crystals (HPDLCs) containing triallyl isocyanurate monomer. Polymer 2007, 48, 5979–5987. [Google Scholar] [CrossRef]
- Shalit, A.; Lucchetta, D.E.; Piazza, V.; Simoni, F.; Bizzarri, R.; Castagna, R. Polarization-dependent laser-light structured directionality with polymer composite materials. Mater. Lett. 2012, 81, 232–234. [Google Scholar] [CrossRef]
- Castagna, R.; Lucchetta, D.E.; Rippa, M.; Xu, J.H.; Di Donato, A. Near-frequency photons Y-splitter. Appl. Mater. Today 2020, 19, 100636. [Google Scholar] [CrossRef]
- Anuja, K.-J.; Deshmukh, R.R. An overview of HPDLC films and their applications. Liq. Cryst. 2022, 49, 589–604. [Google Scholar]
- Palik, E.D. Handbook of Optical Constants of Solids; Academic Press: Orlando, FL, USA, 1998. [Google Scholar]
- Sokolowski-Tinten, K.; von der Linde, D. Generation of dense electron-hole plasmas in silicon. Phys. Rev. B 2000, 61, 2643–2650. [Google Scholar] [CrossRef] [Green Version]
- Danilov, P.A.; Kudryashov, S.I.; Rupasov, A.E.; Smirnov, N.A.; Oleynichuk, E.A.; Rivnyuk, A.S.; Zakoldaev, R.A. Formation of Nanogratings on the Surface of Nanoporous Glass Irradiated by Femtosecond Visible Laser Pulses. JETP Lett. 2021, 113, 622–625. [Google Scholar] [CrossRef]
- Raether, H. Surface Plasmons on Smooth and Rough Surfaces and on Gratings; Springer: Berlin/Heidelberg, Germany, 1988. [Google Scholar]
- Bell, R.J.; Alexander, R.W., Jr.; Parks, W.F.; Kovener, G. Surface excitations in absorbing media. Opt. Commun. 1973, 8, 147–150. [Google Scholar] [CrossRef]
- Ionin, A.A.; Kudryashov, S.I.; Makarov, S.V.; Rudenko, A.A.; Saltuganov, P.N.; Seleznev, L.V.; Sinitsyn, D.V.; Sunchugasheva, E.S. Femtosecond laser fabrication of sub-diffraction nanoripples on wet Al surface in multi-filamentation regime: High optical harmonics effects? Appl. Surf. Sci. 2014, 292, 678–681. [Google Scholar] [CrossRef]
- Shinoda, M.; Gattass, R.R.; Mazur, E. Femtosecond laser-induced formation of nanometer-width grooves on synthetic single-crystal diamond surfaces. J. Appl. Phys. 2009, 105, 053102. [Google Scholar] [CrossRef]
- Rebollar, E.; de Aldana, J.R.V.; Pérez-Hernández, J.A.; Ezquerra, T.A.; Moreno, P.; Castillejo, M. Ultraviolet and infrared femtosecond laser induced periodic surface structures on thin polymer films. Appl. Phys. Lett. 2012, 100, 041106. [Google Scholar] [CrossRef] [Green Version]
- Buividas, R.; Rosa, L.; Šliupas, R.; Kudrius, T.; Šlekys, G.; Datsyuk, V.; Juodkazis, S. Mechanism of fine ripple formation on surfaces of (semi) transparent materials via a half-wavelength cavity feedback. Nanotechnology 2010, 22, 055304. [Google Scholar] [CrossRef]
- Kudryashov, S.I.; Nguyen, L.V.; Kirilenko, D.A.; Brunkov, P.N.; Rudenko, A.A.; Busleev, N.I.; Shakhmin, A.L.; Semencha, A.V.; Khmelnitsky, R.A.; Melnik, N.N.; et al. Large-scale laser fabrication of anti-fouling Si surface nanosheet arrays via nanoplasmonic ablative self-organization in liquid CS2 tracked by sulfur dopant. ACS Appl. Nano Mater. 2018, 1, 2461–2468. [Google Scholar] [CrossRef]
- Kudryashov, S.I.; Makarov, S.V.; Ionin, A.A.; Nathala, C.S.R.; Ajami, A.; Ganz, T.; Assion, A.; Husinsky, W. Dynamic polarization flip in nanoripples on photoexcited Ti surface near its surface plasmon resonance. Opt. Lett. 2015, 40, 4967–4970. [Google Scholar] [CrossRef] [PubMed]
- Gottmann, J.D.; Wortmann, M. Horstmann-Jungemann, Fabrication of sub-wavelength surface ripples and in-volume nanostructures by fs-laser induced selective etching. Appl. Surf. Sci. 2009, 255, 5641–5646. [Google Scholar] [CrossRef]

Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Kudryashov, S.; Rupasov, A.; Kosobokov, M.; Akhmatkhanov, A.; Krasin, G.; Danilov, P.; Lisjikh, B.; Abramov, A.; Greshnyakov, E.; Kuzmin, E.; et al. Hierarchical Multi-Scale Coupled Periodical Photonic and Plasmonic Nanopatterns Inscribed by Femtosecond Laser Pulses in Lithium Niobate. Nanomaterials 2022, 12, 4303. https://doi.org/10.3390/nano12234303
Kudryashov S, Rupasov A, Kosobokov M, Akhmatkhanov A, Krasin G, Danilov P, Lisjikh B, Abramov A, Greshnyakov E, Kuzmin E, et al. Hierarchical Multi-Scale Coupled Periodical Photonic and Plasmonic Nanopatterns Inscribed by Femtosecond Laser Pulses in Lithium Niobate. Nanomaterials. 2022; 12(23):4303. https://doi.org/10.3390/nano12234303
Chicago/Turabian StyleKudryashov, Sergey, Alexey Rupasov, Mikhail Kosobokov, Andrey Akhmatkhanov, George Krasin, Pavel Danilov, Boris Lisjikh, Alexander Abramov, Evgeny Greshnyakov, Evgeny Kuzmin, and et al. 2022. "Hierarchical Multi-Scale Coupled Periodical Photonic and Plasmonic Nanopatterns Inscribed by Femtosecond Laser Pulses in Lithium Niobate" Nanomaterials 12, no. 23: 4303. https://doi.org/10.3390/nano12234303
APA StyleKudryashov, S., Rupasov, A., Kosobokov, M., Akhmatkhanov, A., Krasin, G., Danilov, P., Lisjikh, B., Abramov, A., Greshnyakov, E., Kuzmin, E., Kovalev, M., & Shur, V. (2022). Hierarchical Multi-Scale Coupled Periodical Photonic and Plasmonic Nanopatterns Inscribed by Femtosecond Laser Pulses in Lithium Niobate. Nanomaterials, 12(23), 4303. https://doi.org/10.3390/nano12234303










