Dynamic Analysis and Experiment of 6-DOF Compliant Platform Based on Bridge-Type Amplifier
Abstract
:1. Introduction
2. Dynamic Modeling
2.1. Platform Description
2.2. Dynamic Model of Bridge-Type Amplifier
2.3. Dynamic Model of Platform
3. FEA Verification
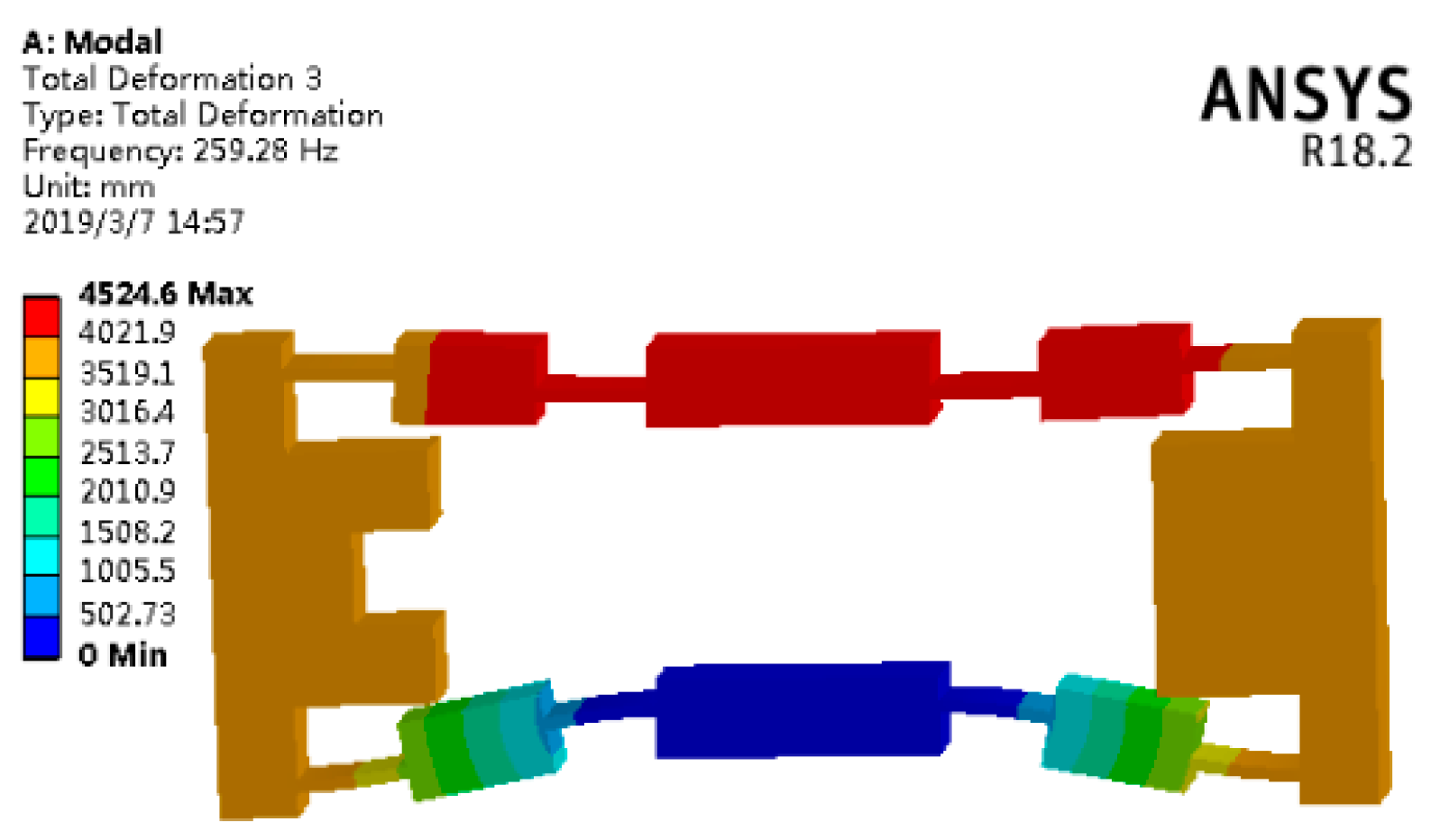
3.1. Natural Frequency Verification of Bridge-type Amplifier
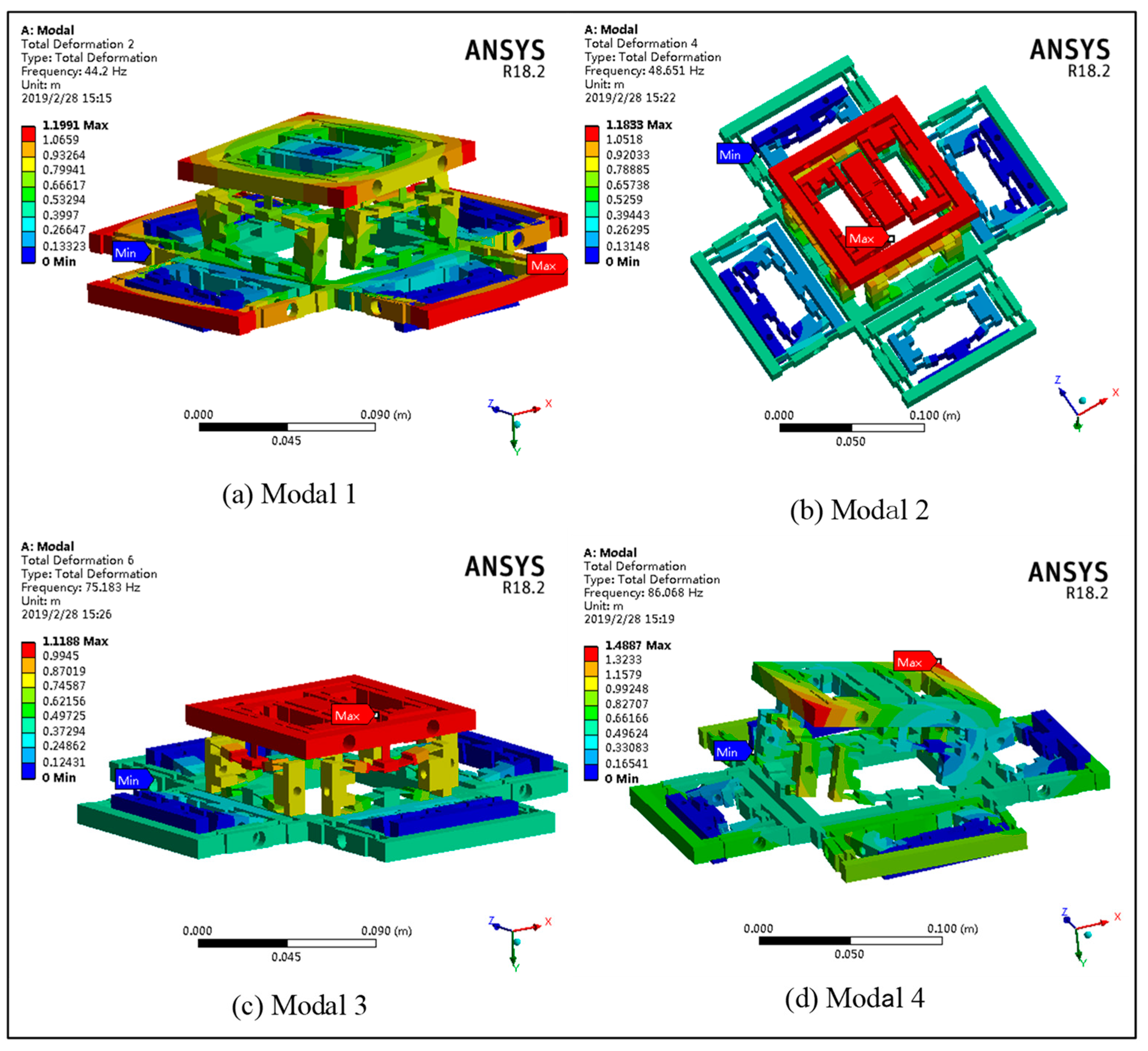
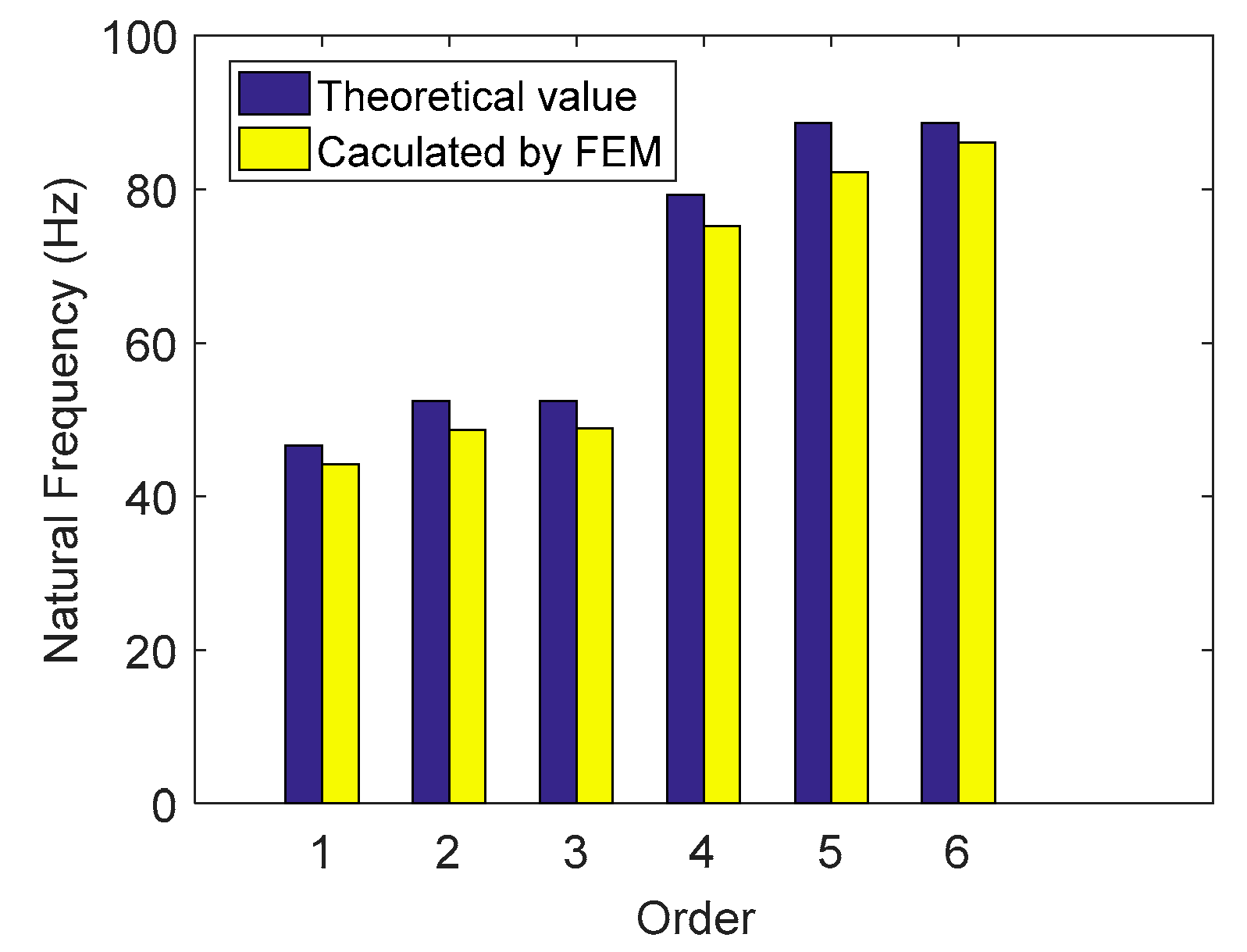
3.2. Natural Frequency Verification of Platform
4. Experimental Verification
4.1. Modal Experiment
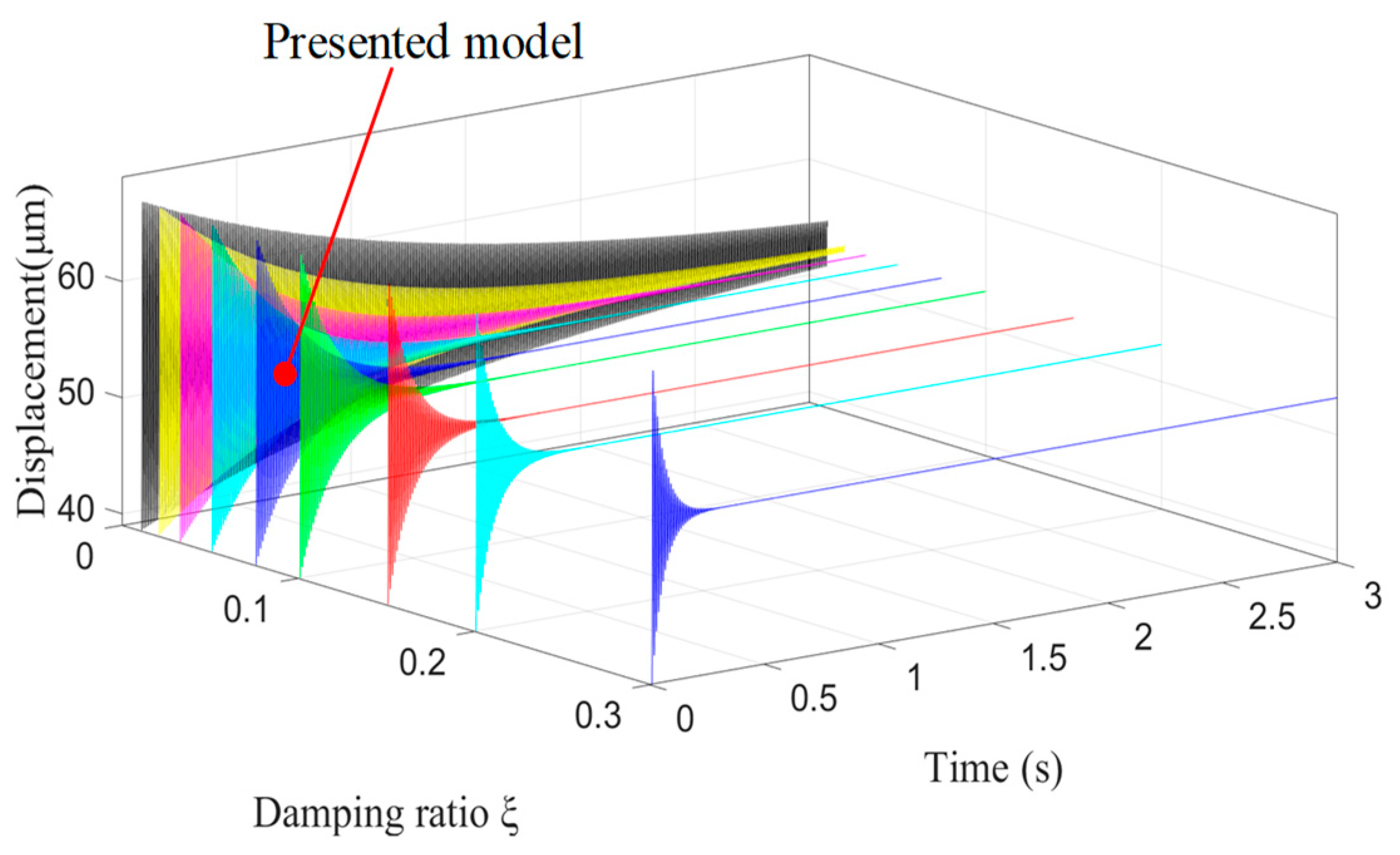
4.2. Step Response Experiment
5. Conclusions
Author Contributions
Funding
Conflicts of Interest
References
- Mallick, R.; Ganguli, R.; Bhat, M.S. A feasibility study of a post-buckled beam for actuating helicopter trailing edge flap. Acta Mech. 2014, 225, 2783–2787. [Google Scholar] [CrossRef]
- Xi, X.; Clancy, T.; Wu, X.; Sun, Y.; Liu, X. A MEMSXY-stage integrating compliant mechanism for nanopositioning at sub-nanometer resolution. J. Micromech. Microeng. 2016, 26, 025014. [Google Scholar] [CrossRef] [Green Version]
- Kim, J.-J.; Choi, Y.-M.; Ahn, D.; Hwang, B.; Gweon, D.-G.; Jeong, J. A millimeter-range flexure-based nano-positioning stage using a self-guided displacement amplification mechanism. Mech. Mach. Theory 2012, 50, 109–120. [Google Scholar] [CrossRef]
- Ma, H.-W.; Yao, S.-M.; Wang, L.-Q.; Zhong, Z. Analysis of the displacement amplification ratio of bridge-type flexure hinge. Sens. Actuators A Phys. 2006, 132, 730–736. [Google Scholar] [CrossRef]
- Zelenika, S.; Munteanu, M.G.; De Bona, F. Optimized flexural hinge shapes for microsystems and high-precision applications. Mech. Mach. Theory 2009, 44, 1826–1839. [Google Scholar] [CrossRef]
- Dechev, N.; Ren, L.; Liu, W.; Cleghorn, W.L.; Mills, J.K. Development of a 6-degree of freedom robotic micromanipulator for use in 3DMEMS micro assembly. In Proceedings of the 2006 IEEE International Conference on Robotics and Automation, Orlando, FL, USA, 15–19 May 2006; pp. 281–288. [Google Scholar]
- Kang, D.; Gweon, D. Development of flexure based 6-degrees of freedom parallel nano-positioning system with large displacement. Rev. Sci. Instrum. 2012, 83, 035003. [Google Scholar] [CrossRef] [PubMed]
- Tang, H.; Li, Y. A New Flexure-Based Yθ Nanomanipulator With Nanometer-Scale Resolution and Millimeter-Scale Workspace. IEEE/ASME Trans. Mechatron. 2014, 20, 1320–1330. [Google Scholar] [CrossRef]
- Qi, K.-Q.; Xiang, Y.; Fang, C.; Zhang, Y.; Yu, C.-S. Analysis of the displacement amplification ratio of bridge-type mechanism. Mech. Mach. Theory 2015, 87, 45–56. [Google Scholar] [CrossRef]
- Chen, J.; Zhang, C.; Xu, M.; Zi, Y.; Zhang, X. Rhombic micro-displacement amplifier for piezoelectric actuator and its linear and hybrid model. Mech. Syst. Signal. Process. 2015, 50, 580–593. [Google Scholar] [CrossRef]
- Tian, Y.; Shirinzadeh, B.; Zhang, D.; Alici, G. Development and dynamic modelling of a flexure-based Scott–Russell mechanism for nano-manipulation. Mech. Syst. Signal. Process. 2009, 23, 957–978. [Google Scholar] [CrossRef]
- Qu, J.; Chen, W.; Zhang, J.; Chen, W. A large-range compliant micropositioning stage with remote-center-of-motion characteristic for parallel alignment. Microsyst. Technol. 2016, 22, 777–789. [Google Scholar] [CrossRef]
- Jiang, Y.; Li, T.-M.; Wang, L.-P. Stiffness modeling of compliant parallel mechanisms and applications in the performance analysis of a decoupled parallel compliant stage. Rev. Sci. Instrum. 2015, 86, 095109. [Google Scholar] [CrossRef] [PubMed]
- Kenton, B.J.; Leang, K.K. Design and Control of a Three-Axis Serial-Kinematic High-Bandwidth Nanopositioner. IEEE/ASME Trans. Mechatron. 2011, 17, 356–369. [Google Scholar] [CrossRef]
- Zhu, W.-L.; Zhu, Z.; Guo, P.; Ju, B.-F. A novel hybrid actuation mechanism based XY nanopositioning stage with totally decoupled kinematics. Mech. Syst. Signal. Process. 2018, 99, 747–759. [Google Scholar] [CrossRef]
- Kim, H.; Kim, J.; Ahn, D.; Gweon, D. Development of a Nanoprecision 3-DOF Vertical Positioning System With a Flexure Hinge. IEEE Trans. Nanotechnol. 2013, 12, 234–245. [Google Scholar] [CrossRef]
- Cai, K.; Tian, Y.; Liu, X.; Fatikow, S.; Wang, F.; Cui, L.; Zhang, D.; Shirinzadeh, B. Modeling and controller design of a 6-DOF precision positioning system. Mech. Syst. Signal. Process. 2018, 104, 536–555. [Google Scholar] [CrossRef] [Green Version]
- Lin, C.; Shen, Z.; Wu, Z.; Yu, J. Kinematic characteristic analysis of a micro-/nano positioning stage based on bridge-type amplifier. Sens. Actuators A Phys. 2018, 271, 230–242. [Google Scholar]
- Shen, Y.; Luo, X.; Wang, S.; Li, X. Dynamic Analysis of a 5-DOF Flexure-Based Nanopositioning Stage. Math. Probl. Eng. 2019, 2019, 1–11. [Google Scholar] [CrossRef]
- Du, Y.; Li, T.; Gao, G. Dynamic analysis of a flexure-based compliant stage. J. Mech. Sci. Technol. 2018, 32, 5223–5231. [Google Scholar] [CrossRef]
- Hongzhe, Z.; Shusheng, B.; Bo, P. Dynamic analysis and experiment of a novel ultra-precision compliant linear-motion mechanism. Precis. Eng. 2015, 42, 352–359. [Google Scholar] [CrossRef]
- Xu, Q.; Li, Y. Analytical modeling, optimization and testing of a compound bridge-type compliant displacement amplifier. Mech. Mach. Theory 2011, 46, 183–200. [Google Scholar]
- Dao, T.-P.; Huang, S.-C. Design and analysis of a compliant micro-positioning platform with embedded strain gauges and viscoelastic damper. Microsyst. Technol. 2016, 23, 441–456. [Google Scholar] [CrossRef]
- Ling, M.; Howell, L.; Cao, J.; Jiang, Z. A pseudo-static model for dynamic analysis on frequency domain of distributed compliant mechanisms. J. Mech. Robot. 2018, 10, 051011. [Google Scholar]
- Ling, M.; Chen, S.; Li, Q.; Tian, G. Dynamic stiffness matrix for free vibration analysis of flexure hinges based on non-uniform Timoshenko beam. J. Sound Vib. 2018, 437, 40–52. [Google Scholar]
- Ling, M.; Cao, J.; Pehrson, N. Kinetostatic and dynamic analyses of planar compliant mechanisms with a two-port dynamic stiffness model. Precis. Eng. 2019, 57, 149–161. [Google Scholar]
- Yu, J.; Lu, D.; Hao, G. Design and analysis of a compliant parallel pan-tilt platform. Meccanica 2016, 51, 1559–1570. [Google Scholar]
- Gu, Y.; Chen, X.; Lu, F.; Lin, J.; Yi, A.; Feng, J.; Sun, Y. Development of a Novel Three Degrees-of-Freedom Rotary Vibration-Assisted Micropolishing System Based on Piezoelectric Actuation. Micromachines 2019, 10, 502. [Google Scholar]
- Lin, C.; Yu, S.; Cheng, K.; Cui, X.; Tao, Y.; Wang, J. Analysis and experiment of dynamic characteristics of micro/nano positioning platform. J. Zhejiang Univ. Eng. Science 2012, 46, 1375–1381. [Google Scholar]
- Ye, G.; Li, W.; Wang, Y.Q. Analysis of guiding displacement of parallel four-bar mechanism with right angle flexible hinge. J. Chi. Univ. Min. Technol. 2010, 39, 254–258. [Google Scholar]

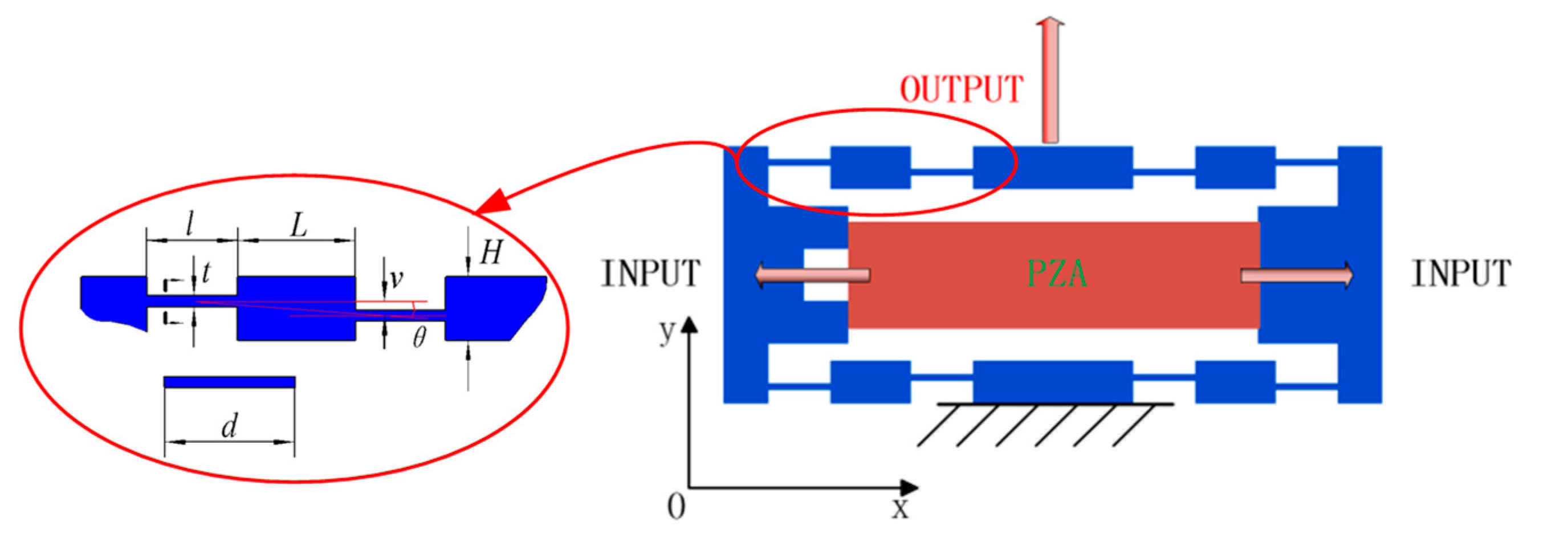
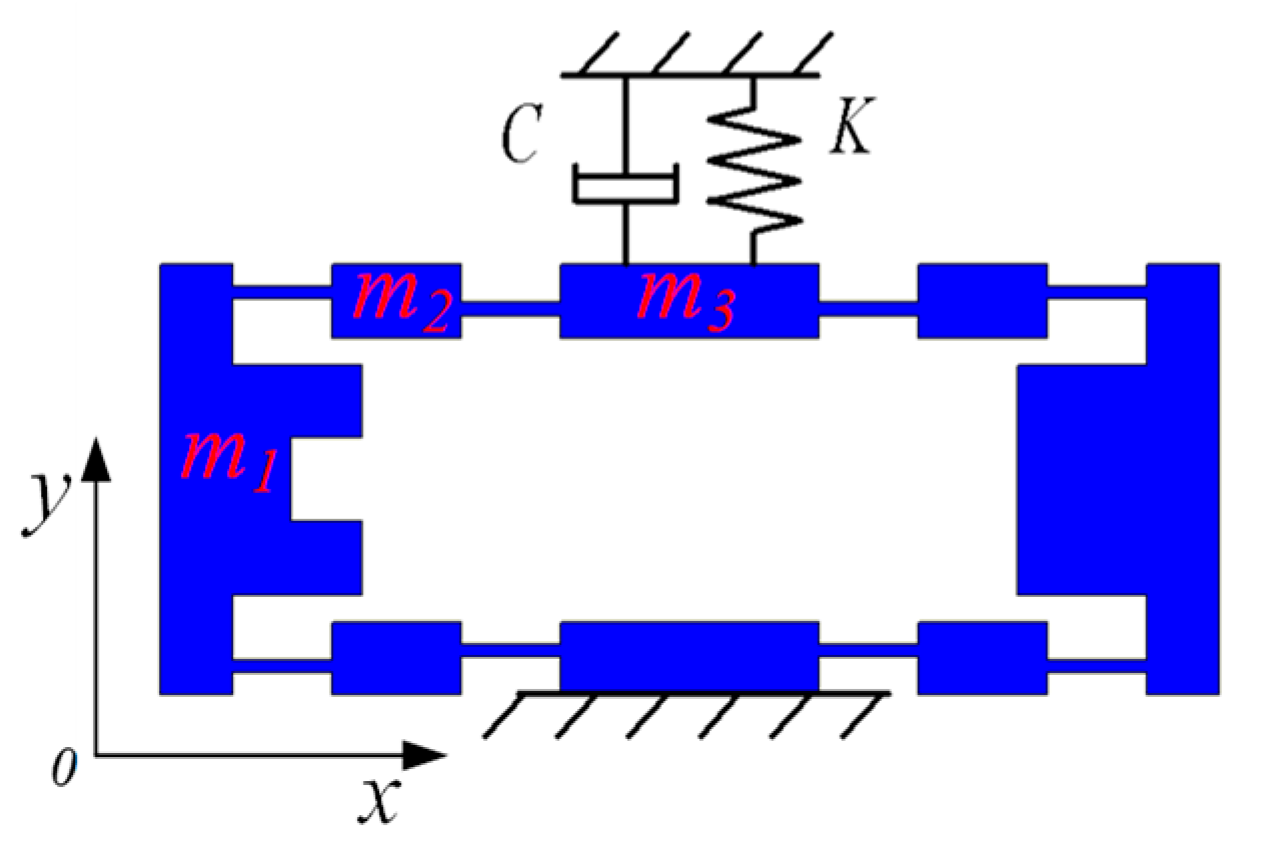
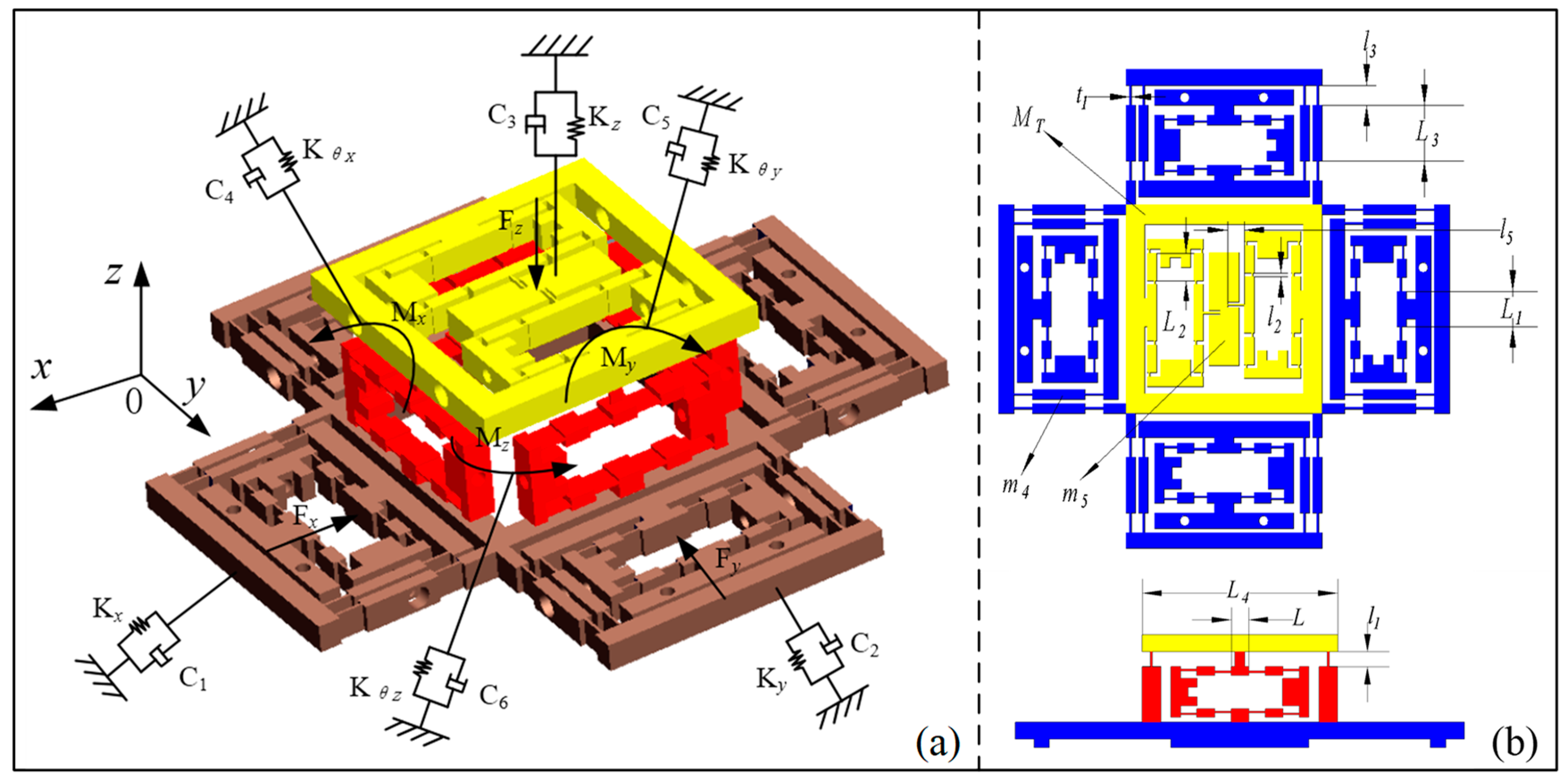

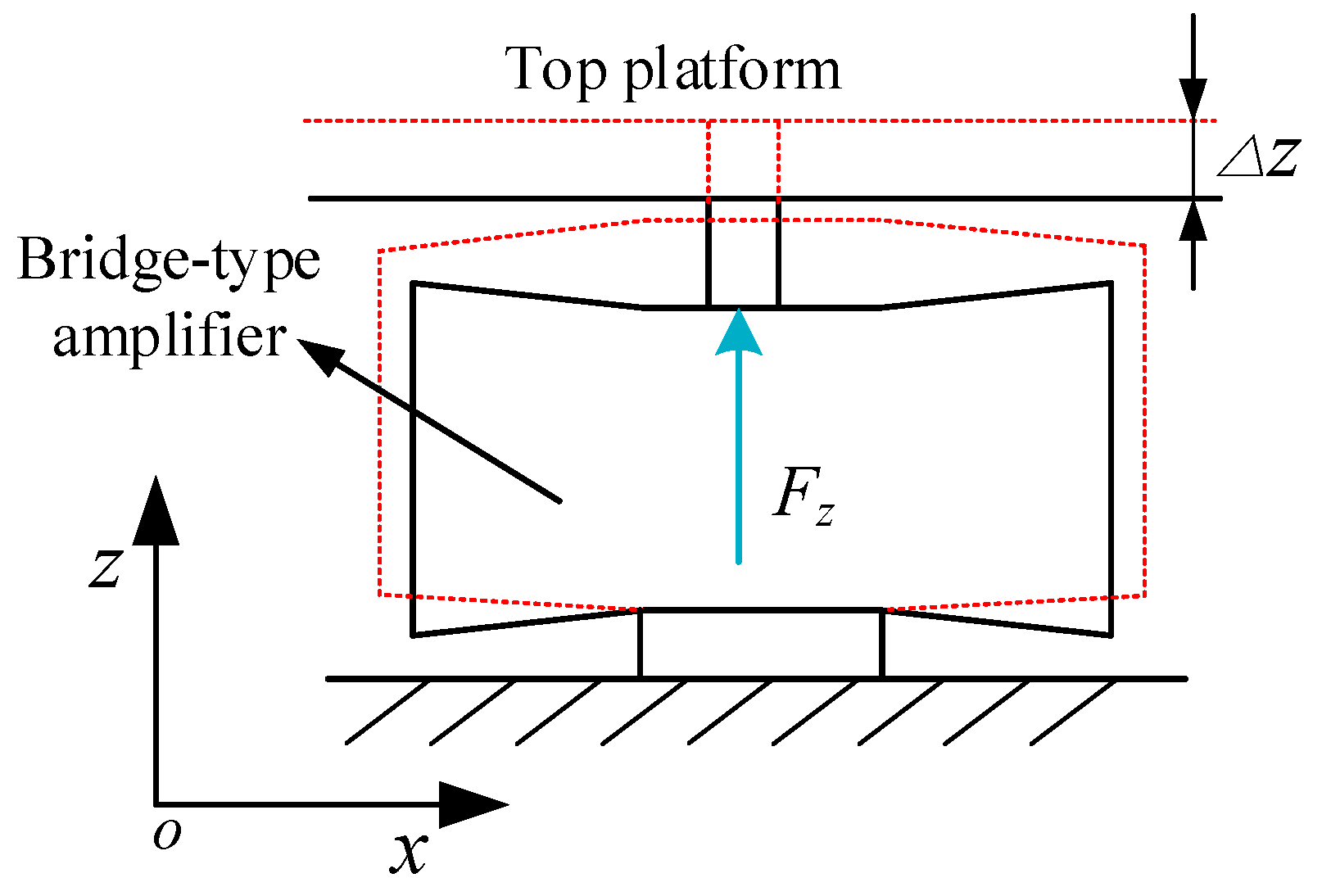






| Parameters | L/mm | l/mm | v/mm | t/mm | d/mm | E/GPa |
|---|---|---|---|---|---|---|
| Value | 9 | 7 | 1.2 | 0.8 | 10 | 210 |
| Parameters | L1 | L2 | L3 | l3 | l5 | B | R |
|---|---|---|---|---|---|---|---|
| Value/mm | 18 | 14 | 28 | 10 | 9 | 16 | 4 |
| Order | Natural frequency fn/Hz | Mode of vibration | ||
|---|---|---|---|---|
| Analytical | FEA | Error/% | ||
| 1 | 46.637 | 44.2 | 5.51 | Rotation along Z axis |
| 2 | 52.421 | 48.651 | 7.75 | Translation along X axis |
| 3 | 52.420 | 48.828 | 7.36 | Translation along Y axis |
| 4 | 79.246 | 75.183 | 5.40 | Translation along Z axis |
| 5 | 88.598 | 82.178 | 7.81 | Rotation along X axis |
| 6 | 88.598 | 86.068 | 2.94 | Rotation along Y axis |
| Order | Natural frequency fn/Hz | Damping ratio ξ/% | ||
|---|---|---|---|---|
| Theory | Experiment | Error/% | ||
| 1 | 46.637 | 41.2 | 11.66 | 0.28 |
| 2 | 52.421 | 46.8 | 10.72 | 0.73 |
| 3 | 52.420 | 48.4 | 7.671 | 0.86 |
| 4 | 79.246 | 70.8 | 10.66 | 0.16 |
| 5 | 88.598 | 80.2 | 9.479 | 0.79 |
| 6 | 88.598 | 82.1 | 7.334 | 0.34 |
| Directions | Step output displacement Sp/μm | Settling time ts/s | ||||
|---|---|---|---|---|---|---|
| Theory | Experiment | Error | Theory | Experiment | Error | |
| X/Y | 68.01 | 58.94 | 13.34% | 1.43 | 1.62 | 13.29% |
| Z | 65.72 | 58.75 | 10.61% | 1.31 | 1.54 | 17.55% |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2020 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Lin, C.; Zheng, S.; Jiang, M. Dynamic Analysis and Experiment of 6-DOF Compliant Platform Based on Bridge-Type Amplifier. Micromachines 2020, 11, 1024. https://doi.org/10.3390/mi11111024
Lin C, Zheng S, Jiang M. Dynamic Analysis and Experiment of 6-DOF Compliant Platform Based on Bridge-Type Amplifier. Micromachines. 2020; 11(11):1024. https://doi.org/10.3390/mi11111024
Chicago/Turabian StyleLin, Chao, Shan Zheng, and Mingdong Jiang. 2020. "Dynamic Analysis and Experiment of 6-DOF Compliant Platform Based on Bridge-Type Amplifier" Micromachines 11, no. 11: 1024. https://doi.org/10.3390/mi11111024
APA StyleLin, C., Zheng, S., & Jiang, M. (2020). Dynamic Analysis and Experiment of 6-DOF Compliant Platform Based on Bridge-Type Amplifier. Micromachines, 11(11), 1024. https://doi.org/10.3390/mi11111024





