Microlens Fabrication by Replica Molding of Electro-Hydrodynamic Printing Liquid Mold
Abstract
:1. Introduction
2. Materials and Methods
2.1. Chemicals and Materials
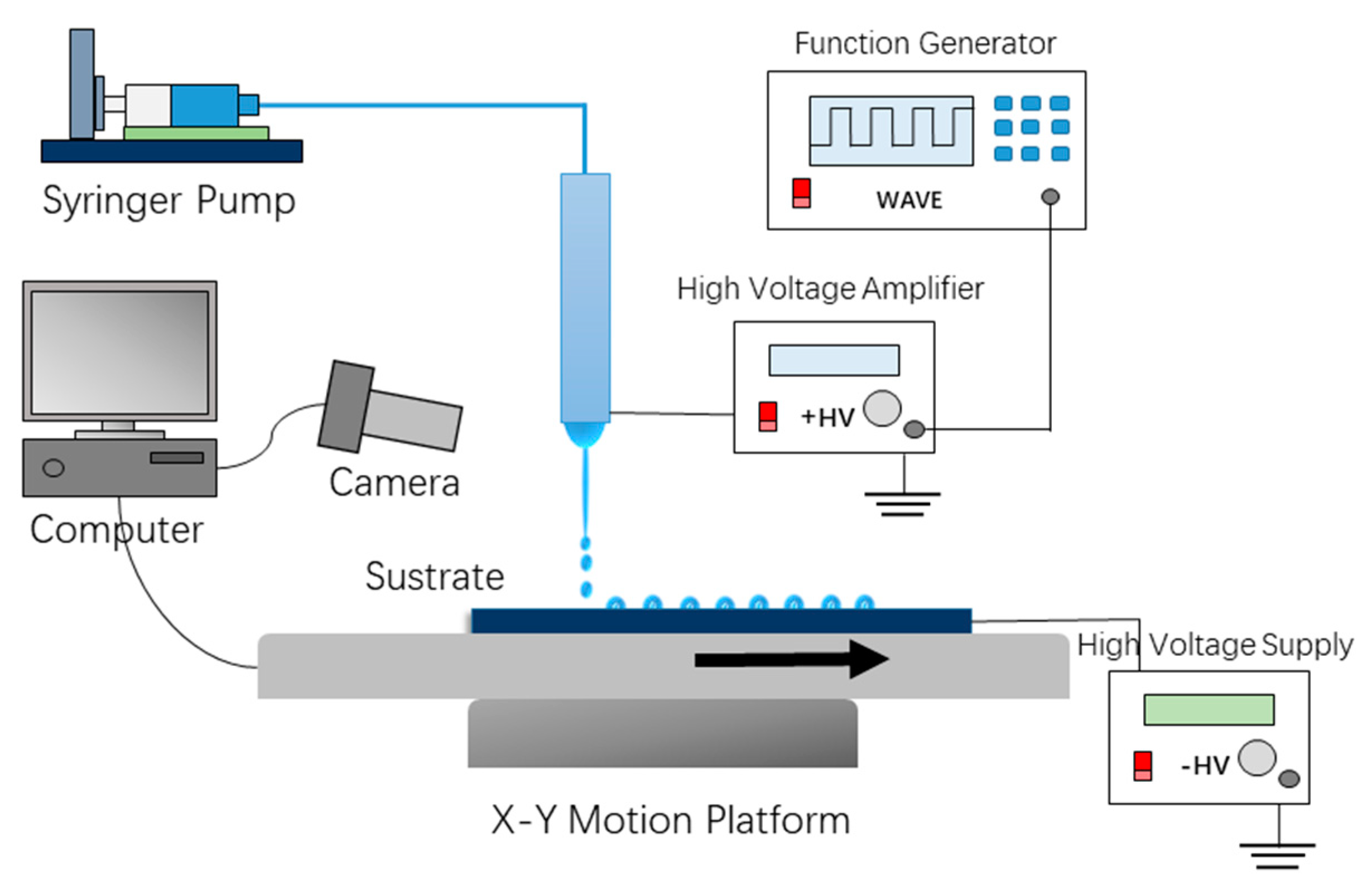
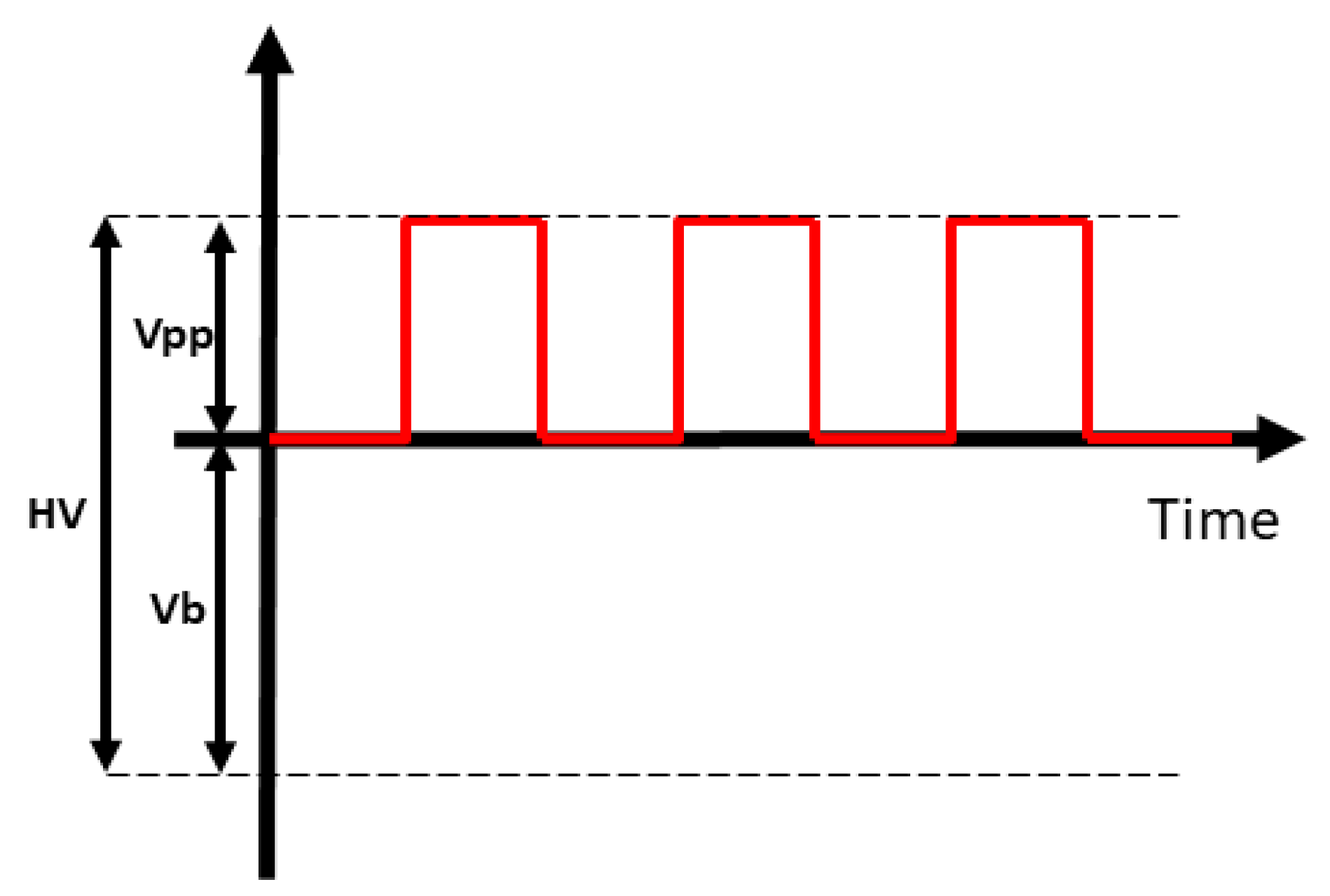
2.2. EHD Printing System
2.3. PDMS Mold and Microlens Fabrication
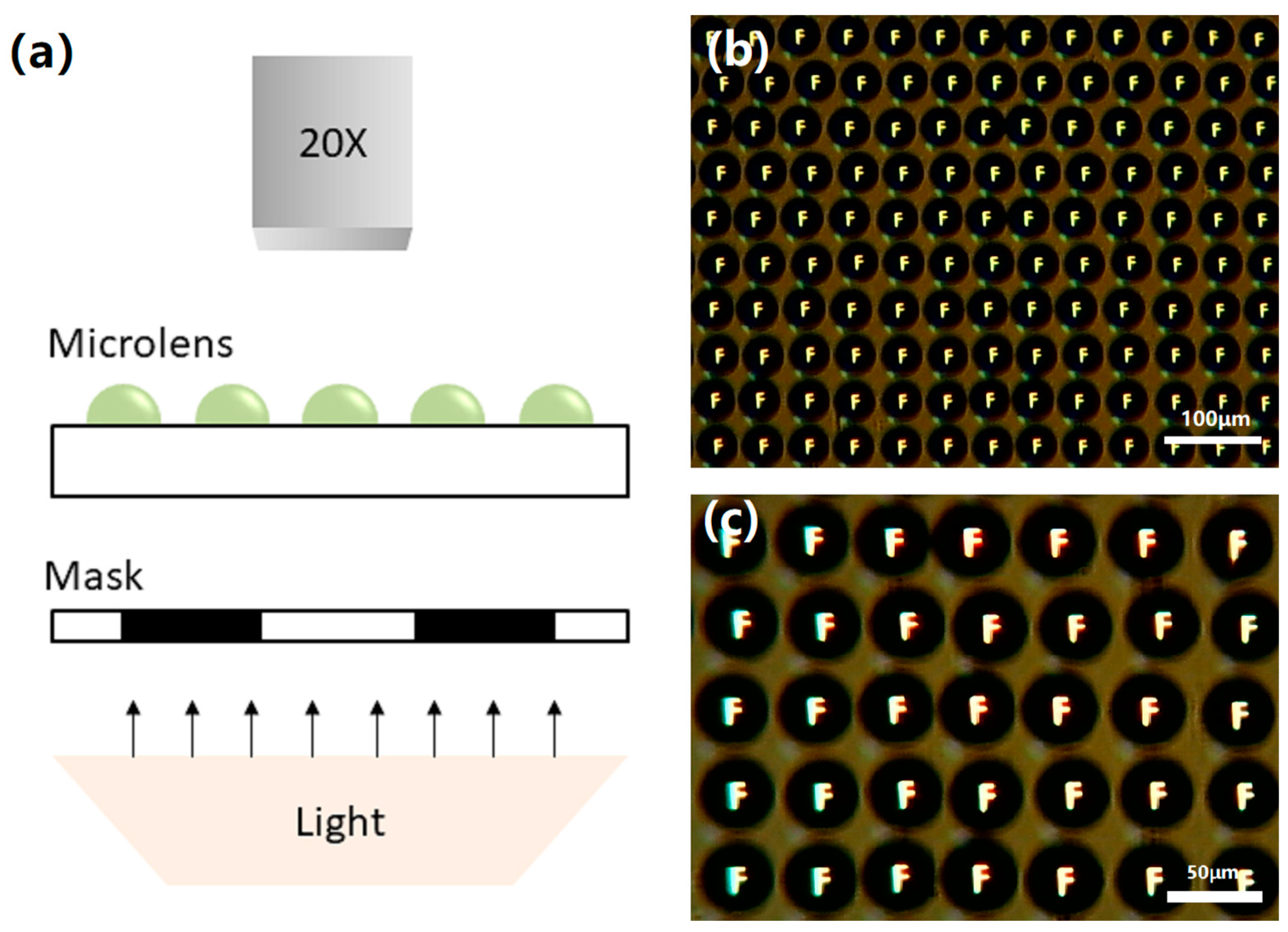
2.4. Morphological and Optical Characterization
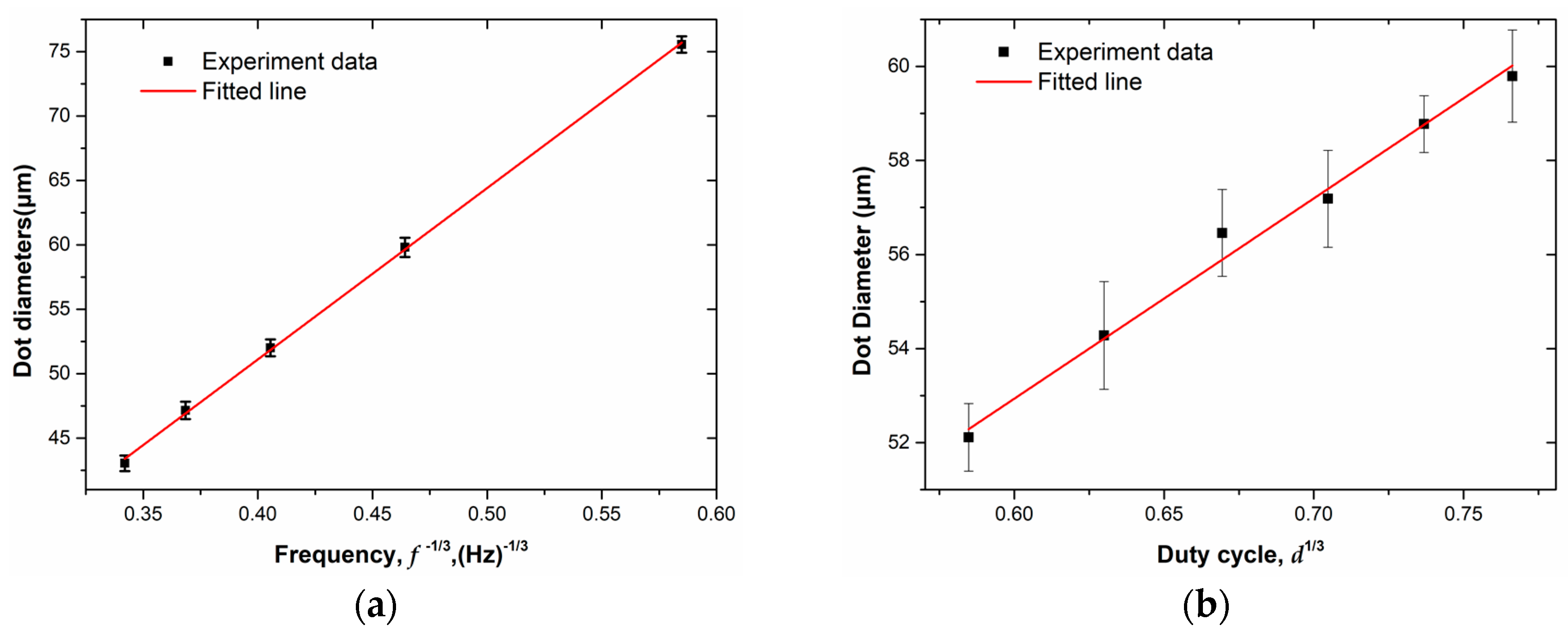
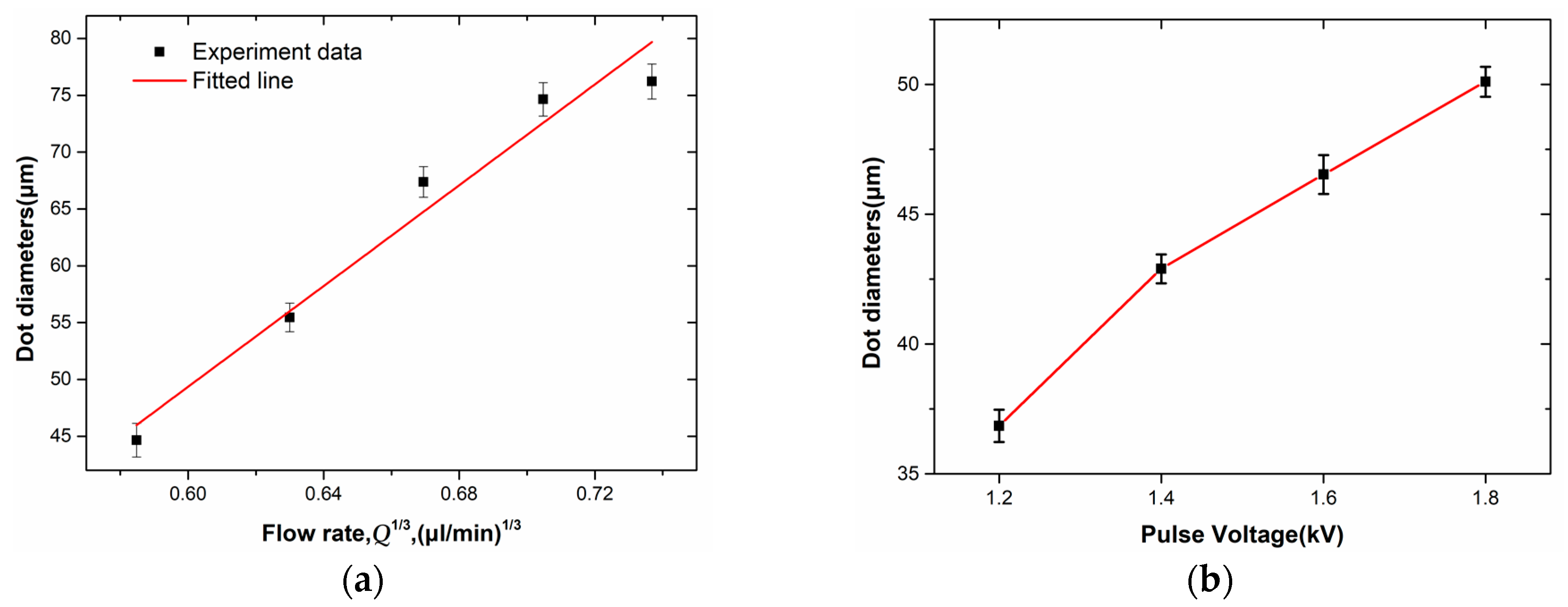
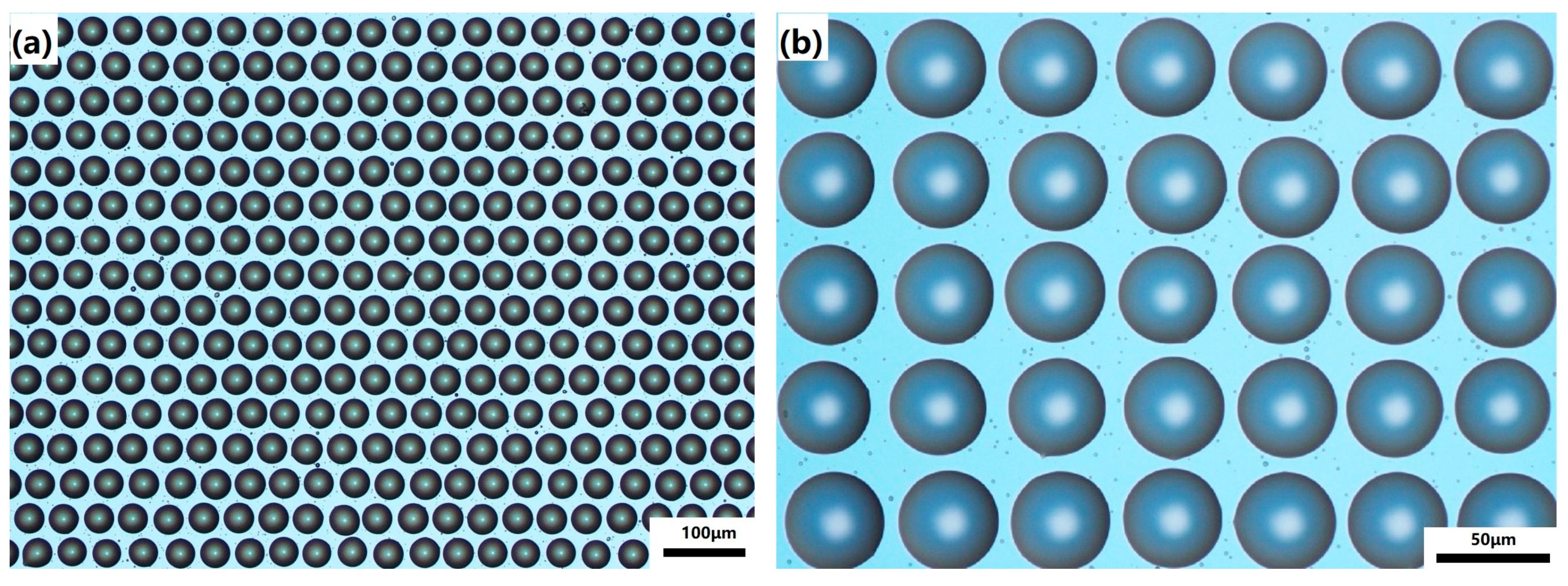
3. Results and Discussion
4. Conclusions
Author Contributions
Funding
Conflicts of Interest
References
- Ma, Z.C.; Hu, X.Y.; Zhang, Y.L.; Liu, X.Q.; Hou, Z.S.; Niu, L.G.; Zhu, L.; Han, B.; Chen, Q.D.; Sun, H.B. Smart Compound Eyes Enable Tunable Imaging. Adv. Funct. Mater. 2019, 29, 1903340. [Google Scholar] [CrossRef]
- Yu, R.; Yin, F.; Huang, X.; Ji, W. Molding hemispherical microlens arrays on flexible substrates for highly efficient inverted quantum dot light emitting diodes. J. Mater. Chem. C 2017, 5, 6682–6687. [Google Scholar] [CrossRef]
- Holzner, G.; Du, Y.; Cao, X.; Choo, J.; deMello, A.J.; Stavrakis, S. An optofluidic system with integrated microlens arrays for parallel imaging flow cytometry. Lab Chip 2018, 18, 3631–3637. [Google Scholar] [CrossRef] [PubMed]
- Elsherif, M.; Moreddu, R.; Hassan, M.U.; Yetisen, A.K.; Butt, H. Real-time optical fiber sensors based on light diffusing microlens arrays. Lab Chip 2019, 19, 2060–2070. [Google Scholar] [CrossRef] [PubMed]
- Olivieri, F.; Todino, M.; Coppola, S.; Vespini, V.; Pagliarulo, V.; Grilli, S.; Ferraro, P. Fabrication of polymer lenses and microlens array for lab-on-a-chip devices. Opt. Eng. 2016, 55, 081319. [Google Scholar] [CrossRef]
- Jung, H.; Jeong, K.H. Monolithic polymer microlens arrays with high numerical aperture and high packing density. ACS Appl. Mater. Interfaces 2015, 7, 2160–2165. [Google Scholar] [CrossRef]
- Gao, P.; Liang, Z.; Wang, X.; Zhou, T.; Xie, J.; Li, S.; Shen, W. Fabrication of a Micro-Lens Array Mold by Micro Ball End-Milling and Its Hot Embossing. Micromachines 2018, 9, 96. [Google Scholar] [CrossRef]
- Zheng, C.; Hu, A.; Kihm, K.D.; Ma, Q.; Li, R.; Chen, T.; Duley, W.W. Femtosecond Laser Fabrication of Cavity Microball Lens (CMBL) inside a PMMA Substrate for Super-Wide Angle Imaging. Small 2015, 11, 3007–3016. [Google Scholar] [CrossRef]
- Wang, L.; Luo, Y.; Liu, Z.; Feng, X.; Lu, B. Fabrication of microlens array with controllable high NA and tailored optical characteristics using confined ink-jetting. Appl. Surf. Sci. 2018, 442, 417–422. [Google Scholar] [CrossRef]
- Surdo, S.; Diaspro, A.; Duocastella, M. Microlens fabrication by replica molding of frozen laser-printed droplets. Appl. Surf. Sci. 2017, 418, 554–558. [Google Scholar] [CrossRef]
- Du, Z.; Yu, X.; Han, Y. Inkjet printing of viscoelastic polymer inks. Chin. Chem. Lett. 2018, 29, 399–404. [Google Scholar] [CrossRef]
- Rose, M.A.; Bowen, J.J.; Morin, S.A. Emergent Soft Lithographic Tools for the Fabrication of Functional Polymeric Microstructures. ChemPhysChem 2019, 20, 909–925. [Google Scholar] [CrossRef] [PubMed]
- Chang, C.-Y.; Yang, S.-Y.; Huang, L.-S.; Jeng, T.-M. A novel method for rapid fabrication of microlens arrays using micro-transfer molding with soft mold. J. Micromech. Microeng. 2006, 16, 999–1005. [Google Scholar] [CrossRef]
- Shin, T.K.; Ho, J.R.; Cheng, J.W.J. A New Approach to Polymeric Microlens Array Fabrication Using Soft Replica Molding. IEEE Photonics Technol. Lett. 2004, 16, 2078–2080. [Google Scholar] [CrossRef]
- Hu, C.-N.; Hsieh, H.-T.; Su, G.-D.J. Fabrication of microlens arrays by a rolling process with soft polydimethylsiloxane molds. J. Micromech. Microeng. 2011, 21, 065013. [Google Scholar] [CrossRef]
- Liu, X.; Wang, Q.; Qin, J.; Lin, B. A facile “liquid-molding” method to fabricate PDMS microdevices with 3-dimensional channel topography. Lab Chip 2009, 9, 1200–1205. [Google Scholar] [CrossRef] [PubMed]
- Zhu, X.; Zhu, L.; Chen, H.; Yang, L.; Zhang, W. Micro-ball lens structure fabrication based on drop on demand printing the liquid mold. Appl. Surf. Sci. 2016, 361, 80–89. [Google Scholar] [CrossRef]
- Lu, Y.; Lin, B.; Qin, J. Patterned paper as a low-cost, flexible substrate for rapid prototyping of PDMS microdevices via “liquid molding”. Anal. Chem. 2011, 83, 1830–1835. [Google Scholar] [CrossRef]
- Jiang, J.; Wang, X.; Li, W.; Liu, J.; Liu, Y.; Zheng, G. Electrohydrodynamic Direct-Writing Micropatterns with Assisted Airflow. Micromachines 2018, 9, 456. [Google Scholar] [CrossRef] [Green Version]
- Guo, L.; Duan, Y.; Huang, Y.; Yin, Z. Experimental Study of the Influence of Ink Properties and Process Parameters on Ejection Volume in Electrohydrodynamic Jet Printing. Micromachines 2018, 9, 522. [Google Scholar] [CrossRef] [Green Version]
- Onses, M.S.; Sutanto, E.; Ferreira, P.M.; Alleyne, A.G.; Rogers, J.A. Mechanisms, Capabilities, and Applications of High-Resolution Electrohydrodynamic Jet Printing. Small 2015, 11, 4237–4266. [Google Scholar] [CrossRef] [PubMed]
- Fang, F.; Chen, X.; Du, Z.; Zhu, Z.; Chen, X.; Wang, H.; Wu, P. Controllable Direct-Writing of Serpentine Micro/Nano Structures via Low Voltage Electrospinning. Polymers 2015, 7, 1577–1586. [Google Scholar] [CrossRef] [Green Version]
- Han, Y.; Dong, J. Electrohydrodynamic (EHD) Printing of Molten Metal Ink for Flexible and Stretchable Conductor with Self-Healing Capability. Adv. Mater. Technol. 2018, 3, 1700268. [Google Scholar] [CrossRef]
- Zhu, M.; Duan, Y.; Liu, N.; Li, H.; Li, J.; Du, P.; Tan, Z.; Niu, G.; Gao, L.; Huang, Y.; et al. Electrohydrodynamically Printed High-Resolution Full-Color Hybrid Perovskites. Adv. Funct. Mater. 2019, 29, 1903294. [Google Scholar] [CrossRef]
- Zeng, J.; Wang, H.; Lin, Y.; Zhang, J.; Liang, F.; Fang, F.; Yang, F.; Wang, P.; Zhu, Z.; Chen, X.; et al. Fabrication of microfluidic channels based on melt-electrospinning direct writing. Microfluid. Nanofluidics 2018, 22, 23. [Google Scholar] [CrossRef]
- Ding, Y.; Zhu, C.; Liu, J.; Duan, Y.; Yi, Z.; Xiao, J.; Wang, S.; Huang, Y.; Yin, Z. Flexible small-channel thin-film transistors by electrohydrodynamic lithography. Nanoscale 2017, 9, 19050–19057. [Google Scholar] [CrossRef]
- Grimaldi, I.A.; Coppola, S.; Loffredo, F.; Villani, F.; Nenna, G.; Minarini, C.; Vespini, V.; Miccio, L.; Grilli, S.; Ferraro, P. Graded-size microlens array by the pyro-electrohydrodynamic continuous printing method. Appl. Opt. 2013, 52, 7699–7705. [Google Scholar] [CrossRef]
- Sun, J.; Bao, B.; He, M.; Zhou, H.; Song, Y. Recent Advances in Controlling the Depositing Morphologies of Inkjet Droplets. ACS Appl. Mater. Interfaces 2015, 7, 28086–28099. [Google Scholar] [CrossRef]
- Jiang, J.; Zheng, G.; Zhu, P.; Liu, J.; Liu, Y.; Wang, X.; Li, W.; Guo, S. Controlling of Electrospray Deposition for Micropatterns. Micromachines 2018, 9, 72. [Google Scholar] [CrossRef] [Green Version]
- Stringer, J.; Derby, B. Limits to feature size and resolution in ink jet printing. J. Eur. Ceram. Soc. 2009, 29, 913–918. [Google Scholar] [CrossRef]
- Park, J.; Kim, B.; Kim, S.-Y.; Hwang, J. Prediction of drop-on-demand (DOD) pattern size in pulse voltage-applied electrohydrodynamic (EHD) jet printing of Ag colloid ink. Appl. Phys. A 2014, 117, 2225–2234. [Google Scholar] [CrossRef]
- Xu, L.; Wang, X.; Lei, T.; Sun, D.; Lin, L. Electrohydrodynamic deposition of polymeric droplets under low-frequency pulsation. Langmuir 2011, 27, 6541–6548. [Google Scholar] [CrossRef] [PubMed]


© 2020 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Fang, F.; Tao, X.; Chen, X.; Wang, H.; Wu, P.; Zhang, J.; Zeng, J.; Zhu, Z.; Liu, Z. Microlens Fabrication by Replica Molding of Electro-Hydrodynamic Printing Liquid Mold. Micromachines 2020, 11, 161. https://doi.org/10.3390/mi11020161
Fang F, Tao X, Chen X, Wang H, Wu P, Zhang J, Zeng J, Zhu Z, Liu Z. Microlens Fabrication by Replica Molding of Electro-Hydrodynamic Printing Liquid Mold. Micromachines. 2020; 11(2):161. https://doi.org/10.3390/mi11020161
Chicago/Turabian StyleFang, Feiyu, Xulei Tao, Xun Chen, Han Wang, Peixuan Wu, Jiarong Zhang, Jun Zeng, Zimin Zhu, and Zhen Liu. 2020. "Microlens Fabrication by Replica Molding of Electro-Hydrodynamic Printing Liquid Mold" Micromachines 11, no. 2: 161. https://doi.org/10.3390/mi11020161
APA StyleFang, F., Tao, X., Chen, X., Wang, H., Wu, P., Zhang, J., Zeng, J., Zhu, Z., & Liu, Z. (2020). Microlens Fabrication by Replica Molding of Electro-Hydrodynamic Printing Liquid Mold. Micromachines, 11(2), 161. https://doi.org/10.3390/mi11020161



