Effect of Microstructure on Micro-Mechanical Properties of Composite Solid Propellant
Abstract
:1. Introduction
2. Construction of Singular Crack Element
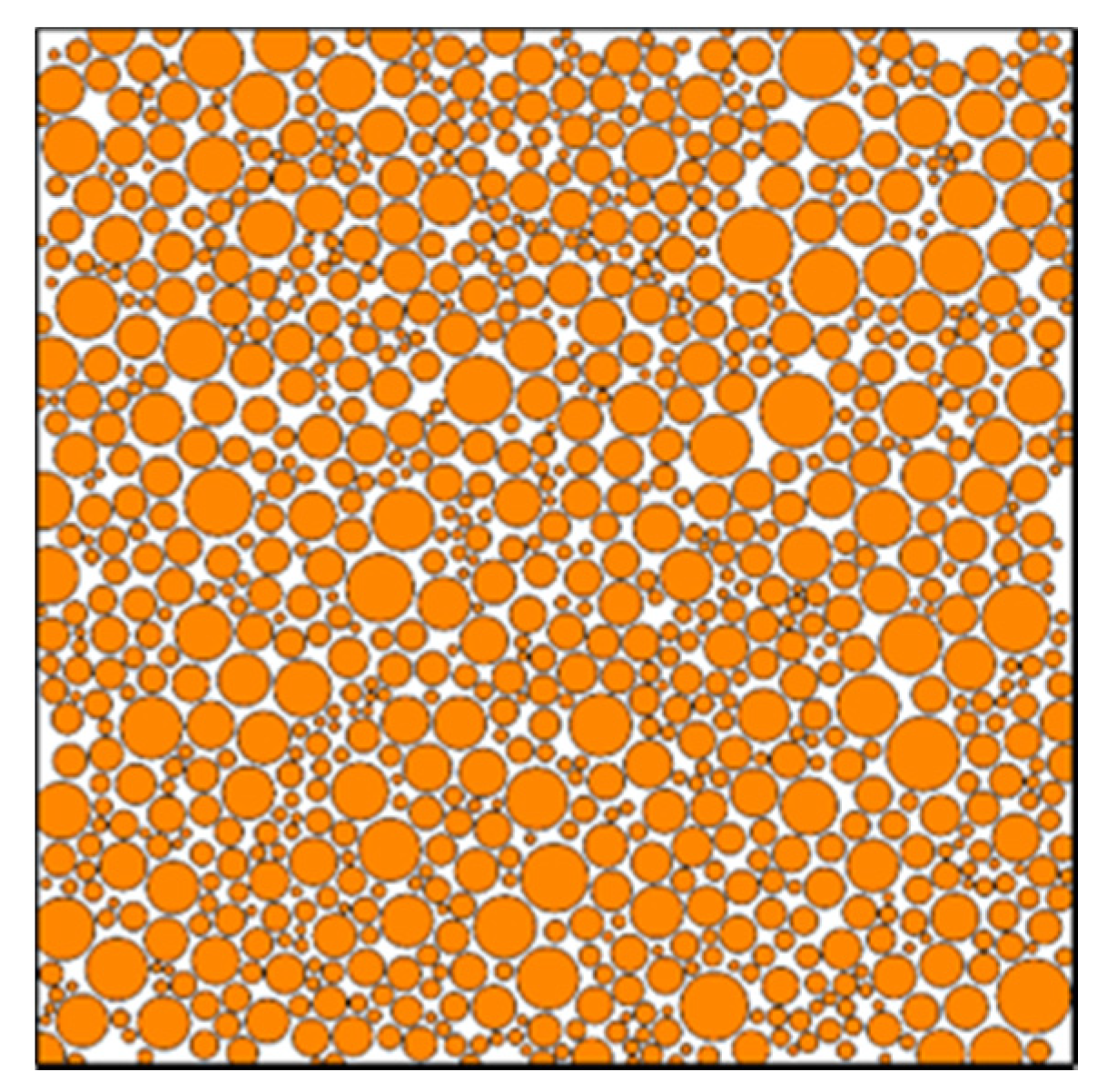
2.1. Mesoscale Model of Composite Solid Propellants
2.2. Simulation Parameters of the Meso-Mechanical Model
3. Effect of Mesostructure on Mechanical Properties of Propellant
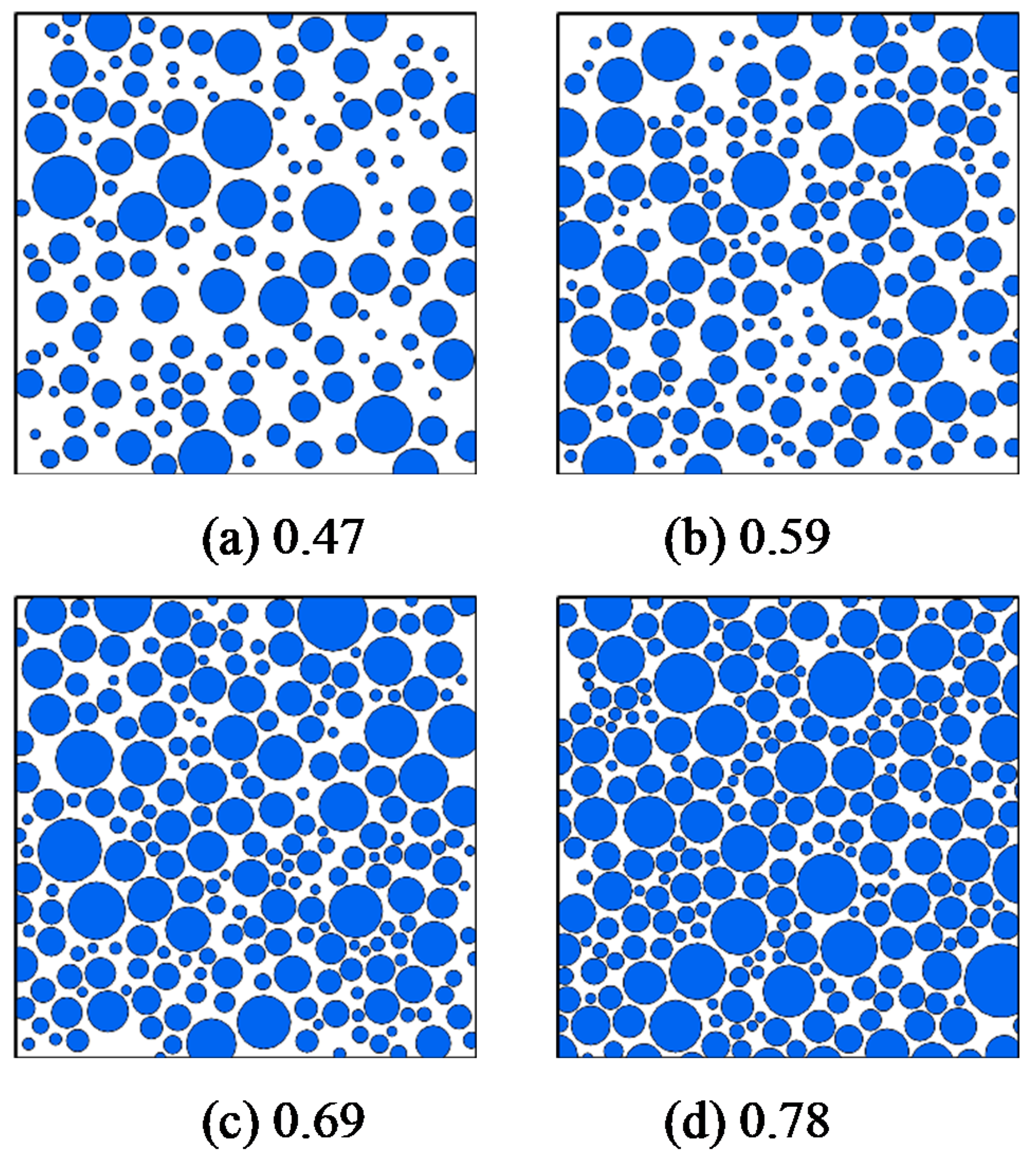
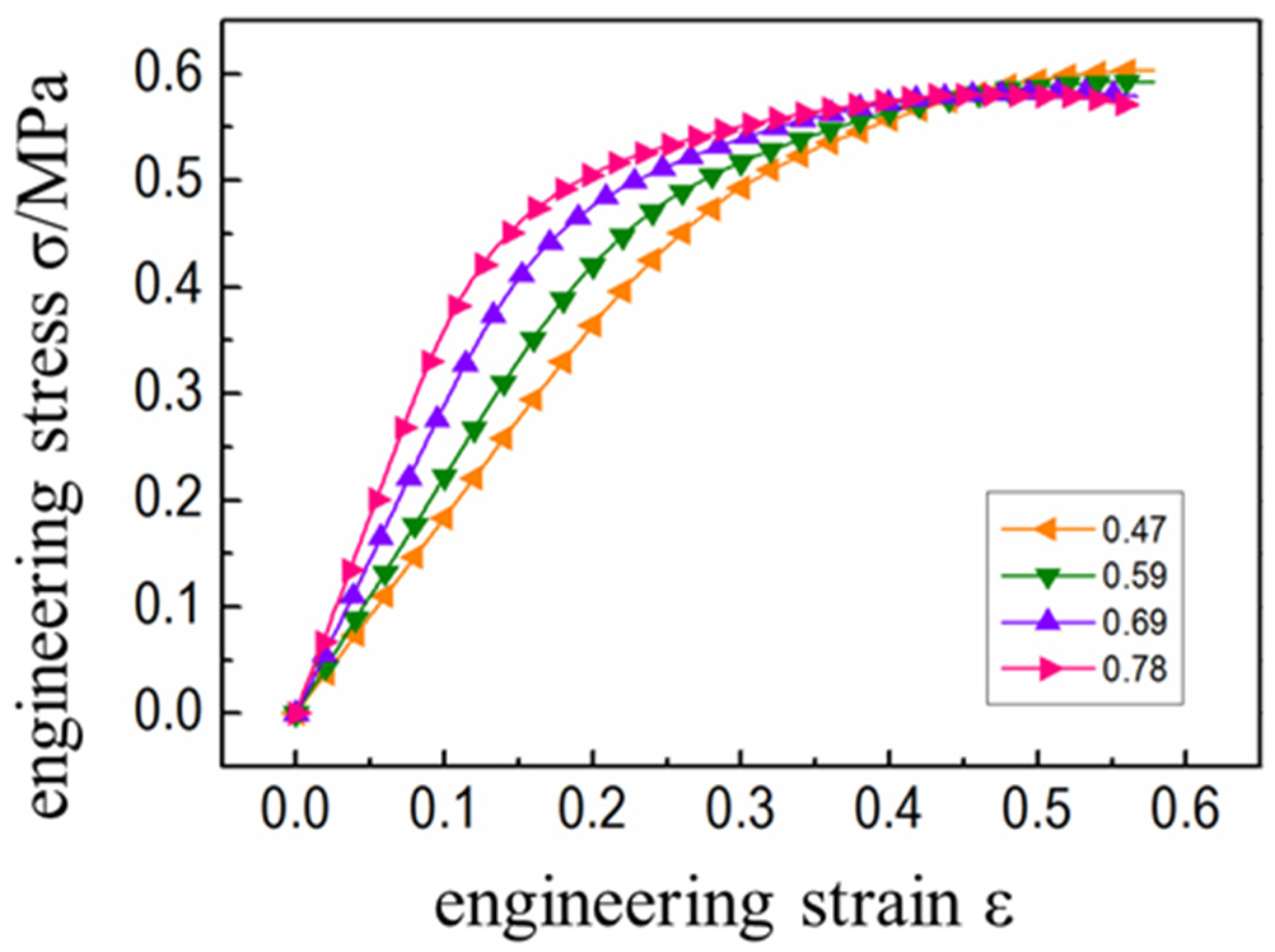
3.1. Influence of Particle Volume Fraction
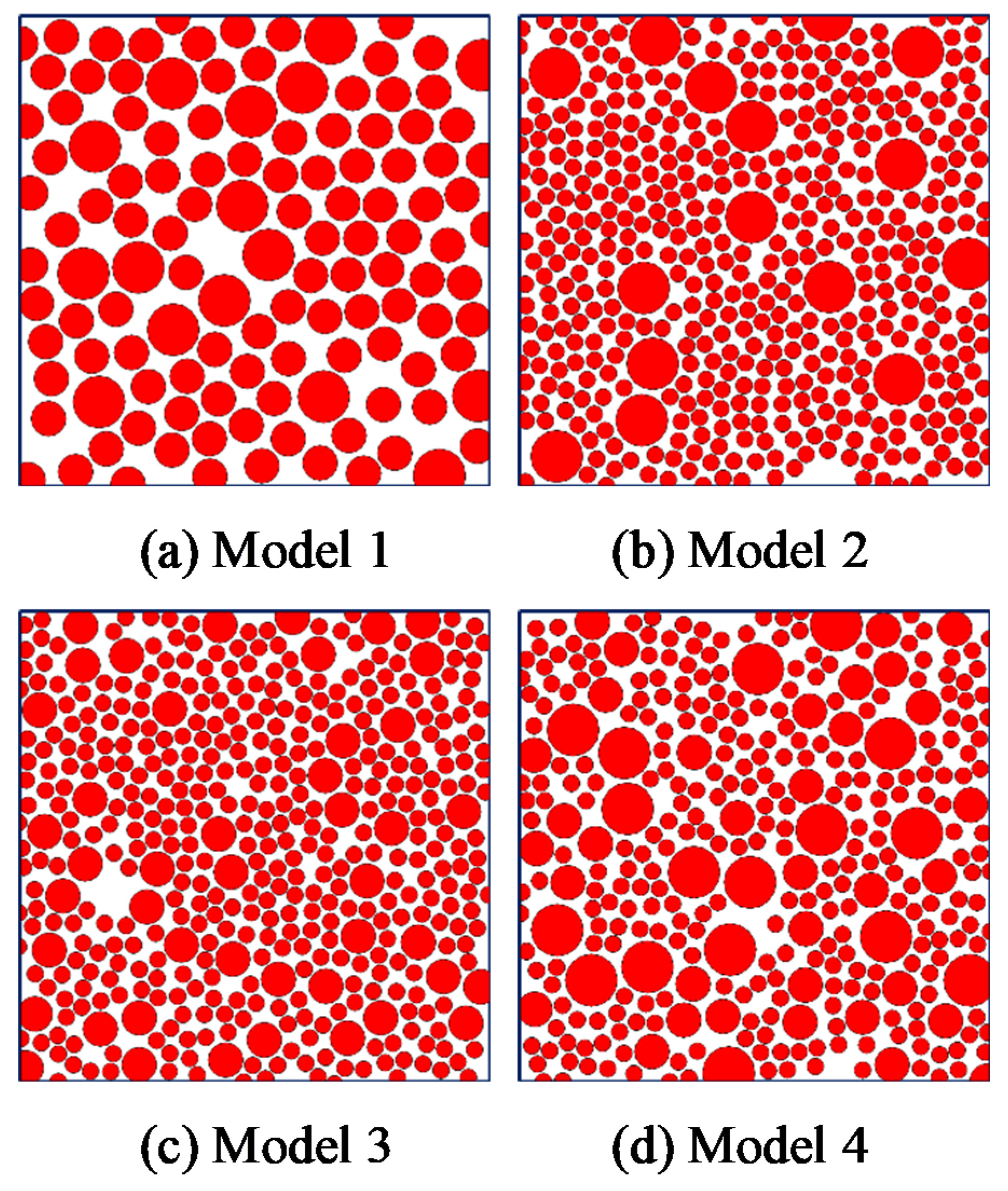
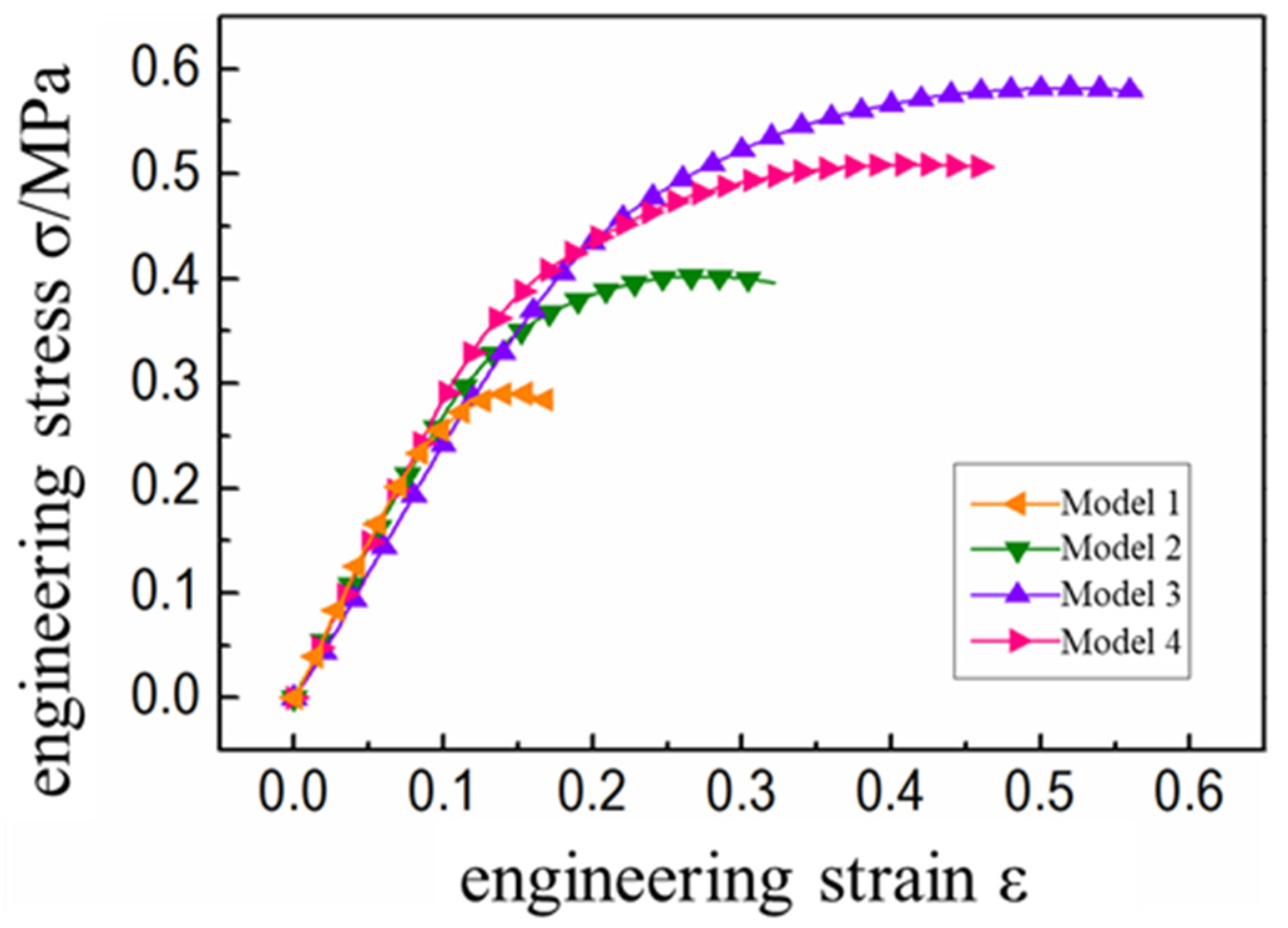
3.2. Influence of Multi-Particle Gradation
4. Effect of Initial Defects on Mechanical Properties of Propellant
4.1. Definition and Modeling of Initial Interface Defects
- The initial defects are uniformly and randomly distributed in the interface element.
- For the defect interface, the failure bonding element is used to simulate.
- Define the interface defect content as p, p = Nd/N. N is the total number of interface units and Nd is the total number of defective units.
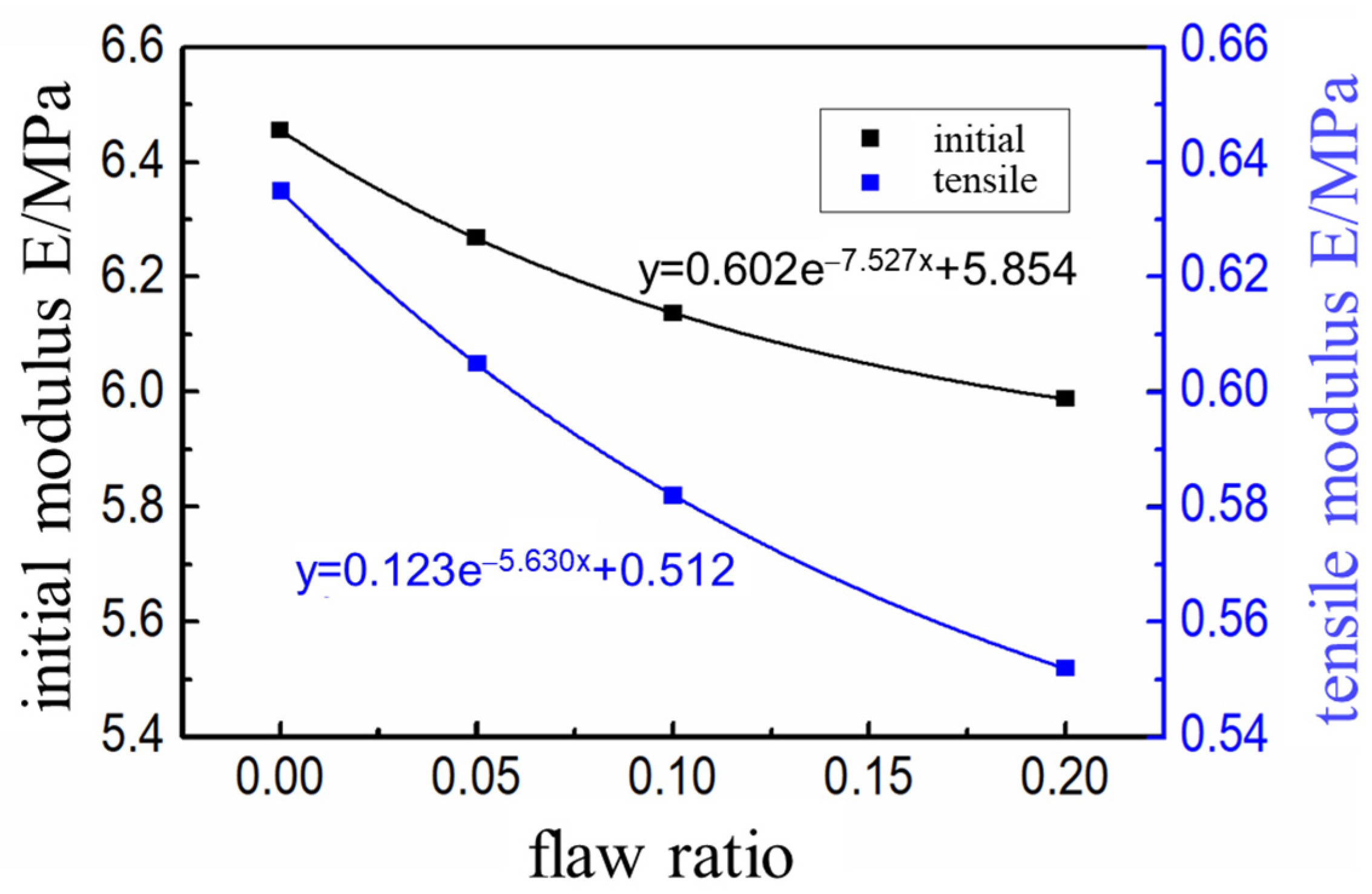
4.2. Effects of Initial Interface Defects on Tensile Mechanical Properties of the Propellant
4.3. Effects of Initial Interface Defects on Mechanical Properties of Propellant Relaxation
5. Conclusions
Author Contributions
Funding
Conflicts of Interest
References
- Tussiwand, G.S.; Saouma, V.E.; Terzenbach, R.; De Luca, L.T. Fracture Mechanics of Composite Solid Rocket Propellant Grains: Material Testing. J. Propuls. Power 2009, 25, 60–73. [Google Scholar] [CrossRef]
- Bencher, C.D.; Dauskardt, R.H.; Ritchie, R.O. Microstructural damage and fracture processes in a composite solid rocket propellant. J. Spacecr. Rocket. 1995, 32, 328–334. [Google Scholar] [CrossRef]
- Liu, C.T. Crack growth behavior in a solid propellant. Eng. Fract. Mech. 1997, 56, 127–135. [Google Scholar] [CrossRef]
- Van Ramshorst, M.C.; Di Benedetto, G.L.; Duvalois, W.; Hooijmeijer, P.A.; van der Heijden, A.E. Investigation of the failure mechanism of HTPB/AP/Al propellant by insitu uniaxial tensile Experimentation in SEM. Propellants Explos. Pyrotech. 2016, 41, 700–708. [Google Scholar] [CrossRef]
- Hu, W.; Qi, C.; Guo, X.; Pang, A.; Zhou, N.; Lu, J.; Tang, G.; Gan, L.; Huang, J. Alkynylfunctionalization of carbon nanotubes to promote anchoring potential in glycidyl azide polymer-based binders via Huisgen reaction for solid propellant application. J. Polym. Res. 2021, 28, 1–11. [Google Scholar] [CrossRef]
- Naito, K. Interfacial mechanical properties of carbon/glass hybrid thermoplastic epoxy composite rods. Compos. Struct. 2020, 257, 113129. [Google Scholar] [CrossRef]
- Matouš, K.; Inglis, H.M.; Gu, X.; Rypl, D.; Jackson, T.L.; Geubelle, P.H. Multiscale modeling of solid propellants: From particle packing to failure. Compos. Sci. Technol. 2007, 67, 1694–1708. [Google Scholar] [CrossRef]
- Chang, W.J.; Ju, Y.T.; Han, B. Numerical simulation of particle/matrix interface failure in composite propellant. Def. Technol. 2012, 8, 35–60. [Google Scholar]
- Arora, H.; Tarleton, E.; Li-Mayer, J.; Charalambids, M.N.; Lewis, D. Modelling the damage and deformation process in a plastic bonded explosive microstructure under tension using the finite element method. Comput. Mater. Sci. 2015, 110, 91–101. [Google Scholar] [CrossRef] [Green Version]
- Li, G.; Xing, Y.; Ji, Z.; Xie, L. Finite element analysis of microscale interfacial debonding in composite solid propellants. Acta Mater. Compos. Sin. 2011, 28, 229–235. [Google Scholar]
- Zhi, S.J.; Sun, B.; Zhang, J.W. Multiscale modeling of heterogeneous propellants from particle packing to grain failure using a surface-based cohesive approach. Acta Mech. Sin. 2012, 28, 746–759. [Google Scholar] [CrossRef]
- Han, B.; Ju, Y.; Zhou, C. Simulation of crack propagation in HTPB propellant using cohesive zone model. Eng. Fail. Anal. 2012, 26, 304–317. [Google Scholar] [CrossRef]
- Cui, H.R.; Shen, Z.B.; Li, H.Y. A novel time dependent cohesive zone model for the debonding interface between solid propellant and insulation. Meccanica 2018, 53, 3527–3544. [Google Scholar] [CrossRef]
- Ahmad, N.; Khan, M.B.; Ma, X.; Ul-Haq, N. The influence of cross-linking/chain extension structures on mechanical properties of HTPB-based polyurethane elastomers. Arab. J. Sci. Eng. 2014, 39, 43–51. [Google Scholar] [CrossRef]
- Zhi, S.; Cao, F.; Shen, Z. Parameters inversion of particle dewetting damage of composite solid propellants. J. Propuls. Technol. 2016, 37, 1977–1983. [Google Scholar]
- He, G.Q.; Cai, T.M.; Li, J. Experimental study of anomalous combustion in solid propellant gain with defects. J. Propuls. Technol. 1999, 20, 30–35. [Google Scholar]
- Du, X.; Jin, L. Research on the influence of pores and micro-cracks on the macro-mechanical properties of concrete. Eng. Mech. 2012, 29, 101–107. [Google Scholar]
- Erkkilä, A.L.; Tuovinen, T.; Kurki, M. A review of the analytical and numerical modeling of composites. Rep. Dep. Math. Inf. Technol. Ser. B Sci. Comput. 2016. [Google Scholar] [CrossRef]
- Xiao, S.; Zhu, L. Numerical experiment study of initial damage microstructural behaviors of concrete. J. Dalian Univ. Technol. 2017, 57, 78–86. [Google Scholar]
- Buckmaster, J.; Jackson, T.L.; Ulrich, M. Numerical modeling of heterogeneous propellant combustion. Reston Am. Inst. Aeronaut. Astronaut. 2001, 2001, 3579. [Google Scholar]
- Qiao, D.; Qiang, H.; Feng, S. Automatic application of periodic boundary conditions in meso analysis of composite solid propellant. IOP Conf. Series Earth Environ. Sci. 2021, 634, 12083. [Google Scholar] [CrossRef]
- Feng, T.; Xu, J.S.; Han, L.; Chen, X. Modeling and simulation of the debonding process of composite solid propellants. IOP Conf. Ser. Mater. Sci. Eng. 2017, 220, 12020. [Google Scholar] [CrossRef] [Green Version]
- Tunç, B.; Özüpek, S. Constitutive modeling of solid propellants for three dimensional nonlinear finite Element analysis. Aerosp. Sci. Technol. 2017, 69, 290–297. [Google Scholar] [CrossRef]
- Wang, H.; Xu, J.; Liu, Z.; Tong, X. Research on the viscoelasticity-viscoplasticity-viscodamage constitutive model of composite modified double base propellant. Acta Armamentarii 2018, 39, 1308–1315. [Google Scholar]
- Shen, Z.; Zhang, L.; Zhi, S. Wide temperature range-gas ambient pressure test system and experiments for solid propellant. Solid Rocket Technol. 2019, 42, 340–344. [Google Scholar]
- Liu, S.; Li, Z.; Niu, Y.; Yin, S.; Kong, Y.; Chen, F.; Wei, Y. Study on electric potential characteristics of coal fracture under uniaxial compression and gas confining pressure. Saf. Coal Mines 2017, 48, 5–8. [Google Scholar]
- Tunç, B.; Özüpek, S. Implementation and validation of a three dimensional damaging finite strain viscoelastic model. Int. J. Solids Struct. 2016, 102–103, 275–285. [Google Scholar] [CrossRef]
- Xu, H.; Xu, D.; Yang, L. A finite element method with generalized DOFs for stress intensity factors of crack groups. Appl. Math. Mech. 2016, 37, 1039–1049. [Google Scholar]





| Component | Adhesive | AP | Al | Other Components |
|---|---|---|---|---|
| mass fraction (%) | 8.0 | 69.5 | 18.5 | 4 |
| density (g/cm3) | 0.90 | 1.95 | 2.70 | - |
| volume fraction (%) | 23.9 | 63.8 | 12.3 | - |
| μ1/MPa | α1 | μ2/MPa | α2 | E1/MPa | τ1/s | E2/MPa | τ2/s |
|---|---|---|---|---|---|---|---|
| 0.235 | 0.525 | 7.529 × 10−5 | 6.705 | 0.0552 | 29.319 | 0.0216 | 392.201 |
| Rate of Extension (mm·min−1) | 1 | 5 | 20 | 100 | 500 | |
|---|---|---|---|---|---|---|
| AP-1 (250–300 μm) | 550 | 610 | 680 | 760 | 860 | |
| 0.346 | 0.454 | 0.586 | 0.723 | 0.831 | ||
| 0.012 | 0.012 | 0.012 | 0.012 | 0.012 | ||
| AP-2 (100–150 μm) | 520 | 580 | 650 | 720 | 800 | |
| 0.382 | 0.472 | 0.604 | 0.756 | 0.862 | ||
| 0.015 | 0.015 | 0.015 | 0.015 | 0.015 | ||
| AP-3 (10–20 μm) | 500 | 560 | 620 | 700 | 780 | |
| 0.442 | 0.558 | 0.683 | 0.779 | 0.921 | ||
| 0.018 | 0.018 | 0.018 | 0.018 | 0.018 | ||
| Model | 1 | 2 | 3 | 4 |
|---|---|---|---|---|
| grain size/μm | 246-165 | 246-80 | 165-80 | 246-165-80 |
| volume ratio | 3:7 | 3:7 | 3:7 | 1:1:1 |
| Model | 1 | 2 | 3 | 4 |
|---|---|---|---|---|
| Initial modulus/MPa | 2.84 | 2.80 | 2.41 | 2.88 |
| Elongation | 0.17 | 0.32 | 0.46 | 0.57 |
| Performance Index | Parameter | |||
|---|---|---|---|---|
| Defect ratio (%) | 0 | 5 | 10 | 20 |
| Initial modulus (MPa) | 6.456 | 6.268 | 6.137 | 5.988 |
| Tensile strength (MPa) | 0.635 | 0.605 | 0.582 | 0.552 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Li, T.; Xu, J.; Han, J.; He, Y. Effect of Microstructure on Micro-Mechanical Properties of Composite Solid Propellant. Micromachines 2021, 12, 1378. https://doi.org/10.3390/mi12111378
Li T, Xu J, Han J, He Y. Effect of Microstructure on Micro-Mechanical Properties of Composite Solid Propellant. Micromachines. 2021; 12(11):1378. https://doi.org/10.3390/mi12111378
Chicago/Turabian StyleLi, Tianpeng, Jinsheng Xu, Junli Han, and Yong He. 2021. "Effect of Microstructure on Micro-Mechanical Properties of Composite Solid Propellant" Micromachines 12, no. 11: 1378. https://doi.org/10.3390/mi12111378
APA StyleLi, T., Xu, J., Han, J., & He, Y. (2021). Effect of Microstructure on Micro-Mechanical Properties of Composite Solid Propellant. Micromachines, 12(11), 1378. https://doi.org/10.3390/mi12111378





