Sawing Force Prediction Model and Experimental Study on Vibration-Assisted Diamond Wire Sawing
Abstract
:1. Introduction
2. Materials and Methods
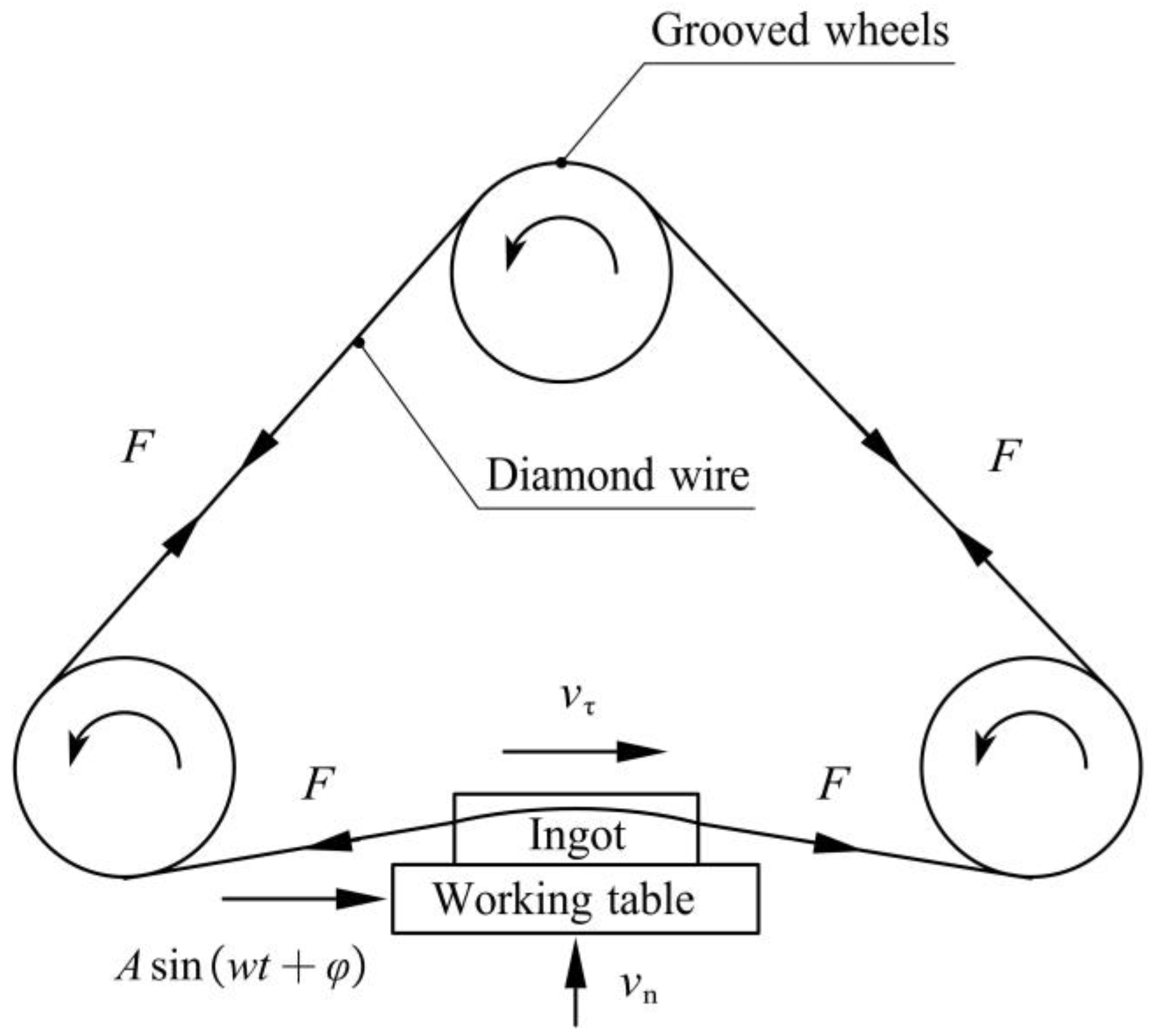
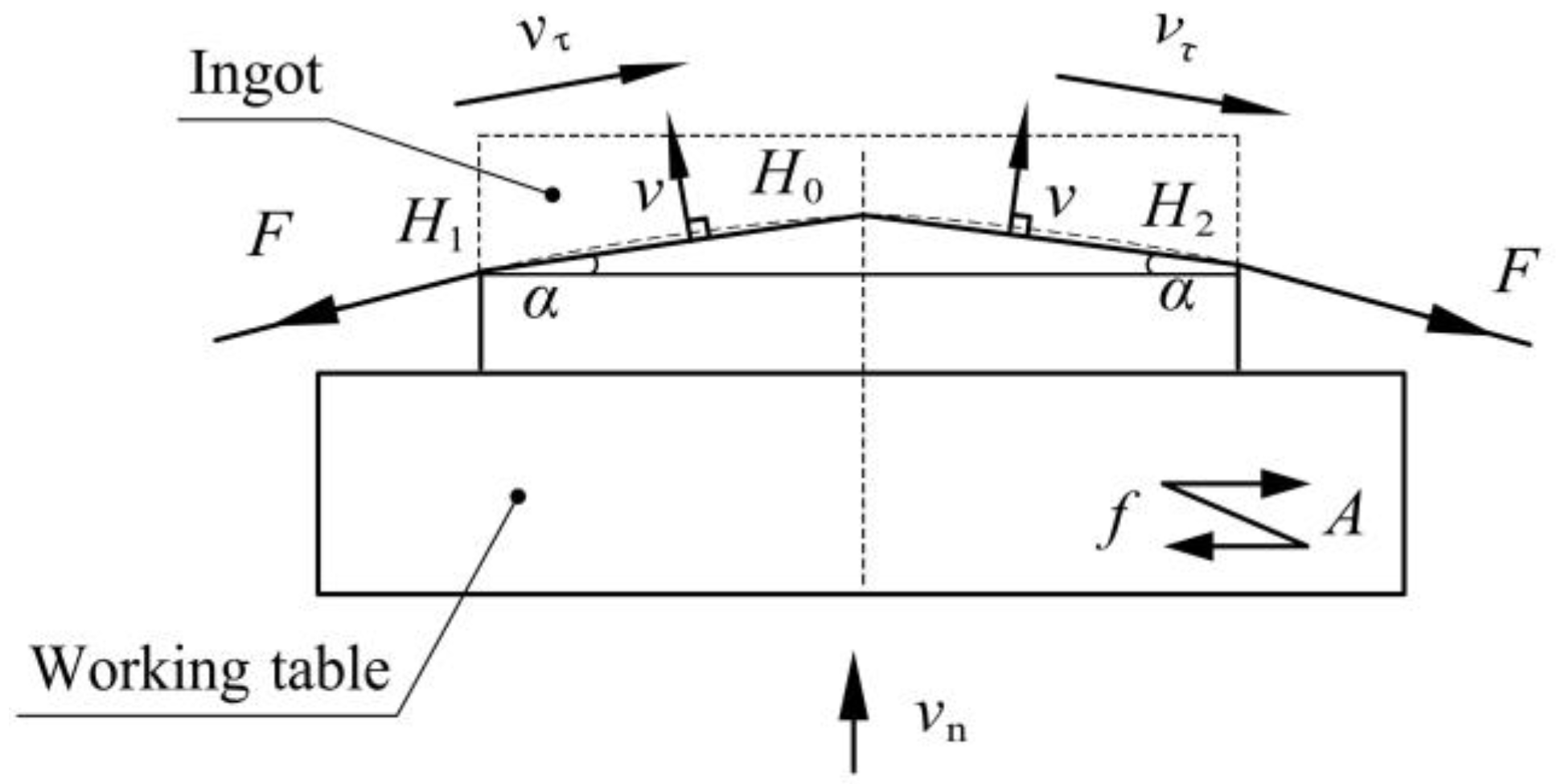
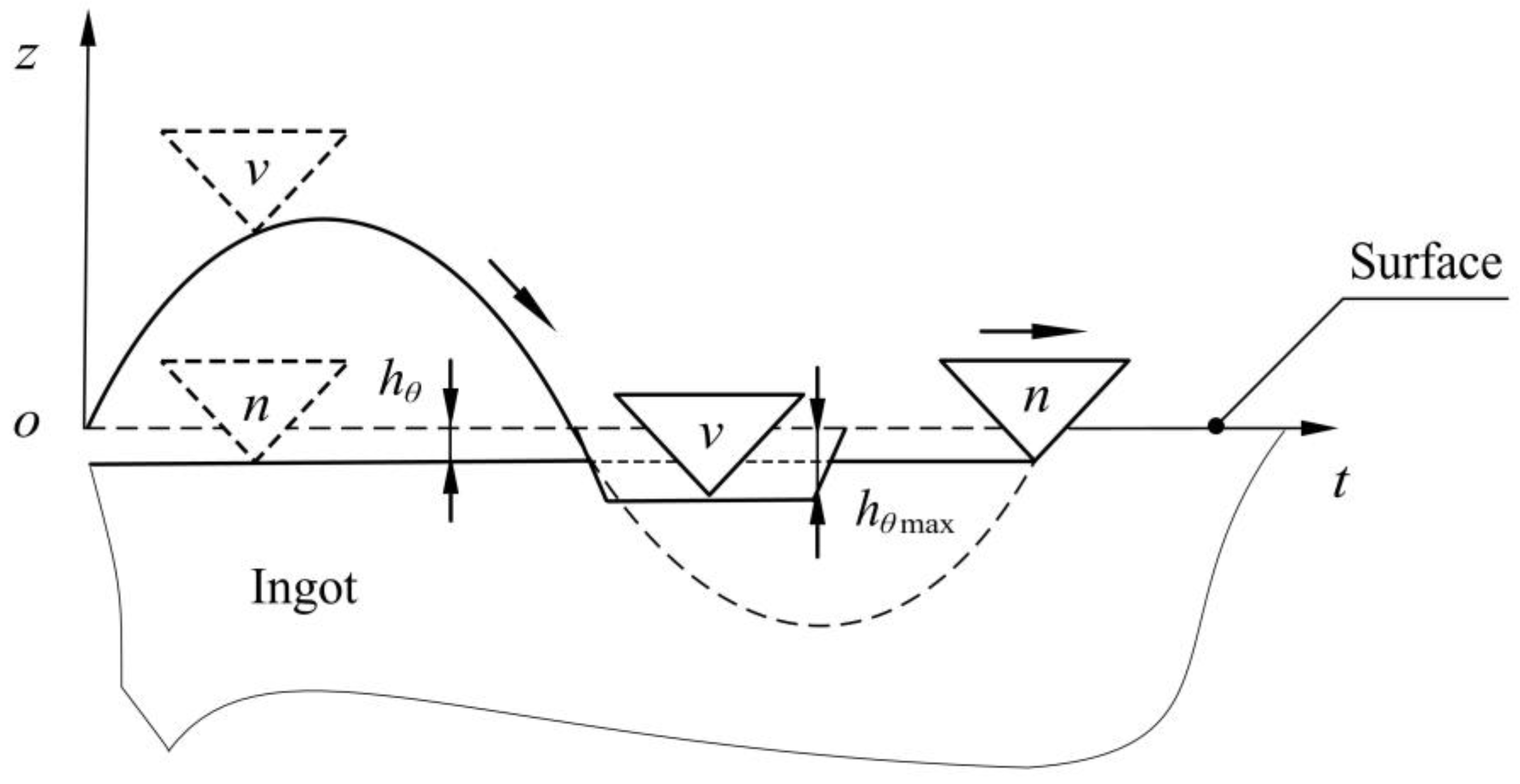
2.1. Sawing Force Prediction Model of Vibration-Assisted Sawing
2.2. Vibration-Assisted Diamond-Wire-Sawing Experiment
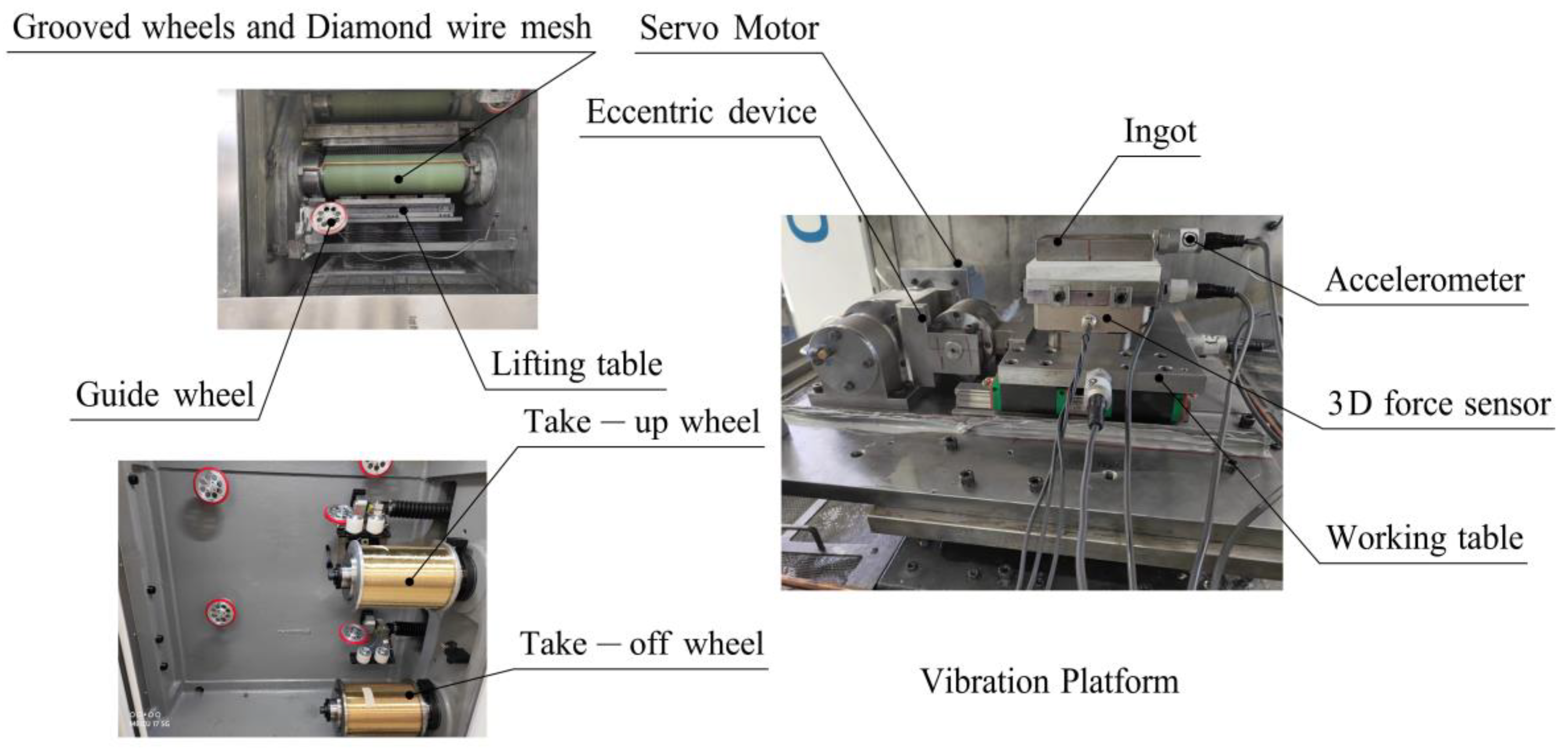
2.2.1. Design of Experimental Device
2.2.2. Experimental Measurements
3. Results and Discussion
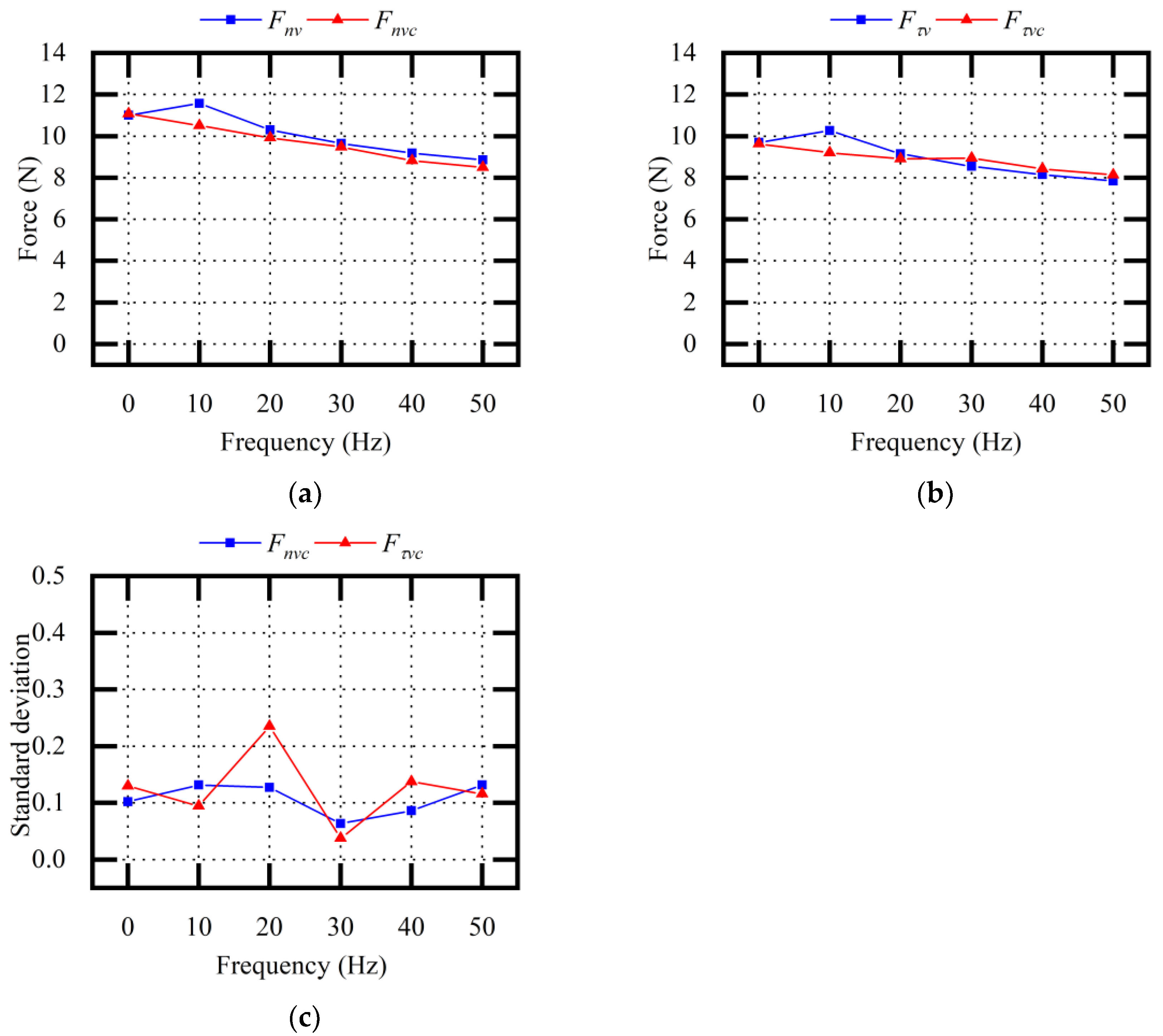
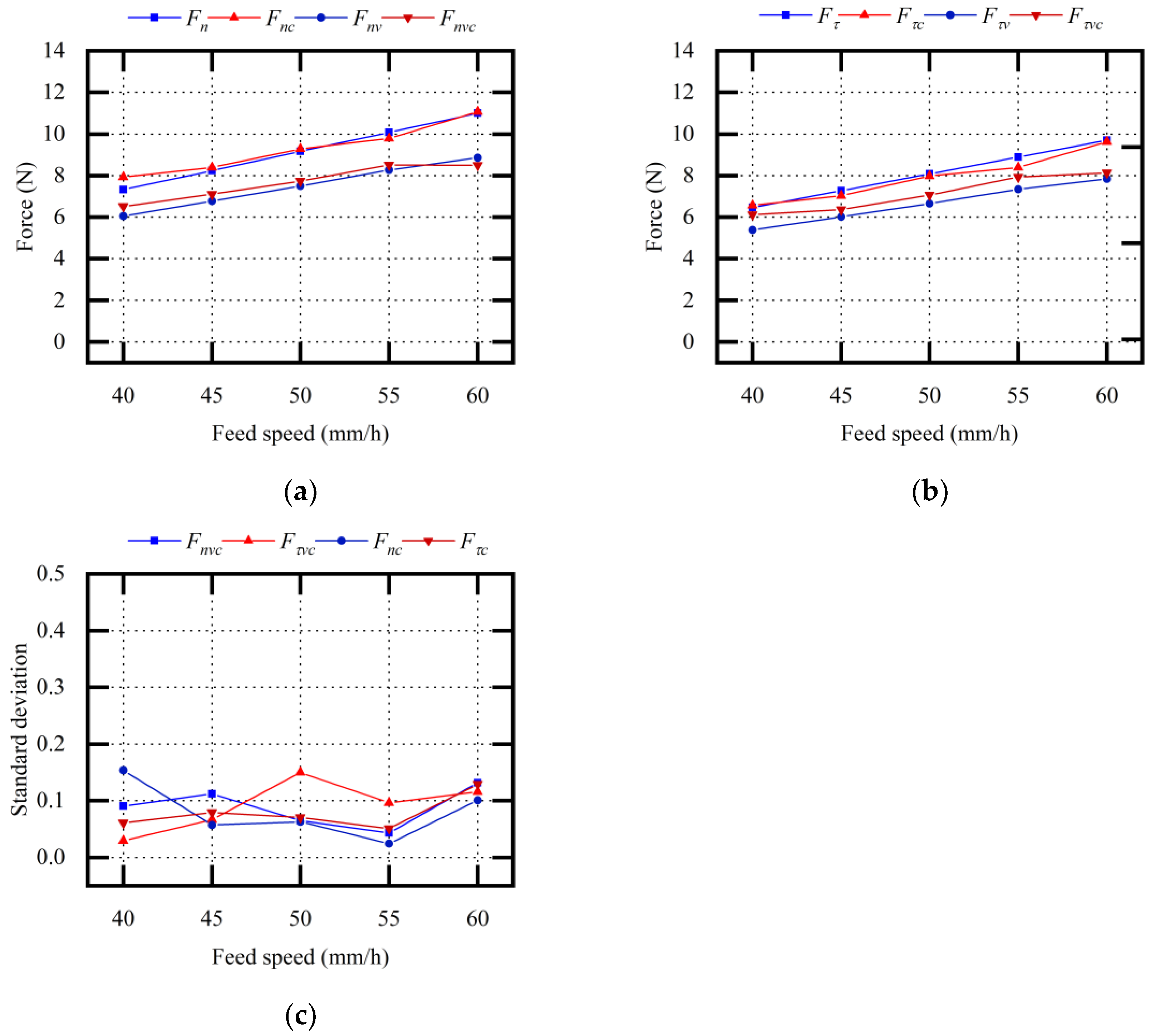
3.1. Vibration Equivalent Sawing Force Analysis
- The relative error between the experimental values and the theoretical values was relatively small, which verified the accuracy of the sawing force prediction model;
- With increase in the vibration frequency (0–50 Hz), the sawing forces and gradually decreased;
- Sawing parameters, including wire speed, feed speed, and wire tension, affected the impact load between the abrasive particle and the ingot and further influenced the optimization effect of vibration assistance on the sawing forces and .
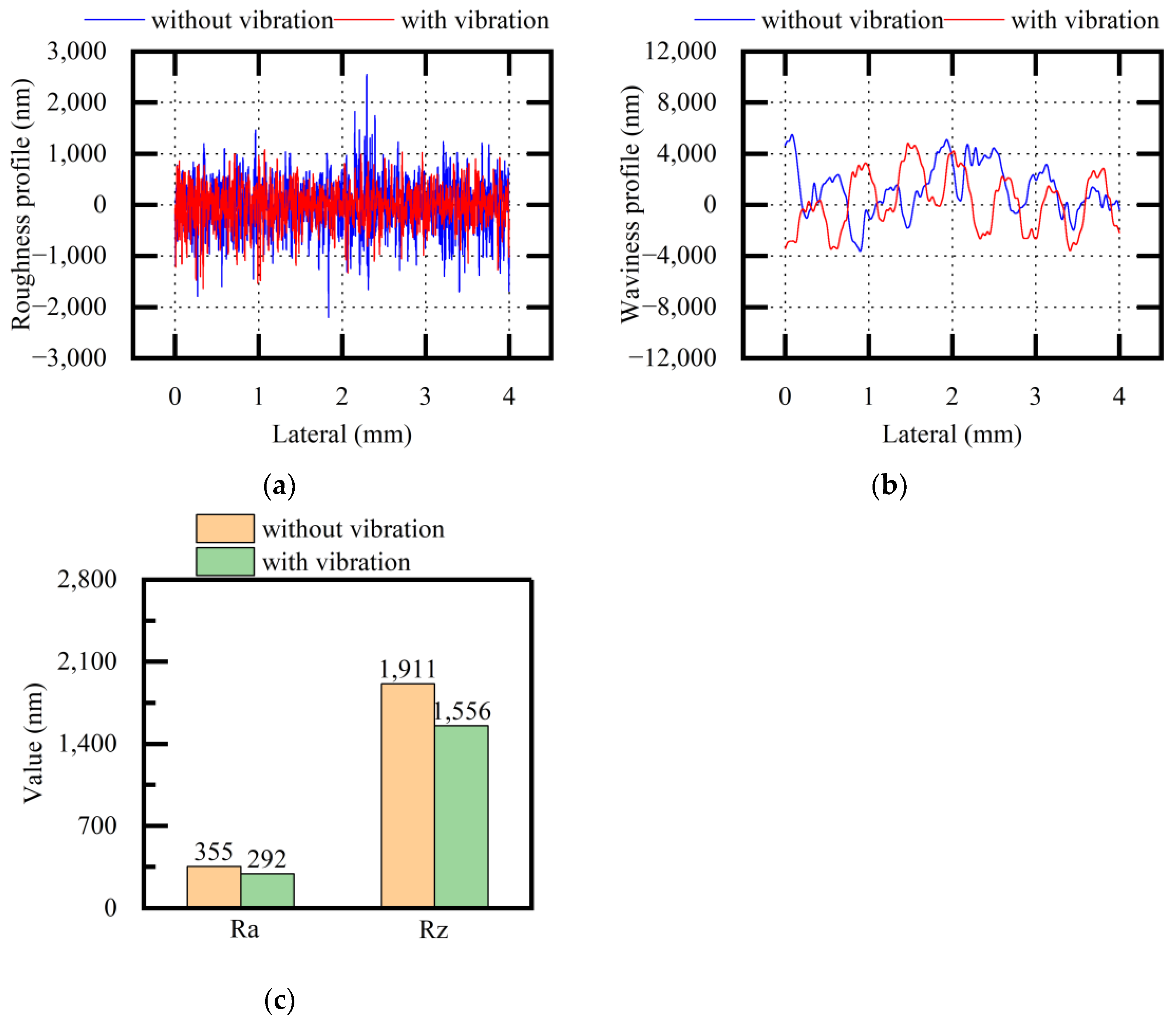
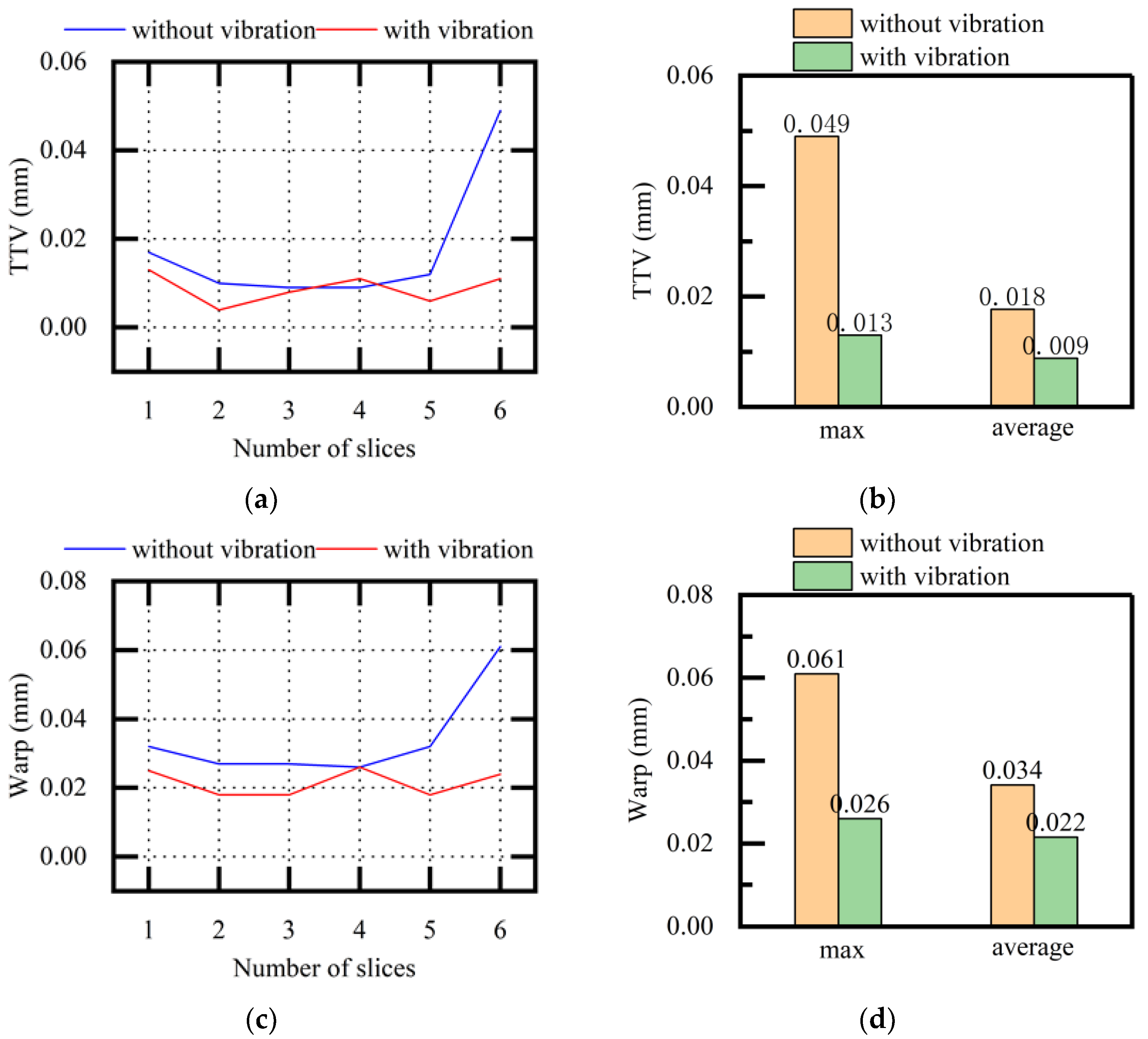
3.2. Study on the Surface Quality of Vibration-Assisted Sawing
- The abrasive particles produced a polishing effect on the surfaces during vibration-assisted sawing;
- Vibration assistance could reduce the surface roughness values of Ra and Rz for different materials;
- The worse the stability of the surface quality in conventional sawing, the more significant the optimization effect of vibration assistance on quality difference.
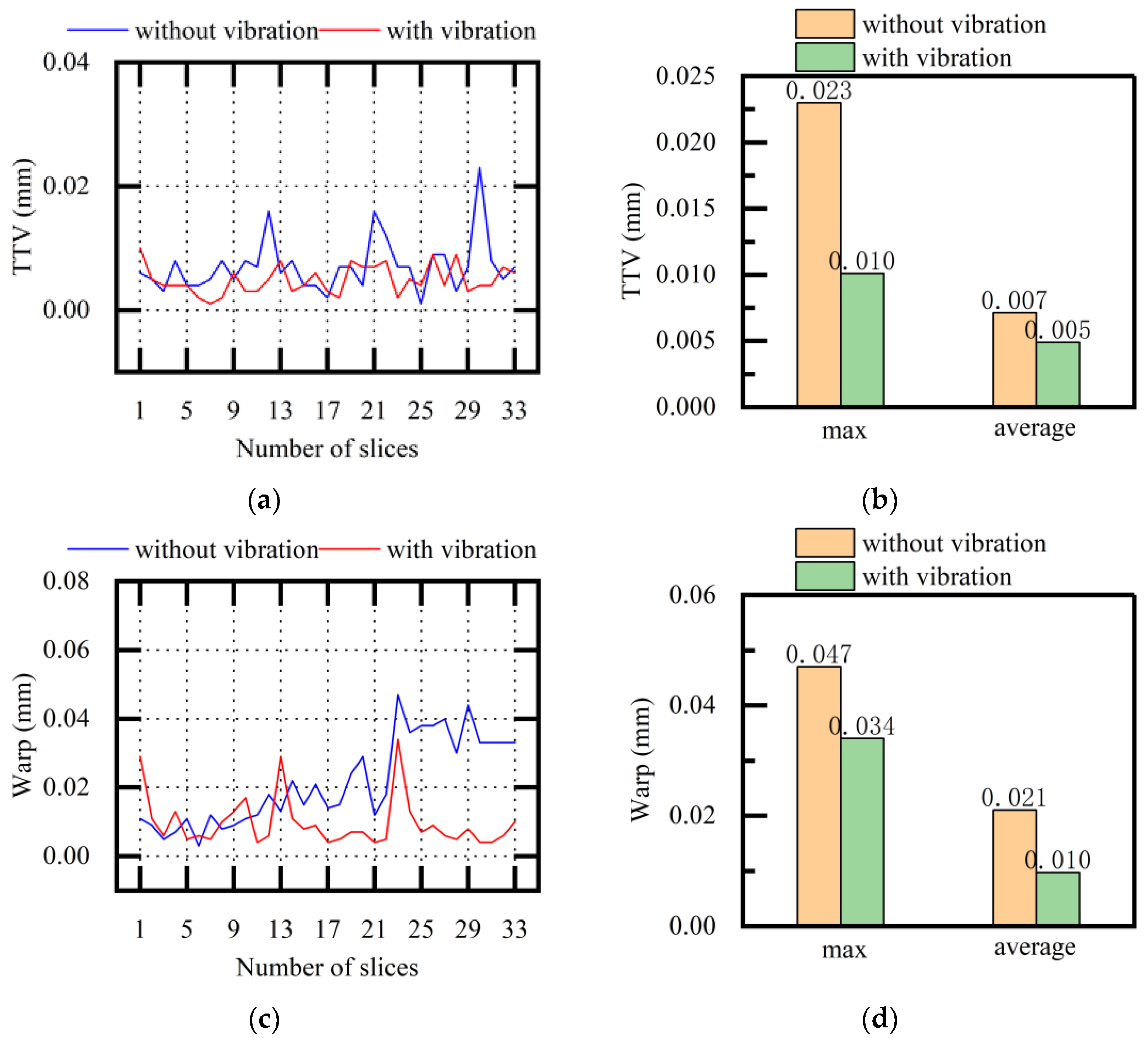
3.3. Experimental Results of Vibration-Assisted Multi-Wire Sawing
4. Conclusions
- In wire sawing, the deformation of the wire saw and the vibration assistance affected each other;
- During vibration-assisted wire sawing, the sawing forces could be reduced by different sawing parameters;
- Vibration frequency, feed speed, wire speed, and wire tension were important factors that affected the sawing force, and the effect of vibration assistance on the sawing force was comprehensively affected by the sawing parameters and vibration parameters;
- The surface roughness, quality difference on the same surface, TTV, and warp were all reduced during vibration-assisted wire sawing;
- It was predicted that the polishing effect of abrasive particles on the surface would be enhanced and that chip removal would be improved when using vibration-assisted sawing;
- The selection and matching of materials, vibration parameters, and sawing parameters were based on the prediction force model and the experiments described in this paper.
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Yang, C.; Wu, H.; Melkote, S.; Danyluk, S. Comparative Analysis of Fracture Strength of Slurry and Diamond Wire Sawn Multicrystalline Silicon Solar Wafers. Adv. Eng. Mater. 2013, 15, 358–365. [Google Scholar] [CrossRef]
- Kumar, A.; Melkote, S.N. The chemo-mechanical effect of sawing fluid on material removal in diamond scribing of silicon. Appl. Phys. Lett. 2017, 111, 011901. [Google Scholar] [CrossRef]
- Gao, Y.; Ge, P.; Zhang, L.; Bi, W. Material removal and surface generation mechanisms in diamond wire sawing of silicon crystal. Mater. Sci. Semicond. Process. 2019, 103, 104642. [Google Scholar] [CrossRef]
- Clark, W.I.; Shih, A.J.; Lemaster, R.L.; McSpadden, S.B. Fixed abrasive diamond wire machining—Part II: Experiment design and results. Int. J. Mach. Tools Manuf. 2003, 43, 533–542. [Google Scholar] [CrossRef]
- Hardin, C.W.; Qu, J.; Shih, A.J. Fixed abrasive diamond wire saw slicing of single-crystal silicon carbide wafers. Mater. Manuf. Process. 2004, 19, 355–367. [Google Scholar] [CrossRef]
- Ge, P.Q.; Zhang, L.; Gao, W.; Liu, Z.C. Development of endless diamond wire saw and sawing experiments. Mater. Sci. Forum 2004, 471–472, 481–484. [Google Scholar] [CrossRef]
- Pala, U.; Kuster, F.; Wegener, K. Characterization of electroplated diamond wires and the resulting ingot quality in silicon sawing. J. Mater. Process. Technol. 2020, 276, 116390. [Google Scholar] [CrossRef]
- Huang, H.; Li, X.X.; Xu, X.P. An Experimental Research on the Force and Energy During the Sapphire Sawing Using Reciprocating Electroplated Diamond Wire Saw. J. Manuf. Sci. Eng. 2017, 139, 121011. [Google Scholar] [CrossRef]
- Wang, P.; Ge, P.; Gao, Y.; Bi, W. Prediction of sawing force for single-crystal silicon carbide with fixed abrasive diamond wire saw. Mater. Sci. Semicond. Process. 2017, 63, 25–32. [Google Scholar] [CrossRef]
- Wang, P.; Ge, P.; Li, Z.; Ge, M.; Gao, Y. A scratching force model of diamond abrasive particles in wire sawing of single crystal sic. Mater. Sci. Semicond. Process. 2017, 68, 21–29. [Google Scholar] [CrossRef]
- Wu, C.; Jiang, Z.; Fan, W.; Chen, L. Finite element analysis of multi-wire saw silicon rods with consolidated abrasive diamonds. Int. J. Adv. Manuf. Technol. 2017, 90, 241–248. [Google Scholar] [CrossRef]
- Li, S.; Tang, A.; Liu, Y.; Wang, J.; Cui, D.; Landers, R.G. Analytical Force Modeling of Fixed Abrasive Diamond Wire Saw Machining With Application to SiC Monocrystal Wafer Processing. J. Manuf. Sci. Eng. 2017, 139, 041003. [Google Scholar] [CrossRef]
- Li, S.; Wang, J.; Tang, A.; Landers, R.G. Force modeling of silicon monocrystal wire saw machining. In Proceedings of the International Symposium on Flexible Automation, Cleveland, OH, USA, 1–3 August 2016. [Google Scholar]
- Yang, S.; Zhang, L.; Wu, Z. Subsurface damage minimization of KDP crystals. Appl. Surf. Sci. 2022, 604, 154592. [Google Scholar] [CrossRef]
- Shi, J.; Wang, J.; Yi, X.; Lu, Y.; Hua, D.; Zhou, Q.; Fan, X. Nanoscratching-induced plastic deformation mechanism and tribology behavior of Cu/Ta bilayer and multilayer by a molecular dynamics study. Appl. Surf. Sci. 2022, 586, 152775. [Google Scholar] [CrossRef]
- Wang, B.; Zhang, Z.; Chang, K.; Cui, J.; Rosenkranz, A.; Yu, J.; Guo, D. New Deformation-Induced Nanostructure in Silicon. Nano Lett. 2018, 18, 4611–4617. [Google Scholar] [CrossRef]
- Zhang, Z.; Wang, X.; Meng, F.; Liu, D.; Huang, S.; Cui, J.; Wen, W. Origin and evolution of a crack in silicon induced by a single grain grinding. J. Manuf. Process. 2022, 75, 617–626. [Google Scholar] [CrossRef]
- Zhang, Z.; Huo, F.; Zhang, X.; Guo, D. Fabrication and size prediction of crystalline nanoparticles of silicon induced by nanogrinding with ultrafine diamond grits. Scr. Mater. 2012, 67, 657–660. [Google Scholar] [CrossRef]
- Zhang, Z.; Wang, B.; Kang, R.; Zhang, B.; Guo, D. Changes in surface layer of silicon wafers from diamond scratching. Cirp Ann. Manuf. Technol. 2015, 64, 349–352. [Google Scholar] [CrossRef]
- Zan, Z.; Guo, K.; Sun, J.; Lu, Y.; Yang, B.; Jia, X.; Xi, J. Investigation on scratching force and material removal mechanism of 3D SiCf/C-SiC composites during single grain scratching. J. Eur. Ceram. Soc. 2022, 42, 5366–5379. [Google Scholar] [CrossRef]
- Zhang, L.; Wang, S.; Shao, Z.; Lv, Z. Electroplated Diamond Wire Saw Applying Ultrasonic Vibration to Cut Polysilicon Experiment and Simulation Analysis. Presented at the 3rd International Conference on Advanced Design and Manufacturing Engineering (ADME 2013), Anshan, China, 13–14 July 2013. [Google Scholar]
- Li, S.; Wan, B.; Landers, R.G. Surface roughness optimization in processing SiC monocrystal wafers by wire saw machining with ultrasonic vibration. Proc. Inst. Mech. Eng. Part B J. Eng. Manuf. 2014, 228, 725–739. [Google Scholar] [CrossRef]
- Li, L.; Yang, S.D.; Du, B.F.; Xu, S.Y.; Li, J.S. Study on Processing Process of SiC Single Crystal Cut with Wire Saw Excited by Ultrasonic. Presented at the 4th International Conference on Mechanical, Control and Computer Engineering (ICMCCE), Hohhot, China, 25–27 October 2019. [Google Scholar]
- Wang, Y.; Li, D.-L.; Ding, Z.-J.; Liu, J.-G.; Wang, R. Modeling and verifying of sawing force in ultrasonic vibration assisted diamond wire sawing (UAWS) based on impact load. Int. J. Mech. Sci. 2019, 164, 105161. [Google Scholar] [CrossRef]
- Wang, Y.; Zhao, B.; Huang, S.; Qian, Z. Study on the subsurface damage depth of monocrystalline silicon in ultrasonic vibration assisted diamond wire sawing. Eng. Fract. Mech. 2021, 258, 108077. [Google Scholar] [CrossRef]
- Wang, Y.; Zhang, S.; Dong, G.; Su, J.; Qian, Z.; Zhou, J. Theoretical study on sawing force of ultrasonic vibration assisted diamond wire sawing (UAWS) based on abrasives wear. Wear 2022, 496, 204291. [Google Scholar] [CrossRef]
- Yan, L.; Zhang, X.; Li, H.; Zhang, Q. Machinability improvement in three-dimensional (3D) ultrasonic vibration assisted diamond wire sawing of SiC. Ceram. Int. 2022, 48, 8051–8068. [Google Scholar] [CrossRef]
- Huang, X.; Huang, H.; Guo, H. Simulation and Experimental Research on the Slicing Temperature of the Sapphire with Diamond Wire. Int. J. Comput. Methods 2019, 16, 1843003. [Google Scholar] [CrossRef]
- Wang, Y.; Song, L.-X.; Liu, J.-G.; Wang, R.; Zhao, B.-C. Investigation on the sawing temperature in ultrasonic vibration assisted diamond wire sawing monocrystalline silicon. Mater. Sci. Semicond. Process. 2021, 135, 106070. [Google Scholar] [CrossRef]
- Qiu, J.; Li, X.; Ge, R.; Liu, C. Surface formation, morphology, integrity and wire marks in diamond wire slicing of mono-crystalline silicon in the photovoltaic industry. Wear 2022, 488–489, 204186. [Google Scholar] [CrossRef]
- Gao, Y.; Ge, P.; Liu, T. Experiment study on electroplated diamond wire saw slicing single-crystal silicon. Mater. Sci. Semicond. Process. 2016, 56, 106–114. [Google Scholar] [CrossRef]
- Bhagavat, S.; Kao, I. A finite element analysis of temperature variation in silicon wafers during wire saw slicing. Int. J. Mach. Tools Manuf. 2008, 48, 95–106. [Google Scholar] [CrossRef]







| /Hz | /mm/h | /m/s | /N | /mm | /mm | /mm |
|---|---|---|---|---|---|---|
| 0–50 | 0–60 | 0–20 | 20–40 | 100–430 | 0–180 | 0.05–0.15 |
| Material | Variable | /Hz | /m/s | /mm/h | /N | /mm | /mm |
|---|---|---|---|---|---|---|---|
| HT250 | Frequency | 0 | 10.0 | 60 | 30 | 120 | 200 |
| 10 | |||||||
| 20 | |||||||
| 30 | |||||||
| 40 | |||||||
| 50 | |||||||
| HT250 | Wire speed | 50 | 10.0 | 60 | 30 | 120 | 200 |
| 12.5 | |||||||
| 15.0 | |||||||
| 17.5 | |||||||
| 20.0 | |||||||
| HT250 | Feed speed | 50 | 10.0 | 40 | 30 | 120 | 200 |
| 45 | |||||||
| 50 | |||||||
| 55 | |||||||
| 60 | |||||||
| HT250 | Wire tension | 50 | 15.0 | 60 | 20 | 120 | 200 |
| 25 | |||||||
| 30 | |||||||
| 35 | |||||||
| 40 |
| Material | Variable | /Hz | /m/s | /mm/h | /N | /mm | /mm |
|---|---|---|---|---|---|---|---|
| HT250 | Frequency | 0 | 10.0 | 60 | 30 | 120 | 200 |
| 50 | |||||||
| Stainless steel | Frequency | 0 | 10.0 | 60 | 30 | 120 | 200 |
| 50 | |||||||
| NdFeB | Frequency | 0 | 10.0 | 60 | 30 | 100 | 200 |
| 50 |
| Material | Variable | /Hz | /m/s | /mm/h | /N | /mm | /mm |
|---|---|---|---|---|---|---|---|
| NdFeB | Frequency | 0 | 15.0 | 40 | 30 | 100 | 430 |
| 50 | |||||||
| SiC | Frequency | 0 | 20.0 | 8 | 35 | 22 | 430 |
| 50 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Zhang, C.; Dong, Z.; Zhao, Y.; Liu, Z.; Wu, S.; Yang, J. Sawing Force Prediction Model and Experimental Study on Vibration-Assisted Diamond Wire Sawing. Micromachines 2022, 13, 2026. https://doi.org/10.3390/mi13112026
Zhang C, Dong Z, Zhao Y, Liu Z, Wu S, Yang J. Sawing Force Prediction Model and Experimental Study on Vibration-Assisted Diamond Wire Sawing. Micromachines. 2022; 13(11):2026. https://doi.org/10.3390/mi13112026
Chicago/Turabian StyleZhang, Chenpu, Zhikui Dong, Yanheng Zhao, Ziliang Liu, Shang Wu, and Jiahao Yang. 2022. "Sawing Force Prediction Model and Experimental Study on Vibration-Assisted Diamond Wire Sawing" Micromachines 13, no. 11: 2026. https://doi.org/10.3390/mi13112026
APA StyleZhang, C., Dong, Z., Zhao, Y., Liu, Z., Wu, S., & Yang, J. (2022). Sawing Force Prediction Model and Experimental Study on Vibration-Assisted Diamond Wire Sawing. Micromachines, 13(11), 2026. https://doi.org/10.3390/mi13112026






