Precise Droplet Dispensing in Digital Microfluidics with Dumbbell-Shaped Electrodes
Abstract
:1. Introduction
2. Materials and Methods
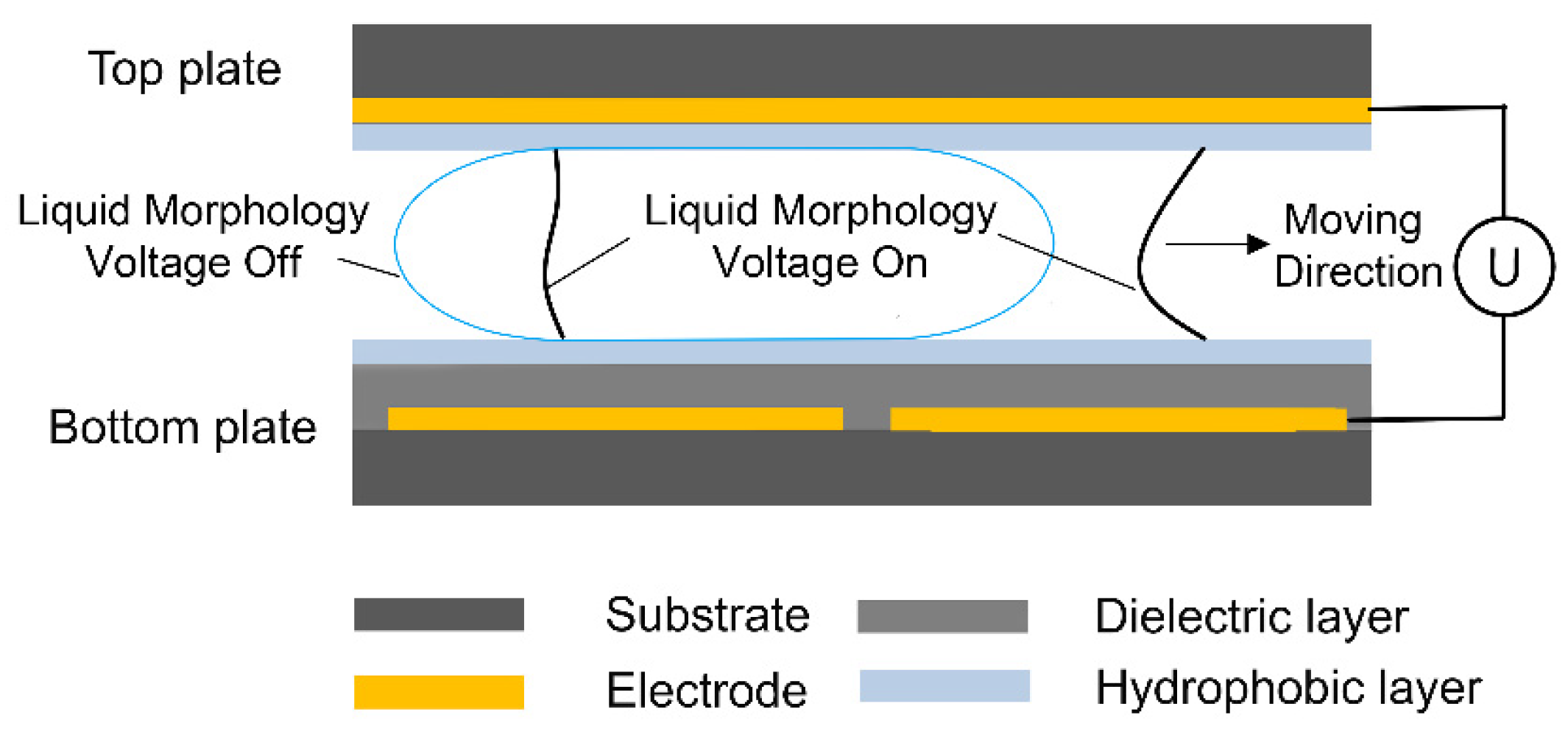
2.1. EWOD Chip on ITO Glass
2.2. Measurement of Droplet Volumes
3. Results
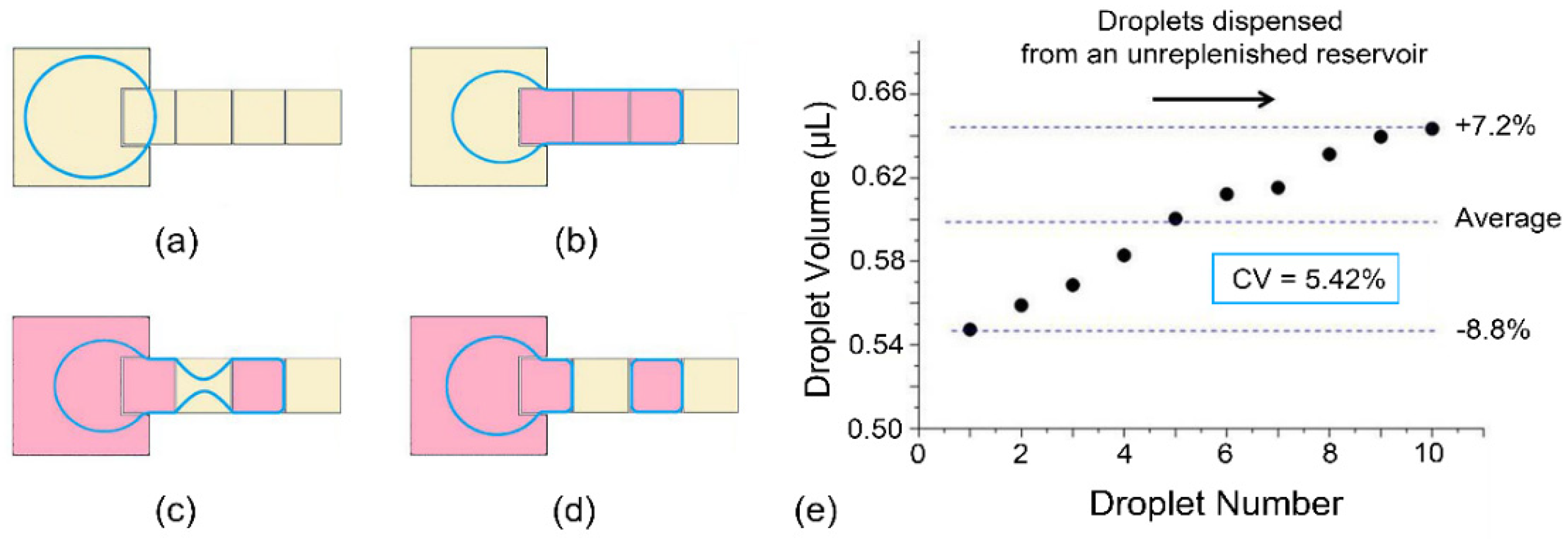
3.1. Droplet Dispensing with the Conventional Electrowetting Electrodes
3.2. Square Sub-Electrode Design for Droplet Dispensing
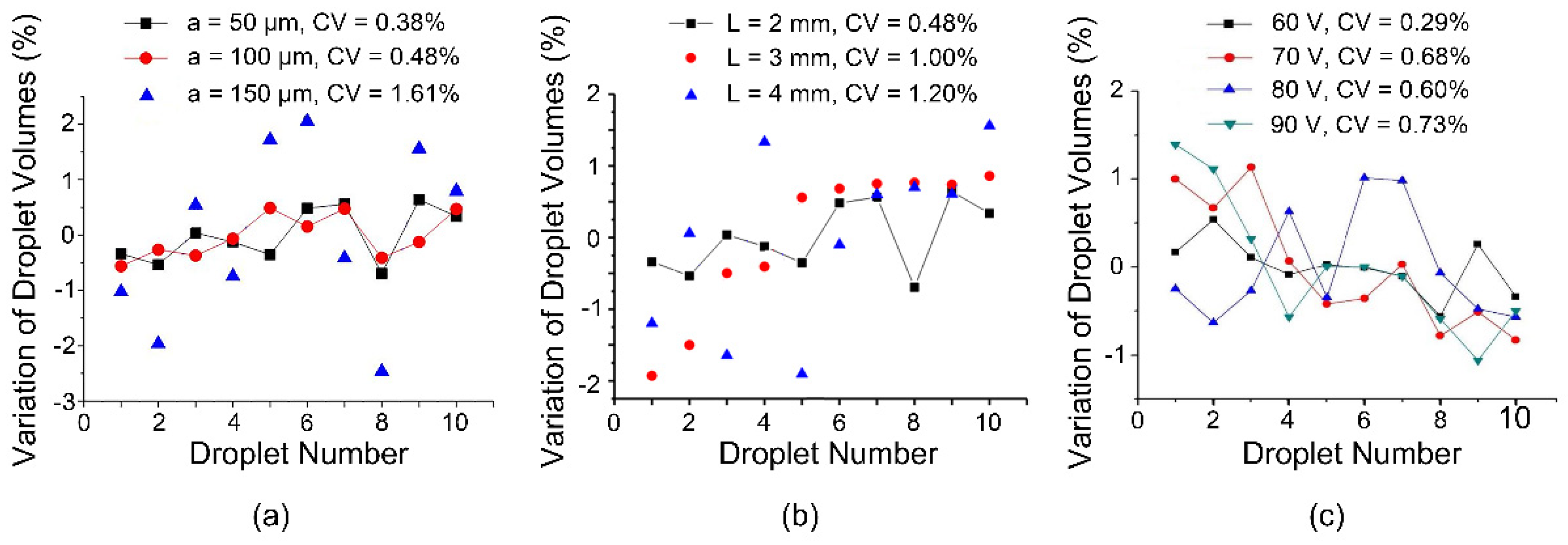
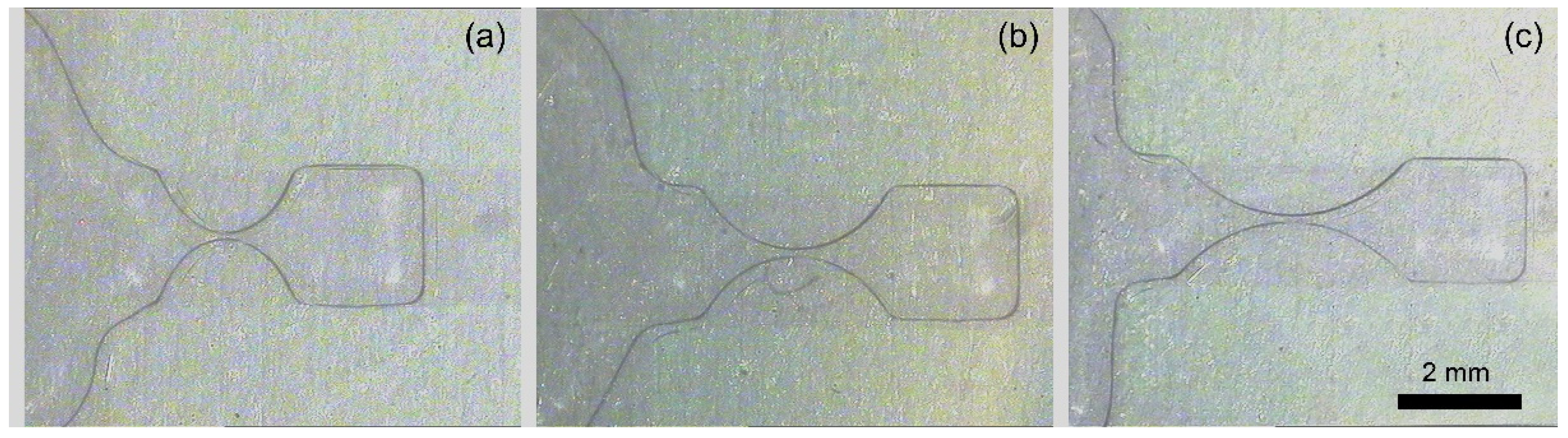
3.3. Dumbbell-Shaped Sub-Electrode Design for Droplet Dispensing
4. Conclusions
Funding
Institutional Review Board Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Dittrich, P.S.; Manz, A. Lab-on-a-chip: Microfluidics in drug discovery. Nat. Rev. Drug Discov. 2006, 5, 210–218. [Google Scholar] [CrossRef] [PubMed]
- Garstecki, P.; Fuerstman, M.J.; Stone, H.A.; Whitesides, G.M. Formation of droplets and bubbles in a microfluidic T-junction–scaling and mechanism of break-up. Lab Chip 2006, 6, 437–446. [Google Scholar] [CrossRef] [PubMed]
- Zhou, H.; Yao, S. A facile on-demand droplet microfluidic system for lab-on-a-chip applications. Microfluid. Nanofluid. 2014, 16, 667–675. [Google Scholar] [CrossRef]
- Baroud, C.N.; Gallaire, F.; Dangla, R. Dynamics of microfluidic droplets. Lab Chip 2010, 10, 2032–2045. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Böhm, S.; Timmer, B.; Olthuis, W.; Bergveld, P. A closed-loop controlled electrochemically actuated micro-dosing system. J. Micromech. Microeng. 2000, 10, 498. [Google Scholar] [CrossRef]
- Vestad, V.; Marr, D.W.M.; Oakey, J. Flow control for capillary-pumped microfluidic systems. J. Micromech. Microeng. 2004, 14, 1503. [Google Scholar] [CrossRef]
- Pollack, M.G.; Shendorov, A.D.; Fair, R.B. Electrowetting-based actuation of droplets for integrated microfluidics. Lab Chip 2002, 2, 96–101. [Google Scholar] [CrossRef] [PubMed]
- Nisisako, T.; Torii, T.; Higuchi, T. Droplet formation in a microchannel network. Lab Chip 2002, 2, 24–26. [Google Scholar] [CrossRef] [PubMed]
- Gong, J.; Kim, C.-J. All-electronic droplet generation on-chip with real-time feedback control for EWOD digital microfluidics. Lab Chip 2008, 8, 898–906. [Google Scholar] [CrossRef] [PubMed]
- Berthier, J.; Clementz, P.; Raccurt, O.; Jary, D.; Claustre, P.; Peponnet, C.; Fouillet, Y. Computer aided design of an EWOD microdevice. Sens. Actuators A 2006, 127, 283–294. [Google Scholar] [CrossRef]
- Luk, V.N.; Wheeler, A.R. A digital microfluidic approach to proteomic sample processing. Anal. Chem. 2009, 81, 4524–4530. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Ding, H.; Sadeghi, S.; Shah, G.J.; Chen, S.; Keng, P.Y.; Kim, C.-J.; van Dam, R.M. Accurate dispensing of volatile reagents on demand for chemical reactions in EWOD chips. Lab Chip 2012, 12, 3331–3340. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Ren, H.; Fair, R.B.; Pollack, M.G. Automated on-chip droplet dispensing with volume control by electro-wetting actuation and capacitance metering. Sens. Actuators B 2004, 98, 319–327. [Google Scholar] [CrossRef]
- Eydelnant, I.A.; Uddayasankar, U.; Li, B.; Liao, M.W.; Wheeler, A.R. Virtual microwells for digital microfluidic reagent dispensing and cell culture. Lab Chip 2012, 12, 750–757. [Google Scholar] [CrossRef] [PubMed]
- Chen, J.; Yu, Y.; Zhang, K.; Wu, C.; Liu, A.; Zhou, J. Study of cyanoethyl pullulan as insulator for electrowetting. Sens. Actuators B 2014, 199, 183–189. [Google Scholar] [CrossRef]
- Cho, S.K.; Moon, H.; Kim, C.J. Creating, transporting, cutting, and merging liquid droplets by electrowetting-based actuation for digital microfluidic circuits. Microelectromech. Syst. 2003, 12, 70–80. [Google Scholar]
- Elvira, K.S.; Leatherbarrow, R.; Edel, J.; de Mello, A. Droplet dispensing in digital microfluidic devices: Assessment of long-term reproducibility. Biomicrofluidics 2012, 6, 022003. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Nikapitiya, N.Y.; Jagath, B.; Nahar, M.M.; Moon, H. Accurate, consistent, and fast droplet splitting and dispensing in electrowetting on dielectric digital microfluidics. Micro. Nano Syst. Lett. 2017, 5, 24. [Google Scholar] [CrossRef] [Green Version]
- Guan, Y.; Tu, J.; Li, B.; Fu, J.; Zhu, M.; Chen, X.; Zhou, C. Stripped electrode based electrowetting-on-dielectric digital microfluidics for precise and controllable parallel microdrop generation. Langmuir 2020, 36, 9540–9550. [Google Scholar] [CrossRef] [PubMed]
- Wang, H.; Chen, L. Novel electrodes for precise and accurate droplet dispensing and splitting in digital microfluidics. Nanotechnol. Rev. 2021, 10, 857–869. [Google Scholar] [CrossRef]


Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the author. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Wang, W. Precise Droplet Dispensing in Digital Microfluidics with Dumbbell-Shaped Electrodes. Micromachines 2022, 13, 484. https://doi.org/10.3390/mi13030484
Wang W. Precise Droplet Dispensing in Digital Microfluidics with Dumbbell-Shaped Electrodes. Micromachines. 2022; 13(3):484. https://doi.org/10.3390/mi13030484
Chicago/Turabian StyleWang, Wei. 2022. "Precise Droplet Dispensing in Digital Microfluidics with Dumbbell-Shaped Electrodes" Micromachines 13, no. 3: 484. https://doi.org/10.3390/mi13030484
APA StyleWang, W. (2022). Precise Droplet Dispensing in Digital Microfluidics with Dumbbell-Shaped Electrodes. Micromachines, 13(3), 484. https://doi.org/10.3390/mi13030484




