Electric-Driven Polarization Meta-Optics for Tunable Edge-Enhanced Images
Abstract
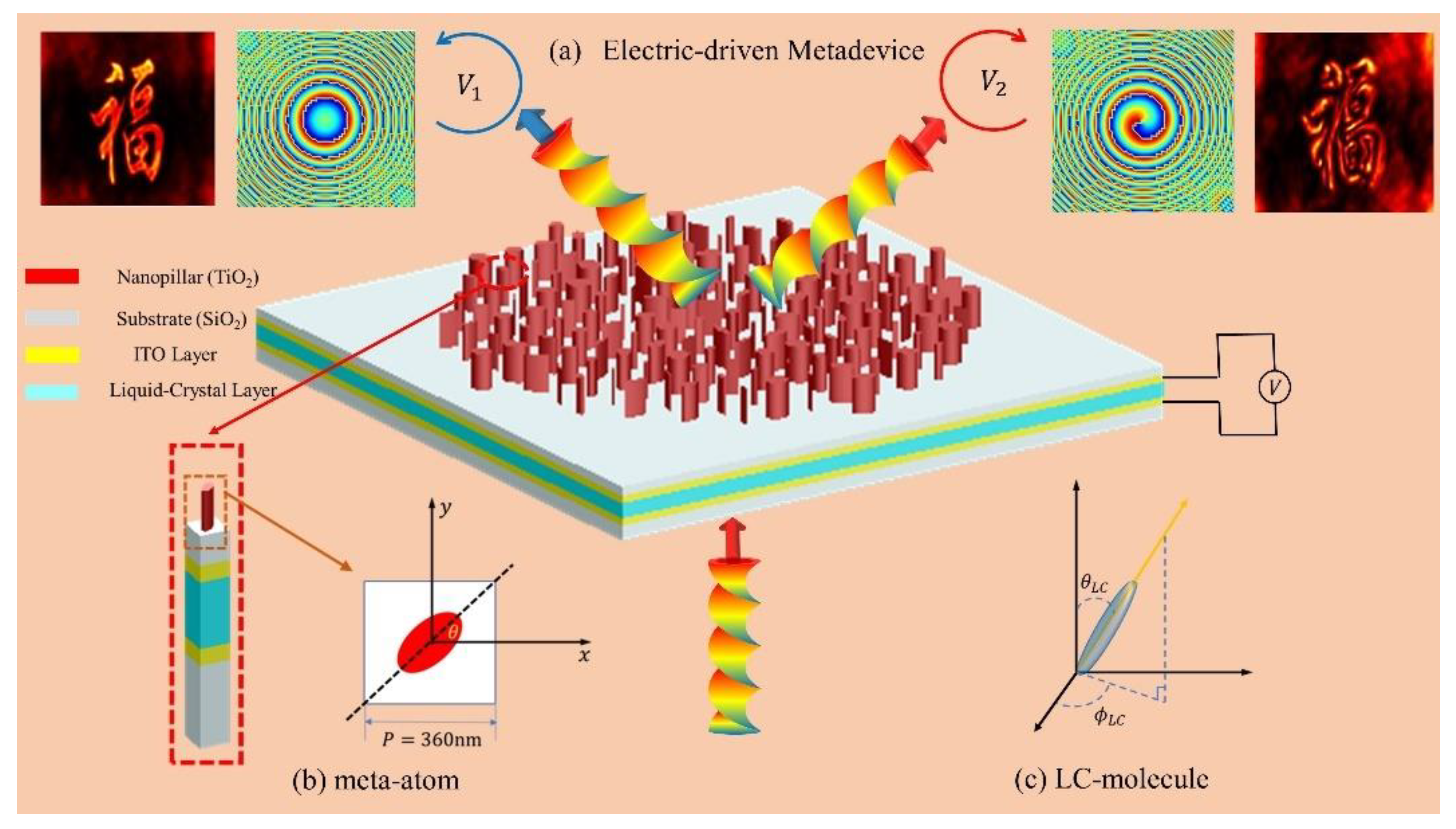
:1. Introduction
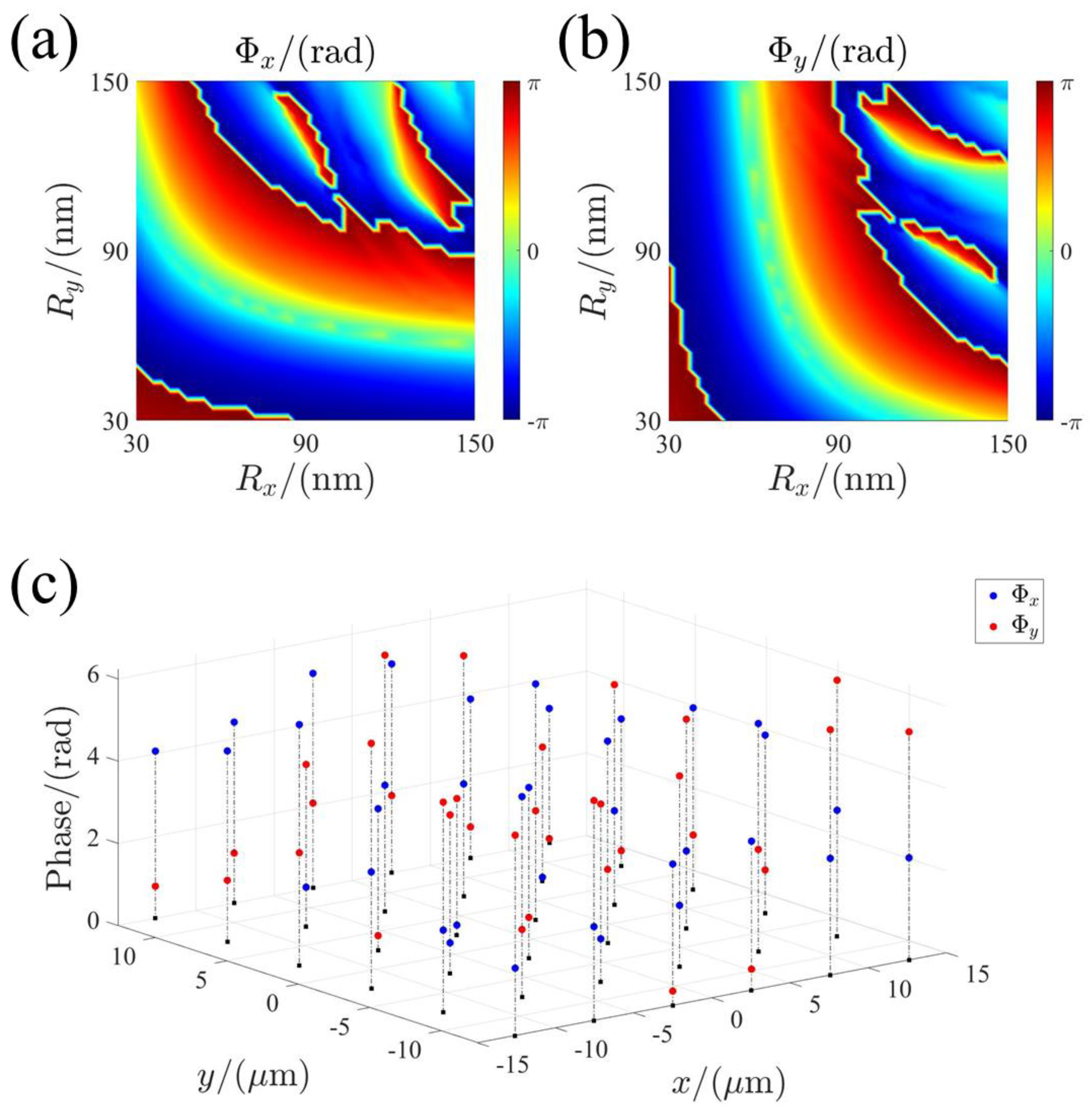
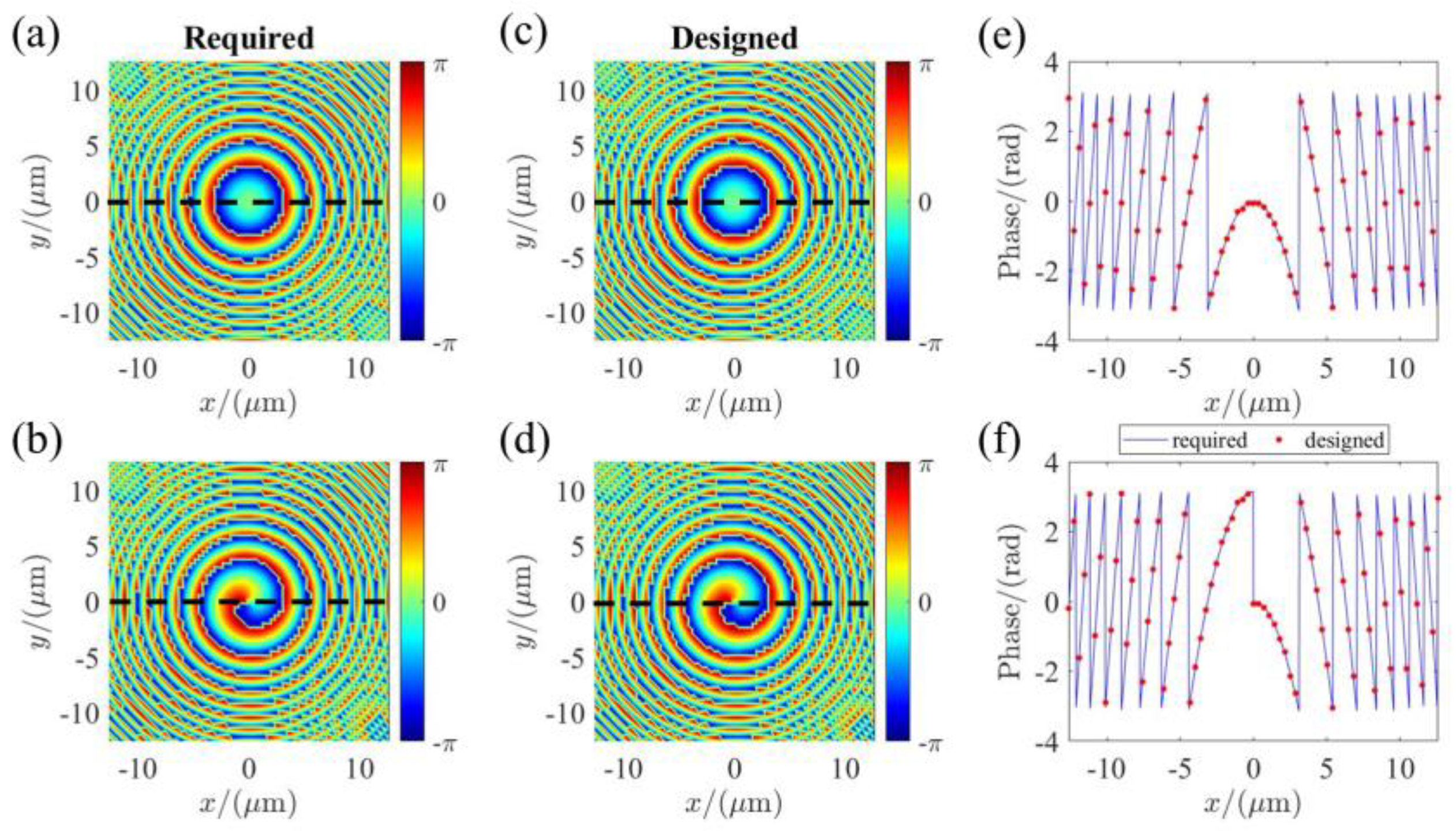
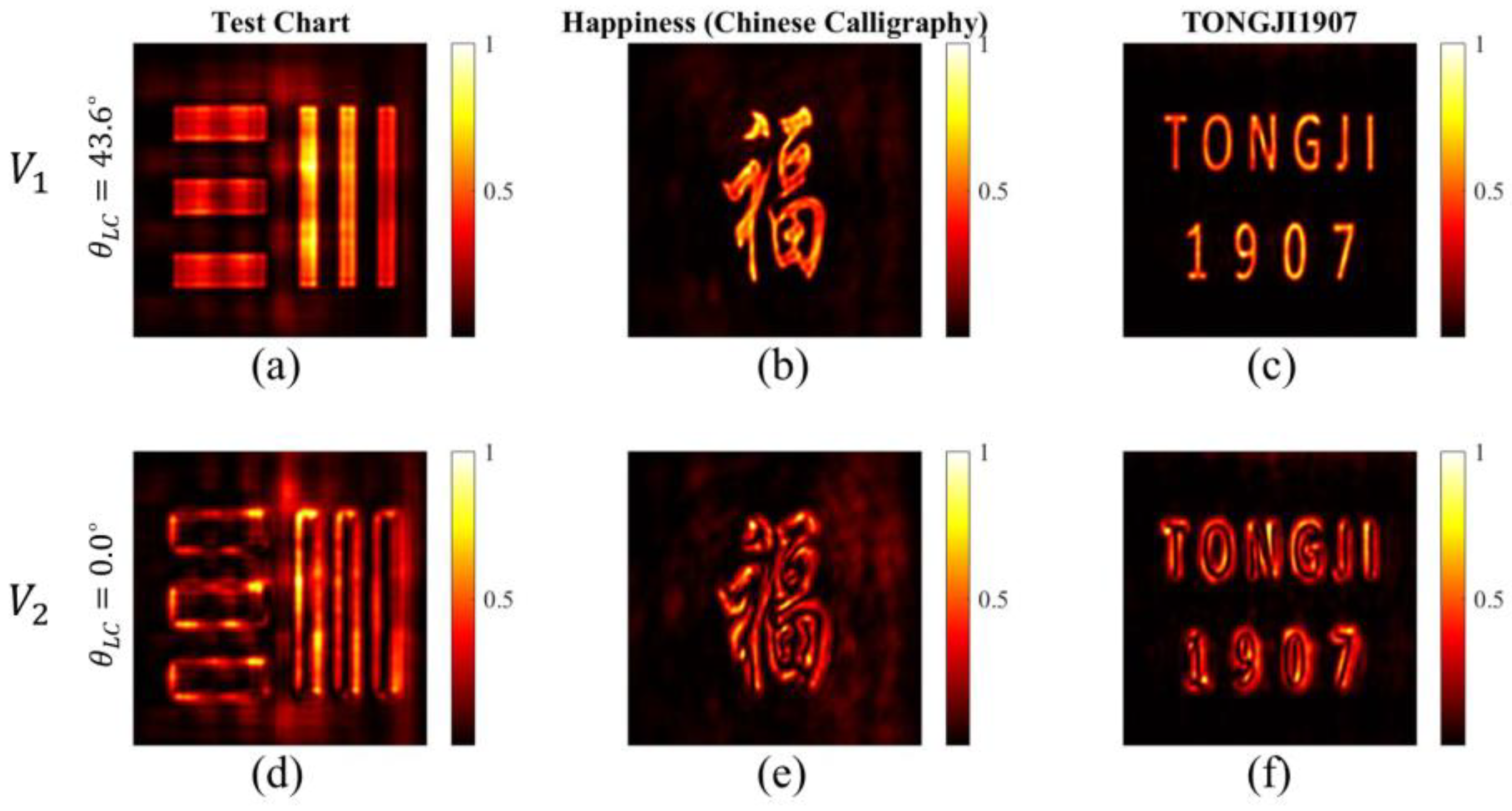
2. Design and Numerical Results
3. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Wu, J.; Lin, X.; Guo, Y.; Liu, J.; Fang, L.; Jiao, S.; Dai, Q. Analog Optical Computing for Artificial Intelligence. Engineering, 2021; in press. [Google Scholar]
- Han, J.-H.; Boeuf, F.; Fujikata, J.; Takahashi, S.; Takagi, S.; Takenaka, M. Efficient low-loss InGaAsP/Si hybrid MOS optical modulator. Nat. Photonics 2017, 11, 486–490. [Google Scholar] [CrossRef]
- Solli, D.R.; Jalali, B. Analog optical computing. Nat. Photonics 2015, 9, 704–706. [Google Scholar] [CrossRef]
- Xu, H.X.; Hu, G.; Han, L.; Jiang, M.; Huang, Y.; Li, Y.; Yang, X.; Ling, X.; Chen, L.; Zhao, J. Chirality-assisted high-efficiency metasurfaces with independent control of phase, amplitude, and polarization. Adv. Opt. Mater. 2019, 7, 1801479. [Google Scholar] [CrossRef]
- Liu, L.; Zhang, X.; Kenney, M.; Su, X.; Xu, N.; Ouyang, C.; Shi, Y.; Han, J.; Zhang, W.; Zhang, S. Broadband metasurfaces with simultaneous control of phase and amplitude. Adv. Mater. 2014, 26, 5031–5036. [Google Scholar] [CrossRef]
- Zhang, J.; Wei, X.; Rukhlenko, I.D.; Chen, H.-T.; Zhu, W. Electrically tunable metasurface with independent frequency and amplitude modulations. ACS Photonics 2019, 7, 265–271. [Google Scholar] [CrossRef]
- Bao, Y.; Weng, Q.; Li, B. Conversion between Arbitrary Amplitude, Phase, and Polarization with Minimal Degrees of Freedom of Metasurface. Laser Photonics Rev. 2022, 16, 2100280. [Google Scholar] [CrossRef]
- Overvig, A.C.; Shrestha, S.; Malek, S.C.; Lu, M.; Stein, A.; Zheng, C.; Yu, N. Dielectric metasurfaces for complete and independent control of the optical amplitude and phase. Light Sci. Appl. 2019, 8, 92. [Google Scholar] [CrossRef]
- Wu, T.; Zhang, X.; Xu, Q.; Plum, E.; Chen, K.; Xu, Y.; Lu, Y.; Zhang, H.; Zhang, Z.; Chen, X. Dielectric Metasurfaces for Complete Control of Phase, Amplitude, and Polarization. Adv. Opt. Mater. 2022, 10, 2101223. [Google Scholar] [CrossRef]
- Liu, M.; Zhu, W.; Huo, P.; Feng, L.; Song, M.; Zhang, C.; Chen, L.; Lezec, H.J.; Lu, Y.; Agrawal, A. Multifunctional metasurfaces enabled by simultaneous and independent control of phase and amplitude for orthogonal polarization states. Light Sci. Appl. 2021, 10, 107. [Google Scholar] [CrossRef]
- Minatti, G.; Caminita, F.; Martini, E.; Sabbadini, M.; Maci, S. Synthesis of modulated-metasurface antennas with amplitude, phase, and polarization control. IEEE Trans. Antennas Propag. 2016, 64, 3907–3919. [Google Scholar] [CrossRef]
- Bao, L.; Wu, R.Y.; Fu, X.; Ma, Q.; Bai, G.D.; Mu, J.; Jiang, R.; Cui, T.J. Multi-beam forming and controls by metasurface with phase and amplitude modulations. IEEE Trans. Antennas Propag. 2019, 67, 6680–6685. [Google Scholar] [CrossRef]
- Ding, G.; Chen, K.; Luo, X.; Qian, G.; Zhao, J.; Jiang, T.; Feng, Y. Direct routing of intensity-editable multi-beams by dual geometric phase interference in metasurface. Nanophotonics 2020, 9, 2977–2987. [Google Scholar] [CrossRef]
- Wesemann, L.; Davis, T.J.; Roberts, A. Meta-optical and thin film devices for all-optical information processing. Appl. Phys. Rev. 2021, 8, 031309. [Google Scholar] [CrossRef]
- Zhou, Y.; Zheng, H.; Kravchenko, I.I.; Valentine, J. Flat optics for image differentiation. Nat. Photonics 2020, 14, 316–323. [Google Scholar] [CrossRef]
- Silva, A.; Monticone, F.; Castaldi, G.; Galdi, V.; Alù, A.; Engheta, N. Performing mathematical operations with metamaterials. Science 2014, 343, 160–163. [Google Scholar] [CrossRef]
- Li, S.-Q.; Xu, X.; Maruthiyodan Veetil, R.; Valuckas, V.; Paniagua-Domínguez, R.; Kuznetsov, A.I. Phase-only transmissive spatial light modulator based on tunable dielectric metasurface. Science 2019, 364, 1087–1090. [Google Scholar] [CrossRef] [Green Version]
- Bartholomew, R.; Williams, C.; Khan, A.; Bowman, R.; Wilkinson, T. Plasmonic nanohole electrodes for active color tunable liquid crystal transmissive pixels. Opt. Lett. 2017, 42, 2810–2813. [Google Scholar] [CrossRef] [Green Version]
- Buchnev, O.; Ou, J.; Kaczmarek, M.; Zheludev, N.; Fedotov, V. Electro-optical control in a plasmonic metamaterial hybridised with a liquid-crystal cell. Opt. Express 2013, 21, 1633–1638. [Google Scholar] [CrossRef] [Green Version]
- Li, J.; Yu, P.; Zhang, S.; Liu, N. Electrically-controlled digital metasurface device for light projection displays. Nat. Commun. 2020, 11, 3574. [Google Scholar] [CrossRef]
- Cordaro, A.; Kwon, H.; Sounas, D.; Koenderink, A.F.; Alù, A.; Polman, A. High-index dielectric metasurfaces performing mathematical operations. Nano Lett. 2019, 19, 8418–8423. [Google Scholar] [CrossRef] [Green Version]
- Wan, L.; Pan, D.; Feng, T.; Liu, W.; Potapov, A.A. A review of dielectric optical metasurfaces for spatial differentiation and edge detection. Front. Optoelectron. 2021, 14, 187–200. [Google Scholar] [CrossRef]
- Zhou, J.; Liu, S.; Qian, H.; Li, Y.; Luo, H.; Wen, S.; Zhou, Z.; Guo, G.; Shi, B.; Liu, Z. Metasurface enabled quantum edge detection. Sci. Adv. 2020, 6, eabc4385. [Google Scholar] [CrossRef]
- Zhou, J.; Qian, H.; Zhao, J.; Tang, M.; Wu, Q.; Lei, M.; Luo, H.; Wen, S.; Chen, S.; Liu, Z. Two-dimensional optical spatial differentiation and high-contrast imaging. Natl. Sci. Rev. 2021, 8, nwaa176. [Google Scholar] [CrossRef] [PubMed]
- Davis, T.; Eftekhari, F.; Gómez, D.; Roberts, A. Metasurfaces with asymmetric optical transfer functions for optical signal processing. Phys. Rev. Lett. 2019, 123, 013901. [Google Scholar] [CrossRef] [PubMed]
- Kwon, H.; Sounas, D.; Cordaro, A.; Polman, A.; Alù, A. Nonlocal metasurfaces for optical signal processing. Phys. Rev. Lett. 2018, 121, 173004. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Xiao, T.; Yang, H.; Yang, Q.; Xu, D.; Wang, R.; Chen, S.; Luo, H. Realization of tunable edge-enhanced images based on computing metasurfaces. Opt. Lett. 2022, 47, 925–928. [Google Scholar] [CrossRef]
- Huo, P.; Zhang, C.; Zhu, W.; Liu, M.; Zhang, S.; Zhang, S.; Chen, L.; Lezec, H.J.; Agrawal, A.; Lu, Y. Photonic spin-multiplexing metasurface for switchable spiral phase contrast imaging. Nano Lett. 2020, 20, 2791–2798. [Google Scholar] [CrossRef]
- Pérez, A.M.; Fabián, C.M.; Zurita, G.R. Spatial Filtering and Optical Tomography of Edge Enhancement of a Phase Object; Seventh Symposium Optics in Industry, Guadalajara, Jalisco, Mexico, International Society for Optics and Photonics: Guadalajara, Jalisco, Mexico, 2009; p. 74990Y. [Google Scholar]
- Yang, H.; Xie, Z.; He, H.; Zhang, Q.; Li, J.; Zhang, Y.; Yuan, X. Switchable imaging between edge-enhanced and bright-field based on a phase-change metasurface. Opt. Lett. 2021, 46, 3741–3744. [Google Scholar] [CrossRef]
- Badloe, T.; Kim, I.; Kim, Y.; Kim, J.; Rho, J. Electrically Tunable Bifocal Metalens with Diffraction-Limited Focusing and Imaging at Visible Wavelengths. Adv. Sci. 2021, 8, 2102646. [Google Scholar] [CrossRef]
- Kim, Y.; Lee, G.Y.; Sung, J.; Jang, J.; Lee, B. Spiral metalens for phase contrast imaging. Adv. Funct. Mater. 2022, 32, 2106050. [Google Scholar] [CrossRef]


Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Cheng, C.; Ou, K.; Yang, H.; Wan, H.; Wei, Z.; Wang, Z.; Cheng, X. Electric-Driven Polarization Meta-Optics for Tunable Edge-Enhanced Images. Micromachines 2022, 13, 541. https://doi.org/10.3390/mi13040541
Cheng C, Ou K, Yang H, Wan H, Wei Z, Wang Z, Cheng X. Electric-Driven Polarization Meta-Optics for Tunable Edge-Enhanced Images. Micromachines. 2022; 13(4):541. https://doi.org/10.3390/mi13040541
Chicago/Turabian StyleCheng, Cheng, Kai Ou, Hui Yang, Hengyi Wan, Zeyong Wei, Zhanshan Wang, and Xinbin Cheng. 2022. "Electric-Driven Polarization Meta-Optics for Tunable Edge-Enhanced Images" Micromachines 13, no. 4: 541. https://doi.org/10.3390/mi13040541
APA StyleCheng, C., Ou, K., Yang, H., Wan, H., Wei, Z., Wang, Z., & Cheng, X. (2022). Electric-Driven Polarization Meta-Optics for Tunable Edge-Enhanced Images. Micromachines, 13(4), 541. https://doi.org/10.3390/mi13040541






