Limit Efficiency of a Silicon Betavoltaic Battery with Tritium Source
Abstract
:1. Introduction
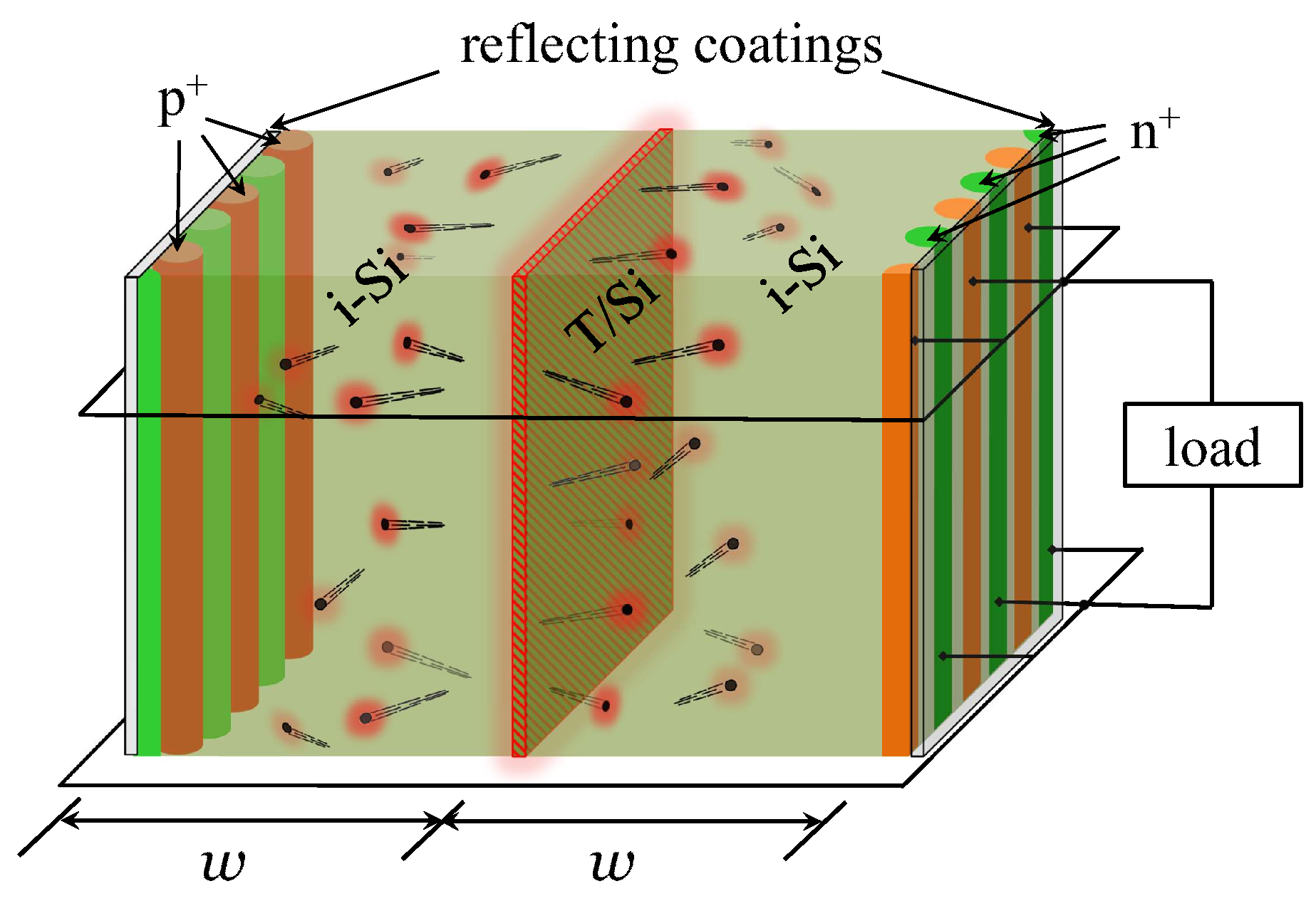
2. The Model
3. Thin Base Approximation
4. Analytical Evaluation of the Betaconversion Parameters
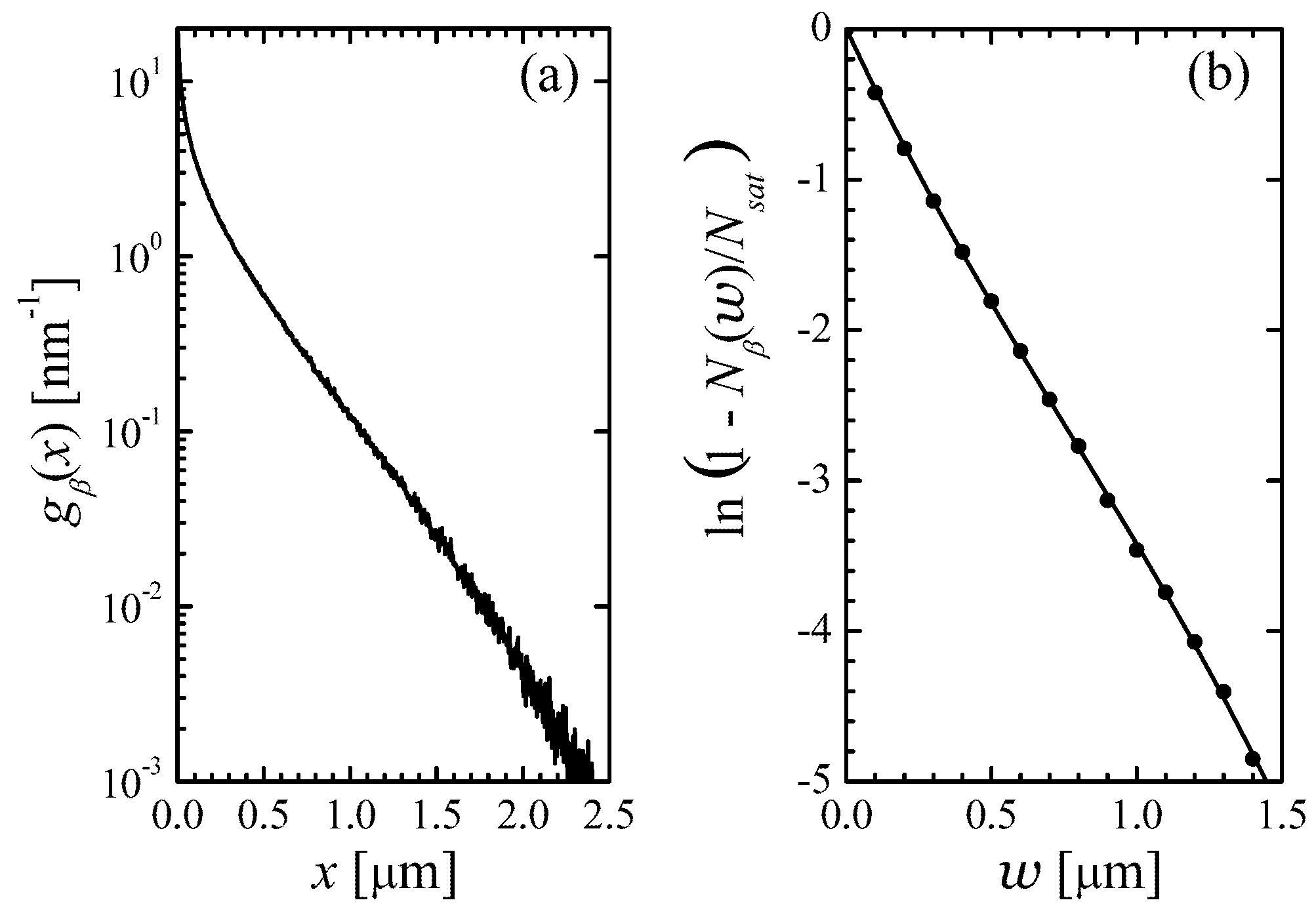
4.1. Generation Function
4.2. Recombination Rate
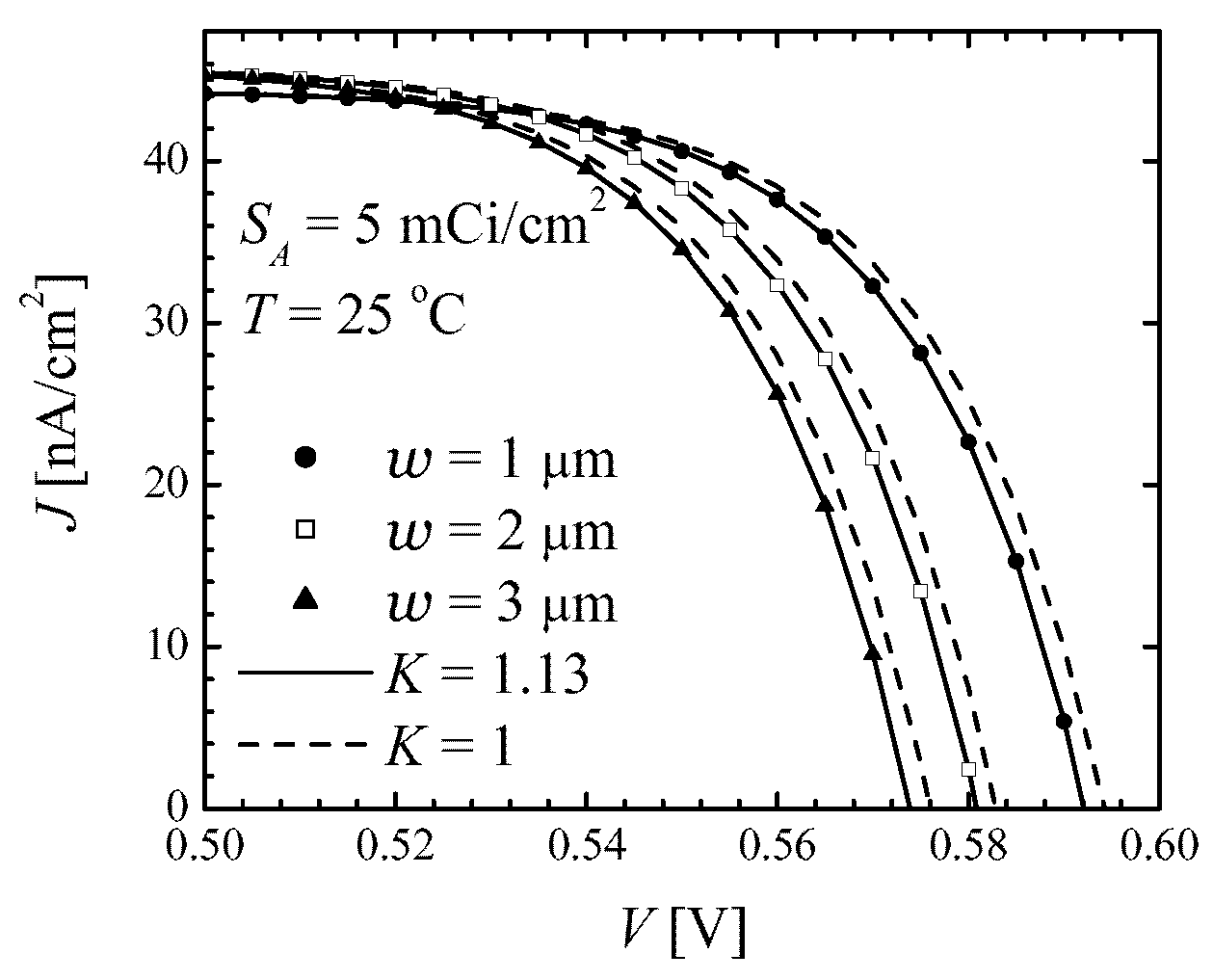
4.3. Analytical Approximation of the Current-Voltage Curve
4.3.1. Recombination Current
4.3.2. Betaconversion Parameters
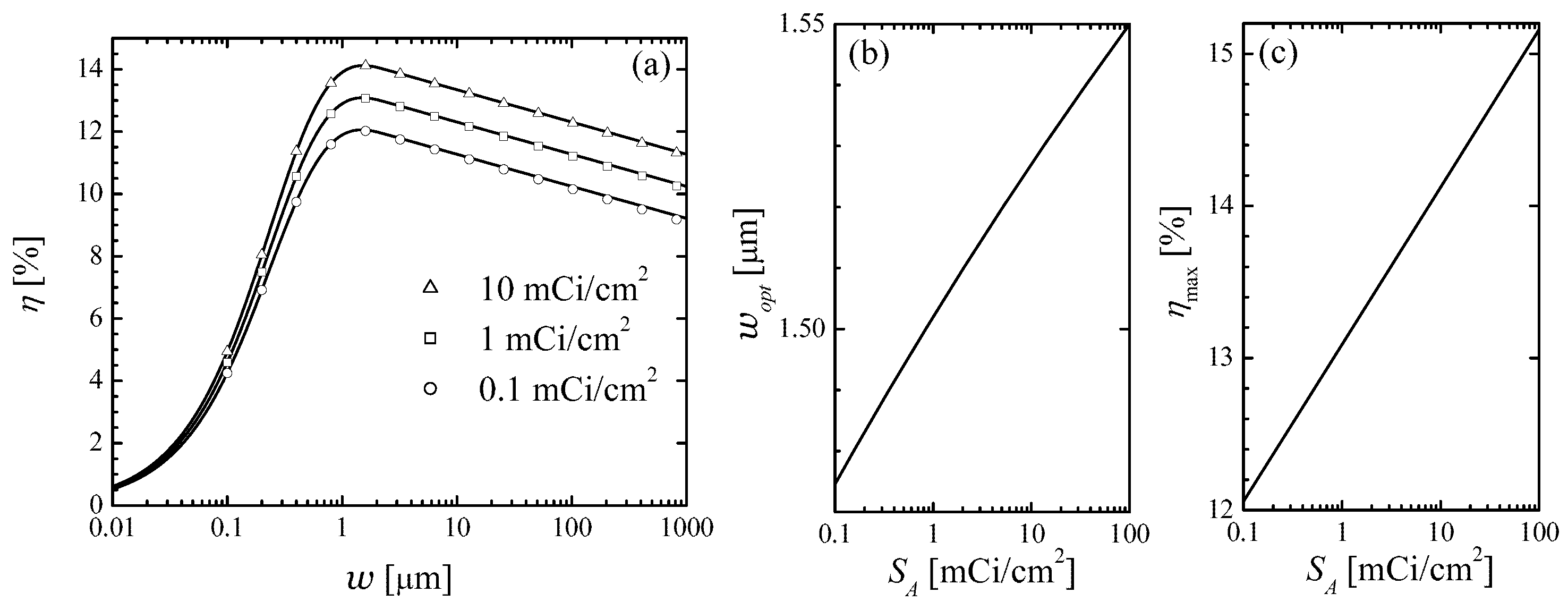
4.4. Optimal Thickness and Maximal Efficiency
5. Conclusions
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
Abbreviations
| EHP | electron-hole pair |
References
- Pfann, W.G.; van Roosbroeck, W. Radioactive and Photoelectric pn Junction Power Sources. J. Appl. Phys. 1954, 25, 1422–1434. [Google Scholar] [CrossRef]
- Rappaport, P. The Electron-Voltaic Effect in p-n Junctions Induced by Beta-Particle Bombardment. Phys. Rev. 1954, 93, 246–247. [Google Scholar] [CrossRef]
- Naseem, M.B.; Kim, H.S.; Lee, J.; Kim, C.H.; In, S.-I. Betavoltaic Nuclear Battery: A Review of Recent Progress and Challenges as an Alternative Energy Source. J. Phys. Chem. C 2023, 127, 7565–7579. [Google Scholar] [CrossRef]
- Landis, G.A.; Bailey, S.G.; Clark, E.B.; Myers, M.G.; Piszczor, M.F.; Murbach, M.S. Non-solar photovoltaics for small space missions. In Proceedings of the 38th IEEE Photovoltaic Specialists Conference, Austin, TX, USA, 3–8 June 2012; pp. 002819–002824. [Google Scholar] [CrossRef]
- O’Connor, A.; Manuel, M.V.; Shaw, H. An extended-temperature, volumetric source model for betavoltaic power generation. Trans. Am. Nucl. Soc. 2019, 121, 542–545. [Google Scholar] [CrossRef] [PubMed]
- Olsen, L.C.; Cabauy, P.; Elkind, B.J. Betavoltaic power sources. Phys. Today 2012, 65, 35–38. [Google Scholar] [CrossRef]
- Prelas, M.A.; Weaver, C.L.; Watermann, M.L.; Lukosi, E.D.; Schott, R.J.; Wisniewski, D.A. A review of nuclear batteries. Prog. Nucl. Energy 2014, 75, 117–148. [Google Scholar] [CrossRef]
- Lei, Y.; Yang, Y.; Liu, Y.; Li, Y.; Wang, G.; Hu, R.; Xiong, S.; Luo, S. The radiation damage of crystalline silicon PN diode in tritium betavoltaic battery. Appl. Radiat. Isot. 2014, 90, 165–169. [Google Scholar] [CrossRef] [PubMed]
- Klein, C.A. Bandgap dependence and related features of radiation ionization energies in semiconductors. J. Appl. Phys. 1968, 39, 2029–2038. [Google Scholar] [CrossRef]
- Whitaker, M.D.C.; Lioliou, G.; Krysa, A.B.; Barnett, A.M. Al0.6Ga0.4As X-ray avalanche photodiodes for spectroscopy. Semicond. Sci. Technol. 2020, 35, 095026. [Google Scholar] [CrossRef]
- Grushko, V.; Beliuskina, O.; Mamalis, A.; Lysakovskiy, V.; Mitskevich, E.; Kiriev, A.; Petrosyan, E.; Chaplynskyi, R.; Bezshyyko, O.; Lysenko, O. Energy conversion efficiency in betavoltaic cells based on the diamond Schottky diode with a thin drift layer. Appl. Radiat. Isot. 2020, 157, 109017. [Google Scholar] [CrossRef]
- Shimaoka, T.; Umezawa, H.; Ichikawa, K.; Pernot, J.; Koizumi, S. Ultrahigh conversion efficiency of betavoltaic cell using diamond pn junction. Appl. Phys. Lett. 2020, 117, 103902. [Google Scholar] [CrossRef]
- Eiting, C.J.; Krishnamoorthy, V.; Rodgers, S.; George, T.; Robertson, J.D.; Brockman, J. Demonstration of a radiation resistant, high efficiency SiC betavoltaic. Appl. Phys. Lett. 2006, 88, 064101. [Google Scholar] [CrossRef]
- Oh, K.; Prelas, M.A.; Rothenberger, J.B.; Lukosi, E.D.; Jeong, J.; Montenegro, D.E.; Schott, R.J.; Weaver, C.L.; Wisniewski, D.A. Theoretical Maximum Efficiencies of Optimized Slab and Spherical Betavoltaic Systems Utilizing Sulfur-35, Strontium-90, and Yttrium-90. Nucl. Technol. 2011, 179, 234–242. [Google Scholar] [CrossRef]
- Zhang, L.; Cheng, H.-L.; Hu, X.-C.; Xu, X.-B. Model and optimal design of 147Pm SiC-based betavoltaic cell. Superlattices Microstruct. 2018, 123, 60–70. [Google Scholar] [CrossRef]
- Kang, T.; Kim, J.; Park, S.; Son, K.; Park, K.; Lee, J.; Kang, S.; Choi, B.-G. Evaluation of a betavoltaic energy converter supporting scalable modular structure. ETRI J. 2018, 41, 254–261. [Google Scholar] [CrossRef]
- Lin, Z. Simulation and Optimization Design of SiC-Based PN Betavoltaic Microbattery Using Tritium Source. Crystals 2020, 10, 105. [Google Scholar] [CrossRef]
- Butera, S.; Lioliou, G.; Barnett, A.M. Temperature effects on gallium arsenide 63Ni betavoltaic cell. Appl. Rad. Isotopes 2017, 125, 42–47. [Google Scholar] [CrossRef]
- Ding, Z.; Jiang, T.-X.; Zheng, R.-R.; Wang, N.; Zhang, L.-F.; Liu, S.-C.; Li, X.; San, H.-S. Quantitative modeling, optimization, and verification of 63Ni powered betavoltaic cells based on three-dimensional ZnO nanorod arrays. Nucl. Sci. Tech. 2022, 33, 144. [Google Scholar] [CrossRef]
- Chen, Z.; Zheng, R.; Lu, J.; Li, X.; Wang, Y.; Zhang, X.; Zhang, Y.; Cui, Q.; Yuan, X.; Zhao, Y.; et al. Modeling and optimization of GaN-based betavoltaic batteries: Comparison of p–n and p–i–n junctions. AIP Adv. 2022, 12, 085112. [Google Scholar] [CrossRef]
- Sze, S.M.; Ng, K.K. Physics of Semiconductor Devices; Willey-Interscience: Hoboken, NJ, USA, 2007. [Google Scholar]
- Green, M.A. Limits on the open-circuit voltage and efficiency of silicon solar cells imposed by intrinsic Auger processes. IEEE Trans. Electron Devices 1984, ED-31, 671–678. [Google Scholar] [CrossRef]
- Richter, A.; Hermle, M.; Glunz, S. Reassessment of the Limiting Efficiency for Crystalline Silicon Solar Cells. IEEE J. Photovolt. 2013, 3, 1184–1191. [Google Scholar] [CrossRef]
- Andreani, L.C.; Bozzola, A.; Kowalczewski, P.; Liscidini, M.; Redorici, L. Silicon solar cell: Toward the efficiency limit. Adv. Phys. X 2019, 4, 125–148. [Google Scholar] [CrossRef]
- Evstigneev, M.; Farahani, F. Photon recycling in a solar cell with two Lambertian surfaces. IEEE J. Photovolt. 2023, 13, 260–266. [Google Scholar] [CrossRef]
- Li, H.; Liu, Y.; Yang, Y.; Wang, G.; Zhong, Z.; Luo, S. Simulations about self-absorption of tritium in titanium tritide and the energy deposition in a silicon Schottky barrier diode. Appl. Radiat. Isot. 2016, 70, 2559–2563. [Google Scholar] [CrossRef] [PubMed]
- Kosteski, T.; Kherani, N.P.; Gaspari, F.; Zukotynski, S.; Shmayda, W.T. Tritiated amorphous silicon films and devices. J. Vac. Sci. Technol. A 1998, 16, 893–896. [Google Scholar] [CrossRef]
- Liu, B.; Chen, K.P.; Kherani, N.P.; Kosteski, T.; Costea, S.; Zukotynski, S.; Antoniazzi, A.B. Tritiation of amorphous and crystalline silicon using T2 gas. Appl. Phys. Lett. 2006, 89, 044104. [Google Scholar] [CrossRef]
- Kanaya, K.; Okayama, S. Penetration and energy-loss theory of electron in solid targets. J. Phys. D Appl. Phys. 1972, 5, 43–58. [Google Scholar] [CrossRef]
- Schenk, A. Finite-temperature full random-phase approximation model of band gap narrowing for silicon device simulation. J. Appl. Phys. 1998, 84, 3684–3695. [Google Scholar] [CrossRef]
- Couderc, R.; Amara, M.; Lemiti, M. Reassessment of the intrinsic carrier density temperature dependence in crystalline silicon. J. Appl. Phys. 2014, 115, 093705. [Google Scholar] [CrossRef]
- Pässler, R. Dispersion-related description of temperature dependencies of band gaps in semiconductors. Phys. Rev. B 2002, 66, 085201. [Google Scholar] [CrossRef]
- Kleesiek, M.; Behrens, J.; Drexlin, G.; Eitel, K.; Erhard, M.; Formaggio, J.A.; Glück, F.; Groh, S.; Hötzel, M.; Mertens, S.; et al. β-Decay spectrum, response function and statistical model for neutrino mass measurements with the KATRIN experiment. Eur. Phys. J. C 2019, 79, 204. [Google Scholar] [CrossRef]
- Joy, D.C.; Luo, S. An empirical stopping power relationship for low-energy electrons. Scanning 1989, 11, 176–180. [Google Scholar] [CrossRef]
- Sachenko, A.V.; Sokolovskyi, I.O.; Evstigneev, M. Betavoltaic generation function in silicon. In Proceedings of the 44th IEEE Photovoltaic Specialist Conference (PVSC), Washington, DC, USA, 25–30 June 2017; pp. 663–666. [Google Scholar] [CrossRef]
- Henoc, J.; Maurice, F. Characteristics of a Monte Carlo Program for Microanalysis Study of Energy Loss. In Use of Monte Carlo Calculations in Electron Probe Microanalysis and Scanning Electron Microscopy; Heinrich, K.J., Newbury, D.E., Yakowitz, H., Eds.; National Bureau of Standards Special Publicaiton: Washington, DC, USA, 1975; pp. 61–95. [Google Scholar] [CrossRef]
- Gauvin, R.; Drouin, D. A formula to compute total elastic Mott cross-sections. Scanning 1993, 15, 140–150. [Google Scholar] [CrossRef]
- Cole, I.R. Modelling CPV. Doctoral Thesis, Loughborough University, Loughborough, UK, 2015. Available online: https://repository.lboro.ac.uk/articles/thesis/Modelling_CPV/9523520 (accessed on 1 September 2023).
- Black, L.E.; Macdonald, D.H. On the quantification of Auger recombination in crystalline silicon. Sol. Energy Mater. Sol. Cells 2022, 234, 111428. [Google Scholar] [CrossRef]
- Niewelt, T.; Steinhauser, B.; Richter, A.; Veith-Wolf, B.; Fell, A.; Hammann, B.; Grant, N.E.; Black, L.; Tan, J.; Youssef, A.; et al. Reassessment of the intrinsic bulk recombination in crystalline silicon. Sol. Energy Mater. Sol. Cells 2022, 235, 111467. [Google Scholar] [CrossRef]
- Rüdiger, M.; Greulich, J.; Richter, A.; Hermle, M. Parameterization of free carrier absorption in highly doped silicon for solar cells. IEEE Trans. Electron Devices 2013, 60, 2156–2163. [Google Scholar] [CrossRef]
- Green, M. Improved silicon optical parameters at 25 °C, 295 K and 300 K including temperature coefficients. Prog. Photovolt. Res. Appl. 2022, 30, 164–179. [Google Scholar] [CrossRef]
- Würfel, P. The chemical potential of radiation. J. Phys. C: Solid State Phys. 1982, 15, 3967–3985. [Google Scholar] [CrossRef]
- Corless, R.M.; Gonnet, G.H.; Hare, D.E.G.; Jeffrey, D.J.; Knuth, D.E. On the Lambert W function. Adv. Comput. Math. 1996, 5, 329–359. [Google Scholar] [CrossRef]

Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Evstigneev, M.; Afkani, M.; Sokolovskyi, I. Limit Efficiency of a Silicon Betavoltaic Battery with Tritium Source. Micromachines 2023, 14, 2015. https://doi.org/10.3390/mi14112015
Evstigneev M, Afkani M, Sokolovskyi I. Limit Efficiency of a Silicon Betavoltaic Battery with Tritium Source. Micromachines. 2023; 14(11):2015. https://doi.org/10.3390/mi14112015
Chicago/Turabian StyleEvstigneev, Mykhaylo, Mohammad Afkani, and Igor Sokolovskyi. 2023. "Limit Efficiency of a Silicon Betavoltaic Battery with Tritium Source" Micromachines 14, no. 11: 2015. https://doi.org/10.3390/mi14112015
APA StyleEvstigneev, M., Afkani, M., & Sokolovskyi, I. (2023). Limit Efficiency of a Silicon Betavoltaic Battery with Tritium Source. Micromachines, 14(11), 2015. https://doi.org/10.3390/mi14112015








