High-Efficiency 3D-Printed Three-Chamber Electromagnetic Peristaltic Micropump
Abstract
:1. Introduction
2. Principle and Design
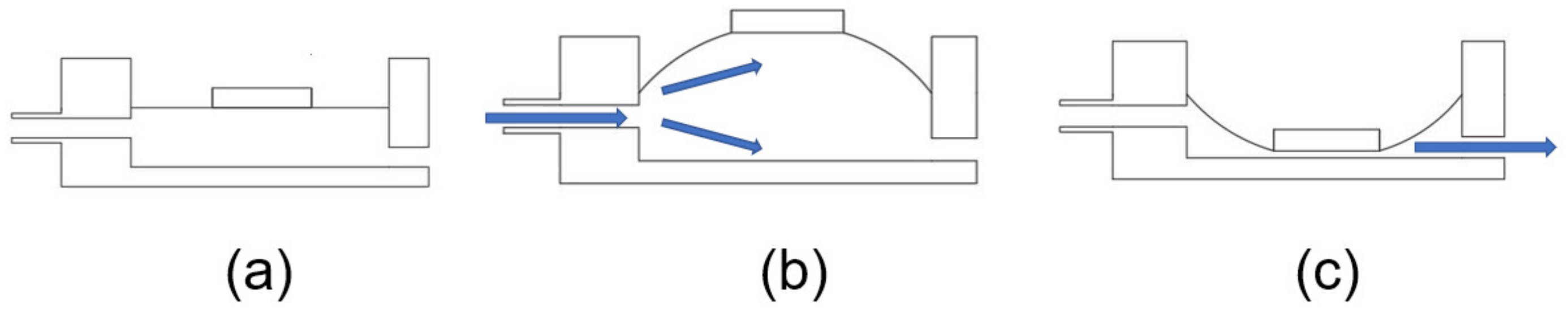
2.1. Physical Model
2.2. Electromagnetic Theory
2.3. PDMS Membrane Deformation Theory
3. Finite Element Simulation
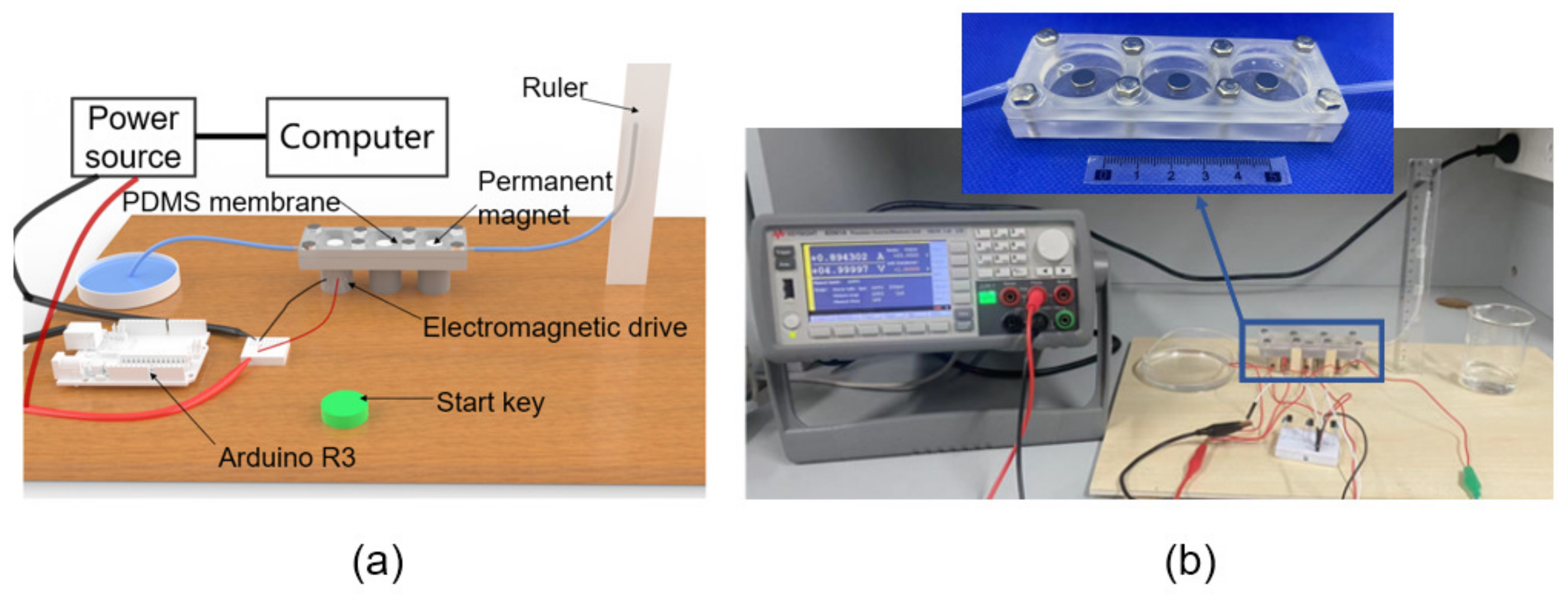
4. Measurement and Evaluation
5. Results and Discussion
6. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Forouzandeh, F.; Arevalo, A.; Alfadhel, A.; Borkholder, D.A. A review of peristaltic micropumps. Sens. Actuators A-Phys. 2021, 326, 112602. [Google Scholar] [CrossRef] [PubMed]
- Swerdlow, H.; Jones, B.J.; Wittwer, C.T. Fully automated DNA reaction and analysis in a fluidic capillary instrument. Anal. Chem. 1997, 69, 848–855. [Google Scholar] [CrossRef] [PubMed]
- Dekker, S.; Buesink, W.; Blom, M.; Alessio, M.; Verplanck, N.; Hihoud, M.; Dehan, C.; César, W.; Le Nel, A.; van den Berg, A.J.S.; et al. Standardized and modular microfluidic platform for fast Lab on Chip system development. Sens. Actuators B: Chem. 2018, 272, 468–478. [Google Scholar] [CrossRef] [Green Version]
- Shoji, S.; Esashi, M. Microflow Devices and Systems. J. Micromech. Microeng. 1994, 4, 157–171. [Google Scholar] [CrossRef] [Green Version]
- Peng, Y.H.; Wang, D.H. A novel multi-channel silicon-based piezoelectric micropump with active piezoelectric valve array. Smart Mater. Struct. 2022, 31, 075010. [Google Scholar] [CrossRef]
- Abhari, F.; Jaafar, H.; Yunus, N.A.M. A Comprehensive Study of Micropumps Technologies. Int. J. Electrochem. Sci. 2012, 7, 9765–9780. [Google Scholar]
- Yu, H.W.; Ye, W.X.; Zhang, W.; Yue, Z.; Liu, G.H. Design, fabrication, and characterization of a valveless magnetic travelling-wave micropump. J. Micromech. Microeng. 2015, 25, 065019. [Google Scholar] [CrossRef]
- Tang, Z.Y.; Shao, X.F.; Huang, J.Z.; Yao, J.Y.; Ding, G.F. Manipulate microfluid with an integrated butterfly valve for micropump application. Sens. Actuators A-Phys. 2020, 306, 111965. [Google Scholar] [CrossRef]
- Laser, D.J.; Santiago, J.G. A review of micropumps. J. Micromech. Microeng. 2004, 14, R35–R64. [Google Scholar] [CrossRef]
- Lin, J.L. Design and Fabrication of Pneumatically Actuated Valveless Pumps. Micromachines 2022, 13, 16. [Google Scholar] [CrossRef] [PubMed]
- Jerman, J.H. The Fabrication and Use of Micromachined Corrugated Silicon Diaphragms. Sens. Actuators A-Phys. 1990, 23, 988–992. [Google Scholar] [CrossRef]
- Huang, Y.-F.; Tsou, C.-H.; Hsu, C.-J.; Lin, Y.-C.; Ono, T.; Tsai, Y.-C. Metallic glass thin film integrated with flexible membrane for electromagnetic micropump application. Jpn. J. Appl. Phys. 2020, 59, SIIK03. [Google Scholar] [CrossRef]
- Tahmasebipour, M.; Paknahad, A.A. Unidirectional and bidirectional valveless electromagnetic micropump with PDMS-Fe<sub>3</sub>O<sub>4</sub> nanocomposite magnetic membrane. J. Micromech. Microeng. 2019, 29, 075014. [Google Scholar]
- Gidde, R.R.; Pawar, P.M.; Ronge, B.P.; Dhamgaye, V.P. Design optimization of an electromagnetic actuation based valveless micropump for drug delivery application. Microsyst. Technol. 2019, 25, 509–519. [Google Scholar] [CrossRef]
- Miao, X.; Lu, H.; Chen, H.; Liu, S. Design and research of the one-way microsphere valve and the PDMS film electromagnetic micropump. Microfluid. Nanofluidics 2022, 26, 075014. [Google Scholar] [CrossRef]






| Electromagnet Parameters | |
|---|---|
| Rated current | 0.3 A |
| Rated voltage | 5 V |
| Rated power | 1.5 W |
| Size | 20 mm × 20 mm |
| Number of Magnetic Blocks | |
|---|---|
| A | Each chamber has two magnetic blocks |
| B | Each chamber has three magnetic blocks |
| C | There are three, four, four and a half magnetic blocks in turn |
| D | There are three, four, and five magnetic blocks in turn |
| E | There are three, four, five and a half magnetic blocks in turn |
| Ref. | Qmax | Pmax | Voltage | Current | Power | Specific Flow | Specific Back Pressure |
|---|---|---|---|---|---|---|---|
| Unit | μL/min | Pa | V | A | W | μL/min·W | Pa/W |
| Y.H. [12] | 0.17 | N/A | 20 | 0.12 | 2.4 | 0.071 | N/A |
| M.T. [13] | 1.25 | N/A | N/A | N/A | 2.2 | 0.568 | N/A |
| R.G. [14] | 51 | 350 | 7.5 | 0.13 | 0.975 | 52.308 | 358.97 |
| X.M. [15] | 8915.8 | 356.72 | 9 | 2.5 | 22.5 | 396.26 | 15.85 |
| This work | 2407.2 | 1127 | 5 | 0.9 | 4.5 | 534.933 | 250.4 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Chen, H.; Miao, X.; Lu, H.; Liu, S.; Yang, Z. High-Efficiency 3D-Printed Three-Chamber Electromagnetic Peristaltic Micropump. Micromachines 2023, 14, 257. https://doi.org/10.3390/mi14020257
Chen H, Miao X, Lu H, Liu S, Yang Z. High-Efficiency 3D-Printed Three-Chamber Electromagnetic Peristaltic Micropump. Micromachines. 2023; 14(2):257. https://doi.org/10.3390/mi14020257
Chicago/Turabian StyleChen, He, Xiaodan Miao, Hongguang Lu, Shihai Liu, and Zhuoqing Yang. 2023. "High-Efficiency 3D-Printed Three-Chamber Electromagnetic Peristaltic Micropump" Micromachines 14, no. 2: 257. https://doi.org/10.3390/mi14020257
APA StyleChen, H., Miao, X., Lu, H., Liu, S., & Yang, Z. (2023). High-Efficiency 3D-Printed Three-Chamber Electromagnetic Peristaltic Micropump. Micromachines, 14(2), 257. https://doi.org/10.3390/mi14020257







