Thermal Fatigue Effect on the Grain Groove Profile in the Case of Diffusion in Thin Polycrystalline Films of Power Electronic Devices
Abstract
:1. Introduction
2. Mathematical Formulation in the Diffusion Case
3. New Study of Mullins’s Case
3.1. Exact Resolution of Mullins’ Problem
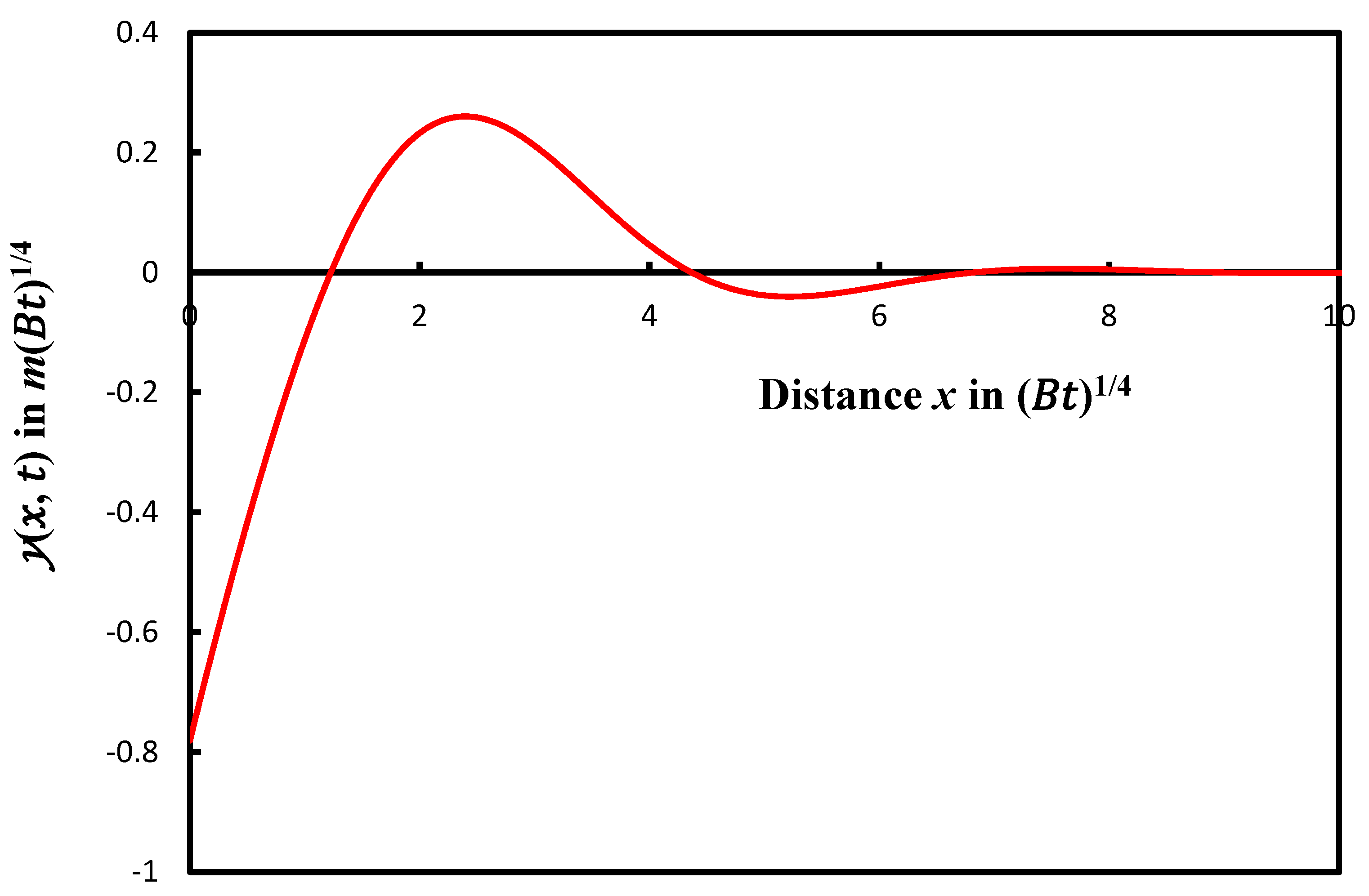
3.2. Profile of the Groove Shape in the Diffusion Case
3.3. Competition between Evaporation and Diffusion
4. Comparison with Experimental Data
4.1. Consequence of Theoretical Results on the Depth of the Groove
4.2. Consequences of the New Solution on the Thermodynamic Parameters
4.3. Validity of the Approximation of
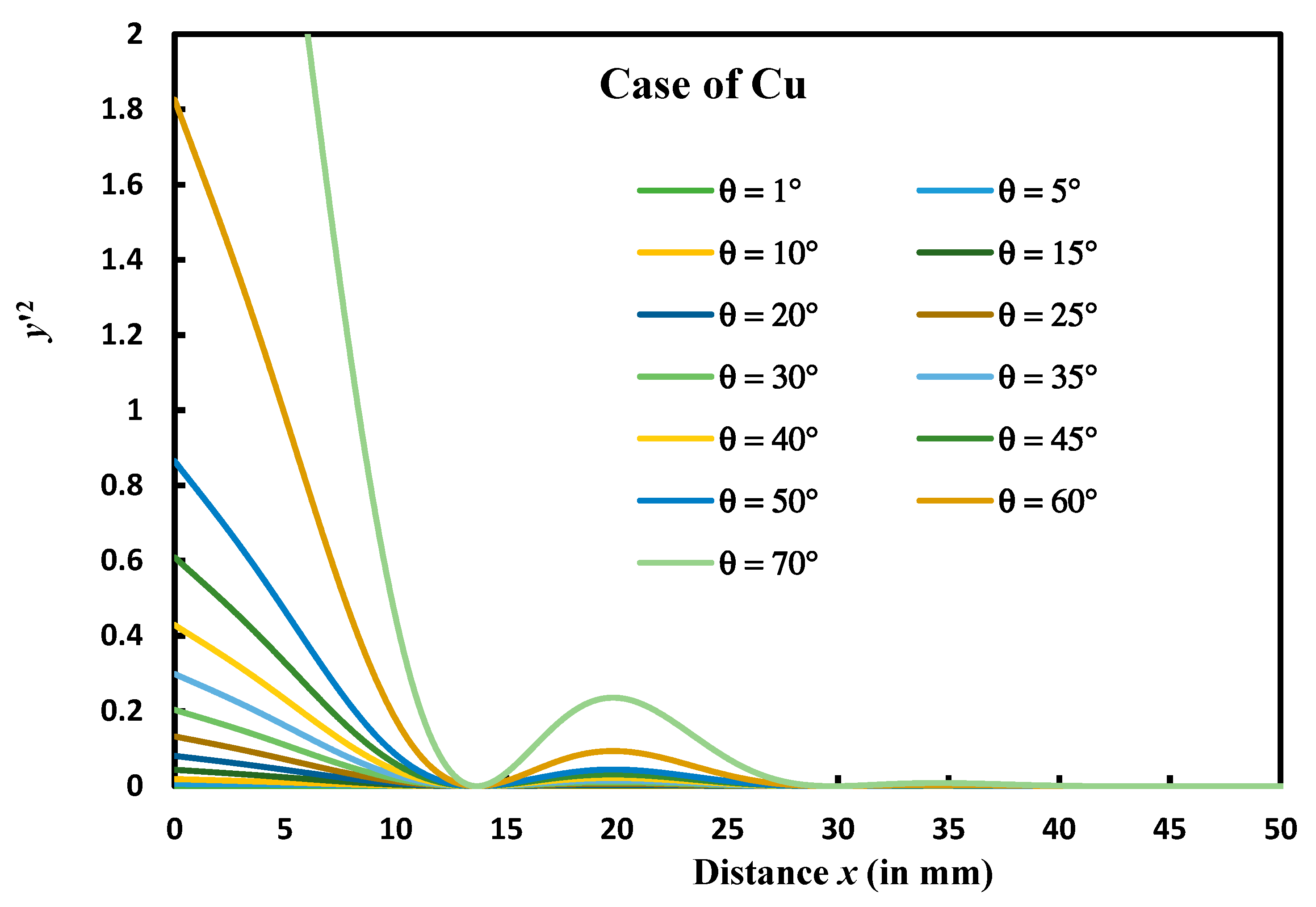
4.4. Variations in the Groove Profile y(x) and the Derivative y′(x) as a Function of the Distance x of Cu
5. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
Appendix A
Appendix B
Appendix B.1. First Case and
Appendix B.2. Second Case and
Appendix C
References
- Zhang, G.P.; Volkert, C.A.; Schwaiger, R.; Mönig, R.; Kraft, O.O. Fatigue and thermal fatigue damage analysis of thin metal films. Microelectron. Reliab. 2007, 47, 2007–2013. [Google Scholar] [CrossRef]
- Zaefferer, S.; Kuo, J.C.; Zhao, Z.; Winning, M.; Raabe, D. On the influence of the grain boundary misorientation on the plastic deformation of aluminum bicrystals. Acta Mater. 2003, 51, 4719–4735. [Google Scholar] [CrossRef]
- Martineau, D.; Levade, C.; Legros, M.; Dupuy, P.; Mazeaud, T. Microelectronics Reliability Universal mechanisms of Al metallization ageing in power MOSFET devices. Microelectron. Reliab. 2014, 54, 2432–2439. [Google Scholar] [CrossRef]
- Broll, M.S.; Geissler, U.; Höfer, J.; Schmitz, S.; Wittler, O.; Lang, K.D. Microstructural evolution of ultrasonic-bonded aluminum wires. Microelectron. Reliab. 2015, 55, 961–968. [Google Scholar] [CrossRef]
- Loh, W.; Corfield, M.; Lu, H.; Hogg, S.; Tilford, T.; Johnson, C.M. Wire Bond Reliability for Power Electronic Modules—Effect of Bonding Temperature. In Thermal, Mechanical and Multi-Physics Simulation Experiments in Microelectronics and Micro-Systems; Wymysłowski, A., Dziedzic, A., Eds.; Euro Sim E: Wroclaw, Poland, 2007; Volume 48, Issue 6; pp. 803–956. [Google Scholar]
- Agyakwa, P.A.; Yang, L.; Arjmand, E.; Evans, P.; Corfield, M.R.; Johnson, C.M. Damage Evolution in Al Wire Bonds Subjected to a Junction Temperature Fluctuation of 30 K. J. Electron. Mater. 2016, 45, 3659–3672. [Google Scholar] [CrossRef]
- Tseng, H.K.; Wu, M.L. Electro-thermal-mechanical modeling of wire bonding failures in IGBT. In Proceedings of the 2013 8th International Microsystems, Packaging, Assembly and Circuits Technology Conference (IMPACT), Taipei, Taiwan, 22–25 October 2013; pp. 152–157. [Google Scholar]
- Medjahed, H.; Vidal, P.E.; Nogarede, B. Thermo-mechanical stress of bonded wires used in high power modules with alternating and direct current modes. Microelectron. Reliab. 2012, 52, 1099–1104. [Google Scholar] [CrossRef]
- Mullins, W.W. Theory of linear facet growth during thermal etching. Philos. Mag. 1961, 6, 1313–1341. [Google Scholar] [CrossRef]
- Mullins, W.W. Theory of thermal grooving. J. Appl. Phys. 1957, 28, 333–339. [Google Scholar] [CrossRef]
- Mullins, W.W. The effect of thermal grooving on grain boundary motion. Acta Metall. 1958, 6, 414–427. [Google Scholar] [CrossRef]
- Mullins, W.W. Grain boundary grooving by volume diffusion. Trans. Metall. Soc. AIME 1960, 218, 354–361. [Google Scholar]
- Zhang, H.; Wong, H. Coupled grooving and migration of inclined grain boundaries: Regime I. Acta Mater. 2002, 50, 1983–1994. [Google Scholar] [CrossRef]
- Bouville, M.; Dongzhi, C.; Srolovitz, D.J. Grain-boundary grooving and agglomeration of alloy thin films with a slow diffusing species. Phys. Rev. Lett. 2007, 98, 085503. [Google Scholar] [CrossRef] [PubMed]
- Bouville, M. Effect of grain shape on the agglomeration of polycrystalline thin films. Appl. Phys. Lett. 2007, 90, 061904. [Google Scholar] [CrossRef]
- Genin, F.Y.; Mullins, W.W.; Wynblatt, P. The effect of stress on grain-boundary grooving. Acta Metall. 1993, 41, 3541–3547. [Google Scholar] [CrossRef]
- Hackney, S.A. Grain-boundary grooving at Þnite grain size. Scr. Metall. 1988, 22, 1731. [Google Scholar] [CrossRef]
- Klinger, L.; Glickman, E.; Fradkov, V.; Mullins, W.W.; Bauer, C. Extension of thermal grooving for arbitrary grain-boundary ßux. J. Appl. Phys. 1995, 78, 3833–3838. [Google Scholar] [CrossRef]
- Klinger, L.; Glickman, E.; Fradkov, V.; Mullins, W.W.; Bauer, C. Effect of surface and grain-boundary diffusion on interconnect reliability. Mater. Res. Soc. Symp. Proc. 1995, 391, 1995. [Google Scholar] [CrossRef]
- Brokman, A.K.R.; Mullins, W.W.; Vilenkin, A.J. Analysis of boundary motion in thin films. Scr. Metall. Mater. 1995, 32, 1341–1346. [Google Scholar] [CrossRef]
- Stone, H.A.; Aziz, M.J.; Margetis, D. Grooving of a grain boundary by evaporation–condensation below the roughening. J. Appl. Phys. 2005, 97, 113535. [Google Scholar] [CrossRef]
- Tritscher, P. An integrable fourth-order nonlinear evolution equation applied to surface redistribution due to capillary. J. Austral. Math. Soc. Ser. B 1997, 38, 518–541. [Google Scholar] [CrossRef]
- Hristov, J. Multiple integral-balance method: Basic idea and an example with Mullin’s model of thermal grooving. Therm. Sci. 2017, 21, 1555–1560. [Google Scholar] [CrossRef]
- Hristov, J. Fourth-order fractional diffusion model of thermal grooving: Integral approach to approximate closed form solution of the Mullins model. Math. Model. Nat. Phenom. 2018, 13, 6. [Google Scholar] [CrossRef]
- Tritscher, P.; Broadbridge, P. Grain boundary grooving by surface diffusion: An analytic nonlinear model for a symmetric groove. Proc. R. Soc. Lond. A 1995, 450, 569–587. [Google Scholar]
- Schwartz, L.W.; Roy, R.V. Theoretical and numerical results for spin coating of viscous liquids. Phys. Fluids 2004, 16, 569–584. [Google Scholar] [CrossRef]
- Myers, T.G. Thin films with high surface tension. SIAM Rev. 1998, 40, 441–462. [Google Scholar] [CrossRef]
- Broadbridge, P.; Tritscher, P. An integrable fourth-order nonlinear evolution equation applied to thermal grooving of metal surfaces. IMA J. Appl. Math. 1994, 53, 249–265. [Google Scholar] [CrossRef]
- Broadbridge, P. Entropy Diagnostics for Fourth Order Partial Differential Equations in Conservation Form. Entropy 2008, 10, 365–379. [Google Scholar] [CrossRef]
- Broadbridge, P. Exact solvability of the Mullins nonlinear diffusion model of groove Development. J. Math. Phys. 1989, 30, 1648–1651. [Google Scholar] [CrossRef]
- Chugunova, M.; Taranets, R. Existence and long-time behaviour of weak solutions for the Mullins equation. Nonlinear Anal. 2012, 75, 6550–6561. [Google Scholar] [CrossRef]
- Gurtin, M.; Jabbour, M. Interface Evolution in Three Dimensions with Curvature-Dependent Energy and Surface Diffusion: Interface-Controlled Evolution, Phase Transitions, Epitaxial Growth of Elastic Films. Arch. Rational Mech. Anal. 2002, 163, 171–208. [Google Scholar] [CrossRef]
- Huang, R. Isothermal stress relaxation in electroplated Cu films. II. Kinetic modeling. J. Appl. Phys. 2005, 97, 103532. [Google Scholar] [CrossRef]
- Asai, T.; Giga, Y. On self-similar solutions to the surface diffusion flow equations with contact angle boundary conditions. Interfaces Free. Boundaries 2014, 16, 539–573. [Google Scholar] [CrossRef]
- Escher, J.; Mayer, U.F.; Simonett, G. The Surface Diffusion Flow for Immersed Hypersurfaces. SIAM J. Math. Anal. 1998, 29, 1419–1433. [Google Scholar] [CrossRef]
- Simonnin, P.; Schreiber, D.K.; Uberuaga, B.P.; Rosso, K.M. Atomic diffusion, segregation, and grain boundary migration in nickel-based alloys from molecular dynamics simulations. Mater. Today Commun. 2023, 35, 105768. [Google Scholar] [CrossRef]
- Beyerlein, I.J.; Caro, A.; Demkowicz, M.J.; Mara, N.A.; Misra, A.; Uberuaga, B.P. Radiation damage tolerant nanomaterials. Mater. Today 2013, 16, 443–449. [Google Scholar] [CrossRef]
- Liu, Y.; Wang, Q.; Chen, L.; Xiao, Z.; Fan, X.; Ma, S.; Ming, L.; Tayal, A.; Zhang, B.; Wu, F.; et al. Diffusion-induced stress optimization by boosted surface Li-concentration for single-crystal Ni-rich layered cathodes. Mater. Today 2022, 61, 40–53. [Google Scholar] [CrossRef]
- Wang, H.; Chao, Q.; Cui, X.; Chen, Z.; Breen, A.; Cabral, M.; Haghdadi, N.; Huang, Q.; Niu, R.; Chen, H.; et al. Introducing C phase in additively manufactured Ti-6Al-4V: A new oxygen-stabilized face-centred cubic solid solution with improved mechanical properties. Mater. Today 2022, 61, 11–21. [Google Scholar] [CrossRef]
- Wei, H.-X.; Tang, L.-B.; Huang, Y.-D.; Wang, Z.-Y.; Luo, Y.-H.; He, Z.-J.; Yan, C.; Mao, J.; Dai, K.-H.; Zheng, J.-C. Comprehensive understanding of Li/Ni intermixing in layered transition metal oxides. Mater. Today 2021, 51, 365–392. [Google Scholar] [CrossRef]
- Li, H.; Tian, C.; Wang, L.; Mo, Y.; Li, J.; Wang, C.; Li, C.; Zheng, L.; Huang, F.; Li, Q. Insight into the Co/Fe intrinsically assembled structure in cobalt-iron-layered double hydroxides on catalytic oxygen evolution reaction. Mater. Today Energy 2023, 35, 101307. [Google Scholar] [CrossRef]
- Chen, N.; Liu, H.; Yang, C.; Liu, H.; Chen, J.; Dai, Y.; Yang, P.; Feng, Y.; Gui, W.; Peng, W.; et al. A multi-particle cellular automaton modeling method for grain dynamics evolution of nickel-rich cathode material. Mater. Today Energy 2023, 35, 101291. [Google Scholar] [CrossRef]
- Mullins, W.W.; Sekerka, R.F. Morphological Stability of a Particle Growing by Diffusion or Heat Flow. J. Appl. Phys. 1963, 34, 323–329. [Google Scholar] [CrossRef]
- Brailsford, A.D.; Gjostein, N.A. Influence of surface energy anisotropy on morphological changes occurring by surface diffusion. J. Appl. Phys. 1975, 46, 2390. [Google Scholar] [CrossRef]
- Bailey, G.L.J.; Watkins, H.C. Surface Tensions in the System Solid Copper-Molten Lead. Proc. Phys. Soc. B 1950, 63, 350. [Google Scholar] [CrossRef]
- Tritscher, P. Thermal Grooving by Surface Diffusion for an Extended Bicrystal Abutting a Half-Space. Proc. Math. Phys. Eng. Sci. 1999, 455, 1957–1977. Available online: http://www.jstor.org/stable/53309 (accessed on 17 April 2023). [CrossRef]
- Martin, P.A. Thermal grooving by surface diffusion: Mullins revisited and extended to multiple grooves. Q. Appl. Math. 2009, 67, 125–136. [Google Scholar] [CrossRef]
- Hamieh, T.; Khatir, Z.; Ibrahim, A. New solution of the partial differential equation of the grain groove profile problem in the case of evaporation/condensation. Sci. Rep. 2019, 9, 10143. [Google Scholar] [CrossRef]




| Number N | |||||||
|---|---|---|---|---|---|---|---|
| 1 | 2.4 | 2.60 × 10−1 | −1.35 | 5.22 | −4.02 × 10−2 | 3.21 | 1.22 |
| 2 | 7.62 | 6.44 × 10−3 | −5.05 | 9.66 | −1.05 × 10−3 | 6.86 | 4.35 |
| 3 | 11.62 | 1.70 × 10−4 | −8.68 | 13.7 | −2.57 × 10−5 | 10.57 | 6.78 |
| 4 | 15.26 | 4.50 × 10−6 | −12.31 | 16.98 | −7.33 × 10−7 | 14.13 | 9 |
| 5 | 18.62 | 1.19 × 10−7 | −15.94 | 20.26 | −1.95 × 10−8 | 17.76 | 11 |
| 6 | 21.82 | 3.17 × 10−9 | −19.57 | 23.34 | −5.17 × 10−10 | 21.38 | 12.89 |
| 7 | 24.82 | 8.42 × 10−11 | −23.20 | 26.3 | −1.37 × 10−11 | 25.01 | 14.69 |
| 8 | 27.74 | 2.24 × 10−12 | −26.83 | 29.14 | −3.64 × 10−13 | 28.64 | 16.44 |
| 9 | 30.54 | 5.94 × 10−14 | −30.45 | 31.9 | −9.67 × 10−15 | 32.27 | 18.08 |
| 10 | 33.26 | 1.58 × 10−15 | −34.08 | 34.58 | −2.57 × 10−16 | 35.90 | 19.72 |
| 11 | 35.94 | 4.19 × 10−17 | −37.71 | 37.22 | −6.84 × 10−18 | 39.52 | 21.27 |
| 12 | 38.5 | 1.11 × 10−18 | −41.34 | 39.78 | −1.82 × 10−19 | 43.15 | 22.83 |
| Number | ||||
|---|---|---|---|---|
| 1 | - | - | - | - |
| 2 | 5.22 | 3.70 | 4.44 | 3.65 |
| 3 | 4.00 | 3.63 | 4.04 | 3.71 |
| 4 | 3.64 | 3.63 | 3.28 | 3.56 |
| 5 | 3.36 | 3.63 | 3.28 | 3.63 |
| 6 | 3.20 | 3.63 | 3.08 | 3.63 |
| 7 | 3.00 | 3.63 | 2.96 | 3.63 |
| 8 | 2.92 | 3.63 | 2.84 | 3.63 |
| 9 | 2.80 | 3.63 | 2.76 | 3.63 |
| 10 | 2.72 | 3.63 | 2.68 | 3.63 |
| 11 | 2.68 | 3.63 | 2.64 | 3.63 |
| 12 | 2.56 | 3.63 | 2.56 | 3.63 |
| Parameters of the Groove | Equation of Interpolation | Linear Regression Coefficient R2 |
|---|---|---|
| in (Bt)1/4 = f(N) | = −0.0929 N2 + 4.3906N − 1.1605 | 0.9991 |
| = f(N) | = 0.0012 N2 − 3.6476N + 2.2688 | 1.0000 |
| in (Bt)1/4 | = −0.0579 N2 + 2.6546N − 0.9316 | 0.9990 |
| in (Bt)1/4 = f(N) | = −0.0767 N2 + 4.0748N + 1.6466 | 0.9996 |
| = f(N) | = −0.0006 N2 + 3.6352N − 0.3982 | 1.0000 |
| + 0.6885 | 0.9998 | |
| − 0.2429 | 1.0000 | |
| − 1.0789 | 1.0000 | |
| − 2.1302 | 0.9999 | |
| = −0.0436 N2 + 2.3829 N + 1.378 | 0.9996 |
| Studied Parameter | Results Obtained by Using Our Solution | Results Obtained by Mullins |
|---|---|---|
| Approached equation of the groove profile | ||
| First zero of y | 1.22 | 1.14 |
| Coordinates of the principal maximum | (2.40; 0.260) | (2.30; 0.193) |
| Coordinates of the first inflexion point | (3.475; 0.131) | 3.43 |
| = −0.0436 N2 + 2.3829N + 1.378 R2 = 0.9996 | Not given | |
| Positive inflexion point relation | + 0.3252 R2 = 0.9999 | Not given |
| Negative inflexion point relation | − 0.6231 R2 = 1 | Not given |
| Separation Distance | Equation of Interpolation | |
|---|---|---|
| Between two consecutive maxima | ||
| Between two consecutive minima |
| Studied Parameter | Results from Our Solution | Results of Mullins |
|---|---|---|
With an error of 6.5% | ||
| Separation distance between the two first maxima | With an error of 11.88% | |
With an error of 5.78% |
| Number | Ordinate of the Positive Inflexion | |
| 1 | 3.475 | 1.310 × 10−1 |
| 2 | 8.295 | 3.436 × 10−3 |
| 3 | 12.275 | 9.068 × 10−5 |
| 4 | 15.855 | 2.410 × 10−6 |
| 5 | 19.185 | 6.503 × 10−8 |
| 6 | 22.325 | 1.744 × 10−9 |
| Equation | = −0.0134 − 0.6214 + 0.3252; R2 = 0.9999 | |
| Number | Abscissa of the Negative Inflexion Point | Ordinate of the Negative Inflexion Point |
| 1 | 6.055 | −2.109 × 10−2 |
| 2 | 10.355 | −5.568 × 10−4 |
| 3 | 14.105 | −1.487 × 10−5 |
| 4 | 17.545 | −4.013 × 10−7 |
| 5 | 20.775 | −1.040 × 10−8 |
| 6 | 23.845 | −2.823 × 10−10 |
| Equation | = 0.012 + 0.6638 − 0.6231; R2 = 1.0000 | |
| Molecular mass m | |
| Temperature T (K) | 725.15 K |
| Surface energy γ | 1 J/m2 |
| kT | 10−20 J |
| 10−7 m2/s | |
| Vapor pressure P0 of Au | |
| P0 of Mg |
| Parameter | Our Results | Mullins Results |
|---|---|---|
| C | ||
| B | ||
| Metal | M (g/mol) | γ (J/m2) | ω (m3) | TMP (K) | T (K) | P0 (Pa) | |
|---|---|---|---|---|---|---|---|
| Cu | 63.546 | 1.808 | 1.18 × 10−29 | 1358.2 | 2200 | 11,490.38 | 1.2 × 10−5 |
| Al | 26.9815 | 1.152 | 2.32 × 10−29 | 933.5 | 2000 | 2956.96 | 1.9 × 10−5 |
| Ti | 47.867 | 2.045 | 1.77 × 10−29 | 1941.2 | 2370 | 286.35 | 2.5 × 10−4 |
| Cs | 132.905 | 0.095 | 1.18 × 10−28 | 302.96 | 530 | 425.19 | 2.0 × 10−4 |
| Li | 6.941 | 0.524 | 2.18 × 10−29 | 453.7 | 970 | 294.34 | 1.5 × 10−4 |
| Co | 58.933 | 2.536 | 1.11× 10−29 | 1768.2 | 2120 | 303.04 | 3.8 × 10−4 |
| Ga | 69.723 | 0.991 | 1.96 × 10−29 | 302.96 | 1570 | 278.52 | 4.1 × 10−4 |
| Tl | 204.383 | 0.639 | 2.86 × 10−29 | 577.2 | 1070 | 318.79 | 5.2 × 10−4 |
| Sr | 87.62 | 0.415 | 5.60 × 10−29 | 1050.2 | 1030 | 1008.65 | 6.9 × 10−5 |
| Metal | for 24 h | ||
|---|---|---|---|
| Co | 5.9 × 10−15 | 1.6 × 10−26 | 6.1 × 10−6 |
| Ti | 9.6 × 10−15 | 2.9 × 10−26 | 7.1 × 10−6 |
| Ga | 1.0 × 10−14 | 2.6 × 10−26 | 6.9 × 10−6 |
| Li | 1.5 × 10−14 | 2.8 × 10−26 | 7.0 × 10−6 |
| Tl | 2.9 × 10−14 | 5.3 × 10−26 | 8.2 × 10−6 |
| Al | 1.2 × 10−13 | 3.4 × 10−26 | 7.3 × 10−6 |
| Cu | 1.7 × 10−13 | 1.2 × 10−26 | 5.7 × 10−6 |
| Sr | 2.4 × 10−13 | 1.4 × 10−25 | 1.0 × 10−5 |
| Cs | 2.8 × 10−13 | 2.7 × 10−25 | 1.2 × 10−5 |
| Metal | 1 s | 1 min | 1 h | 1 Half-Day | 1 Day | 5 Days | 10 Days |
|---|---|---|---|---|---|---|---|
| Co | 7.4 × 10−8 | 2.1 × 10−7 | 5.7 × 10−7 | 1.1 × 10−6 | 1.3 × 10−6 | 1.9 × 10−6 | 2.3 × 10−6 |
| Ti | 8.6 × 10−8 | 2.4 × 10−7 | 6.7 × 10−7 | 1.2 × 10−6 | 1.5 × 10−6 | 2.2 × 10−6 | 2.6 × 10−6 |
| Ga | 8.4 × 10−8 | 2.3 × 10−7 | 6.5 × 10−7 | 1.2 × 10−6 | 1.4 × 10−6 | 2.1 × 10−6 | 2.6 × 10−6 |
| Li | 8.5 × 10−8 | 2.4 × 10−7 | 6.6 × 10−7 | 1.2 × 10−6 | 1.5 × 10−6 | 2.2 × 10−6 | 2.6 × 10−6 |
| Tl | 1.0 × 10−7 | 2.8 × 10−7 | 7.7 × 10−7 | 1.4 × 10−6 | 1.7 × 10−6 | 2.6 × 10−6 | 3.0 × 10−6 |
| Al | 8.9 × 10−8 | 2.5 × 10−7 | 6.9 × 10−7 | 1.3 × 10−6 | 1.5 × 10−6 | 2.3 × 10−6 | 2.7 × 10−6 |
| Cu | 6.9 × 10−8 | 1.9 × 10−7 | 5.4 × 10−7 | 1.0 × 10−6 | 1.2 × 10−6 | 1.8 × 10−6 | 2.1 × 10−6 |
| Sr | 1.3 × 10−7 | 3.5 × 10−7 | 9.8 × 10−7 | 1.8 × 10−6 | 2.2 × 10−6 | 3.2 × 10−6 | 3.9 × 10−6 |
| Cs | 1.5 × 10−7 | 4.2 × 10−7 | 1.2 × 10−6 | 2.2 × 10−6 | 2.6 × 10−6 | 3.8 × 10−6 | 4.6 × 10−6 |
| Metal | 1 s | 1 min | 1 h | 1 Half-Day | 1 Day | 5 Days | 10 Days |
|---|---|---|---|---|---|---|---|
| Co | 1.7 × 10−6 | 4.8 × 10−6 | 1.3 × 10−5 | 2.5 × 10−5 | 2.9 × 10−5 | 4.4 × 10−5 | 5.2 × 10−5 |
| Ti | 2.0 × 10−6 | 5.5 × 10−6 | 1.5 × 10−5 | 2.9 × 10−5 | 3.4 × 10−5 | 5.1 × 10−5 | 6.1 × 10−5 |
| Ga | 1.9 × 10−6 | 5.4 × 10−6 | 1.5 × 10−5 | 2.8 × 10−5 | 3.3 × 10−5 | 5.0 × 10−5 | 5.9 × 10−5 |
| Li | 2.0 × 10−6 | 5.5 × 10−6 | 1.5 × 10−5 | 2.8 × 10−5 | 3.4 × 10−5 | 5.0 × 10−5 | 6.0 × 10−5 |
| Tl | 2.3 × 10−6 | 6.4 × 10−6 | 1.8 × 10−5 | 3.3 × 10−5 | 4.0 × 10−5 | 5.9 × 10−5 | 7.0 × 10−5 |
| Al | 2.1 × 10−6 | 5.7 × 10−6 | 1.6 × 10−5 | 3.0 × 10−5 | 3.5 × 10−5 | 5.3 × 10−5 | 6.3 × 10−5 |
| Cu | 1.6 × 10−6 | 4.5 × 10−6 | 1.2 × 10−5 | 2.3 × 10−5 | 2.7 × 10−5 | 4.1 × 10−5 | 4.9 × 10−5 |
| Sr | 2.9 × 10−6 | 8.1 × 10−6 | 2.3 × 10−5 | 4.2 × 10−5 | 5.0 × 10−5 | 7.5 × 10−5 | 8.9 × 10−5 |
| Cs | 3.5 × 10−6 | 9.6 × 10−6 | 2.7 × 10−5 | 5.0 × 10−5 | 5.9 × 10−5 | 8.9 × 10−5 | 1.1 × 10−4 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Hamieh, T.; Ibrahim, A.; Khatir, Z. Thermal Fatigue Effect on the Grain Groove Profile in the Case of Diffusion in Thin Polycrystalline Films of Power Electronic Devices. Micromachines 2023, 14, 1781. https://doi.org/10.3390/mi14091781
Hamieh T, Ibrahim A, Khatir Z. Thermal Fatigue Effect on the Grain Groove Profile in the Case of Diffusion in Thin Polycrystalline Films of Power Electronic Devices. Micromachines. 2023; 14(9):1781. https://doi.org/10.3390/mi14091781
Chicago/Turabian StyleHamieh, Tayssir, Ali Ibrahim, and Zoubir Khatir. 2023. "Thermal Fatigue Effect on the Grain Groove Profile in the Case of Diffusion in Thin Polycrystalline Films of Power Electronic Devices" Micromachines 14, no. 9: 1781. https://doi.org/10.3390/mi14091781
APA StyleHamieh, T., Ibrahim, A., & Khatir, Z. (2023). Thermal Fatigue Effect on the Grain Groove Profile in the Case of Diffusion in Thin Polycrystalline Films of Power Electronic Devices. Micromachines, 14(9), 1781. https://doi.org/10.3390/mi14091781






