Inverse Tesla Valve as Micromixer for Water Purification
Abstract
:1. Introduction
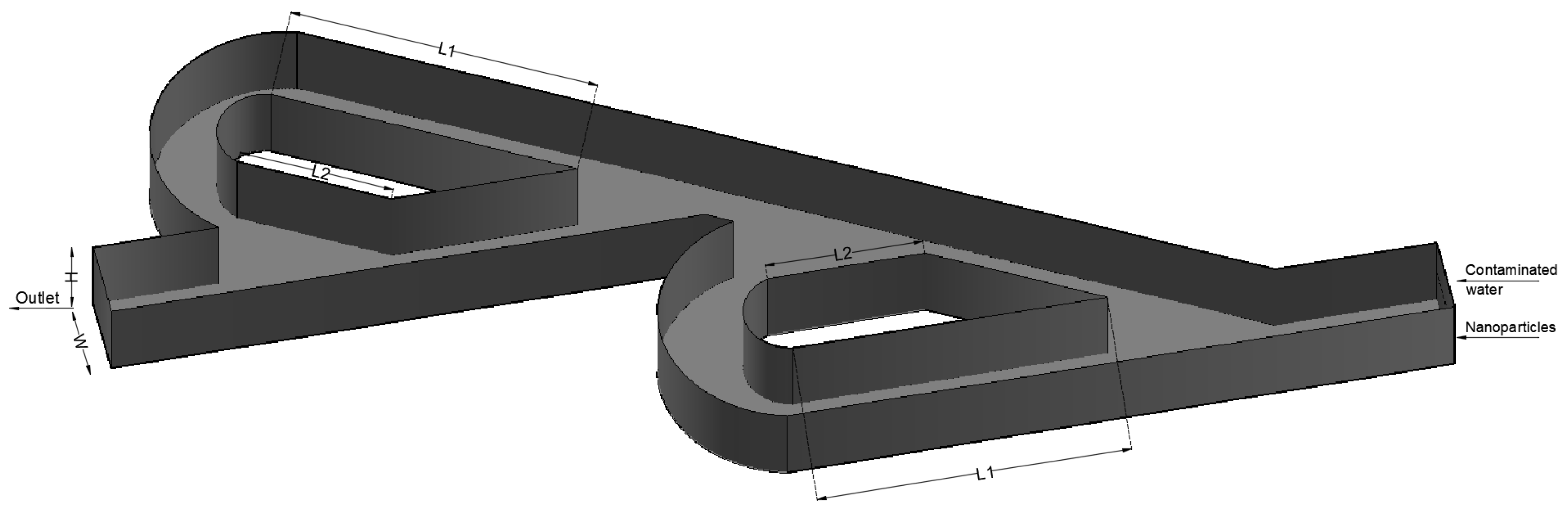
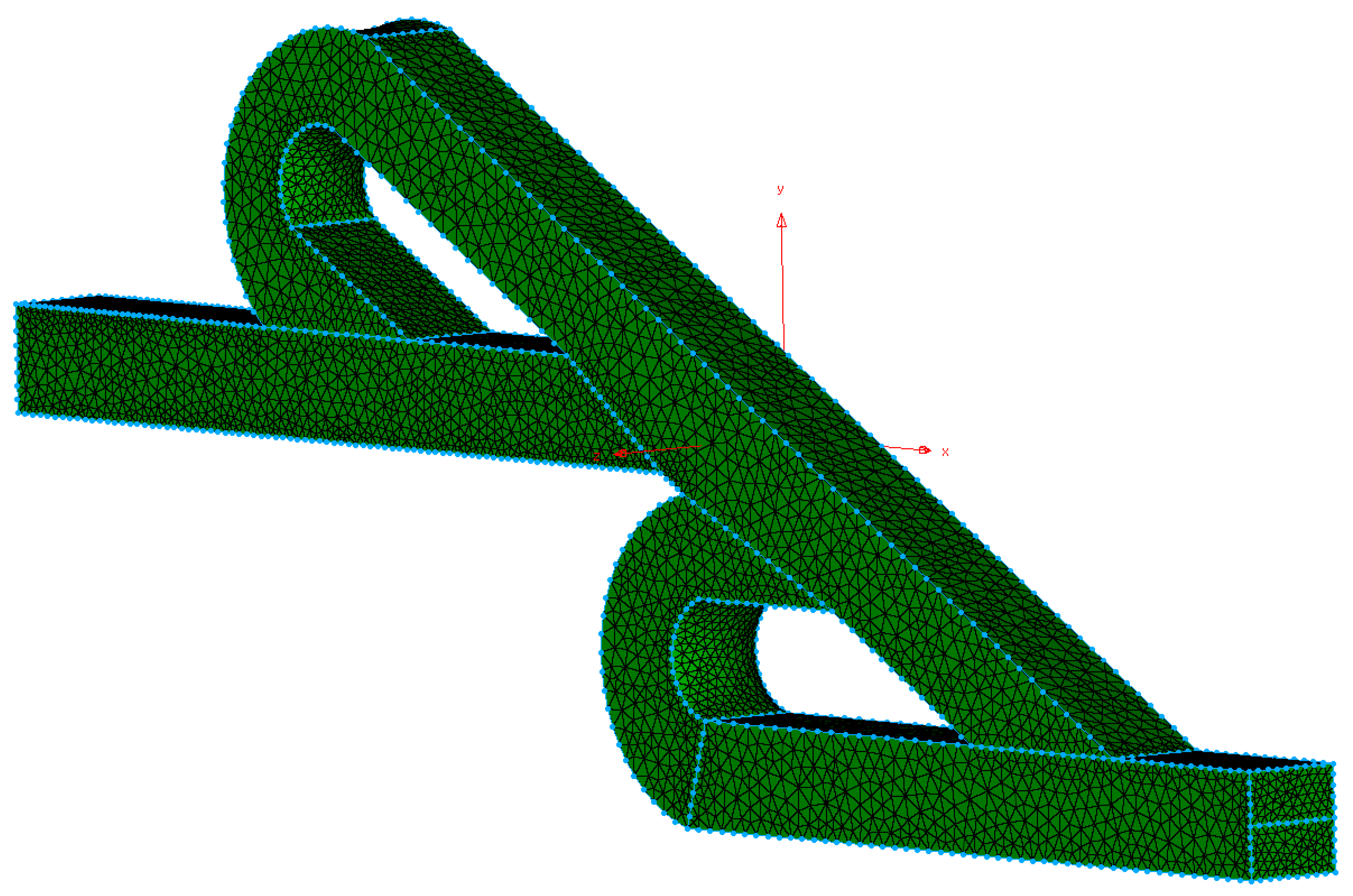
2. Materials and Methods
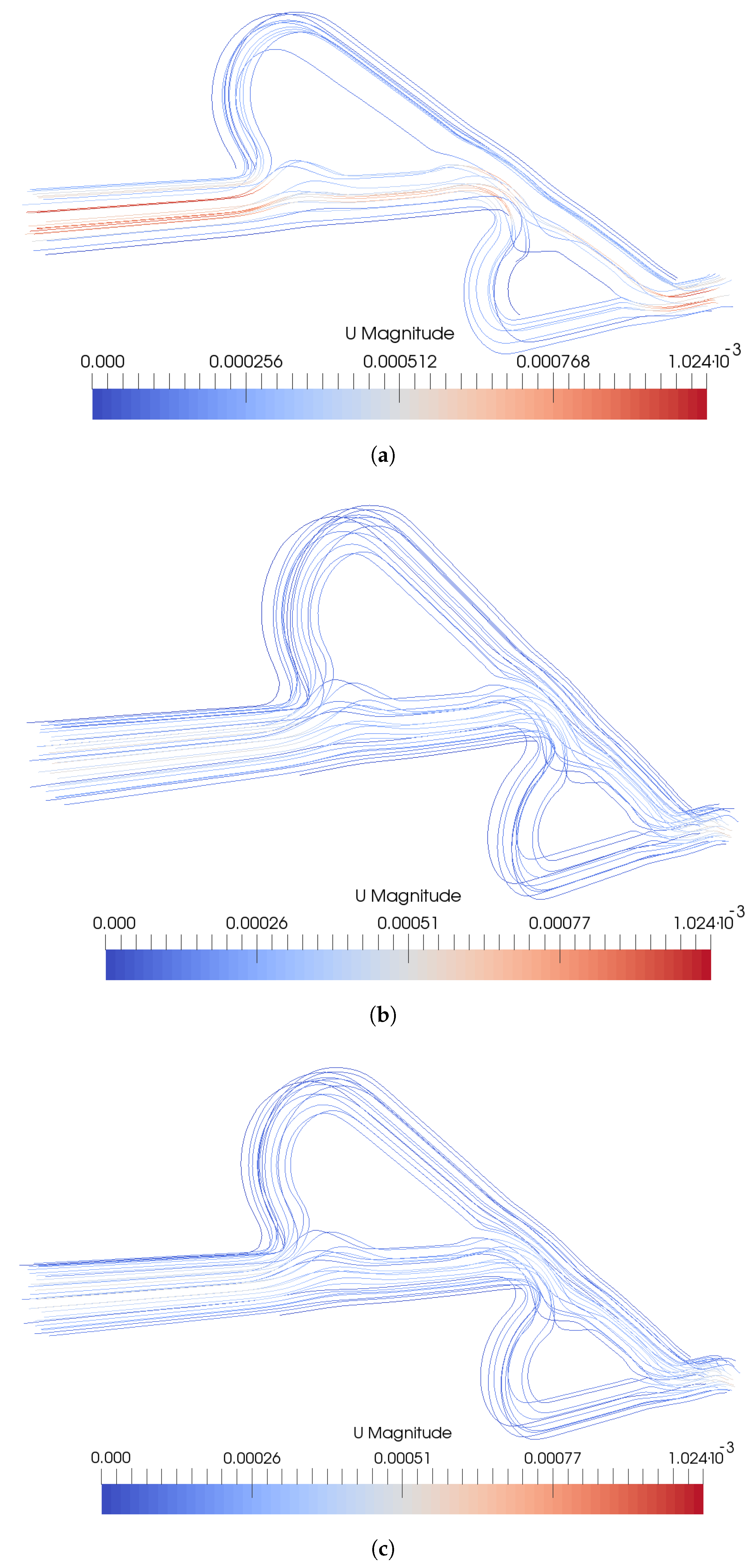
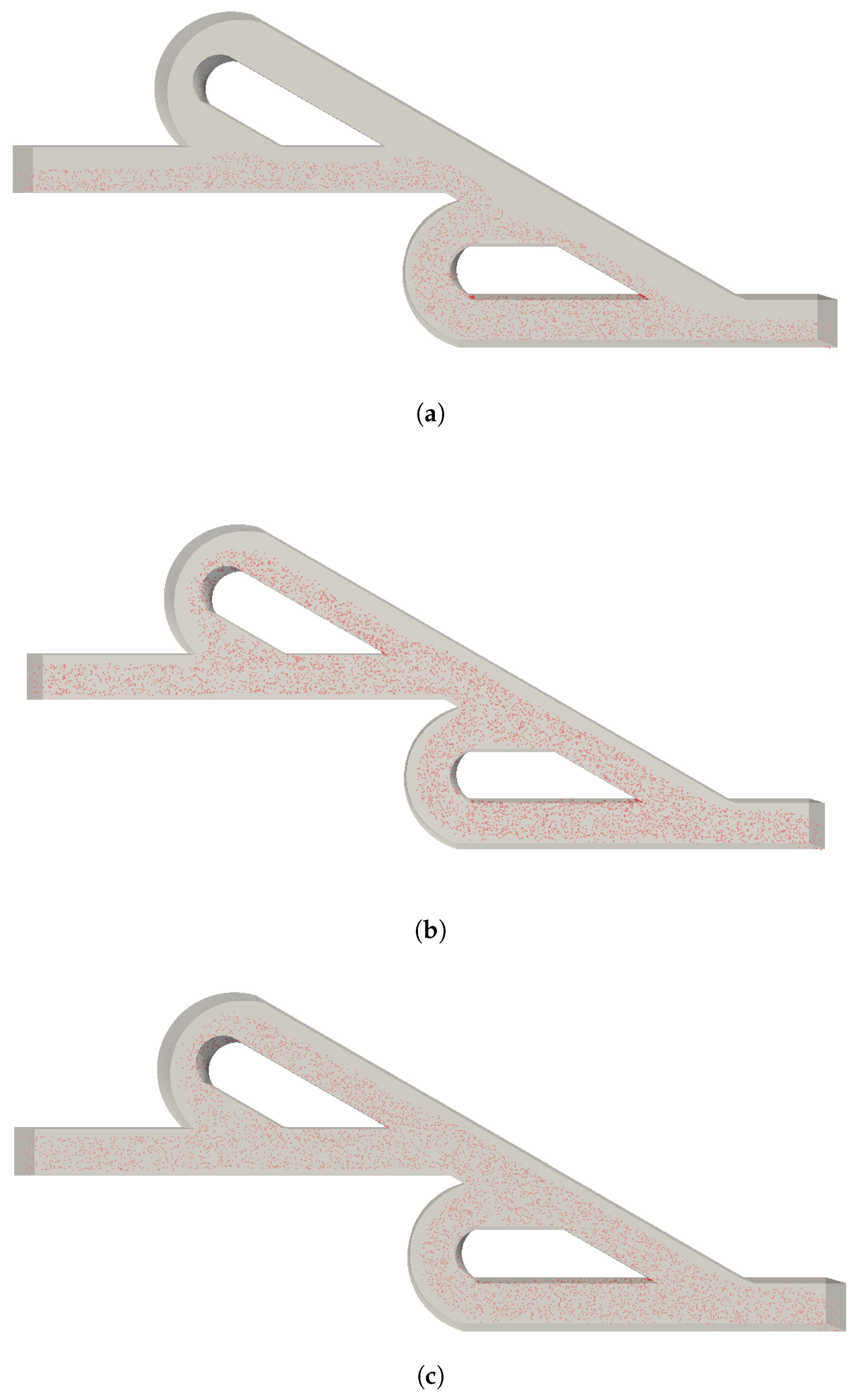
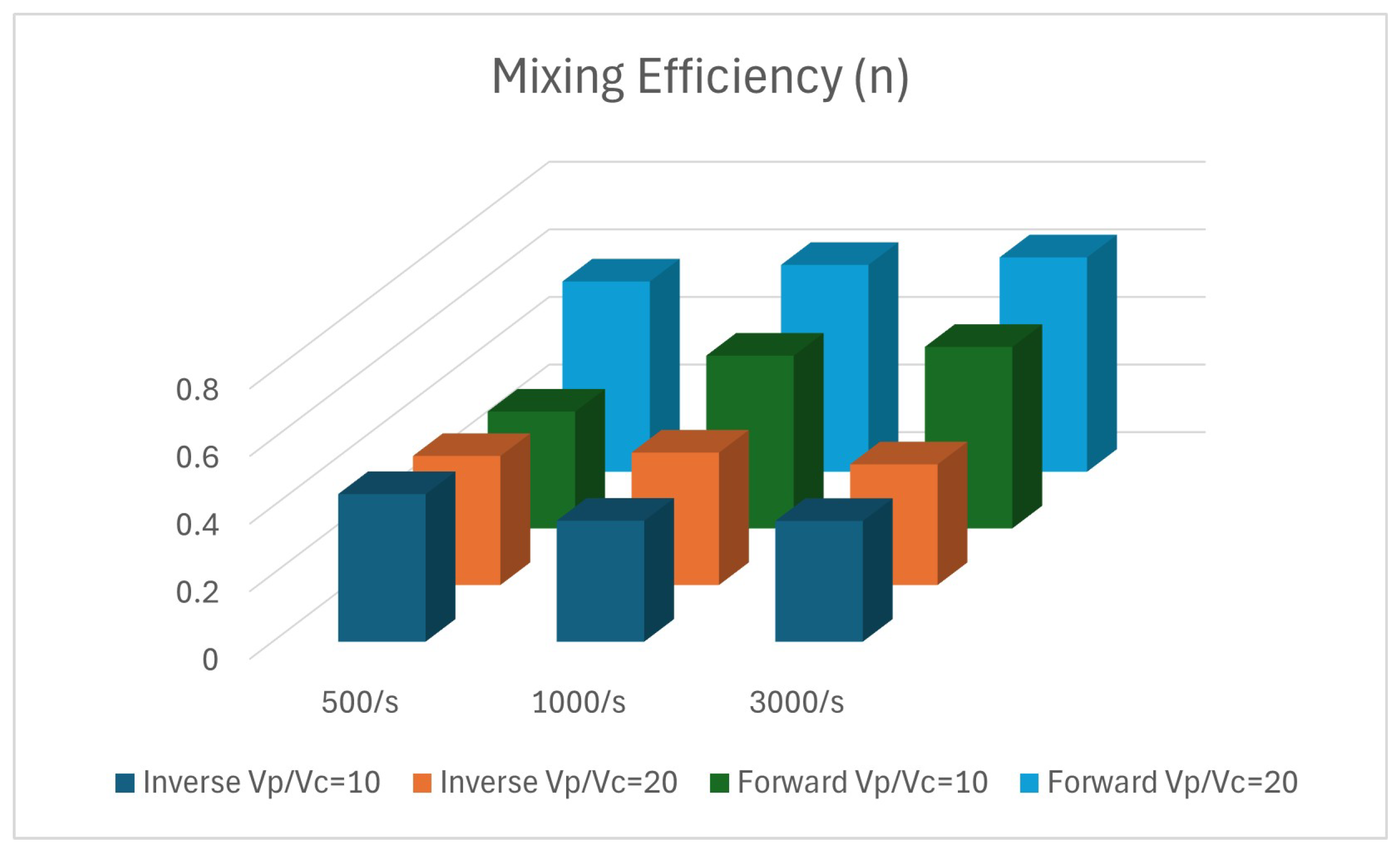
3. Results
4. Discussion
5. Conclusions
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
Abbreviations
| CFD | Computational Fluid Dynamics |
| Re | Reynolds number |
| Velocity of water with magnetic nanoparticles | |
| Velocity of contaminated water | |
| Inlet velocity ratio | |
| Length ratio of micromixer | |
| u | Velocity |
| p | Pressure |
| t | Time |
| Kinematic viscosity | |
| viscosity | |
| density | |
| Transversal velocity | |
| Rotational velocity | |
| Mass | |
| Linear accelerations | |
| Normal contact force | |
| Tangential contact force | |
| Hydrodynamic drag force | |
| Gravity and buoyancy force | |
| Mass moment of the inertia matrix | |
| Angular accelerations | |
| Drag moment | |
| Contact moment | |
| n | Mixing efficiency |
| Concentration variance | |
| Maximum possible variance |
References
- Kim, K.H.; Keller, A.A.; Yang, J.K. Removal of heavy metals from aqueous solution using a novel composite of recycled materials. Colloids Surfaces Physicochem. Eng. Asp. 2013, 425, 6–14. [Google Scholar] [CrossRef]
- Liosis, C.; Papadopoulou, A.; Karvelas, E.; Karakasidis, T.E.; Sarris, I.E. Heavy Metal Adsorption Using Magnetic Nanoparticles for Water Purification: A Critical Review. Materials 2021, 14, 7500. [Google Scholar] [CrossRef] [PubMed]
- Karvelas, E.; Liosis, C.; Karakasidis, T.; Sarris, I. Micromixing Nanoparticles and Contaminated Water Under Different Velocities for Optimum Heavy Metal Ions Adsorption. Environ. Sci. Proc. 2020, 2, 65. [Google Scholar] [CrossRef]
- Essajai, R.; Benhouria, Y.; Rachadi, A.; Qjani, M.; Mzerd, A.; Hassanain, N. Shape-dependent structural and magnetic properties of Fe nanoparticles studied through simulation methods. RSC Adv. 2019, 9, 22057–22063. [Google Scholar] [CrossRef] [PubMed]
- Niu, Y.; Zhang, X.; Kang, Y.; Sun, P.; Liu, H.; Xiao, Z.; Zhao, D. Magnetic microcapsules based on Fe3O4 nanoparticles: Preparation, properties, and applications. Mater. Today Commun. 2024, 39, 108660. [Google Scholar] [CrossRef]
- Liosis, C.; Karvelas, E.; Karakasidis, T.; Sarris, I. Mixing of Fe3O4 nanoparticles under electromagnetic and shear conditions for wastewater treatment applications. AQUA Water Infrastruct. Ecosyst. Soc. 2022, 71, 671–681. [Google Scholar] [CrossRef]
- Razavi Bazaz, S.; Sayyah, A.; Hazeri, A.H.; Salomon, R.; Abouei Mehrizi, A.; Ebrahimi Warkiani, M. Micromixer research trend of active and passive designs. Chem. Eng. Sci. 2024, 293, 120028. [Google Scholar] [CrossRef]
- Zhou, T.; Xu, Y.; Liu, Z.; Joo, S.W. An Enhanced One-Layer Passive Microfluidic Mixer With an Optimized Lateral Structure With the Dean Effect. J. Fluids Eng. 2015, 137, 091102. [Google Scholar] [CrossRef]
- Lee, C.Y.; Wang, W.T.; Liu, C.C.; Fu, L.M. Passive mixers in microfluidic systems: A review. Chem. Eng. J. 2016, 288, 146–160. [Google Scholar] [CrossRef]
- Cao, Q.; Han, X.; Li, L. An active microfluidic mixer utilizing a hybrid gradient magnetic field. Int. J. Appl. Electromagn. Mech. 2015, 47, 583–592. [Google Scholar] [CrossRef]
- Veldurthi, N.; Ghoderao, P.; Sahare, S.; Kumar, V.; Bodas, D.; Kulkarni, A.; Bhave, T. Magnetically active micromixer assisted synthesis of drug nanocomplexes exhibiting strong bactericidal potential. Mater. Sci. Eng. C 2016, 68, 455–464. [Google Scholar] [CrossRef] [PubMed]
- Afzal, A.; Kim, K.Y. Active and Passive Micromixers. In Analysis and Design Optimization of Micromixers; Springer: Singapore, 2021; pp. 11–34. [Google Scholar] [CrossRef]
- Nguyen, N.T.; Wu, Z. Micromixers—A review. J. Micromech. Microeng. 2004, 15, R1. [Google Scholar] [CrossRef]
- Wang, C.T.; Chen, Y.M.; Hong, P.A.; Wang, Y.T. Tesla Valves in Micromixers. Int. J. Chem. React. Eng. 2014, 12, 397–403. [Google Scholar] [CrossRef]
- Vaferi, K.; Vajdi, M.; Shadian, A.; Ahadnejad, H.; Moghanlou, F.; Nami, H.; Jafarzadeh, H. Modeling and Optimization of Hydraulic and Thermal Performance of a Tesla Valve Using a Numerical Method and Artificial Neural Network. Entropy 2023, 25, 967. [Google Scholar] [CrossRef]
- Hong, C.C.; Choi, J.W.; Ahn, C.H. A novel in-plane passive microfluidic mixer with modified Tesla structures. Lab Chip 2004, 4, 109–113. [Google Scholar] [CrossRef]
- Zhang, Y.; Tong, J.B.; Zhu, Z.C. Numerical calculation of forward and reverse flow in Tesla valves with different longitudinal width-to-narrow ratios. Sci. Rep. 2023, 13. [Google Scholar] [CrossRef]
- Bachman, H.; Chen, C.; Rufo, J.; Zhao, S.; Yang, S.; Tian, Z.; Nama, N.; Huang, P.H.; Huang, T.J. An acoustofluidic device for efficient mixing over a wide range of flow rates. Lab Chip 2020, 20, 1238–1248. [Google Scholar] [CrossRef]
- Liosis, C.; Sofiadis, G.; Karvelas, E.; Karakasidis, T.; Sarris, I. A Tesla Valve as a Micromixer for Fe3O4 Nanoparticles. Processes 2022, 10, 1648. [Google Scholar] [CrossRef]
- Weng, X.; Yan, S.; Zhang, Y.; Liu, J.; Shen, J. Design, simulation and experimental study of a micromixer based on Tesla valve structure. Chem. Ind. Eng. Prog. 2021, 40, 4173–4178. [Google Scholar]
- Wang, Y.; He, Y.; Xie, X.; Huang, Z.; Xu, H.; Hu, Q.; Ma, C. Design and Simulation of a New Near Zero-Wear Non-Contact Self-Impact Seal Based on the Tesla Valve Structure. Lubricants 2023, 11, 102. [Google Scholar] [CrossRef]
- Chávez-Guajardo, A.; Medina Llamas, J.; Maqueira, L.; Andrade, C.; Alves, K.; de Melo, C. Efficient removal of Cr (VI) and Cu (II) ions from aqueous media by use of polypyrrole/maghemite and polyaniline/maghemite magnetic nanocomposites. Chem. Eng. J. 2015, 281, 826–836. [Google Scholar] [CrossRef]
- Liu, Y.; Fu, R.; Sun, Y.; Zhou, X.; Baig, S.; Xu, X. Multifunctional nanocomposites Fe3O4@SiO2-EDTA for Pb(II) and Cu(II) removal from aqueous solutions. Appl. Surf. Sci. 2016, 369, 267–276. [Google Scholar] [CrossRef]
- Pang, Y.; Zeng, G.; Tang, L.; Zhang, Y.; Liu, Y.; Lei, X.; Li, Z.; Xie, G. PEI-grafted magnetic porous powder for highly effective adsorption of heavy metal ions. Desalination 2011, 281, 278–284. [Google Scholar] [CrossRef]
- Parham, H.; Zargar, B.; Shiralipour, R. Fast and efficient removal of mercury from water samples using magnetic iron oxide nanoparticles modified with 2-mercaptobenzothiazole. J. Hazard. Mater. 2012, 205–206, 94–100. [Google Scholar] [CrossRef] [PubMed]
- Jin, Y.; Liu, F.; Tong, M.; Hou, Y. Removal of arsenate by cetyltrimethylammonium bromide modified magnetic nanoparticles. J. Hazard. Mater. 2012, 227–228, 461–468. [Google Scholar] [CrossRef]
- Kalantari, K.; Ahmad, M.B.; Masoumi, H.R.F.; Shameli, K.; Basri, M.; Khandanlou, R. Rapid Adsorption of Heavy Metals by Fe3O4/Talc Nanocomposite and Optimization Study Using Response Surface Methodology. Int. J. Mol. Sci. 2014, 15, 12913–12927. [Google Scholar] [CrossRef]
- Zhang, Y.; Ni, S.; Wang, X.; Zhang, W.; Lagerquist, L.; Qin, M.; Willför, S.; Xu, C.; Fatehi, P. Ultrafast adsorption of heavy metal ions onto functionalized lignin-based hybrid magnetic nanoparticles. Chem. Eng. J. 2019, 372, 82–91. [Google Scholar] [CrossRef]
- Qiang, C.; Xu, J.; Zhang, Z.; Tian, L.; Xiao, S.; Liu, Y.; Xu, P. Magnetic properties and microwave absorption properties of carbon fibers coated by Fe3O4 nanoparticles. J. Alloy. Compd. 2010, 506, 93–97. [Google Scholar] [CrossRef]
- Chang, Y.C.; Chen, D.H. Preparation and adsorption properties of monodisperse chitosan-bound Fe3O4 magnetic nanoparticles for removal of Cu(II) ions. J. Colloid Interface Sci. 2005, 283, 446–451. [Google Scholar] [CrossRef]
- Teja, A.S.; Koh, P.Y. Synthesis, properties, and applications of magnetic iron oxide nanoparticles. Prog. Cryst. Growth Charact. Mater. 2009, 55, 22–45. [Google Scholar] [CrossRef]
- Chicot, D.; Mendoza, J.; Zaoui, A.; Louis, G.; Lepingle, V.; Roudet, F.; Lesage, J. Mechanical properties of magnetite (Fe3O4), hematite (α-Fe2O3) and goethite (α-FeO·OH) by instrumented indentation and molecular dynamics analysis. Mater. Chem. Phys. 2011, 129, 862–870. [Google Scholar] [CrossRef]
- Liosis, C.; Sofiadis, G.; Karvelas, E.; Karakasidis, T.; Sarris, I. Simulations of Tesla Valve Micromixer for Water Purification with Fe3O4 Nanoparticles. Environ. Sci. Proc. 2022, 21, 1082. [Google Scholar] [CrossRef]
- Arockiam, S.; Cheng, Y.H.; Armenante, P.M.; Basuray, S. Experimental determination and computational prediction of the mixing efficiency of a simple, continuous, serpentine-channel microdevice. Chem. Eng. Res. Des. 2021, 167, 303–317. [Google Scholar] [CrossRef]
- Endaylalu, S.A.; Tien, W.H. A Numerical Investigation of the Mixing Performance in a Y-Junction Microchannel Induced by Acoustic Streaming. Micromachines 2022, 13, 338. [Google Scholar] [CrossRef] [PubMed]
- Su, Y.; Lautenschleger, A.; Chen, G.; Kenig, E.Y. A Numerical Study on Liquid Mixing in Multichannel Micromixers. Ind. Eng. Chem. Res. 2014, 53, 390–401. [Google Scholar] [CrossRef]
- Hossain, S.; Ansari, M.A.; Husain, A.; Kim, K.Y. Analysis and optimization of a micromixer with a modified Tesla structure. Chem. Eng. J. 2010, 158, 305–314. [Google Scholar] [CrossRef]
- Bhagat, A.A.S.; Papautsky, I. Enhancing particle dispersion in a passive planar micromixer using rectangular obstacles. J. Micromech. Microeng. 2008, 18, 085005. [Google Scholar] [CrossRef]
- Yang, A.S.; Chuang, F.C.; Chen, C.K.; Lee, M.H.; Chen, S.W.; Su, T.L.; Yang, Y.C. A high-performance micromixer using three-dimensional Tesla structures for bio-applications. Chem. Eng. J. 2015, 263, 444–451. [Google Scholar] [CrossRef]

| Heavy Metal | Health Impact |
|---|---|
| Arsenic (As) | Skin damage, circulatory system issues, protein coagulation, nerve inflammation, muscle weakness, carcinogenicity |
| Cadmium (Cd) | Kidney damage, carcinogenicity, DNA damage, gastrointestinal irritation, hyperactivity, renal failure |
| Chromium (Cr) | Allergic dermatitis, diarrhea, nausea, vomiting, headache, neurotoxicity, kidney and liver damage |
| Copper (Cu) | Gastrointestinal issues, liver and kidney damage, anorexia, Wilson’s disease |
| Lead (Pb) | Kidney damage, reduced neural development, carcinogenicity, high blood pressure |
| Mercury (Hg) | Kidney damage, nervous system damage, carcinogenicity, gingivitis, stomatitis, gastrointestinal issues, abortions |
| Nickel (Ni) | Allergic dermatitis, nausea, chronic asthma, coughing, carcinogenicity, hair loss |
| Zinc (Zn) | Depression, lethargy, neurological signs, increased thirst, hyperactivity, physical dysfunction |
| Iron Oxide Compound | Heavy Metal Ion | Adsorption Capacity (mg/g) | Time (min) | References |
|---|---|---|---|---|
| PPY- | Cr (VI) | 209 | 15 | [22] |
| Zn (II) | 138.8 | 10 | [23] | |
| Cd (II) | 37.59 | 10 | [24] | |
| Hg (II) | 4 | [25] | ||
| As (V) | 23.07 | 2 | [26] | |
| Ni (II) | 65.78 | 2 | [27] | |
| Pb (II) | 150.33 | 0.5 | [28] | |
| Cu (II) | 70.7 | 0.5 | [28] |
| inlet and outlet dimensions (m) | Height (H) = Width (W) = | |
| diameter of nanoparticles (nm) | 13.5 | |
| inlet rate of nanoparticles | 500/s, 1000/s, 3000/s | |
| Boundary Conditions | Velocity (m/s) | Pressure (Pa) |
| velocity (m/s) of contaminated water () | , , | zero gradient |
| velocity (m/s) of water with nanoparticles () | zero gradient | |
| Outlet | zero gradient | 0 |
| Walls | 0 | zero gradient |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Liosis, C.; Sofiadis, G.; Karvelas, E.; Karakasidis, T.; Sarris, I. Inverse Tesla Valve as Micromixer for Water Purification. Micromachines 2024, 15, 1371. https://doi.org/10.3390/mi15111371
Liosis C, Sofiadis G, Karvelas E, Karakasidis T, Sarris I. Inverse Tesla Valve as Micromixer for Water Purification. Micromachines. 2024; 15(11):1371. https://doi.org/10.3390/mi15111371
Chicago/Turabian StyleLiosis, Christos, George Sofiadis, Evangelos Karvelas, Theodoros Karakasidis, and Ioannis Sarris. 2024. "Inverse Tesla Valve as Micromixer for Water Purification" Micromachines 15, no. 11: 1371. https://doi.org/10.3390/mi15111371
APA StyleLiosis, C., Sofiadis, G., Karvelas, E., Karakasidis, T., & Sarris, I. (2024). Inverse Tesla Valve as Micromixer for Water Purification. Micromachines, 15(11), 1371. https://doi.org/10.3390/mi15111371









