Experimental and Theoretical Investigations of Direct and Indirect Band Gaps of WSe2
Abstract
1. Introduction
2. Materials and Methods
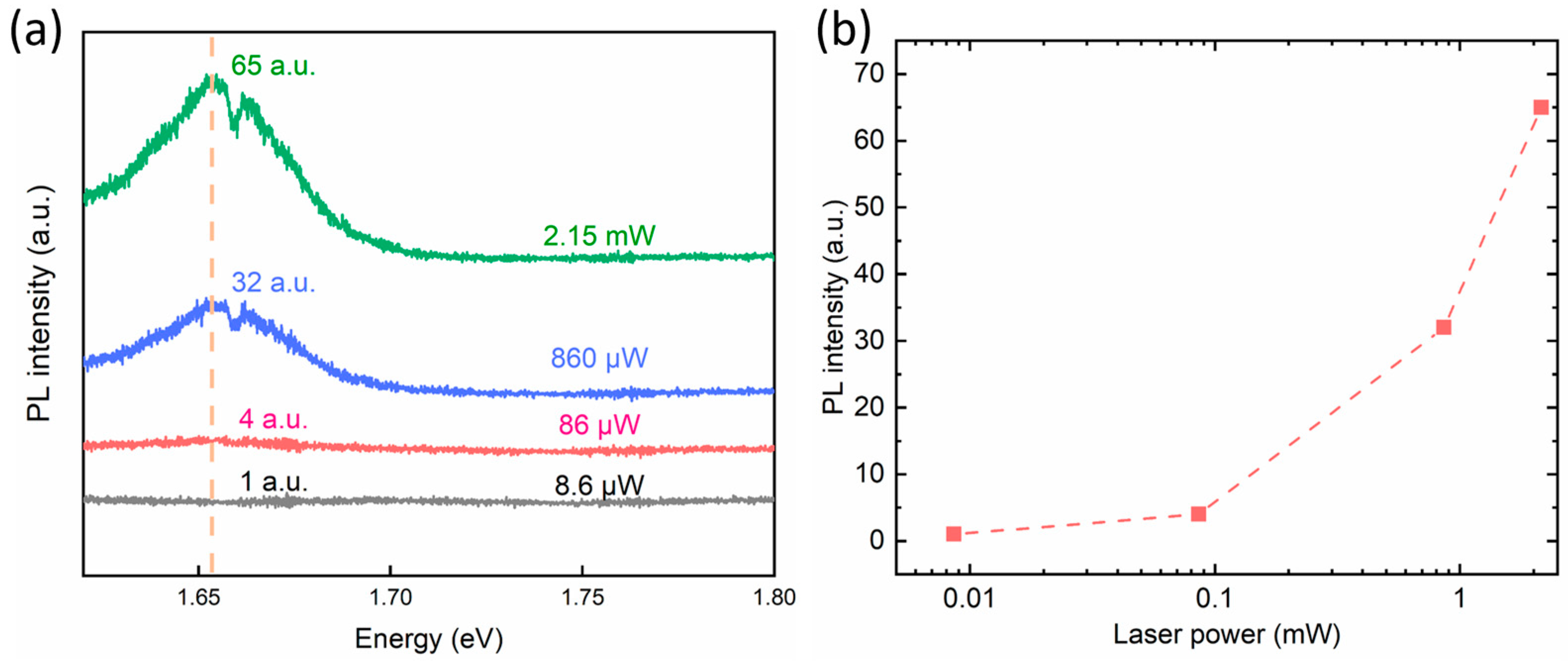
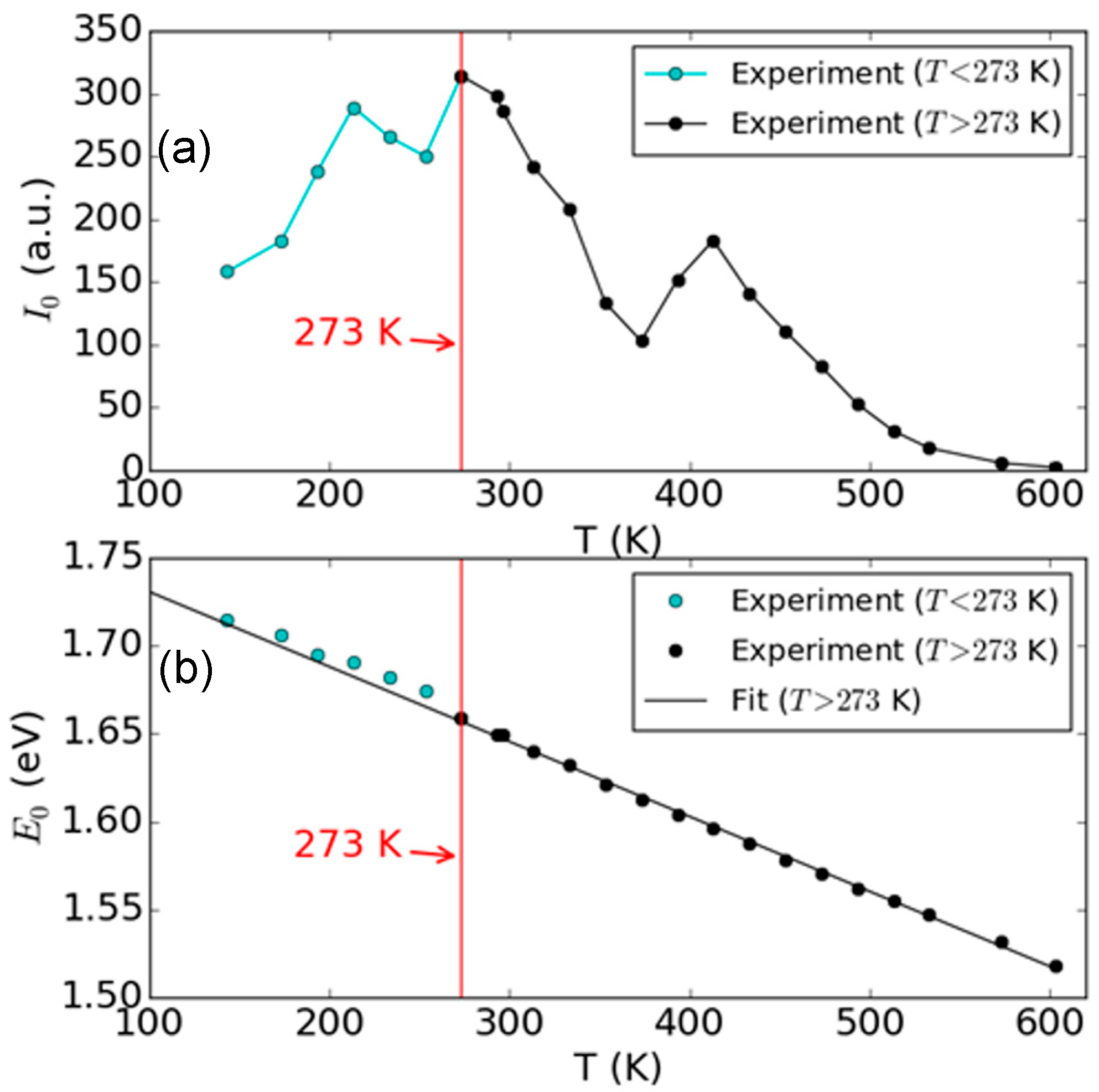
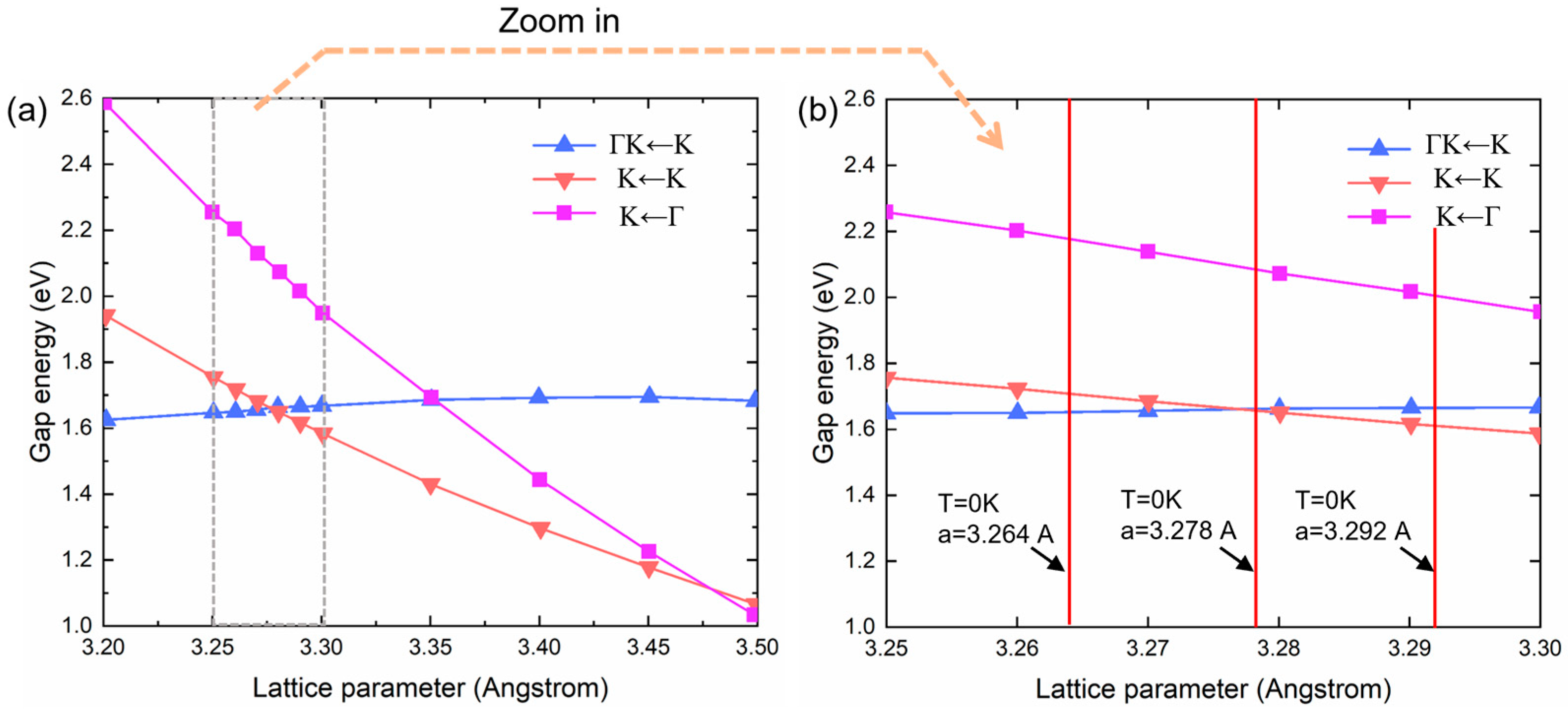
3. Results
4. Discussion
5. Conclusions
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Novoselov, K.S.; Geim, A.K.; Morozov, S.V.; Jiang, D.; Zhang, Y.; Dubonos, S.V.; Grigorieva, I.V.; Firsov, A.A. Electric Field Effect in Atomically Thin Carbon Films. Science 2004, 306, 666–669. [Google Scholar] [CrossRef] [PubMed]
- Radisavljevic, B.; Radenovic, A.; Brivio, J.; Giacometti, V.; Kis, A. Single-layer MoS2 transistors. Nat. Nanotechnol. 2011, 6, 147–150. [Google Scholar] [CrossRef]
- Dean, C.R.; Young, A.F.; Meric, I.; Lee, C.; Wang, L.; Sorgenfrei, S.; Watanabe, K.; Taniguchi, T.; Kim, P.; Shepard, K.L.; et al. Boron nitride substrates for high-quality graphene electronics. Nat. Nanotechnol. 2010, 5, 722–726. [Google Scholar] [CrossRef]
- Mak, K.F.; Lee, C.; Hone, J.; Shan, J.; Heinz, T.F. Atomically Thin MoS2: A New Direct-Gap Semiconductor. Phys. Rev. Lett. 2010, 105, 136805. [Google Scholar] [CrossRef] [PubMed]
- Novoselov, K.S.; Geim, A.K.; Morozov, S.V.; Jiang, D.; Katsnelson, M.I.; Grigorieva, I.V.; Dubonos, S.V.; Firsov, A.A. Two-dimensional gas of massless Dirac fermions in graphene. Nature 2005, 438, 197–200. [Google Scholar] [CrossRef] [PubMed]
- Geim, A.K.; Grigorieva, I.V. Van der Waals heterostructures. Nature 2013, 499, 419–425. [Google Scholar] [CrossRef]
- Wang, Q.H.; Kalantar-Zadeh, K.; Kis, A.; Coleman, J.N.; Strano, M.S. Electronics and optoelectronics of two-dimensional transition metal dichalcogenides. Nat. Nanotechnol. 2012, 7, 699–712. [Google Scholar] [CrossRef]
- Mahjoubi, S.; Ye, F.; Bao, Y.; Meng, W.; Zhang, X. Identification and classification of exfoliated graphene flakes from microscopy images using a hierarchical deep convolutional neural network. Eng. Appl. Artif. Intell. 2023, 119, 105743. [Google Scholar] [CrossRef]
- Cui, X.; Lee, G.-H.; Kim, Y.D.; Arefe, G.; Huang, P.Y.; Lee, C.-H.; Chenet, D.A.; Zhang, X.; Wang, L.; Ye, F.; et al. Multi-terminal transport measurements of MoS2 using a van der Waals heterostructure device platform. Nat. Nanotechnol. 2015, 10, 534–540. [Google Scholar] [CrossRef]
- Britnell, L.; Ribeiro, R.M.; Eckmann, A.; Jalil, R.; Belle, B.D.; Mishchenko, A.; Kim, Y.J.; Gorbachev, R.V.; Georgiou, T.; Morozov, S.V.; et al. Strong Light-Matter Interactions in Heterostructures of Atomically Thin Films. Science 2013, 340, 1311–1314. [Google Scholar] [CrossRef]
- Li, Y.; Chernikov, A.; Zhang, X.; Rigosi, A.; Hill, H.M.; van der Zande, A.M.; Chenet, D.A.; Shih, E.-M.; Hone, J.; Heinz, T.F. Measurement of the optical dielectric function of monolayer transition-metal dichalcogenides: MoS2, MoSe2, WS2, and WSe2. Phys. Rev. B 2014, 90, 205422. [Google Scholar] [CrossRef]
- Yuan, H.; Wang, X.; Lian, B.; Zhang, H.; Fang, X.; Shen, B.; Xu, G.; Xu, Y.; Zhang, S.-C.; Hwang, H.Y.; et al. Generation and electric control of spin–valley-coupled circular photogalvanic current in WSe2. Nat. Nanotechnol. 2014, 9, 851–857. [Google Scholar] [CrossRef] [PubMed]
- Lee, C.; Wei, X.; Kysar Jeffrey, W.; Hone, J. Measurement of the Elastic Properties and Intrinsic Strength of Monolayer Graphene. Science 2008, 321, 385–388. [Google Scholar] [CrossRef] [PubMed]
- Wu, W.; Wang, L.; Li, Y.; Zhang, F.; Lin, L.; Niu, S.; Chenet, D.; Zhang, X.; Hao, Y.; Heinz, T.F.; et al. Piezoelectricity of single-atomic-layer MoS2 for energy conversion and piezotronics. Nature 2014, 514, 470–474. [Google Scholar] [CrossRef] [PubMed]
- Wu, S.; Wang, L.; Lai, Y.; Shan, W.-Y.; Aivazian, G.; Zhang, X.; Taniguchi, T.; Watanabe, K.; Xiao, D.; Dean, C.; et al. Multiple hot-carrier collection in photo-excited graphene Moiré superlattices. Sci. Adv. 2016, 2, e1600002. [Google Scholar] [CrossRef] [PubMed]
- Kalantari, M.H.; Zhang, X. Thermal Transport in 2D Materials. Nanomaterials 2023, 13, 117. [Google Scholar] [CrossRef] [PubMed]
- Xia, F.; Mueller, T.; Lin, Y.-M.; Valdes-Garcia, A.; Avouris, P. Ultrafast graphene photodetector. Nat. Nanotechnol. 2009, 4, 839–843. [Google Scholar] [CrossRef] [PubMed]
- Bunch, J.S.; van der Zande Arend, M.; Verbridge Scott, S.; Frank Ian, W.; Tanenbaum David, M.; Parpia Jeevak, M.; Craighead Harold, G.; McEuen Paul, L. Electromechanical Resonators from Graphene Sheets. Science 2007, 315, 490–493. [Google Scholar] [CrossRef] [PubMed]
- Kim, Y.D.; Kim, H.; Cho, Y.; Ryoo, J.H.; Park, C.-H.; Kim, P.; Kim, Y.S.; Lee, S.; Li, Y.; Park, S.-N.; et al. Bright visible light emission from graphene. Nat. Nanotechnol. 2015, 10, 676–681. [Google Scholar] [CrossRef]
- Wang, Y.; Savalia, M.; Zhang, X. Novel wet transfer technology of manufacturing flexible suspended two-dimensional material devices. J. Vac. Sci. Technol. B 2023, 41, 062810. [Google Scholar] [CrossRef]
- Ye, F.; Liu, Q.; Xu, B.; Feng, P.X.L.; Zhang, X. Ultra-High Interfacial Thermal Conductance via Double hBN Encapsulation for Efficient Thermal Management of 2D Electronics. Small 2023, 19, 2205726. [Google Scholar] [CrossRef] [PubMed]
- Pan, Z.; Zhang, X.; DiSturco, I.; Mao, Y.; Zhang, X.; Wang, H. The Potential of Tellurene-Like Nanosheets as a Solution-Processed Room-Temperature Thermoelectric Material. Small Sci. 2024, 2300272. [Google Scholar] [CrossRef]
- Wang, Y.; Zhang, X. On the role of crystal defects on the lattice thermal conductivity of monolayer WSe2 (P63/mmc) thermoelectric materials by DFT calculation. Superlattices Microstruct. 2021, 160, 107057. [Google Scholar] [CrossRef]
- Easy, E.; Gao, Y.; Wang, Y.; Yan, D.; Goushehgir, S.M.; Yang, E.-H.; Xu, B.; Zhang, X. Experimental and Computational Investigation of Layer-Dependent Thermal Conductivities and Interfacial Thermal Conductance of One- to Three-Layer WSe2. ACS Appl. Mater. Interfaces 2021, 13, 13063–13071. [Google Scholar] [CrossRef] [PubMed]
- Wang, Y.; Zhang, X. Thermal Laser-Assisted Manufacturing of Two-Dimensional Atomic Layer Heterostructures. In Proceedings of the TMS 2021 150th Annual Meeting & Exhibition Supplemental Proceedings, Virtual, 15–18 March 2021; Springer International Publishing: Cham, Switzerland, 2021; pp. 25–34. [Google Scholar]
- Li, Y.; Draher, T.; Comstock, A.H.; Xiong, Y.; Haque, M.A.; Easy, E.; Qian, J.; Polakovic, T.; Pearson, J.E.; Divan, R.; et al. Probing intrinsic magnon bandgap in a layered hybrid perovskite antiferromagnet by a superconducting resonator. Phys. Rev. Res. 2023, 5, 043031. [Google Scholar] [CrossRef]
- Mak, K.F.; He, K.; Shan, J.; Heinz, T.F. Control of valley polarization in monolayer MoS2 by optical helicity. Nat. Nanotechnol. 2012, 7, 494–498. [Google Scholar] [CrossRef] [PubMed]
- Mak, K.F.; He, K.; Lee, C.; Lee, G.H.; Hone, J.; Heinz, T.F.; Shan, J. Tightly bound trions in monolayer MoS2. Nat. Mater. 2013, 12, 207–211. [Google Scholar] [CrossRef] [PubMed]
- Li, Y.; Rao, Y.; Mak, K.F.; You, Y.; Wang, S.; Dean, C.R.; Heinz, T.F. Probing Symmetry Properties of Few-Layer MoS2 and h-BN by Optical Second-Harmonic Generation. Nano Lett. 2013, 13, 3329–3333. [Google Scholar] [CrossRef] [PubMed]
- Xie, Z.; Li, G.; Xia, S.; Liu, C.; Zhang, S.; Zeng, Z.; Liu, X.; Flandre, D.; Fan, Z.; Liao, L.; et al. Ultimate Limit in Optoelectronic Performances of Monolayer WSe2 Sloping-Channel Transistors. Nano Lett. 2023, 23, 6664–6672. [Google Scholar] [CrossRef]
- Tonndorf, P.; Schmidt, R.; Böttger, P.; Zhang, X.; Börner, J.; Liebig, A.; Albrecht, M.; Kloc, C.; Gordan, O.; Zahn, D.R.T.; et al. Photoluminescence emission and Raman response of monolayer MoS2, MoSe2, and WSe2. Opt. Express 2013, 21, 4908–4916. [Google Scholar] [CrossRef]
- Yan, T.; Qiao, X.; Liu, X.; Tan, P.; Zhang, X. Photoluminescence properties and exciton dynamics in monolayer WSe2. Appl. Phys. Lett. 2014, 105, 101901. [Google Scholar] [CrossRef]
- Łopion, A.; Goryca, M.; Smoleński, T.; Oreszczuk, K.; Nogajewski, K.; Molas, M.R.; Potemski, M.; Kossacki, P. Temperature dependence of photoluminescence lifetime of atomically-thin WSe2 layer. Nanotechnology 2020, 31, 135002. [Google Scholar] [CrossRef] [PubMed]
- Zhang, X. Characterization of Layer Number of Two-Dimensional Transition Metal Diselenide Semiconducting Devices Using Si-Peak Analysis. Adv. Mater. Sci. Eng. 2019, 2019, 7865698. [Google Scholar] [CrossRef]
- Sierra-Castillo, A.; Haye, E.; Acosta, S.; Bittencourt, C.; Colomer, J.-F. Synthesis and characterization of highly crystalline vertically aligned WSe2 nanosheets. Appl. Sci. 2020, 10, 874. [Google Scholar] [CrossRef]
- Zhang, R.; Drysdale, D.; Koutsos, V.; Cheung, R. Controlled layer thinning and p-type doping of WSe2 by vapor XeF2. Adv. Funct. Mater. 2017, 27, 1702455. [Google Scholar] [CrossRef]
- Yun, W.S.; Han, S.W.; Hong, S.C.; Kim, I.G.; Lee, J.D. Thickness and strain effects on electronic structures of transition metal dichalcogenides: 2H-MX2 semiconductors (M = Mo, W; X = S, Se, Te). Phys. Rev. B 2012, 85, 033305. [Google Scholar] [CrossRef]
- Murray, R.; Evans, B. The thermal expansion of 2H-MoS2 and 2H-WSe2 between 10 and 320 K. J. Appl. Crystallogr. 1979, 12, 312–315. [Google Scholar] [CrossRef]
- Mounet, N.; Marzari, N. First-principles determination of the structural, vibrational and thermodynamic properties of diamond, graphite, and derivatives. Phys. Rev. B 2005, 71, 205214. [Google Scholar] [CrossRef]
- El-Mahalawy, S.H.; Evans, B.L. The thermal expansion of 2H-MoS2, 2H-MoSe2 and 2H-WSe2 between 20 and 800 °C. J. Appl. Crystallogr. 1976, 9, 403–406. [Google Scholar] [CrossRef]
- Zhang, L.; Lu, Z.; Song, Y.; Zhao, L.; Bhatia, B.; Bagnall, K.R.; Wang, E.N. Thermal Expansion Coefficient of Monolayer Molybdenum Disulfide Using Micro-Raman Spectroscopy. Nano Lett. 2019, 19, 4745–4751. [Google Scholar] [CrossRef]
- Kumar, D.; Kumar, V.; Kumar, R.; Kumar, M.; Kumar, P. Electron-phonon coupling, thermal expansion coefficient, resonance effect, and phonon dynamics in high-quality CVD-grown monolayer and bilayer MoSe2. Phys. Rev. B 2022, 105, 085419. [Google Scholar] [CrossRef]
- Late, D.J.; Shirodkar, S.N.; Waghmare, U.V.; Dravid, V.P.; Rao, C.N.R. Thermal Expansion, Anharmonicity and Temperature-Dependent Raman Spectra of Single- and Few-Layer MoSe2 and WSe2. ChemPhysChem 2014, 15, 1592–1598. [Google Scholar] [CrossRef] [PubMed]
- Zhang, X.-X.; You, Y.; Zhao, S.Y.F.; Heinz, T.F. Experimental Evidence for Dark Excitons in Monolayer WSe2. Phys. Rev. Lett. 2015, 115, 257403. [Google Scholar] [CrossRef] [PubMed]
- Hsu, W.-T.; Lu, L.-S.; Wang, D.; Huang, J.-K.; Li, M.-Y.; Chang, T.-R.; Chou, Y.-C.; Juang, Z.-Y.; Jeng, H.-T.; Li, L.-J.; et al. Evidence of indirect gap in monolayer WSe2. Nat. Commun. 2017, 8, 929. [Google Scholar] [CrossRef] [PubMed]


Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Wang, Y.; Zhang, X. Experimental and Theoretical Investigations of Direct and Indirect Band Gaps of WSe2. Micromachines 2024, 15, 761. https://doi.org/10.3390/mi15060761
Wang Y, Zhang X. Experimental and Theoretical Investigations of Direct and Indirect Band Gaps of WSe2. Micromachines. 2024; 15(6):761. https://doi.org/10.3390/mi15060761
Chicago/Turabian StyleWang, Yingtao, and Xian Zhang. 2024. "Experimental and Theoretical Investigations of Direct and Indirect Band Gaps of WSe2" Micromachines 15, no. 6: 761. https://doi.org/10.3390/mi15060761
APA StyleWang, Y., & Zhang, X. (2024). Experimental and Theoretical Investigations of Direct and Indirect Band Gaps of WSe2. Micromachines, 15(6), 761. https://doi.org/10.3390/mi15060761






