Preamble Design and Noncoherent ToA Estimation for Pulse-Based Wireless Networks-on-Chip Communications in the Terahertz Band
Abstract
1. Introduction
- We design a preamble consisting of a signaling part and a guard interval to achieve synchronization. The design is based on solving a nonlinear optimization problem to optimize the energy allocated to the pulses.
- We consider a THz-WiNoC channel based on the iWISE-256 architecture, a popular WiNoC framework [8]. The package uses flip-chip technology, and the chip design is modeled as a multilayer structure. Specifically, we adopt the EM field analytical channel model in [28] to derive the channel frequency and impulse responses. Additionally, we present the noncoherent energy-detection receiver architecture, which is simple and energy-efficient.
- Based on the energy-detection receiver, we propose a synchronization scheme for ToA estimation. We evaluate the performance of this scheme in terms of synchronization probability and error, providing a comprehensive assessment of its effectiveness.
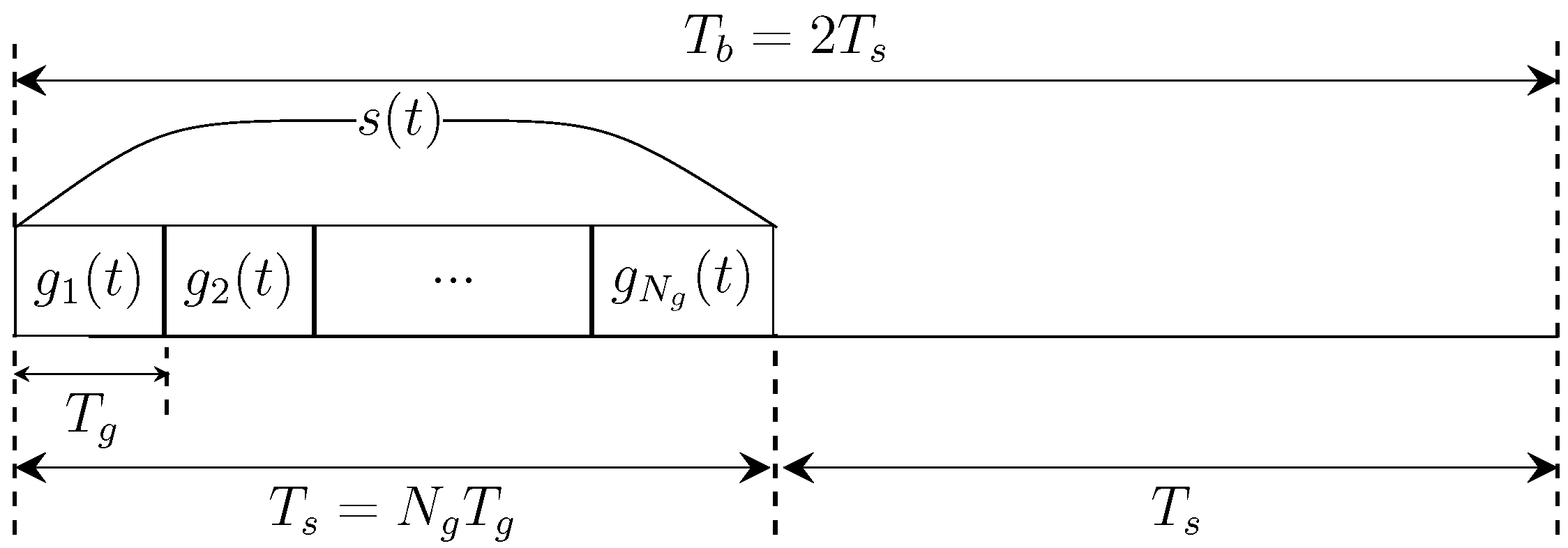
2. Preamble Definition
THz Signal Generation and Detection
3. WiNoC Architecture and Channel Model
THz Channel Influence on the Synchronization Scheme Design
- Attenuation effects: The THz frequency band experiences significantly higher attenuation compared to lower-frequency bands due to molecular absorption and scattering. In the context of intra-chip WiNoC, while atmospheric absorption is negligible, material properties such as silicon substrate and packaging layers contribute to signal loss. To address this, the synchronization scheme employs an energy-detection-based receiver, which accumulates energy over time rather than relying on the precise phase or polarity of the received signal. This design mitigates the impact of attenuation, ensuring accurate synchronization.
- Dispersion effects: In the WiNoC environment, the stratified medium causes dispersion as signals reflect and interact with various chip layers. To manage these effects, the scheme incorporates multiple integration windows, with each integrator accumulating energy over a specific time interval. The peak energy output among these windows is then used to estimate the ToA. This design ensures robust synchronization despite dispersion induced by the THz channel.
- Ultrashort pulse durations: The ultrashort duration of THz pulses, typically on the order of femtoseconds, demands precise timing to avoid missing pulses. Even minor timing offsets can lead to significant synchronization errors. To address this, the preamble design includes a guard interval between consecutive symbols, reducing the likelihood of inter-symbol interference. Additionally, the energy collection mechanism employs high-resolution integration windows to efficiently capture the energy of these ultrashort pulses.
- Time-varying channel effects: Although the intra-chip channel is relatively stable, factors such as thermal variations or fabrication inconsistencies can introduce time-varying effects. To enhance reliability under such conditions, the preamble signal is transmitted multiple times (N repetitions). This repetition averages out temporal variations and increases robustness against minor channel fluctuations.
- Energy efficiency and hardware simplicity: Energy efficiency and minimal hardware simplicity are essential in WiNoC systems to reduce power consumption and heat dissipation. To meet these constraints, the synchronization scheme avoids complex coherent detection mechanisms, such as PLLs. Instead, it uses a simple, noncoherent energy detection approach, which significantly reduces hardware and computational complexity while maintaining synchronization accuracy.
- Channel modeling insights: The synchronization scheme design is informed by an EM-field-based WiNoC channel model that accounts for guided and surface wave effects. This model predicts channel responses with high accuracy and helps optimize parameters such as preamble structure and integration window settings, ensuring compatibility with the specific characteristics of THz-WiNoC systems.
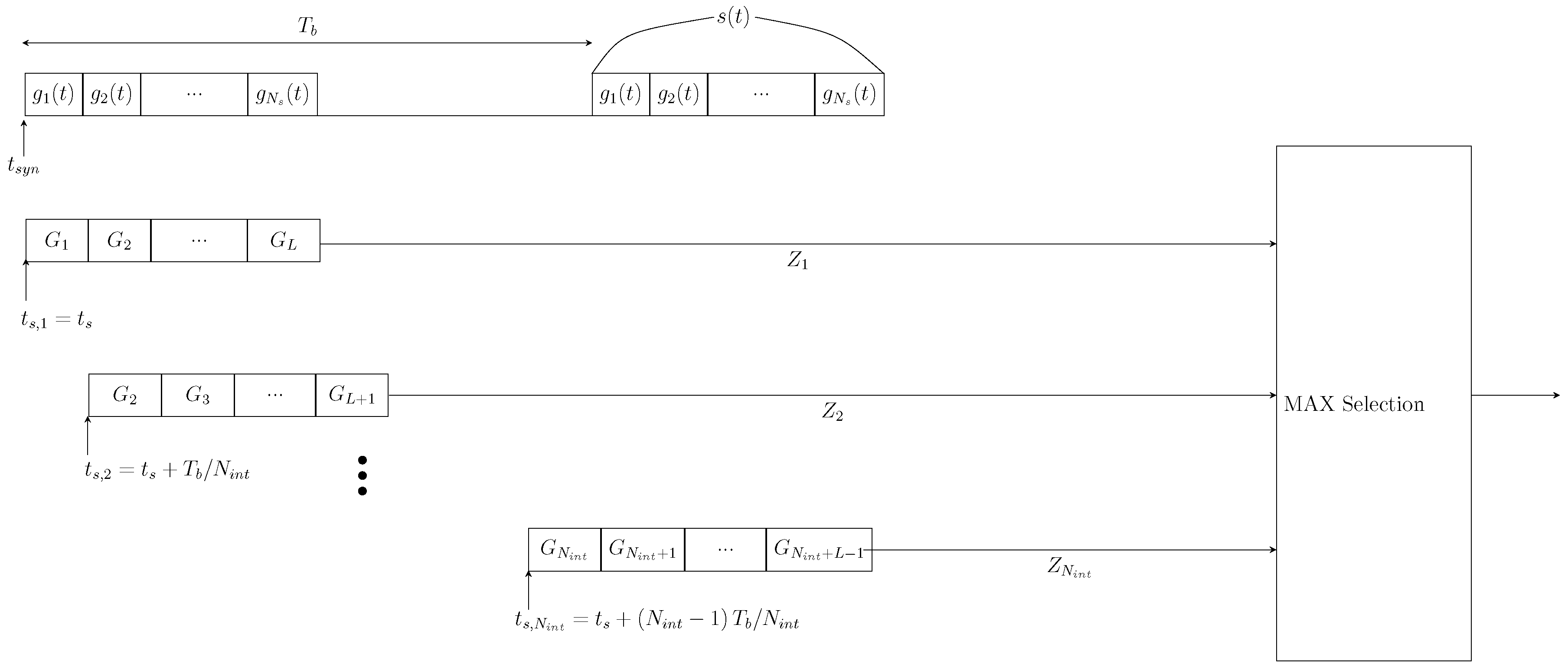
4. Proposed Synchronization Mechanism
4.1. THz-WiNoC Receiver Architecture
4.2. Synchronization Scheme
4.3. Preamble Structure Design
5. Simulations
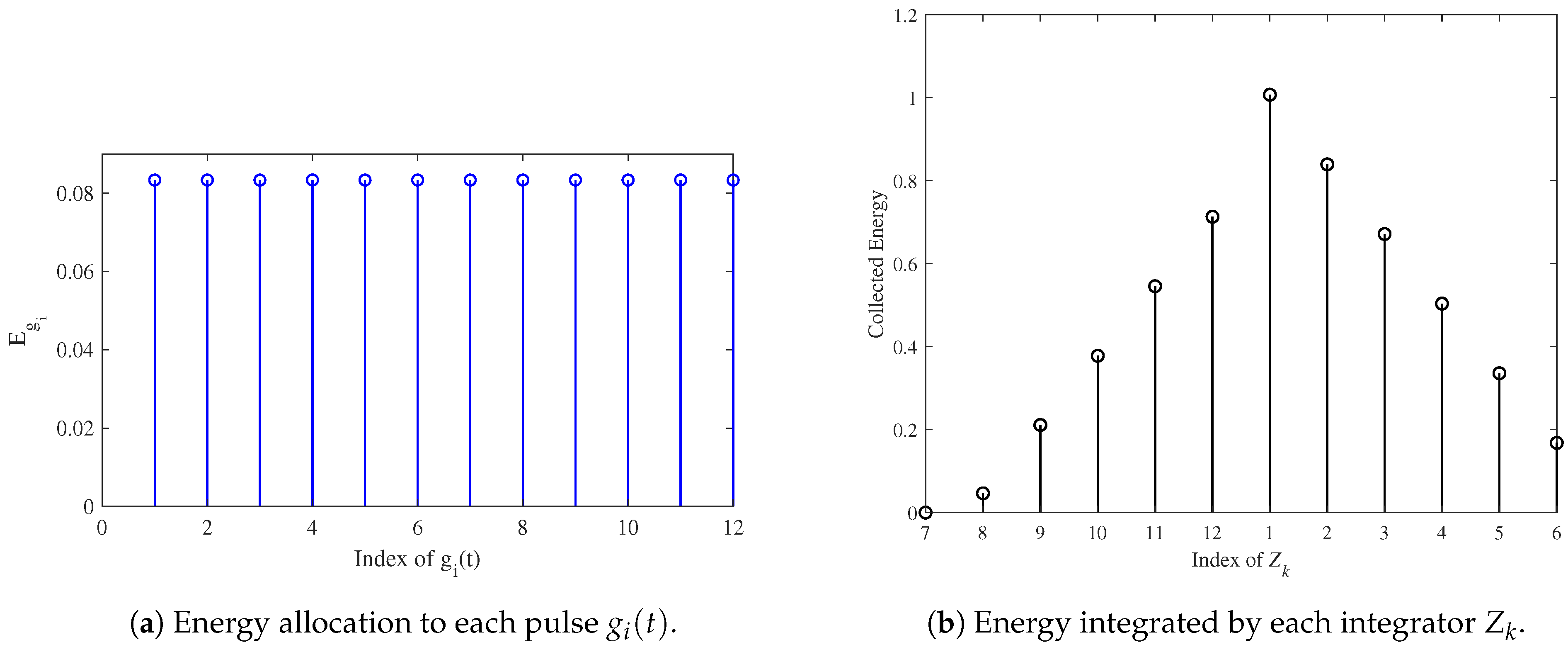
5.1. Optimal Preamble Energy Allocation
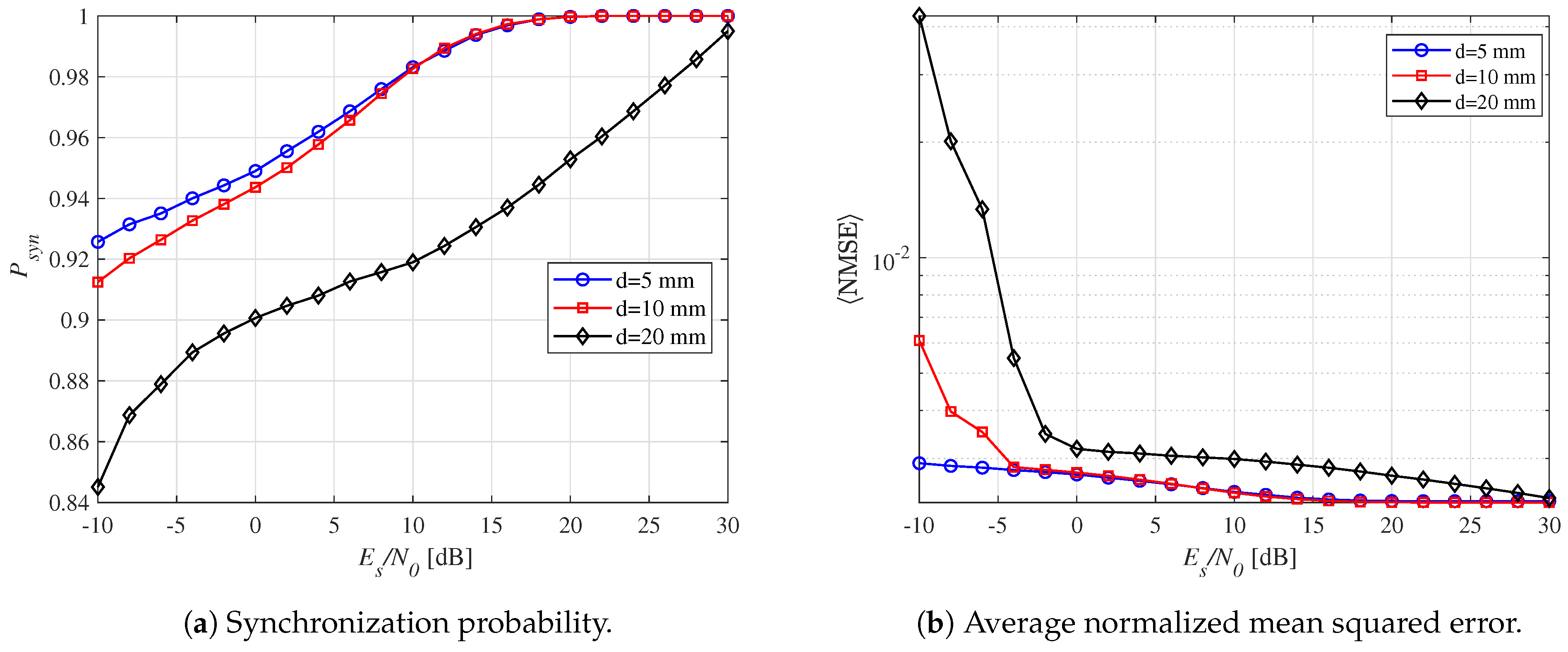
5.2. Synchronization Performance
5.3. Challenges in Hardware Feasibility
6. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Jantsch, A.; Tenhunen, H. Networks on Chip; Springer: New York, NY, USA, 2003; Volume 396. [Google Scholar]
- Bjerregaard, T.; Mahadevan, S. A survey of research and practices of network-on-chip. ACM Comput. Surv. (CSUR) 2006, 38, 1-es. [Google Scholar] [CrossRef]
- Marculescu, R.; Ogras, U.Y.; Peh, L.S.; Jerger, N.E.; Hoskote, Y. Outstanding research problems in NoC design: System, microarchitecture, and circuit perspectives. IEEE Trans. Comput.-Aided Des. Integr. Circuits Syst. 2008, 28, 3–21. [Google Scholar] [CrossRef]
- Nychis, G.P.; Fallin, C.; Moscibroda, T.; Mutlu, O.; Seshan, S. On-chip networks from a networking perspective: Congestion and scalability in many-core interconnects. ACM SIGCOMM Comput. Commun. Rev. 2012, 42, 407–418. [Google Scholar] [CrossRef]
- Bertozzi, D.; Dimitrakopoulos, G.; Flich, J.; Sonntag, S. The fast evolving landscape of on-chip communication: Selected future challenges and research avenues. Des. Autom. Embed. Syst. 2015, 19, 59–76. [Google Scholar] [CrossRef]
- Lemic, F.; Abadal, S.; Tavernier, W.; Stroobant, P.; Colle, D.; Alarcón, E.; Marquez-Barja, J.; Famaey, J. Survey on terahertz nanocommunication and networking: A top-down perspective. IEEE J. Sel. Areas Commun. 2021, 39, 1506–1543. [Google Scholar] [CrossRef]
- Kim, J.; Choi, K.; Loh, G. Exploiting new interconnect technologies in on-chip communication. IEEE J. Emerg. Sel. Top. Circuits Syst. 2012, 2, 124–136. [Google Scholar] [CrossRef]
- Matolak, D.W.; Kodi, A.; Kaya, S.; Ditomaso, D.; Laha, S.; Rayess, W. Wireless networks-on-chips: Architecture, wireless channel, and devices. IEEE Wirel. Commun. 2012, 19, 58–65. [Google Scholar] [CrossRef]
- Matolak, D.W.; Kaya, S.; Kodi, A. Channel modeling for wireless networks-on-chips. IEEE Commun. Mag. 2013, 51, 180–186. [Google Scholar]
- Ganguly, A.; Chang, K.; Pande, P.P.; Belzer, B.; Nojeh, A. Performance evaluation of wireless networks on chip architectures. In Proceedings of the 2009 10th International Symposium on Quality Electronic Design, San Jose, CA, USA, 16–18 March 2009; pp. 350–355. [Google Scholar]
- Abadal, S.; Nemirovsky, M.; Alarcón, E.; Cabellos-Aparicio, A. Networking challenges and prospective impact of broadcast-oriented wireless networks-on-chip. In Proceedings of the 9th International Symposium on Networks-on-Chip, Vancouver, BC, Canada, 28–30 September 2015; pp. 1–8. [Google Scholar]
- Ganguly, A.; Chang, K.; Deb, S.; Pande, P.P.; Belzer, B.; Teuscher, C. Scalable hybrid wireless network-on-chip architectures for multicore systems. IEEE Trans. Comput. 2010, 60, 1485–1502. [Google Scholar] [CrossRef]
- Wang, C.; Hu, W.H.; Bagherzadeh, N. A wireless network-on-chip design for multicore platforms. In Proceedings of the 2011 19th International Euromicro Conference on Parallel, Distributed and Network-Based Processing, Ayia Napa, Cyprus, 9–11 February 2011; pp. 409–416. [Google Scholar]
- Abadal, S.; Alarcón, E.; Cabellos-Aparicio, A.; Lemme, M.C.; Nemirovsky, M. Graphene-enabled wireless communication for massive multicore architectures. IEEE Commun. Mag. 2013, 51, 137–143. [Google Scholar] [CrossRef]
- Abadal, S.; Llatser, I.; Mestres, A.; Solé-Pareta, J.; Alarcón, E.; Cabellos-Aparicio, A. Fundamentals of graphene-enabled wireless on-chip networking. In Modeling, Methodologies and Tools for Molecular and Nano-Scale Communications: Modeling, Methodologies and Tools; Springer: Cham, Switzerland, 2017; pp. 293–317. [Google Scholar]
- Llatser, I.; Cabellos-Aparicio, A.; Alarcón, E.; Jornet, J.M.; Mestres, A.; Lee, H.; Solé-Pareta, J. Scalability of the channel capacity in graphene-enabled wireless communications to the nanoscale. IEEE Trans. Commun. 2014, 63, 324–333. [Google Scholar] [CrossRef]
- Abadal, S.; Hosseininejad, S.E.; Lemme, M.; Bolivar, P.H.; Solé-Pareta, J.; Alarcón, E.; Cabellos-Aparicio, A. Graphene-based antenna design for communications in the terahertz band. In Nanoscale Networking and Communications Handbook; CRC Press: Boca Raton, FL, USA, 2019; pp. 25–45. [Google Scholar]
- Mrunalini, S.; Manoharan, A. Dual-band re-configurable graphene-based patch antenna in terahertz band for wireless network-on-chip applications. IET Microw. Antennas Propag. 2017, 11, 2104–2108. [Google Scholar] [CrossRef]
- Abadal, S.; Llatser, I.; Mestres, A.; Lee, H.; Alarcón, E.; Cabellos-Aparicio, A. Time-domain analysis of graphene-based miniaturized antennas for ultra-short-range impulse radio communications. IEEE Trans. Commun. 2015, 63, 1470–1482. [Google Scholar] [CrossRef]
- Saxena, S.; Manur, D.S.; Shamim, M.S.; Ganguly, A. A folded wireless network-on-chip using graphene based THz-band antennas. In Proceedings of the 4th ACM International Conference on Nanoscale Computing and Communication, Washington, DC, USA, 27–29 September 2017; pp. 1–6. [Google Scholar]
- Saxena, S.; Manur, D.S.; Mansoor, N.; Ganguly, A. Scalable and energy efficient wireless inter chip interconnection fabrics using THz-band antennas. J. Parallel Distrib. Comput. 2020, 139, 148–160. [Google Scholar] [CrossRef]
- Shamim, M.S.; Mansoor, N.; Narde, R.S.; Kothandapani, V.; Ganguly, A.; Venkataraman, J. A wireless interconnection framework for seamless inter and intra-chip communication in multichip systems. IEEE Trans. Comput. 2016, 66, 389–402. [Google Scholar] [CrossRef]
- Paudel, B.; Li, X.J. A terahertz on-chip antenna for intra-chip wireless communications. In Proceedings of the 2022 IEEE Conference on Antenna Measurements and Applications (CAMA), Guangzhou, China, 14–17 December 2022; pp. 1–3. [Google Scholar]
- Crabb, J.; Cantos-Roman, X.; Aizin, G.R.; Jornet, J.M. Amplitude and Frequency Modulation with an On-chip Graphene-based Plasmonic Terahertz Nanogenerator. IEEE Trans. Nanotechnol. 2022, 21, 539–546. [Google Scholar] [CrossRef]
- Asgari, M.; Riccardi, E.; Balci, O.; De Fazio, D.; Shinde, S.M.; Zhang, J.; Mignuzzi, S.; Koppens, F.H.; Ferrari, A.C.; Viti, L.; et al. Chip-scalable, room-temperature, zero-bias, graphene-based terahertz detectors with nanosecond response time. ACS Nano 2021, 15, 17966–17976. [Google Scholar] [CrossRef] [PubMed]
- Cantos Roman, X. Multiphysics Modeling of Graphene-based Plasmonic Devices for Terahertz-Band Communication. Bachelor’s Thesis, Universitat Politècnica de Catalunya · BarcelonaTech (UPC), Barcelona, Spain, 2019. [Google Scholar]
- Petrov, V.; Moltchanov, D.; Komar, M.; Antonov, A.; Kustarev, P.; Rakheja, S.; Koucheryavy, Y. Terahertz band intra-chip communications: Can wireless links scale modern x86 CPUs? IEEE Access 2017, 5, 6095–6109. [Google Scholar] [CrossRef]
- Chen, Y.; Han, C. Channel modeling and characterization for wireless networks-on-chip communications in the millimeter wave and terahertz bands. IEEE Trans. Mol. Biol. Multi-Scale Commun. 2019, 5, 30–43. [Google Scholar] [CrossRef]
- Chen, Y.; Han, C. Channel modeling and analysis for wireless networks-on-chip communications in the millimeter wave and terahertz bands. In Proceedings of the IEEE INFOCOM 2018-IEEE Conference on Computer Communications Workshops (INFOCOM WKSHPS), Honolulu, HI, USA, 15–19 April 2018; pp. 651–656. [Google Scholar]
- Abadal, S.; Han, C.; Jornet, J.M. Wave propagation and channel modeling in chip-scale wireless communications: A survey from millimeter-wave to terahertz and optics. IEEE Access 2019, 8, 278–293. [Google Scholar] [CrossRef]
- Timoneda, X.; Abadal, S.; Franques, A.; Manessis, D.; Zhou, J.; Torrellas, J.; Alarcón, E.; Cabellos-Aparicio, A. Engineer the channel and adapt to it: Enabling wireless intra-chip communication. IEEE Trans. Commun. 2020, 68, 3247–3258. [Google Scholar] [CrossRef]
- Vien, Q.T.; Agyeman, M.O.; Le, T.A.; Mak, T. On the nanocommunications at THz band in graphene-enabled wireless network-on-chip. Math. Probl. Eng. 2017, 2017. [Google Scholar] [CrossRef]
- Laha, S.; Kaya, S.; Matolak, D.W.; Rayess, W.; DiTomaso, D.; Kodi, A. A new frontier in ultralow power wireless links: Network-on-chip and chip-to-chip interconnects. IEEE Trans. Comput.-Aided Des. Integr. Circuits Syst. 2014, 34, 186–198. [Google Scholar] [CrossRef]
- Yi, C.; Kim, D.; Solanki, S.; Kwon, J.H.; Kim, M.; Jeon, S.; Ko, Y.C.; Lee, I. Design and performance analysis of THz wireless communication systems for chip-to-chip and personal area networks applications. IEEE J. Sel. Areas Commun. 2021, 39, 1785–1796. [Google Scholar] [CrossRef]
- Dobkin, R.; Ginosar, R.; Sotiriou, C.P. Data synchronization issues in GALS SoCs. In Proceedings of the 10th International Symposium on Asynchronous Circuits and Systems, 2004. Proceedings, Crete, Greece, 19–23 April 2004; pp. 170–179. [Google Scholar]
- Jung, S.Y. Design of a preamble signal for synchronization in ultra-wideband noncoherent energy detection receivers. Int. J. Commun. Syst. 2013, 26, 465–480. [Google Scholar] [CrossRef]
- Carbonelli, C.; Mengali, U. Synchronization algorithms for UWB signals. IEEE Trans. Commun. 2006, 54, 329–338. [Google Scholar] [CrossRef]
- Stoica, L.; Rabbachin, A.; Repo, H.O.; Tiuraniemi, T.S.; Oppermann, I. An ultrawideband system architecture for tag based wireless sensor networks. IEEE Trans. Veh. Technol. 2005, 54, 1632–1645. [Google Scholar] [CrossRef]
- Gupta, A.; Medley, M.; Jornet, J.M. Joint synchronization and symbol detection design for pulse-based communications in the THz band. In Proceedings of the 2015 IEEE Global Communications Conference (GLOBECOM), San Diego, CA, USA, 6–10 December 2015; pp. 1–7. [Google Scholar]
- Singh, P.; Kim, B.W.; Jung, S.Y. Preamble-based synchronisation scheme for electromagnetic wireless nanocommunications. IET Commun. 2017, 11, 1097–1105. [Google Scholar] [CrossRef]
- Jornet, J.M.; Akyildiz, I.F. Channel modeling and capacity analysis for electromagnetic wireless nanonetworks in the terahertz band. IEEE Trans. Wirel. Commun. 2011, 10, 3211–3221. [Google Scholar] [CrossRef]
- Sarieddeen, H.; Saeed, N.; Al-Naffouri, T.Y.; Alouini, M.S. Next generation terahertz communications: A rendezvous of sensing, imaging, and localization. IEEE Commun. Mag. 2020, 58, 69–75. [Google Scholar] [CrossRef]
- Liu, C.; Wang, L.; Chen, X.; Zhou, J.; Hu, W.; Wang, X.; Li, J.; Huang, Z.; Zhou, W.; Tang, W.; et al. Room-temperature photoconduction assisted by hot-carriers in graphene for sub-terahertz detection. Carbon 2018, 130, 233–240. [Google Scholar] [CrossRef]
- Ludwig, F.; Generalov, A.; Holstein, J.; Murros, A.; Viisanen, K.; Prunnila, M.; Roskos, H.G. Terahertz Detection with Graphene FETs: Photothermoelectric and Resistive Self-Mixing Contributions to the Detector Response. ACS Appl. Electron. Mater. 2024, 6, 2197–2212. [Google Scholar] [CrossRef]
- Jornet, J.M.; Akyildiz, I.F. Femtosecond-long pulse-based modulation for terahertz band communication in nanonetworks. IEEE Trans. Commun. 2014, 62, 1742–1754. [Google Scholar] [CrossRef]
- Katzmarek, D.A.; Pradeepkumar, A.; Ziolkowski, R.W.; Iacopi, F. Review of graphene for the generation, manipulation, and detection of electromagnetic fields from microwave to terahertz. 2D Mater. 2022, 9, 022002. [Google Scholar] [CrossRef]
- Jin, M.; Wang, Y.; Chai, M.; Chen, C.; Zhao, Z.; He, T. Terahertz detectors based on carbon nanomaterials. Adv. Funct. Mater. 2022, 32, 2107499. [Google Scholar] [CrossRef]
- Yachmenev, A.; Khabibullin, R.; Ponomarev, D. Recent advances in THz detectors based on semiconductor structures with quantum confinement: A review. J. Phys. D Appl. Phys. 2022, 55, 193001. [Google Scholar] [CrossRef]
- Massicotte, M.; Soavi, G.; Principi, A.; Tielrooij, K.J. Hot carriers in graphene–fundamentals and applications. Nanoscale 2021, 13, 8376–8411. [Google Scholar] [CrossRef] [PubMed]
- Amirmazlaghani, M.; Raissi, F. Feasibility of room-temperature GHz-THz direct detection in graphene through hot-carrier effect. IEEE Trans. Device Mater. Reliab. 2018, 18, 429–437. [Google Scholar] [CrossRef]
- Sun, M.; Zhang, Y.P.; Zheng, G.X.; Yin, W.Y. Performance of intra-chip wireless interconnect using on-chip antennas and UWB radios. IEEE Trans. Antennas Propag. 2009, 57, 2756–2762. [Google Scholar] [CrossRef]
- Yan, L.; Hanson, G.W. Wave propagation mechanisms for intra-chip communications. IEEE Trans. Antennas Propag. 2009, 57, 2715–2724. [Google Scholar]
- Chen, Y.; Cai, X.; Han, C. Wave propagation modeling for mmWave and terahertz wireless networks-on-chip communications. In Proceedings of the ICC 2019—2019 IEEE International Conference on Communications (ICC), Shanghai, China, 20–24 May 2019; pp. 1–6. [Google Scholar]
- Cid-Fuentes, R.G.; Jornet, J.M.; Akyildiz, I.F.; Alarcón, E. A receiver architecture for pulse-based electromagnetic nanonetworks in the terahertz band. In Proceedings of the 2012 IEEE International Conference on Communications (ICC), Ottawa, ON, Canada, 10–15 June 2012; pp. 4937–4942. [Google Scholar]
- He, N.; Tepedelenlioglu, C. Performance analysis of non-coherent UWB receivers at different synchronization levels. IEEE Trans. Wirel. Commun. 2006, 5, 1266–1273. [Google Scholar]
- Denis, B.; Pierrot, J.B.; Abou-Rjeily, C. Joint distributed synchronization and positioning in UWB ad hoc networks using TOA. IEEE Trans. Microw. Theory Tech. 2006, 54, 1896–1911. [Google Scholar] [CrossRef]
- Jung, S.Y.; Park, D.J. Design of preamble signal for synchronization with UWB non-coherent energy detection receiver. In Proceedings of the 2005 IEEE International Conference on Ultra-Wideband, Zurich, Switzerland, 5–8 September 2005; pp. 464–468. [Google Scholar]
- Fletcher, R.; Powell, M.J. A rapidly convergent descent method for minimization. Comput. J. 1963, 6, 163–168. [Google Scholar] [CrossRef]
- Goldfarb, D. A family of variable metric updates derived by variational means, v. 24. Math. Comput. 1970, 24, 21–55. [Google Scholar] [CrossRef]
- Akyildiz, I.F.; Kak, A.; Nie, S. 6G and beyond: The future of wireless communications systems. IEEE Access 2020, 8, 133995–134030. [Google Scholar] [CrossRef]
- Akyildiz, I.F.; Han, C.; Hu, Z.; Nie, S.; Jornet, J.M. Terahertz band communication: An old problem revisited and research directions for the next decade. IEEE Trans. Commun. 2022, 70, 4250–4285. [Google Scholar] [CrossRef]


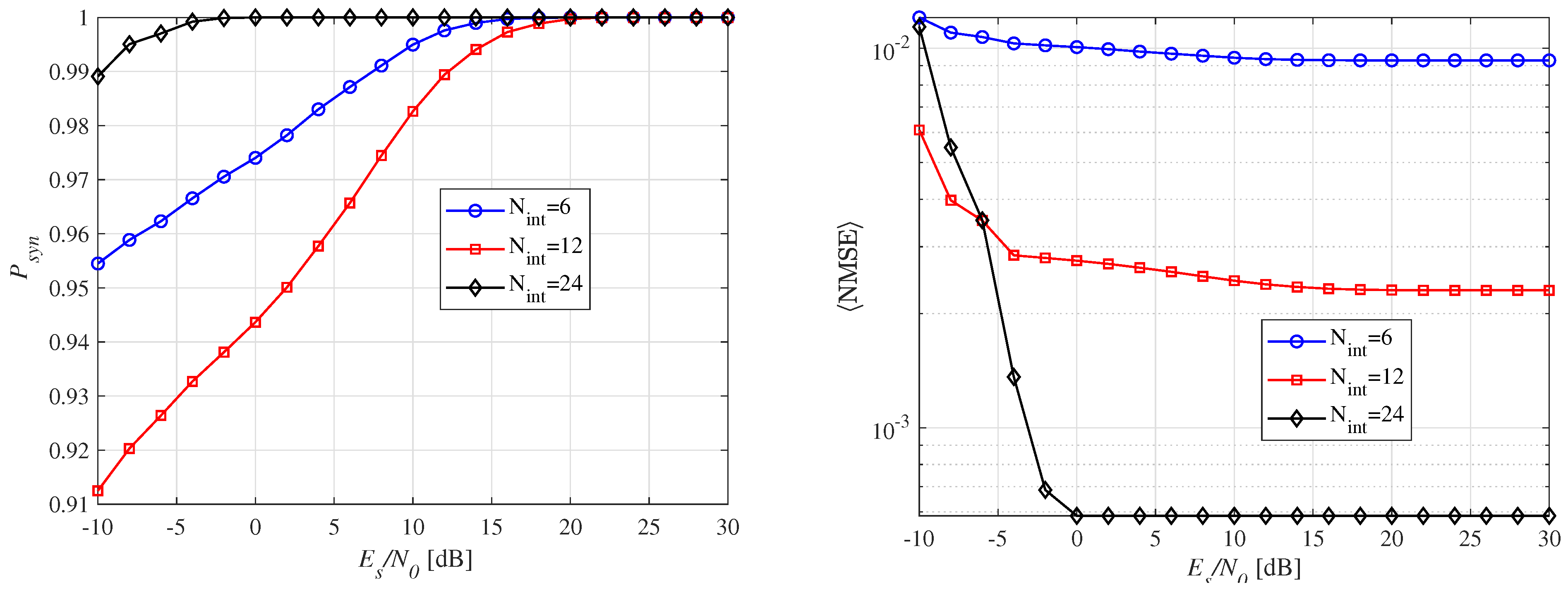
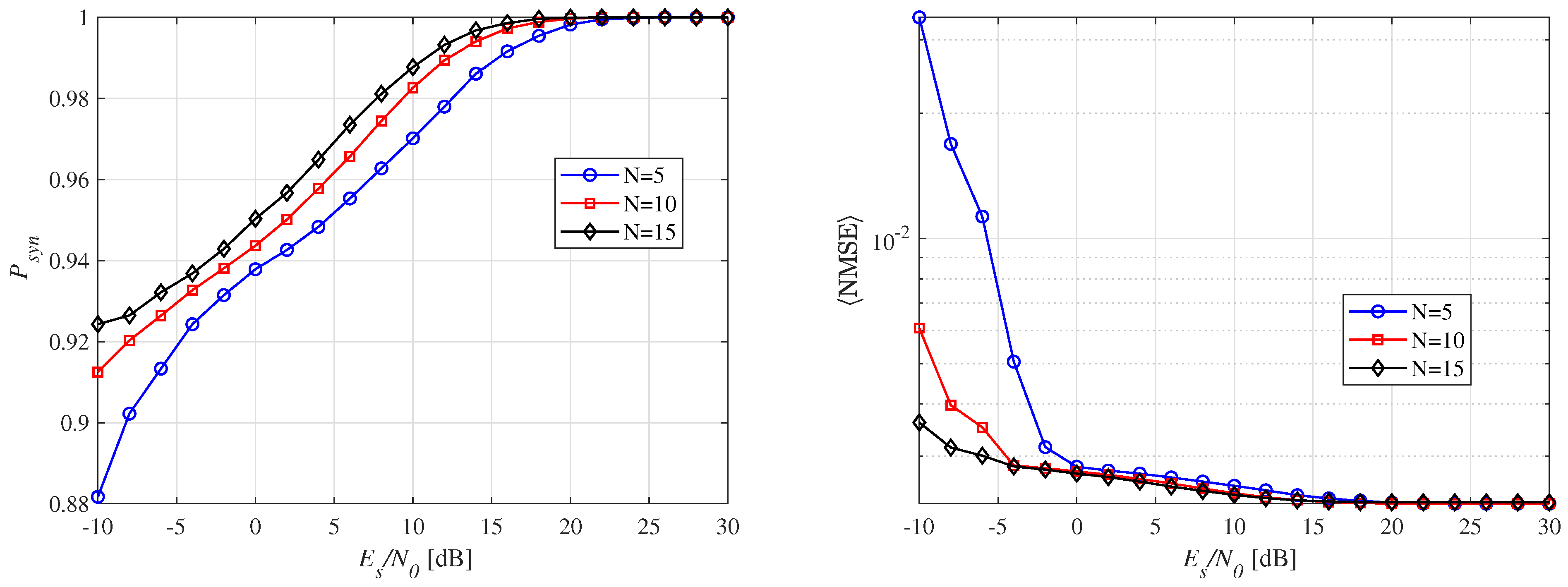
| Parameter | Values |
|---|---|
| Distance (d) | 10 mm |
| Pulse width () | 2500 fs |
| Pulse variance | 100 fs |
| Pulses in one preamble, | 12 |
| Number of integrators, | 12 |
| Number of repetitions of the basic preamble, N | 10 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2025 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Singh, P.; Jung, S.-Y. Preamble Design and Noncoherent ToA Estimation for Pulse-Based Wireless Networks-on-Chip Communications in the Terahertz Band. Micromachines 2025, 16, 70. https://doi.org/10.3390/mi16010070
Singh P, Jung S-Y. Preamble Design and Noncoherent ToA Estimation for Pulse-Based Wireless Networks-on-Chip Communications in the Terahertz Band. Micromachines. 2025; 16(1):70. https://doi.org/10.3390/mi16010070
Chicago/Turabian StyleSingh, Pankaj, and Sung-Yoon Jung. 2025. "Preamble Design and Noncoherent ToA Estimation for Pulse-Based Wireless Networks-on-Chip Communications in the Terahertz Band" Micromachines 16, no. 1: 70. https://doi.org/10.3390/mi16010070
APA StyleSingh, P., & Jung, S.-Y. (2025). Preamble Design and Noncoherent ToA Estimation for Pulse-Based Wireless Networks-on-Chip Communications in the Terahertz Band. Micromachines, 16(1), 70. https://doi.org/10.3390/mi16010070











