Synthesis Dependent Structural Magnetic and Electrical Properties of CR-Doped Lead-Free Multiferroic AlFeO3
Abstract
:1. Introduction
2. Experimental Section
3. Results and Discussion
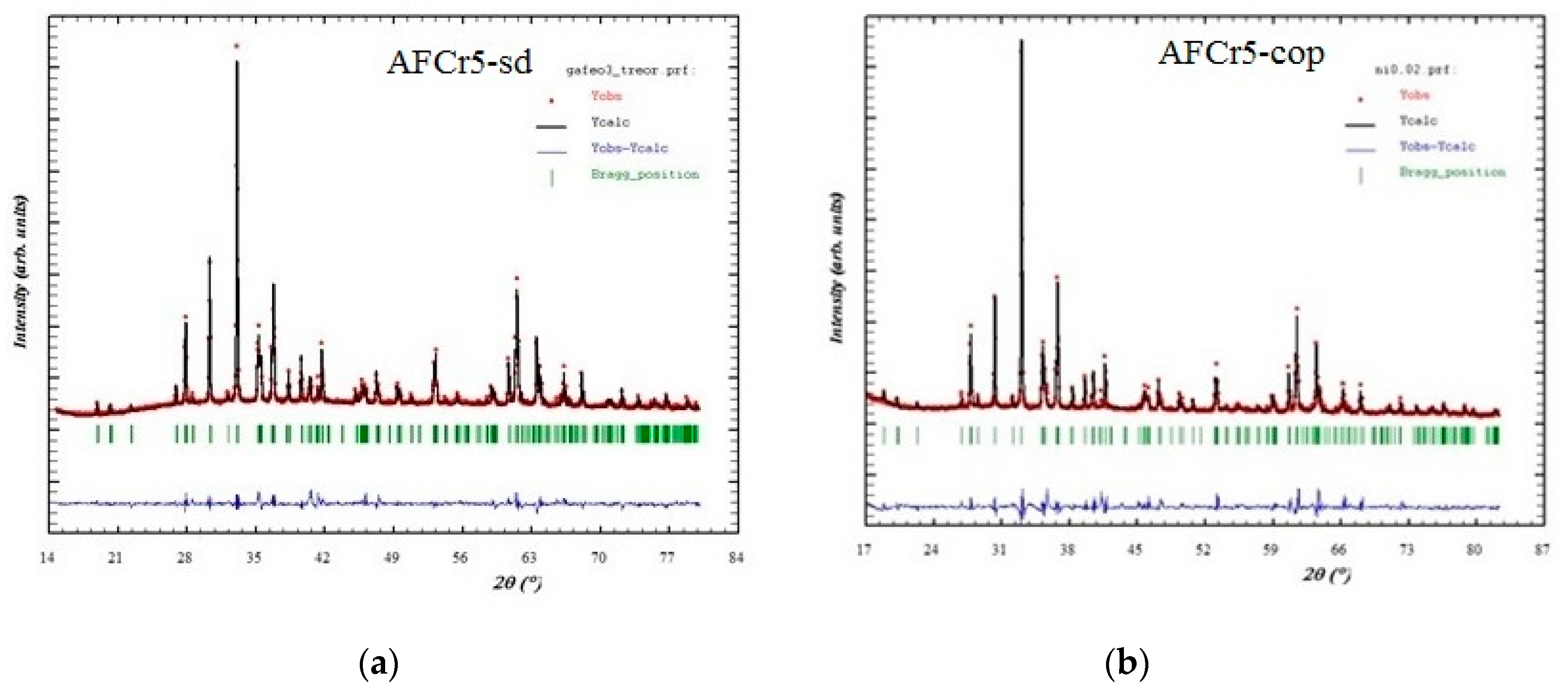
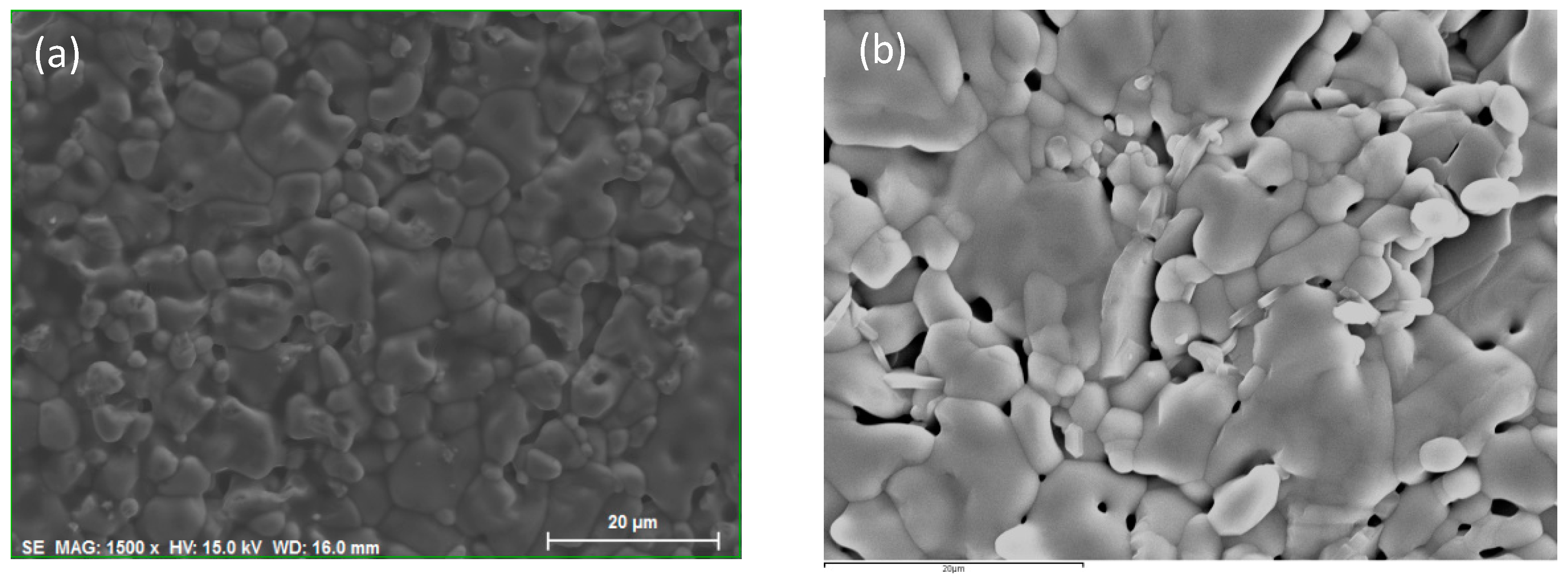
3.1. Structural Characterization
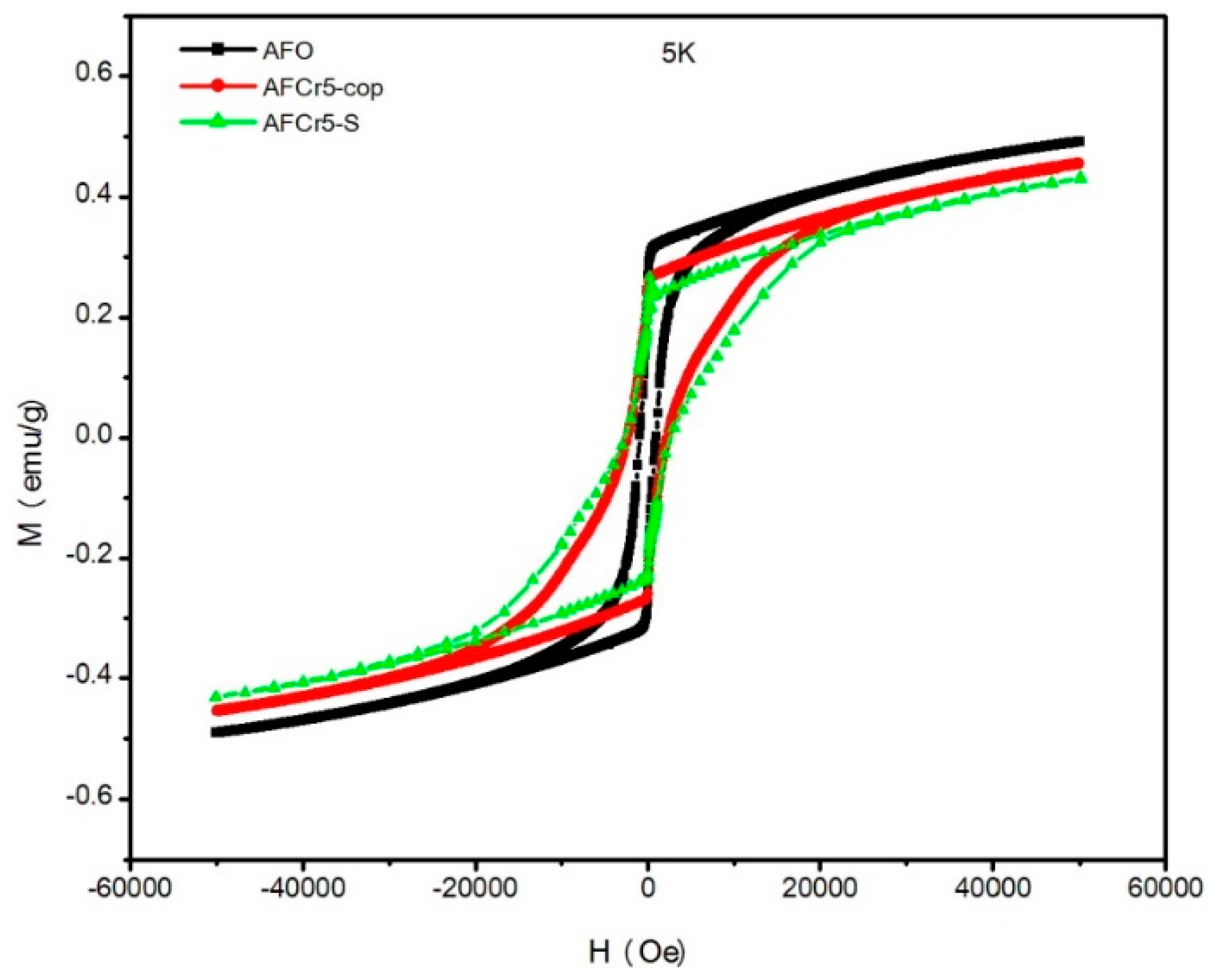
3.2. Magnetic Properties
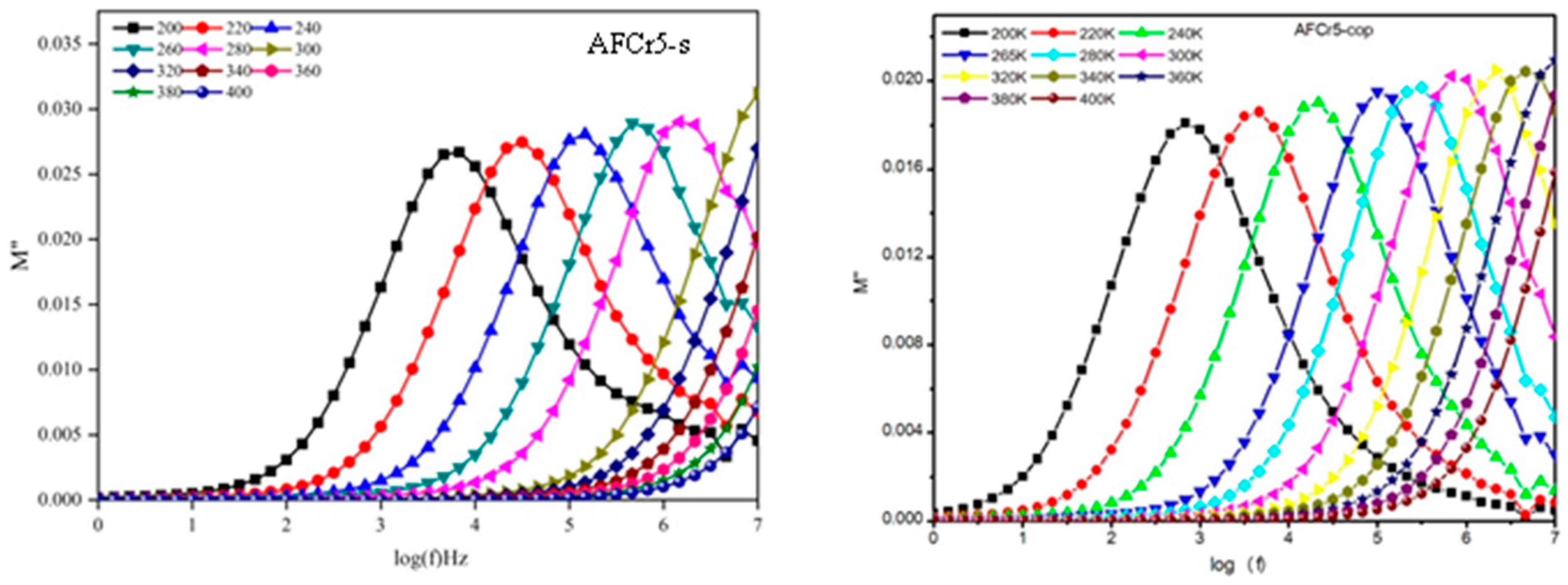
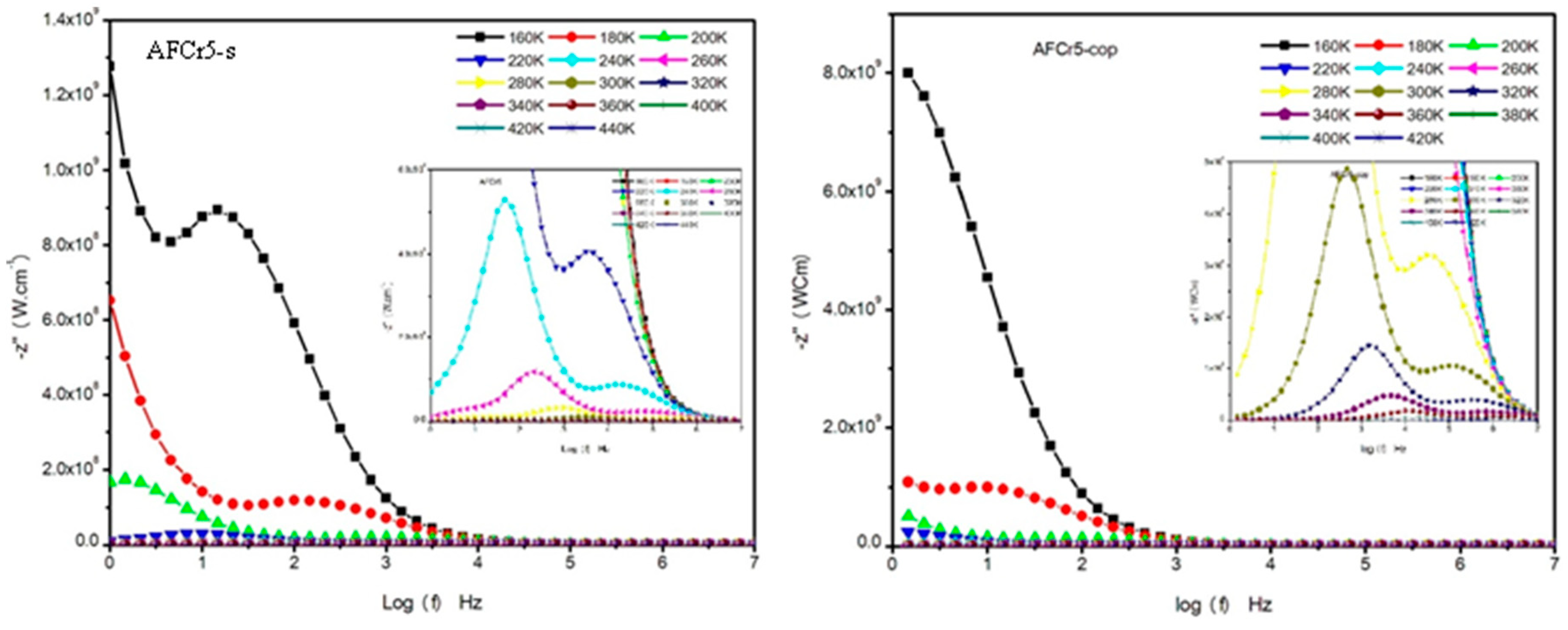
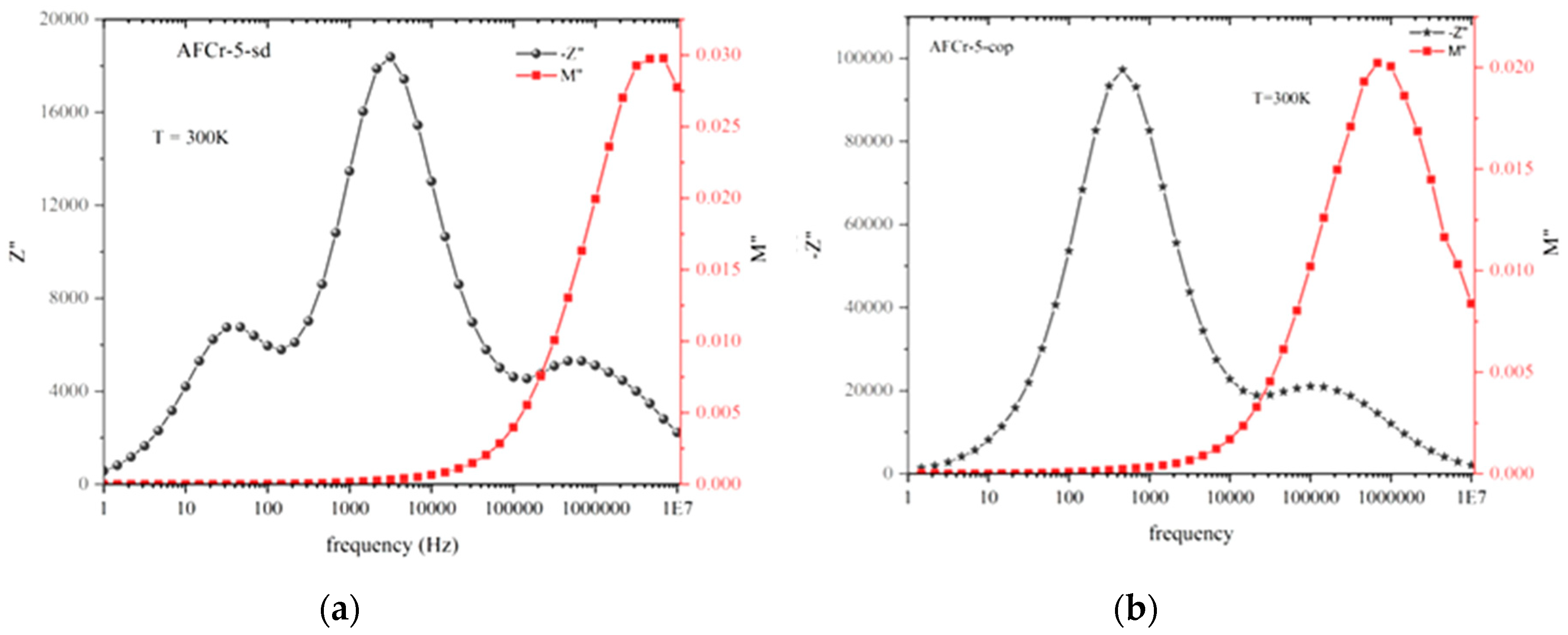
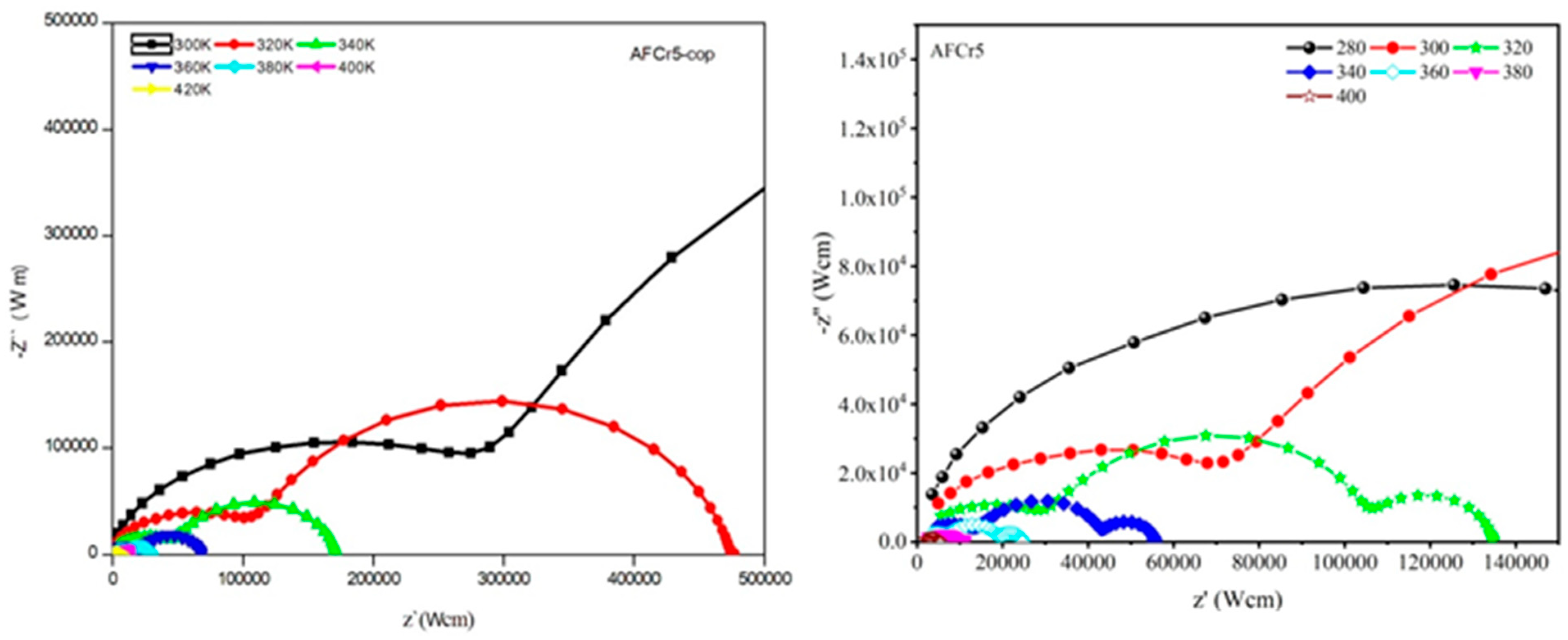
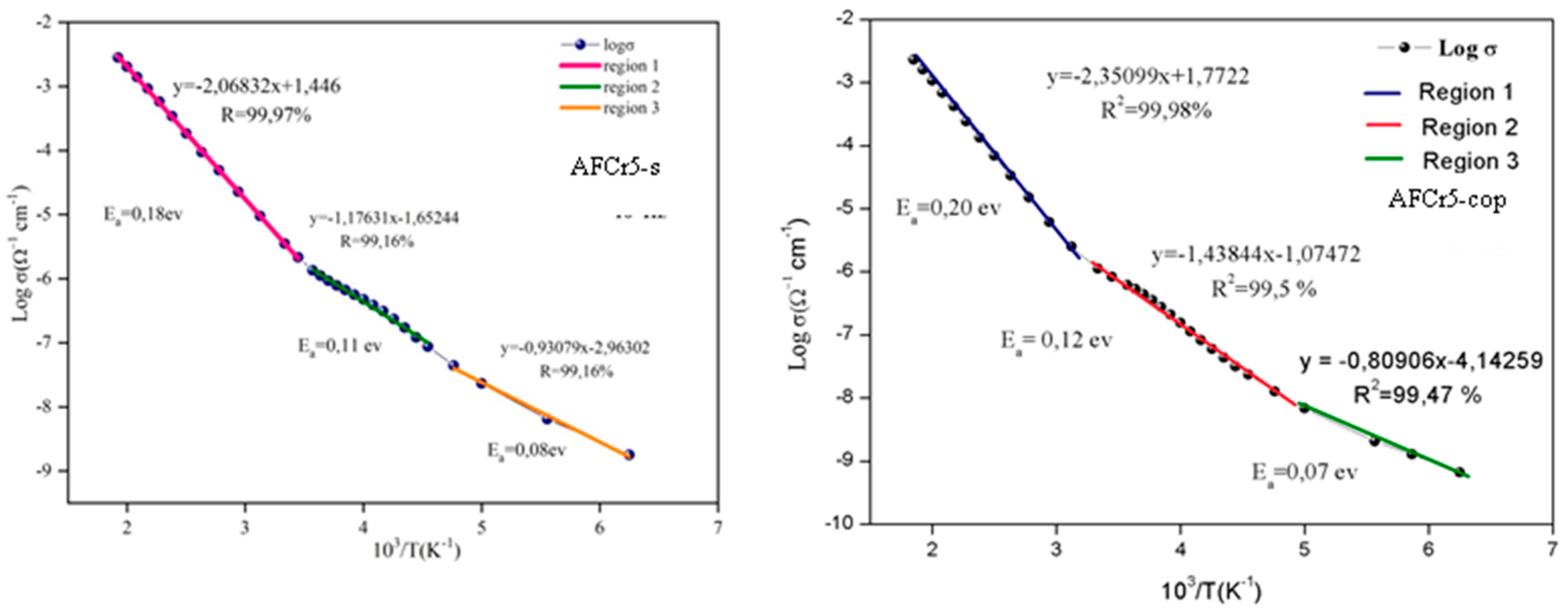
3.3. Dielectric Measurements
4. Conclusions
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
References
- Catalan, G.; Scott, J.F. Physics and applications of bismuth ferrite. Adv. Mater. 2009, 21, 2463–2485. [Google Scholar] [CrossRef]
- Hill, N.A. Why are there so few magnetic ferroelectrics? J. Phys. Chem. B 2000, 104, 6694–6709. [Google Scholar] [CrossRef]
- Ma, J.; Hu, J.; Li, Z.; Nan, C.W. Recent progress in multiferroic magnetoelectric composites: From bulk to thin films. Adv. Mater. 2011, 23, 1062–1087. [Google Scholar] [CrossRef] [PubMed]
- Shirolkar, M.M.; Hao, C.; Dong, X.; Guo, T.; Zhang, L.; Li, M.; Wang, H. Tunable multiferroic and bistable/complementary resistive switching properties of dilutely Li-doped BiFeO3 nanoparticles: An effect of aliovalent substitution. Nanoscale 2014, 6, 4735–4744. [Google Scholar] [CrossRef]
- Rao, C.N.; Sundaresan, A.; Saha, R. Multiferroic and magnetoelectric oxides: The emerging scenario. J. Phys. Chem. Lett. 2012, 3, 2237–2246. [Google Scholar] [CrossRef]
- Tokura, Y.; Seki, S. Multiferroics with spiral spin orders. Adv. Mater. 2010, 22, 1554–1565. [Google Scholar] [CrossRef]
- Edri, E.; Kirmayer, S.; Cahen, D.; Hodes, G. High open-circuit voltage solar cells based on organic–inorganic lead bromide perovskite. J. Phys. Chem. Lett. 2013, 4, 897–902. [Google Scholar] [CrossRef]
- Oishi, Y.; Tsukamoto, E.; Shimoda, M.; Takamuku, T.; Narita, T.; Era, M. A novel preparation method of lead-based layered perovskite Langmuir films with a negligible amount of PbBr2. New J. Chem. 2013, 37, 568. [Google Scholar] [CrossRef]
- Saha, R.; Shireen, A.; Shirodkar, S.N.; Singh, M.S.; Waghmare, U.V.; Waghmare, A.; Rao, C.N. Phase Transitions of AlFeO3 and GaFeO3 from the Chiral Orthorhombic (Pna 21) Structure to the Rhombohedral (R c). Struct. Inorg. Chem. 2011, 50, 9527–9532. [Google Scholar]
- Saha, R.; Shireen, A.; Shirodkar, S.N.; Waghmare, U.V.; Sundaresan, A.; Rao, C.N.R. Multiferroic and magnetoelectric nature of GaFeO3, AlFeO3 and related oxides. Solid State Commun. 2012, 152, 1964–1968. [Google Scholar] [CrossRef] [Green Version]
- Shireen, A.; Saha, R.; Mandal, P.; Sundaresan, A.; Rao, C.N.R. Multiferroic and magnetodielectric properties of the Al1−xGaxFeO3 family of oxides. J. Mater. Chem. 2011, 21, 57–59. [Google Scholar] [CrossRef]
- Saha, R.; Sundaresan, A.; Rao, C.N.R. Novel features of multiferroic and magnetoelectric ferrites and chromites exhibiting magnetically driven ferroelectricity. Mater. Horiz. 2014, 1, 20–31. [Google Scholar] [CrossRef]
- Hamasaki, Y.; Shimizu, T.; Taniguchi, H.; Taniyama, T.; Yasui, S.; Itoh, M. Epitaxial growth of metastable multiferroic AlFeO3 film on SrTiO3 (111) substrate. Appl. Phys. 2014, 104, 082906. [Google Scholar] [CrossRef]
- Pirc, R.; Blinc, R. Nonlinear magnetoelectric effect in magnetically disordered relaxor ferroelectrics. Ferroelectrics 2010, 400, 387–394. [Google Scholar] [CrossRef]
- Pirc, R.; Blinc, R.; Scott, J.F. Mesoscopic model of a system possessing both relaxor ferroelectric and relaxor ferromagnetic properties. Phys. Rev. B 2009, 79, 214114. [Google Scholar] [CrossRef]
- Liao, L.; An, R.; Shi, S.; Xu, Y.; Luo, Y.; Liao, W. One New Species of the Genus Dryomys (Rodentia, Glirgae) from Xinjiang China, Dryomys Yarkandensis sp. nov. Magn 2020, 33, 795–808. [Google Scholar] [CrossRef]
- Kumar, A.; Mishra, V.; Warshi, M.K.; Sati, A.; Sagdeo, A.; Kumar, R.; Sagdeo, P.R. Strain induced disordered phonon modes in Cr doped PrFeO3. J. Phys. Condens. Matter 2019, 31, 275602. [Google Scholar] [CrossRef]
- Sharma, N.; Mall, A.K.; Gupta, R.; Garg, A.; Singh, A.K.; Kumar, S. Temperature dependent structural and electrical analysis of Cr-doped multiferroic GaFeO3 ceramics. Mater. Res. Express 2019, 6, 115704. [Google Scholar] [CrossRef]
- Mohamed, M.B.; Senyshyn, A.; Ehrenberg, H.; Fuess, H. Structural, magnetic, dielectric properties of multiferroic GaFeO3 prepared by solid state reaction and sol–gel methods. J. Alloys Compd. 2010, 492, L20–L27. [Google Scholar] [CrossRef]
- Sharma, K.; Reddy, V.R.; Kothari, D.; Gupta, A.; Banerjee, A.; Sathe, V.G. Low temperature Raman and high field 57Fe Mossbauer study of polycrystalline GaFeO3. Phys. Condens. Matter 2010, 22, 146005. [Google Scholar] [CrossRef]
- Goodenough, J.B. Theory of the Role of Covalence in the Perovskite-Type Manganites[La, M(II)]MnO3. Phys. Rev. 1955, 100, 564–573. [Google Scholar] [CrossRef] [Green Version]
- Bora, T.; Ravi, S. Study of magnetization reversal in LaCr1−xFexO3compounds. J. Appl. Phys. 2013, 114, 033906. [Google Scholar] [CrossRef]
- Selvadurai, A.P.B.; Pazhanivelu, V.; Jagadeeshwaran, C.; Murugaraj, R.; Muthuselvam, I.P.; Chou, F.C. Influence of Cr substitution on structural, magnetic and electrical conductivity spectra of LaFeO3. J. Alloys Compd. 2015, 646, 924–931. [Google Scholar] [CrossRef]
- Bakr, M.; Mohamed; Wang, H.; Fuess, H. Dielectric relaxation and magnetic properties of Cr doped GaFeO3. J. Phys. D Appl. Phys. 2010, 43, 43455409. [Google Scholar]
- Lunkenheimer, P.; Bobnar, V.; Pronin, A.V.; Ritus, A.I.; Volkov, A.A.; Loidl, A. Origin of apparent colossal dielectric constants. Phys. Rev. B 2002, 66, 052105. [Google Scholar] [CrossRef] [Green Version]
- Cohen, M.H.; Neaton, J.B.; He, L.; Vanderbilt, D.J. Extrinsic models for the dielectric response of CaCu3Ti4O12. Appl. Phys. 2003, 94, 3299. [Google Scholar] [CrossRef] [Green Version]
- Catalan, G. Magnetocapacitance without magnetoelectric coupling. Appl. Phys. Lett. 2006, 88, 102902. [Google Scholar] [CrossRef]
- Santos, G.M.; Silva, D.M.; Freitas, V.F.; Dias, G.S.; Coelho, A.A.; Pál, M.; Santos, I.; Cotica, L.; Guo, R.; Bhalla, A.S. Multiferroic Behavior of Lead-free AlFeO3 and Mn, Nb Doped Compositions. Ferroelectrics 2014, 460, 108–116. [Google Scholar] [CrossRef]
- Khan, A.A.; Satapathy, S.; AnjuAhlawat; Deshmukh, P.; Karnal, A.K. Magneto-dielectric coupling in SmFeO3: A study on anomalous dielectric, conductivity, impedance at spin reorientation temperature. Ceram. Int. 2018, 44, 12401–12413. [Google Scholar] [CrossRef]
- Penn, S.J.; Alford, N.M.; Templeton, A.; Wang, X.; Xu, M.; Reece, M.; Schrapel, K. Effect of porosity and grain size on the microwave dielectric properties of sintered alumina. J. Am. Ceram. Soc. 1997, 80, 1885. [Google Scholar] [CrossRef]
- Ortega, N.; Kumar, A.; Bhattacharya, P.; Majumder, S.B.; Katiyar, R.S. Impedance spectroscopy of multiferroic PbZrxTi1−xO3/CoFe2O4 layered thin films. Phys. Rev. B 2008, 77, 014111. [Google Scholar] [CrossRef]
- Pike, G.E. AC conductivity of scandium oxide and a new hopping model for conductivity. Phys. Rev. B 1972, 6, 1572. [Google Scholar] [CrossRef]
- Elliot, S.R. Frequency-dependent conductivity in ionic glasses: A possible model. Solid State Ion. 1988, 27, 131–149. [Google Scholar] [CrossRef]


| a | b | c | V | |
|---|---|---|---|---|
| AFCr5-s | 8.7427(7) | 9.3875(5) | 5.0803(7) | 416.96(2) |
| AFCr5-cop | 8.7577(3) | 9.4104(6) | 5.0853(5) | 419.10(5) |
| Ms(emu/Fe) | Hc(Oe) | Mr(emu/Fe) | |
|---|---|---|---|
| AFCr5-s | 0.77 | 6435 | 0.34 |
| AFCr5-cop | 0.89 | 4035 | 0.49 |
© 2020 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Aldulmani, S.A.A.; Raies, I.; Amami, M.; Ben Farhat, L. Synthesis Dependent Structural Magnetic and Electrical Properties of CR-Doped Lead-Free Multiferroic AlFeO3. Crystals 2020, 10, 440. https://doi.org/10.3390/cryst10060440
Aldulmani SAA, Raies I, Amami M, Ben Farhat L. Synthesis Dependent Structural Magnetic and Electrical Properties of CR-Doped Lead-Free Multiferroic AlFeO3. Crystals. 2020; 10(6):440. https://doi.org/10.3390/cryst10060440
Chicago/Turabian StyleAldulmani, Sharah Ali A., Imen Raies, Mongi Amami, and Lamia Ben Farhat. 2020. "Synthesis Dependent Structural Magnetic and Electrical Properties of CR-Doped Lead-Free Multiferroic AlFeO3" Crystals 10, no. 6: 440. https://doi.org/10.3390/cryst10060440
APA StyleAldulmani, S. A. A., Raies, I., Amami, M., & Ben Farhat, L. (2020). Synthesis Dependent Structural Magnetic and Electrical Properties of CR-Doped Lead-Free Multiferroic AlFeO3. Crystals, 10(6), 440. https://doi.org/10.3390/cryst10060440




