Metallic Conduction and Carrier Localization in Two-Dimensional BEDO-TTF Charge-Transfer Solid Crystals
Abstract
:1. Introduction
2. Materials and Methods
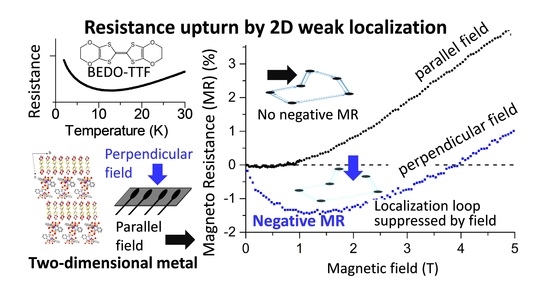
3. Results
4. Discussion
5. Conclusions
Supplementary Materials
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Ishiguro, T.; Yamaji, K.; Saito, G. Organic Superconductors, 2nd ed.; Springer: Berlin, Germany, 1998. [Google Scholar]
- Yamada, J.; Sugimoto, T. TTF Chemistry; Kodansha & Springer: Tokyo, Japan, 2004. [Google Scholar]
- Horiuchi, S.; Yamochi, H.; Saito, G.; Sakaguchi, K.; Kusunoki, M. Nature and Origin of Stable Metallic State in Organic Charge-Transfer Complexes of Bis(ethylenedioxy)tetrathiafulvalene. J. Am. Chem. Soc. 1996, 118, 8604–8622. [Google Scholar] [CrossRef]
- Beno, M.A.; Wang, H.H.; Kini, A.M.; Carlson, K.D.; Geiser, U.; Kwok, W.K.; Thompson, J.E.; Williams, J.M.; Ren, J.; Whangbo, M.H. The first ambient pressure organic superconductor containing oxygen in the donor molecule, βm-(BEDO-TTF)3Cu2(NCS)3, Tc = 1.06 K. Inorg. Chem. 1990, 29, 1599–1601. [Google Scholar] [CrossRef]
- Kahlich, S.; Schweitzer, D.; Heinen, I.; Lan, S.E.; Nuber, B.; Keller, H.J.; Winzer, K.; Helberg, H.W. (BEDO-TTF)2ReO4(H2O): A new organic superconductor. Solid State Comm. 1991, 80, 191–195. [Google Scholar] [CrossRef] [Green Version]
- Ishizaki, Y.; Izumi, M.; Ohnuki, H.; Lipinska, K.K.; Imakubo, T.; Kobayashi, K. Formation of two-dimensional weak localization in conducting Langmuir-Blodgett films. Phys. Rev. B 2001, 63, 134201. [Google Scholar] [CrossRef] [Green Version]
- Ishizaki, Y.; Izumi, M.; Ohnuki, H.; Imakubo, T.; Kalita-Lipinska, K. Observation of two-dimensional weak localization as a sign of coherent carrier transport in the conducting Langmuir–Blodgett films of BEDO-TTF and stearic acid. Colloids Surf. A 2002, 198–200, 723–728. [Google Scholar] [CrossRef]
- Ito, H.; Tamura, H.; Kuroda, S.; Yamochi, H. Structural and Transport Studies of BEDO-TTF-Arachidic-Acid Conducting Langmuir-Blodgett Films. Trans. MRS-J 2005, 30, 131–134. [Google Scholar]
- Lee, P.A.; Ramakrishhnan, T.V. Disordered electronic systems. Rev. Mod. Phys. 1985, 57, 287–337. [Google Scholar] [CrossRef]
- Hikami, S.; Larkin, A.I.; Nagaoka, Y. Spin-Orbit Interaction and Magnetoresistance in the Two Dimensional Random System. Prog. Theor. Phys. 1980, 63, 707–710. [Google Scholar] [CrossRef]
- Ulmet, J.P.; Bachere, L.; Askenazy, S.; Ousset, J.C. Negative magnetoresistance in some dimethyltri-methylene-tetraselenafulvalenium salts: A signature of weak-localization effects. Phys. Rev. B 1988, 38, 7782–7788. [Google Scholar] [CrossRef]
- Prokhorova, T.G.; Simonov, S.V.; Khasanov, S.S.; Zorina, L.V.; Buravov, L.I.; Shibaeva, R.P.; Yagubskii, E.B.; Morgunov, R.B.; Foltynowiczc, D.; S’wietlikc, R. Bifunctional molecular metals based on BEDO-TTF radical cation salts with paramagnetic [MIII(CN)6]3− anions, M= Fe, Cr, (Fe0.5Co0.5). Synth. Met. 2008, 158, 749–757. [Google Scholar] [CrossRef]
- Dubrovakii, A.D.; Spitsina, N.G.; Buravov, L.I.; Shilov, G.V.; Dyachenko, O.A.; Yagubskii, E.B.; Laukhin, V.N.; Canadell, E. New molecular metals based on BEDO radical cation salts with the square planar Ni(CN)42− anion. J. Mater. Chem. 2005, 15, 1248–1254. [Google Scholar]
- Shen, Y.; Ito, H.; Zhang, H.; Yamochi, H.; Cosquer, G.; Herrmann, C.; Ina, T.; Yoshina, S.K.; Breedlove, B.K.; Otsuka, A.; et al. Emergence of Metallic Conduction and Cobalt(II)-Based Single-Molecule Magnetism in the Same Temperature Range. J. Am. Chem. Soc. 2021, 143, 4891–4895. [Google Scholar] [CrossRef] [PubMed]
- Fettouhi, M.; Ouahab, L.; Serhani, D.; Fabre, J.-M.; Ducasse, L.; Arniell, J.; Canet, R.; Delhaesd, P. Structural and physical properties of BEDO-TTF charge-transfer salts: κ-phase with CF3SO3−. J. Mater. Chem. 1993, 3, 1101–1107. [Google Scholar] [CrossRef]
- Shen, Y.; Ito, H.; Zhang, H.; Yamochi, H.; Katagiri, S.; Yoshina, S.K.; Otsuka, A.; Ishikawa, M.; Cosquer, G.; Uchida, K.; et al. Simultaneous manifestation of metallic conductivity and single-molecule magnetism in a layered molecule-based compound. Chem. Sci. 2020, 11, 11154–11161. [Google Scholar] [CrossRef] [PubMed]
- Fettouhi, M.; Ouahab, L.; Gomez-Garcia, C.; Ducasse, L.; Delhaes, P. Structural and physical properties of κ-(BEDT-TTF)2(CF3SO3). Synth. Met. 1995, 70, 1131–1132. [Google Scholar] [CrossRef]
- Ito, H.; Asai, T.; Shimizu, Y.; Hayama, H.; Yoshida, Y.; Saito, G. Pressure-induced superconductivity in the antiferromagnet κ-(ET)2CF3SO3 with quasi-one-dimensional triangular spin lattice. Phys. Rev. B 2016, 94, 020503(R). [Google Scholar] [CrossRef]
- Ito, H.; Suzuki, D.; Yokochi, Y.; Kuroda, S.; Umemiya, M.; Miyasaka, H.; Sugiura, K.I.; Yamashita, M.; Tajima, H. Charge Carriers in Divalent BEDT-TTF Conductor (BEDT-TTF)Cu2Br4. Phys. Rev. B. 2005, 71, 085202. [Google Scholar] [CrossRef]
- Altshuler, B.L.; Aronov, A.G.; Khmelnitsky, D.E. Effects of electron-electron collisions with small energy transfers on quantum localization. J. Phys. C Solid State Phys. 1982, 15, 7367–7386. [Google Scholar] [CrossRef]
- Belitz, D.; Das Sarma, S. Inelastic phase-coherence time in thin metal films. Phys. Rev. B 1987, 36, 7701–7704. [Google Scholar] [CrossRef]
- Sergeev, A.; Mitin, V. Electron-phonon interaction in disordered conductors: Static and vibrating scattering potentials. Phys. Rev. B 2000, 61, 6041–6047. [Google Scholar] [CrossRef] [Green Version]
- Homma, Y.; Itoh, K.; Masunaga, H.; Fujiwara, A.; Nishizaki, T.; Iguchi, S.; Sasaki, T. Mesoscopic 2D Charge Transport in Commonplace PEDOT:PSS Films. Adv. Electron. Mater. 2018, 4, 1700490. [Google Scholar]






Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Ito, H.; Matsuno, M.; Katagiri, S.; Yoshina, S.K.; Takenobu, T.; Ishikawa, M.; Otsuka, A.; Yamochi, H.; Yoshida, Y.; Saito, G.; et al. Metallic Conduction and Carrier Localization in Two-Dimensional BEDO-TTF Charge-Transfer Solid Crystals. Crystals 2022, 12, 23. https://doi.org/10.3390/cryst12010023
Ito H, Matsuno M, Katagiri S, Yoshina SK, Takenobu T, Ishikawa M, Otsuka A, Yamochi H, Yoshida Y, Saito G, et al. Metallic Conduction and Carrier Localization in Two-Dimensional BEDO-TTF Charge-Transfer Solid Crystals. Crystals. 2022; 12(1):23. https://doi.org/10.3390/cryst12010023
Chicago/Turabian StyleIto, Hiroshi, Motoki Matsuno, Seiu Katagiri, Shinji K. Yoshina, Taishi Takenobu, Manabu Ishikawa, Akihiro Otsuka, Hideki Yamochi, Yukihiro Yoshida, Gunzi Saito, and et al. 2022. "Metallic Conduction and Carrier Localization in Two-Dimensional BEDO-TTF Charge-Transfer Solid Crystals" Crystals 12, no. 1: 23. https://doi.org/10.3390/cryst12010023
APA StyleIto, H., Matsuno, M., Katagiri, S., Yoshina, S. K., Takenobu, T., Ishikawa, M., Otsuka, A., Yamochi, H., Yoshida, Y., Saito, G., Shen, Y., & Yamashita, M. (2022). Metallic Conduction and Carrier Localization in Two-Dimensional BEDO-TTF Charge-Transfer Solid Crystals. Crystals, 12(1), 23. https://doi.org/10.3390/cryst12010023