Band Alignments of GeS and GeSe Materials
Abstract
:1. Introduction
2. Materials and Methods
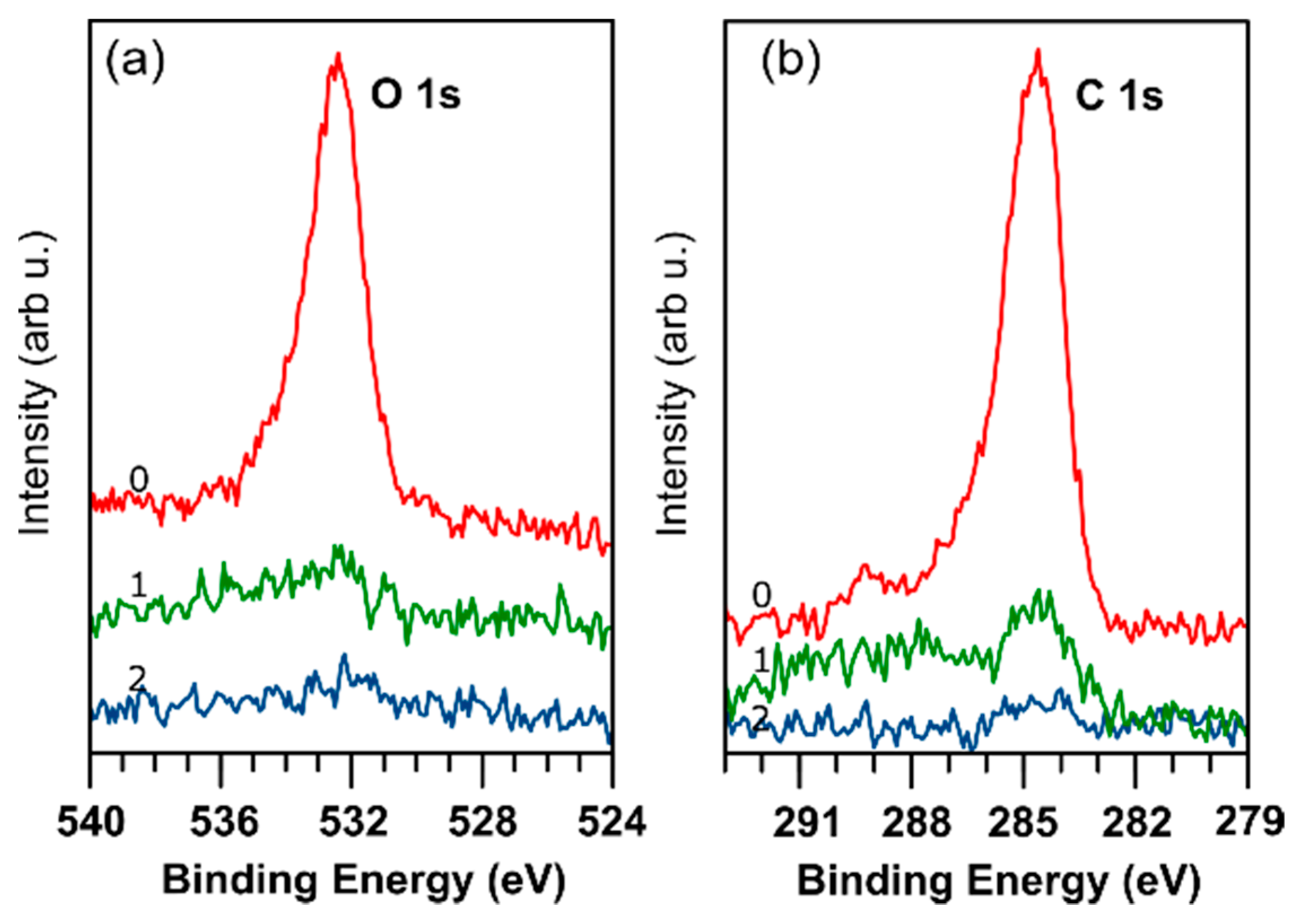
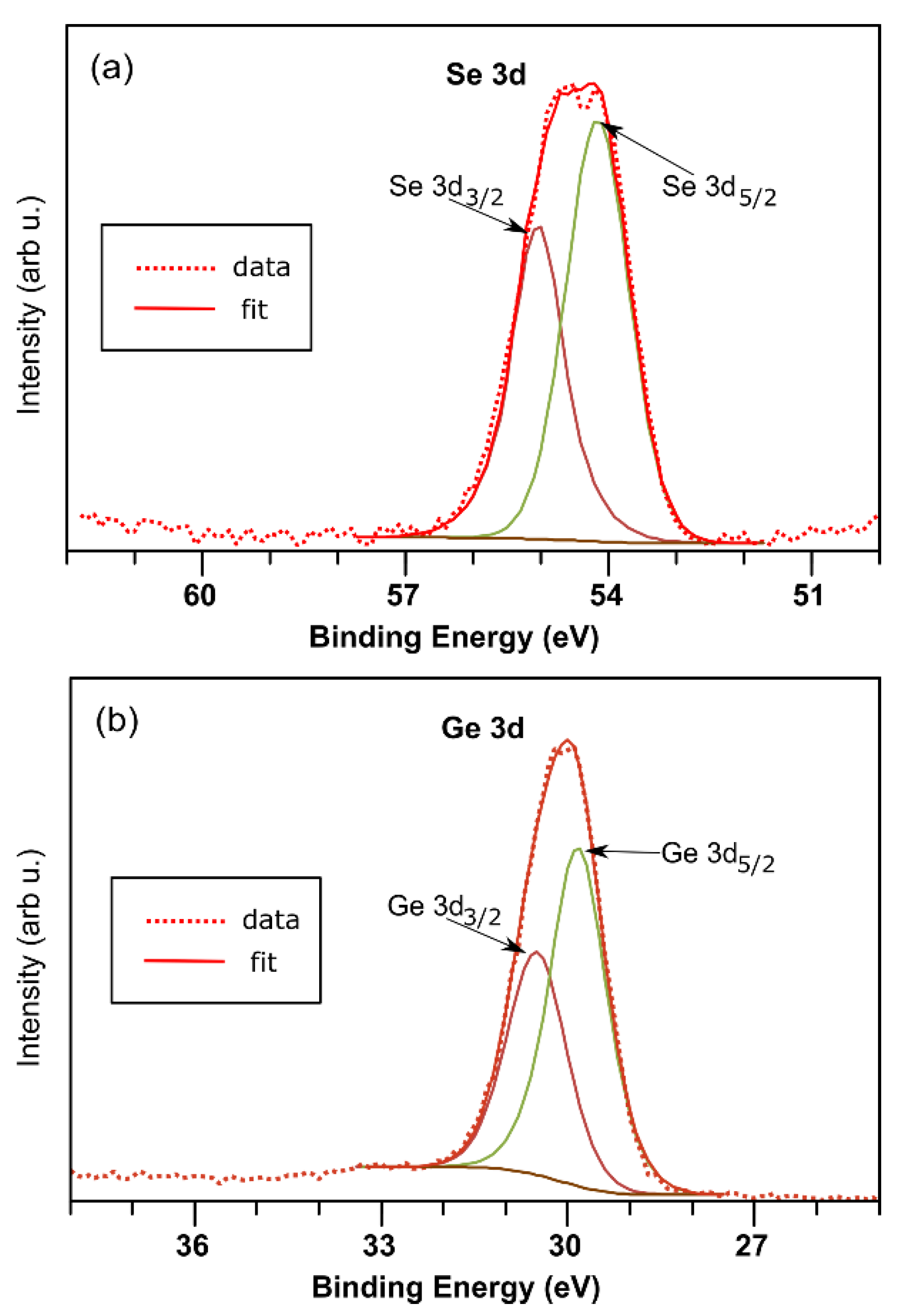
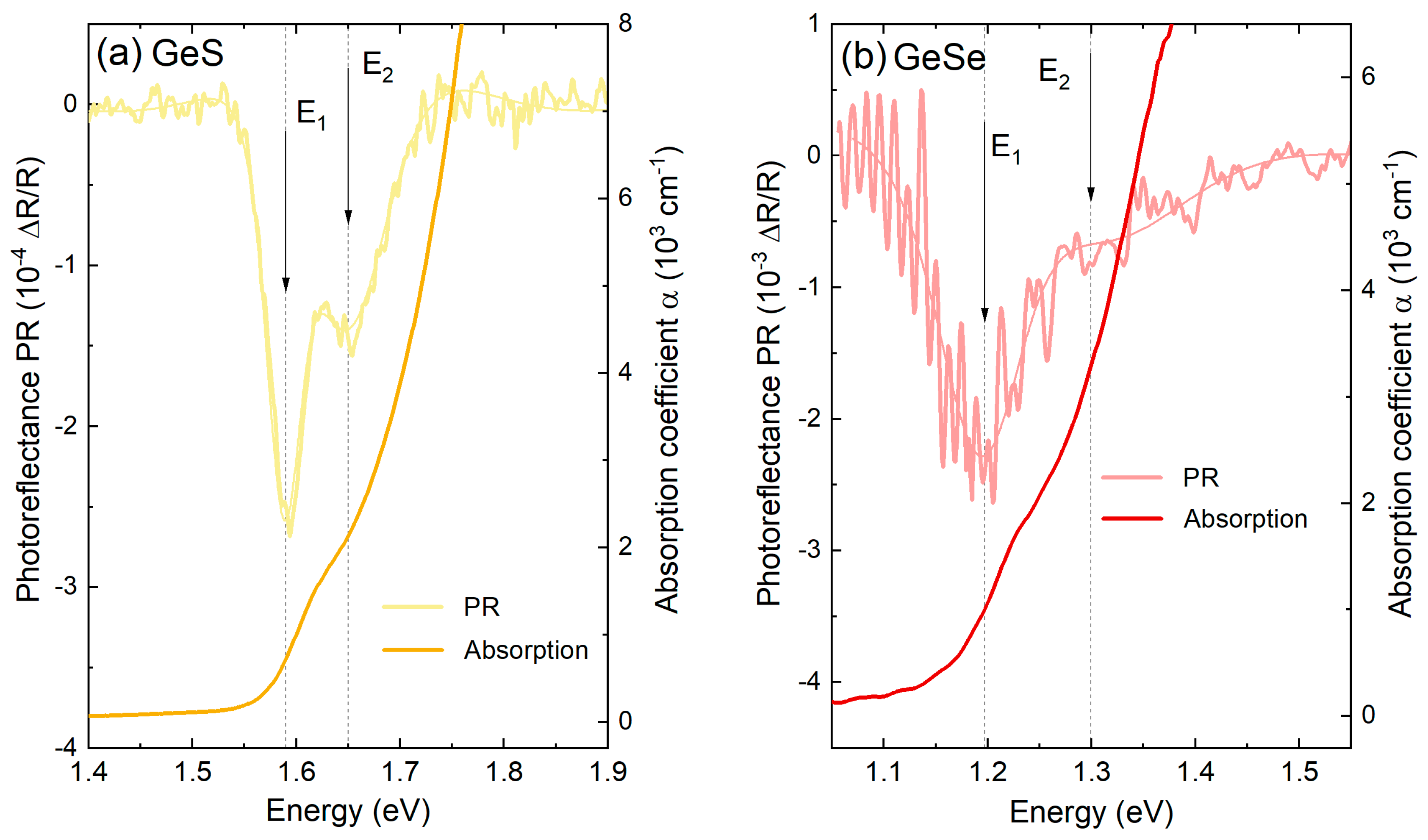
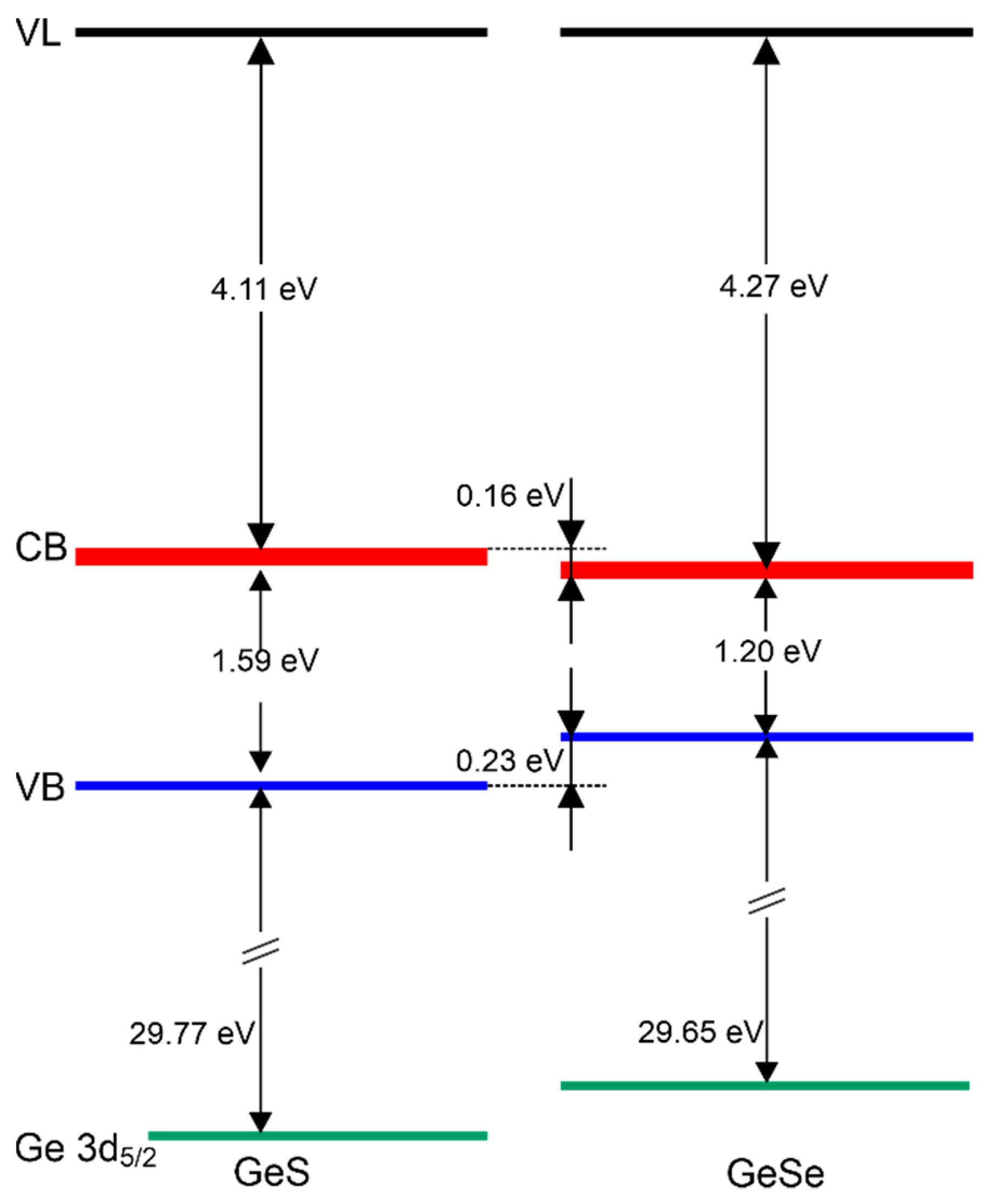
3. Results and Discussion
4. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Tołłoczko, A.; Oliva, R.; Woźniak, T.; Kopaczek, J.; Scharoch, P.; Kudrawiec, R. Anisotropic optical properties of GeS investigated by optical absorption and photoreflectance. Mater. Adv. 2020, 1, 1886–1894. [Google Scholar] [CrossRef]
- Feng, M.; Liu, S.-C.; Hu, L.; Wu, J.; Liu, X.; Xue, D.-J.; Hu, J.-S.; Wan, L.-J. Interfacial Strain Engineering in Wide-Bandgap GeS Thin Films for Photovoltaics. J. Am. Chem. Soc. 2021, 143, 9664–9671. [Google Scholar] [CrossRef] [PubMed]
- Kim, Y.; Choi, I.-H. Optical and electrical properties of GeSe and SnSe single crystals. J. Korean Phys. Soc. 2018, 72, 238–242. [Google Scholar] [CrossRef]
- Zhao, H.; Mao, Y.; Mao, X.; Shi, X.; Xu, C.; Wang, C.; Zhang, S.; Zhou, D. Band Structure and Photoelectric Characterization of GeSe Monolayers. Adv. Funct. Mater. 2018, 28, 1704855. [Google Scholar] [CrossRef]
- Tołłoczko, A.; Zelewski, S.J.; Błaszczak, M.; Woźniak, T.; Siudzińska, A.; Bachmatiuk, A.; Scharoch, P.; Kudrawiec, R. Optical properties of orthorhombic germanium selenide: An anisotropic layered semiconductor promising for optoelectronic applications. J. Mater. Chem. C 2021, 9, 14838–14847. [Google Scholar] [CrossRef]
- Murgatroyd, P.A.E.; Smiles, M.J.; Savory, C.N.; Shalvey, T.P.; Swallow, J.E.N.; Fleck, N.; Robertson, C.M.; Jäckel, F.; Alaria, J.; Major, J.D.; et al. GeSe: Optical Spectroscopy and Theoretical Study of a van der Waals Solar Absorber. Chem. Mater. 2022, 30, 3245–3253. [Google Scholar] [CrossRef] [PubMed]
- Ding, Y.; Liu, Y.-S.; Yang, G.; Gu, Y.; Fan, Q.; Lu, N.; Zhao, H.; Yu, Y.; Zhang, X.; Huo, X.; et al. High-Performance Ballistic Quantum Transport of Sub-10 nm Monolayer GeS Field-Effect Transistors. ACS Appl. Electron. Mater. 2021, 3, 1151–1161. [Google Scholar] [CrossRef]
- Kumar Ulaganathan, R.; Lu, Y.-Y.; Kuo, C.-J.; Reddy Tamalampudi, S.; Sankar, R.; Moorthy Boopathi, K.; Anand, A.; Yadav, K.; Jesus Mathew, R.; Liu, C.-R.; et al. High photosensitivity and broad spectral response of multi-layered germanium sulfide transistors. Nanoscale 2016, 8, 2284–2292. [Google Scholar] [CrossRef] [PubMed]
- Li, H.; Wang, Q.; Liu, F.; Lu, J. Lifting on-state currents for GeS-based tunneling field-effect transistors with electrode optimization. Appl. Surf. Sci. 2022, 602, 154297. [Google Scholar] [CrossRef]
- Liu, S.-C.; Yang, Y.; Li, Z.; Xue, D.-J.; Hu, J.-S. GeSe thin-film solar cells. Mater. Chem. Front. 2020, 4, 775–787. [Google Scholar] [CrossRef]
- Ramasamy, P.; Kwak, D.; Lim, D.-H.; Ra, H.-S.; Lee, J.-S. Solution synthesis of GeS and GeSe nanosheets for high-sensitivity photodetectors. J. Mater. Chem. C 2016, 4, 479–485. [Google Scholar] [CrossRef]
- Vaughn, D.D.; Patel, R.J.; Hickner, M.A.; Schaak, R.E. Single-Crystal Colloidal Nanosheets of GeS and GeSe. J. Am. Chem. Soc. 2010, 132, 15170–15172. [Google Scholar] [CrossRef] [PubMed]
- Shalvoy, R.B.; Fisher, G.B.; Stiles, P.J. Bond ionicity and structural stability of some average-valence-five materials studied by x-ray photoemission. Phys. Rev. B 1977, 15, 1680–1697. [Google Scholar] [CrossRef]
- Ueno, T. X-ray Photoelectron and Anger Electron Spectroscopic Studies of Chemical Shifts in Amorphous Ge–Se System. Jpn. J. Appl. Phys. 1983, 22, 1349. [Google Scholar] [CrossRef]
- Wei, Y.; He, J.; Zhang, Q.; Liu, C.; Wang, A.; Li, H.; Zhai, T. Synthesis and investigation of layered GeS as a promising large capacity anode with low voltage and high efficiency in full-cell Li-ion batteries. Mater. Chem. Front. 2017, 1, 1607–1614. [Google Scholar] [CrossRef]
- Lam, D.; Chen, K.-S.; Kang, J.; Liu, X.; Hersam, M.C. Anhydrous Liquid-Phase Exfoliation of Pristine Electrochemically Active GeS Nanosheets. Chem. Mater. 2018, 30, 2245–2250. [Google Scholar] [CrossRef]
- Smiles, M.J.; Skelton, J.M.; Shiel, H.; Jones, L.A.H.; Swallow, J.E.N.; Edwards, H.J.; Murgatroyd, P.A.E.; Featherstone, T.J.; Thakur, P.K.; Lee, T.-L.; et al. Ge 4s2 lone pairs and band alignments in GeS and GeSe for photovoltaics. J. Mater. Chem. A 2021, 9, 22440–22452. [Google Scholar] [CrossRef]
- Xue, D.-J.; Liu, S.-C.; Dai, C.-M.; Chen, S.; He, C.; Zhao, L.; Hu, J.-S.; Wan, L.-J. GeSe Thin-Film Solar Cells Fabricated by Self-Regulated Rapid Thermal Sublimation. J. Am. Chem. Soc. 2017, 8, 958–965. [Google Scholar] [CrossRef]
- Hou, G.-J.; Wang, D.-L.; Ali, R.; Zhou, Y.-R.; Zhu, Z.-G.; Su, G. CH3NH3PbI3/GeSe Bilayer Heterojunction Solar Cell with High Performance. arXiv 2017, arXiv:1712.01369. Available online: http://arxiv.org/abs/1712.01369 (accessed on 28 July 2022). [CrossRef] [Green Version]
- Hosokawa, S.; Hari, Y.; Kouchi, T.; Ono, I.; Sato, H.; Taniguchi, M.; Hiraya, A.; Takata, Y.; Kosugi, N.; Watanabe, M. Electronic structures and local atomic configurations in amorphous GeSe and GeTe. J. Phys. Condens. Matter 1998, 10, 1931–1950. [Google Scholar] [CrossRef]
- Greczynski, G.; Hultman, L. C 1s Peak of Adventitious Carbon Aligns to the Vacuum Level: Dire Consequences for Material’s Bonding Assignment by Photoelectron Spectroscopy. ChemPhysChem 2017, 18, 1507–1512. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Greczynski, G.; Hultman, L. X-ray photoelectron spectroscopy: Towards reliable binding energy referencing. Prog. Mater. Sci. 2020, 107, 100591. [Google Scholar] [CrossRef]
- Greczynski, G.; Hultman, L. Reliable determination of chemical state in x-ray photoelectron spectroscopy based on sample-work-function referencing to adventitious carbon: Resolving the myth of apparent constant binding energy of the C 1s peak. Appl. Surf. Sci. 2018, 451, 99–103. [Google Scholar] [CrossRef]
- Martin, W.C. Energy Levels of Neutral Helium (4He I). J. Phys. Chem. Ref. Data 1973, 2, 257–266. [Google Scholar] [CrossRef] [Green Version]
- Liu, S.-C.; Dai, C.-M.; Min, Y.; Hou, Y.; Proppe, A.H.; Zhou, Y.; Chen, C.; Chen, S.; Tang, J.; Xue, D.-J.; et al. An antibonding valence band maximum enables defect-tolerant and stable GeSe photovoltaics. Nat. Commun. 2021, 12, 670. [Google Scholar] [CrossRef] [PubMed]
- Solanki, G.K.; Deshpande, M.P.; Agarwal, M.K.; Patel, P.D.; Vaidya, S.N. Thermoelectric power factor measurements in GeSe single crystals grown using different transporting agents. J. Mater. Sci. Lett. 2003, 22, 985–987. [Google Scholar] [CrossRef]
- Aspnes, D.E. Third-derivative modulation spectroscopy with low-field electroreflectance. Surf. Sci. 1973, 37, 418–442. [Google Scholar] [CrossRef]
- Gomes, L.C.; Carvalho, A. Phosphorene analogues: Isoelectronic two-dimensional group-IV monochalcogenides with orthorhombic structure. Phys. Rev. B 2015, 92, 085406. [Google Scholar] [CrossRef] [Green Version]
- Oliva, R.; Woźniak, T.; Dybala, F.; Tołłoczko, A.; Kopaczek, J.; Scharoch, P.; Kudrawiec, R. Valley polarization investigation of GeS under high pressure. Phys. Rev. B 2020, 101, 235205. [Google Scholar] [CrossRef]
- Grodzicki, M.; Sito, J.; Lewandków, R.; Mazur, P.; Ciszewski, A. Interfacial Polarization of Thin Alq3, Gaq3, and Erq3 Films on GaN(0001). Materials 2022, 15, 1671. [Google Scholar] [CrossRef]
- Zelewski, S.J.; Kudrawiec, R. Photoacoustic and modulated reflectance studies of indirect and direct band gap in van der Waals crystals. Sci. Rep. 2017, 7, 15365. [Google Scholar] [CrossRef] [PubMed]



| EVBM (eV) | ϕ (eV) | χ (eV) | EI (eV) | EB (eV) | Ge 3d5/2 (eV) | S 2p3/2 (eV) | Se 3d5/2 (eV) | |
|---|---|---|---|---|---|---|---|---|
| GeS | 0.38 | 5.32 | 4.11 | 5.70 | 1.59 | 30.15 | 162 | - |
| GeSe | 0.20 | 5.27 | 4.27 | 5.47 | 1.20 | 29.85 | - | 54.15 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Grodzicki, M.; Tołłoczko, A.K.; Majchrzak, D.; Hommel, D.; Kudrawiec, R. Band Alignments of GeS and GeSe Materials. Crystals 2022, 12, 1492. https://doi.org/10.3390/cryst12101492
Grodzicki M, Tołłoczko AK, Majchrzak D, Hommel D, Kudrawiec R. Band Alignments of GeS and GeSe Materials. Crystals. 2022; 12(10):1492. https://doi.org/10.3390/cryst12101492
Chicago/Turabian StyleGrodzicki, Miłosz, Agata K. Tołłoczko, Dominika Majchrzak, Detlef Hommel, and Robert Kudrawiec. 2022. "Band Alignments of GeS and GeSe Materials" Crystals 12, no. 10: 1492. https://doi.org/10.3390/cryst12101492
APA StyleGrodzicki, M., Tołłoczko, A. K., Majchrzak, D., Hommel, D., & Kudrawiec, R. (2022). Band Alignments of GeS and GeSe Materials. Crystals, 12(10), 1492. https://doi.org/10.3390/cryst12101492







