On the Definition of Phase Diagram
Abstract
:1. Introduction
- phase diagram n. Chemistry a diagram which represents the limits of stability of the various phases of a chemical system at equilibrium, with respect to two or more variables (commonly composition and temperature); an equilibrium diagram.
2. Glossary of the Key Terms Related to Phase Diagrams
- An ideal phase diagram is a P–T plot of thermodynamically stable phases in the equilibrium state. There is only one ideal phase diagram for any stoichiometric assembly of atomic species. It is impossible to experimentally determine an ideal phase diagram because all experiments are dynamic, hysteresis in first-order phase transitions cannot be completely eliminated, and nonhydrostatic stresses and P,T gradients are inevitable;
- A phase diagram is the best estimate of the ideal phase diagram based on experimental and theoretical constraints;
- A dynamic P–T diagram represents observed or predicted phases that can be produced during the course of dynamic compression or decompression (or cooling/heating). A dynamic P–T diagram can include metastable or transitional states and must include descriptions of the necessary conditions: the compression or decompression rate, cooling or heating rate, stress–strain conditions, etc. Note that some authors refer to dynamic P–T diagrams as “dynamic phase diagrams”. The reason for this discrepancy is related to the definition of a phase, and the question of whether the thermodynamic metastable state can be regarded a phase. While a full discussion of this issue is beyond this opinion paper’s scope, I am leaning towards using the first option (i.e., a dynamic P–T diagram);
- A transitional P–T diagram (sometimes referred to as a transitional phase diagram) represents the P–T diagram that includes metastable states (i.e., the states outside of the stability region in the ideal phase diagram). Contrary to an ideal phase diagram, a transitional P–T diagram depicts a system that is far from thermodynamic equilibrium;
- The difference between dynamic and transitional diagrams can be characterized as semantic rather than phenomenological. The term dynamic P–T diagram is used mainly in dynamic-loading studies, where fast pressure variation is the intrinsic feature of the experimental methodology (shock compression, ramp compression, piezo-electrically driven dynamic diamond-anvil cells), and the observed states often have very short lifetimes. A transitional P–T diagram applies mostly to quasistatic experiments, where metastable states are “quenched” from the initial thermodynamic equilibrium and can be stabilized for a relatively long time (from minutes to years). This type of P–T diagram is largely related to multicomponent materials, but it can also describe chemical elements. For example, a transitional P–T diagram of buckminsterfullerene (C60) reports the forms of carbon in which the molecular integrity of a C60 molecule is preserved. It is different than the ideal phase diagram of carbon, as all the states of carbon consisting of C60 molecules are metastable under any P–T condition. Note that there are also other terms used to convey similar notions, such as phase evolution diagram or kinetic phase diagram [6]. The term metastable phase diagram, which sometimes occurs in the literature, is ambiguous and should be abandoned;
- By using terms such as reaction P–T diagram [7] or transformation P–T diagram [8], some authors intend to emphasize that the transitions between different phases or states displayed on a diagram involve chemical reactions and the rearrangement of the bonding pattern (e.g., transformations from a molecular to an extended solid), although this additional specification seems redundant given the previously defined types of P–T diagrams.
3. Classification of Phase Transitions and Definitions of Phase Stability
4. Case Studies
4.1. Stable or Metastable, That Is the Question
4.1.1. Chemical Elements
4.1.2. Chemical Compounds
4.2. Pressure-Induced Amorphization, Glassy States, and Liquid–Liquid Transitions
4.3. Critical Points and Supercritical States
4.4. Nonequilibrium Studies and Dynamic P–T Diagrams
5. Conclusions and Call for Actions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Ogden, C.K.; Richards, I.A. The Meaning of Meaning: A Study of the Influence of Language upon Thought and of the Science of Symbolism, 1st ed.; Routledge & Kegan Paul: London, UK, 1923. [Google Scholar]
- IUPAC Gold Book. Available online: https://goldbook.iupac.org (accessed on 25 July 2022).
- IUCr Online Dictionary of Crystallography. Available online: https://dictionary.iucr.org (accessed on 25 July 2022).
- Grochala, W. Pressure-induced phase transitions. In Chemical Reactivity in Confined Systems: Theory, Modelling and Applications, 1st ed.; Chattaraj, P.K., Chakraborty, A., Eds.; Wiley: Hoboken, NJ, USA, 2021; pp. 25–47. [Google Scholar]
- Oxford English Dictionary Online. Available online: https://www.oed.com (accessed on 25 July 2022).
- Luo, K.; Liu, B.; Hu, W.; Dong, X.; Wang, Y.; Huang, Q.; Gao, Y.; Sun, L.; Zhao, Z.; Wu, Y.; et al. Coherent interfaces with mixed hybridization govern direct transformation from graphite to diamond. Nature 2022, 607, 486–491. [Google Scholar] [CrossRef] [PubMed]
- Turnbull, R.; Hanfland, M.; Binns, J.; Martinez-Canales, M.; Frost, M.; Marqués, M.; Howie, R.T.; Gregoryanz, E. Unusually complex phase of dense nitrogen at extreme conditions. Nat. Commun. 2018, 9, 4717. [Google Scholar] [CrossRef] [PubMed]
- Bundy, F.P.; Bassett, W.A.; Weathers, M.S.; Hemley, R.J.; Mao, H.K.; Goncharov, A.F. The pressure-temperature phase and transformation diagram for carbon; updated through 1994. Carbon 1996, 34, 141–153. [Google Scholar] [CrossRef]
- Ehrenfest, P. Phasenumwandlungen im ueblichen und erweiterten Sinn, Classifiziert nach dem Entsprechenden Singularitaeten des Thermodynamischen Potentiales; N. V. Noord-Hollandsche Uitgevers Maatschappij: Amsterdam, The Netherlands, 1933; Volume 36, pp. 153–157. [Google Scholar]
- Jaeger, G. The Ehrenfest classification of phase transitions: Introduction and evolution. Arch. Hist. Exact Sci. 1998, 53, 51–81. [Google Scholar] [CrossRef]
- Sauer, T. A look back at the Ehrenfest classification. Translation and commentary of Ehrenfest’s 1933 paper introducing the notion of phase transitions of different order. Eur. Phys. J. Spec. Topics 2017, 226, 539–549. [Google Scholar] [CrossRef] [Green Version]
- Landau, L.D.; Lifshitz, E.M. Statistical Physics, 3rd ed.; Pergamon Press: Oxford, UK, 1980. [Google Scholar]
- Frenkel, J. Kinetic Theory of Liquids, 1st ed.; Clarendon Press: Oxford, UK, 1946. [Google Scholar]
- Turnbull, D. Phase changes. In Solid State Physics, 1st ed.; Seitz, F., Turnbull, D., Eds.; Academic Press: New York, NY, USA, 1956; Volume 3, pp. 225–306. [Google Scholar]
- Dash, J.G. Melting from one to two to three dimensions. Contemp. Phys. 2002, 43, 427–436. [Google Scholar] [CrossRef]
- Mnyuk, Y. Mechanism and kinetics of phase transitions and other reactions in solids. Am. J. Condens. Matter Phys. 2013, 3, 89–103. [Google Scholar]
- Muller, P. Glossary of terms used in physical organic chemistry (IUPAC Recommendations 1994). Pure Appl. Chem. 1994, 66, 1077–1184. [Google Scholar] [CrossRef] [Green Version]
- Tschoegl, N.W. Fundamentals of Equilibrium and Steady-State Thermodynamics, 1st ed.; Elsevier: Amsterdam, The Netherlands, 2000. [Google Scholar]
- Wallace, D.C. Thermodynamics of Crystals, 1st ed.; Wiley: New York, NY, USA, 1972. [Google Scholar]
- Born, M. On the stability of crystal lattices. I. Math. Proc. Cambridge Philos. Soc. 1940, 36, 160–172. [Google Scholar] [CrossRef]
- Mouhat, F.; Coudert, F.-X. Necessary and sufficient elastic stability conditions in various crystal systems. Phys. Rev. B 2014, 90, 224104. [Google Scholar] [CrossRef] [Green Version]
- Abu-Jafar, M.S.; Leonhardi, V.; Jaradat, R.; Mousa, A.A.; Al-Qaisi, S.; Mahmoud, N.T.; Bassalat, A.; Khenata, R.; Bouhemadou, A. Structural, electronic, mechanical, and dynamical properties of scandium carbide. Results Phys. 2021, 21, 103804. [Google Scholar] [CrossRef]
- Kadkhodaei, S.; Hong, Q.-J.; van de Walle, A. Free energy calculation of mechanically unstable but dynamically stabilized bcc titanium. Phys. Rev. B 2017, 95, 064101. [Google Scholar] [CrossRef] [Green Version]
- Young, D.A. Phase Diagrams of the Elements, 1st ed.; University of California Press: Berkeley, CA, USA, 1991. [Google Scholar]
- Tonkov, E.Y.; Ponyatovsky, E.G. Phase Transformations of the Elements Under High Pressure, 1st ed.; CRC Press: Boca Raton, FL, USA, 2005. [Google Scholar]
- Holzapfel, W.B. Structures of the elements—Crystallography and art. Acta Crystallogr. B 2014, 70, 429–435. [Google Scholar] [CrossRef] [PubMed]
- SACADA—Samara Carbon Allotrope Database. Available online: https://www.sacada.info (accessed on 25 July 2022).
- Hoffmann, R.; Kabanov, A.A.; Golov, A.A.; Proserpio, D.M. Homo Citans and Carbon Allotropes: For an Ethics of Citation. Angew. Chem. Int. Ed. Engl. 2016, 55, 10962–10976. [Google Scholar] [CrossRef] [PubMed]
- Bundy, F.P. Pressure-temperature phase diagram of elemental carbon. Phys. A Stat. Mech. Its Appl. 1989, 156, 169–178. [Google Scholar] [CrossRef]
- Zazula, J.M. On Graphite Transformations at High Temperature and Pressure Induced by Absorption of the LHC Beam; CERN-LHC-Project-Note-78/97; CERN: Geneva, Switzerland, 1997. [Google Scholar]
- Benedict, L.X.; Driver, K.P.; Hamel, S.; Militzer, B.; Qi, T.; Correa, A.A.; Saul, A.; Schwegler, E. Multiphase equation of state for carbon addressing high pressures and temperatures. Phys. Rev. B 2014, 89, 224109. [Google Scholar] [CrossRef] [Green Version]
- Lazicki, A.; McGonegle, D.; Rygg, J.R.; Braun, D.; Swift, D.C.; Gorman, M.G.; Smith, R.F.; Heighway, P.; Higginbotham, A.; Suggit, M.J.; et al. Metastability of diamond ramp-compressed to 2 terapascals. Nature 2021, 589, 532–535. [Google Scholar] [CrossRef]
- Wang, Y.; Panzik, J.E.; Kiefer, B.; Lee, K.K.M. Crystal structure of graphite under room-temperature compression and decompression. Sci. Rep. 2012, 2, 520. [Google Scholar] [CrossRef]
- Németh, P.; Garvie, L.A.J.; Aoki, T.; Natalia, D.; Dubrovinsky, L.; Buseck, P.R. Lonsdaleite is faulted and twinned cubic diamond and does not exist as a discrete material. Nat. Commun. 2014, 5, 5447. [Google Scholar] [CrossRef] [Green Version]
- Salzmann, C.G.; Murray, B.J.; Shephard, J.J. Extent of stacking disorder in diamond. Diam. Relat. Mater. 2015, 59, 69–72. [Google Scholar] [CrossRef] [Green Version]
- Correa, A.A.; Bonev, S.A.; Galli, G. Carbon under extreme conditions: Phase boundaries and electronic properties from first-principles theory. Proc. Natl. Acad. Sci. USA 2006, 103, 1204–1208. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Sun, J.; Klug, D.D.; Martoňák, R. Structural transformations in carbon under extreme pressure: Beyond diamond. J. Chem. Phys. 2009, 130, 194512. [Google Scholar] [CrossRef] [PubMed]
- Ackland, G.J.; Dunuwille, M.; Martinez-Canales, M.; Loa, I.; Zhang, R.; Sinogeikin, S.; Cai, W.; Deemyad, S. Quantum and isotope effects in lithium metal. Science 2017, 356, 1254–1259. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Barrett, C.S. A low temperature transformation in lithium. Phys. Rev. 1947, 72, 245. [Google Scholar] [CrossRef]
- Overhauser, A.W. Crystal structure of lithium at 4.2 K. Phys. Rev. Lett. 1984, 53, 64–65. [Google Scholar] [CrossRef]
- Smith, H.G. Martensitic phase transformation of single-crystal lithium from bcc to a 9R-related structure. Phys. Rev. Lett. 1987, 58, 1228–1231. [Google Scholar] [CrossRef]
- Scelta, D.; Baldassarre, A.; Serrano-Ruiz, M.; Dziubek, K.; Cairns, A.B.; Peruzzini, M.; Bini, R.; Ceppatelli, M. Interlayer bond formation in black phosphorus at high pressure. Angew. Chem. Int. Ed. Engl. 2017, 56, 14135–14140. [Google Scholar] [CrossRef]
- Bridgman, P.W. Two new modifications of phosphorus. J. Am. Chem. Soc. 1914, 36, 1344–1363. [Google Scholar] [CrossRef] [Green Version]
- Henry, L.; Svitlyk, V.; Mezouar, M.; Sifré, D.; Garbarino, G.; Ceppatelli, M.; Serrano-Ruiz, M.; Peruzzini, M.; Datchi, F. Anisotropic thermal expansion of black phosphorus from nanoscale dynamics of phosphorene layers. Nanoscale 2020, 12, 4491–4497. [Google Scholar] [CrossRef]
- Zhao, J.-J.; Scandolo, S.; Kohanoff, J.; Chiarotti, G.L.; Tosatti, E. Elasticity and mechanical instabilities of diamond at megabar stresses: Implications for diamond-anvil-cell research. Appl. Phys. Lett. 1999, 75, 487–488. [Google Scholar] [CrossRef]
- Levitas, V.I. High pressure phase transformations revisited. J. Phys. Condens. Matter 2018, 30, 163001. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Boehler, R.; von Bargen, N.; Chopelas, A. Melting, thermal expansion, and phase transitions of iron at high pressures. J. Geophys. Res. 1990, 95, 21731–21736. [Google Scholar] [CrossRef]
- Errandonea, D.; Meng, Y.; Somayazulu, M.; Häusermann, D. Pressure-induced α→ω transition in titanium metal: A systematic study of the effects of uniaxial stress. Phys. B Condens. Matter 2005, 355, 116–125. [Google Scholar] [CrossRef] [Green Version]
- Santoro, M.; Gorelli, F.A.; Bini, R.; Haines, J.; Cambon, O.; Levelut, C.; Montoya, J.A.; Scandolo, S. Partially collapsed cristobalite structure in the non molecular phase V in CO2. Proc. Natl. Acad. Sci. USA 2012, 109, 5176–5179. [Google Scholar] [CrossRef] [Green Version]
- Datchi, F.; Mallick, B.; Salamat, A.; Ninet, S. Structure of polymeric carbon dioxide CO2-V. Phys. Rev. Lett. 2012, 108, 125701. [Google Scholar] [CrossRef]
- Iota, V.; Yoo, C.-S.; Klepeis, J.-H.; Jenei, Z.; Evans, W.; Cynn, H. Six-fold coordinated carbon dioxide VI. Nat. Mater. 2007, 6, 34–38. [Google Scholar] [CrossRef]
- Yoo, C.-S.; Sengupta, A.; Kim, M. Carbon dioxide carbonates in the Earth’s mantle: Implications to the deep carbon cycle. Angew. Chem. Int. Ed. 2011, 50, 11219–11222. [Google Scholar] [CrossRef]
- Dziubek, K.F.; Ende, M.; Scelta, D.; Bini, R.; Mezouar, M.; Garbarino, G.; Miletich, R. Crystalline polymeric carbon dioxide stable at megabar pressures. Nat. Comm. 2018, 9, 3148. [Google Scholar] [CrossRef]
- Scelta, D.; Dziubek, K.F.; Ende, M.; Miletich, R.; Mezouar, M.; Garbarino, G.; Bini, R. Extending the stability field of polymeric carbon dioxide phase V beyond the Earth’s geotherm. Phys. Rev. Lett. 2021, 126, 065701. [Google Scholar] [CrossRef]
- Tschauner, O.; Mao, H.-k.; Hemley, R.J. New transformations of CO2 at high pressures and temperatures. Phys. Rev. Lett. 2001, 87, 075701. [Google Scholar] [CrossRef]
- Seto, Y.; Hamane, D.; Nagai, T.; Fujino, K. Fate of carbonates within oceanic plates subducted to the lower mantle, and a possible mechanism of diamond formation. Phys. Chem. Miner. 2008, 35, 223–229. [Google Scholar] [CrossRef]
- Litasov, K.D.; Goncharov, A.F.; Hemley, R.J. Crossover from melting to dissociation of CO2 under pressure: Implications for the lower mantle. Earth Planet. Sci. Lett. 2011, 309, 318–323. [Google Scholar] [CrossRef]
- Giordano, V.M.; Datchi, F.; Dewaele, A. Melting curve and fluid equation of state of carbon dioxide at high pressure and high temperature. J. Chem. Phys. 2006, 125, 054504. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Giordano, V.M.; Datchi, F. Molecular carbon dioxide at high pressure and high temperature. EPL 2007, 77, 46002. [Google Scholar] [CrossRef]
- Iota, V.; Yoo, C.S. Phase diagram of carbon dioxide: Evidence for a new associated phase. Phys. Rev. Lett. 2001, 86, 5922–5925. [Google Scholar] [CrossRef]
- Cogollo-Olivo, B.H.; Biswas, S.; Scandolo, S.; Montoya, J.A. Ab initio determination of the phase diagram of CO2 at high pressures and temperatures. Phys. Rev. Lett. 2020, 124, 095701. [Google Scholar] [CrossRef] [Green Version]
- Teweldeberhan, A.M.; Boates, B.; Bonev, S.A. CO2 in the mantle: Melting and solid-solid phase boundaries. Earth Planet. Sci. Lett. 2013, 373, 228–232. [Google Scholar] [CrossRef] [Green Version]
- Datchi, F.; Weck, G. X-ray crystallography of simple molecular solids up to megabar pressures: Application to solid oxygen and carbon dioxide. Z. Kristallogr. 2014, 229, 135–157. [Google Scholar] [CrossRef] [Green Version]
- Zhu, Q.; Oganov, A.R.; Glass, C.W.; Stokes, H.T. Constrained evolutionary algorithm for structure prediction of molecular crystals: Methodology and applications. Acta Crystallogr. B 2012, 68, 215–226. [Google Scholar] [CrossRef]
- Smith, S.J.; Montgomery, J.M.; Vohra, Y.K. High-pressure high-temperature phase diagram of organic crystal paracetamol. J. Phys. Condens. Matter 2016, 28, 035101. [Google Scholar] [CrossRef]
- Brazhkin, V.V. Metastable phases and ‘metastable’ phase diagrams. J. Phys. Condens. Matter 2006, 18, 9643–9650. [Google Scholar] [CrossRef]
- Ciabini, L.; Santoro, M.; Gorelli, F.A.; Bini, R.; Schettino, V.; Raugei, S. Triggering dynamics of the high-pressure benzene amorphization. Nat. Mater. 2006, 6, 39–43. [Google Scholar] [CrossRef] [PubMed]
- Dziubek, K.; Citroni, M.; Fanetti, S.; Cairns, A.B.; Bini, R. Synthesis of high-quality crystalline carbon nitride oxide by selectively driving the high-temperature instability of urea with pressure. J. Phys. Chem. C 2017, 121, 19872–19879. [Google Scholar] [CrossRef] [Green Version]
- Zakharov, B.A.; Seryotkin, Y.V.; Tumanov, N.A.; Paliwoda, D.; Hanfland, M.; Kurnosov, A.V.; Boldyreva, E.V. The role of fluids in high-pressure polymorphism of drugs: Different behaviour of β-chlorpropamide in different inert gas and liquid media. RSC Adv. 2016, 6, 92629–92637. [Google Scholar] [CrossRef] [Green Version]
- Guńka, P.A.; Olejniczak, A.; Fanetti, S.; Bini, R.; Collings, I.E.; Svitlyk, V.; Dziubek, K.F. Crystal structure and non-hydrostatic stress-induced phase transition of urotropine under high pressure. Chem. Eur. J. 2021, 27, 1094–1102. [Google Scholar] [CrossRef]
- Amsler, M.; Hegde, V.I.; Jacobsen, S.D.; Wolverton, C. Exploring the high-pressure materials genome. Phys. Rev. X 2018, 8, 041021. [Google Scholar] [CrossRef] [Green Version]
- Hilleke, K.P.; Bi, T.; Zurek, E. Materials under high pressure: A chemical perspective. Appl. Phys. A 2022, 128, 441. [Google Scholar] [CrossRef]
- Conway, L.J.; Pickard, C.J.; Hermann, A. Rules of formation of H–C–N–O compounds at high pressure and the fates of planetary ices. Proc. Natl. Acad. Sci. USA 2021, 118, e2026360118. [Google Scholar] [CrossRef]
- Machon, D.; Meersman, F.; Wilding, M.C.; Wilson, M.; McMillan, P.F. Pressure-induced amorphization and polyamorphism: Inorganic and biochemical systems. Prog. Mater. Sci. 2014, 61, 216–282. [Google Scholar] [CrossRef]
- McMillan, P.F.; Wilding, M.C. Polyamorphism and liquid–liquid phase transitions. In Encyclopedia of Glass Science, Technology, History, and Culture, 1st ed.; Richet, P., Ed.; Wiley: Hoboken, NJ, USA, 2021; Volume 1, pp. 359–370. [Google Scholar]
- Santoro, M.; Gorelli, F.A.; Bini, R.; Ruocco, G.; Scandolo, S.; Crichton, W.A. Amorphous silica-like carbon dioxide. Nature 2006, 441, 857–860. [Google Scholar] [CrossRef]
- Tulk, C.A.; Molaison, J.J.; Makhluf, A.R.; Manning, C.E.; Klug, D.D. Absence of amorphous forms when ice is compressed at low temperature. Nature 2019, 569, 542–545. [Google Scholar] [CrossRef] [PubMed]
- Amann-Winkel, K.; Böhmer, R.; Fujara, F.; Gainaru, C.; Geil, B.; Loerting, T. Colloquium: Water’s controversial glass transitions. Rev. Mod. Phys. 2016, 88, 011002. [Google Scholar] [CrossRef]
- Katayama, Y.; Inamura, Y.; Mizutani, T.; Yamakata, M.; Utsumi, W.; Shimomura, O. Macroscopic separation of dense fluid phase and liquid phase of phosphorus. Science 2004, 306, 848–851. [Google Scholar] [CrossRef]
- Henry, L.; Mezouar, M.; Garbarino, G.; Sifré, D.; Weck, G.; Datchi, F. Liquid–liquid transition and critical point in sulfur. Nature 2020, 584, 382–386. [Google Scholar] [CrossRef] [PubMed]
- Simeoni, G.G.; Bryk, T.; Gorelli, F.A.; Krisch, M.; Ruocco, G.; Santoro, M.; Scopigno, T. The Widom line as the crossover between liquid-like and gas-like behaviour in supercritical fluids. Nat. Phys. 2010, 6, 503–507. [Google Scholar] [CrossRef]
- Bridgman, P.W. The compressibility and pressure coefficient of resistance of ten elements. Proc. Am. Acad. Arts. Sci. 1927, 62, 207–226. [Google Scholar] [CrossRef]
- Decremps, F.; Belhadi, L.; Farber, D.L.; Moore, K.T.; Occelli, F.; Gauthier, M.; Polian, A.; Antonangeli, D.; Aracne-Ruddle, C.M.; Amadon, B. Diffusionless γ⇄α phase transition in polycrystalline and single-crystal cerium. Phys. Rev. Lett. 2011, 106, 065701. [Google Scholar] [CrossRef] [Green Version]
- Devaux, N.; Casula, M.; Decremps, F.; Sorella, S. Electronic origin of the volume collapse in cerium. Phys. Rev. B 2015, 91, 081101. [Google Scholar] [CrossRef] [Green Version]
- Lipp, M.J.; Jackson, D.; Cynn, H.; Aracne, C.; Evans, W.J.; McMahan, A.K. Thermal signatures of the Kondo volume collapse in cerium. Phys. Rev. Lett. 2008, 101, 165703. [Google Scholar] [CrossRef]
- Lipp, M.J.; Jenei, Z.; Ruddle, D.; Aracne-Ruddle, C.; Cynn, H.; Evans, W.J.; Kono, Y.; Kenney-Benson, C.; Park, C. Equation of state measurements by radiography provide evidence for a liquid-liquid phase transition in cerium. J. Phys. Conf. Ser. 2014, 500, 032011. [Google Scholar] [CrossRef] [Green Version]
- Munro, K.A.; Daisenberger, D.; MacLeod, S.G.; McGuire, S.; Loa, I.; Popescu, C.; Botella, P.; Errandonea, D.; McMahon, M.I. The high-pressure, high-temperature phase diagram of cerium. J. Phys. Condens. Matter 2020, 32, 335401. [Google Scholar] [CrossRef] [PubMed]
- McBride, E.E.; Krygier, A.; Ehnes, A.; Galtier, E.; Harmand, M.; Konôpková, Z.; Lee, H.J.; Liermann, H.-P.; Nagler, B.; Pelka, A.; et al. Phase transition lowering in dynamically compressed silicon. Nat. Phys. 2019, 15, 89–94. [Google Scholar] [CrossRef]
- Coleman, A.L.; Gorman, M.G.; Briggs, R.; McWilliams, R.S.; McGonegle, D.; Bolme, C.A.; Gleason, A.E.; Fratanduono, D.E.; Smith, R.F.; Galtier, E.; et al. Identification of phase transitions and metastability in dynamically compressed antimony using ultrafast X-ray diffraction. Phys. Rev. Lett. 2019, 122, 255704. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Husband, R.J.; O’Bannon, E.F.; Liermann, H.-P.; Lipp, M.J.; Méndez, A.S.J.; Konôpková, Z.; McBride, E.E.; Evans, W.J.; Jenei, Z. Compression-rate dependence of pressure-induced phase transitions in Bi. Sci. Rep. 2021, 11, 14859. [Google Scholar] [CrossRef] [PubMed]
- Fisch, M.; Lanza, A.; Boldyreva, E.; Macchi, P.; Casati, N. Kinetic control of high-pressure solid-state phase transitions: A Case Study on L-serine. J. Phys. Chem. C 2015, 119, 18611–18617. [Google Scholar] [CrossRef] [Green Version]
- Gramsch, S.A. A closer look at phase diagrams for the general chemistry course. J. Chem. Ed. 2000, 77, 718–723. [Google Scholar] [CrossRef]
- International Association for the Advancement of High Pressure Science and Technology (Association Internationale pour l’Avancement de la Recherche et de la Technologie aux Hautes Pressions). Available online: https://www.airapt.org (accessed on 20 July 2022).

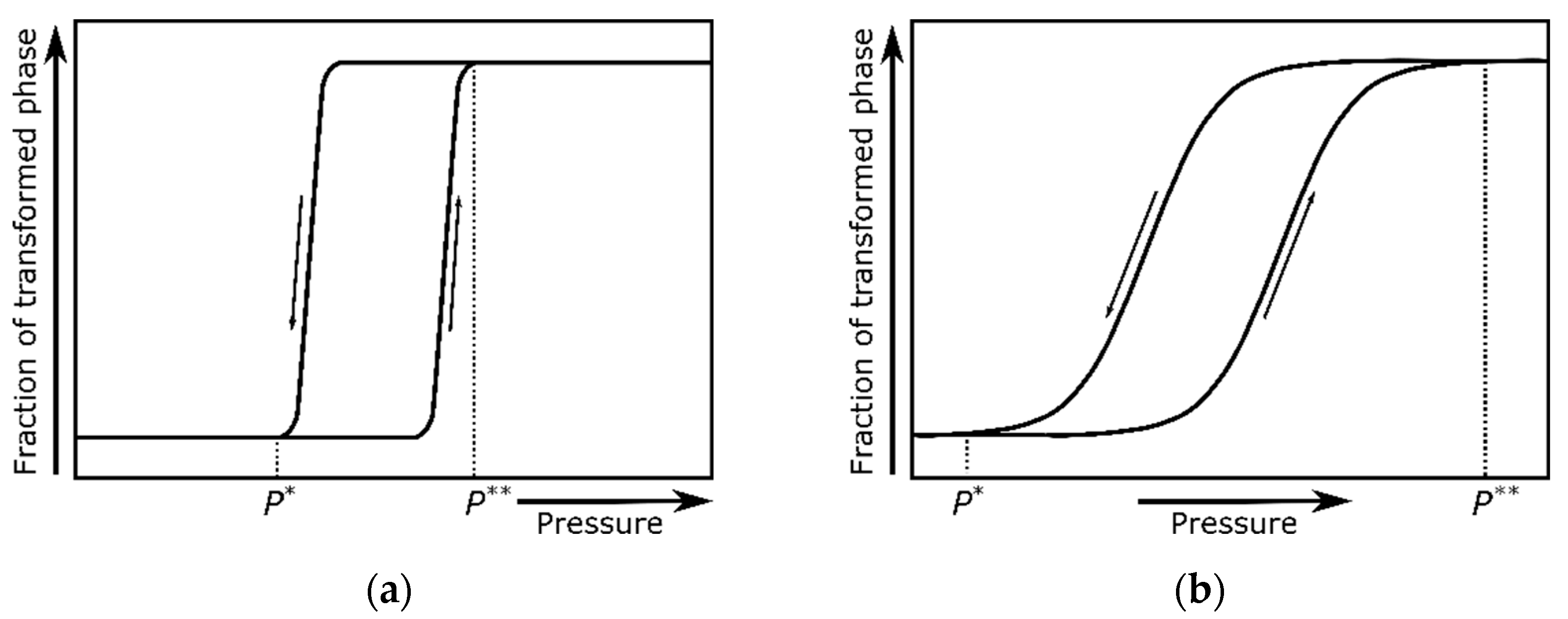

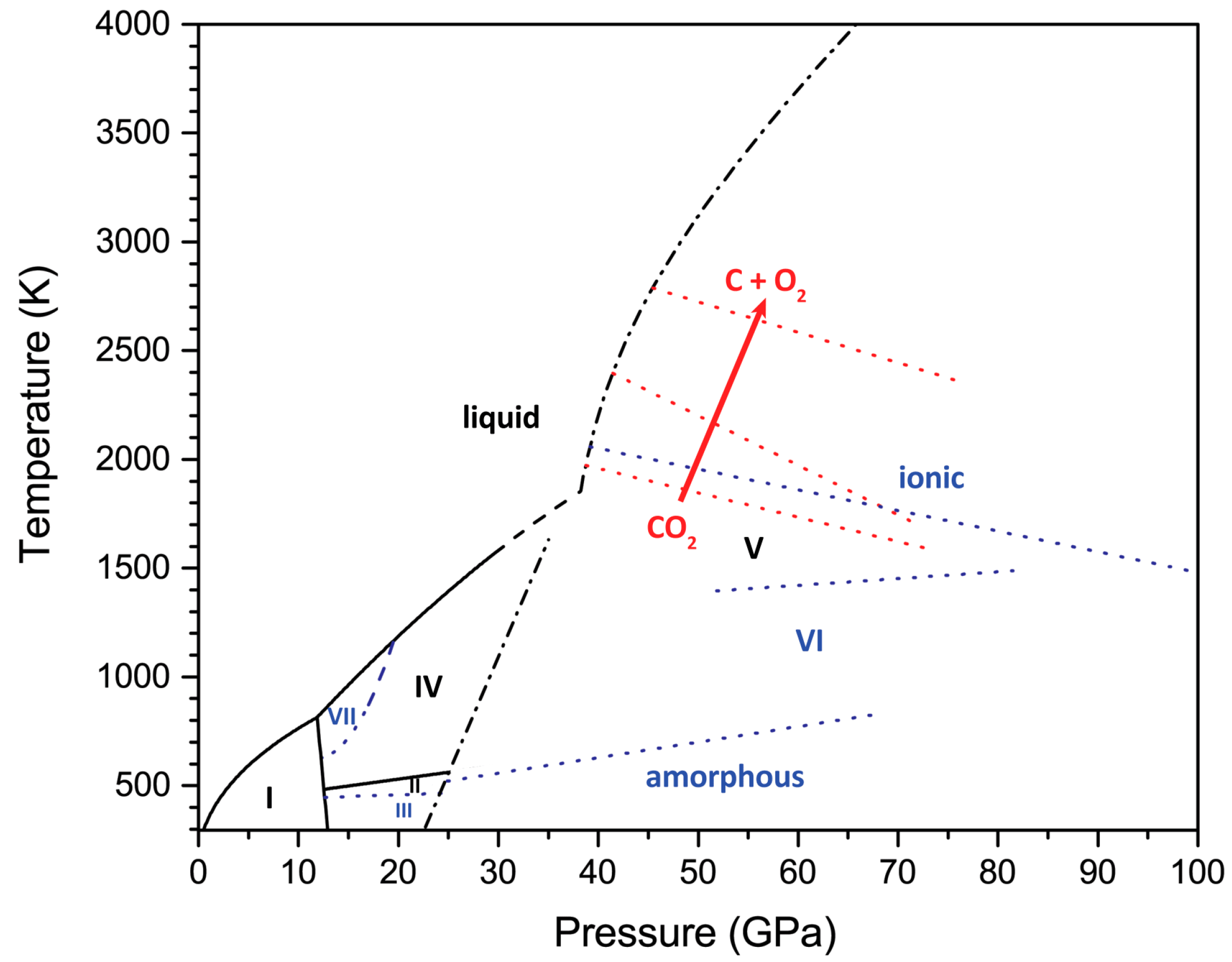
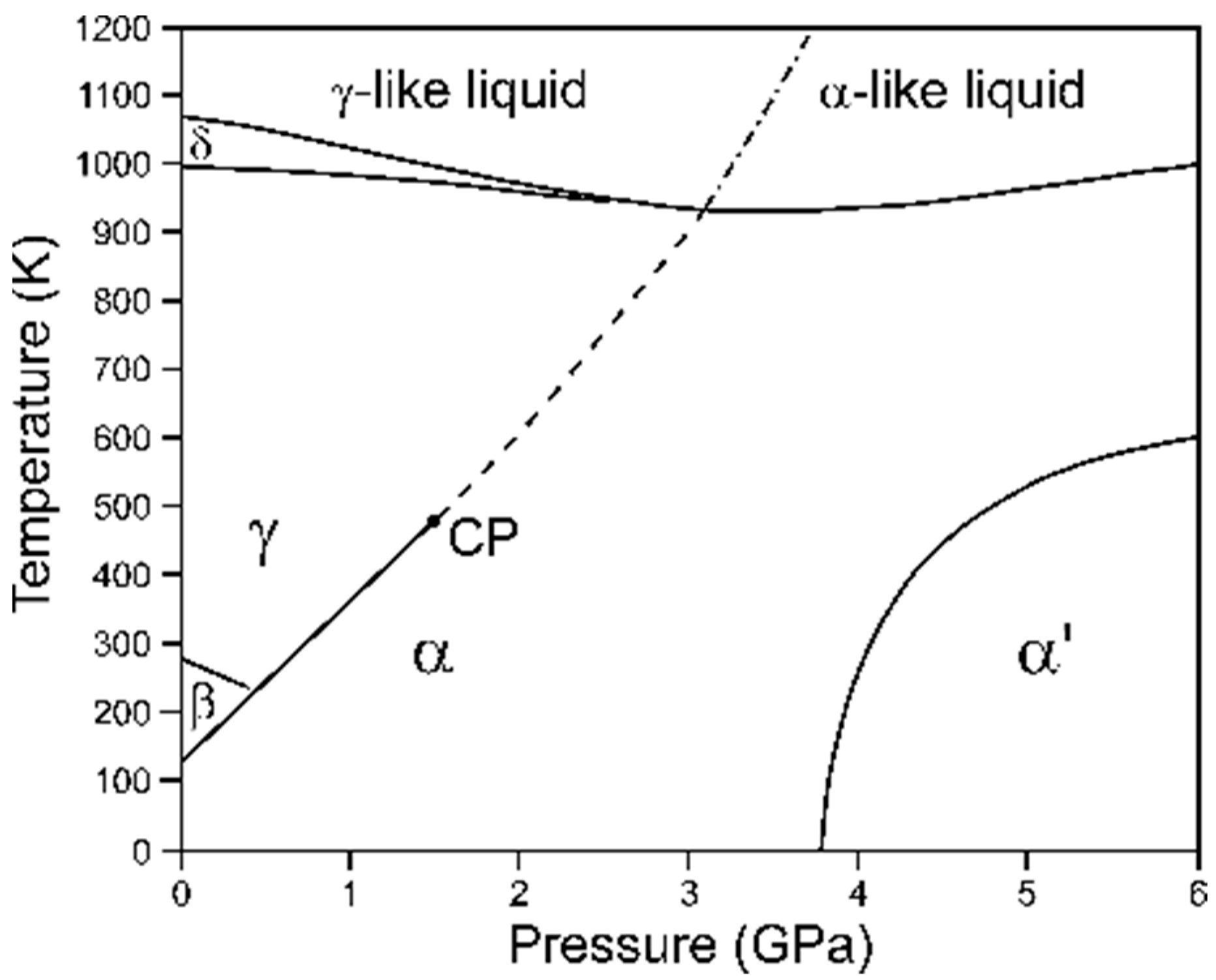
| Issues | Potential Solutions |
|---|---|
| The definition of an ideal phase diagram is quite limited to the elements and simplest chemical compounds. P–T plots of more complex systems, often reported as phase diagrams, are transitional P–T diagrams or “metastable phase diagrams.” Even when regarding only chemical elements, one should be extremely careful with wording 1. | It should be acknowledged that a presented phase diagram is the best estimate of the ideal phase diagram based on experimental and theoretical constraints. At the base of the wording phase diagram lies the assumption that: (1) the presented states are thermodynamically stable phases; (2) the described situation is as close to the thermodynamic equilibrium as possible. |
| There is no harm in presenting nonequilibrium P–T diagrams if only experimental conditions are described in detail. Reporting nonequilibrium states (in particular, amorphous glasses) incorporated within an equilibrium phase diagram may be utterly confusing when not described appropriately. | Transitional P–T diagrams that include nonequilibrium states can be reported only if additional information is indicated and commented on in the legend and caption. The careful description is substantial, especially to distinguish between the thermodynamically stable phases and kinetically trapped metastable states. In such plots, the position of the kinetic transition lines may strongly depend on the experimental conditions and the P–T pathway. Such P–T diagrams should not be referred to as phase diagrams. |
| Undoubtedly, discovering new stable phases is of paramount importance in condensed-matter chemistry and physics. However, some literature P–T plots report numerous “phases” that appear to be metastable states after a more thorough investigation. Articles containing the catchphrase “we revised the phase diagram of…” make little sense if the authors of previous studies understand the term differently. To paraphrase Occam’s razor, supposedly stable phases should not be multiplied beyond necessity. | The reported phases should conform to the definition of a thermodynamically stable phase. Under no circumstances can a claim of the revision of a phase diagram be accepted if the reported states are metastable. |
| Some specific boundary lines (such as liquid–liquid-transition curves and Widom lines) and critical points may appear in a phase diagram or transitional P–T diagram. It is essential, however, to characterize them well in the plot or figure caption. | All the elements of the reported diagrams should be communicated in a clear and unambiguous fashion. |
| Dynamic P–T diagrams (based on the results of compression experiments) do not comply with the phase-diagram definition, as they do not correspond to an equilibrium state. The compression rate can shift phase boundaries and, as such, may be regarded as an additional variable, but one has to bear in mind that many other factors can also influence the boundary lines under nonequilibrium conditions. Having said that, an ideally static P–T diagram does not exist, as any compression and decompression is a time-dependent process. The crucial factor is, therefore, the ratio between the rate of transformation and the rate of pressurization or depressurization. | The terms dynamic P–T diagram and transitional P–T diagrams are almost synonymous; however, the second one is used more often to report the results of quasistatic compression experiments. As there is no universally accepted compression-rate threshold that unequivocally distinguishes these two notions, the term dynamic P–T diagram appears to be redundant. |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the author. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Dziubek, K.F. On the Definition of Phase Diagram. Crystals 2022, 12, 1186. https://doi.org/10.3390/cryst12091186
Dziubek KF. On the Definition of Phase Diagram. Crystals. 2022; 12(9):1186. https://doi.org/10.3390/cryst12091186
Chicago/Turabian StyleDziubek, Kamil Filip. 2022. "On the Definition of Phase Diagram" Crystals 12, no. 9: 1186. https://doi.org/10.3390/cryst12091186
APA StyleDziubek, K. F. (2022). On the Definition of Phase Diagram. Crystals, 12(9), 1186. https://doi.org/10.3390/cryst12091186






