Heterogeneity in La Distribution in Highly La-Doped SrTiO3 Crystals
Abstract
:1. Introduction
2. Point Defect Chemistry of La-Doped SrTiO3
3. X-ray Diffraction Measurements and Density Determination
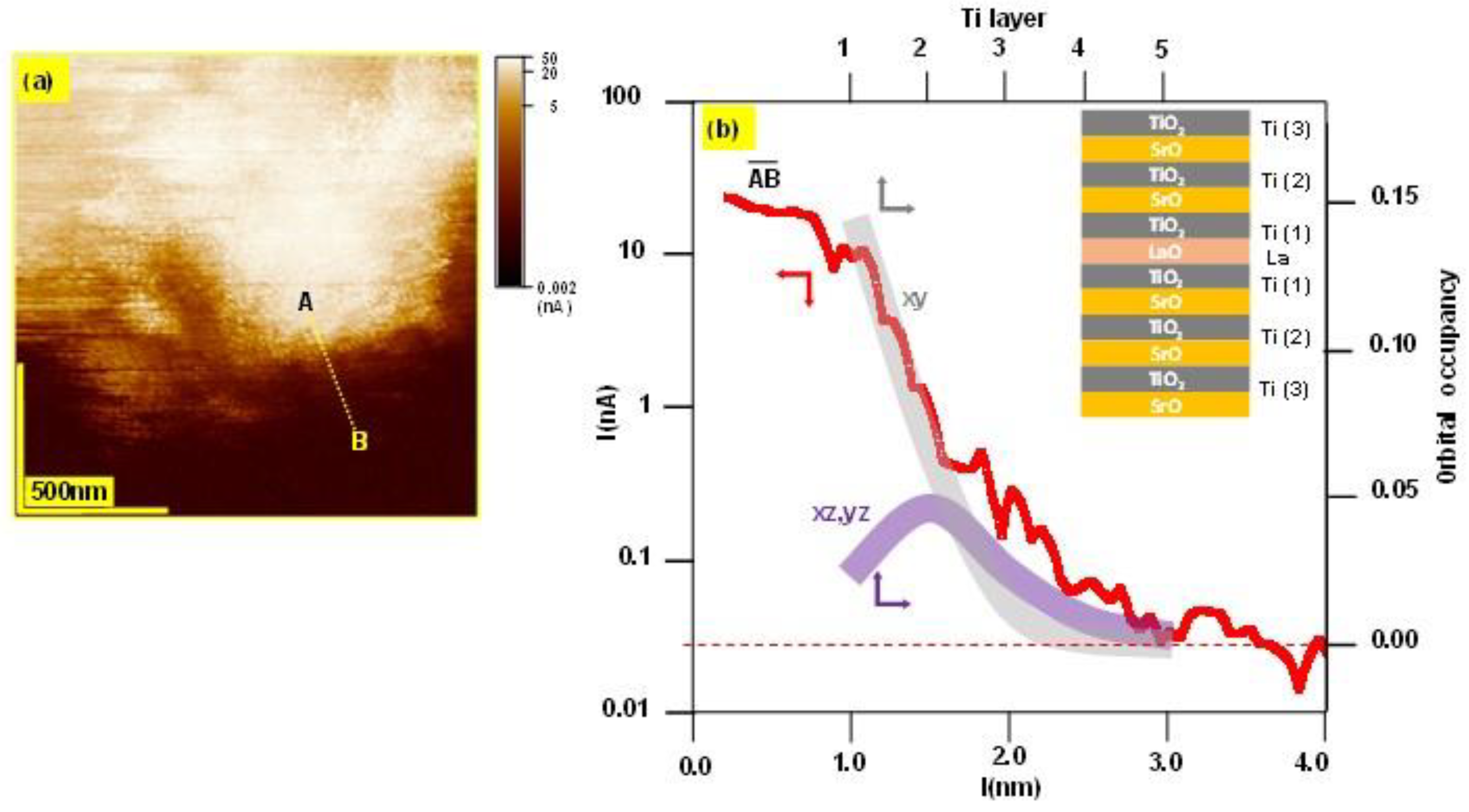
4. TEM, EELS, EDX, and SAD Analysis
5. Optical Inspections and Morphological Analysis of Cleaved Plane
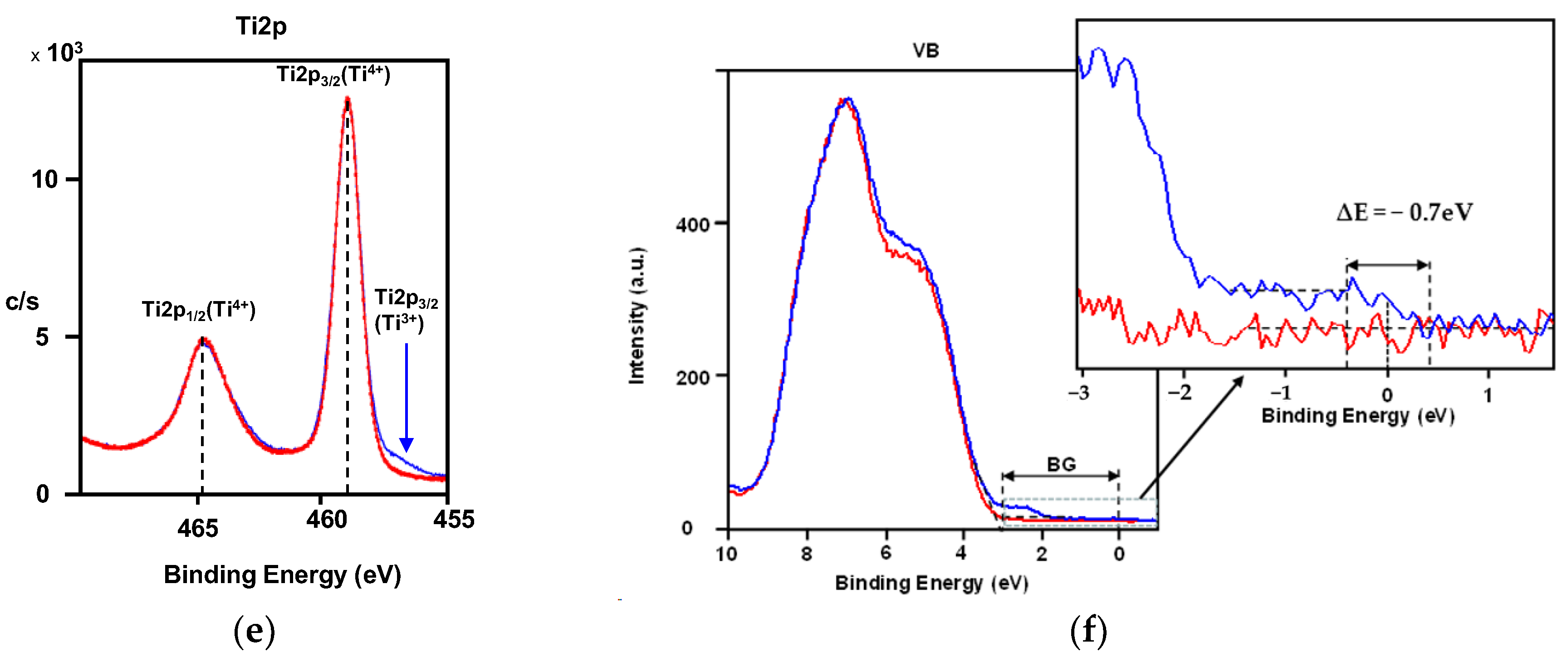
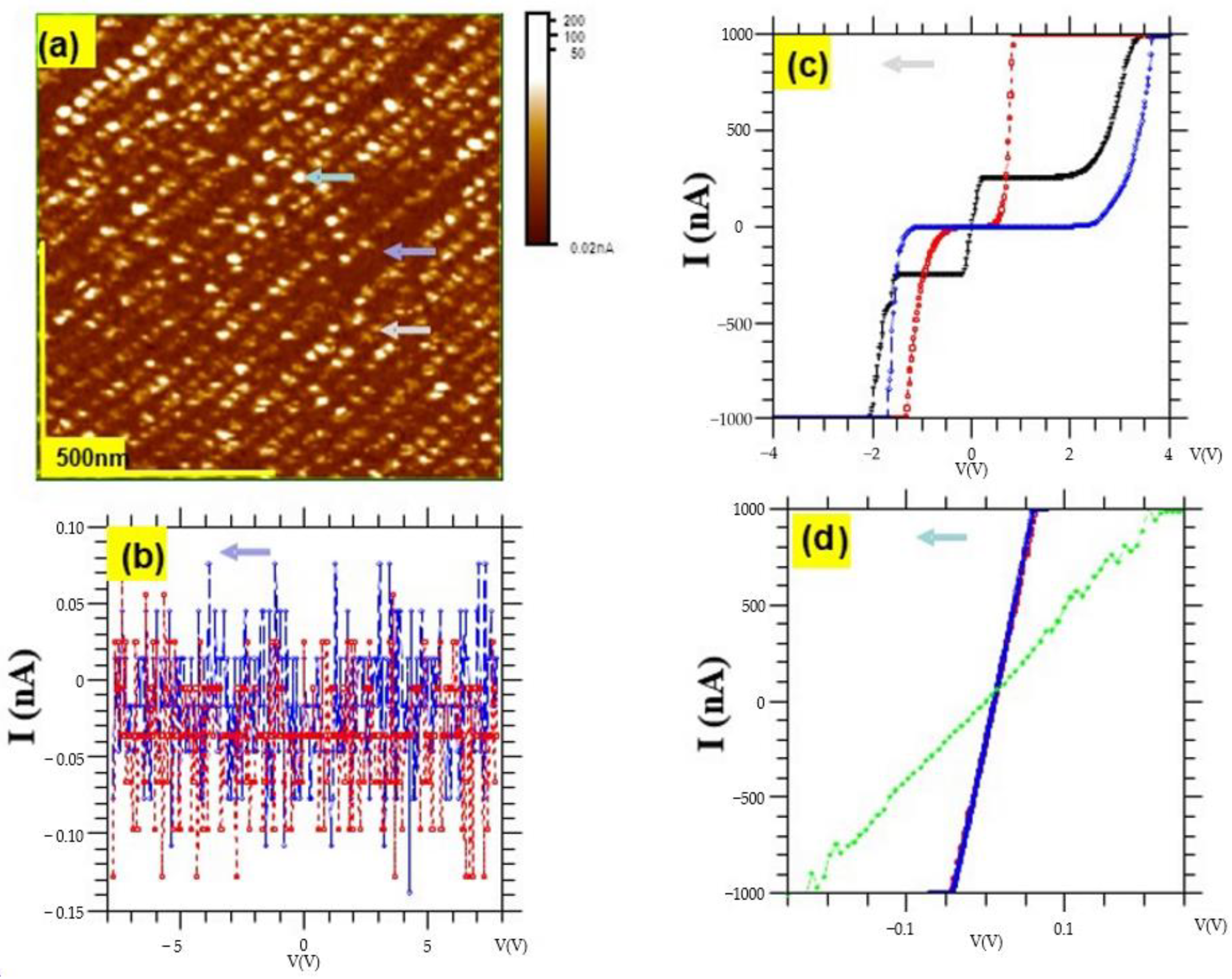
6. Surface-Sensitive Studies of the Electronic Structure and Electrical Conductivity on the Surface of LSTO; XPS and LCAFM Investigation
7. Summary
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Yu, K.; Zhang, C.; Chang, Y.; Feng, Y.; Yang, Z.; Yang, T.; Lou, L.-L.; Liu, S. Novel three-dimensionally ordered macroporous SrTiO3 photocatalysts with remarkably enhanced hydrogen production performance. Appl. Catal. B Environ. 2017, 200, 514–520. [Google Scholar] [CrossRef]
- Su, K.; Liu, X.; Zhang, T.; Li, J.; Luo, F.; Yuan, C.; Liu, F. Effect of Cr and La co-doping on the photocatalytic hydrogen production performance of Sr1-xLaxTi1-xCrxO3 nanofibers. Catal. Commun. 2023, 175, 106601. [Google Scholar] [CrossRef]
- Sikam, P.; Thirayatorn, R.; Kaewmaraya, T.; Thongbai, P.; Moontragoon, P.; Ikonic, Z. Improved Thermoelectric Properties of SrTiO3 via (La, Dy and N) Co-Doping: DFT Approach. Molecules 2022, 27, 7923. [Google Scholar] [CrossRef]
- Moos, R.; Härdtl, K.H. Electronic transport properties of Sr1−xLaxTiO3 ceramics. J. Appl. Phys. 1996, 80, 393–400. [Google Scholar] [CrossRef]
- Moos, R. Donatordotierungen im Strontiumtitanat: Elektrische Eigenschaften und modellhafte Beschreibung. Ph.D. Thesis, VDI GmbH, Alexisbad, Germany, 1994. (In German). [Google Scholar]
- Moos, R.; Bishoff, T.; Menesklu, W.; Hardtl, K.H. Solubility of lanthanum in strontium titanate in oxygen-rich atmospheres. J. Mater. Sci. 1997, 32, 4247–4252. [Google Scholar] [CrossRef]
- Menesklou, W. Kompensationsmechanismen der Überschlußladung im lanthandotiertem Barium- und Strontiumtitanat. Ph.D. Thesis, VDI GmbH, Alexisbad, Germany, 1997. (In German). [Google Scholar]
- Canales-Vázquez, J.; Smith, M.J.; Irvine, J.T.; Zhou, W. Studies on the Reorganization of Extended Defects with Increasing n in the Perovskite-Based La4Srn–4TinO3n+2 Series. Adv. Funct. Mater. 2005, 15, 1000–1008. [Google Scholar] [CrossRef]
- Battle, P.D.; Bennett, J.E.; Sloan, J.; Tilley, R.J.D.; Vente, J.F. A-site cation-vacancy ordering in Sr1−3x/2LaxTiO3: A study by HRTEM. J. Solid State Chem. 2000, 149, 360–369. [Google Scholar] [CrossRef]
- Ruiz-Morales, J.; Canales-Vázquez, J.; Savaniu, C.; Marrero-López, D.; Zhou, W.; Irvine, J.T. Disruption of extended defects in solid oxide fuel cell anodes for methane oxidation. Nature 2006, 439, 568–571. [Google Scholar] [CrossRef]
- Pilch, M. Rola Domieszki La w Monokryształach SrTiO3. Ph.D. Thesis, University of Silesia, Katowice, Poland, 2010. (In Polish). [Google Scholar]
- Kobayashi, S.; Ikuhara, Y.; Mizoguchi, T. Lattice expansion and local lattice distortion in Nb-and La-doped SrTiO3 single crystals investigated by x-ray diffraction and first-principles calculations. Phys. Rev. B 2018, 98, 134114. [Google Scholar] [CrossRef]
- Wang, Z.; Okude, M.; Saito, M.; Tsukimoto, S.; Ohtomo, A.; Tsukada, M.; Kawasaki, M.; Ikuhara, Y. Dimensionality-driven insulator—Metal transition in A-site excess non-stoichiometric perovskites. Nat. Commun. 2010, 1, 106. [Google Scholar] [CrossRef]
- Bäurer, M. Kornwachstum in Strontiumtitanat Karlsruhe. Ph.D. Thesis, Institut für Keramik im, Maschinenbau Universität Karlsruhe (TH), Berlin, Germany, 2009. [Google Scholar]
- Pilch, M.; Szot, K. Resistive Switching in Sr1−0.05La0.05TiO3. In Proceedings of the ISAF-ECAPD-PFM 2012, Aveiro, Portugal, 9–13 July 2012; pp. 1–2. [Google Scholar]
- Rodenbücher, C.; Speier, W.; Bihlmayer, G.; Breuer, U.; Waser, R.; Krzysztof, S. Cluster-like resistive switching of SrTiO3: Nb surface layers. New J. Phys. 2013, 15, 103017. [Google Scholar] [CrossRef]
- Rodenbücher, C.; Menzel, S.; Wrana, D.; Gensch, T.; Korte, C.; Krok, F.; Szot, K. Current channeling along extended defects during electroreduction of SrTiO3. Sci. Rep. 2019, 9, 2502. [Google Scholar] [CrossRef]
- Rodenbücher, C.; Gensch, T.; Speier, W.; Breuer, U.; Pilch, M.; Hardtdegen, H.; Mikulics, M.; Zych, E.; Waser, R.; Szot, K. Inhomogeneity of donor doping in SrTiO3 substrates studied by fluorescence-lifetime imaging microscopy. Appl. Phys. Lett. 2013, 103, 162904. [Google Scholar] [CrossRef]
- Szot, K.; Speier, W.; Bihlmayer, G.; Waser, R. Switching the electrical resistance of individual dislocations in single-crystalline SrTiO3. Nat. Mater. 2006, 5, 312–320. [Google Scholar] [CrossRef] [PubMed]
- Pellegrino, L.; Bellingeri, E.; Pallecchi, I.; Siri, A.S.; Marré, D. Submicrometric SrTiO3-δ based devices realized by an atomic force microscope. Solid-State Electron. 2003, 47, 2193–2198. [Google Scholar] [CrossRef]
- Shanthi, N.; Sarma, D.D. Electronic structure of electron doped SrTiO3: SrTiO3−δ and Sr1−xLaxTiO3. Phys. Rev. B 1998, 57, 2153–2158. [Google Scholar] [CrossRef]
- Bakhshi, H.; Sarraf-Mamoory, R.; Yourdkhani, A.; Song, S.; Tseng, Y.-C.; Mozharivskyj, Y. Improvements in the thermoelectric efficiency of SrTiO3 through donor doping. Ceram. Int. 2022, 48, 5831–5839. [Google Scholar] [CrossRef]
- Guo, X.; Pu, Y.; Wang, W.; Zhang, L.; Ji, J.; Shi, R.; Shi, Y.; Yang, M.; Li, J. High insulation resistivity and ultralow dielectric loss in La-doped SrTiO3 colossal permittivity ceramics through defect chemistry optimization. ACS Sustain. Chem. Eng. 2019, 7, 13041–13052. [Google Scholar] [CrossRef]
- Marshall, M.S.J.; Newell, D.T.; Payne, D.J.; Egdell, R.G.; Castell, M.R. Atomic and electronic surface structures of dopants in oxides: STM and XPS of Nb-and La-doped SrTiO3 (001). Phys. Rev. B 2011, 83, 035410. [Google Scholar] [CrossRef]
- Matsushima, S.; Kohiki, S.; Oku, M. Effect of La Doping on the Electronic Structure of SrTiO3. J. Ceram. Soc. Jpn. 2000, 108, 518–520. [Google Scholar] [CrossRef]
- Shan, K.; Dastan, D.; Yi, Z.Z.; Mohammed, M.K.A.; Yin, X.T.; Timoumi, A.; Weidenbach, A.S. Conductivity and aging behavior of Sr (Ti0.6Fe0.4)1−xO3−δ mixed conductor materials. RSC Adv. 2023, 13, 8683–8691. [Google Scholar] [CrossRef] [PubMed]
- Larson, P.; Popović, Z.S.; Satpathy, S. Lattice relaxation effects on the interface electron states in the perovskite oxide heterostructures: LaTiO3 monolayer embedded in SrTiO3. Phys. Rev. B 2008, 77, 245122. [Google Scholar] [CrossRef]
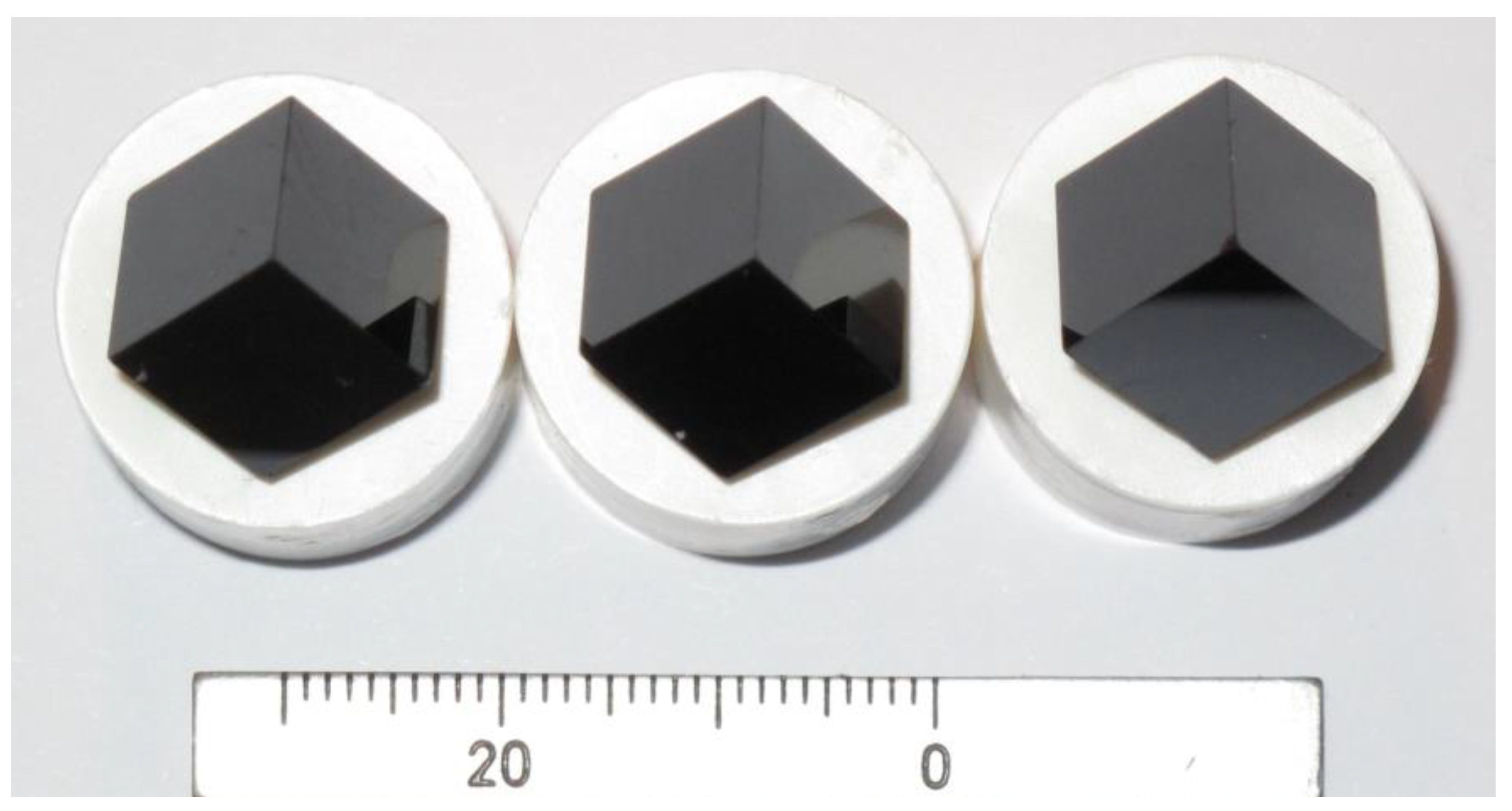


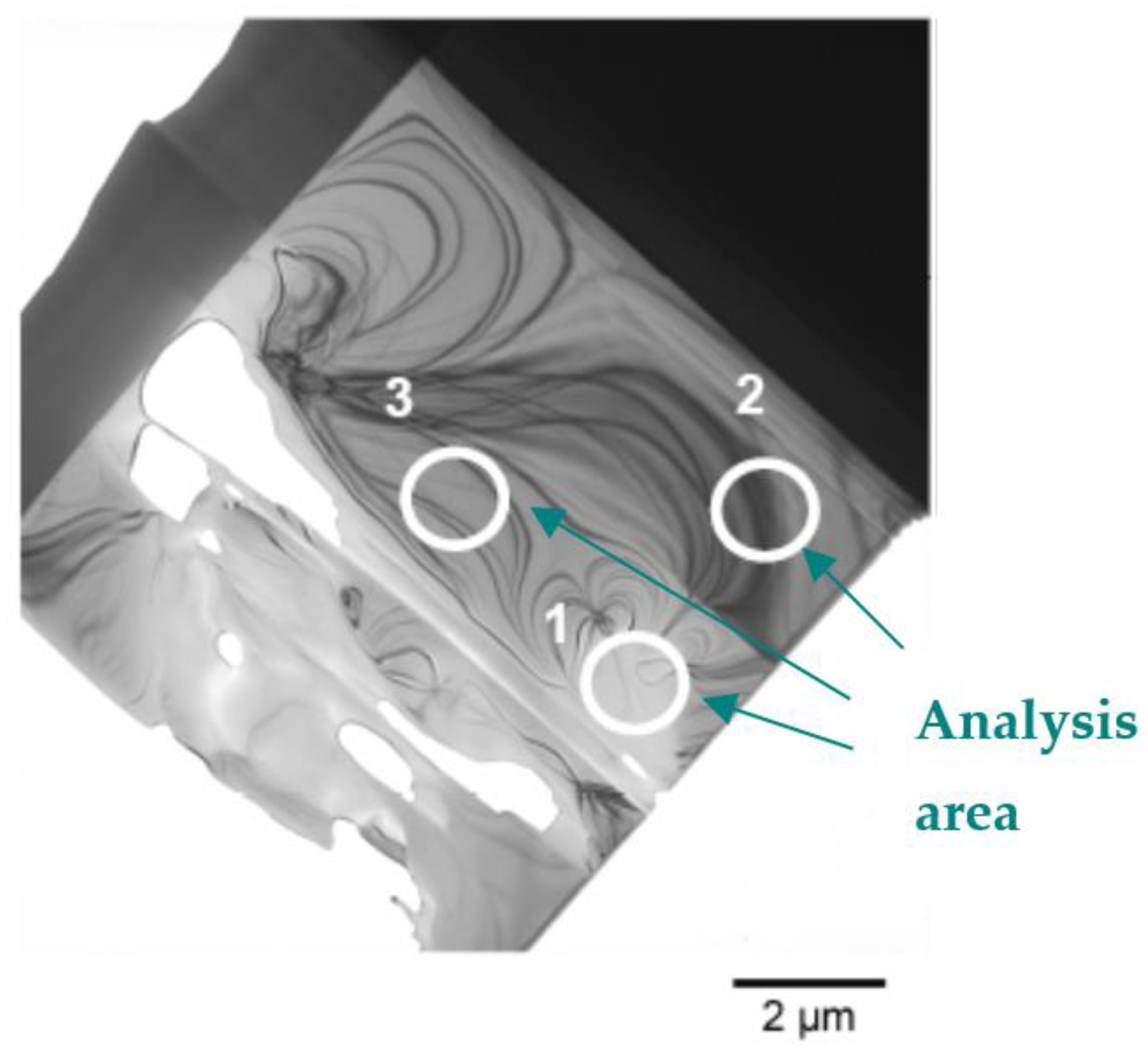

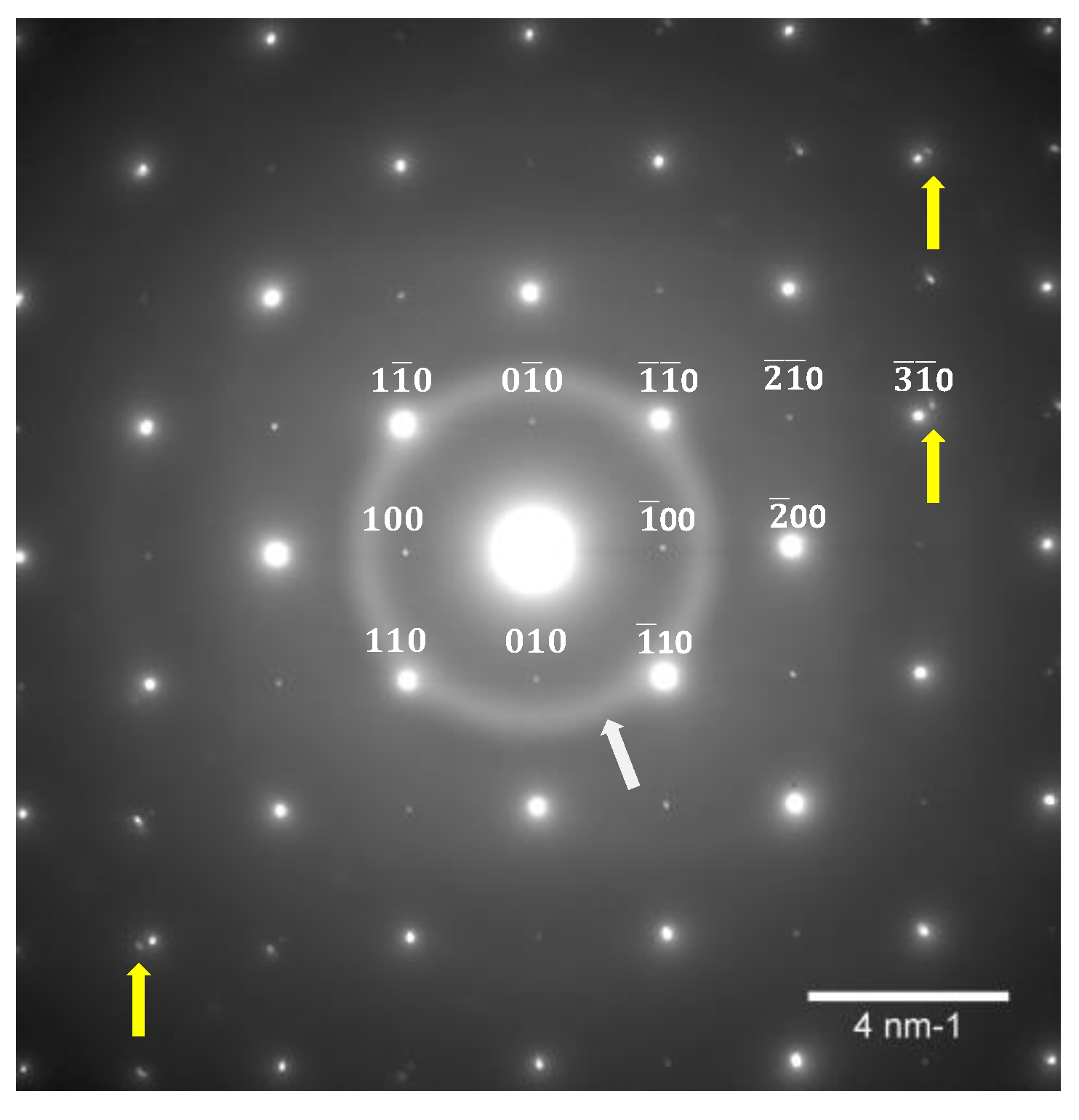


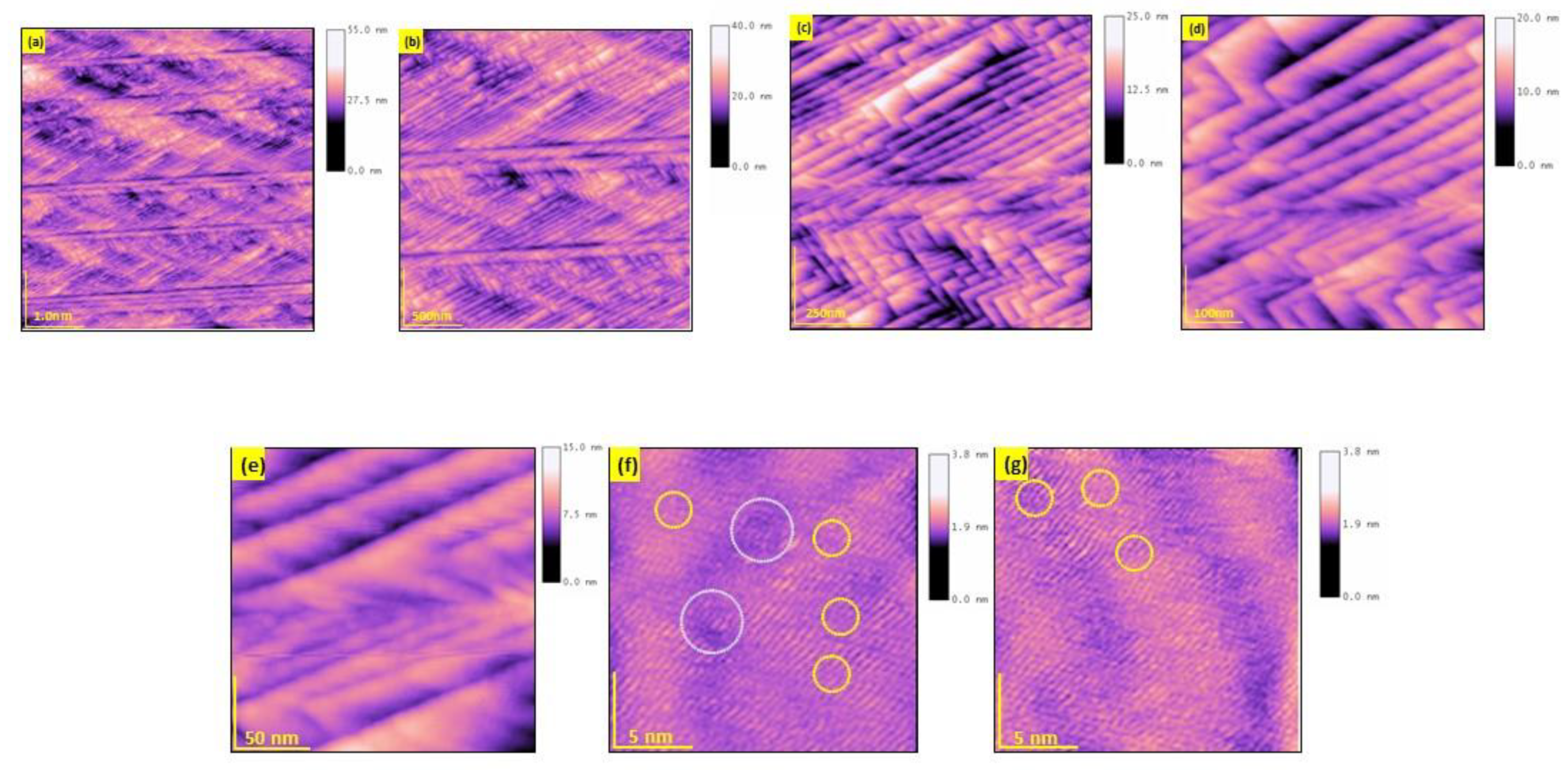



| Chemical Composition | Lattice Constant (nm) | Theoretical Density (X-ray), (g/cm3) | Experimental Density (g/cm3) |
|---|---|---|---|
| SrTiO3 | 0.39054 (±0.00002) | 5.115 (±0.002) | 5.112 (99.99%) |
| Sr0.999La0.001TiO3 | 0.39027 (±0.00001) | 5.116 (±0.001) | 5.116 (100%) |
| Sr0.99La0.01TiO3 | 0.39037 (±0.00002) | 5.137 (±0.002) | 5.128 (99.82%) |
| Sr0.95La0.05TiO3 | 0.39073 (±0.00002) | 5.235 (±0.002) | 5.180 (98.9%) |
| Element | EDX 1 | EDX 2 | EDX 3 |
|---|---|---|---|
| Ti (% at.) | 52.37 | 47.94 | 57.73 |
| Sr (% at.) | 44.93 | 49.43 | 37.17 |
| La (% at.) | 2.70 | 2.63 | 5.10 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Pilch, M.M.; Rodenbücher, C.; Krok, F.; Szot, K. Heterogeneity in La Distribution in Highly La-Doped SrTiO3 Crystals. Crystals 2023, 13, 1552. https://doi.org/10.3390/cryst13111552
Pilch MM, Rodenbücher C, Krok F, Szot K. Heterogeneity in La Distribution in Highly La-Doped SrTiO3 Crystals. Crystals. 2023; 13(11):1552. https://doi.org/10.3390/cryst13111552
Chicago/Turabian StylePilch, Michał Marek, Christian Rodenbücher, Franciszek Krok, and Kristof Szot. 2023. "Heterogeneity in La Distribution in Highly La-Doped SrTiO3 Crystals" Crystals 13, no. 11: 1552. https://doi.org/10.3390/cryst13111552
APA StylePilch, M. M., Rodenbücher, C., Krok, F., & Szot, K. (2023). Heterogeneity in La Distribution in Highly La-Doped SrTiO3 Crystals. Crystals, 13(11), 1552. https://doi.org/10.3390/cryst13111552





