Effect of Grain-Size in Nanocrystalline Tungsten on Hardness and Dislocation Density: A Molecular Dynamics Study
Abstract
1. Introduction
2. Materials and Methods
2.1. Interatomic Potential
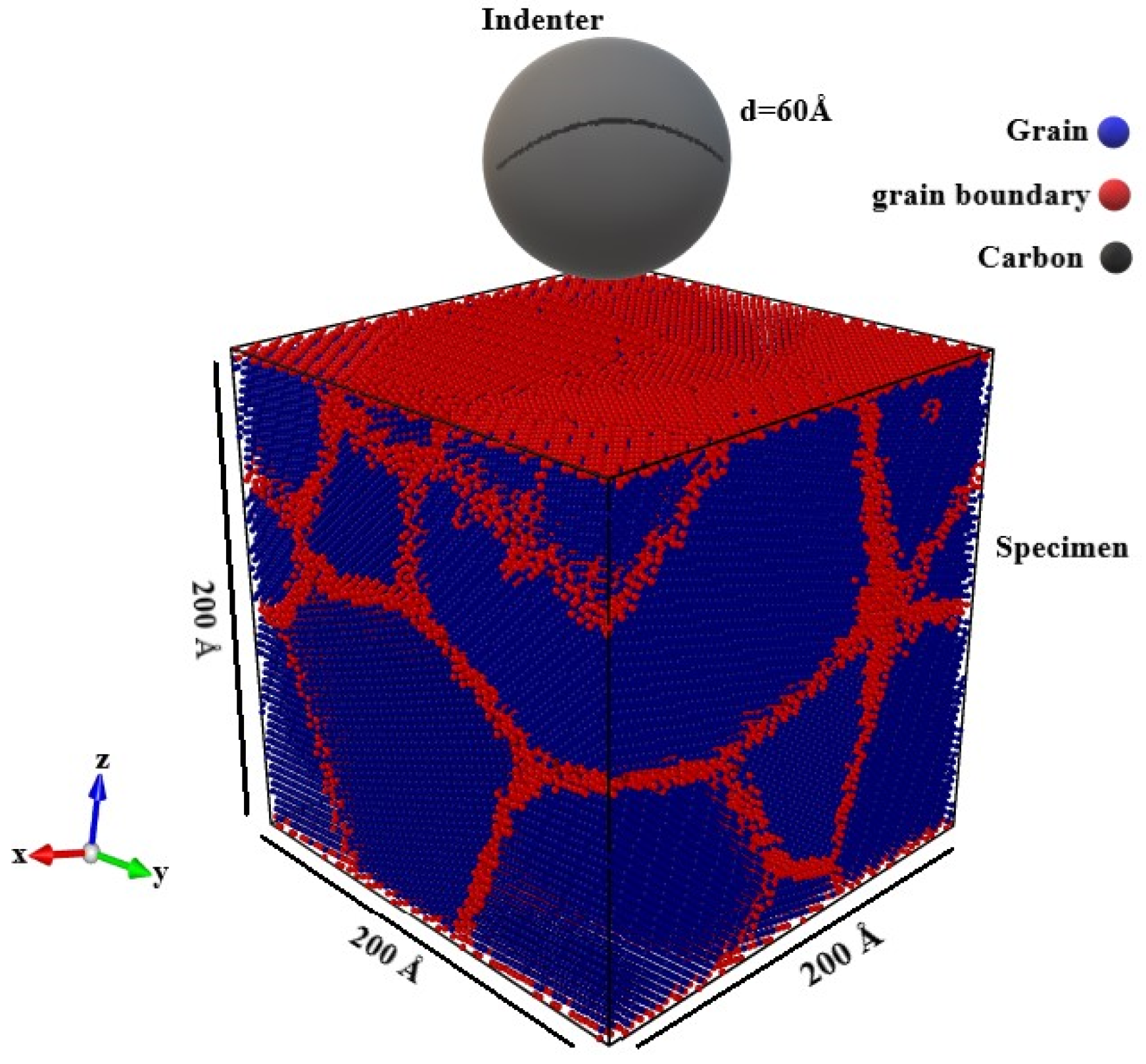
2.2. Sample Preparation
3. Results and Discussions
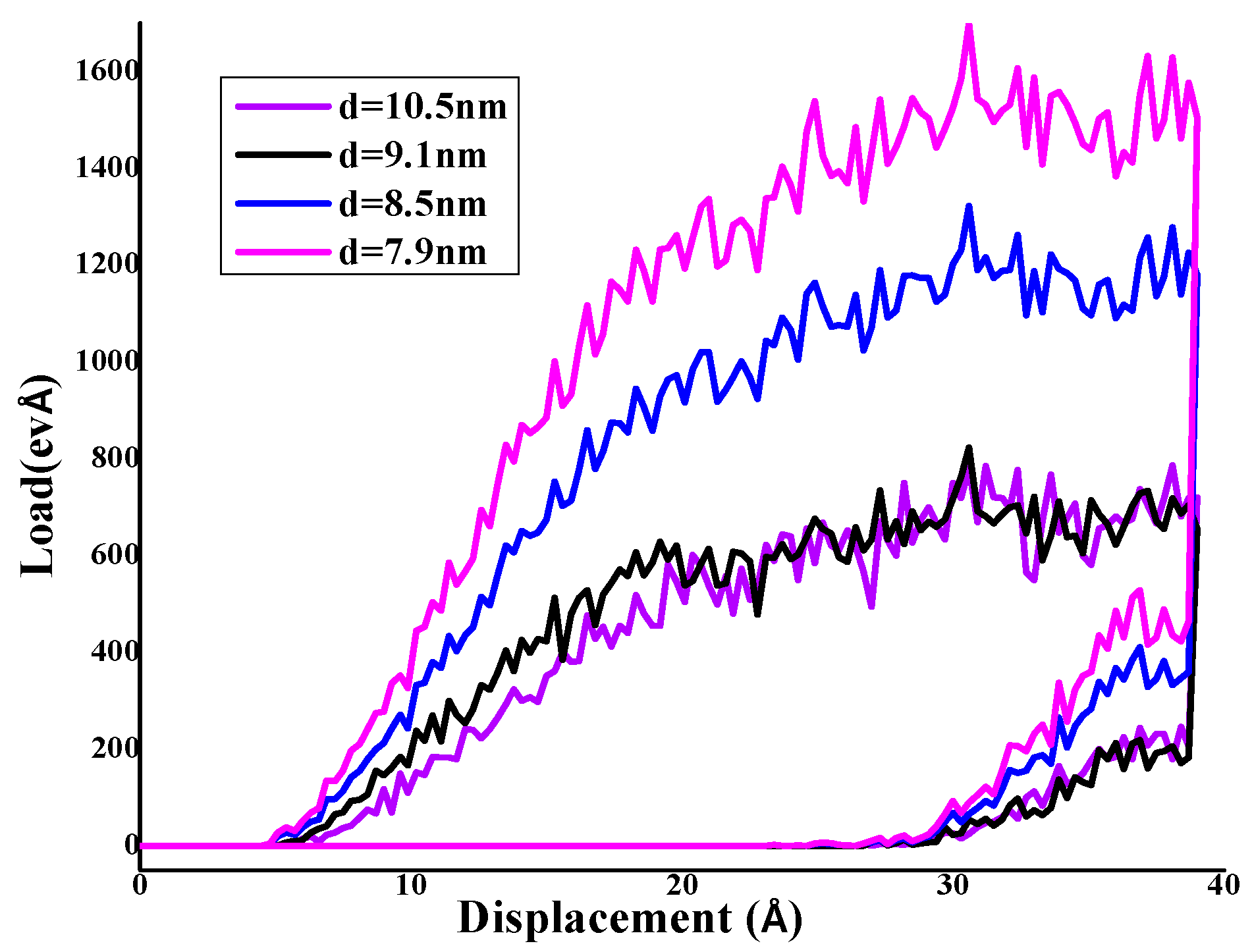
3.1. Effect of Size-Grain on Hardness and Elastic Modulus
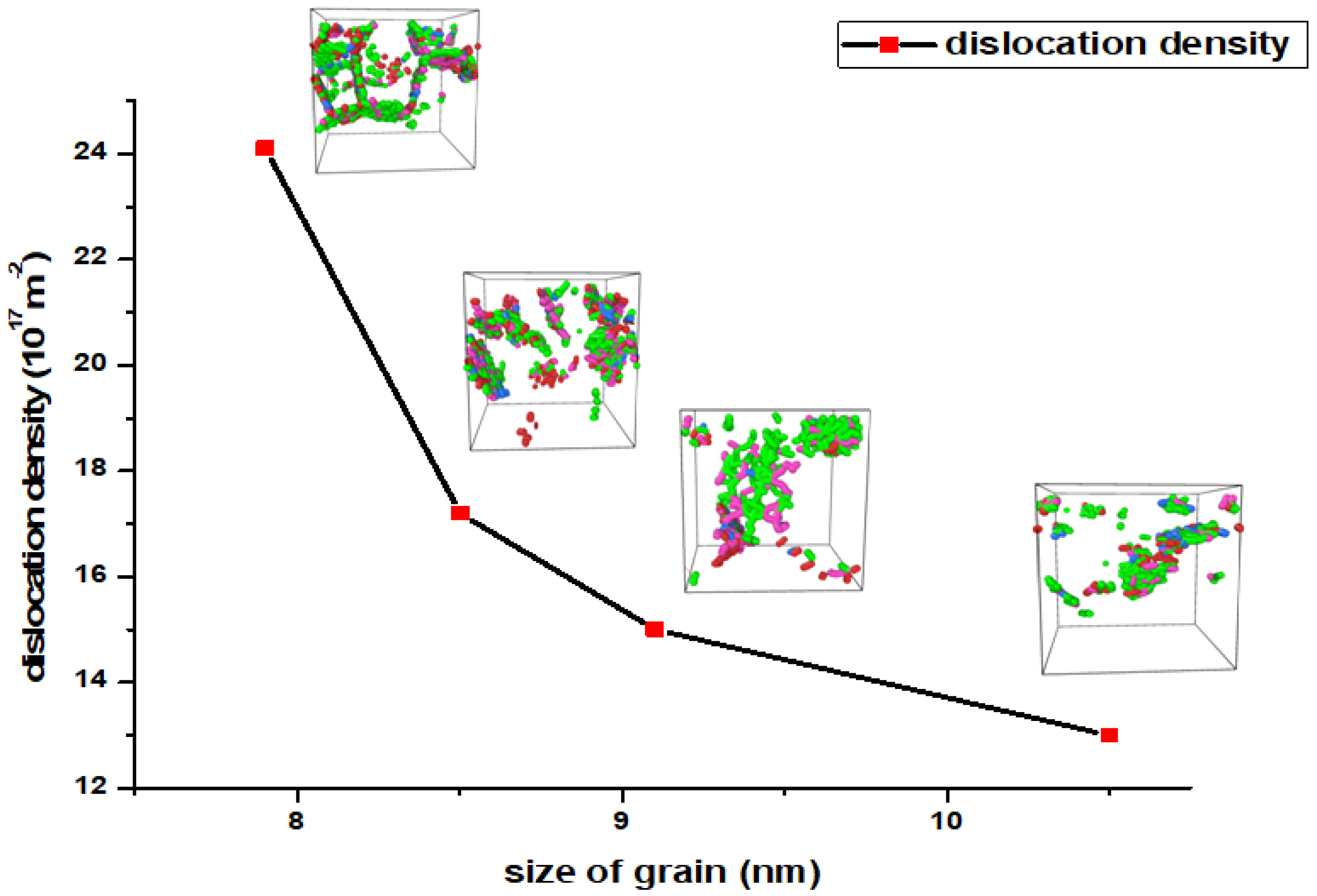
3.2. Mechanisms of Dislocation during Nanoindetation Process
4. Conclusions
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Chookajorn, T.; Murdoch, H.A.; Schuh, C.A. Design of stable nanocrystalline alloys. Science 2012, 337, 951–954. [Google Scholar] [CrossRef]
- Li, L.; Jiang, L.; Huang, G.; Wang, Z.; Chen, R.; Wei, Y.; Chen, X.; Zhang, L.; Liu, X. Mechanical properties of nanocrystalline tungsten and its behavior under neutron irradiation. Procedia Struct. Integr. 2020, 27, 702–709. [Google Scholar]
- Li, H.; Yang, R.; Yin, X.; Kang, X.; Wang, Z.; Li, L. Effect of grain size on the mechanical behavior and structural properties of nanocrystalline tungsten by molecular dynamics simulations. Metall. Mater. Trans. A 2020, 51, 5632–5642. [Google Scholar]
- Li, N.; Huang, H.; Wu, Y.; Wang, Y.; Zhang, Z.; Han, X.; Chen, M. Effect of grain size and temperature on mechanical behavior of nanocrystalline tungsten: In situ transmission electron microscopy study. J. Mater. Sci. 2018, 53, 346–359. [Google Scholar] [CrossRef]
- Zhang, T.; Huang, H.; Han, X.; Chen, M.; Wu, Y.; Zhang, Z. Microstructure and mechanical properties of nanocrystalline tungsten fabricated by spark plasma sintering. J. Mater. Sci. Technol. 2019, 35, 1296–1302. [Google Scholar] [CrossRef]
- Kato, M. Hallpetch relationship and dislocation model for deformation of ultrafine-grained and nanocrystalline metals. Mater. Trans. 2014, 55, 19–24. [Google Scholar] [CrossRef]
- Tahiri, A.; Idiri, M.; Boubeker, B. Molecular Dynamics Studies of Temperature and Grain Size Effects on Mechanical Properties of Nanocrystalline Tungsten. J. Nanomater. Mol. Nanotechnol. 2018, 7, 1. [Google Scholar] [CrossRef]
- Okamoto, H.; Massalski, T.B. Binary Alloy Phase Diagrams, 2nd ed.; ASM International: Materials Park, OH, USA, 1990; Volume 3. [Google Scholar]
- Umnov, A.G.; Shirator, Y.; Hiraoka, H. Giant field amplification in tungsten nanowires. Appl. Phys. A Mater. Sci. Process. 2003, 77, 159–161. [Google Scholar] [CrossRef]
- Armstrong, R.W.; Elban, W.L.; Walley, S.M. Elastic, plastic, cracking aspects of the hardness of materials. Int. J. Mod. Phys. B 2013, 27, 1–79. [Google Scholar] [CrossRef]
- Fischer-Cripps, A.C. Factors Affecting Nanoindentation Test Data. In Nanoindentation. Mechanical Engineering Series; Springer: New York, NY, USA, 2004. [Google Scholar] [CrossRef]
- Weppelmann, E.; Wittling, M.; Swain, M.V.; Munz, D. Indentation cracking of brittle thin films on brittle substrates. In Fracture Mechanics of Ceramics: Fatigue, Composites, and High-Temperature Behavior; Springer: Boston, MA, USA, 1996; pp. 475–486. [Google Scholar]
- Volinsky, A.A.; Vella, J.B.; Gerberich, W.W. Fracture toughness, adhesion and mechanical properties of low-K dielectric thin films measured by nanoindentation. Thin Solid Film. 2003, 429, 201–210. [Google Scholar] [CrossRef]
- Zhang, Y.; Wang, W.H.; Greer, A.L. Making metallic glasses plastic by control of residual stress. Nat. Mater. 2006, 5, 857–860. [Google Scholar] [CrossRef] [PubMed]
- Sargent, P.M.; Ashby, M.F. Indentation creep. Mater. Sci. Technol. 1992, 8, 594–601. [Google Scholar] [CrossRef]
- Bendavid, A.; Martin, P.J.; Takikawa, H. Deposition and modification of titanium dioxide thin films by filtered arc deposition. Thin Solid Film. 2000, 360, 241–249. [Google Scholar] [CrossRef]
- Karafi, T.; Tahiri, A.; Idiri, M.; Boubeker, B. Effect of temperature on hardness and elastic modulus in tungsten-rhenium alloy: Study by dynamics molecular. Int. J. Sci. Technol. Res. 2021, 10, 107–111. [Google Scholar]
- Oliver, W.C.; Pharr, G.M.; Introduction, I. An improved technique for determining hardness and elastic modulus using load and displacement sensing indentation experiments. J. Mater. Res. 1992, 7, 1564–1583. [Google Scholar] [CrossRef]
- Liang, X.; Hu, C.; Zhou, L.; Xiong, L.; Li, X. Embedded atom method potential: Theory, development, and applications. Mater. Sci. Technol. 2018, 34, 663–675. [Google Scholar] [CrossRef]
- Frenkel, D.; Smith, B. Understanding Molecular Dynamics Simulation from Algorithms to Applications, 2nd ed.; Academic Press: Cambridge, MA, USA, 1996; Volume 66. [Google Scholar]
- Dea, P.; Frauenheim, T.; Pederson, M.R. Computer Simulation of Materials at Atomic Level; John Wiley & Sons: Hoboken, NJ, USA, 2000; Volume 1. [Google Scholar]
- Rapaport, D.C. The Art of Molecular Dynamics Simulation, 2nd ed.; Cambridge University Press: Cambridge, UK, 1995. [Google Scholar]
- Daw, M.S.; Baskes, M.I. Embedded-atom method: Derivation and application to impurities, surfaces, and other defects in metals. Phys. Rev. B 1984, 29, 6443–6453. [Google Scholar] [CrossRef]
- Bonny, G.; Bakaev, A.; Terentyev, D.; Mastrikov, Y.A. Interatomic potential to study plastic deformation in tungsten-rhenium alloys. J. Appl. Phys. 2017, 121, 165107. [Google Scholar] [CrossRef]
- Plimpton, S. Fast parallel algorithms for short-range molecular dynamics. J. Comput. Phys. 1995, 117, 1–19. [Google Scholar] [CrossRef]
- Stukowski, A. Visualization and analysis of atomistic simulation data with OVITO-the Open Visualization Tool. Model. Simul. Mater. Sci. Eng. 2010, 18, 015012. [Google Scholar] [CrossRef]
- Stukowski, A.; Albe, K. Extracting dislocations and non-dislocation crystal defects from atomistic simulation data. Model. Simul. Mater. Sci. Eng. 2009, 17, 055011. [Google Scholar] [CrossRef]
- Wang, B.; Gao, Y.; Urbassek, H.M. Microstructure and magnetic disorder induced by nanoindentation in single-crystalline Fe. Phys. Rev. B Condens. Matter Mater. Phys. 2014, 89, 1–8. [Google Scholar] [CrossRef]
- Lu, K.; Lu, J. Nanostructured Metals and Alloys: Processing, Microstructure, Mechanical Properties and Applications; Springer Science & Business Media: Berlin/Heidelberg, Germany, 2004. [Google Scholar]
- Zhechen, Y. Study on Young’s Modulus of Nanocrystalline Tungsten. Phys. Procedia 2013, 50, 159–164. [Google Scholar]
- Valiev, R.Z.; Alexandrov, I.V.; Zhu, Y.T. Nanostructuring of metals by severe plastic deformation for advanced properties. Nat. Mater. 2004, 3, 511–516. [Google Scholar] [CrossRef] [PubMed]
- Barbee, T.W., Jr.; Yang, L.H.; Germann, T.C. The Hall–Petch relationship in nanocrystalline metals: A simple description based on homogeneously nucleated dislocations. J. Mater. Sci. 2013, 48, 1–13. [Google Scholar]
- Nieh, T.G.; Wang, S.J.; Wang, Y.M. High-strain-rate superplasticity in nanocrystalline copper at room temperature. Science 2006, 312, 1624–1627. [Google Scholar]
- Goel, S.; Gerberich, W.W. Mechanical response of single crystal tungsten under indentation. Mater. Sci. Eng. A 2006, 418, 126–131. [Google Scholar]
- Scruggs, B.; Maclennan, D.G.; Scattergood, R.O. The Hall–Petch equation and deformation mechanisms in nanocrystalline tungsten produced by mechanical attrition. Scr. Mater. 2005, 52, 171–175. [Google Scholar]
- Van Swygenhoven, H.; Spaczer, M.; Caro, A. Dislocation microstructure and mechanical properties of ultrafine polycrystalline molybdenum produced by powder metallurgy. Acta Mater. 1999, 47, 3211–3221. [Google Scholar]
- Wei, Q.; Cheng, S.; Ramesh, K.T.; Ma, E. Effect of nanocrystalline and ultrafine grain sizes on the strain rate sensitivity and activation volume: Fcc versus bcc metals. Mater. Sci. Eng. A 2004, 381, 71–79. [Google Scholar] [CrossRef]
- Wei, Q.; Zhang, H.T.; Schuster, B.E.; Ramesh, K.T.; Valiev, R.Z.; Kecskes, L.J.; Dowding, R.J.; Magness, L.; Cho, K. Microstructure and mechanical properties of super-strong nanocrystalline tungsten processed by high-pressure torsion. Acta Mater. 2006, 54, 4079–4089. [Google Scholar] [CrossRef]
- Wei, Q.; Pan, Z.L.; Wu, X.L.; Schuster, B.E.; Kecskes, L.J.; Valiev, R.Z. Microstructure and mechanical properties at different length scales and strain rates of nanocrystalline tantalum produced by high-pressure torsion. Acta Mater. 2011, 59, 2423–2436. [Google Scholar] [CrossRef]

| Material | W |
|---|---|
| Number of atoms | ~504,948 |
| Box size | 200 (x) × 200 (y) × 200 (z) Å |
| Time-step (fs) | 1 |
| Temperature (K) | 300 |
| Potential | EAM |
| Pressure (Pa) | Ambient atmospheric |
| Ensemble | NVT |
| Boundary Conditions | PPP |
| Runs | 30,000 |
| Indenter velocity | 3 Å/ps |
| Indenter radius | 30 Å |
| Size of Grain (nm) | d = 7.9 | d = 8.5 | d = 9.1 | d = 10.5 |
|---|---|---|---|---|
| Reduced elastic modulus Er (GPa) | 497.25 | 424.12 | 292.5 | 235.2 |
| Hardness H (GPa) | 54.7 | 42.58 | 26.51 | 24.73 |
| Yield stress σe (GPa) | 57.2 | 43.89 | 28.2 | 24.07 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Karafi, T.; Tahiri, A.; Chabba, H.; Idiri, M.; Boubeker, B. Effect of Grain-Size in Nanocrystalline Tungsten on Hardness and Dislocation Density: A Molecular Dynamics Study. Crystals 2023, 13, 469. https://doi.org/10.3390/cryst13030469
Karafi T, Tahiri A, Chabba H, Idiri M, Boubeker B. Effect of Grain-Size in Nanocrystalline Tungsten on Hardness and Dislocation Density: A Molecular Dynamics Study. Crystals. 2023; 13(3):469. https://doi.org/10.3390/cryst13030469
Chicago/Turabian StyleKarafi, Toufik, Abdellah Tahiri, Hanae Chabba, Mohamed Idiri, and Brahim Boubeker. 2023. "Effect of Grain-Size in Nanocrystalline Tungsten on Hardness and Dislocation Density: A Molecular Dynamics Study" Crystals 13, no. 3: 469. https://doi.org/10.3390/cryst13030469
APA StyleKarafi, T., Tahiri, A., Chabba, H., Idiri, M., & Boubeker, B. (2023). Effect of Grain-Size in Nanocrystalline Tungsten on Hardness and Dislocation Density: A Molecular Dynamics Study. Crystals, 13(3), 469. https://doi.org/10.3390/cryst13030469










