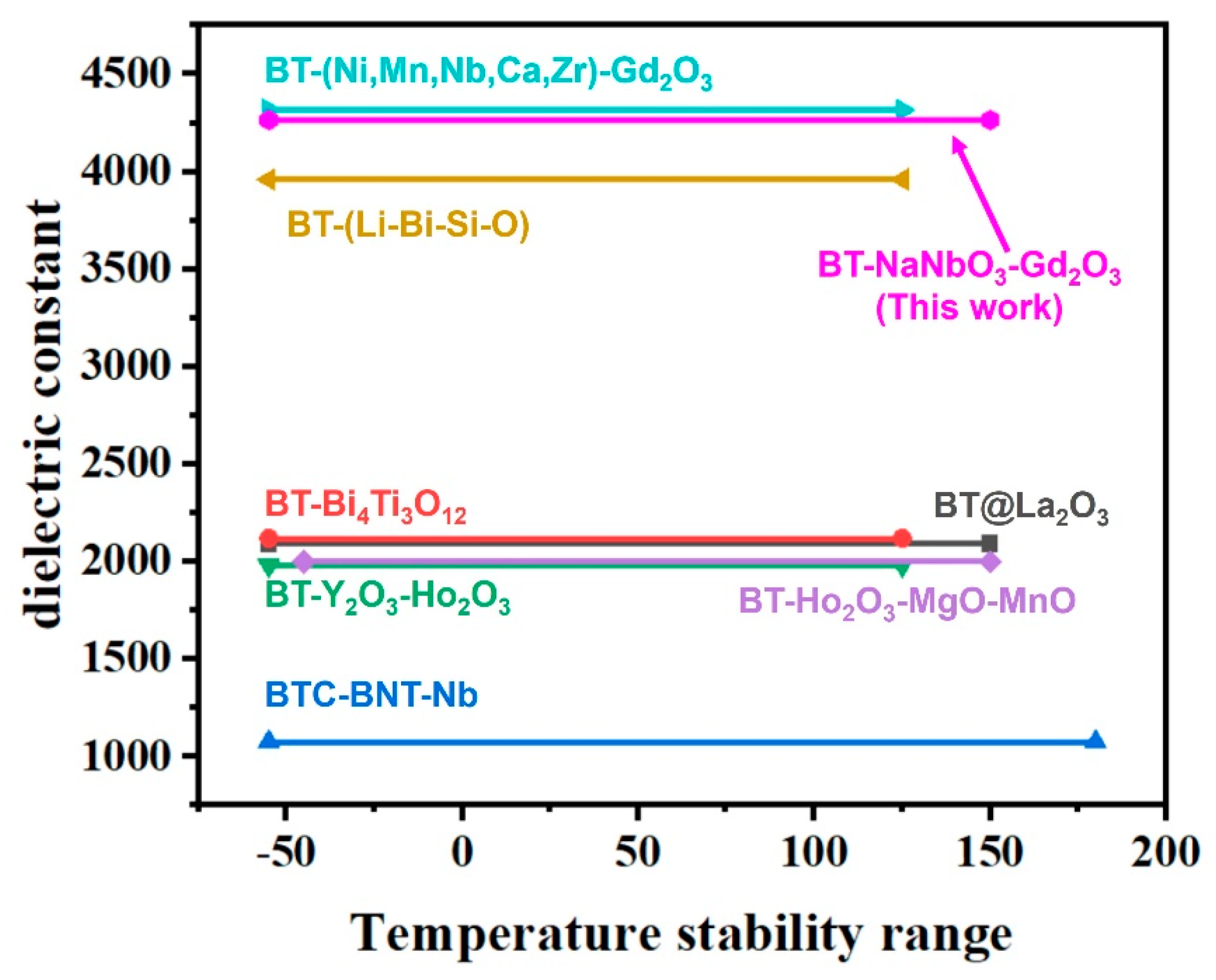
Wide Temperature Stability of BaTiO3-NaNbO3-Gd2O3 Dielectric Ceramics with Grain Core–Shell Structure
Abstract
:1. Introduction
2. Experiments
2.1. Sample Preparation
2.2. Sample Characterization and Testing
2.3. Research Methods
3. Results and Discussion
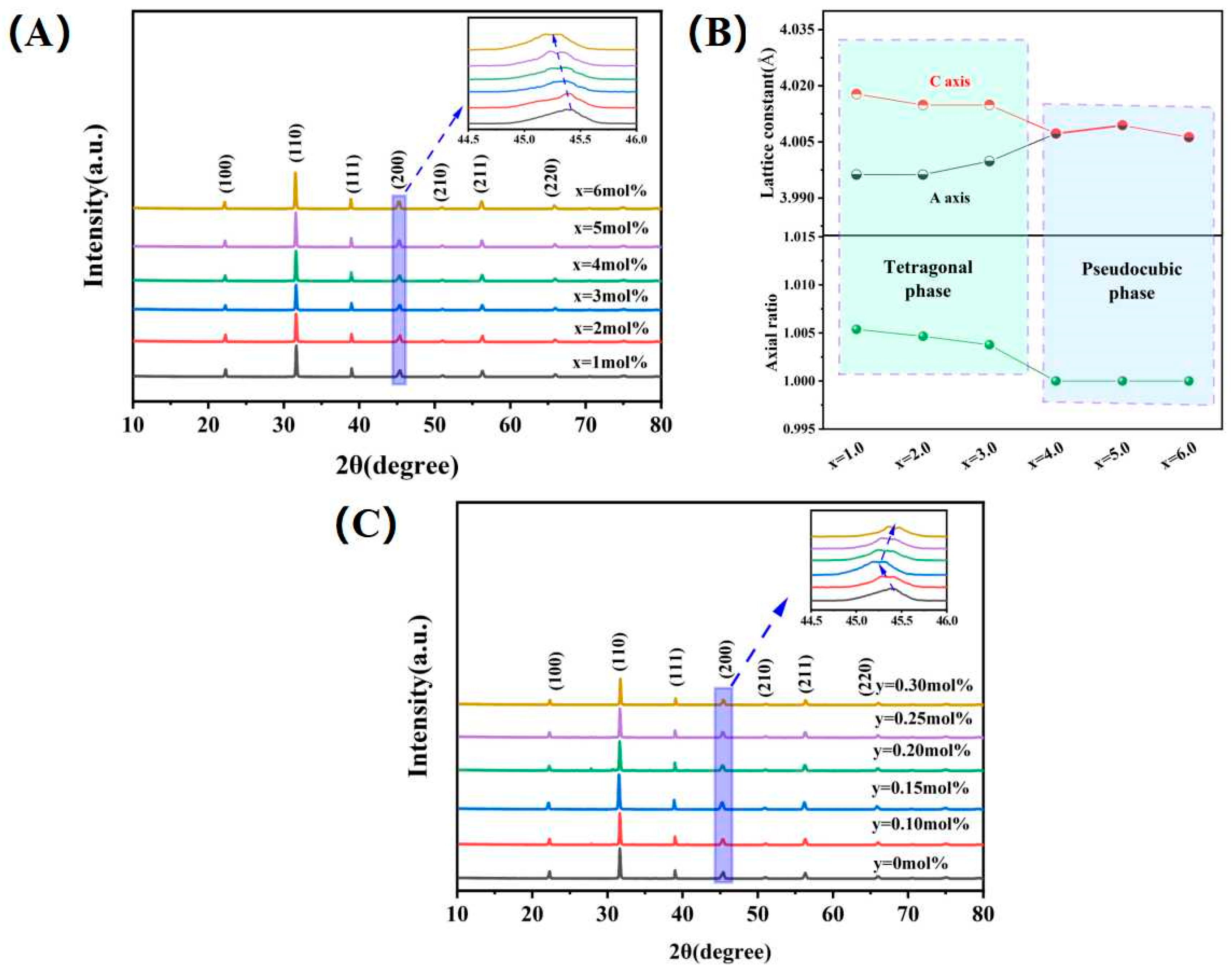
3.1. Phase Analysis
| Ions | Coordination Number (CN) | Ionic Radius (nm) |
|---|---|---|
| Ba2+ | 12 | 0.1610 |
| Na+ | 12 | 0.1020 |
| Gd3+ | 12 | 0.1253 |
| Gd3+ | 6 | 0.0938 |
| Nb5+ | 6 | 0.0720 |
| Ti4+ | 6 | 0.0610 |
| O2- | 6 | 0.1400 |
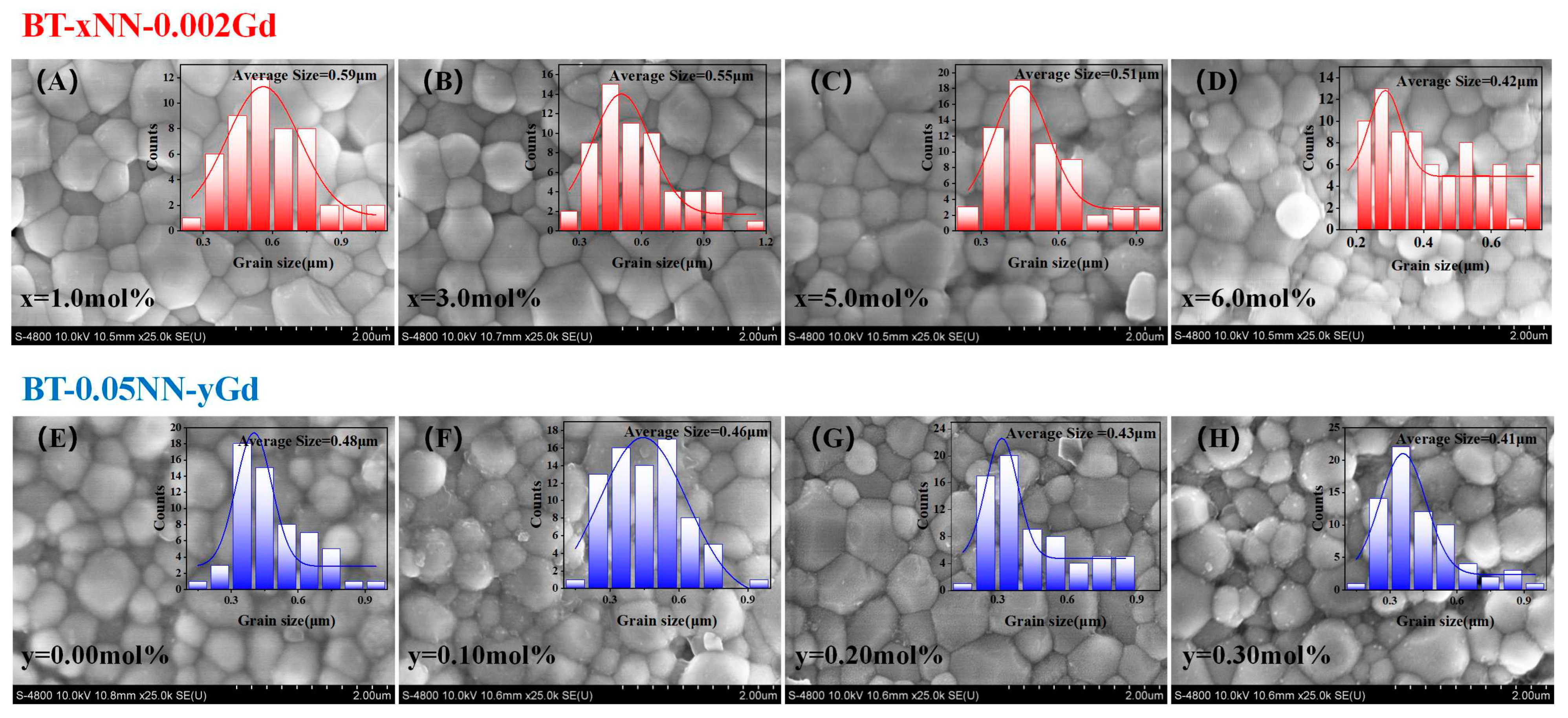
3.2. Morphological Analysis
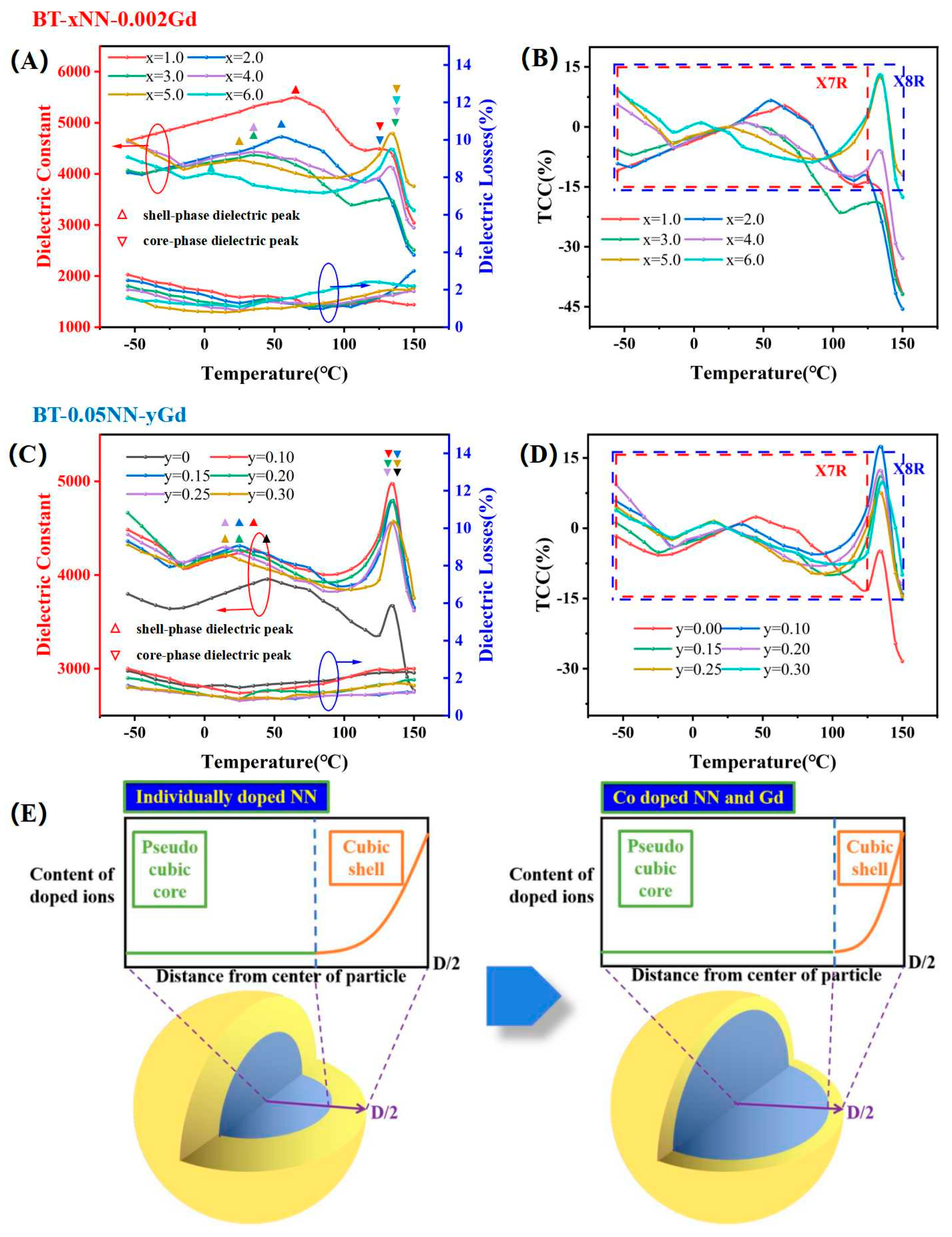
3.3. Dielectric and Insulation Properties
4. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Hong, K.; Lee, T.H.; Suh, J.M.; Yoon, S.-H.; Jang, H.W. Perspectives and challenges in multilayer ceramic capacitors for next generation electronics. J. Mater. Chem. C 2019, 7, 9782–9802. [Google Scholar] [CrossRef]
- Zhao, P.; Cai, Z.; Wu, L.; Zhu, C.; Li, L.; Wang, X. Perspectives and challenges for lead-free energy-storage multilayer ceramic capacitors. J. Adv. Ceram. 2021, 10, 1153–1193. [Google Scholar] [CrossRef]
- Wang, H.; Huang, R.; Hao, H.; Yao, Z.; Liu, H.; Cao, M. Multiscale grain synergistic by microstructure designed hierarchically structured in BaTiO3-based ceramics with enhanced energy storage density and X9R high-temperature dielectrics application. J. Mater. Sci. 2022, 57, 11839–11851. [Google Scholar] [CrossRef]
- Li, M.; Bai, L.; Zhang, Y.; Luo, G.; Han, Y.; Meng, D.; Tu, R.; Shen, Q. A Review of Titanate-based Multi-layer Ceramic Capacitors with High Capacitance and Stablility. China’s Ceram. 2022, 58, 7–19. [Google Scholar]
- Jayakrishnan, A.R.; Silva, J.P.B.; Kamakshi, K.; Dastan, D.; Annapureddy, V.; Pereira, M.; Sekhar, K.C. Are lead-free relaxor ferroelectric materials the most promising candidates for energy storage capacitors? Prog. Mater. Sci. 2023, 132, 101046. [Google Scholar] [CrossRef]
- Jiang, B.B.; Iocozzia, J.; Zhao, L.; Zhang, H.F.; Harn, Y.W.; Chen, Y.H.; Lin, Z.Q. Barium titanate at the nanoscale: Controlled synthesis and dielectric and ferroelectric properties. Chem. Soc. Rev. 2019, 48, 1194–1228. [Google Scholar] [CrossRef]
- Venkatachalam, V.; Vaidhyanathan, B.; Binner, J. Synthesis of nanocrystalline barium titanate: Effect of microwave power on phase evolution. J. Eur. Ceram. Soc. 2020, 40, 3974–3983. [Google Scholar] [CrossRef]
- Prado, L.R.; de Resende, N.S.; Silva, R.S.; Egues, S.M.S.; Salazar-Banda, G.R. Influence of the synthesis method on the preparation of barium titanate nanoparticles. Chem. Eng. Process. Process Intensif. 2016, 103, 12–20. [Google Scholar] [CrossRef]
- Zhai, Y.; Xie, X.; Zhou, R.; Li, X.; Liu, X.; Liu, S. High performance room temperature ferroelectric barium strontium titanate ceramics by spark-plasma-sintering ultrafine nanocrystals. Ceram. Int. 2019, 45, 15526–15531. [Google Scholar] [CrossRef]
- Gong, H.; Wang, X.; Zhang, S.; Wen, H.; Li, L. Grain size effect on electrical and reliability characteristics of modified fine-grained BaTiO3 ceramics for MLCCs. J. Eur. Ceram. Soc. 2014, 34, 1733–1739. [Google Scholar] [CrossRef]
- Jo, S.K.; Park, J.S.; Han, Y.H. Effects of multi-doping of rare-earth oxides on the microstructure and dielectric properties of BaTiO3. J. Alloys Compd. 2010, 501, 259–264. [Google Scholar] [CrossRef]
- Jeon, S.-C.; Kang, S.-J.L. Coherency strain enhanced dielectric-temperature property of rare-earth doped BaTiO3. Appl. Phys. Lett. 2013, 102, 112915. [Google Scholar] [CrossRef]
- Yao, F.-Z.; Yuan, Q.; Wang, Q.; Wang, H. Multiscale structural engineering of dielectric ceramics for energy storage applications: From bulk to thin films. Nanoscale 2020, 12, 17165–17184. [Google Scholar] [CrossRef] [PubMed]
- Kim, D.; Kim, J.; Noh, T.; Ryu, J.; Kim, Y.-n.; Lee, H. Dielectric properties and temperature stability of BaTiO3 co-doped La2O3 and Tm2O3. Curr. Appl. Phys. 2012, 12, 952–956. [Google Scholar] [CrossRef]
- Bein, N.; Kmet, B.; Rojac, T.; Golob, A.B.; Malič, B.; Moxter, J.; Schneider, T.; Fulanovic, L.; Azadeh, M.; Frömling, T.; et al. Fermi energy, electrical conductivity, and the energy gap of NaNbO3. Phys. Rev. Mater. 2022, 6, 084404. [Google Scholar] [CrossRef]
- Li, P.; Abe, H.; Ye, J. Band-Gap Engineering of NaNbO3 for Photocatalytic H2 Evolution with Visible Light. Int. J. Photoenergy 2014, 2014, 380421. [Google Scholar] [CrossRef]
- Montecillo, R.; Chen, C.-S.; Feng, K.-C.; Chien, R.R.; Haw, S.-C.; Chen, P.-Y.; Tu, C.-S. Achieving superb electric energy storage in relaxor ferroelectric BiFeO3-BaTiO3-NaNbO3 ceramics via O2 atmosphere. J. Eur. Ceram. Soc. 2023, 43, 7446–7454. [Google Scholar] [CrossRef]
- Zhang, C.; Du, Q.; Li, W.; Su, D.; Shen, M.; Qian, X.; Li, B.; Zhang, H.; Jiang, S.; Zhang, G. High electrocaloric effect in barium titanate-sodium niobate ceramics with core-shell grain assembly. J. Mater. 2020, 6, 618–627. [Google Scholar] [CrossRef]
- Benedek, N.A.; Fennie, C.J. Why Are There So Few Perovskite Ferroelectrics? J. Phys. Chem. C 2013, 117, 13339–13349. [Google Scholar] [CrossRef]
- Muhammad, R.; Ali, A.; Camargo, J.; Castro, M.S.; Lei, W.; Song, K.X.; Wang, D.W. Enhanced Thermal Stability in Dielectric Properties of NaNbO3-Modified BaTiO3-BiMg1/2Ti1/2O3 Ceramics for X9R-MLCC Applications. Crystals 2022, 12, 141. [Google Scholar] [CrossRef]
- Raengthon, N.; Brown-Shaklee, H.J.; Brennecka, G.L.; Cann, D.P. Dielectric properties of BaTiO3-Bi(Zn1/2Ti1/2)O3-NaNbO3 solid solutions. J. Mater. Sci. 2013, 48, 2245–2250. [Google Scholar] [CrossRef]
- Muhammad, R.; Ali, A.; Camargo, J.; Castro, M.S. Temperature Stable Dielectric Properties in BaTiO3-Bi(Mg2/3Nb1/3)O3-NaNbO3 Solid Solution. Chemistryselect 2020, 5, 3730–3734. [Google Scholar] [CrossRef]
- Li, L.; Guo, D.; Xia, W.; Liao, Q.; Han, Y.; Peng, Y.; Alford, N. An Ultra-Broad Working Temperature Dielectric Material of BaTiO3-Based Ceramics with Nd2O3 Addition. J. Am. Ceram. Soc. 2012, 95, 2107–2109. [Google Scholar] [CrossRef]
- Li, L.; Fu, R.; Liao, Q.; Ji, L. Doping behaviors of NiO and Nb2O5 in BaTiO3 and dielectric properties of BaTiO3-based X7R ceramics. Ceram. Int. 2012, 38, 1915–1920. [Google Scholar] [CrossRef]
- Yashima, M.; Hoshina, T.; Ishimura, D.; Kobayashi, S.; Nakamura, W.; Tsurumi, T.; Wada, S. Size effect on the crystal structure of barium titanate nanoparticles. J. Appl. Phys. 2005, 98, 014313. [Google Scholar] [CrossRef]
- Miller, V.L.; Tidrow, S.C. Perovskites: Some Polarization Induced Structural Phase Transitions Using “Effective” Temperature and Coordination Dependent Radii and Polarizabilities of Ions. Integr. Ferroelectr. 2015, 166, 206–224. [Google Scholar] [CrossRef]
- Ben, L.B.; Sinclair, D.C. Anomalous Curie temperature behavior of A-site Gd-doped BaTiO3 ceramics: The influence of strain. Appl. Phys. Lett. 2011, 98, 092907. [Google Scholar] [CrossRef]
- Hou, Z.W.; Kang, A.G.; Ma, W.Q.; Zhao, X.L. Dimension effects on the dielectric properties of fine BaTiO3 ceramics. Chin. Phys. B 2014, 23, 117701. [Google Scholar] [CrossRef]
- Yang, Z.T.; Du, H.L.; Jin, L.; Poelman, D. High-performance lead-free bulk ceramics for electrical energy storage applications: Design strategies and challenges. J. Mater. Chem. A 2021, 9, 18026–18085. [Google Scholar] [CrossRef]
- Hu, Z.Y.; Cui, B.; Li, M.; Li, L.L. Novel X8R-type BaTiO3-based ceramics with a high dielectric constant created by doping nanocomposites with Li-Ti-Si-O. J. Mater. Sci.-Mater. Electron. 2013, 24, 3850–3855. [Google Scholar] [CrossRef]
- Jain, T.A.; Chen, C.C.; Fung, K.Z. Effects of Bi4Ti3O12 addition on the microstructure and dielectric properties of modified BaTiO3 under a reducing atmosphere. J. Eur. Ceram. Soc. 2009, 29, 2595–2601. [Google Scholar] [CrossRef]
- Li, L.X.; Wang, M.J.; Guo, D.; Fu, R.X.; Meng, Q.L. Effect of Gd amphoteric substitution on structure and dielectric properties of BaTiO3-based ceramics. J. Electroceramics 2013, 30, 129–132. [Google Scholar] [CrossRef]
- Luo, G.Q.; Zhang, G.R.; Zhang, Y.; Li, A.; Sun, Y.; Tu, R.; Shen, Q. Wide temperature range of stable dielectric properties in relaxor BaTiO3-based ceramics by co-doping synergistic engineering. Mater. Chem. Phys. 2023, 302, 127629. [Google Scholar] [CrossRef]
- Wang, X.H.; Chen, R.Z.; Zhou, H.; Li, L.T.; Gui, Z.L. Dielectric properties of BaTiO3-based ceramics sintered in reducing atmospheres prepared from nano-powders. Ceram. Int. 2004, 30, 1895–1898. [Google Scholar] [CrossRef]
- Wang, Y.; Cui, B.; Liu, Y.; Zhao, X.T.; Hu, Z.Y.; Yan, Q.Q.; Wu, T.; Zhao, L.L.; Wang, Y.Y. Fabrication of submicron La2O3-coated BaTiO3 particles and fine-grained ceramics with temperature-stable dielectric properties. Scr. Mater. 2014, 90–91, 49–52. [Google Scholar] [CrossRef]
- Zhang, Y.C.; Wang, X.H.; Kim, J.Y.; Li, L.T. Effect of Rare Earth Oxide Content on Nanograined Base Metal Electrode Multilayer Ceramic Capacitor Powder Prepared by Aqueous Chemical Coating Method. Jpn. J. Appl. Phys. 2013, 52, 021501. [Google Scholar] [CrossRef]


Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Zhao, Z.; Bai, Y.; Li, M.; Ji, H. Wide Temperature Stability of BaTiO3-NaNbO3-Gd2O3 Dielectric Ceramics with Grain Core–Shell Structure. Crystals 2024, 14, 488. https://doi.org/10.3390/cryst14060488
Zhao Z, Bai Y, Li M, Ji H. Wide Temperature Stability of BaTiO3-NaNbO3-Gd2O3 Dielectric Ceramics with Grain Core–Shell Structure. Crystals. 2024; 14(6):488. https://doi.org/10.3390/cryst14060488
Chicago/Turabian StyleZhao, Zicheng, Yaoning Bai, Mingwei Li, and Huiming Ji. 2024. "Wide Temperature Stability of BaTiO3-NaNbO3-Gd2O3 Dielectric Ceramics with Grain Core–Shell Structure" Crystals 14, no. 6: 488. https://doi.org/10.3390/cryst14060488
APA StyleZhao, Z., Bai, Y., Li, M., & Ji, H. (2024). Wide Temperature Stability of BaTiO3-NaNbO3-Gd2O3 Dielectric Ceramics with Grain Core–Shell Structure. Crystals, 14(6), 488. https://doi.org/10.3390/cryst14060488