Localized Structural and Electronic Perturbations Induced by Mono-Vacancy in MgH₂: A Comprehensive First-Principles Investigation

Abstract

In the pursuit of sustainable energy, magnesium hydride (MgH₂) stands out as a promising candidate for hydrogen storage due to its high capacity. Nevertheless, its high thermodynamic stability necessitates elevated operating temperatures, thereby hindering practical applications. To mitigate this limitation, our study employs a defect engineering approach by introducing a mono-vacancy to decrease its thermodynamic stability. Utilizing first-principles density functional theory calculations, we investigate the influence of a mono-vacancy on the structural and electronic properties of MgH₂ crystal. Introducing the defect results in a $0.57\%$ contraction of the $a/b$ lattice parameters and a $1.03\%$ expansion along the c-axis, causing lattice distortion. Electronically, the band gap narrows by 0.67 eV, indicating an increase in metallic character. We observe a distinct vacancy-affected zone, characterized by substantial alterations in electron density within a 26.505 ų volume and modifications to the potential energy distribution encompassing a 19.514 ų volume. The mono-vacancy enhances the polarity of the Mg-H bonds and maximally decreases the bond energy by 0.065 eV. A localized high-energy region of 0.354 eV emerges, functioning as an energy barrier to atomic diffusion. This energy barrier is encompassed by low-energy pathways, potentially facilitating H atom migration within the MgH₂ crystal.

1. Introduction

Hydrogen, known for its high energy density, environmental friendliness, and abundance, is crucial in the pursuit of sustainable energy solutions. As global efforts to reduce carbon emissions intensify, hydrogen is emerging as a key energy carrier, paving the way for a low-carbon economy [1,2]. Among solid-state hydrogen storage materials, magnesium hydride (MgH₂) has attracted considerable attention due to its high storage capacity of 7.6 wt.%, reversibility, stability, and resistance to contamination [3,4,5].

Nevertheless, the practical applications of MgH₂ are limited by its elevated thermodynamic stability and slow hydrogen sorption kinetics, requiring high operating temperatures [6,7]. Research efforts have focused on reducing reaction temperatures and accelerating hydrogen absorption and desorption kinetics in MgH₂ to overcome these challenges. Approaches involve incorporating catalysts like transition metals [8], oxides [9], and carbides [10,11], as well as developing nanostructured MgH₂ [12,13].

In addition to catalysis and nanostructuring, defect engineering is considered an effective method for reducing thermodynamic stability and enhancing the reaction kinetics of materials [14,15]. This approach can improve hydrogen storage performance by diminishing dehydrogenation enthalpies and weakening Mg-H bonds in defective crystals [16,17]. Among the various types of defects, vacancies, which are common in metals and arise from processes such as deformation, irradiation, or quenching, have a notable influence on the material’s properties [18]. Vacancies can disrupt the periodicity of the crystal lattice, creating regions with altered electronic and chemical characters [19]. Studies have shown that H atoms are inclined to be captured in the vicinity of vacancies and vacancy clusters, underscoring the atomic-level role that vacancies play in the hydrogen storage process [20].

However, achieving an atomic-level understanding of these phenomena remains a formidable challenge due to the limitations of experimental measurement [21]. The complexity of defect–hydrogen interactions and the difficulty in isolating these interactions in experiments highlight the need for computational approaches to supplement and extend experimental findings [22]. In this context, first-principles density functional theory (DFT) calculations offer a detailed depiction of electronic structures and atomic interactions, providing essential theoretical support for understanding and optimizing defect engineering in the hydrogen storage process [23].

In this context, this study aims to investigate the influence of a mono-vacancy defect on the fundamental structural and electronic properties of a MgH₂ crystal, including lattice distortion, electron distribution, bonding nature, and the potential energy distribution. The findings from this study are anticipated to enrich current knowledge, potentially guiding future approaches for optimizing MgH₂-based hydrogen storage materials.

2. Computational Methods

2.1. DFT Calculation Details

This study employed DFT calculations using the plane-wave pseudopotential method implemented in the Quantum ESPRESSO package [24,25], a widely used open-source software suite. For visualization and analysis, we utilized VMD (Visual Molecular Dynamics) [26], a powerful 3D molecular visualization tool, and Matplotlib [27], a Python plotting library.

The DFT calculations utilized projector-augmented wave (PAW) pseudopotentials [28] and the Perdew–Burke–Ernzerhof (PBE) functional [29], which belongs to the generalized gradient approximation (GGA). A plane-wave basis set with a 60 Ry energy cutoff was used, and the Brillouin zone was sampled with a 4 × 4 × 4 k-point mesh using the Monkhorst–Pack scheme [30]. Gaussian smearing with 0.01 Ry spreading was applied, which helps to smooth the occupation numbers around the Fermi level.

Structural relaxation was performed iteratively using the Broyden–Fletcher–Goldfarb–Shanno (BFGS) algorithm, with convergence criteria set as follows: the atomic forces were below ${10}^{-3}$ atomic units (a.u.), and the changes in total energy were below ${10}^{-4}$ a.u. The density of states (DOS) was calculated using non-self-consistent field (NSCF) calculations with a denser 12 × 12 × 12 k-point mesh after the initial self-consistent field (SCF) calculations [31], to refine the electronic states.

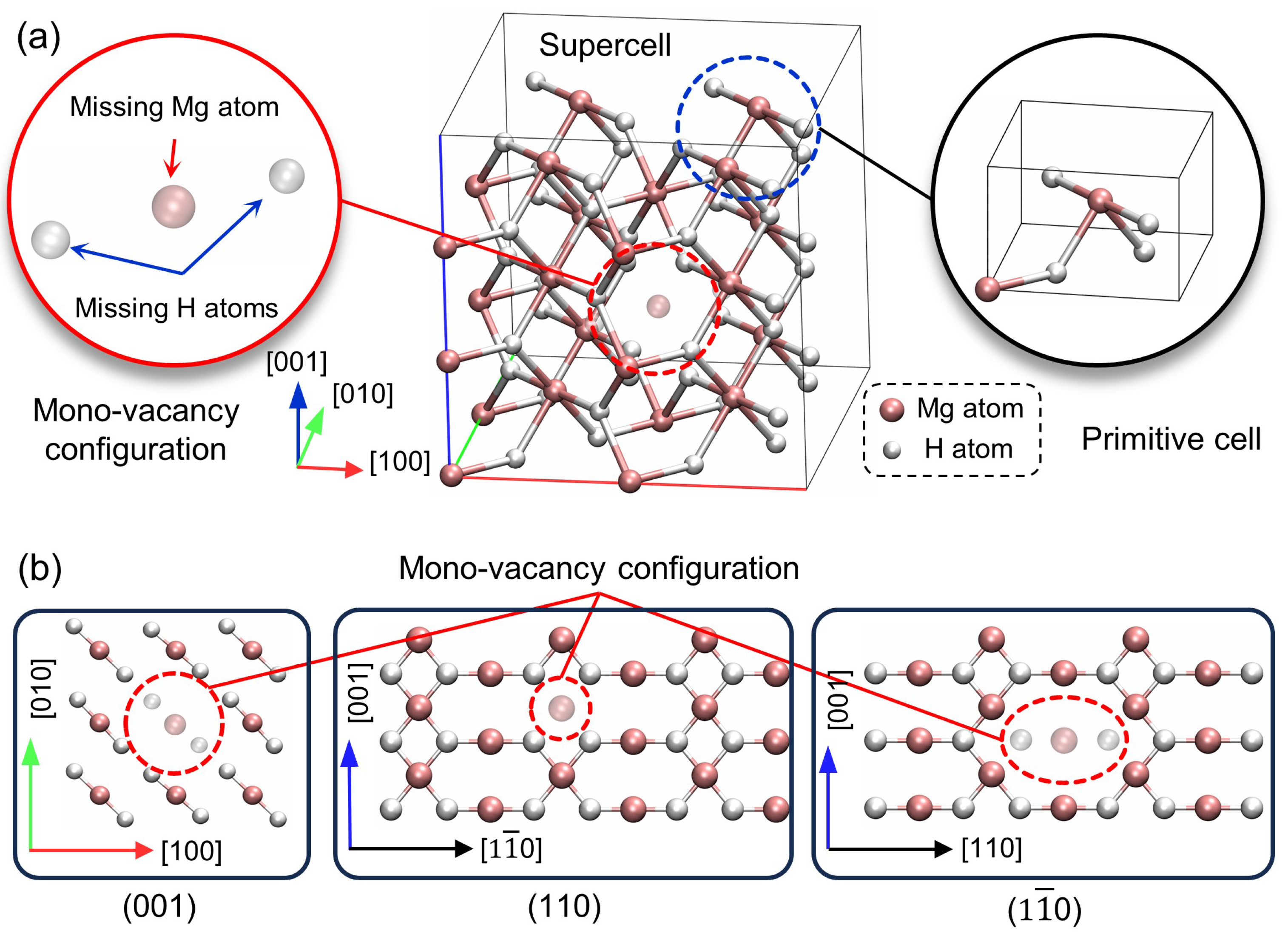
2.2. Model Configuration

A 2 × 2 × 3 supercell model of pristine MgH₂ crystal was initially created, containing 24 Mg atoms and 48 H atoms. Subsequently, a mono-vacancy was introduced by removing one Mg atom and its two first-neighbor H atoms, resulting in a vacancy concentration of $4.17\%$. The configuration of the supercell with the mono-vacancy is illustrated in Figure 1a.

The reason for not simply removing a single Mg atom to create a mono-vacancy is based on the potential for significant perturbation of local structural and chemical equilibrium. Removing only one Mg atom could significantly alter the local environment, resulting in changed local stoichiometry, inducing charge imbalance and causing computational instabilities. These instabilities could, in turn, hinder the convergence of ionic positions and electronic states to their minimum energy configurations, complicating the analysis and interpretation of computational results.

Removing both a Mg atom and its two first-neighbor H atoms maintains a more balanced local environment, which is essential for preserving the integrity of computational simulations and providing a stable framework. This approach maximizes lattice symmetry and periodicity while preserving local stoichiometry, thereby ensuring computational stability.

It should be noted that the mono-vacancy configuration (H-Mg-H) in the MgH₂ crystal can exist in two distinct configurations: one along the [110] direction and another along the [$1\overline{1}0$] direction. This study specifically examines the configuration along the [110] direction. Microscopically, the results from this direction exhibit minor differences characterized by a 90° rotational difference relative to c-axis. Notwithstanding, the differences do not exert an influence on the macroscopic properties of the crystal, as the [110] and [$1\overline{1}0$] directions are crystallographically equivalent.

2.3. Verification

To validate our DFT calculations, we first calculated the lattice parameters, band gap, and formation energy of the MgH₂ primitive cell. These results were compared with experimental measurements and prior DFT studies, as shown in

Table 1. A comparison of the key parameters from the current calculations to those in references.

Parameters	In Present Calculations	In References	Max Deviation
$a/b$ (Å)	4.511	4.518 [32], 4.512 [33], 4.501 [34]	$0.222\%$
c (Å)	3.016	3.021 [32], 3.016 [33], 3.010 [34]	$0.364\%$
band gap (eV)	3.72	3.90 [33], 3.60 [35]	$3.226\%$
formation energy (eV/atom)	−0.276	−0.278 [33]	$0.088\%$

.

Our calculated values show good agreement with both experimental measurements and previous DFT calculations, with a maximum deviation of $3.226\%$. This agreement validates the robustness and reliability of our DFT methods, models, and parameter selections, establishing a solid foundation for subsequent analyses.

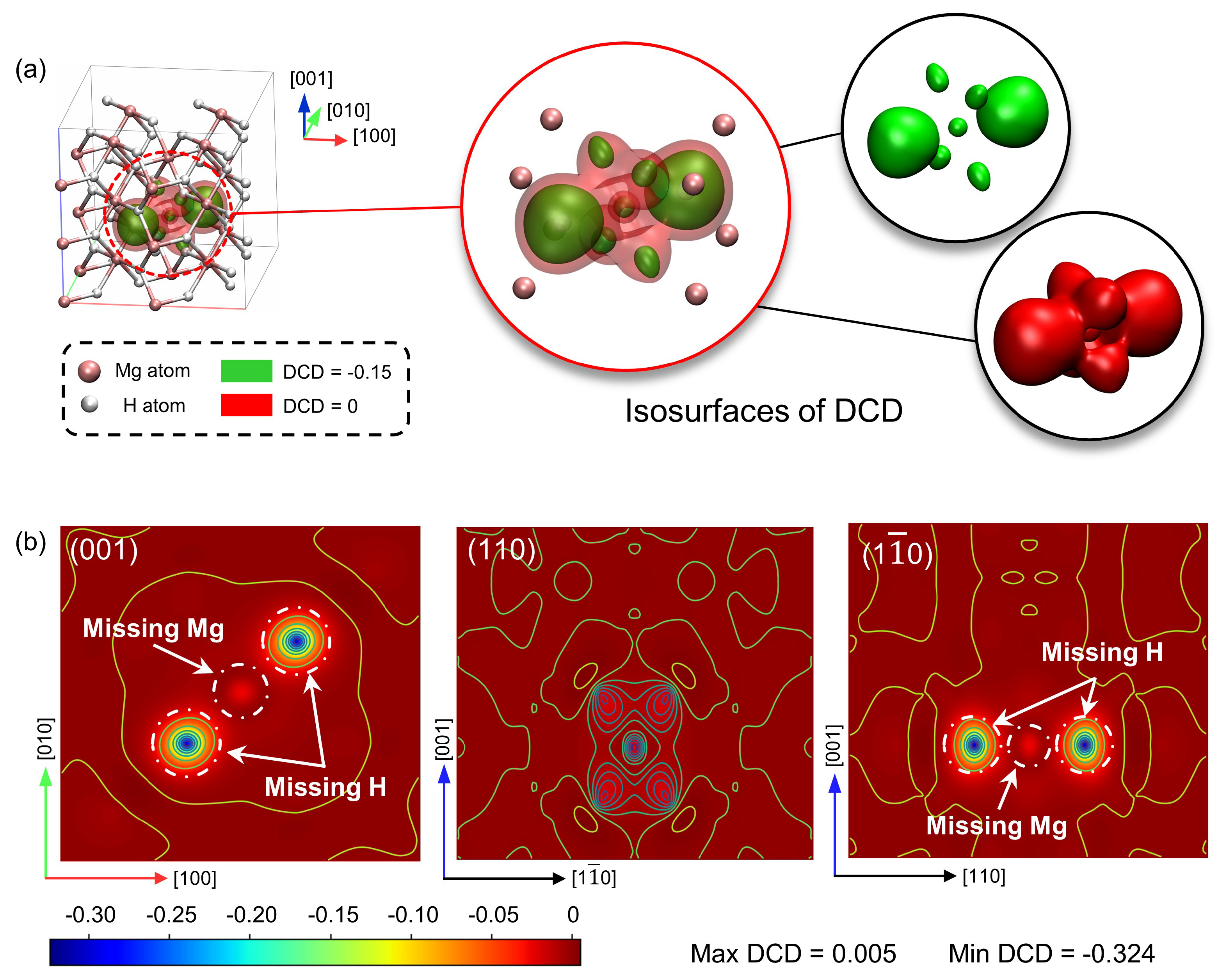
3. Results

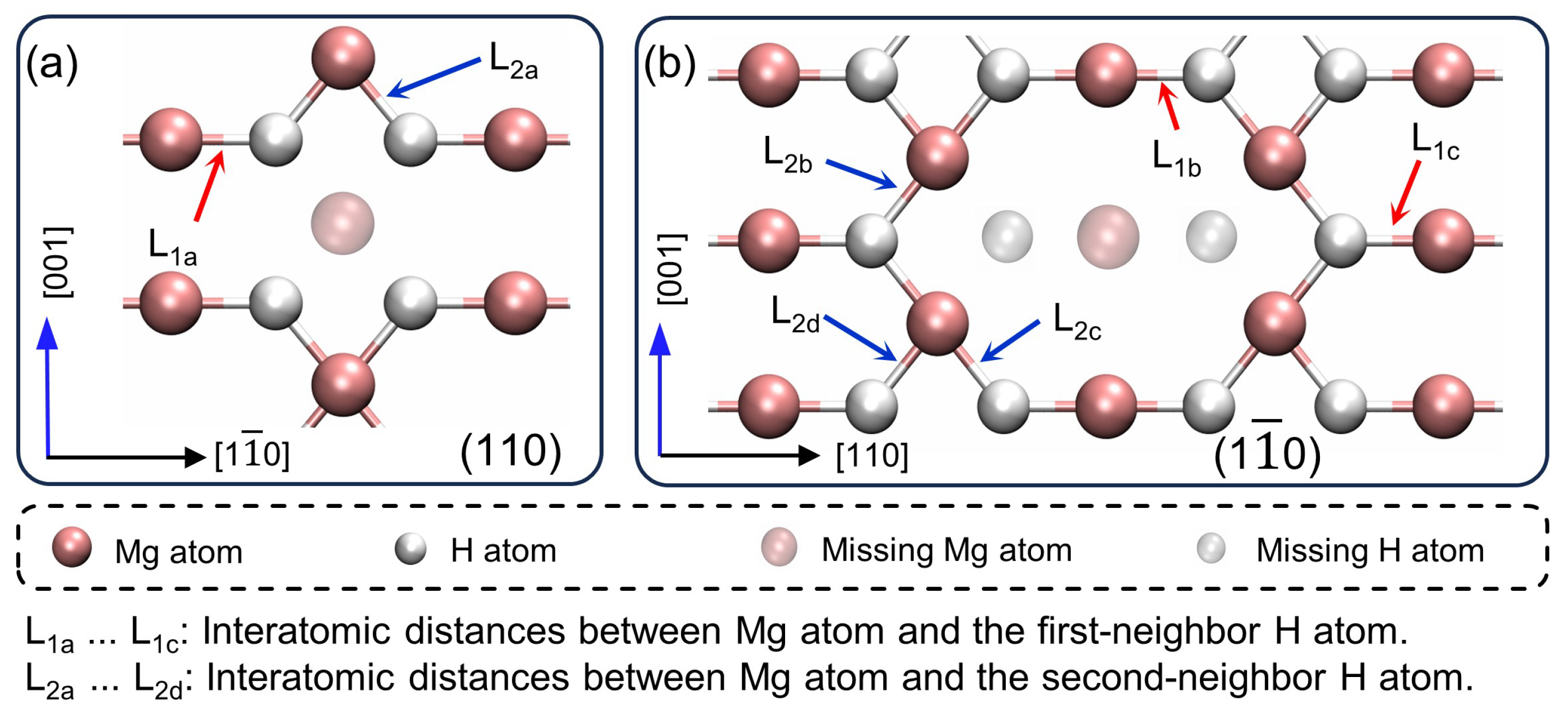
3.1. Crystal Structure

Our optimized pristine MgH₂ crystal exhibits a tetragonal rutile structure ($P{4}_2/mnm$, Group No. 136), with Mg atoms occupying 2a Wyckoff sites and H atoms at 4f positions, as illustrated in the primitive cell in Figure 1a. The atomic arrangements on the (001), (110), and ($1\overline{1}0$) crystallographic planes intersected by the vacancy are depicted in Figure 1b.

Figure 2 represents the local atomic environments in the MgH₂ crystal, focusing on the coordination of H and Mg atoms. In Figure 2a, a central H atom is surrounded by five Mg atoms, with four on a coplanar basal plane forming a rhombus. The H atom is coordinated by one first-neighbor Mg atom and two second-neighbor Mg atoms. Similarly, Figure 2b shows a central Mg atom surrounded by six H atoms, with four second-neighbors coplanar and two first-neighbors aligned collinearly, placing the Mg atom at the center of an octahedral void. These diagrams highlight the tetrahedral coordination environment of the MgH₂ crystal structure, where H atoms exhibit an approximate hexagonal close-packed (HCP) structure, while Mg atoms occupy the octahedral voids created by H atoms. This arrangement is characteristic of the rutile-type structure [36].

The introduction of a mono-vacancy induces local lattice distortions and minor alterations in the lattice parameters. For our mono-vacancy-defective supercell, corresponding to a $4.17\%$ vacancy concentration, the optimized lattice parameters are $a=b=4.486$ Å, $c=3.047$ Å, corresponding to a $0.57\%$ decrease and a $1.03\%$ increase in the $a/b$ and c parameters, respectively. The supercell volume contracts by $6.49\%$ and the density decreases from 1.424 g/cm³ to 1.366 g/cm³.

Figure 3 illustrates the local atomic arrangements on the (110) and ($1\overline{1}0$) planes containing the vacancy. The atomic arrangements remain largely consistent with the standard configuration, deviating minimally except in the region immediately surrounding the vacancy. Consequently, the lattice’s overall integrity and stability are preserved.

Table 2. Atomic distance comparison between pristine and vacancy-defective MgH₂ crystal.

Interatomic Distances 1	In Pristine Crystal (Å)	In Defective Crystal (Å)	Deviations
${\mathrm{L}}_{1a}$	1.9403	1.8238	−6.39%
${\mathrm{L}}_{1b}$		1.9796	1.99%
${\mathrm{L}}_{1c}$		2.1126	8.16%
${\mathrm{L}}_{2a}$	1.9587	1.8589	−5.37%
${\mathrm{L}}_{2b}$		1.9129	−2.39%
${\mathrm{L}}_{2c}$		1.8994	−3.12%
${\mathrm{L}}_{2d}$		1.8553	−5.57%

¹ The notation ${\mathrm{L}}_{1a}$, ${\mathrm{L}}_{1b}$ … ${\mathrm{L}}_{2d}$ are presented in Figure 3.

presents the variations in interatomic distances within the first coordination sphere surrounding the vacancy site. On the same (001) plane as the vacancy site, the interatomic distance ${\mathrm{L}}_{1c}$ shows the largest increase of $8.16\%$. The ${\mathrm{L}}_{1b}$ distance, one atomic layer from the vacancy, increases by $1.99\%$. All the interlayer distances (${\mathrm{L}}_{2a}$, ${\mathrm{L}}_{2b}$ … ${\mathrm{L}}_{2d}$) decrease, with a maximum reduction of $5.57\%$. These results suggest that changes in interatomic distances are predominantly localized on the (001) crystallographic plane containing the vacancy.

${\mathrm{L}}_1$ represents the distance between Mg atoms and their first-neighbor H atoms, effectively describing the Mg-H bond length. Thus, on the (001) plane with the vacancy, the Mg-H bond length increases by up to $8.16\%$, while on the adjacent (001) plane, the increase is $1.99\%$. To investigate the energetic implications of these bond length changes, we analyze the ${\mathrm{L}}_{1c}$ bond, which exhibits the most substantial length increase. $\Delta E_{bond}$ is defined as the change in bond energy of ${\mathrm{L}}_{1c}$ induced by the vacancy to quantify the energetic effects of structural modifications in the crystal. We compare two energy states using Equation (1),

$$\Delta E_{bond}=E_v-E_p$$

(1)

where $\Delta E_{bond}$ represents the change in bond energy of ${\mathrm{L}}_{1c}$, $E_v$ denotes the total energy of the defective crystal with ${\mathrm{L}}_{1c}$ at its new equilibrium length of 2.1126 Å, and $E_p$ denotes the total energy of the crystal with ${\mathrm{L}}_{1c}$ at its original equilibrium length of 1.9403 Å.

Our calculations indicate that the bond energy of ${\mathrm{L}}_{1c}$ decreases by 0.065 eV. This result confirms that the vacancy reduces the bond energy of surrounding Mg-H bonds, with the maximum reduction of 0.065 eV occurring on the (001) plane containing the vacancy.

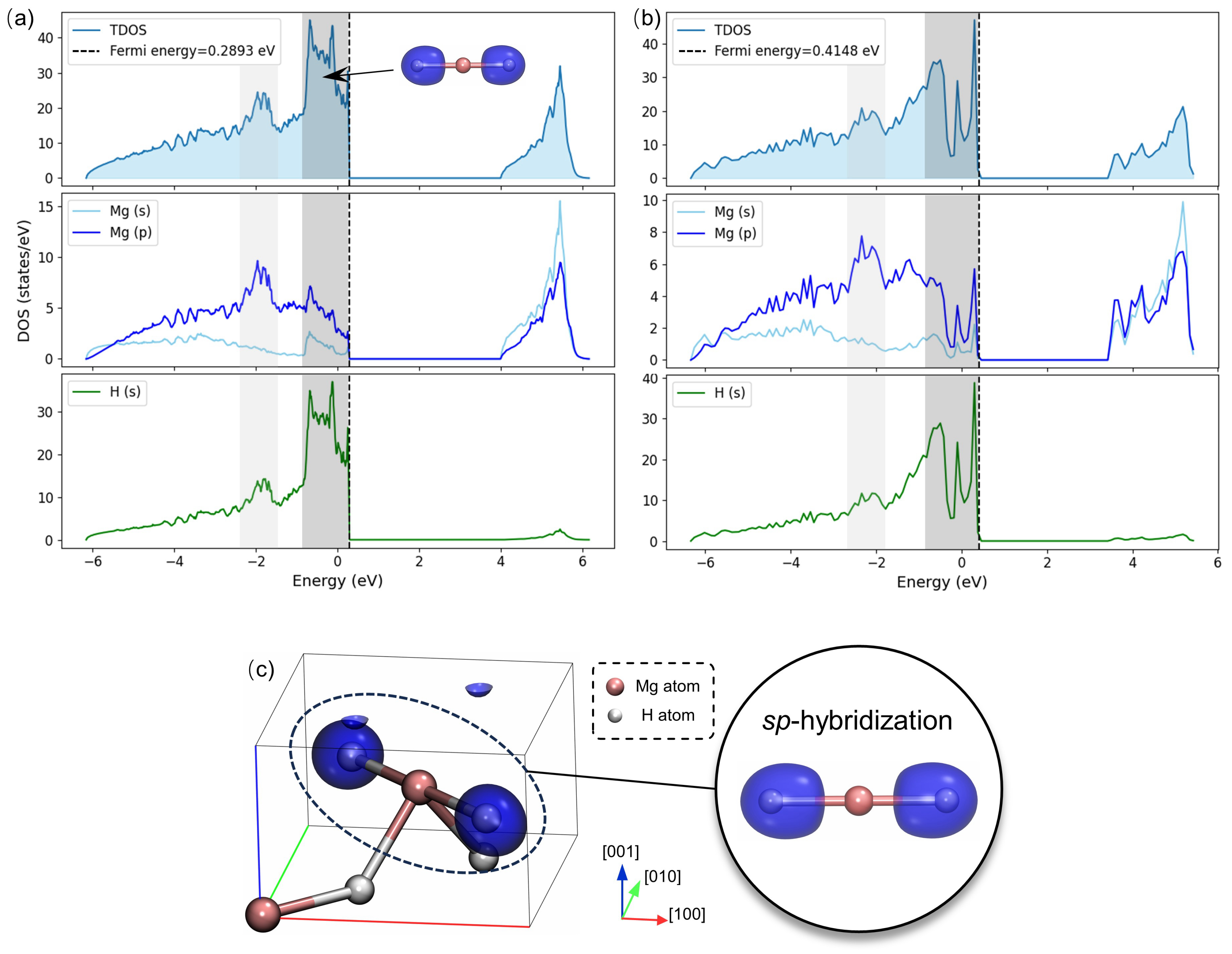
3.2. Electronic Structures and Bonding Nature

The incorporation of a mono-vacancy in MgH₂ substantially modifies its local electronic structure and bonding nature, impacting H atom stability within the system. These modifications were investigated through analysis of the density of states (DOS) and electron localization functions (ELF) for both pristine and vacancy-defective MgH₂ crystals.

Figure 4a,b illustrate the total density of states (TDOS) and projected density of states (PDOS) for pristine and vacancy-defective MgH₂ crystals, depicting the distribution of total electronic states and orbital-projected states as a function of energy, respectively. For the pristine crystal, the TDOS below the Fermi level (0.2893 eV) exhibits multiple peaks corresponding to occupied states, while a prominent peak above the Fermi level signifies conduction band states. A band gap of 3.72 eV is observed. Previous studies report band gaps for MgH₂ crystals ranging from 3.372 eV to 5.104 eV, depending on the pseudopotentials and exchange–correlation functionals employed in DFT calculations [35,37,38]. Our calculated band gap is consistent with reported values, corroborating the semiconductor properties of MgH₂.

The PDOS analysis reveals that both Mg s- and p-states occupy the energy range from −6 eV to the Fermi level, with significant contributions between −1 eV and the Fermi level. H s-states predominantly occupy the energy range from −1 eV to the Fermi level, exhibiting a prominent peak that aligns with Mg s- and p-states, as highlighted in dark gray in Figure 4a. This alignment indicates strong interactions and suggests sp-hybridization character of Mg-H bonding. Furthermore, notable hybridization peaks between Mg p-states and H s-states are observed at −2 eV, as highlighted in light gray.

Figure 4c illustrates sp-hybridization derived from wavefunctions of the crystal, exhibiting a distorted spherical morphology character of sp-hybridization. The 180° symmetric configuration of the H-Mg-H bond aligns with the geometric characters of the sp-hybridization, reflecting a linear arrangement that is typical for such hybridization. This configuration results in a strong and directional bond.

Analysis of the DOS and orbital visualization reveals the complex nature of the Mg-H bond, exhibiting both covalent (sp-hybridization) and ionic (electron distribution) properties, characterizing it as a strong polar covalent bond. These findings corroborate previous study that determined ionic charges of ${\mathrm{Mg}}^{1.91+}$ and ${\mathrm{H}}^{0.26-}$ using X-ray synchrotron radiation [39].

In vacancy-defective MgH₂ crystal, while the general DOS profile is preserved, peak intensities are diminished (Figure 4b). Concurrently, the band gap narrows from 3.72 eV to 3.05 eV and the Fermi level elevates from 0.2893 eV to 0.4148 eV, indicating a localized enhancement in metallic character and additional electronic states.

These electronic structure changes are complemented by insights from the ELF, which serves as an effective tool for elucidating electron pair distribution and bonding characters [40]. Figure 5a illustrates the ELF isosurfaces within the crystal, where the green isosurfaces denote moderate electron localization (ELF = 0.5) and the red isosurfaces signify high localization (ELF = 0.99). The red isosurfaces surrounding H atoms indicate substantial electron transfer from Mg to H, underscoring the Mg atom’s function as an electron donor and the pronounced polarity of the bonds.

The ELF cross-sections of the (001) plane in the pristine crystal (left half of Figure 5b) reveal spherical and well-defined separations, indicating an isotropic nature of the Mg-H bond within this plane. While on the (110) and ($1\overline{1}0$) planes, the non-spherical morphology of the red regions demonstrates an anisotropic character. Upon introduction of the vacancy, the right half of Figure 5b exhibits distortions and elongations of high ELF regions towards the vacancy site. The vacancy induces localized asymmetry in electron distribution, modifying electron localization around H atoms and promoting partial electron delocalization in its proximity.

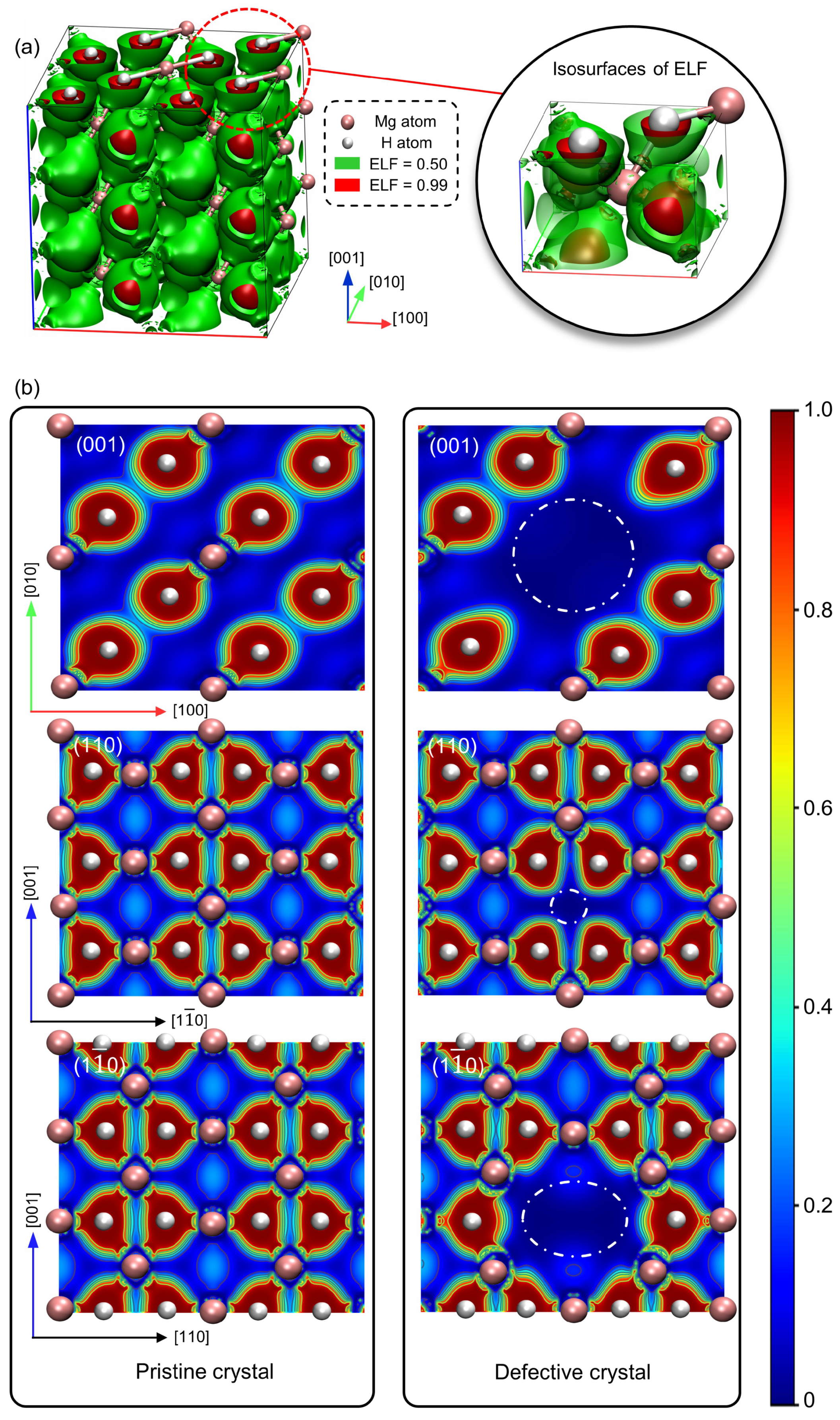
3.3. The Vacancy-Affected Zone (VAZ)

To elucidate the local effects of mono-vacancy introduction in MgH₂ crystal, we perform a comprehensive investigation of the VAZ. This study integrates analyses of the deformation charge density (DCD) and potential energy distribution (PED) for both pristine and vacancy-defective crystals. The DCD analysis provides crucial insights into the local redistribution of electrons, which are essential for predicting reactivity and bonding characters, while the PED examination reveals changes in the local energy distribution [41]. By combining these complementary approaches, we gain a multifaceted understanding of the electronic and energetic perturbations induced by the vacancy.

The DCD is calculated as Equation (2),

$$\Delta \rho ={\rho }_v-{\rho }_p$$

(2)

where $\Delta \rho$ represents the DCD, ${\rho }_v$ denotes the electron density within the MgH₂ crystal containing the mono-vacancy, and ${\rho }_p$ denotes the electron density of the pristine crystal.

Figure 6 illustrates a three-dimensional representation and cross-sections of the DCD on the (001), (110), and ($1\overline{1}0$) crystallographic planes of the vacancy-defective crystal. Negative values of DCD denote regions of decreased electron density. The red isosurface in Figure 6a corresponds to a DCD value of 0, indicating negligible electron density changes beyond these boundaries. The volume encompassed by the red isosurface is 26.505 ų, encompassing the negative value region of the DCD space. Detailed analysis of the isosurface, particularly in the vicinity of the vacancy site, reveals an elongated dumbbell shape aligned with the bond axis, corresponding to the electron density distribution in the H-Mg-H bonding structure.

The green isosurfaces (corresponding to a DCD value of −0.15 a.u.) demarcate regions of substantial electron depletion, localized in the vicinity of the missing Mg and H atoms. The vacancy induces electron depletion at its site, with effects constrained within the volume enclosed by the red isosurface, indicating that the mono-vacancy’s influence on the electronic structure is predominantly localized to the immediate vicinity of the defect.

Following the analysis of the DCD, we now direct our attention to the PED, which refers to the distribution of potential energy within the lattice. This allows us to visualize and examine the localized variations of potential energy within a three-dimensional framework. The potential utilized in this study refers to the total effective potential experienced by electrons within the crystal lattice, calculated according to Equation (3) [42],

$$V_{\mathrm{tot}}=V_{\mathrm{bare}}+V_H+V_{\mathrm{xc}}$$

(3)

where $V_{\mathrm{tot}}$ represents the total effective potential, $V_{\mathrm{bare}}$ denotes the bare ion potential, $V_H$ denotes the Hartree potential, and $V_{\mathrm{xc}}$ denotes the exchange–correlation potential.

Figure 7a presents a three-dimensional illustration of a red isosurface corresponding to a potential value of 0.1 eV in the defective MgH₂ crystal. This isosurface encompasses a volume of 19.514 ų, within which the potential values exceed 0.1 eV. A closer examination of the isosurface reveals its complex structure, illustrating the spatial distribution and magnitude of the potential energy perturbation induced by the vacancy defect. The isosurface delineates a localized high-potential region with a peak energy of 0.354 eV, affecting atomic diffusion pathways or serving as a trap for other defects.

Figure 7b illustrates PED cross-sections along various crystallographic planes of the defective crystal. A central high-energy region, demarcated by a yellow contour line (potential value of 0.1 eV), corresponds to the localized high-potential area depicted in Figure 7a. To enhance the comprehensibility of the PED, three-dimensional visualizations are provided, positioned adjacent to each cross-sectional illustration. These illustrations reveal that the prominent local high-potential region is surrounded by continuous low-energy domains. This spatial energy distribution indicates a clear demarcation between the high-energy region and the more expansive, lower-energy regions encompassing it. This configuration suggests a structured energy distribution within the crystal lattice, where high-potential regions may serve as energy barriers, while low-energy regions offer more favorable pathways for atomic migration.

4. Discussion

The incorporation of a mono-vacancy in MgH₂ crystal causes a cascade of structural and electronic perturbations. The crystal lattice undergoes a minor distortion, characterized by a 0.57% decrease in the $a/b$ lattice parameters and a 1.03% increase in the c parameter. In terms of electronic properties, noticeable modifications occur in the band structure: the band gap narrows by 0.67 eV and the Fermi level elevates by approximately 0.12 eV. These changes suggest a trend towards enhanced metallic character and the emergence of additional electronic states. Notwithstanding these alterations, the overall crystalline structure and the general profile of the DOS remain largely preserved, indicating an intrinsic stability of the MgH₂ crystal.

The pristine MgH₂ crystal exhibits strong polar covalent Mg-H bonds, with anisotropic character on various crystallographic planes. This anisotropy stems from the directional nature of the sp-hybridization, particularly in the H-Mg-H bonding configuration. A mono-vacancy alters the bonding nature in the defect’s immediate vicinity, enhancing the polarity of nearby Mg-H bonds. These changes are primarily localized on the (001) crystallographic plane containing the vacancy, where the Mg-H bond length increases by up to $8.16\%$ and the bond energy decreases by 0.065 eV.

The formation of a distinct VAZ is quantified by volumes of 26.505 ų for electron density changes and 19.514 ų for potential energy modifications. The DCD analysis reveals substantial electron depletion within this zone, suggesting that while the mono-vacancy’s influence is spatially limited, it induces significant local distortions. These distortions manifest as variations in surrounding the vacancy site, underscoring the substantial impact of even localized defects on the material’s atomic structure.

Alternatively, the VAZ can be characterized as a localized high-energy region induced by the mono-vacancy, which is typically considered a energy barrier, as delineated through the PED analysis. The region, exhibiting a peak energy of 0.354 eV, demarcates an energy barrier surrounding the vacancy site. This barrier hinders atomic motion and diffusion. However, it may also function as a trapping locale for additional defects.

While this localized energy barrier impedes atomic motion and diffusion, it is surrounded by continuous low-energy regions, as shown in Figure 7b, which facilitate a more conducive environment for atomic migration. These low-energy regions constitute preferred diffusion pathways for H atoms, as atoms inherently favor lower energy trajectories. From the perspective of defect engineering, vacancy-induced energy barriers present an opportunity to modulate the energy distribution within the MgH₂ lattice. Vacancies enable the creation of a network of energy barriers, guiding atomic movement along predetermined pathways and enhancing the performance in hydrogen diffusion.

5. Conclusions

(1)

The incorporation of a mono-vacancy in the MgH₂ crystal results in a 0.57% reduction of the $a/b$ lattice parameters and a 1.03% expansion of the c parameter. Notwithstanding these alterations, the overall crystalline structure remains largely intact.

(2)

Mg-H bonds exhibit strong polar covalent characters with anisotropic effects. The mono-vacancy enhances the polarity of adjacent bonds. These alterations are predominantly localized on the (001) plane, resulting in an increase of up to $8.16\%$ in bond length and a decrease of 0.065 eV in bond energy.

(3)

The VAZ encompasses volumes of 26.505 ų for electron density depletion and 19.514 ų for potential energy modifications.

(4)

The mono-vacancy generates a localized high-potential region, characterized by a peak energy of 0.354 eV. This barrier is encompassed by low-energy regions that offer more energetically favorable pathways for H atom migration.