Crystal Structure, Microstructure, and Dielectric and Electrical Properties of Ceramic Material Prepared Using Volcanic Ash
Abstract
1. Introduction
2. Materials and Methods
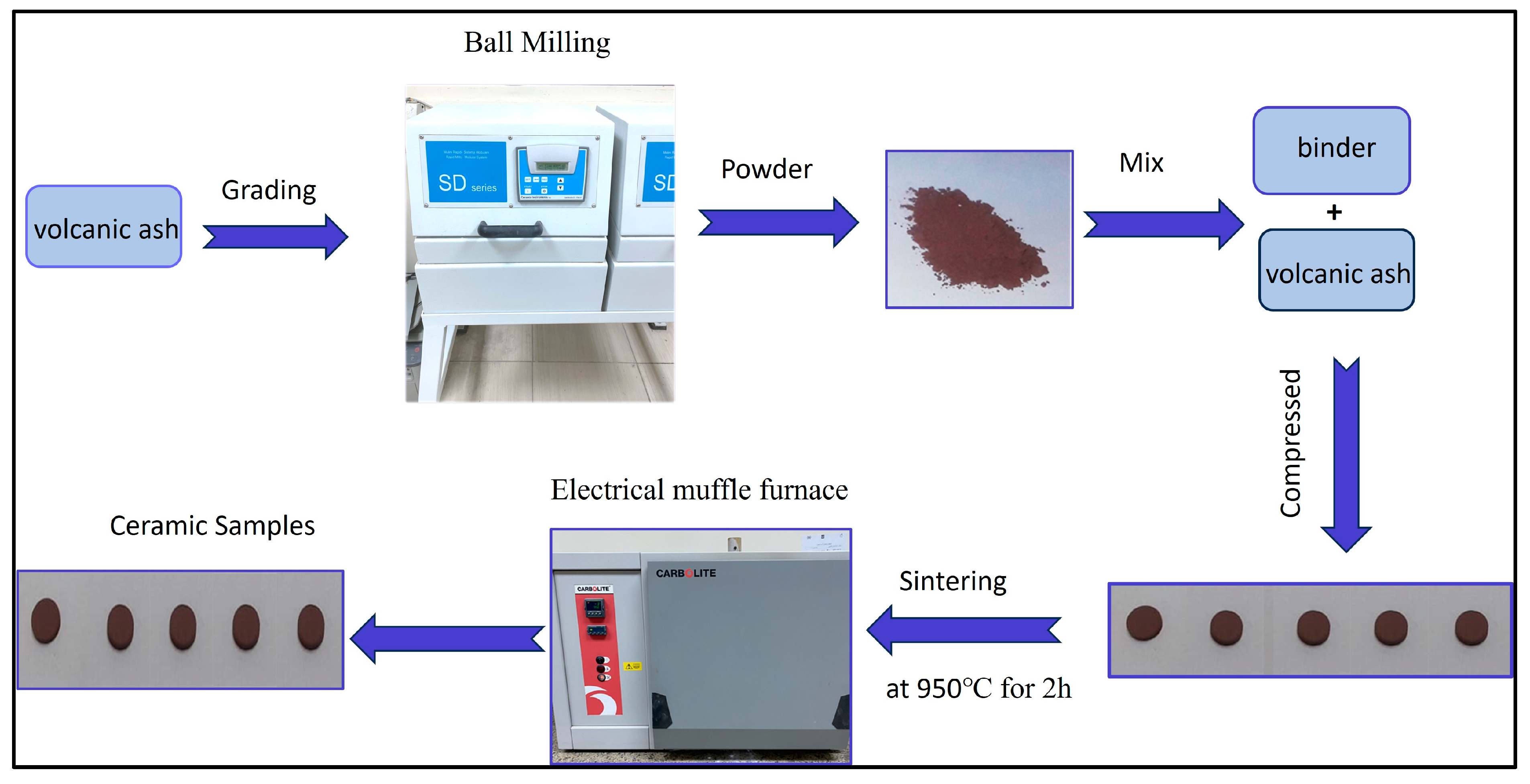
2.1. Materials and Ceramic Sample Preparation
2.2. Chemical Composition and Differential Thermal Analysis (DTA)
2.3. XRD Analysis and SEM
2.4. Electrical and Dielectric Measurements
3. Results and Discussion
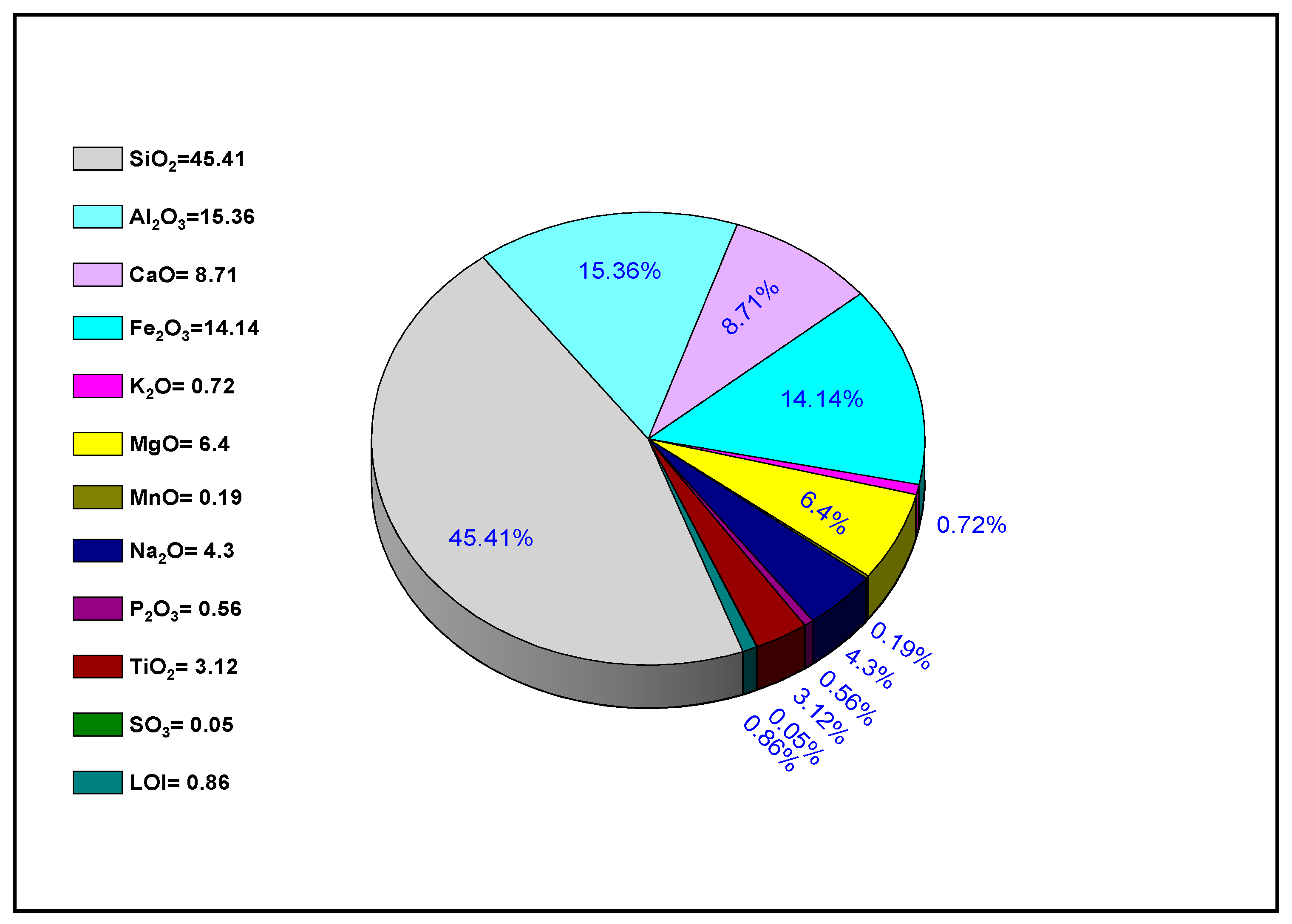
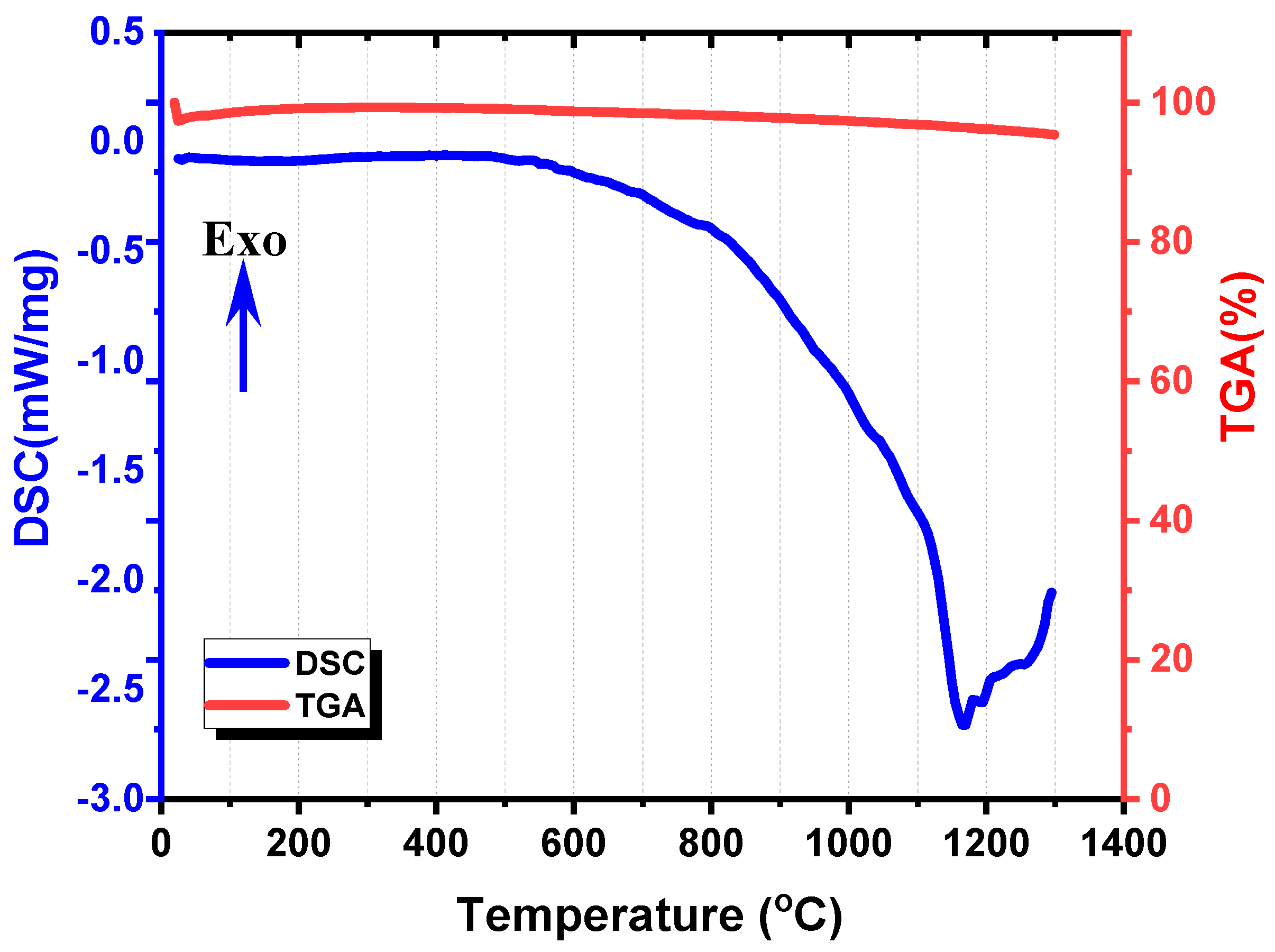
3.1. Chemical Composition and Thermal Analyses of Volcanic Ash
3.2. XRD and SEM Analysis
3.3. Dielectric and Electrical Properties
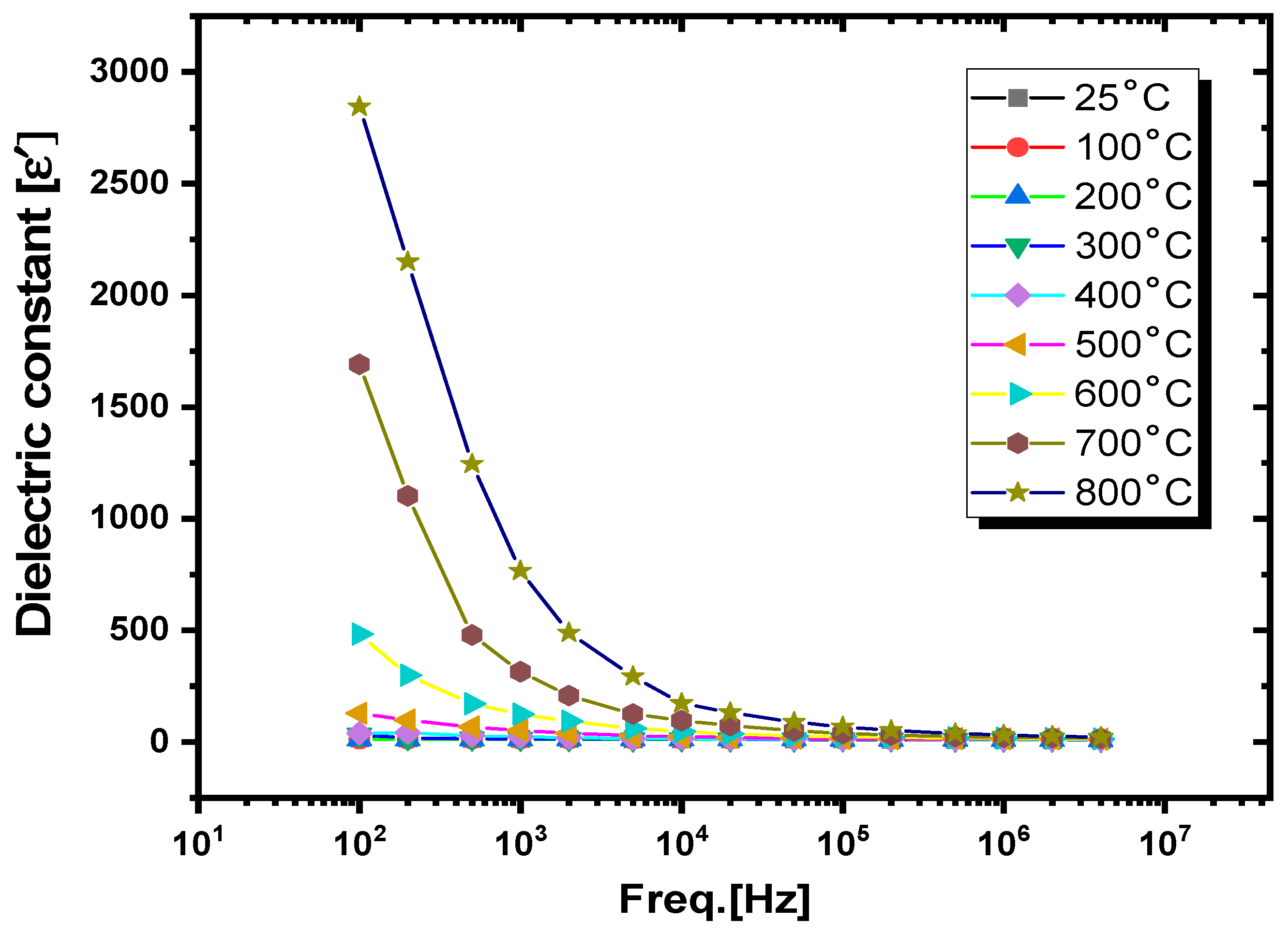
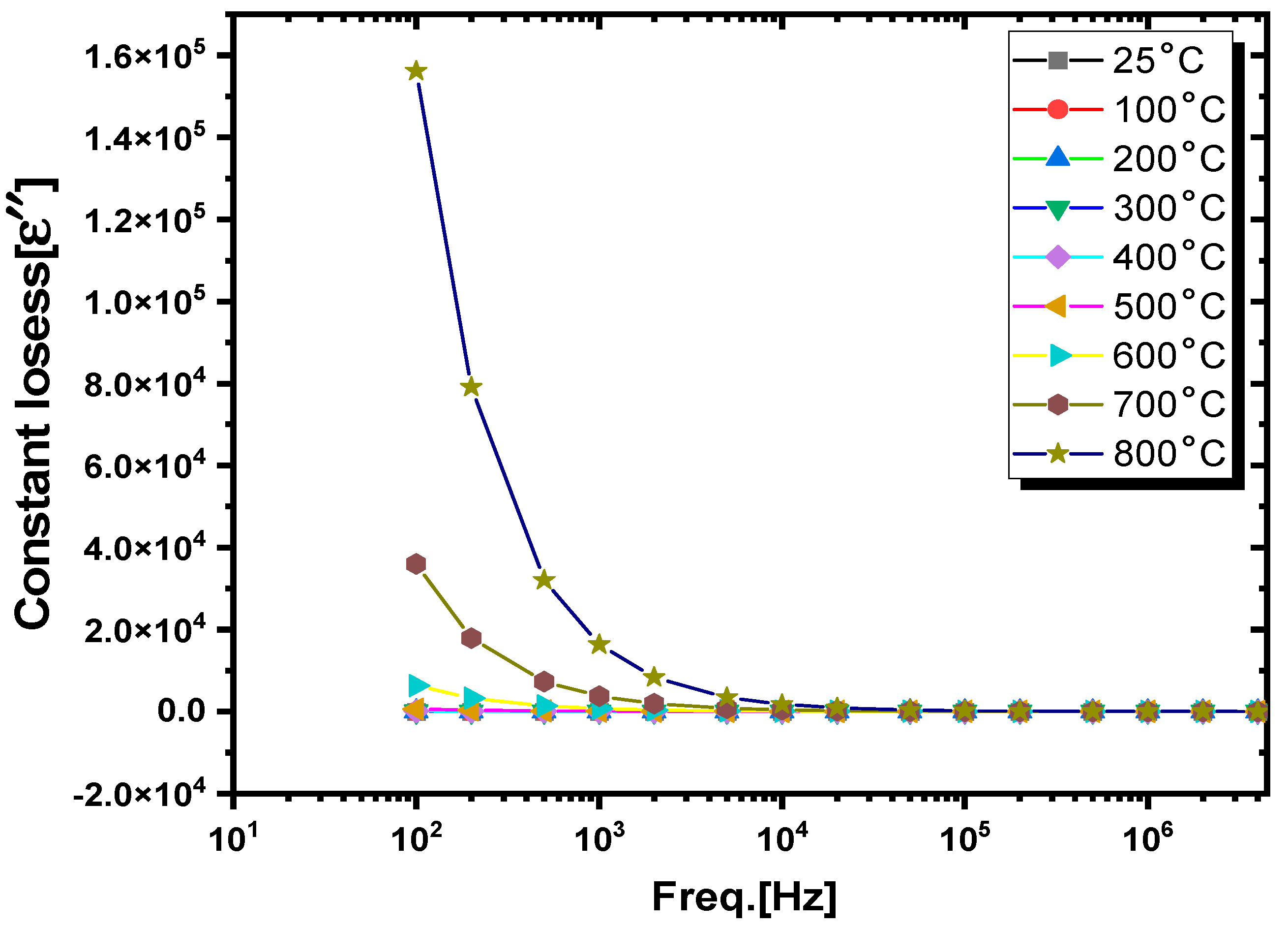
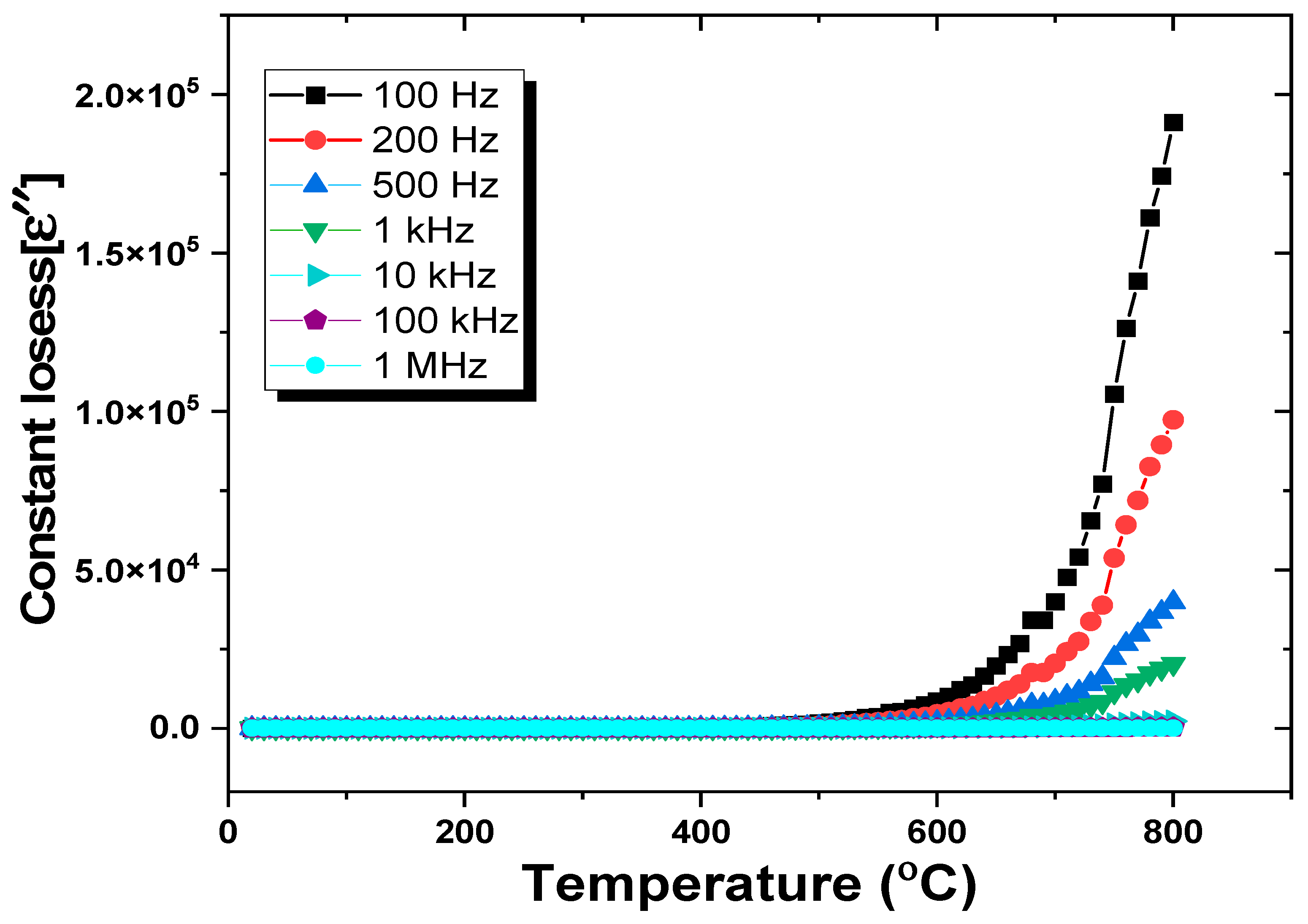
3.3.1. Dielectric Constants and Dielectric Losses
- (i).
- Frequency-dependent dielectric analysis
- (ii).
- Temperature-dependent dielectric analysis
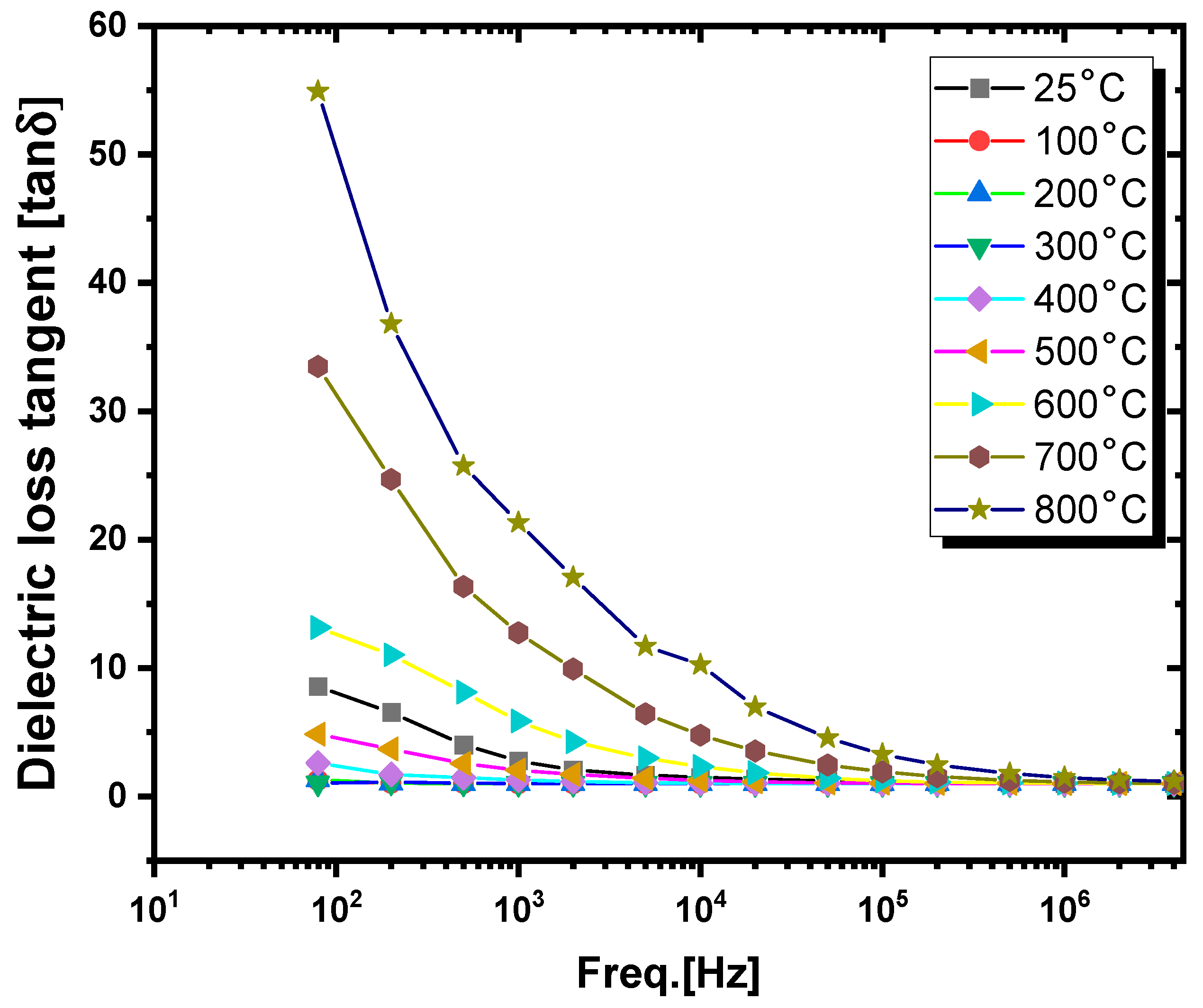
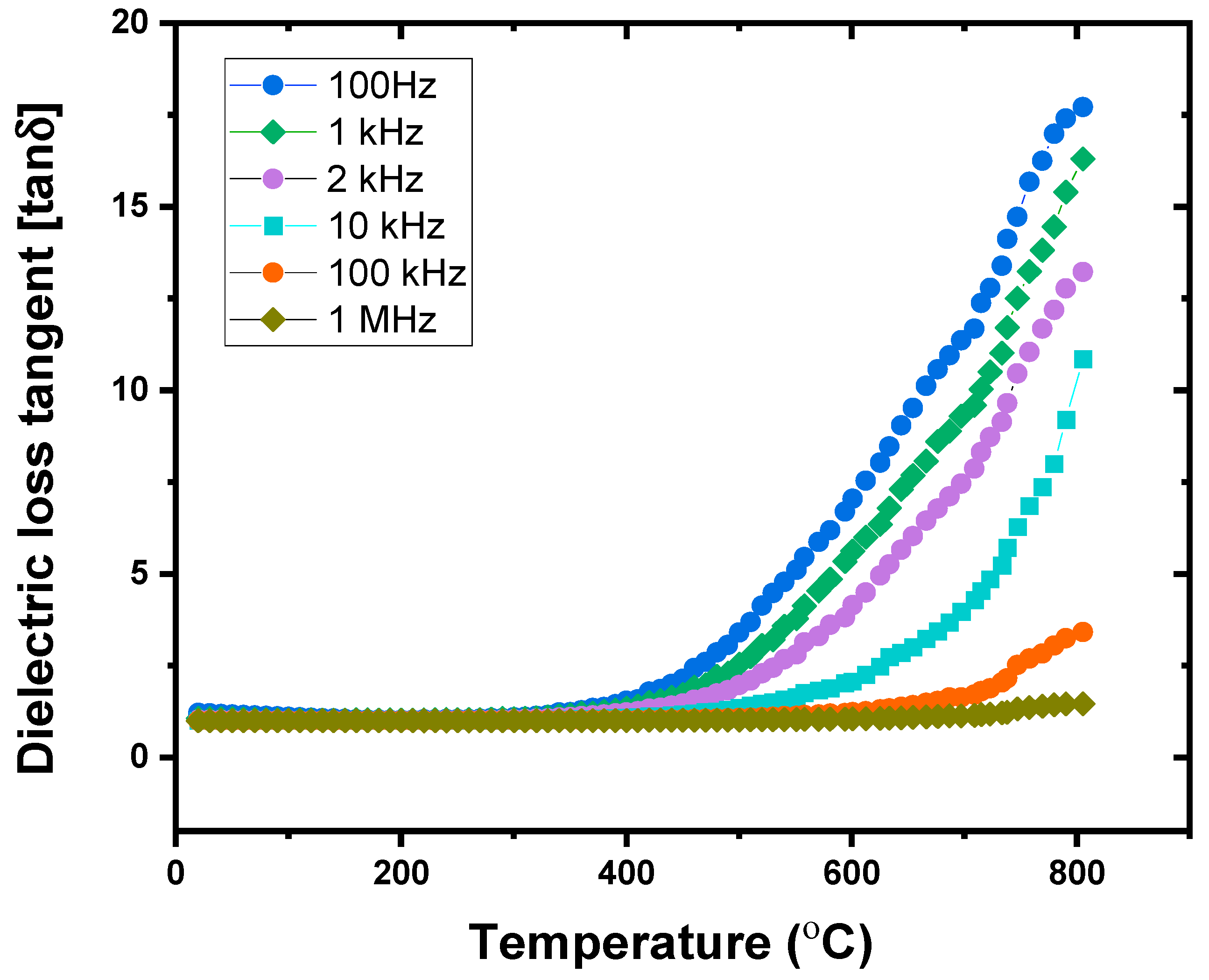
3.3.2. Tangent Dielectric Loss Study
- (i).
- Frequency-dependent dielectric analysis
- (ii).
- Temperature-dependent dielectric analysis
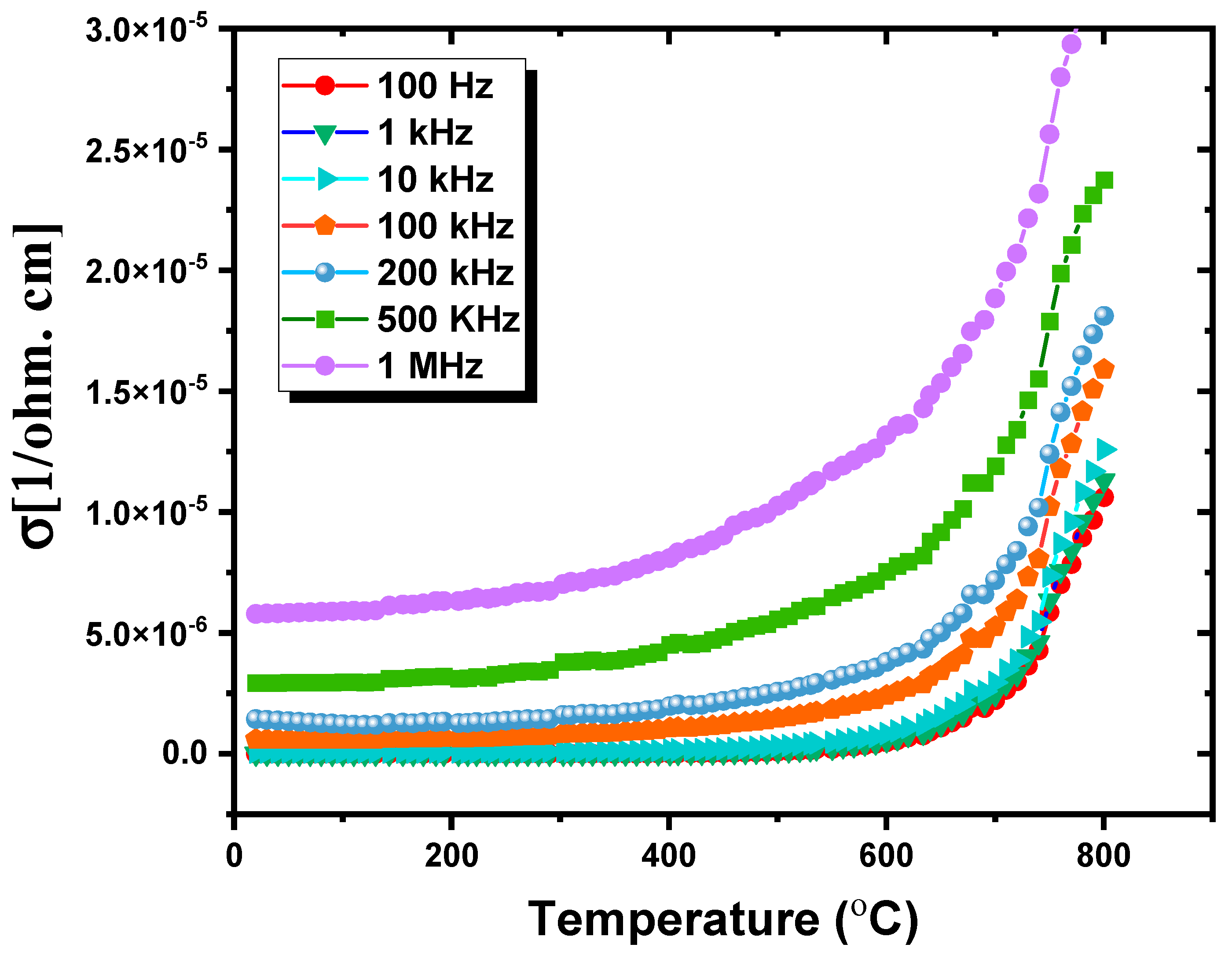
3.3.3. AC Conductivity Study
4. Conclusions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Otitoju, T.A.; Okoye, P.U.; Chen, G.; Li, Y.; Okoye, M.O.; Li, S. Advanced ceramic components: Materials, fabrication, and applications. J. Ind. Eng. Chem. 2020, 85, 34–65. [Google Scholar] [CrossRef]
- Khater, G.A.; Nabawy, B.S.; El-Kheshen, A.A.; Abdel-Baki, M.; Farag, M.M.; Abd Elsatar, A.G. Preparation and characterization of low-cost wollastonite and gehlenite ceramics based on industrial wastes. Constr. Build. Mater. 2021, 310, 125214. [Google Scholar] [CrossRef]
- Quesada, D.E.; Villarejo, L.P.; Soto, P.S. (Eds.) Ceramic Materials: Synthesis, Characterization, Applications and Recycling; BoD–Books on Demand: Norderstedt, Germany, 2019. [Google Scholar]
- Khater, G.A.; Nabawy, B.S.; El-Kheshen, A.A.; Abdel-Baki, M.; Farag, M.M. Utilizing of solid waste materials for producing porous and lightweight ceramics. Mater. Chem. Phys. 2022, 280, 125784. [Google Scholar] [CrossRef]
- Tchadjie, L.N.; Ekolu, S.O. Enhancing the reactivity of aluminosilicate materials toward geopolymer synthesis. J. Mater. Sci. 2018, 53, 4709–4733. [Google Scholar] [CrossRef]
- Alraddadi, S.; Assaedi, H. Thermal and mechanical properties of glass–ceramics based on slate and natural raw materials. Silicon 2023, 15, 1871–1882. [Google Scholar] [CrossRef]
- Siddique, R. Effect of volcanic ash on the properties of cement paste and mortar. Resour. Conserv. Recycl. 2011, 56, 66–70. [Google Scholar] [CrossRef]
- Zheng, Y.; Wang, S.; Ouyang, Z.; Zou, Y.; Liu, J.; Li, C.; Li, X.; Feng, J. CAS-1 lunar soil simulant. Adv. Space Res. 2009, 43, 448–454. [Google Scholar] [CrossRef]
- Takeda, H.; Hashimoto, S.; Kanie, H.; Honda, S.; Iwamoto, Y. Fabrication and characterization of hardened bodies from Japanese volcanic ash using geopolymerization. Ceram. Int. 2014, 40, 4071–4076. [Google Scholar] [CrossRef]
- Ndjock, B.I.D.L.; Elimbi, A.; Cyr, M. Rational utilization of volcanic ashes based on factors affecting their alkaline activation. J. Non-Cryst. Solids 2017, 463, 31–39. [Google Scholar] [CrossRef]
- Lemougna, P.N.; Wang, K.-T.; Tang, Q.; Nzeukou, A.N.; Billong, N.; Melo, U.C.; Cui, X.-M. Review on the use of volcanic ashes for engineering applications. Resour. Conserv. Recycl. 2018, 137, 177–190. [Google Scholar] [CrossRef]
- Kamseu, E.; Boccaccini, D.N.; Sola, A.; Rizzuti, A.; Leonelli, C.; Melo, C.U.; Billong, N. Sintering behaviour, microstructure and mechanical properties of low quartz content vitrified ceramics using volcanic ash. Adv. Appl. Ceram. 2008, 107, 19–26. [Google Scholar] [CrossRef]
- Husain, A.; Kupwade-Patil, K.; Al-Aibani, A.F.; Abdulsalam, M.F. In situ electrochemical impedance characterization of cement paste with volcanic ash to examine early stage of hydration. Constr. Build. Mater. 2017, 133, 107–117. [Google Scholar] [CrossRef]
- Kupwade-Patil, K.; Chin, S.; Ilavsky, J.; Andrews, R.N.; Bumajdad, A.; Büyüköztürk, O. Hydration kinetics and morphology of cement pastes with pozzolanic volcanic ash studied via synchrotron-based techniques. J. Mater. Sci. 2018, 53, 1743–1757. [Google Scholar] [CrossRef]
- Bondar, D.; Lynsdale, C.; Milestone, N.; Hassani, N. Sulfate resistance of alkali activated pozzolans. Int. J. Concr. Struct. Mater. 2015, 9, 145–158. [Google Scholar] [CrossRef]
- Manfredini, T.; Hanuskova, M. Natural raw materials in ”traditional” ceramic manufacturing. J. Univ. Chem. Technol. Metall. 2012, 47, 465–470. [Google Scholar]
- Serra, M.F.; Conconi, M.S.; Suarez, G.; Aglietti, E.F.; Rendtorff, N.M. Volcanic ash as flux in clay based triaxial ceramic materials, effect of the firing temperature in phases and mechanical properties. Ceram. Int. 2015, 41, 6169–6177. [Google Scholar] [CrossRef]
- Candamano, S.; De Luca, P.; Garofalo, P.; Crea, F. Ceramic Materials Containing Volcanic Ash and Characterized by Photoluminescent Activity. Environments 2023, 10, 172. [Google Scholar] [CrossRef]
- Nasution, N.J.F.; Sembiring, K.; Sitorus, Z. Analysis of the Structure of Ceramic Materials of Clay, Mount Kelud Volcanic Ash and Sea Water. J. Phys. Conf. Ser. 2021, 2019, 012103. [Google Scholar] [CrossRef]
- Tassongwa, B.; Njoya, A.; Pouepene, R.A.M.; Mbog, M.B.; Kenfack, J.V.; Dongmo, A.K.; Wouatong, A.S.L. Effect of volcanic ashes addition on ceramic properties of clayey materials from Koutaba (Western Cameroon). Arab. J. Geosci. 2022, 15, 541. [Google Scholar] [CrossRef]
- Majó, M.; Svobodova-Sedlackova, A.; Fernández, A.I.; Calderón, A.; Barreneche, C. Evaluation of volcanic ash as a low-cost high-temperature thermal energy storage material for concentrated solar power. J. Energy Storage 2024, 89, 111729. [Google Scholar] [CrossRef]
- Alraddadi, S.; Assaedi, H. Characterization and potential applications of different powder volcanic ash. J. King Saud Univ. Sci. 2020, 32, 2969–2975. [Google Scholar] [CrossRef]
- Leonelli, C.; Kamseu, E.; Boccaccini, D.N.; Melo, U.C.; Rizzuti, A.; Billong, N.; Miselli, P. Volcanic ash as alternative raw materials for traditional vitrified ceramic products. Adv. Appl. Ceram. 2007, 106, 135–141. [Google Scholar] [CrossRef]
- Bloise, A. Thermal behaviour of actinolite asbestos. J. Mater. Sci. 2019, 54, 11784–11795. [Google Scholar] [CrossRef]
- Alraddadi, S.; Saeed, A.; Assaedi, H. Effect of thermal treatment on the structural, electrical, and dielectric properties of volcanic scoria. J. Mater. Sci. Mater. Electron. 2020, 31, 11688–11699. [Google Scholar] [CrossRef]
- Pritam; Arya, A.; Sharma, A.L. Dielectric relaxations and transport properties parameter analysis of novel blended solid polymer electrolyte for sodium-ion rechargeable batteries. J. Mater. Sci. 2019, 54, 7131–7155. [Google Scholar] [CrossRef]
- Sun, J.; Ahmed, R.; Wang, G.J.; Wang, S.T.; Wang, J.; Suhaib, S.A.; Xie, Y.M.; Bi, H.; Wang, C.C. Colossal dielectric behavior and dielectric anomalies in Sr2TiCrO6 ceramics. J. Mater. Sci. 2019, 54, 6323–6331. [Google Scholar] [CrossRef]
- Vyas, M.K.; Chandra, A. Synergistic effect of conducting and insulating fillers in polymer nanocomposite films for attenuation of X-band. J. Mater. Sci. 2019, 54, 1304–1325. [Google Scholar] [CrossRef]
- Barsoum, M. Fundamentals of Ceramics; CRC Press: Boca Raton, FL, USA, 2019. [Google Scholar]
- Verma, R.; Tiwari, S.P.; Kumari, R.; Srivastava, R. Study of enhancement in the dielectric and electrical properties of WO3-doped LiF nano-composite. J. Mater. Sci. 2018, 53, 4199–4208. [Google Scholar] [CrossRef]
- Oliveira, F.; Dencheva, N.; Martins, P.; Lanceros-Méndez, S.; Denchev, Z. A new approach for preparation of metal-containing polyamide/carbon textile laminate composites with tunable electrical conductivity. J. Mater. Sci. 2018, 53, 11444–11459. [Google Scholar] [CrossRef]
- Singh, S.; Kaur, A.; Kaur, P.; Singh, L. High-temperature dielectric relaxation and electric conduction mechanisms in a LaCoO3-modified Na0.5Bi0.5TiO3 system. ACS Omega 2023, 8, 25623–25638. [Google Scholar] [CrossRef]
- Salem, S.M.; Antar, E.M.; Mostafa, A.G.; Salem, S.M.; El-badry, S.A. Compositional dependence of the structural and dielectric properties of Li2O–GeO2–ZnO–Bi2O3–Fe2O3 glasses. J. Mater. Sci. 2011, 46, 1295–1304. [Google Scholar] [CrossRef]
- Khater, G.A.; Nabawy, B.S.; El-Kheshen, A.A.; Abdel Latif, M.A.B.; Farag, M.M. Use of arc furnace slag and ceramic sludge for the production of lightweight and highly porous ceramic materials. Materials 2022, 15, 1112. [Google Scholar] [CrossRef]
- Khater, G.A.; Nabawy, B.; Kang, J.; Yue, Y.; Mahmoud, M.A. Magnetic and Electrical Properties of Glass and Glass-Ceramics Based on Weathered Basalt. Silicon 2020, 12, 2921–2940. [Google Scholar] [CrossRef]
- Shanker, J.; Buchi Suresh, M.; Narsinga Rao, G.; Suresh Babu, D. Colossal dielectric, relaxor ferroelectric, diamagnetic and weak ferromagnetic properties of NdCrO3 perovskite nanoparticles. J. Mater. Sci. 2019, 54, 5595–5604. [Google Scholar] [CrossRef]
- Lin, J.-F.; Sturhahn, W.; Zhao, J.; Shen, G.; Mao, H.-k.; Hemley, R.J. Chapter 19—Nuclear resonant inelastic X-ray scattering and synchrotron Mössbauer spectroscopy with laser-heated diamond anvil cells. In Advances in High-Pressure Technology for Geophysical Applications; Chen, J., Wang, Y., Duffy, T.S., Shen, G., Dobrzhinetskaya, L.F., Eds.; Elsevier: Amsterdam, The Netherlands, 2005; pp. 397–411. [Google Scholar]
- Kremer, F.; Schönhals, A. (Eds.) Broadband Dielectric Spectroscopy; Springer Science & Business Media: Berlin/Heidelberg, Germany, 2002. [Google Scholar]
- Park, C.O.; Akbar, S.A. Ceramics for chemical sensing. J. Mater. Sci. 2003, 38, 4611–4637. [Google Scholar] [CrossRef]
- Pan, W.; Cao, M.; Diao, C.; Tao, C.; Hao, H.; Yao, Z.; Yu, Z.; Liu, H. Structures and dielectric properties of (Nb, Zn) co-doped SrTiO3 ceramics at various sintering temperatures. J. Mater. Sci. 2019, 54, 12401–12410. [Google Scholar] [CrossRef]
- Exner, J.; Kita, J.; Moos, R. In- and through-plane conductivity of 8YSZ films produced at room temperature by aerosol deposition. J. Mater. Sci. 2019, 54, 13619–13634. [Google Scholar] [CrossRef]
- Brok, E.; Frandsen, C.; Lefmann, K.; McEnroe, S.; Robinson, P.; Burton, B.P.; Hansen, T.C.; Harrison, R. Spin orientation in solid solution hematite-ilmenite. Am. Mineral. 2017, 102, 1234–1243. [Google Scholar] [CrossRef]




| Freq. (Hz) | RT | 300 °C | 400 °C | 500 °C | 600 °C | 800 °C | ||||||
|---|---|---|---|---|---|---|---|---|---|---|---|---|
| ε′ | σ′ | ε′ | σ′ | ε′ | σ′ | ε′ | σ′ | ε′ | σ′ | ε′ | σ′ | |
| 100 | 17.0 | 7.60 × 10−10 | 32.27 | 1.44 × 10−9 | 39.06 | 3.71 × 10−9 | 128.22 | 2.65 × 10−8 | 482.74 | 2.80 × 10−7 | 2845.17 | 8.68 × 10−6 |
| 1000 | 15.18 | 6.75 × 10−9 | 14.46 | 7.59 × 10−9 | 22.94 | 1.33 × 10−8 | 50.93 | 4.84 × 10−8 | 124.80 | 2.48 × 10−7 | 766.17 | 9.08 × 10−6 |
| 10,000 | 13.35 | 6.63 × 10−8 | 12.45 | 6.73 × 10−8 | 15.18 | 8.12 × 10−8 | 23.31 | 1.44 × 10−7 | 47.10 | 4.20 × 10−7 | 173.42 | 9.88 × 10−6 |
| 100,000 | 11.53 | 5.61 × 10−7 | 11.72 | 6.44 × 10−7 | 12.91 | 6.97 × 10−7 | 16.05 | 8.82 × 10−7 | 23.19 | 1.35 × 10−6 | 68.07 | 1.24 × 10−5 |
| 1,000,000 | 9.51 | 5.55 × 10−6 | 10.73 | 5.90 × 10−6 | 11.46 | 6.21 × 10−6 | 13.51 | 7.36 × 10−6 | 16.88 | 9.20 × 10−6 | 31.09 | 2.54 × 10−5 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the author. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Alraddadi, S. Crystal Structure, Microstructure, and Dielectric and Electrical Properties of Ceramic Material Prepared Using Volcanic Ash. Crystals 2024, 14, 817. https://doi.org/10.3390/cryst14090817
Alraddadi S. Crystal Structure, Microstructure, and Dielectric and Electrical Properties of Ceramic Material Prepared Using Volcanic Ash. Crystals. 2024; 14(9):817. https://doi.org/10.3390/cryst14090817
Chicago/Turabian StyleAlraddadi, Shoroog. 2024. "Crystal Structure, Microstructure, and Dielectric and Electrical Properties of Ceramic Material Prepared Using Volcanic Ash" Crystals 14, no. 9: 817. https://doi.org/10.3390/cryst14090817
APA StyleAlraddadi, S. (2024). Crystal Structure, Microstructure, and Dielectric and Electrical Properties of Ceramic Material Prepared Using Volcanic Ash. Crystals, 14(9), 817. https://doi.org/10.3390/cryst14090817






