Ferroelectric Liquid Crystal Dammann Grating by Patterned Photoalignment
Abstract
:1. Introduction
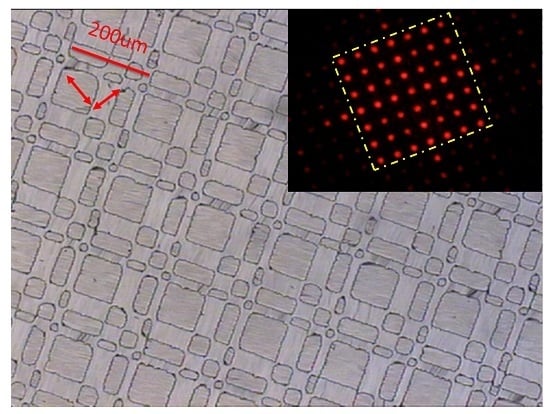
2. Results
3. Discussion
4. Materials and Methods
Acknowledgments
Author Contributions
Conflicts of Interest
References
- Yang, D.K.; Wu, S.T. Fundamentals of Liquid Crystal Devices, 2nd ed.; John Wiley & Sons: Chichester, UK, 2006. [Google Scholar]
- Cao, Z.; Xuan, L.; Hu, L.; Liu, Y.; Mu, Q.; Li, D. Investigation of optical testing with a phase-only liquid crystal spatial light modulator. Opt. Express 2005, 13, 1059–1065. [Google Scholar] [CrossRef] [PubMed]
- Efron, U. Spatial Light Modulator Technology: Materials, Devices, and Applications; Marcel Dekker: New York, NY, USA, 1994. [Google Scholar]
- Slussarenko, S.; Murauski, A.; Du, T.; Chigrinov, V.; Marrucci, L.; Santamato, E. Tunable liquid crystal q-plates with arbitrary topological charge. Opt. Express 2011, 19, 4085–4090. [Google Scholar] [CrossRef] [PubMed]
- Fan, F.; Du, T.; Srivastava, A.K.; Lu, W.; Chigrinov, V.; Kwok, H.S. Axially symmetric polarization converter made of patterned liquid crystal quarter wave plate. Opt. Express 2012, 20, 23036–23043. [Google Scholar] [CrossRef] [PubMed]
- Aerle, N.A.J.M.; Barmentlo, M.; Hollering, R.W.J. Effect of rubbing on the molecular orientation within polyimide orienting layers of liquid crystal displays. J. Appl. Phys. 1993, 74, 3111–3120. [Google Scholar] [CrossRef]
- Chigrinov, V.G.; Kozenkov, V.M.; Kwok, H.S. Photoalignment of Liquid Crystalline Materials; John Wiley & Sons: Chichester, UK, 2008. [Google Scholar]
- Armitage, D. Alignment of liquid crystals on obliquely evaporated silicon oxide films. J. Appl. Phys. 1980, 51, 2552–2555. [Google Scholar] [CrossRef]
- Schadt, M.; Seiberle, H.; Schuster, A.; Kelly, S.M. Photo-generation of linearly polymerized liquid crystal aligning layers comprising novel, integrated optically patterned retarders and color filters. Jpn. J. Appl. Phys. 1995, 34, 3240–3249. [Google Scholar] [CrossRef]
- Wu, Y.J.; Jeng, Y.S.; Yeh, P.C.; Hu, C.J.; Huang, W.M. 20.2: Stereoscopic 3D Display Using Patterned Retarder. In SID Symposium Digest of Technical Papers; Blackwell Publishing Ltd.: Oxford, UK, 2008; Volume 39, pp. 260–263. [Google Scholar]
- Tan, L.; Ho, J.Y.; Kwok, H.S. Extended Jones matrix method for oblique incidence study of polarization gratings. Appl. Phys. Lett. 2012, 101, 51107. [Google Scholar] [CrossRef]
- Oh, C.; Escuti, M.J. Achromatic diffraction from polarization gratings with high efficiency. Opt. Lett. 2008, 33, 2287–2289. [Google Scholar] [CrossRef] [PubMed]
- Gao, K.; Cheng, H.; Bhowmik, A.K.; Bos, P.J. Thin-film Pancharatnam lens with low f-number and high quality. Opt. Express 2015, 23, 26086. [Google Scholar] [CrossRef] [PubMed]
- Fan, F.; Srivastava, A.; Du, T.; Tseng, M.C.; Chigrinov, V.; Kwok, H.S. Low Voltage Tunable Liquid Crystal Lens. Opt. Lett. 2013, 38, 4116–4119. [Google Scholar] [CrossRef] [PubMed]
- Kim, J.; Li, Y.; Miskiewicz, M.N.; Oh, C.; Kudenov, M.W.; Escuti, M.J. Fabrication of ideal geometric-phase holograms with arbitrary wavefronts. Optica 2015, 2, 958–964. [Google Scholar] [CrossRef]
- Chen, P.; Ji, W.; Wei, B.; Hu, W.; Chigrinov, V.; Lu, Y. Generation of arbitrary vector beams with liquid crystal polarization converters and vector-photoaligned q-plates. Appl. Phys. Lett. 2015, 107, 241102. [Google Scholar] [CrossRef]
- Tzeng, Y.Y.; Ke, S.W.; Ting, C.L.; Fuh, A.Y.G.; Lin, T.H. Axially symmetric polarization converters based on photo-aligned liquid crystal films. Opt. Express 2008, 16, 3768–3775. [Google Scholar] [CrossRef] [PubMed]
- Kim, J.; Komanduri, R.K.; Lawler, K.F.; Kekas, D.J.; Escuti, M.J. Efficient and monolithic polarization conversion system based on a polarization grating. Appl. Opt. 2012, 51, 4852–4857. [Google Scholar] [CrossRef] [PubMed]
- Zhao, X.; Boussaid, F.; Bermak, A.; Chigrinov, V.G. Thin Photo-Patterned Micropolarizer Array for CMOS Image Sensors. IEEE Photonics Technol. Lett. 2009, 21, 805–807. [Google Scholar] [CrossRef]
- Dammann, H.; Klotz, E. Coherent Optical Generation and Inspection of Two-dimensional Periodic Structures. Opt. Acta 1977, 24, 505–515. [Google Scholar] [CrossRef]
- Dammann, H.; Görtler, K. High-efficiency in-line multiple imaging by means of multiple phase holograms. Opt. Commun. 1971, 3, 312–315. [Google Scholar] [CrossRef]
- Veldkamp, W.B.; Leger, J.R.; Swanson, G.J. Coherent summation of laser beams using binary phase gratings. Opt. Lett. 1986, 11, 303–305. [Google Scholar] [CrossRef] [PubMed]
- Morrison, R.L.; Walker, S.L.; Cloonan, T.J. Beam array generation and holographic interconnections in a free-space optical switching network. Appl. Opt. 1993, 32, 2512–2518. [Google Scholar] [CrossRef] [PubMed]
- Yu, J.; Zhou, C.; Jia, W.; Ma, J.; Hu, A.; Wu, J.; Wang, S. Distorted Dammann grating. Opt. Lett. 2013, 38, 474–476. [Google Scholar] [CrossRef] [PubMed]
- Davis, J.A.; Moreno, I.; Martínez, J.L.; Hernandez, T.J.; Cottrell, D.M. Creating three-dimensional lattice patterns using programmable Dammann gratings. Appl. Opt. 2011, 50, 3653–3657. [Google Scholar] [CrossRef] [PubMed]
- Lei, T.; Meng, Z.; Li, Y.; Ping, J.; Liu, G.N.; Xu, X.; Li, Z.; Min, C.; Jiao, L.; Yu, C. Massive individual orbital angular momentum channels for multiplexing enabled by Dammann gratings. Light Sci. Appl. 2015, 4, e257. [Google Scholar] [CrossRef]
- Ge, S.; Chen, P.; Ma, L.; Liu, Z.; Zheng, Z.; Shen, D.; Hu, W.; Lu, Y. Optical array generator based on blue phase liquid crystal Dammann grating. Opt. Mater. Express 2016, 6, 1087. [Google Scholar] [CrossRef]
- Jahns, J.; Downs, M.M.; Prise, M.E.; Streibi, N.; Walker, S.J. Dammann gratings for laser beam shaping. Opt. Eng. 1989, 28, 281267. [Google Scholar] [CrossRef]
- Chen, P.; Ge, S.J.; Ma, L.L.; Hu, W.; Chigrinov, V.; Lu, Y.Q.; Chen, P.; Ge, S.J.; Ma, L.L.; Hu, W. Generation of Equal-Energy Orbital Angular Momentum Beams via Photopatterned Liquid Crystals. Phys. Rev. Appl. 2016, 5, 044009. [Google Scholar] [CrossRef]
- Wang, X.; Srivastava, A.K.; Fan, F.; Zheng, Z.; Shen, D.; Chigrinov, V.G.; Kwok, H. Electrically/optically tunable photo-aligned hybrid nematic liquid crystal Dammann grating. Opt. Lett. 2016, 41, 5668–5671. [Google Scholar] [CrossRef] [PubMed]
- Shteyner, E.A.; Srivastava, A.K.; Chigrinov, V.G.; Kwok, H.S.; Afanasyev, A.D. Submicron-scale liquid crystal photo-alignment. Soft Matter 2013, 9, 5160–5165. [Google Scholar] [CrossRef]
- Srivastava, A.K.; Chigrinov, V.G.; Kwok, H.S. Ferroelectric liquid crystals: Excellent tool for modern displays and photonics. J. Soc. Inf. Disp. 2015, 23, 253–272. [Google Scholar] [CrossRef]
- Srivastava, A.K.; Hu, W.; Chigrinov, V.G.; Kiselev, A.D.; Lu, Y.Q. Fast switchable grating based on orthogonal photo alignments of ferroelectric liquid crystals. Appl. Phys. Lett. 2012, 101, 31112. [Google Scholar] [CrossRef]
- Wang, X.Q.; Fan, F.; Du, T.; Tam, A.M.; Ma, Y.; Srivastava, A.K.; Chigrinov, V.G.; Kwok, H.S. Liquid crystal Fresnel zone lens based on single-side-patterned photoalignment layer. Appl. Opt. 2014, 53, 2026–2029. [Google Scholar] [CrossRef] [PubMed]
- Hu, W.; Kumar Srivastava, A.; Lin, X.W.; Liang, X.; Wu, Z.J.; Sun, J.T.; Zhu, G.; Chigrinov, V.; Lu, Y.Q. Polarization independent liquid crystal gratings based on orthogonal photoalignments. Appl. Phys. Lett. 2012, 100, 111116. [Google Scholar] [CrossRef]
- Zhou, C.; Liu, L. Numerical study of Dammann array illuminators. Appl. Opt. 1995, 34, 5961–5969. [Google Scholar] [CrossRef] [PubMed]




© 2017 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license ( http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Fan, F.; Yao, L.; Wang, X.; Shi, L.; Srivastava, A.K.; Chigrinov, V.G.; Kwok, H.-S.; Wen, S. Ferroelectric Liquid Crystal Dammann Grating by Patterned Photoalignment. Crystals 2017, 7, 79. https://doi.org/10.3390/cryst7030079
Fan F, Yao L, Wang X, Shi L, Srivastava AK, Chigrinov VG, Kwok H-S, Wen S. Ferroelectric Liquid Crystal Dammann Grating by Patterned Photoalignment. Crystals. 2017; 7(3):79. https://doi.org/10.3390/cryst7030079
Chicago/Turabian StyleFan, Fan, Lishuang Yao, Xiaoqian Wang, Liangyu Shi, Abhishek K. Srivastava, Vladimir G. Chigrinov, Hoi-Sing Kwok, and Shuangchun Wen. 2017. "Ferroelectric Liquid Crystal Dammann Grating by Patterned Photoalignment" Crystals 7, no. 3: 79. https://doi.org/10.3390/cryst7030079
APA StyleFan, F., Yao, L., Wang, X., Shi, L., Srivastava, A. K., Chigrinov, V. G., Kwok, H. -S., & Wen, S. (2017). Ferroelectric Liquid Crystal Dammann Grating by Patterned Photoalignment. Crystals, 7(3), 79. https://doi.org/10.3390/cryst7030079