Analysis of Switching Current Data during Polarization Reversal in KTP Single Crystals with Surface Dielectric Layer
Abstract
:1. Introduction
2. Materials and Methods
3. Results
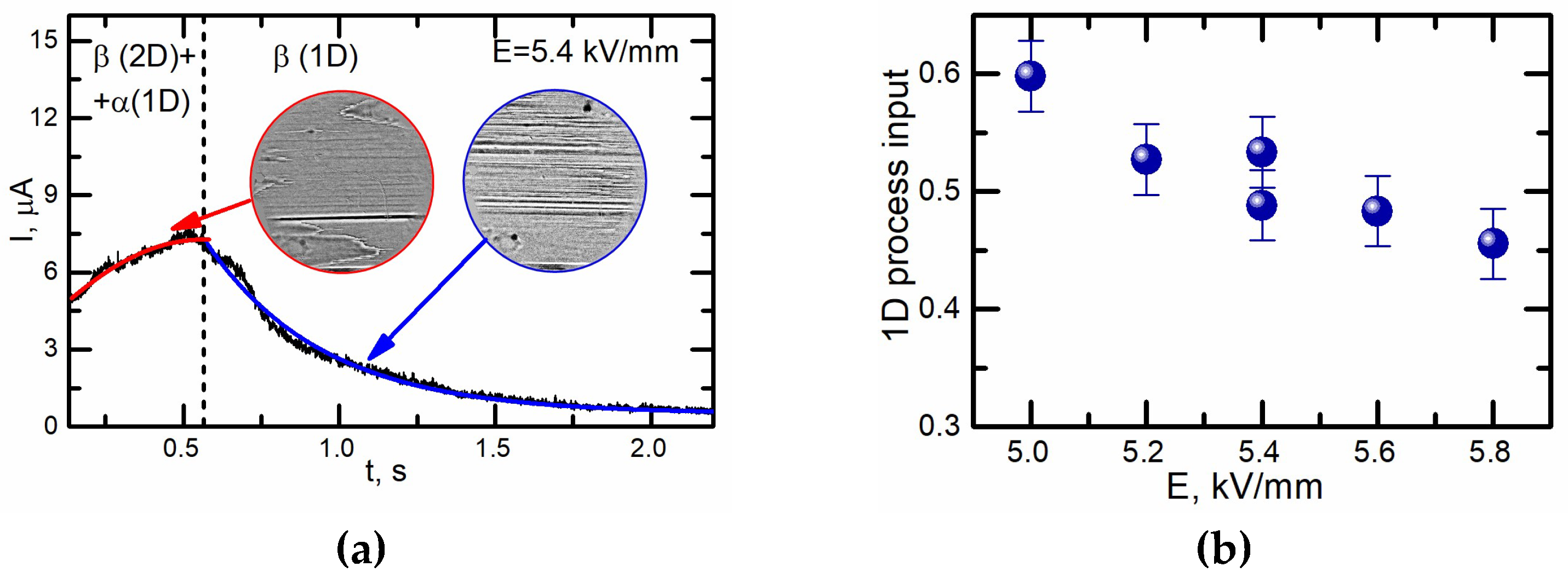
3.1. Polarization Reversal without Artificial Dielectric Layer
3.2. Polarization Reversal with Artificial Dielectric Layer
4. Discussion
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
References
- Tordjman, I.; Masse, R.; Guitel, J.C. Structure cristalline du monophosphate KTiPO5. Zeitschrift Für Kristallographie-Cryst. Mater. 1974, 139, 103–115. [Google Scholar] [CrossRef]
- Zumsteg, F.C.; Bierlein, J.D.; Gier, E.T. KxRb1−xTiOPO4: A new nonlinear optical material. J. Appl. Phys. 1976, 47, 4980–4985. [Google Scholar] [CrossRef]
- Yanovskii, V.K.; Voronkova, V.I. Ferroelectric phase transitions and properties of crystals of the KTiOPO4 family. Phys. Status Solidi 1986, 93, 665–668. [Google Scholar] [CrossRef]
- Bierlein, J.D.; Ahmed, F. Observation and poling of ferroelectric domains in KTiOPO4. Appl. Phys. Lett. 1987, 51, 1322–1324. [Google Scholar] [CrossRef]
- Rosenman, G.; Urenski, P.; Arie, A.; Roth, M.; Angert, N.; Skliar, A.; Tseitlin, M. Polarization reversal and domain grating in flux-grown KTiOPO4 crystals with variable potassium stoichiometry. Appl. Phys. Lett. 2000, 76, 3798–3800. [Google Scholar] [CrossRef]
- Urenski, P.; Rosenman, G.; Molotskii, M. Polarization reversal and domain anisotropy in flux-grown KTiOPO4 and isomorphic crystals. J. Mater. Res. 2001, 16, 1493–1499. [Google Scholar] [CrossRef]
- Canalias, C.; Hirohashi, J.; Pasiskevicius, V.; Laurell, F. Polarization-switching characteristics of flux-grown KTiOPO4 and RbTiOPO4 at room temperature. J. Appl. Phys. 2005, 97, 124105. [Google Scholar] [CrossRef]
- Canalias, C.; Pasiskevicius, V. Mirrorless optical parametric oscillator. Nat. Photonics 2007, 1, 459–462. [Google Scholar] [CrossRef]
- Canalias, C.; Pasiskevicius, V.; Fokine, M.; Laurell, F. Backward quasi-phase-matched second-harmonic generation in submicrometer periodically poled flux-grown KTiOPO4. Appl. Phys. Lett. 2005, 86, 181105. [Google Scholar] [CrossRef]
- Chuchumishev, D.; Marchev, G.; Buchvarov, I.; Pasiskevicius, V.; Laurell, F.; Petrov, V. High-energy picosecond OPO based on PPKTP. Laser Phys. 2013, 10, 115404. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Marchev, G.; Dallocchio, P.; Pirzio, F.; Agnesi, A.; Reali, G.; Petrov, V.; Tyazhev, A.; Pasiskevicius, V.; Thilmann, N.; Laurell, F. Sub-nanosecond, 1–10 kHz, low-threshold, non-critical OPOs based on periodically poled KTP crystal pumped at 1.064 nm. Appl. Phys. B 2012, 109, 211–214. [Google Scholar] [CrossRef]
- Chaitanya Kumar, S.; Das, R.; Samanta, G.K.; Ebrahim-Zadeh, M. Optimally-output-coupled, 17.5 W, fiber-laser-pumped continuous-wave optical parametric oscillator. Appl. Phys. B 2010, 102, 31–35. [Google Scholar] [CrossRef]
- Canalias, C.; Pasiskevicius, V.; Laurell, F. Periodic poling of KTiOPO4: From micrometer to sub-micrometer domain gratings. Ferroelectrics 2006, 340, 27–47. [Google Scholar] [CrossRef]
- Mu, X.; Zotova, I.B.; Ding, Y.J.; Risk, W.P. Backward second-harmonic generation in submicron-period ion-exchanged KTiOPO4 waveguide. Opt. Commun. 2000, 181, 153–159. [Google Scholar] [CrossRef]
- Gu, X.; Makarov, M.; Ding, Y.J.; Khurgin, J.B.; Risk, W.P. Backward second-harmonic and third-harmonic generation in a periodically poled potassium titanyl phosphate waveguide. Opt. Lett. 1999, 24, 127–129. [Google Scholar] [CrossRef] [PubMed]
- Arie, A.; Rosenman, G.; Mahal, V.; Skliar, A.; Oron, M.; Katz, M.; Eger, D. Green and ultraviolet quasi-phase-matched second harmonic generation in bulk periodically-poled KTiOPO4. Opt. Commun. 1997, 142, 265–268. [Google Scholar] [CrossRef]
- Karlsson, H.; Laurell, F.; Cheng, L.K. Periodic poling of RbTiOPO4 for quasi-phase matched blue light generation. Appl. Phys. Lett. 1999, 74, 1519–1521. [Google Scholar] [CrossRef]
- Shur, V.Y.; Pelegova, E.V.; Akhmatkhanov, A.R.; Baturin, I.S. Periodically poled crystals of KTP family: A review. Ferroelectrics 2016, 496, 49–69. [Google Scholar] [CrossRef]
- Shur, V.Y.; Akhmatkhanov, A.R.; Chuvakova, M.A.; Dolbilov, M.A.; Zelenovskiy, P.S.; Lobov, A.I. Formation of self-organized domain structures with charged domain walls in lithium niobate with surface layer modified by proton exchange. J. Appl. Phys. 2017, 121, 104101. [Google Scholar] [CrossRef]
- Shur, V.Y.; Neradovskiy, M.M.; Dolbilov, M.A.; Lobov, A.I.; Zelenovskiy, P.S.; Ushakov, A.D.; Ushakova, E.S.; Quillier, E.; Baldi, P.; De Micheli, M.P. Formation of broad domain boundary in congruent lithium niobate modified by proton exchange. Ferroelectrics 2015, 476, 146–155. [Google Scholar] [CrossRef]
- Dolbilov, M.A.; Shur, V.Y.; Shishkina, E.V.; Angudovich, E.S.; Ushakov, A.D.; Baldi, P.; de Micheli, M.P. Formation of nanodomain structure in front of the moving domain wall in lithium niobate single crystal modified by proton exchange. Ferroelectrics 2013, 442, 82–91. [Google Scholar] [CrossRef]
- Akhmatkhanov, A.R.; Shur, V.Y.; Baturin, I.S.; Zorikhin, D.V.; Lukmanova, A.M.; Zelenovskiy, P.S.; Neradovskiy, M.M. Domain kinetics in lithium niobate single crystals with photoresist dielectric layer. Ferroelectrics 2012, 439, 3–12. [Google Scholar] [CrossRef]
- Shur, V.Y.; Rumyantsev, E.L. Kinetics of ferroelectric domain structure during switching: Theory and experiment. Ferroelectrics 1994, 151, 171–180. [Google Scholar] [CrossRef]
- Shur, V.Y. Kinetics of ferroelectric domains: Application of general approach to LiNbO3 and LiTaO3. J. Mater. Sci. 2006, 41, 199–210. [Google Scholar] [CrossRef]
- Shur, V.; Rumyantsev, E.; Makarov, S. Kinetics of phase transformations in real finite systems: Application to switching in ferroelectrics. J. Appl. Phys. 1998, 84, 445–451. [Google Scholar] [CrossRef]
- Shur, V.Y.; Rumyantsev, E.L.; Makarov, S.D.; Volegov, V.V. How to extract information about domain kinetics in thin ferroelectric films from switching transient current data. Integr. Ferroelectr. 1994, 5, 293–301. [Google Scholar] [CrossRef]
- Kolmogorov, A.N. On the statistical theory of the crystallization of metals. Bull. Acad. Sci. USSR, Math. Ser 1937, 1, 355–359. [Google Scholar]
- Avrami, M. Kinetics of phase change. I General theory. J. Chem. Phys. 1939, 7, 1103–1112. [Google Scholar] [CrossRef]
- Ishibashi, Y.; Takagi, Y. Note on ferroelectric domain switching. J. Phys. Soc. JPN 1971, 31, 506–510. [Google Scholar] [CrossRef]
- Chezganov, D.S.; Vlasov, E.O.; Gimadeeva, L.V.; Chuvakova, M.A.; Shur, V.Y. Domain formation induced in congruent lithium niobate by electron beam irradiation. In Proceedings of the 14th Russia/CIS/Baltic/Japan Symposium on Ferroelectricity, Saint-Petersburg, Russia, 14–18 May 2018. [Google Scholar]
- Akhmatkhanov, A.R.; Vaskina, E.M.; Chuvakova, M.A.; Pelegova, E.V.; Shur, V.Y. Investigation of polarization reversal and analysis of switching current data in KTP single crystals. Ferroelectrics 2017, 508, 1–8. [Google Scholar] [CrossRef]
- Shur, V.Y.; Vaskina, E.M.; Pelegova, E.V.; Chuvakova, M.A.; Akhmatkhanov, A.R.; Kizko, O.V.; Ivanov, M.; Kholkin, A.L. Domain wall orientation and domain shape in KTiOPO4 crystals. Appl. Phys. Lett. 2016, 109, 132901. [Google Scholar] [CrossRef]
- Shur, V.Y.; Nikolaeva, E.V.; Shishkin, E.I.; Chernykh, A.P.; Terabe, K.; Kitamura, K.; Ito, H.; Nakamura, K. Domain shape in congruent and stoichiometric lithium tantalate. Ferroelectrics 2002, 269, 195–200. [Google Scholar] [CrossRef]
- Shur, V.Y.; Nikolaeva, E.V.; Shishkin, E.I.; Kozhevnikov, V.L.; Chernykh, A.P.; Terabe, K.; Kitamura, K. Polarization reversal in congruent and stoichiometric lithium tantalate. Appl. Phys. Lett. 2001, 79, 3146–3148. [Google Scholar] [CrossRef]
- Shur, V.Y.; Gruverman, A.L.; Ponomarev, N.Y.; Rumyantsev, E.L.; Tonkacheva N., A. Domain structure kinetics in ultrafast polarization switching in lead germanate. JETP Lett. 1991, 53, 615–619. [Google Scholar]
- Shur, V.Y.; Rumyantsev, E.L. Kinetics of ferroelectric domain structure: Retardation effects. Ferroelectrics 1997, 191, 319–333. [Google Scholar] [CrossRef]
- Shur, V.Y.; Kosobokov, M.S.; Mingaliev, E.A.; Karpov, V.R.; Lobov, A.I.; Makaev, A.V. Self-assembled formation of quasi-regular finger structure at the domain wall induced by multiple IR pulse laser irradiation of lithium niobate. Acta Mater. 2018, submitted. [Google Scholar]
- Pryakhina, V.I.; Alikin, D.O.; Negashev, S.A.; Shur, V.Y. Domain kinetics in LiNbO3 and LiTaO3 with modified bulk conductivity. Ferroelectrics 2016, 496, 79–84. [Google Scholar] [CrossRef]
- Ro, J.H.; Cha, M. Subsecond relaxation of internal field after polarization reversal in congruent LiNbO3 and LiTaO3 crystals. Appl. Phys. Lett. 2000, 77, 2391–2393. [Google Scholar] [CrossRef]
- Shur, V.Y.; Akhmatkhanov, A.R.; Baturin, I.S.; Nebogatikov, M.S.; Dolbilov, M.A. Complex study of bulk screening processes in single crystals of lithium niobate and lithium tantalate family. Phys. Solid State 2010, 52, 2147–2153. [Google Scholar] [CrossRef]
- Shur, V.Y.; Akhmatkhanov, A.R.; Baturin, I.S. Micro- and nano-domain engineering in lithium niobate. Appl. Phys. Rev. 2015, 2, 040604. [Google Scholar] [CrossRef]









© 2018 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Akhmatkhanov, A.; Vaskina, E.; Gachegova, E.; Shur, V. Analysis of Switching Current Data during Polarization Reversal in KTP Single Crystals with Surface Dielectric Layer. Crystals 2018, 8, 315. https://doi.org/10.3390/cryst8080315
Akhmatkhanov A, Vaskina E, Gachegova E, Shur V. Analysis of Switching Current Data during Polarization Reversal in KTP Single Crystals with Surface Dielectric Layer. Crystals. 2018; 8(8):315. https://doi.org/10.3390/cryst8080315
Chicago/Turabian StyleAkhmatkhanov, Andrey, Ekaterina Vaskina, Ekaterina Gachegova, and Vladimir Shur. 2018. "Analysis of Switching Current Data during Polarization Reversal in KTP Single Crystals with Surface Dielectric Layer" Crystals 8, no. 8: 315. https://doi.org/10.3390/cryst8080315
APA StyleAkhmatkhanov, A., Vaskina, E., Gachegova, E., & Shur, V. (2018). Analysis of Switching Current Data during Polarization Reversal in KTP Single Crystals with Surface Dielectric Layer. Crystals, 8(8), 315. https://doi.org/10.3390/cryst8080315





