Revisit the Role of Steps/Disconnections on Misfit Cancellation at Semi-Coherent Interface—Bridging the O-Line Model and the Topological Model
Abstract
:1. Introduction
2. Materials and Methods
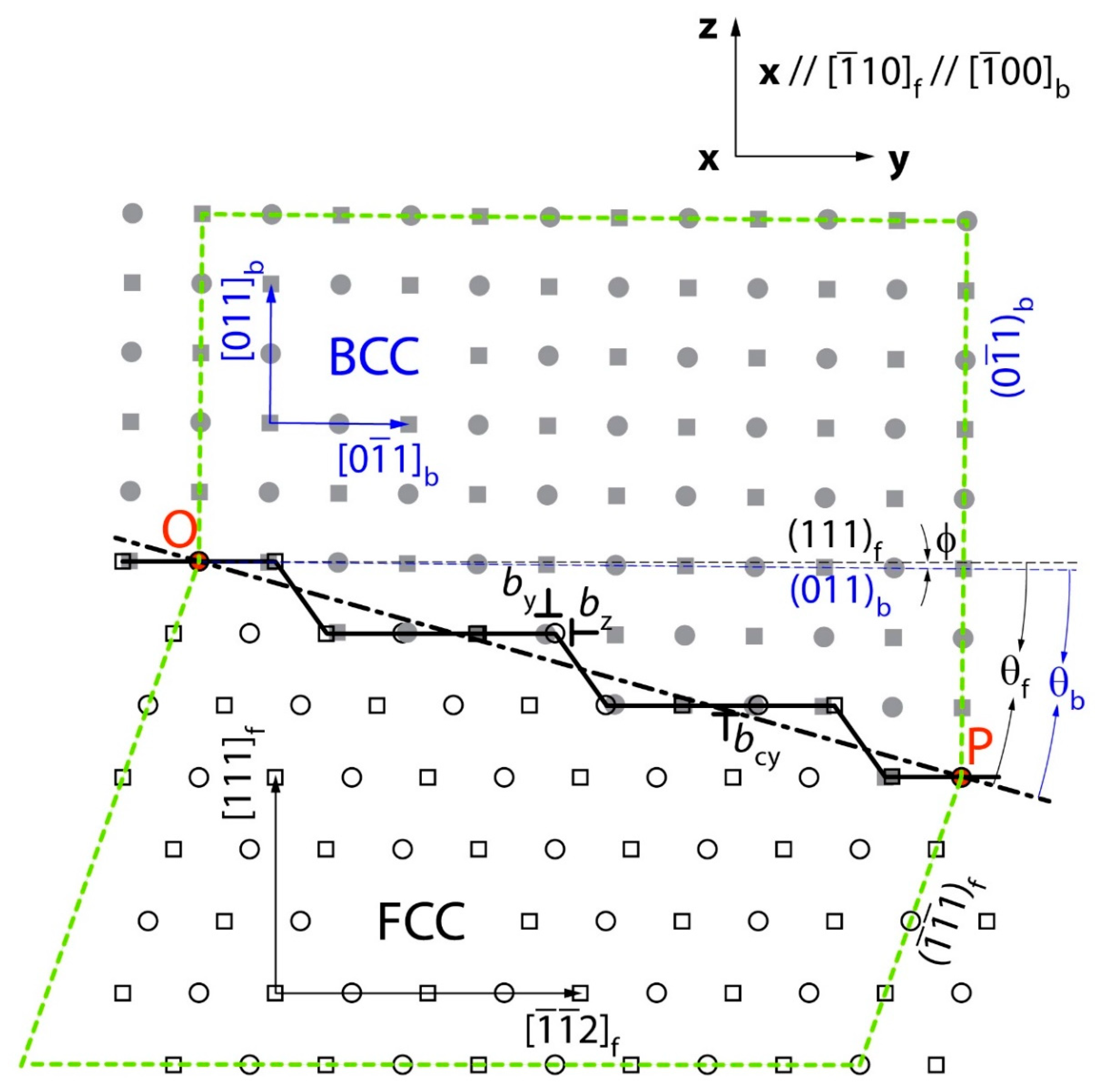
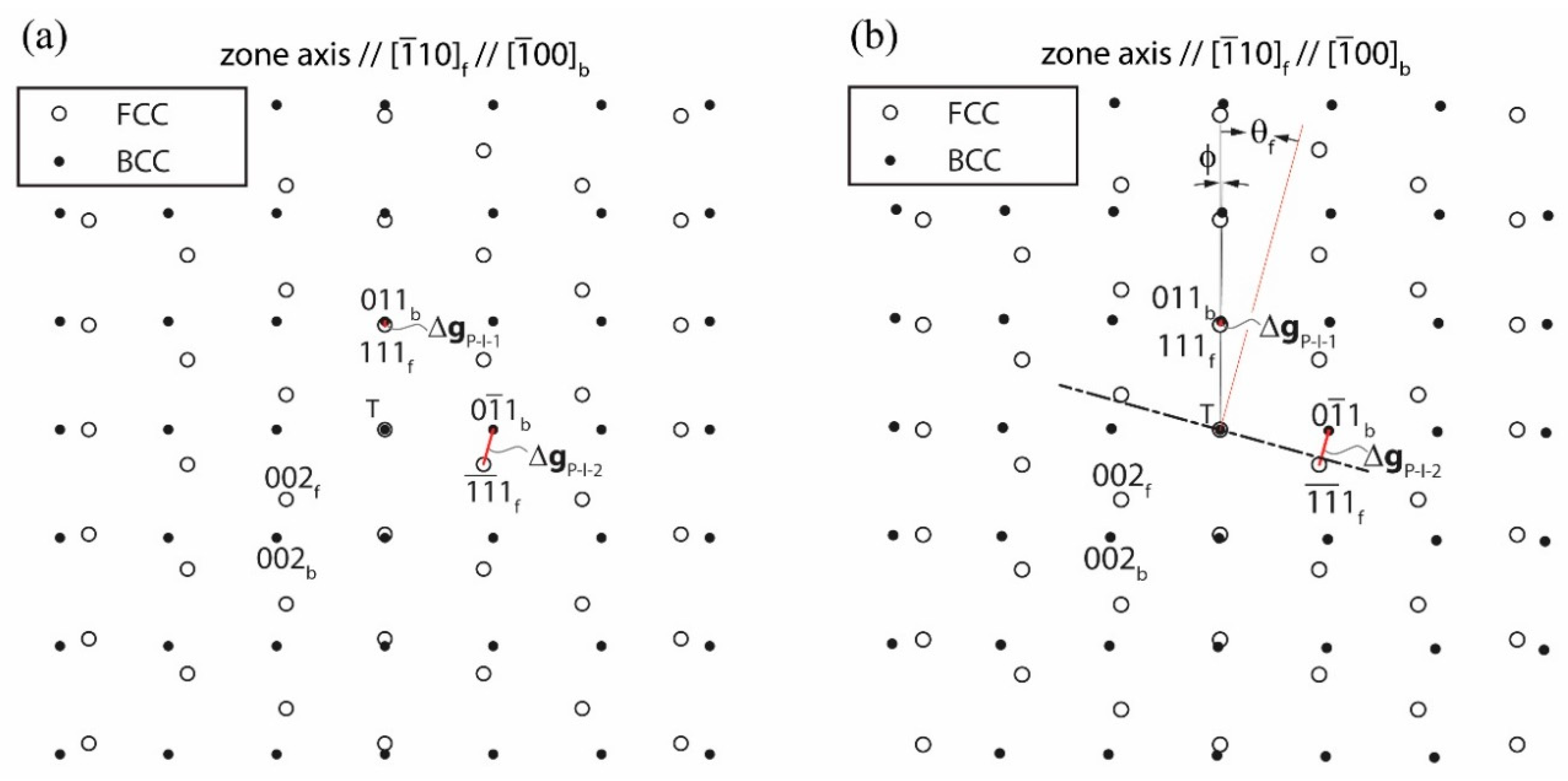
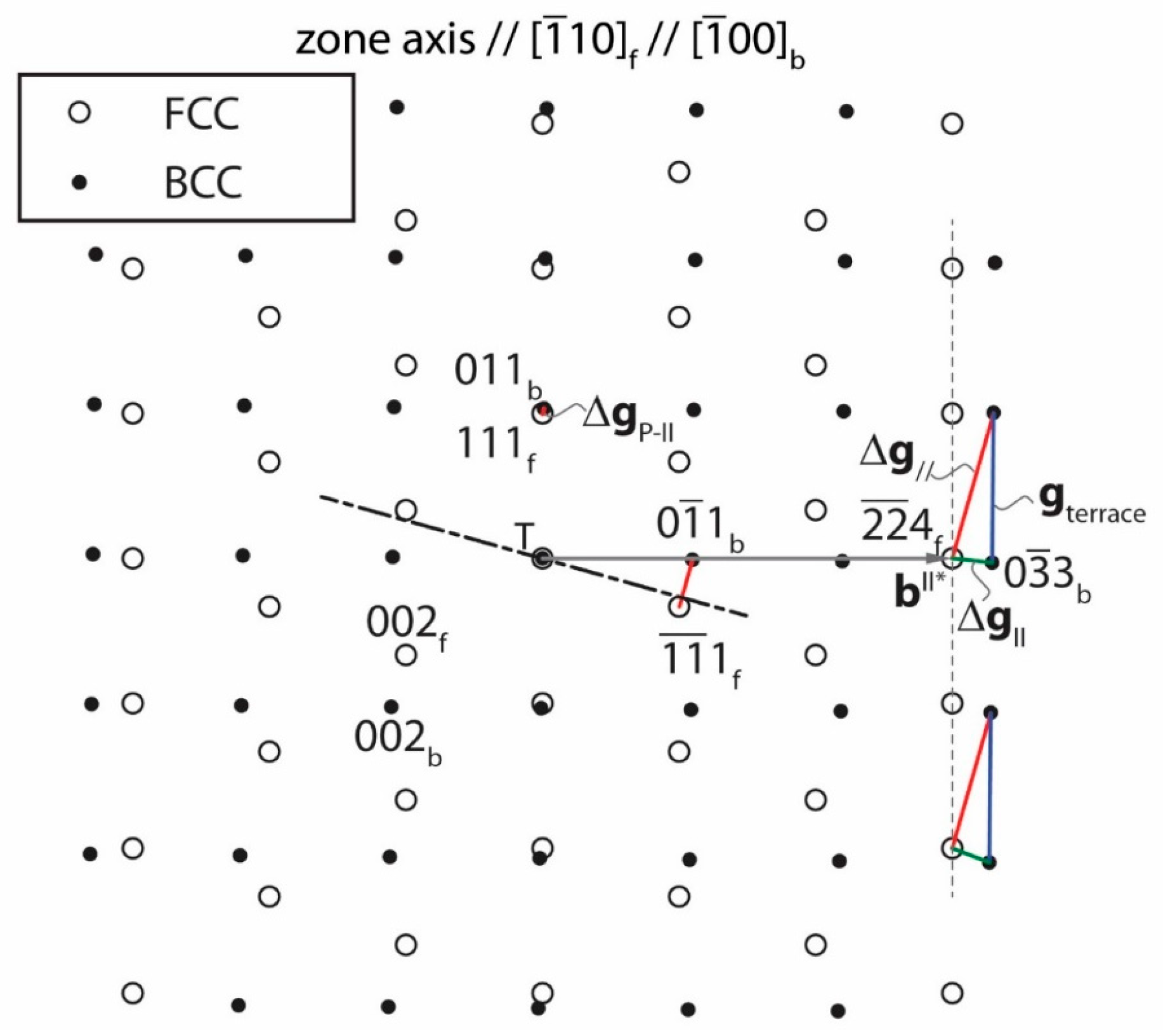
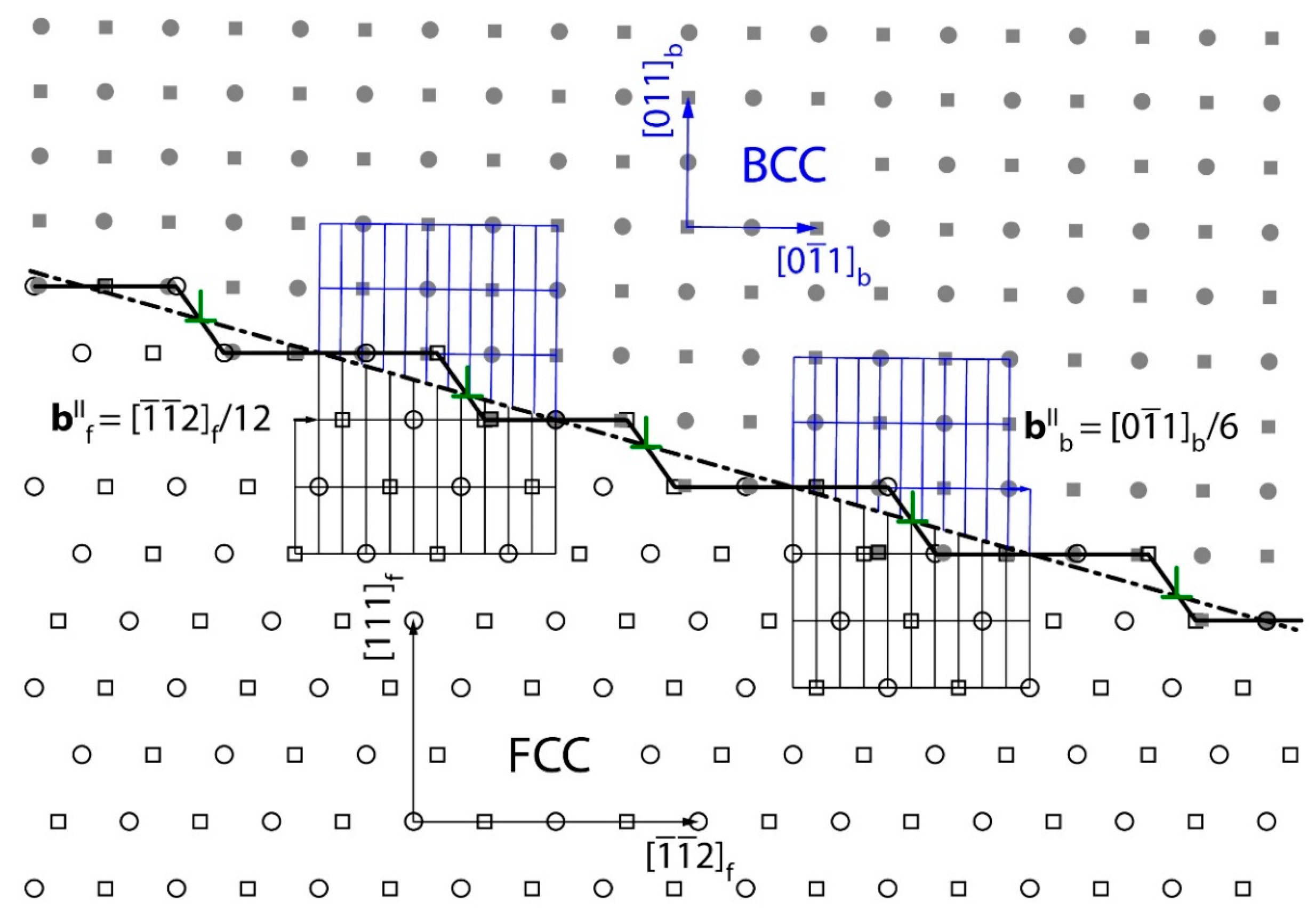
3. Results
4. Discussion
4.1. The Current Work vs. the Topological Model
4.2. The Current Work vs. the O-Line Model
5. Conclusions
- (1)
- The topological model can deliver the same OR and IO of a stepped, semi-coherent interface with those determined by a 2D approach of the O-line model when a 2D reference lattice is correctly defined. The discrepancy between the two models barely rests on the different description of the role of interface steps/disconnections.
- (2)
- The disconnections in the topological model can be explicitly described as regularly spaced interface steps coincident with a set of secondary misfit dislocations. The Burgers vector of the secondary dislocations, bII, is a partial lattice translation vector, defined by a basic vector of the CDSCL based on the OR that enables periodic O-lines.
- (3)
- In contrast to the primary misfit dislocations, which separate coherent patches in terrace planes, this set of secondary misfit dislocations do not destroy the continuity of lattice planes across the interface, and it only accommodates the extra misfit when the terrace plane is off the average interface, which cannot be described by the convention O-line model.
- (4)
- The one-to-one correspondence between the secondary misfit dislocations and the interface steps can be not only achieved in the secondary preferred state, but it can also appear in the primary preferred state when a 2D CCSL/CDSCL can be appropriately constructed.
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
References
- Zhang, W.-Z.; Weatherly, G.C. On the crystallography of precipitation. Prog. Mater. Sci. 2005, 50, 181–292. [Google Scholar] [CrossRef]
- Zhang, W.-Z.; Purdy, G.R. O-lattice analyses of interfacial misfit. II. Systems containing invariant lines. Philos. Mag. A 1993, 68, 291–303. [Google Scholar] [CrossRef]
- Dahmen, U. Orientation relationships in precipitation systems. Acta Metall. 1982, 30, 63–73. [Google Scholar] [CrossRef] [Green Version]
- Kelly, P.M.; Zhang, M.-X. Edge-to-edge matching—A new approach to the morphology and crystallography of precipitates. Mater. Forum 1999, 23, 41–62. [Google Scholar]
- Howe, J.M.; Pond, R.C.; Hirth, J.P. The role of disconnections in phase transformations. Prog. Mater. Sci. 2009, 54, 792–838. [Google Scholar] [CrossRef]
- Pond, R.C.; Celotto, S.; Hirth, J.P. A comparison of the phenomenological theory of martensitic transformations with a model based on interfacial defects. Acta Mater. 2003, 51, 5385–5398. [Google Scholar] [CrossRef]
- Qiu, D.; Zhang, W.-Z. A systematic study of irrational precipitation crystallography in fcc/bcc systems with an analytical O-line method. Philos. Mag. 2003, 83, 3093–3116. [Google Scholar] [CrossRef]
- Wu, J.; Zhang, W.-Z.; Gu, X.-F. A two-dimensional analytical approach for phase transformations involving an invariant line strain. Acta Mater. 2006, 57, 635–645. [Google Scholar] [CrossRef]
- Hall, M.G.; Aaronson, H.I.; Kinsman, K.R. The structure of nearly coherent fcc: bcc boundaries in a Cu-Cr alloy. Surf. Sci. 1972, 31, 257–274. [Google Scholar] [CrossRef]
- Hall, M.G.; Aaronson, H.I. The fine structure f.c.c./b.c.c. boundaries in a Cu-0.3% Cr alloy. Acta Metall. 1986, 34, 1409–1418. [Google Scholar] [CrossRef]
- Fujii, T.; Nakazawa, H.; Kato, M.; Dahmen, U. Crystallography and morphology of nanosized Cr particles in a Cu-0.2% Cr alloy. Acta Mater. 2000, 48, 1033–1045. [Google Scholar] [CrossRef]
- Luo, C.P.; Dahmen, U.; Westmacott, K.H. Morphology and crystallography of Cr precipitates in a Cu-0.33 wt% Cr alloy. Acta Metall. Mater. 1994, 42, 1923–1932. [Google Scholar] [CrossRef]
- Qiu, D.; Shen, Y.-X.; Zhang, W.-Z. An extended invariant line analysis for fcc/bcc precipitation systems. Acta Mater. 2006, 53, 339–347. [Google Scholar] [CrossRef]
- Kelly, P.M.; Qiu, D. The topological model (TM) of martensite transformations—Some improvements. Solid State Phenomena 2011, 172–174, 25–30. [Google Scholar] [CrossRef]
- Zhang, W.-Z.; Purdy, G.R. TEM study of the crystallography and interphase boundary structure of precipitates in a Zr-2.5 wt% Nb Alloy. Acta Metall. Mater. 1993, 41, 543–551. [Google Scholar] [CrossRef]
- Ye, F.; Zhang, W.-Z.; Qiu, D. A TEM study of the habit plane structure of intragranular proeutectoid precipitates in a Ti-7.26 wt% Cr alloy. Acta Mater. 2004, 52, 2449–2460. [Google Scholar] [CrossRef]
- Howe, J.M.; Spanos, G. Atomic structure of the austenite-cementite interface of proeutectoid cementite plates. Philos. Mag. 1999, 79, 9–30. [Google Scholar] [CrossRef]
- Ye, F.; Zhang, W.-Z. Coincidence structures of interfacial steps and secondary misfit dislocations in the habit plane between Widmanstatten cementite and austenite. Acta Mater. 2002, 50, 2761–2777. [Google Scholar] [CrossRef]
- Zhang, M.; Zhang, W.-Z.; Ye, F. Interpretation of precipitation crystallography of Mg17Al12 in a Mg-Al alloy in terms of singular interface structure. Metall. Mater. Trans. 2005, 36A, 1681–1688. [Google Scholar] [CrossRef]
- Qiu, D.; Kelly, P.M.; Zhang, M.-X. The interfacial structure of self-assembled DySi2 nanostructures grown on Si(0 0 1). Scr. Mater. 2009, 60, 787–790. [Google Scholar] [CrossRef]
- Muddle, B.C.; Nie, J.F.; Hugo, G.R. Application of the theory of martensite crystallography to displacive phase transformations in substitutional nonferrous alloys. Metall. Mater. Trans. 1994, 25A, 1841–1856. [Google Scholar] [CrossRef]

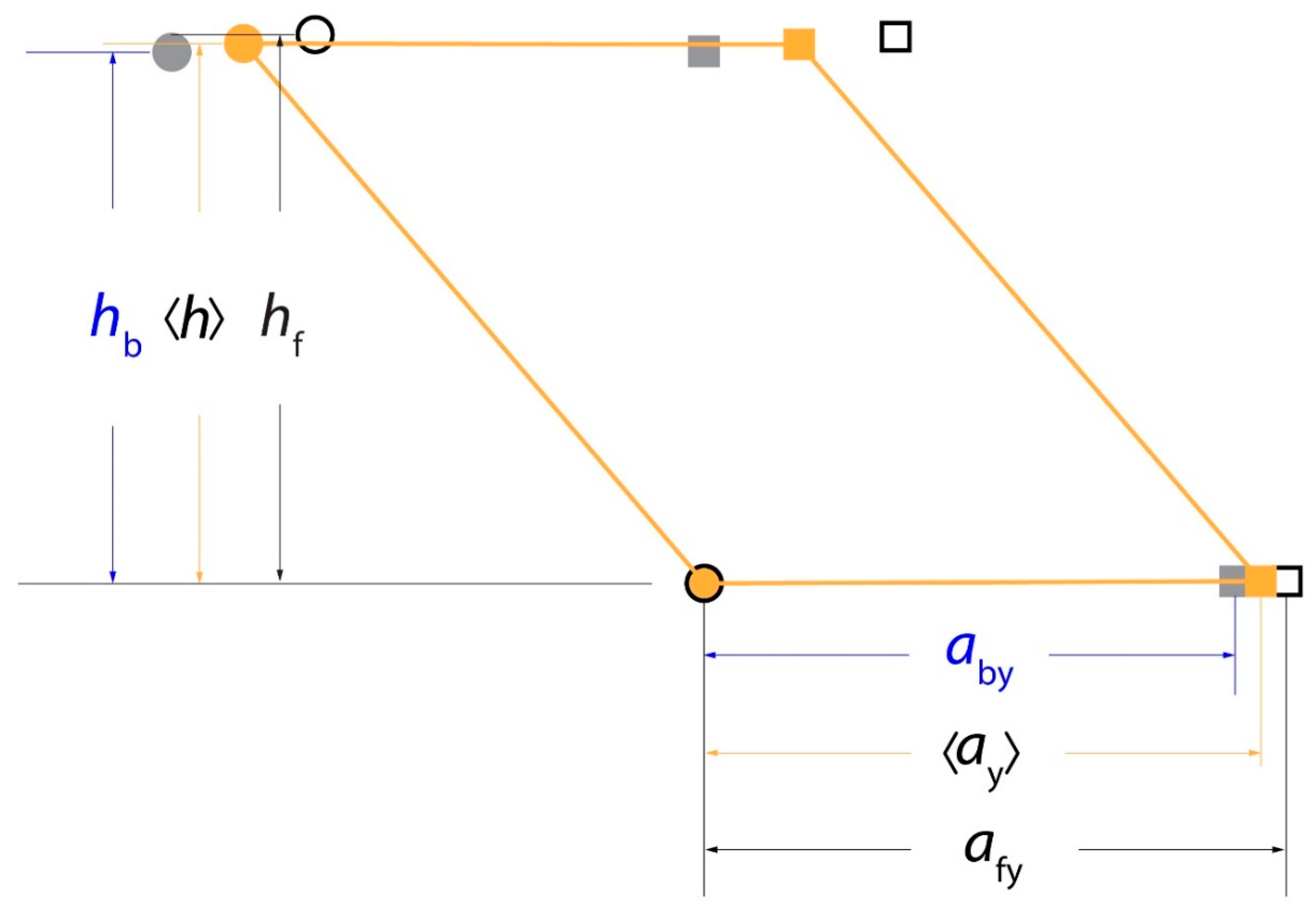

| εyy | by (nm) | bz (nm) | <h> (nm) | θ (°) | φ (°) | θf (°) | θb (°) |
|---|---|---|---|---|---|---|---|
| −0.0928 | 0.0704 | −0.0070 | 0.2052 | 15.56 | 0.5420 | 15.83 | 15.28 |
| a | b | s | φ (°) | θf (°) |
|---|---|---|---|---|
| 1.0973 | 1.0346 | −0.3658 | 0.5420 | 15.83 |
© 2019 by the author. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Qiu, D. Revisit the Role of Steps/Disconnections on Misfit Cancellation at Semi-Coherent Interface—Bridging the O-Line Model and the Topological Model. Crystals 2019, 9, 525. https://doi.org/10.3390/cryst9100525
Qiu D. Revisit the Role of Steps/Disconnections on Misfit Cancellation at Semi-Coherent Interface—Bridging the O-Line Model and the Topological Model. Crystals. 2019; 9(10):525. https://doi.org/10.3390/cryst9100525
Chicago/Turabian StyleQiu, Dong. 2019. "Revisit the Role of Steps/Disconnections on Misfit Cancellation at Semi-Coherent Interface—Bridging the O-Line Model and the Topological Model" Crystals 9, no. 10: 525. https://doi.org/10.3390/cryst9100525
APA StyleQiu, D. (2019). Revisit the Role of Steps/Disconnections on Misfit Cancellation at Semi-Coherent Interface—Bridging the O-Line Model and the Topological Model. Crystals, 9(10), 525. https://doi.org/10.3390/cryst9100525





