Ni Doping: A Viable Route to Make Body-Centered-Cubic Fe Stable at Earth’s Inner Core
Abstract
:1. Introduction
2. Methodology
3. Results
4. Discussion
5. Concluding Remarks and Implications
Supplementary Materials
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
References
- Allégre, C.J.; Poirier, J.-P.; Humler, E.; Hofmann, A.W. The chemical composition of the Earth. Earth Planet. Sci. Lett. 1995, 134, 515–526. [Google Scholar] [CrossRef]
- Anderson, D.L. Theory of the Earth; Blackwell Scientific Publications: Hoboken, NJ, USA, 1989. [Google Scholar]
- Vočadlo, L.; Brodholt, J.; Alfe, D.; Price, G.D. The structure of iron under the conditions of the Earth’s inner core. Geophys. Res. Lett. 1999, 26, 1231–1234. [Google Scholar] [CrossRef] [Green Version]
- Belonoshko, A.B.; Ahuja, R.; Johansson, B. Stability of the body-centred-cubic phase of iron in the Earth’s inner core. Nature 2003, 424, 1032. [Google Scholar] [CrossRef]
- Anzellini, S.; Dewaele, A.; Mezouar, M.; Loubeyre, P.; Morard, G. Melting of iron at Earth’s inner core boundary based on fast X-ray diffraction. Science 2013, 340, 464–466. [Google Scholar] [CrossRef]
- Denoeud, A.; Ozaki , N.; Benuzzi-Mounaix, A.; Uranishi , H.; Kondon, Y.; Kodama, R.; Brambrink, E.; Ravasio, A.; Bocoum, M.; Boudenne , J.-M.; et al. Dynamic X-ray diffraction observation of shocked solid iron up to 170 GPa. Proc. Natl. Acad. Sci. USA 2016, 113, 7745–7749. [Google Scholar] [CrossRef] [Green Version]
- Tateno, S.; Hirose, K.; Ohishi, Y.; Tatsumi, Y. The structure of iron in Earth’s inner core. Science 2010, 330, 359–361. [Google Scholar] [CrossRef]
- Luo, W.; Johansson, B.; Eriksson, O.; Arapan, S.; Souvatzis, P.; Katsnelson, M.I.; Ahuja, R. Dynamical stability of body center cubic iron at the Earth’s core conditions. Proc. Natl. Acad. Sci. USA 2010, 107, 9962. [Google Scholar] [CrossRef] [Green Version]
- Aquilanti, G.; Trapananti, A.; Karandikar, A.; Kantor, I.; Marini, C.; Mathon, O.; Pascarelli, S.; Boehler, R. Melting of iron determined by X-ray absorption spectroscopy to 100 GPa. Proc. Natl. Acad. Sci. USA 2015, 112, 12042–12045. [Google Scholar] [CrossRef] [Green Version]
- Tateno, S.; Hirose, K.; Komabayashi, T.; Ozawa, H.; Ohishi, Y. The structure of Fe-Ni alloy in Earth’s inner core. Geophys. Res. Lett. 2012, 39, L12305. [Google Scholar] [CrossRef] [Green Version]
- Hirose, K.; Labrosse, S.; Hernlund, J. Composition and state of the core. Ann. Rev. Earth Planet. Sci. 2013, 41, 657–691. [Google Scholar] [CrossRef]
- Mao, W.L.; Campbell, A.J.; Heinz, D.L.; Shen, G.Y. Phase relations of Fe-Ni alloys at high pressure and temperature. Phys. Earth Planet. Inter. 2006, 155, 146–151. [Google Scholar] [CrossRef]
- Komabayashi, T.; Hirose, K.; Ohishi, Y. In situ X-ray diffraction measurements of the fcc–hcp phase transition boundary of an Fe-Ni alloy in an internally heated diamond anvil cell. Phys. Chem. Miner. 2012, 39, 329–338. [Google Scholar] [CrossRef]
- Kuwayama, Y.; Hirose, K.; Sata, N.; Ohishi, Y. Phase relations of Fe and Fe-Ni alloys up to 300 GPa: Implications for composition and structure of the Earth’s inner core. Earth Planet. Sci. Lett. 2008, 273, 379–385. [Google Scholar] [CrossRef]
- Vočadlo, L.; Dobson, D.P.; Wood, I.G. Ab initio study of nickel substitution into iron. Earth Planet. Sci. Lett. 2006, 248, 132–137. [Google Scholar] [CrossRef]
- Côté, A.S.; Vočadlo, L.; Brodholt, J.P. Ab initio simulations of iron-nickel alloys at Earth’s core conditions. Earth Planet. Sci. Lett. 2012, 345, 126–130. [Google Scholar] [CrossRef] [Green Version]
- Martorell, B.; Brodholt, J.; Wood, I.G.; Vočadlo, L. The effect of nickel on the properties of iron at the conditions of Earth’s inner core: Ab initio calculations of seismic wave velocities of Fe-Ni alloys. Earth Planet. Sci. Lett. 2013, 365, 143–151. [Google Scholar] [CrossRef]
- Ekholm, M.; Mikhaylushkin, A.S.; Simak, S.I.; Johansson, B.; Abrokosov, I.A. Configurational thermodynamis of Fe-Ni alloys at Earth’s core conditions. Earth Planet. Sci. Lett. 2011, 308, 90–96. [Google Scholar] [CrossRef] [Green Version]
- Lin, J.F.; Heinz, D.L.; Campbell, A.J.; Devine, J.M.; Mao, W.L.; Shen, G. Iron-nickel alloy in the Earth’s core. Geophys. Res. Lett. 2002, 29, 1471. [Google Scholar] [CrossRef] [Green Version]
- Sakai, T.; Ohtani, E.; Hirao, N.; Ohishi, Y. Stability field of the hcp-structure for Fe, Fe-Ni, and Fe-Ni-Si alloys up to 3 Mbar. Geophys. Res. Lett. 2011, 38, L09302. [Google Scholar] [CrossRef]
- Torchio, R.; Boccato, S.; Miozzi, F.; Rosa, A.D.; Ishimatsu, N.; Kantor, I.; Sévelin-Radiguet, N.; Briggs, R.; Meneghini, C.; Irifune, T.; et al. Melting curve and phase relations of Fe-Ni alloys: Implications for the Earth’s core composition. Geophys. Res. Lett. 2020, 47, e2020GL088169. [Google Scholar] [CrossRef]
- Belonoshko, A.B.; Lukinov, T.; Fu, J.; Zhao, J.; Davis, S.; Simak, S.I. Stabilization of body-centered cubic iron under inner-core condition. Nat. Geosci. 2017, 10, 312. [Google Scholar] [CrossRef]
- Dubrovinsky, L.S.; Dubrovinskaia, N.A.; Narygina, O. Body-centered cubic iron-nickel alloy in Earth’s core. Science 2007, 316, 1880–1883. [Google Scholar] [CrossRef]
- Morelli, A.; Dziewonski, A.M.; Woodhouse, J.H. Anisotropy of the inner core inferred from PKIKP travel times. Geophys. Res Lett. 1986, 13, 1545–1548. [Google Scholar] [CrossRef]
- Woodhouse, J.H.; Giardini, D.; Li, X.D. Evidence for inner core anisotropy from free oscillations. Geophys. Res. Lett. 1986, 13, 1549–1552. [Google Scholar] [CrossRef]
- Song, X.D. Anisotropy of the Earth’s inner core. Rev. Geophys. 1997, 35, 297–313. [Google Scholar] [CrossRef]
- Beghein, C.; Trampert, J. Robust normal mode constraints on inner-core anisotropy from model space search. Science 2003, 229, 552–555. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Wenk, H.R.; Matthies, S.; Hemley, R.J.; Mao, H.K.; Shu, J. The plastic deformation of iron at pressures of the Earth’s inner core. Nature 2000, 45, 1044–1047. [Google Scholar] [CrossRef] [PubMed]
- Buffett, B.A.; Wenk, H.-R. Texturing of the Earth’s inner core by Maxwell stresses. Nature 2001, 413, 60–63. [Google Scholar] [CrossRef]
- Mattesini, M.; Belonoshko, A.B.; Tkalčić, H. Polymorphic nature of iron and degree of lattice preferred orientations beneath the Earth’s inner core boundary. Geochem. Geophys. Geosyst. 2018, 19, 292–304. [Google Scholar] [CrossRef]
- Belonoshko, A.B.; Skorodumova, N.V.; Rosengren, A.; Johansson, B. Elastic anisotropy of Earth’s inner core. Science 2008, 319, 797–800. [Google Scholar] [CrossRef]
- Mattesini, M.; Belonoshko, A.B.; Tkalčić, H.; Buforn, E.; Udias, A.; Ahuja, R. Candy wrapper for the Earth’s inner core. Sci. Rep. 2013, 3, 1–8. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Ohtani, E.; Shibazaki, Y.; Sakai, T.; Mibe, K.; Fukui, H.; Kamada, S.; Sakamaki, T.; Seto, Y.; Tsutsui, S.; Baron, A.Q.R. Sound velocity of hexagonal close packed iron up to core pressures. Geophys. Res. Lett. 2013, 40, 5089–5094. [Google Scholar] [CrossRef]
- Murphy, C.A.; Jackson, J.M.; Sturhahn, W. Experimental constraints on the thermodynamics and sound velocities of hcp-Fe to core pressures. J. Geophys. Res. Solid Earth 2013, 118, 1999–2016. [Google Scholar] [CrossRef] [Green Version]
- Antonangeli, A.; Morard, G.; Paolasini, L.; Garbarini, G.; Murphy, C.A.; Edmund, E.; Decremps, F.; Fiquet, G.; Bosak, A.; Mezouar, M.; et al. Sound velocities and density measurements of solid hcp-Fe and hcp-Fe-Si (9 wt.%) alloy at high pressure: Constraints on the Si abundance in the Earth’s inner core. Earth Planet. Sci. Lett. 2018, 482, 446–453. [Google Scholar] [CrossRef]
- Kresse, G.; Hafner, J. Ab initio molecular dynamics for liquid metals. Phys. Rev. B 1993, 47, 558–561. [Google Scholar] [CrossRef]
- Kresse, G.; Furthmuller, J. Efficient iterative schemes for ab-initio total-energy calculations using a plane-wave basis set. Phys. Rev. B 1996, 54, 11169–11186. [Google Scholar] [CrossRef]
- Kresse, G.; Joubert, D. From ultrasoft pseudopotentials to the projector augment d-wave method. Phys. Rev. B 1999, 59, 1158–1775. [Google Scholar] [CrossRef]
- Perdew, J.P.; Burke, K.; Ernzerhof, M. Generalized gradient approximation made simple. Phys. Rev. Lett. 1996, 77, 3865–3868. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Iota, V.; Klepeis, J.H.P.; Yoo, C.S.; Lang, J.; Haskel, D.; Srajer, G. Electronic structure and magnetism in compressed 3d transition metals. Appl. Phys. Lett. 2007, 90, 042505. [Google Scholar] [CrossRef]
- Ezenwa, I.C.; Secco, R.A. Fe melting transition: Electrical resistivity, thermal conductivity, and heat flow at the inner core boundaries of mercury and ganymede. Crystals 2019, 9, 359. [Google Scholar] [CrossRef] [Green Version]
- Vočadlo, L.; Alfe, D.; Gillan, M.J.; Wood, I.G.; Brodholt, J.; Price, G.D. Possible thermal and chemical stabilization of body-centred-cubic iron in the Earth’s core. Nature 2003, 424, 536–539. [Google Scholar] [CrossRef]
- Murnaghan, F.D. The compressibility of media under extreme pressures. Proc. Natl. Acad. Sci. USA 1944, 30, 244–247. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Page, Y.L.; Saxe, P. Symmetry-general least-squares extraction of elastic data for strained materials from ab initio calculations of stress. Phys. Rev. B 2002, 65, 104104. [Google Scholar] [CrossRef]
- Voigt, W. Lehrbuch der Kristallphysik; B.G. Teubner: Leipzig, Germany, 1928. [Google Scholar]
- Simmons, G.; Wang, H. Single Crystal Elastic Constants and Calculated Aggregate Properties, A Handbook; MIT Press: Cambridge, UK, 1971; Volume 379. [Google Scholar]
- Sha, X.; Cohen, R.E. Lattice dynamics and thermodynamics of bcc iron under pressure: First principles linear response theory. Phys. Rev. B 2006, 73, 104303. [Google Scholar] [CrossRef] [Green Version]
- Togo and Tanaka 2015 Togo, A.; Tanaka, I. First principles phonon calculations in materials science. Scr. Mater. 2015, 108, 1–5. [Google Scholar] [CrossRef] [Green Version]
- Stixrude, L.; Cohen, R.E. Constraints on the crystalline structure of the inner core: Mechanical instability of bcc iron at high pressure. Geophys. Res. Lett. 1995, 22, 125–128. [Google Scholar] [CrossRef] [Green Version]
- Alfe, D.; Price, G.D.; Gillan, M.J. Thermodynamics of hexagonal close packed iron under Earth’s core conditions. Phys. Rev. B 2001, 64, 04512316. [Google Scholar] [CrossRef] [Green Version]
- Vočadlo, L.; Wood, I.G.; Alfè, D.; Price, G.D. Ab initio calculations on the free energy and high P–T elasticity of face-centred-cubic iron. Earth Planet. Sci. Lett. 2008, 268, 444–449. [Google Scholar] [CrossRef] [Green Version]
- Côté, A.S.; Vočadlo, L.; Brodholt, J.P. Light elements in the core: Effects of impurities on the phase diagram of iron. Geophys. Res. Lett. 2008, 35, L05306. [Google Scholar] [CrossRef] [Green Version]
- Stixrude, L. Structure of Iron to 1 Gbar and 40 000 K. Phys. Rev. Lett. 2012, 108, 055505. [Google Scholar] [CrossRef]
- Mikhaylushkin, A.S.; Simak, S.I.; Dubrovinsky, L.S.; Dubrovinskaia, N.A.; Johansson, B.; Abrikosov, I.A. Pure iron compressed and heated to extreme conditions. Phys. Rev. Lett. 2007, 99, 165505. [Google Scholar] [CrossRef]
- Dziewonski, A.M.; Anderson, D.L. Preliminary reference Earth model. Phys. Earth Planet. Inter. 1981, 25, 297–356. [Google Scholar] [CrossRef]
- Mao, H.K.; Shu, J.; Shen, G.; Hemley, R.J.; Li, B.; Singh, A.K. Elasticity and rheology of iron above 220 GPa and the nature of the Earth’s inner core. Nature 1998, 396, 741–743, (correction Nature 1999, 399, 80). [Google Scholar] [CrossRef]
- Antonangeli, D.; Occelli, F.; Requardt, H.; Badro, J.; Fiquet, G.; Krisch, M. Elastic anisotropy in textured hcp-iron to 112 GPa from sound velocity wave propagation measurements. Earth Planet. Sci. Lett. 2004, 225, 243–251. [Google Scholar] [CrossRef]
- Antonangeli, D.; Komabayashi, T.; Occelli, F.; Borissenko, E.; Walters, A.C.; Fiquest, G.; Fei, Y. Simultaneous sound velocity and density measurements of hcp iron upto 93GPa and 1100K: An experimental test of the Birch’s law at high tempearture. Earth Planet. Sci. Lett. 2012, 331, 210–214. [Google Scholar] [CrossRef]
- Crowhurst, J.C.; Goncharov, A.F.; Zaug, J.M. Impulsive stimulated light scattering from opaque materials at high pressure. J. Phys. Condens. Matter 2004, 16, S1137–S1142. [Google Scholar] [CrossRef]
- Gleason, A.E.; Mao, W.L.; Zhao, J.Y. Sound velocities for hexagonally close packed iron compressed hydrostatically to 136 GPa from phonon density of states. Geophys. Res. Lett. 2013, 40, 2983–2987. [Google Scholar] [CrossRef]
- Decremps, F.; Antonangeli, D.; Gauthier, M.; Ayrinhac, S.; Morand, M.; Le Marchand, G.; Bergame, F.; Philippe, J. Sound velocity measurements of iron up to 152 GPa by picosecond acoustics in diamond anvil cell. Geophys. Res. Lett. 2014, 41, 1459. [Google Scholar] [CrossRef]
- Brown, J.M.; McQueen, R.G. Phase transitions, Grüneisen parameter, and elasticity for shocked iron between 77 GPa and 400 GPa. J. Geophys. Res. Solid Earth 1986, 91, 7485–7494. [Google Scholar] [CrossRef]
- Antonangeli, D.; Ohtani, E. Sound velocity of hcp-Fe at high pressure: Experimental constraints, extrapolations and comparison with seismic models. Prog. Earth Planet. Sci. 2015, 2, 3. [Google Scholar] [CrossRef]
- Laio, A.; Bernard, S.; Chiarotti, G.L.; Scandolo, S.; Tosatti, E. Physics of iron at Earth’s core conditions. Science 2000, 287, 1027–1030. [Google Scholar] [CrossRef] [PubMed]
- Vočadlo, L.; Dobson, D.P.; Wood, I.G. Ab initio calculations of the elasticity of hcp-Fe as a function of temperature at inner-core pressure. Earth Planet. Sci. Lett. 2009, 288, 534–538. [Google Scholar] [CrossRef]
- Martorell, B.; Vočadlo, L.; Brodholt, J.; Wood, I.G. Strong premelting effect in the elastic properties of hcp-Fe under inner-core conditions. Science 2013, 342, 466–468. [Google Scholar] [CrossRef] [Green Version]
- Jackson, I.; Gerald, J.F.; Kokkonen, H. High-temperature viscoelastic relaxation in iron and its implications for the shear modulus and attenuation of the Earth’s inner core. J. Geophys. Res. 2000, 1105, 23605–23634. [Google Scholar] [CrossRef]
- Singh, S.C.; Taylor, M.A.J.; Montagner, J.P. On the presence of liquid in Earth’s inner core. Science 2000, 287, 2471–2474. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Vočadlo, L. Ab initio calculations of the elasticity of iron and iron alloys at inner core conditions: Evidence for a partially molten inner core? Earth Planet. Sci. Lett. 2007, 254, 227–232. [Google Scholar] [CrossRef]
- Steinle-Neumann, G.; Stixrude, L.; Cohen, R.E.; Gülseren, O. Elasticity of iron at the temperature of the Earth’s inner core. Nature 2001, 413, 57–60. [Google Scholar] [CrossRef]
- Gannarelli, C.M.S.; Alfè, D.; Gillan, M.J. The axial ratio of hcp iron at the conditions of the Earth’s inner core. Phys. Earth Planet. Inter. 2005, 152, 67–77. [Google Scholar] [CrossRef] [Green Version]
- Mattesini, M.; Belonoshko, A.B.; Buforn, E.; Ramírez, M.; Simak, S.I.; Udías, A.; Mao, H.K.; Ahuja, R. Hemispherical anisotropic patterns of the Earth’s inner core. Proc. Natl. Acad. Sci. USA 2010, 107, 9507–9512. [Google Scholar] [CrossRef] [Green Version]
- Matsui, M.; Anderson, O.L. The case for a body-centered cubic phase (α′) for iron at inner core conditions. Phys. Earth Planet. Inter. 1997, 103, 55–62. [Google Scholar] [CrossRef]
- Hrubiak, R.; Meng, Y.; Shen, G. Experimental evidence of a body centered cubic iron at the Earth’s core condition. arXiv 2018, arXiv:1804.05109. [Google Scholar]
- Ping, Y.; Coppari, F.; Hicks, D.G.; Yaakobi, B.; Fratanduono, D.E.; Hamel, S.; Eggert, J.H.; Rygg, J.R.; Smith, R.F.; Swift, D.C.; et al. Solid Iron compressed up to 560 GPa. Phys. Rev. Lett. 2013, 111, 065501. [Google Scholar] [CrossRef] [Green Version]
- Stevenson, D.J. Models of the Earth’s core. Science 1981, 214, 611–619. [Google Scholar] [CrossRef] [PubMed]
- Poirier, J.P. Light elements in the Earth’s outer core: A critical review. Phys. Earth Planet. Inter. 1994, 85, 319–337. [Google Scholar] [CrossRef]
- Badro, J.; Côté, A.S.; Brodholt, J.P. A seismologically consistent compositional model of Earth’s core. Proc. Natl. Acad. Sci. USA 2014, 111, 7542–7545. [Google Scholar] [CrossRef] [Green Version]
- Li, J.; Fei, Y. Experimental constraints on core composition, The Mantle and Core. In Treatise on Geochemistry; Carlson, R.W., Ed.; Elsevier: Amsterdam, The Netherland, 2014; Volume 2, pp. 521–546. [Google Scholar]
- Das, T.; Chatterjee, S.; Ghosh, S.; Saha-Dasgupta, T. First-principles prediction of Si-doped Fe carbide as one of the possible constituents of Earth’s inner core. Geophys. Res. Lett. 2017, 44, 8776–8784. [Google Scholar] [CrossRef]
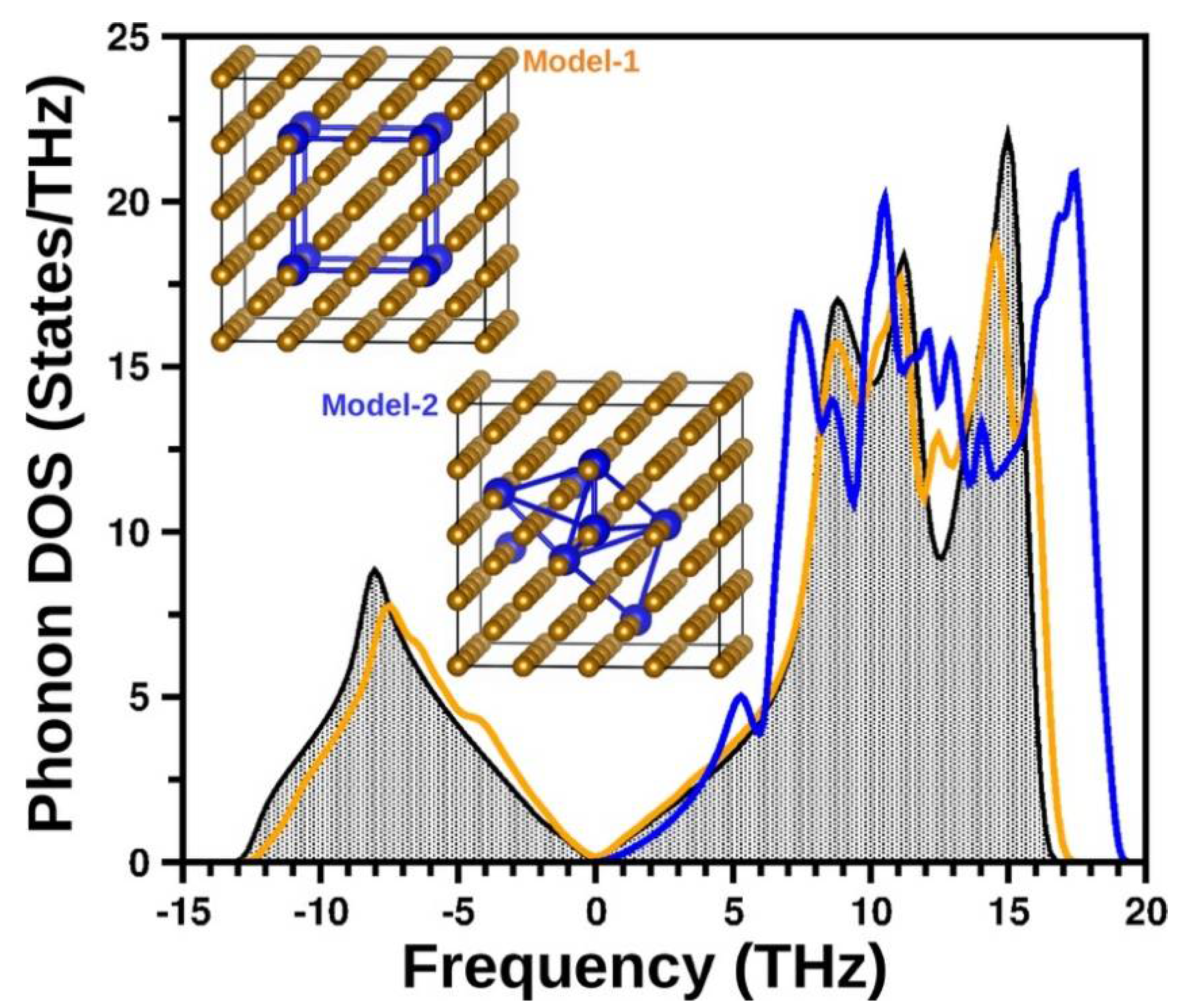

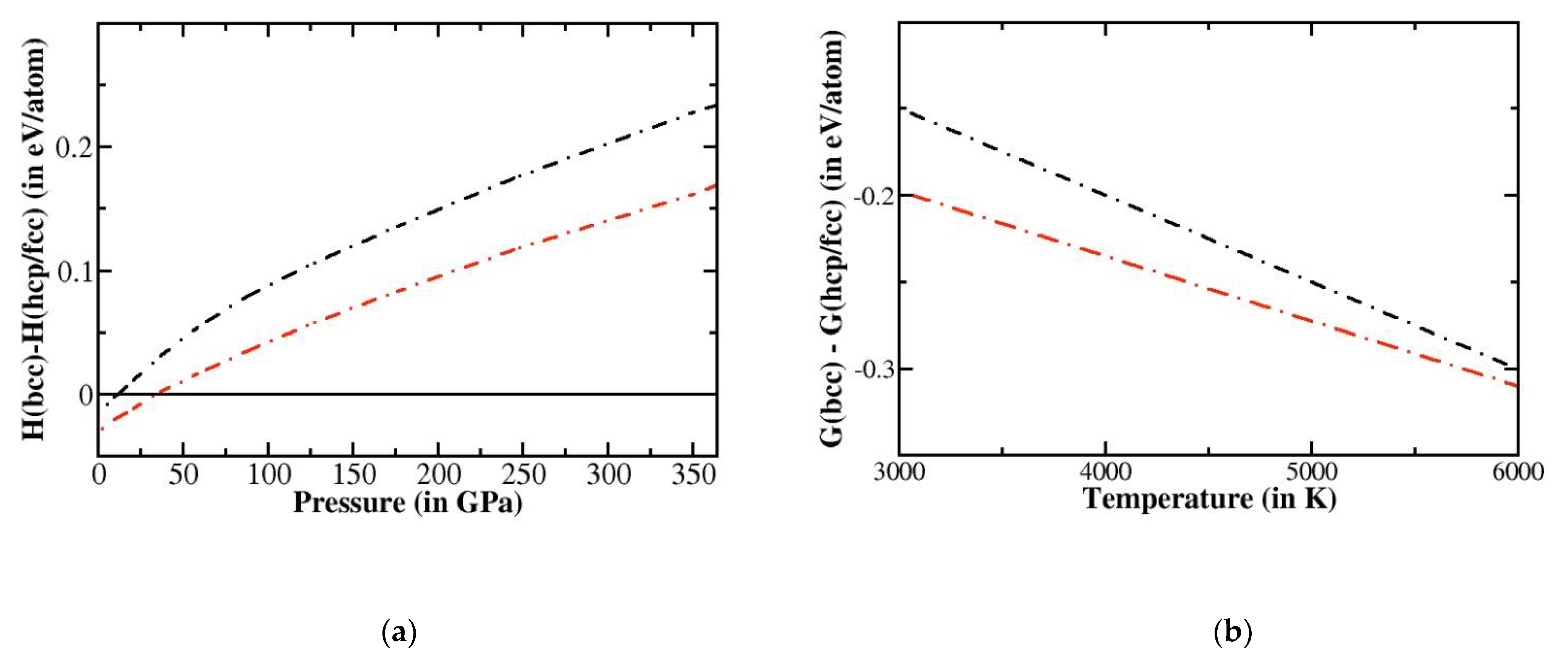
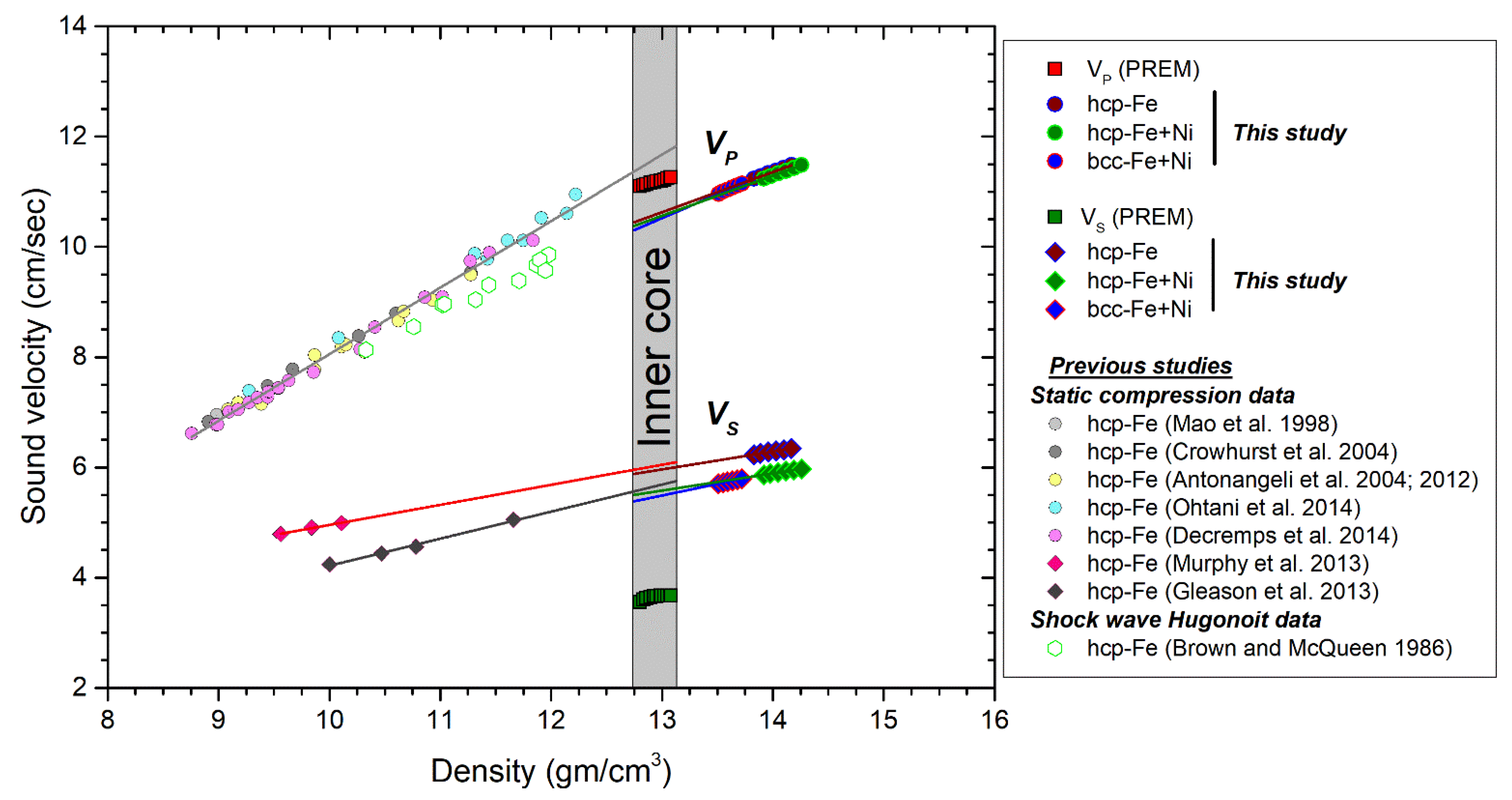
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Chatterjee, S.; Ghosh, S.; Saha-Dasgupta, T. Ni Doping: A Viable Route to Make Body-Centered-Cubic Fe Stable at Earth’s Inner Core. Minerals 2021, 11, 258. https://doi.org/10.3390/min11030258
Chatterjee S, Ghosh S, Saha-Dasgupta T. Ni Doping: A Viable Route to Make Body-Centered-Cubic Fe Stable at Earth’s Inner Core. Minerals. 2021; 11(3):258. https://doi.org/10.3390/min11030258
Chicago/Turabian StyleChatterjee, Swastika, Sujoy Ghosh, and Tanusri Saha-Dasgupta. 2021. "Ni Doping: A Viable Route to Make Body-Centered-Cubic Fe Stable at Earth’s Inner Core" Minerals 11, no. 3: 258. https://doi.org/10.3390/min11030258
APA StyleChatterjee, S., Ghosh, S., & Saha-Dasgupta, T. (2021). Ni Doping: A Viable Route to Make Body-Centered-Cubic Fe Stable at Earth’s Inner Core. Minerals, 11(3), 258. https://doi.org/10.3390/min11030258





