Simultaneous Partition Experiment of Divalent Metal Ions between Sphalerite and 1 mol/L (Ni, Mg, Co, Fe, Mn)Cl2 Aqueous Solution under Supercritical Conditions
Abstract
:1. Introduction
2. Materials and Methods
2.1. Sphalerite
2.2. Solid Starting Material
2.3. Liquid Starting Material
2.4. Experimental Procedure
3. Results and Discussion
3.1. Solid and Liquid Run Products
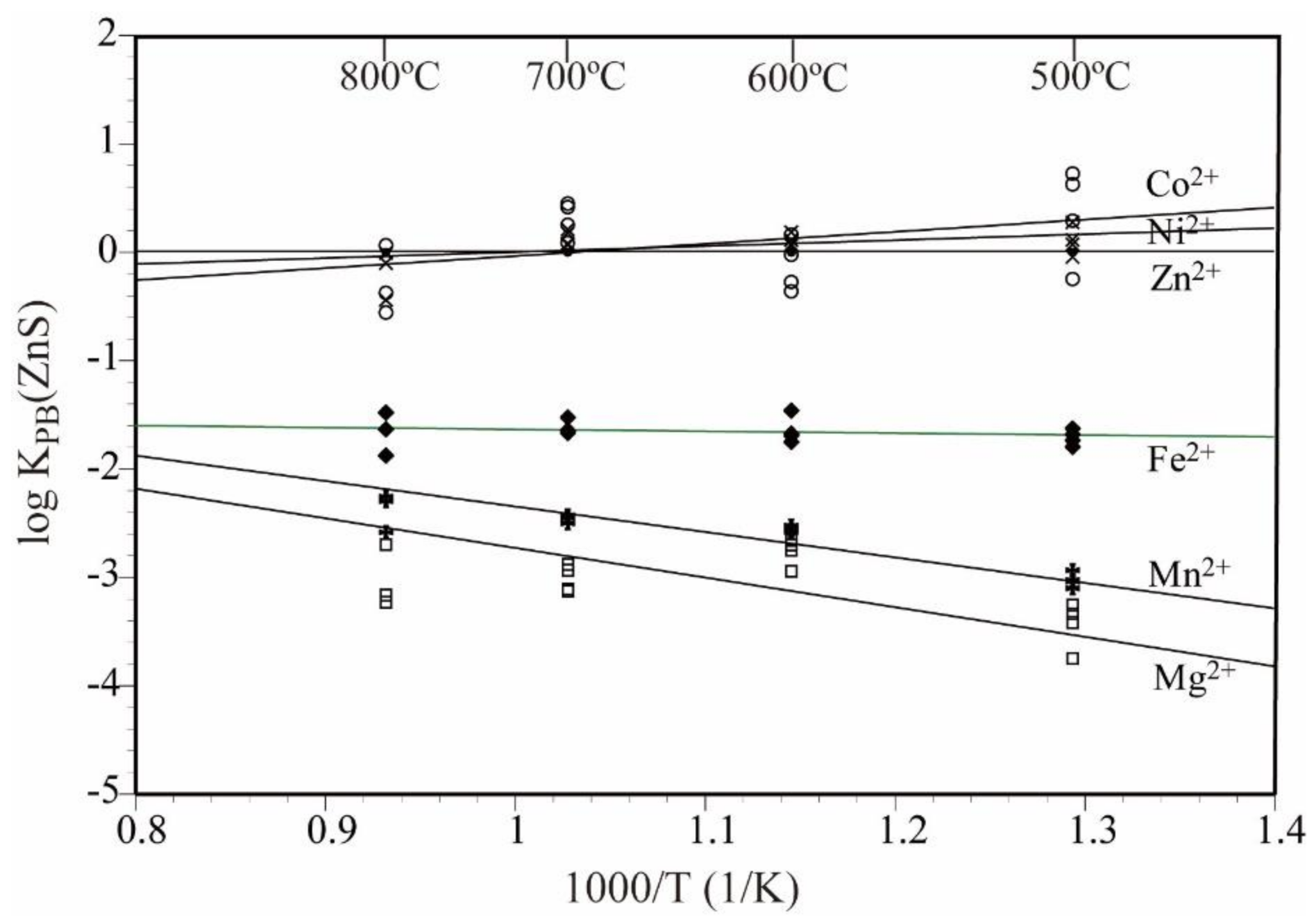
3.2. Element Partitioning
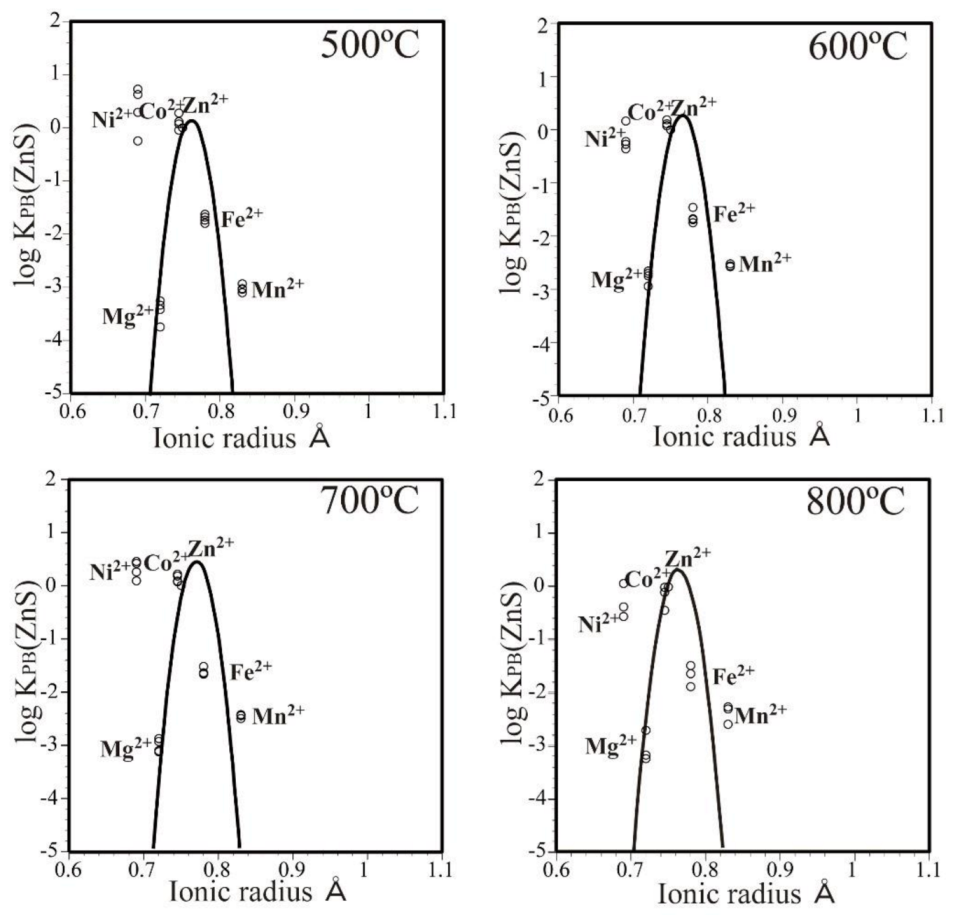
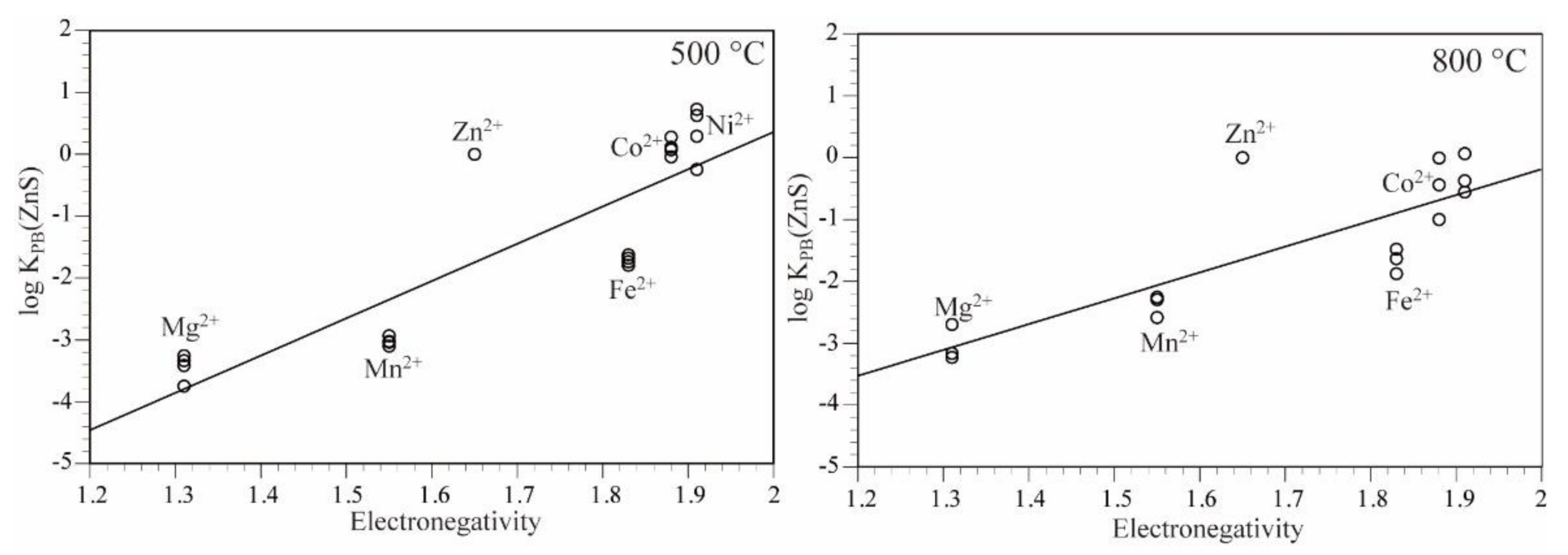
3.3. Partition Behavior of the Elements
3.4. Application of Experimental Results to Natural System
4. Conclusions
- (1)
- The bulk partition coefficient of divalent metal ions (KPB(ZnS) = (xMeS/xZnS)/(mMeaq/mZnaq)) between sphalerite and aqueous chloride solution followed the order Zn ≑ Co ≑ Ni > Fe > Mn > Mg. With the exception of Mg and Mn, almost no temperature dependence was observed for 500–800 °C.
- (2)
- The optimum ionic radius for sphalerite was slightly larger than the Zn ionic radius (~0.76 Å).
- (3)
- Because Zn is in the four-fold coordinated site in sphalerite, unlike minerals with a six-fold coordinated site, no negative partition anomaly was observed, and the partition appeared to follow the ionic radius.
- (4)
- Ni showed a positive partition anomaly in the four-fold coordinated site of sphalerite and in the six-fold coordinated site of sulfide minerals, arsenic sulfide minerals, and arsenide minerals. This is likely because of a large Ni electronegativity.
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Roedder, E. Fluid inclusions. In Reviews in Mineralogy; Mineralogical Society of America: Washington, DC, USA, 1984; Volume 12, pp. 109–148. [Google Scholar]
- Uchida, E.; Hata, Y.; Uemura, H. Experiments on the simultaneous partitioning of divalent cations between pyrite or pyrrhotite and 2M aqueous chloride solution under supercritical conditions. Jpn. Mag. Mineral. Petrol. Sci. 2017, 46, 124–134, (In Japanese with English Abstract). [Google Scholar]
- Uchida, E.; Murasugi, M.; Okuda, S. Simultaneous partitioning of divalent metal ions between alabandite and 1 mol/L (Ni, Mg, Co, Zn, Fe)Cl2 aqueous solutions under supercritical conditions. Minerals 2020, 10, 696. [Google Scholar] [CrossRef]
- Uchida, E.; Izumi, Y.; Watanabe, H. Experiments on the simultaneous partitioning of divalent cations between arsenopyrite or cobaltite and hydrothermal chloride solution under supercritical conditions. Jpn. Mag. Mineral. Petrol. Sci. 2019, 48, 103–112, (In Japanese with English Abstract). [Google Scholar] [CrossRef]
- Uchida, E.; Sugino, Y.; Yokoyama, H. Experimental investigation of the simultaneous partitioning of divalent cations between löllingite or safflorite and 2 mol/L aqueous chloride solutions under supercritical conditions. J. Mineral. Petrol. Sci. 2020, 115, 365–374. [Google Scholar] [CrossRef]
- Uchida, E.; Fukuma, M.; Sato, F.; Endo, S. Experiments on simultaneous partitioning of divalent metal ions between sphalerite or garnet and 2M aqueous chloride solutions under supercritical conditions. Jpn. Mag. Mineral. Petrol. Sci. 2004, 33, 151–162, (In Japanese with English Abstract). [Google Scholar]
- Scott, S.D.; Barnes, H.L. Sphalerite-wurtzite equilibria and stoichiometry. Geochem. Cosmochim. Acta 1972, 36, 1275–1295. [Google Scholar] [CrossRef]
- Gaines, R.V.; Skinner, H.C.W.; Foord, E.E.; Mason, B.; Rosenzweig, A.R. Dana’s New Mineralogy, 8th ed.; John Willey & Sons, Inc.: New York, NY, USA, 1997; pp. 67–69. [Google Scholar]
- Frantz, J.D.; Marshall, W.L. Electrical conductances and ionization constants of calcium chloride and magnesium chloride in aqueous solutions at temperatures to 600 °C and pressures to 4000 bars. Am. J. Sci. 1982, 282, 1666–1693. [Google Scholar] [CrossRef]
- Uchida, E.; Goryozono, Y.; Naito, M. Aqueous speciation of magnesium, strontium, nickel and cobalt chlorides in hydrothermal solutions at 600 °C and 1 kbar. Geochem. J. 1996, 30, 99–109. [Google Scholar] [CrossRef] [Green Version]
- Uchida, E.; Tsutsui, K. Cation leaching from the basalt JB-1a by 2M NaCl hydrothermal solutions. Resour. Geol. 2000, 50, 93–102. [Google Scholar] [CrossRef]
- Shannon, R.D.; Prewitt, C.T. Revised values of effective ionic radii. Acta Crystallogr. 1970, B26, 1046–1048. [Google Scholar] [CrossRef]
- Matsui, Y.; Onuma, N.; Nagasawa, H.; Higuchi, H.; Banno, S. Crystal structure control in trace element partitioning between crystal and magma. Bull. Soc. Franç. Minéral. Cristal. 1977, 100, 315–324. [Google Scholar]
- Uchida, E.; Takahashi, C.; Ohta, N. Experiments on the simultaneous partitioning of divalent metal ions between spinel group minerals (spinel and magnetite) and 2M aqueous chloride solutions under supercritical conditions. Jpn. Mag. Mineral. Petrol. Sci. 2004, 33, 1–11, (In Japanese with English Abstract). [Google Scholar]
- Matsui, Y. Behavior of elements in igneous activity. In Earth Sciences 4, Material Science of the Earth III; Matsui, Y., Banno, S., Eds.; Iwanami Shoten: Tokyo, Japan, 1979; pp. 165–189. (In Japanese) [Google Scholar]
- Pauling, L. The Nature of the Chemical Bond and the Structure of Molecules and Crystals: An Introduction to Modern Structured Chemistry, 3rd ed.; Cornell University Press: New York, NY, USA, 1960; pp. 1–664. [Google Scholar]
- Emsley, J. The Elements, 3rd ed.; Clarendon Press: Oxford, UK, 1998; pp. 1–292. [Google Scholar]
- Scott, S.D. Experimental methods in sulfide synthesis. In Sulfide Mineralogy; Ribbe, P.H., Ed.; Reviews in Mineralogy 1; Mineralogical Society of America: Washington, DC, USA, 1974; pp. S1–S38. [Google Scholar]


| Run No. | Duration | Temperature | Pressure | Solid | Fluid |
|---|---|---|---|---|---|
| Days | (°C) | (MPa) | (mg) | (μL) | |
| 501 | 14 | 500 | 100 | 28.9 | 30 1 |
| 502 | 14 | 500 | 100 | 30.0 | 30 1 |
| 503 | 14 | 500 | 100 | 30.0 | 30 1 |
| 504 | 14 | 500 | 100 | 28.7 | 30 1 |
| 601 | 7 | 600 | 100 | 30.0 | 30 1 |
| 602 | 7 | 600 | 100 | 29.9 | 30 1 |
| 603 | 7 | 600 | 100 | 30.3 | 30 1 |
| 604 | 7 | 600 | 100 | 30.1 | 30 1 |
| 701 | 5 | 700 | 100 | 24.5 | 25 1 |
| 702 | 5 | 700 | 100 | 25.4 | 25 1 |
| 703 | 5 | 700 | 100 | 25.0 | 25 1 |
| 704 | 5 | 700 | 100 | 25.5 | 25 1 |
| 801 | 3 | 800 | 100 | 19.8 | 20 1 |
| 802 | 3 | 800 | 100 | 20.4 | 20 1 |
| 803 | 3 | 800 | 100 | 20.4 | 20 1 |
| Temp., Pressure | Run No. | Mg | Mn | Fe | Co | Ni | Zn | |
|---|---|---|---|---|---|---|---|---|
| 500 °C, 100 MPa | 501 | Solid 1 | 0.0033 | 0.0033 | 0.0025 | 0.0049 | 0.0014 | 0.9845 |
| Fluid (Meaq) 2 | 0.64890 | 0.26652 | 0.01031 | 0.00028 | 0.00019 | 0.07380 | ||
| Fluid (MeCl2aq) 3 | 0.48141 | 0.16060 | 0.00817 | 0.00020 | 0.00017 | 0.05211 | ||
| log KPB | −3.4149 | −3.0374 | −1.7365 | 0.1143 | −0.2463 | 0.0000 | ||
| log KPN | −3.4363 | −2.9685 | −1.7863 | 0.1143 | −0.3542 | 0.0000 | ||
| 502 | Solid 1 | 0.0016 | 0.0034 | 0.0027 | 0.0044 | 0.0016 | 0.9863 | |
| Fluid (Meaq) 2 | 0.65298 | 0.26507 | 0.00939 | 0.00028 | 0.00003 | 0.07226 | ||
| Fluid (MeCl2aq) 3 | 0.48566 | 0.15916 | 0.00742 | 0.00019 | 0.00002 | 0.05089 | ||
| log KPB | −3.7471 | −3.0234 | −1.6795 | 0.0724 | 0.6264 | 0.0000 | ||
| log KPN | −3.7708 | −2.9541 | −1.7297 | 0.0724 | 0.5177 | 0.0000 | ||
| 503 | Solid 1 | 0.0051 | 0.0030 | 0.0022 | 0.0025 | 0.0008 | 0.9865 | |
| Fluid (Meaq) 2 | 0.65069 | 0.26862 | 0.00993 | 0.00020 | 0.00003 | 0.07053 | ||
| Fluid (MeCl2aq) 3 | 0.48307 | 0.16167 | 0.00786 | 0.00014 | 0.00003 | 0.04976 | ||
| log KPB | −3.2555 | −3.1008 | −1.7957 | −0.0429 | 0.2908 | 0.0000 | ||
| log KPN | −3.2776 | −3.0318 | −1.8457 | −0.0429 | 0.1826 | 0.0000 | ||
| 504 | Solid 1 | 0.0036 | 0.0037 | 0.0026 | 0.0043 | 0.0018 | 0.9839 | |
| Fluid (Meaq) 2 | 0.64770 | 0.26159 | 0.00918 | 0.00019 | 0.00003 | 0.08131 | ||
| Fluid (MeCl2aq) 3 | 0.48044 | 0.15774 | 0.00727 | 0.00013 | 0.00003 | 0.05745 | ||
| log KPB | −3.3356 | −2.9341 | −1.6262 | 0.2773 | 0.7265 | 0.0000 | ||
| log KPN | −3.3567 | −2.8653 | −1.6760 | 0.2773 | 0.6187 | 0.0000 | ||
| 600 °C, 100 MPa | 601 | Solid 1 | 0.0064 | 0.0072 | 0.0024 | 0.0044 | 0.0028 | 0.9768 |
| Fluid (Meaq) 2 | 0.45739 | 0.38559 | 0.01699 | 0.00055 | 0.00027 | 0.13922 | ||
| Fluid (MeCl2aq) 3 | 0.35369 | 0.30504 | 0.01538 | 0.00047 | 0.00026 | 0.11934 | ||
| log KPB | −2.7015 | −2.5772 | −1.6910 | 0.0630 | 0.1631 | 0.0000 | ||
| log KPN | −2.6568 | −2.5423 | −1.7145 | 0.0630 | 0.1140 | 0.0000 | ||
| 602 | Solid 1 | 0.0035 | 0.0077 | 0.0022 | 0.0054 | 0.0030 | 0.9781 | |
| Fluid (Meaq) 2 | 0.4585 | 0.3806 | 0.0152 | 0.0005 | 0.0010 | 0.1442 | ||
| Fluid (MeCl2aq) 3 | 0.3550 | 0.3009 | 0.0137 | 0.0004 | 0.0010 | 0.1235 | ||
| Log KPB | −2.9437 | −2.5232 | −1.6733 | 0.1862 | −0.3596 | 0.0000 | ||
| Log KPN | −2.8997 | −2.4882 | −1.6969 | 0.1862 | −0.4089 | 0.0000 | ||
| 603 | Solid 1 | 0.0056 | 0.0070 | 0.0020 | 0.0049 | 0.0020 | 0.9786 | |
| Fluid (Meaq) 2 | 0.46019 | 0.37695 | 0.01698 | 0.00056 | 0.00056 | 0.14477 | ||
| Fluid (MeCl2aq) 3 | 0.35680 | 0.29769 | 0.01535 | 0.00048 | 0.00053 | 0.12394 | ||
| log KPB | −2.7481 | −2.5620 | −1.7497 | 0.1111 | −0.2742 | 0.0000 | ||
| log KPN | −2.7050 | −2.5269 | −1.7734 | 0.1111 | −0.3237 | 0.0000 | ||
| 604 | Solid 1 | 0.0072 | 0.0074 | 0.0041 | 0.0042 | 0.0034 | 0.9737 | |
| Fluid (Meaq) 2 | 0.45951 | 0.38740 | 0.01649 | 0.00049 | 0.00049 | 0.13561 | ||
| Fluid (MeCl2aq) 3 | 0.35554 | 0.30612 | 0.01491 | 0.00042 | 0.00047 | 0.11616 | ||
| log KPB | −2.6582 | −2.5767 | −1.4611 | 0.0726 | −0.0222 | 0.0000 | ||
| log KPN | −2.6140 | −2.5417 | −1.4847 | 0.0726 | −0.0716 | 0.0000 | ||
| 700 °C, 100 MPa | 701 | Solid 1 | 0.0037 | 0.0078 | 0.0022 | 0.0054 | 0.0031 | 0.9777 |
| Fluid (Meaq) 2 | 0.46117 | 0.36207 | 0.01582 | 0.00055 | 0.00018 | 0.16021 | ||
| Fluid (MeCl2aq) 3 | 0.42597 | 0.33521 | 0.01533 | 0.00052 | 0.00018 | 0.15250 | ||
| log KPB | −2.8805 | −2.4501 | −1.6513 | 0.2124 | 0.4508 | 0.0000 | ||
| log KPN | −2.8675 | −2.4381 | −1.6591 | 0.2124 | 0.4349 | 0.0000 | ||
| 702 | Solid 1 | 0.0020 | 0.0079 | 0.0026 | 0.0050 | 0.0036 | 0.9790 | |
| Fluid (Meaq) 2 | 0.4534 | 0.3623 | 0.0149 | 0.0007 | 0.0002 | 0.1684 | ||
| Fluid (MeCl2aq) 3 | 0.4185 | 0.3360 | 0.0144 | 0.0007 | 0.0002 | 0.1605 | ||
| log KPB | −3.1275 | −2.4286 | −1.5241 | 0.0828 | 0.4167 | 0.0000 | ||
| log KPN | −3.1137 | −2.4167 | −1.5317 | 0.0828 | 0.4010 | 0.0000 | ||
| 703 | Solid 1 | 0.0022 | 0.0079 | 0.0022 | 0.0044 | 0.0034 | 0.9799 | |
| Fluid (Meaq) 2 | 0.45455 | 0.36658 | 0.01673 | 0.00062 | 0.00031 | 0.16122 | ||
| Fluid (MeCl2aq) 3 | 0.41947 | 0.33979 | 0.01622 | 0.00059 | 0.00031 | 0.15358 | ||
| log KPB | −3.1067 | −2.4491 | −1.6676 | 0.0696 | 0.2537 | 0.0000 | ||
| log KPN | −3.0929 | −2.4372 | −1.6753 | 0.0696 | 0.2380 | 0.0000 | ||
| 704 | Solid 1 | 0.0035 | 0.0077 | 0.0024 | 0.0056 | 0.0034 | 0.9775 | |
| Fluid (Meaq) 2 | 0.45666 | 0.37653 | 0.01591 | 0.00057 | 0.00043 | 0.14990 | ||
| Fluid (MeCl2) 3 | 0.42119 | 0.34883 | 0.01543 | 0.00054 | 0.00042 | 0.14275 | ||
| log KPB | −2.9347 | −2.5012 | −1.6424 | 0.1775 | 0.0874 | 0.0000 | ||
| log KPN | −2.9208 | −2.4893 | −1.6501 | 0.1775 | 0.0716 | 0.0000 | ||
| 800 °C, 100 MPa | 801 | Solid 1 | 0.0033 | 0.0103 | 0.0026 | 0.0040 | 0.0015 | 0.9783 |
| Fluid (Meaq) 2 | 0.51843 | 0.36967 | 0.01830 | 0.00105 | 0.00052 | 0.09203 | ||
| Fluid (MeCl2aq) 3 | 0.50382 | 0.35731 | 0.01805 | 0.00102 | 0.00052 | 0.09006 | ||
| log KPB | −3.2287 | −2.5835 | −1.8757 | −0.4398 | −0.5559 | 0.0000 | ||
| log KPN | −3.2257 | −2.5781 | −1.8792 | −0.4398 | −0.5629 | 0.0000 | ||
| 802 | Solid 1 | 0.0017 | 0.0101 | 0.0028 | 0.0039 | 0.0031 | 0.9783 | |
| Fluid (Meaq) 2 | 0.46685 | 0.33448 | 0.01578 | 0.00091 | 0.00137 | 0.18061 | ||
| Fluid (MeCl2aq) 3 | 0.45317 | 0.32447 | 0.01558 | 0.00090 | 0.00137 | 0.17717 | ||
| log KPB | −3.1634 | −2.2557 | −1.4793 | −0.0999 | −0.3742 | 0.0000 | ||
| log KPN | −3.1589 | −2.2509 | −1.4824 | −0.0999 | −0.3804 | 0.0000 | ||
| 803 | Solid 1 | 0.0048 | 0.0088 | 0.0019 | 0.0043 | 0.0026 | 0.9775 | |
| Fluid (Meaq) 2 | 0.46094 | 0.33432 | 0.01588 | 0.00085 | 0.00042 | 0.18759 | ||
| Fluid (MeCl2aq) 3 | 0.44731 | 0.32445 | 0.01569 | 0.00083 | 0.00042 | 0.18406 | ||
| log KPB | −2.6965 | −2.2958 | −1.6331 | −0.0069 | 0.0643 | 0.0000 | ||
| log KPN | −2.6917 | −2.2910 | −1.6361 | −0.0069 | 0.0581 | 0.0000 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Uchida, E.; Wakamatsu, K.; Takamatsu, N. Simultaneous Partition Experiment of Divalent Metal Ions between Sphalerite and 1 mol/L (Ni, Mg, Co, Fe, Mn)Cl2 Aqueous Solution under Supercritical Conditions. Minerals 2021, 11, 435. https://doi.org/10.3390/min11040435
Uchida E, Wakamatsu K, Takamatsu N. Simultaneous Partition Experiment of Divalent Metal Ions between Sphalerite and 1 mol/L (Ni, Mg, Co, Fe, Mn)Cl2 Aqueous Solution under Supercritical Conditions. Minerals. 2021; 11(4):435. https://doi.org/10.3390/min11040435
Chicago/Turabian StyleUchida, Etsuo, Keiko Wakamatsu, and Naoki Takamatsu. 2021. "Simultaneous Partition Experiment of Divalent Metal Ions between Sphalerite and 1 mol/L (Ni, Mg, Co, Fe, Mn)Cl2 Aqueous Solution under Supercritical Conditions" Minerals 11, no. 4: 435. https://doi.org/10.3390/min11040435
APA StyleUchida, E., Wakamatsu, K., & Takamatsu, N. (2021). Simultaneous Partition Experiment of Divalent Metal Ions between Sphalerite and 1 mol/L (Ni, Mg, Co, Fe, Mn)Cl2 Aqueous Solution under Supercritical Conditions. Minerals, 11(4), 435. https://doi.org/10.3390/min11040435






