Effects of Freeze–Thaw Cycling on Dynamic Compressive Strength and Energy Dissipation of Sandstone
Abstract
:1. Introduction
2. Specimen Preparation
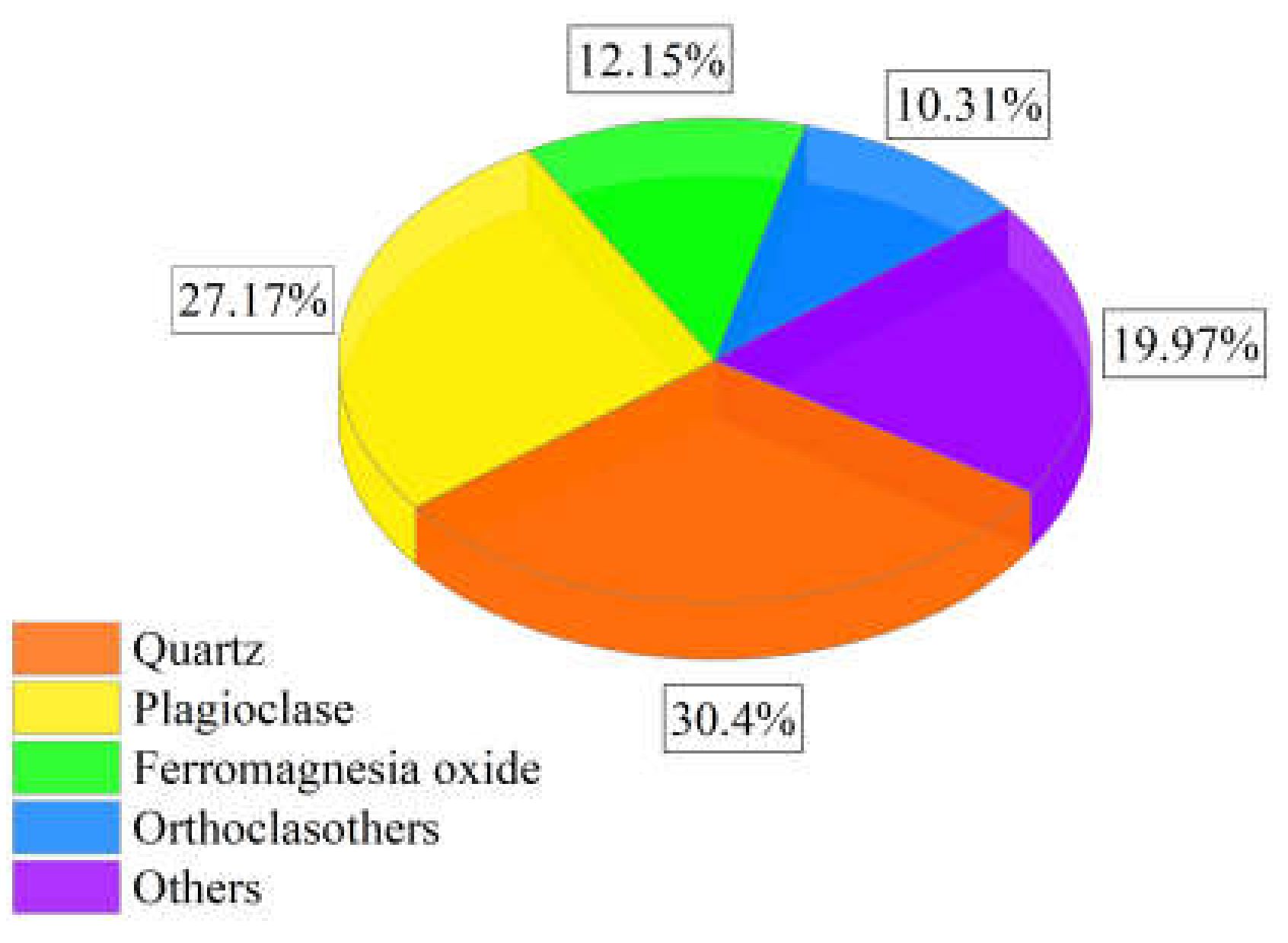
2.1. Specimen Configuration and Basic Properties
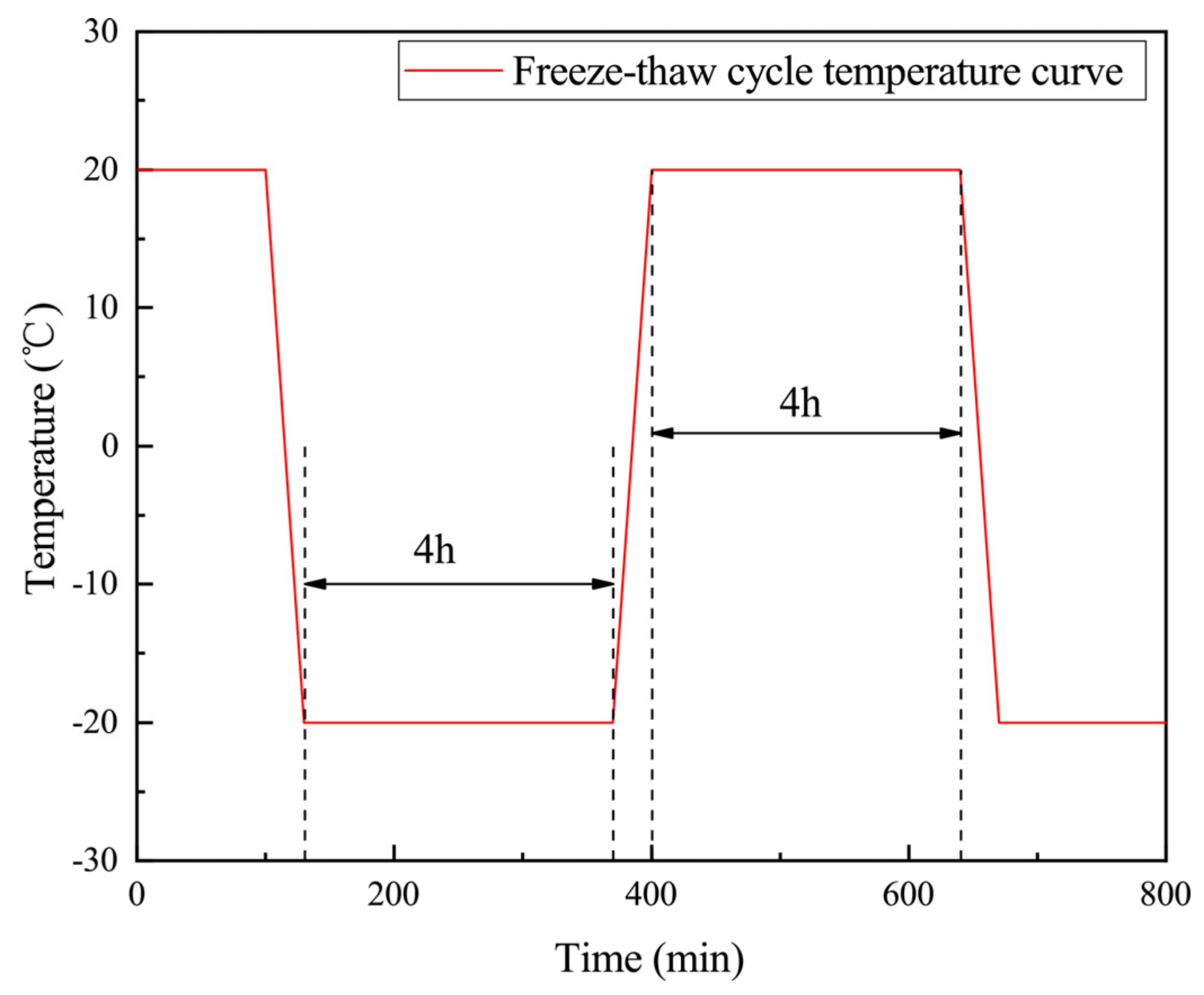
2.2. Freeze–Thaw Cycling Weathering
3. Experimental Techniques
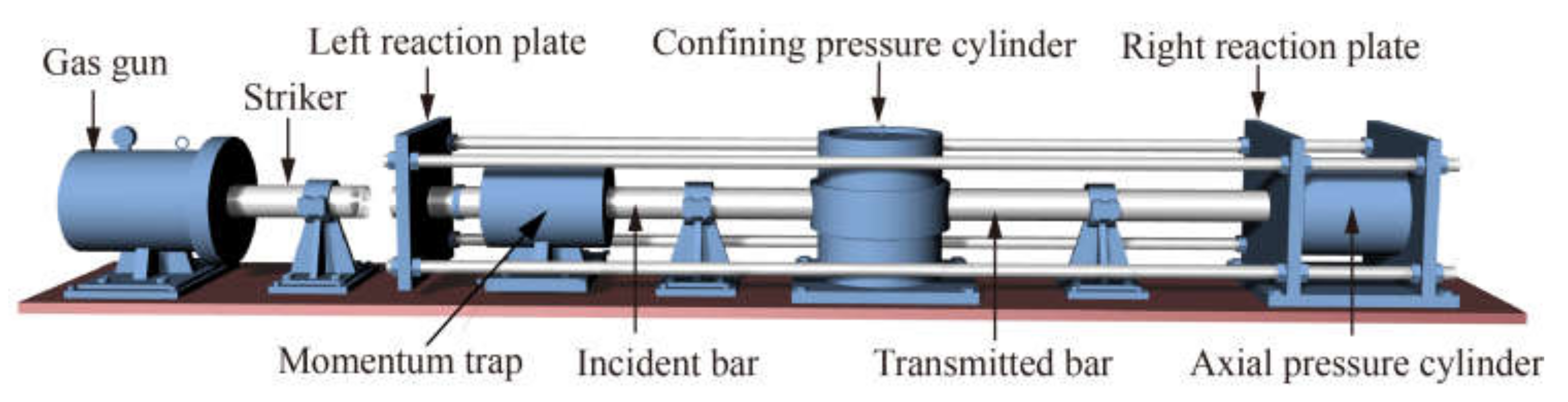
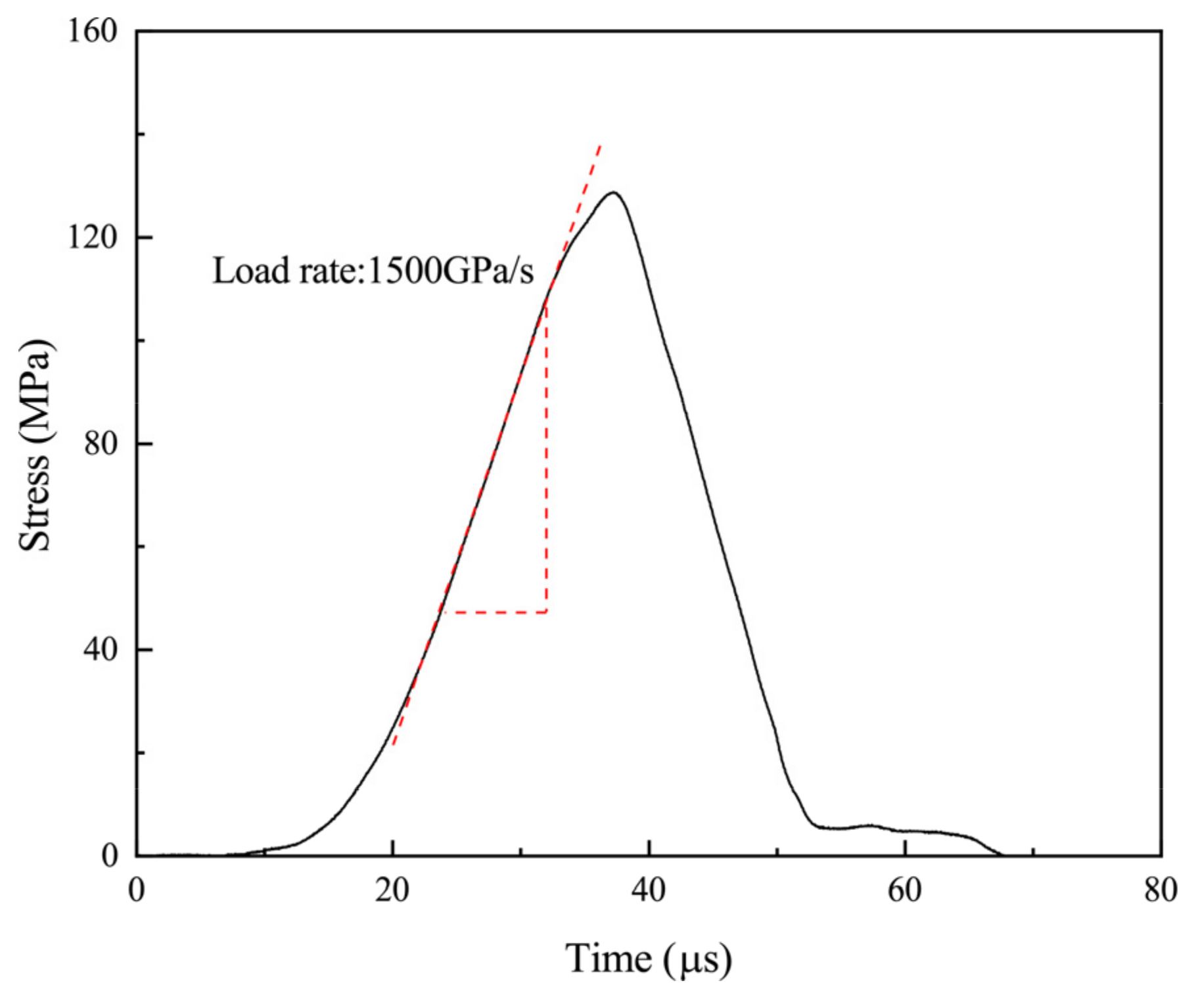
3.1. Loading System
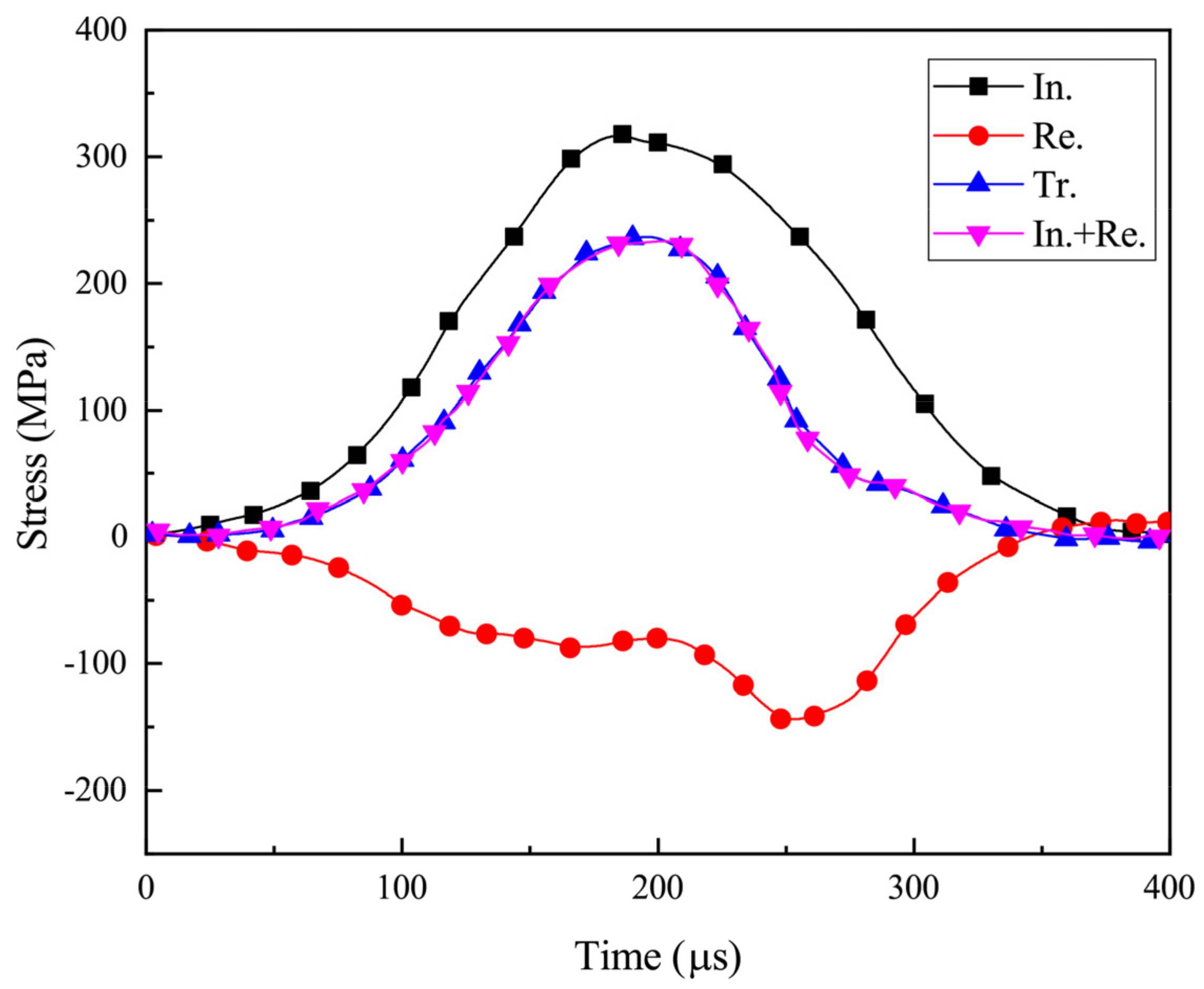
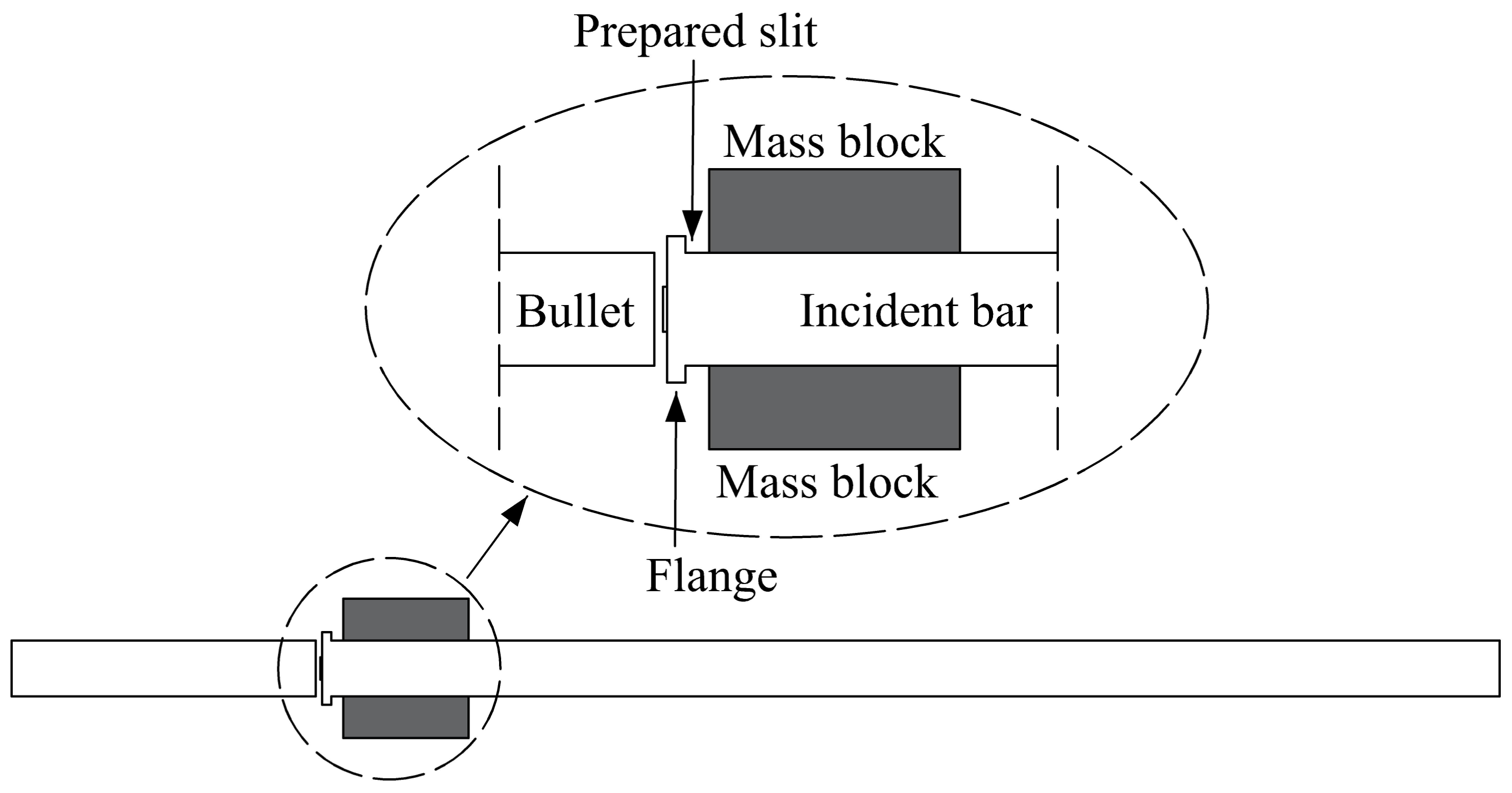
3.2. Single-Loading Technology
4. Results and Discussions
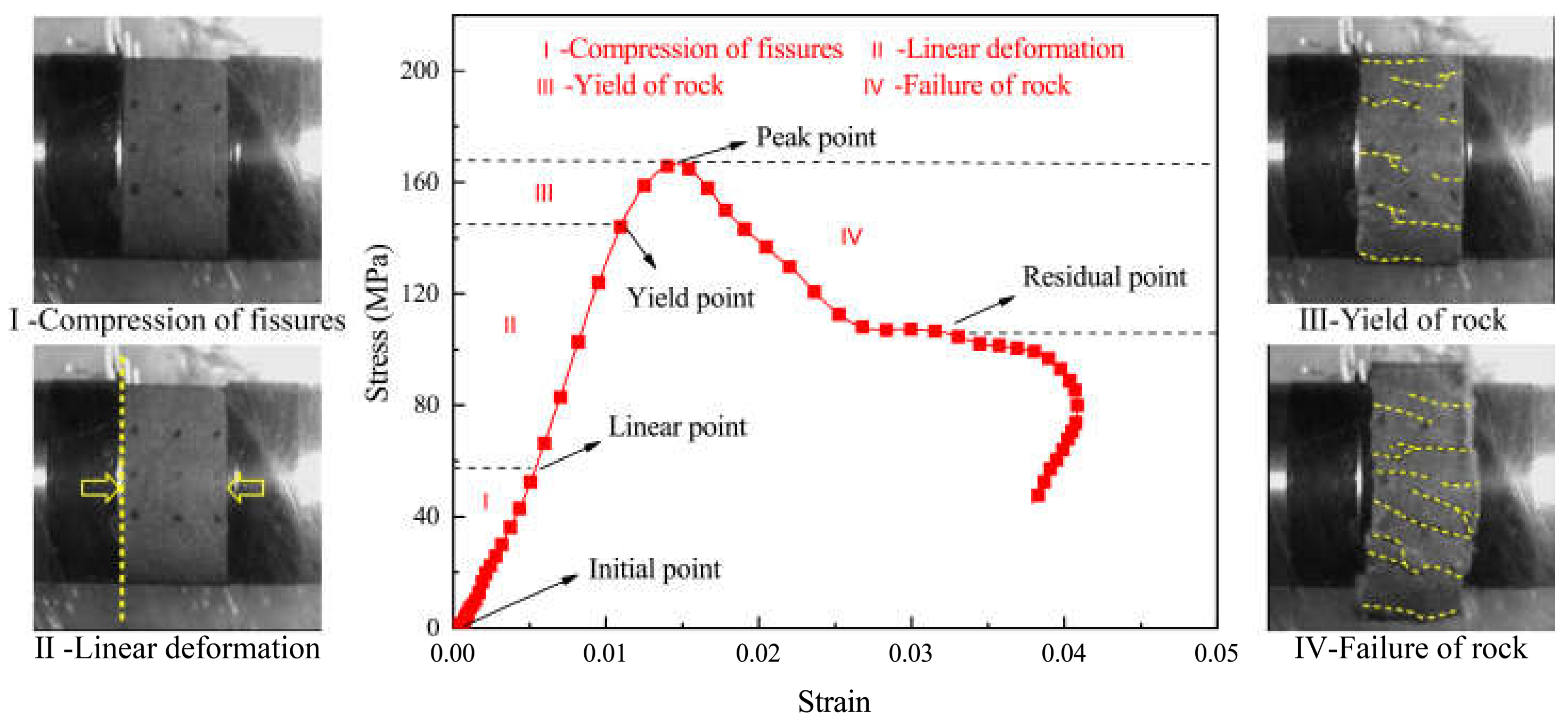
4.1. Stress–Strain Curve
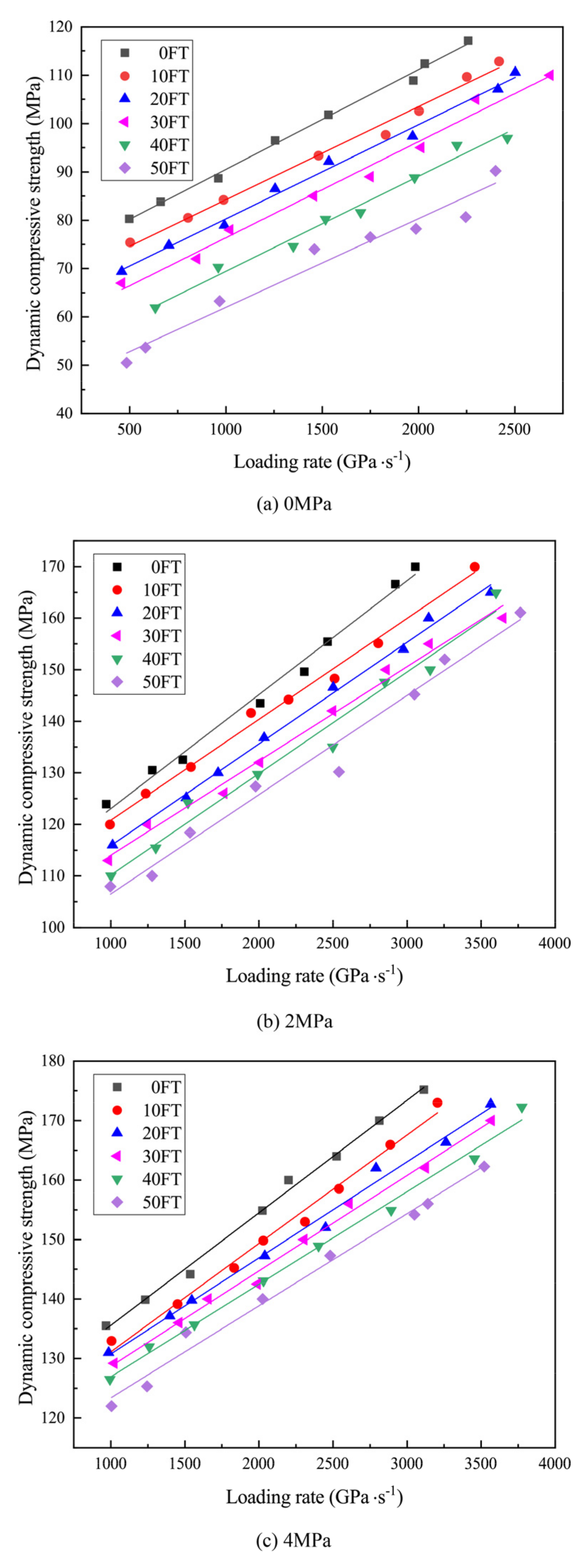
4.2. Dynamic Compressive Strength
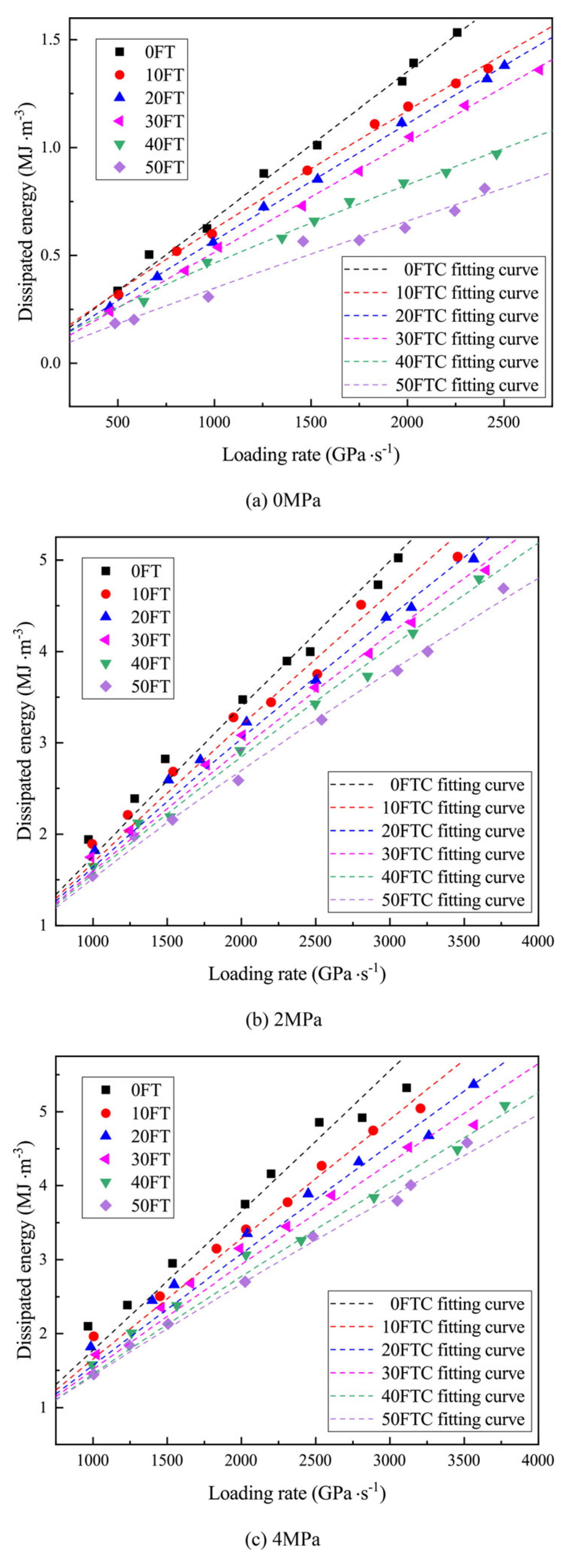
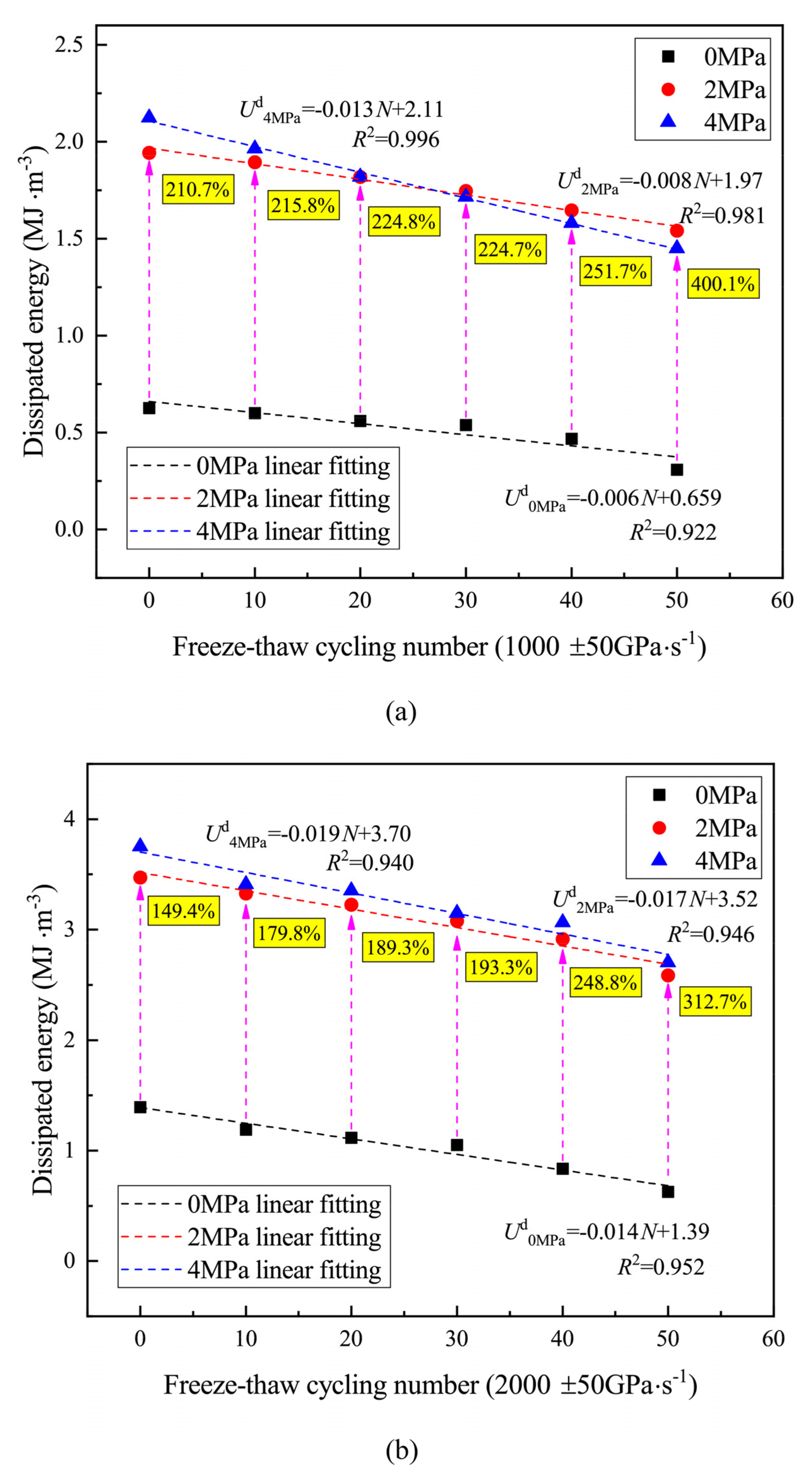
4.3. Dissipated Energy
4.4. Dissipated Energy vs. Dynamic Compression Strength
4.5. Damage Characteristics Analysis
4.5.1. Average Fragment Size
4.5.2. CT Value
5. Conclusions
- (1)
- The dynamic failure process after FTC had obvious stages, and its stress–strain curve could be roughly divided into four stages: compression of fissures, linear deformation of rock, yield of rock, and failure of rock. Under different working conditions, the rock destruction phases were different. Under the effect of high loading rate and hydrostatic pressure, the stress–strain curve rebounded after a relatively gentle stage. This phenomenon became more obvious as the loading rate increased;
- (2)
- The dynamic compression strength of rock increased linearly with the increase in the loading rate and decreased with the increase in FTCs. Under the same conditions of loading rate and FTC, the hydrostatic pressure could enhance dynamic compression strength, and this enhancement effect increased with the increase in the number of FTCs;
- (3)
- The dissipated energy of sandstone specimens was affected significantly by FTC, hydrostatic pressure, and loading rate. The dissipated energy of sandstone specimens was negatively related to the number of FTCs, positively related to hydrostatic pressure, and positively related to the loading rate. An empirical equation of dissipated energy with freeze–thaw cycling, hydrostatic pressure, and loading rate was proposed, and the fitting parameters were provided. After 40 FT cycles, the internal damage of the specimens reached a larger value, which desensitized the energy required for specimens to reach breakage to the loading rate, but the sensitivity did not decrease under the effect of hydrostatic pressure. Freeze–thaw weathering reduced the effect of hydrostatic pressure on dissipated energy, and hydrostatic pressure enhanced the effect of FTC on the dissipated energy of the specimens;
- (4)
- The dissipated energy of the specimen corresponded to the dynamic compression strength according to a quadratic function, and the correspondence was almost independent of the FTCs, loading rate, and hydrostatic pressure;
- (5)
- The average fragment size after damage decreased with the increase in FTCs for specimens that were not subjected to hydrostatic pressure. For the specimens subjected to hydrostatic pressure, the appearance remained intact after loading, and the CT value decreased with the increase in the number of FTCs. Both phenomena indicated that FTC exacerbated the internal damage of rock. It was also found that 40 FTCs was an important number of FTCs for green sandstone whereby the internal damage reached a large value, which was consistent with the result given by the energy analysis.
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Park, J.; Hyun, C.U.; Park, H.D. Changes in microstructure and physical properties of rocks caused by artificial freeze-thaw action. Bull. Eng. Geol. Environ. 2015, 74, 555–565. [Google Scholar] [CrossRef]
- Deprez, M.; De Kock, T.; De Schutter, G.; Cnudde, V. A review on freeze-thaw action and weathering of rocks. Earth-Sci. Rev. 2020, 203, 103143. [Google Scholar] [CrossRef]
- Eppes, M.C.; Keanini, R. Mechanical weathering and rock erosion by climate-dependent subcritical cracking. Rev. Geophys. 2017, 55, 470–508. [Google Scholar] [CrossRef]
- Liu, W.W.; Feng, Q.; Wang, C.X.; Lu, C.K.; Xu, Z.Z.; Li, W.T. Analytical solution for three-dimensional radial heat transfer in a cold-region tunnel. Cold Reg. Sci. Technol. 2019, 164, 102787. [Google Scholar] [CrossRef]
- Luo, X.D.; Jiang, N.; Fan, X.Y.; Mei, N.F.; Luo, H. Effects of freeze-thaw on the determination and application of parameters of slope rock mass in cold regions. Cold Reg. Sci. Technol. 2015, 110, 32–37. [Google Scholar] [CrossRef]
- Pudasaini, S.P.; Krautblatter, M. A two-phase mechanical model for rock-ice avalanches. J. Geophys. Res.-Earth Surf. 2014, 119, 2272–2290. [Google Scholar] [CrossRef] [Green Version]
- Huang, S.B.; Liu, Y.Z.; Guo, Y.L.; Zhang, Z.L.; Cai, Y.T. Strength and failure characteristics of rock-like material containing single crack under freeze-thaw and uniaxial compression. Cold Reg. Sci. Technol. 2019, 162, 1–10. [Google Scholar] [CrossRef]
- Khanlari, G.; Sahamieh, R.Z.; Abdilor, Y. The effect of freeze-thaw cycles on physical and mechanical properties of Upper Red Formation sandstones, central part of Iran. Arab. J. Geosci. 2015, 8, 5991–6001. [Google Scholar] [CrossRef]
- Walbert, C.; Eslami, J.; Beaucour, A.L.; Bourges, A.; Noumowe, A. Evolution of the mechanical behaviour of limestone subjected to freeze-thaw cycles. Environ. Earth Sci. 2015, 74, 6339–6351. [Google Scholar] [CrossRef]
- Yavuz, H. Effect of freeze-thaw and thermal shock weathering on the physical and mechanical properties of an andesite stone. Bull. Eng. Geol. Environ. 2011, 70, 187–192. [Google Scholar] [CrossRef]
- Park, C.; Synn, J.H.; Shin, H.S.; Cheon, D.S.; Lim, H.D.; Jeon, S.W. An experimental study on the thermal characteristics of rock at low temperatures. Int. J. Rock Mech. Min. Sci. 2004, 41, 367–368. [Google Scholar] [CrossRef]
- Thomachot, C.; Matsuoka, N. Dilation of building materials submitted to frost action. In Proceedings of the General Assembly of the European-Union-of-Geosciences, Vienna, Austria, 24–29 April 2005; p. 167. [Google Scholar]
- Tan, X.J.; Chen, W.Z.; Yang, J.P.; Cao, J.J. Laboratory investigations on the mechanical properties degradation of granite under freeze-thaw cycles. Cold Reg. Sci. Technol. 2011, 68, 130–138. [Google Scholar] [CrossRef]
- Del Rio, L.M.; Lopez, F.; Esteban, F.J.; Tejado, J.J.; Mota, M.I.; Gonzalez, I.; Ramos, A.; San Emeterio, J.L. Ultrasonic study of alteration processes in granites caused by freezing and thawing. In Proceedings of the IEEE International Ultrasonics Symposium, Rotterdam, The Netherlands, 18–21 September 2005; pp. 419–422. [Google Scholar]
- Martinez-Martinez, J.; Benavente, D.; Gomez-Heras, M.; Marco-Castano, L.; Garcia-del-Cura, M.A. Non-linear decay of building stones during freeze-thaw weathering processes. Constr. Build. Mater. 2013, 38, 443–454. [Google Scholar] [CrossRef]
- Kodama, J.; Goto, T.; Fujii, Y.; Hagan, P. The effects of water content, temperature and loading rate on strength and failure process of frozen rocks. Int. J. Rock Mech. Min. Sci. 2013, 62, 1–13. [Google Scholar] [CrossRef]
- Li, X.; Lu, Y.; Weng, Y. Research on Damage Model of Single Jointed Rock Masses under Coupling Action of Freeze-Thaw and Loading. Chin. J. Rock Mech. Eng. 2013, 32, 2307–2315. [Google Scholar]
- Nicholson, D.T.; Nicholson, F.H. Physical deterioration of sedimentary rocks subjected to experimental freeze-thaw weathering. Earth Surf. Process. Landf. 2000, 25, 1295–1307. [Google Scholar] [CrossRef]
- Yamabe, T.; Neaupane, K.M. Determination of some thermo-mechanical properties of Sirahama sandstone under subzero temperature condition. Int. J. Rock Mech. Min. Sci. 2001, 38, 1029–1034. [Google Scholar] [CrossRef]
- Jamshidi, A.; Nikudel, M.R.; Khamehchiyan, M.; Zalooli, A. Statistical Models for Predicting the Mechanical Properties of Travertine Building Stones After Freeze-Thaw Cycles. In Proceedings of the 12th International IAEG Congress, Torino, Italy, 15–19 September 2014; pp. 477–481. [Google Scholar]
- Wang, Y.; Song, Z.Y.; Mao, T.Q.; Zhu, C. Macro-Meso Fracture and Instability Behaviors of Hollow-Cylinder Granite Containing Fissures Subjected to Freeze-Thaw-Fatigue Loads. Rock Mech. Rock Eng. 2022, 55, 4051–4071. [Google Scholar] [CrossRef]
- Chen, T.C.; Yeung, M.R.; Mori, N. Effect of water saturation on deterioration of welded tuff due to freeze-thaw action. Cold Reg. Sci. Technol. 2004, 38, 127–136. [Google Scholar] [CrossRef]
- Bayram, F. Predicting mechanical strength loss of natural stones after freeze-thaw in cold regions. Cold Reg. Sci. Technol. 2012, 83–84, 98–102. [Google Scholar] [CrossRef]
- Jamshidi, A. Predicting the Strength of Granitic Stones after Freeze-Thaw Cycles: Considering the Petrographic Characteristics and a New Approach Using Petro-Mechanical Parameter. Rock Mech. Rock Eng. 2021, 54, 2829–2841. [Google Scholar] [CrossRef]
- Ren, J.X.; Yun, M.C.; Cao, X.T.; Zhang, K.; Liang, Y.; Chen, X. Study on the mechanical properties of saturated red sandstone under freeze-thaw conditions. Environ. Earth Sci. 2022, 81, 376. [Google Scholar] [CrossRef]
- Xia, K.; Yao, W. Dynamic rock tests using split Hopkinson (Kolsky) bar system—A review. J. Rock Mech. Geotech. Eng. 2015, 7, 27–59. [Google Scholar] [CrossRef] [Green Version]
- Zhang, Q.B.; Zhao, J. Determination of mechanical properties and full-field strain measurements of rock material under dynamic loads. Int. J. Rock Mech. Min. Sci. 2013, 60, 423–439. [Google Scholar] [CrossRef]
- Dai, F.; Xia, K.W. Loading Rate Dependence of Tensile Strength Anisotropy of Barre Granite. Pure Appl. Geophys. 2010, 167, 1419–1432. [Google Scholar] [CrossRef]
- Ma, Q.Y.; Ma, D.D.; Yao, Z.M. Influence of freeze-thaw cycles on dynamic compressive strength and energy distribution of soft rock specimen. Cold Reg. Sci. Technol. 2018, 153, 10–17. [Google Scholar] [CrossRef]
- Wang, P.; Xu, J.Y.; Liu, S.; Liu, S.H.; Wang, H.Y. A prediction model for the dynamic mechanical degradation of sedimentary rock after a long-term freeze-thaw weathering: Considering the strain-rate effect. Cold Reg. Sci. Technol. 2016, 131, 16–23. [Google Scholar] [CrossRef] [Green Version]
- Weng, L.; Wu, Z.J.; Liu, Q.S. Dynamic Mechanical Properties of Dry and Water-Saturated Siltstones Under Sub-Zero Temperatures. Rock Mech. Rock Eng. 2020, 53, 4381–4401. [Google Scholar] [CrossRef]
- Zhou, K.P.; Li, B.; Li, J.L.; Deng, H.W.; Bin, F. Microscopic damage and dynamic mechanical properties of rock under freeze-thaw environment. Trans. Nonferrous Met. Soc. China 2015, 25, 1254–1261. [Google Scholar] [CrossRef]
- Xie, H.; Ju, Y.; Li, L. Criteria for Strength and Structural Failure of Rocks Based on Energy Dissipation and Energy Release Principles. Chin. J. Rock Mech. Eng. 2005, 24, 3003–3010. [Google Scholar]
- Khazaei, C.; Hazzard, J.; Chalaturnyk, R. Damage quantification of intact rocks using acoustic emission energies recorded during uniaxial compression test and discrete element modeling. Comput. Geotech. 2015, 67, 94–102. [Google Scholar] [CrossRef]
- Wang, P.; Xu, J.Y.; Fang, X.Y.; Wang, P.X. Energy dissipation and damage evolution analyses for the dynamic compression failure process of red-sandstone after freeze-thaw cycles. Eng. Geol. 2017, 221, 104–113. [Google Scholar] [CrossRef]
- Feng, Q.; Jin, J.C.; Zhang, S.; Liu, W.W.; Yang, X.X.; Li, W.T. Study on a Damage Model and Uniaxial Compression Simulation Method of Frozen-Thawed Rock. Rock Mech. Rock Eng. 2022, 55, 187–211. [Google Scholar] [CrossRef]
- Lundberg, B. Split Hopkinson Bar Study of Energy-Absorption in Dynamic Rock Fragmentation. Int. J. Rock Mech. Min. Sci. 1976, 13, 187–197. [Google Scholar] [CrossRef]
- Li, X.B.; Lok, T.S.; Zhao, J. Dynamic characteristics of granite subjected to intermediate loading rate. Rock Mech. Rock Eng. 2005, 38, 21–39. [Google Scholar] [CrossRef]
- Deng, H.W.; Yu, S.T.; Deng, J.R.; Ke, B.; Bin, F. Experimental Investigation on Energy Mechanism of Freezing-Thawing Treated Sandstone under Uniaxial Static Compression. Ksce J. Civ. Eng. 2019, 23, 2074–2082. [Google Scholar] [CrossRef]
- Gong, F.Q.; Si, X.F.; Li, X.B.; Wang, S.Y. Dynamic triaxial compression tests on sandstone at high strain rates and low confining pressures with split Hopkinson pressure bar. Int. J. Rock Mech. Min. Sci. 2019, 113, 211–219. [Google Scholar] [CrossRef]
- Wu, B.; Xia, K. An experimental study of dynamic tensile failure of rocks subjected to hydrostatic confinement. In Proceedings of the 2nd International Conference on Rock Dynamics and Applications (RocDyn), Suzhou, China, 18–19 May 2016; pp. 197–202. [Google Scholar]
- Xu, Y.; Yao, W.; Xia, K.W.; Ghaffari, H.O. Experimental Study of the Dynamic Shear Response of Rocks Using a Modified Punch Shear Method. Rock Mech. Rock Eng. 2019, 52, 2523–2534. [Google Scholar] [CrossRef]
- Zhou, Y.X.; Xia, K.; Li, X.B.; Li, H.B.; Ma, G.W.; Zhao, J.; Zhou, Z.L.; Dai, F. Suggested methods for determining the dynamic strength parameters and mode-I fracture toughness of rock materials. Int. J. Rock Mech. Min. Sci. 2012, 49, 105–112. [Google Scholar] [CrossRef]
- Xu, Y.; Chen, B.Q.; Wu, B.B.; Chen, Z.; Yang, L.; Li, P.F. Influence of freeze-thaw cycling on the dynamic compressive failure of rocks subjected to hydrostatic pressure. Bull. Eng. Geol. Environ. 2022, 81, 276. [Google Scholar] [CrossRef]
- Song, B.; Chen, W. Loading and unloading split Hopkinson pressure bar pulse-shaping techniques for dynamic hysteretic loops. Exp. Mech. 2004, 44, 622–627. [Google Scholar] [CrossRef]
- Rong, C.; FangYun, L.U.; YuLiang, L.I.N.; JinGui, Q.I.N. Investigation of the Pre-fixed Gap in Single-Pulse Loading Technique of Hopkinson Pressure Bars. Chin. J. High Press. Phys. 2008, 22, 187–191. [Google Scholar]
- Ping, Q.; Luo, X.; Ma, Q.Y.; Yuan, P. Broken energy dissipation characteristics of sandstone specimens under impact loads. Chin. J. Rock Mech. Eng. 2015, 34, 4197–4203. (In Chinese) [Google Scholar] [CrossRef]


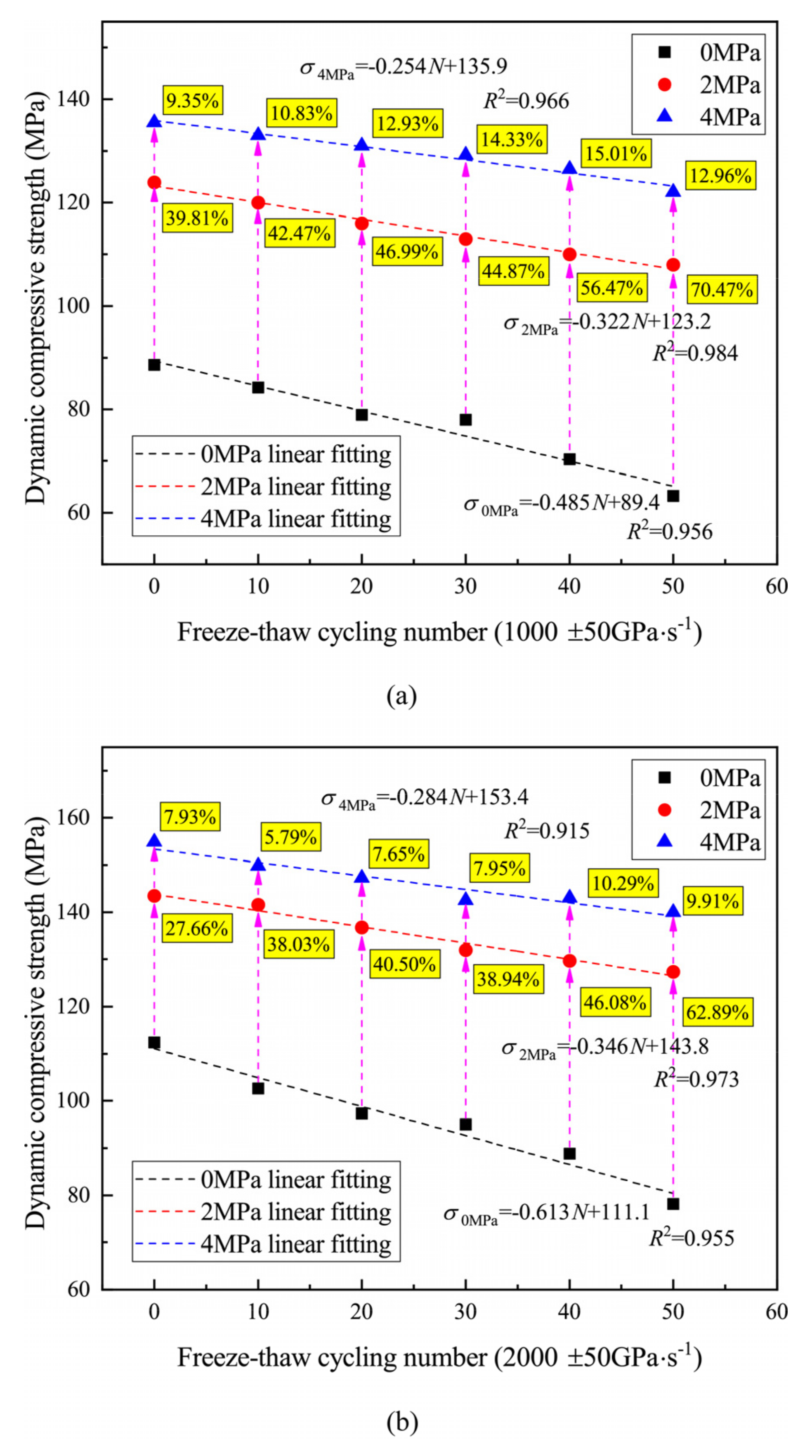



| Loading Rate (GP/s) | Hydrostatic Pressure (MPa) | |||
|---|---|---|---|---|
| 1000 ± 50 | 0 | −0.485 | 89.4 | 0.956 |
| 2 | −0.322 | 123.2 | 0.984 | |
| 4 | −0.254 | 135.9 | 0.966 | |
| 2000 ± 50 | 0 | −0.613 | 111.1 | 0.955 |
| 2 | −0.346 | 143.8 | 0.973 | |
| 4 | −0.284 | 153.4 | 0.915 |
| Confining Pressure (MPa) | FT Cycles | ||||
|---|---|---|---|---|---|
| 0 | 0 | 0.00050 | 2.0 | 1.006 | 0.994 |
| 10 | 0.00061 | 2.0 | 0.937 | 0.997 | |
| 20 | 0.00654 | 2.0 | 0.966 | 0.999 | |
| 30 | 0.00765 | 2.0 | 0.995 | 0.998 | |
| 40 | 0.00561 | 2.0 | 0.835 | 0.991 | |
| 50 | 0.00903 | 2.0 | 0.922 | 0.974 | |
| 2 | 0 | 0.00170 | 1.561 | 0.950 | 0.949 |
| 10 | 0.00169 | 1.553 | 0.920 | 0.956 | |
| 20 | 0.00162 | 1.560 | 0.896 | 0.983 | |
| 30 | 0.00153 | 1.552 | 0.881 | 0.989 | |
| 40 | 0.00141 | 1.561 | 0.865 | 0.992 | |
| 50 | 0.00124 | 1.556 | 0.832 | 0.994 | |
| 4 | 0 | 0.00250 | 1.029 | 1.025 | 0.911 |
| 10 | 0.00243 | 1.012 | 0.975 | 0.962 | |
| 20 | 0.00231 | 0.992 | 0.954 | 0.971 | |
| 30 | 0.00234 | 0.995 | 0.937 | 0.977 | |
| 40 | 0.00212 | 0.982 | 0.914 | 0.986 | |
| 50 | 0.00176 | 0.997 | 0.883 | 0.995 |
| Loading Rate (GP/s) | Hydrostatic Pressure (MPa) | |||
|---|---|---|---|---|
| 1000 ± 50 | 0 | −0.006 | 0.65 | 0.922 |
| 2 | −0.008 | 1.97 | 0.981 | |
| 4 | −0.013 | 2.11 | 0.996 | |
| 2000 ± 50 | 0 | −0.014 | 1.39 | 0.955 |
| 2 | −0.017 | 3.52 | 0.973 | |
| 4 | −0.019 | 3.70 | 0.915 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Wu, B.; Yang, L.; Mei, Y.; Sun, Y.; Liu, J.; Shen, J. Effects of Freeze–Thaw Cycling on Dynamic Compressive Strength and Energy Dissipation of Sandstone. Minerals 2022, 12, 1331. https://doi.org/10.3390/min12101331
Wu B, Yang L, Mei Y, Sun Y, Liu J, Shen J. Effects of Freeze–Thaw Cycling on Dynamic Compressive Strength and Energy Dissipation of Sandstone. Minerals. 2022; 12(10):1331. https://doi.org/10.3390/min12101331
Chicago/Turabian StyleWu, Bangbiao, Ling Yang, Yong Mei, Yunhou Sun, Jinming Liu, and Jun Shen. 2022. "Effects of Freeze–Thaw Cycling on Dynamic Compressive Strength and Energy Dissipation of Sandstone" Minerals 12, no. 10: 1331. https://doi.org/10.3390/min12101331
APA StyleWu, B., Yang, L., Mei, Y., Sun, Y., Liu, J., & Shen, J. (2022). Effects of Freeze–Thaw Cycling on Dynamic Compressive Strength and Energy Dissipation of Sandstone. Minerals, 12(10), 1331. https://doi.org/10.3390/min12101331







