Europium-Doped Carbonated Apatites
1. Introduction
1.1. Charge-Balance Mechanisms
 (
(  represents a vacancy).
represents a vacancy). , Ca4Na2, and so on. Therefore, a channel carbonate ion may be surrounded by six calcium ions that provide a total surrounding charge of +12, by five calcium ions and one sodium ion for a charge of +11, and so on. Because of the difference in the surrounding charge, carbonate ions in different environments have different vibrational frequencies. Fleet [10] utilized the difference in these channel environments to explain the IR spectrum of apatites synthesized at high temperature and pressure. This channel environment model has also been used to explain the IR spectra of calcium and strontium apatites prepared in aqueous solution [11,12].
, Ca4Na2, and so on. Therefore, a channel carbonate ion may be surrounded by six calcium ions that provide a total surrounding charge of +12, by five calcium ions and one sodium ion for a charge of +11, and so on. Because of the difference in the surrounding charge, carbonate ions in different environments have different vibrational frequencies. Fleet [10] utilized the difference in these channel environments to explain the IR spectrum of apatites synthesized at high temperature and pressure. This channel environment model has also been used to explain the IR spectra of calcium and strontium apatites prepared in aqueous solution [11,12].1.2. IR Spectra
 ) environments have been proposed [11].
) environments have been proposed [11].1.3. Substitution of Eu3+
2. Materials and Methods
2.1. Synthesis of Apatites
2.2. Characterization
3. Results
3.1. Composition
3.2. IR and NMR Carbonate Spectra
3.3. Lattice Parameters
4. Discussion
4.1. Charge-Balance Mechanism
4.2. Distribution of Carbonate
4.3. Lattice Parameters
5. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Pasteris, J.D. A mineralogical view of apatitic biomaterials. Am. Mineral. 2016, 101, 2594–2610. [Google Scholar] [CrossRef]
- McConnell, D. The crystal chemistry of carbonate apatites and their relationship to the composition of calcified tissues. J. Dental Res. 1952, 31, 53–63. [Google Scholar] [CrossRef]
- LeGeros, R.Z.; Trautz, O.R.; LeGeros, J.P.; Klein, E.J. Spectral properties of carbonate in carbonate-apatites. Appl. Spectrosc. 1968, 22, 357–358. [Google Scholar]
- LeGeros, R.Z.; Trautz, O.R.; Klein, E.; LeGeros, J.P. Two Types of Carbonate Substitution in the apatite structure. Experientia 1969, 25, 5–7. [Google Scholar] [CrossRef] [PubMed]
- Pan, Y.; Fleet, M.E. Compositions of the apatite-group minerals: Substitution mechanisms and controlling factors. In Reviews in Mineralogy and Geochemistry; Kohn, M., Rakovan, J., Hughes, J.M., Eds.; Mineralogical Society of America: Washington, DC, USA, 2002; Volume 48, pp. 13–49. [Google Scholar]
- Demarco, P.V.; Elzey, T.K.; Lewis, R.B.; Wenkert, E. Tri(dipivalomethanato)europium (III). Shift reagent for use in the proton magnetic resonance analysis of steroids and terpenoids. J. Am. Chem. Soc. 1970, 92, 5737–5739. [Google Scholar] [CrossRef]
- Morales, J.G.; Escamilla, C.V.; Penas, R.F.; Parra-Milla, C.M.; Drouet, C.; Maube-Bosc, F.; Oltolina, F.; Prat, M.; Ffernandez-Sanchez, J.F. Luminescent biomimetic citrate-coated europium-doped carbonated apatite nanoparticles for use in bioimaging: Physico-chemistry and cytocompatibility. RSC Adv. 2018, 9, 2385–2397. [Google Scholar] [CrossRef] [Green Version]
- Perera, T.S.H.; Han, Y.; Lu, X.; Wang, X.; Dai, H.; Li, S. Rare earth doped apatite nanomaterials for biological application. J. Nanomater. 2015, 2015, 705390. [Google Scholar] [CrossRef] [Green Version]
- De Maeyer, E.A.P.; Verbeeck, R.M.H.; Pieters, I.Y. Carbonate and alkalimetal incorporation in calciumhydroxyapatite. Trends Inorg. Chem. 1996, 4, 157–171. [Google Scholar]
- Fleet, M.E. Infrared spectra of carbonate apatites: Evidence for a connection between bone mineral and body fluids. Am. Mineral. 2017, 102, 149–157. [Google Scholar] [CrossRef]
- Yoder, C.H.; Bollmeyer, M.M.; Stepien, K.R.; Dudrick, R.N. The effect of incorporated carbonate and sodium on the IR spectra of A-, B-, and AB-type carbonate apatites. Am. Mineral. 2019, 104, 869–877. [Google Scholar] [CrossRef]
- Bollmeyer, M.M.; Carney, M.C.; Yoder, C.H. A-type carbonate in strontium apatites. Am. Mineral. 2019, 104, 438–446. [Google Scholar] [CrossRef]
- Fleet, M.E. Infrared spectra of carbonate apatites: ν2-Region bands. Biomaterials 2009, 30, 1473–1481. [Google Scholar] [CrossRef] [PubMed]
- Fleet, M.E. Carbonated Hydroxyapatite: Materials, Synthesis, and Application; CRC Press: Boca Raton, FL, USA, 2015. [Google Scholar]
- Pogosova, M.A.; Eliseev, A.A.; Kazin, P.E.; Azarmi, F. Synthesis, structure, luminescence, and color features of the Eu- and Cu-doped calcium apatite. Dye. Pigment. 2017, 141, 209–216. [Google Scholar] [CrossRef]
- Pazik, R.; Nedelec, J.-M.; Wiglusz, R.J. Preferential site substitution of Eu3+ ions in Ca10(PO4)6Cl2 nanoparticles obtained using a microwave stimulated wet chemistry technique. CrystEngComm 2014, 16, 5308–5318. [Google Scholar] [CrossRef]
- Ternane, R.; Trabelsi-Ayedi, M.; Jbir-Ariguib, N.; Piriou, B.L. Luminescent properties of Eu3+ in calcium hydroxyapatite. J. Lumin. 1999, 81, 165–170. [Google Scholar] [CrossRef]
- Piriou, B.; Fahmi, D.; Dexpert-Ghys, J.; Taitai, A.; Lacout, J.L. Unusual fluorescent properties of Eu3+ in oxyapatites. J. Lumin. 1987, 29, 97–103. [Google Scholar] [CrossRef]
- Hosseini, S.M.; Drouet, C.; Al-Kattan, A.; Navrotsky, A. Energetics of lanthanide-doped calcium phosphate apatite. Am. Mineral. 2014, 99, 2320–2327. [Google Scholar] [CrossRef] [Green Version]
- Yoder, C.H.; Havlusch, M.D.; Dudrick, R.N.; Shermerhorn, J.T.; Tran, L.K.; Deymier, A.C. The synthesis of phosphate and vanadate apatites using an aqueous one-step method. Polyhedron 2017, 127, 403–409. [Google Scholar] [CrossRef] [Green Version]
- Vignoles, M.; Bonel, G.; Holcomb, D.W.; Young, R.A. Influence of preparation conditions on the composition of type B carbonated hydroxyapatite and on the localization of the carbonate ions. Calcif. Tissue Int. 1988, 43, 33–40. [Google Scholar] [CrossRef]
- Holland, T.J.B.; Redfern, S.A.T. Unit cell refinement from powder diffraction data: The use of regression diagnostics. Mineral. Mag. 1997, 61, 65–77. [Google Scholar] [CrossRef]
- Bollmeyer, M.M.; Yoder, C.H. Incorporation of fluorophosphate in apatite. Polyhedron 2018, 145, 176–181. [Google Scholar] [CrossRef]
- Al-Kattan, A.; Dufour, P.; Dexpert-Ghys, I.; Drouet, C. Preparation and physicochemical characteristics of luminescent apatite-based colloids. J. Phys. Chem. C. 2010, 113, 2918–2924. [Google Scholar] [CrossRef]
- Yoder, C.H.; Stepien, K.R.; Dudrick, R.N. The distribution of carbonate in apatite: The environment model. Am. Mineral. 2022, 3, 1713–1728. [Google Scholar]
- Vignoles, M.; Bonel, G.; Young, R.A. Occurrence of nitrogenous species in precipitated B-type carbonated hydroxyapatites. Calcif. Tissue Int. 1987, 40, 64–70. [Google Scholar] [CrossRef] [PubMed]
- Fleet, M.E.; Liu, X.; Liu, X. Orientation of channel carbonate ions in apatite: Effect of pressure and composition. Am. Mineral. 2011, 96, 1148–1157. [Google Scholar] [CrossRef]
- Rey, C.; Collins, B.; Goehl, T.; Dickson, I.R.; Glimcher, M.J. The carbonate environment in bone mineral: A resolution-enhanced fourier transform infrared spectroscopy study. Calcif. Tissue Int. 1989, 45, 157–164. [Google Scholar] [CrossRef] [PubMed]
- Bashah, K.; Rey, C.; Gllmcher, M.J.; Schimizu, M.; Griffin, R.G. Solid state carbon-13 and proton NMR studies of carbonate-containing phosphate and enamel. J. Solid State Chem. 1990, 84, 71–81. [Google Scholar] [CrossRef]
- Babonneau, F.; Bonhomme, C.; Hayakawa, S.; Osaka, A. Solid state NMR characterization of nano-crystalline hydroxyl-carbonate apatite using 1H-31P-13C triple resonance experiments. Mater. Res. Soc. Symp. Proc. 2007, 984, 39–44. [Google Scholar]
- Silva, F.R.O.; de Lima, N.B.; Bressiani, A.H.A.; Courrol, L.C.; Gomes, L. Synthesis, characterization and luminescence properties of Eu3+-doped hydroxyapatite nanocrystals and thermal treatment effects. Opt. Mater. 2015, 47, 135–142. [Google Scholar] [CrossRef]
- Han, Y.; Wang, X.; Dai, H.; Li, S. Synthesis and luminescence of Eu3+ doped hydroxyapatite nanocrystallines: Effect of calcinations and Eu3+ content. J. Lumin. 2013, 135, 281–287. [Google Scholar] [CrossRef]
- LeGeros, R.Z. Effect of carbonate on the lattice parameters of apatite. Nature 1965, 206, 403–404. [Google Scholar] [CrossRef] [PubMed]
- Deymier, A.C.; Nair, A.K.; Dapalie, B.; Qin, Z.; Arcot, K.; Drouet, C.; Yoder, C.H.; Buehler, M.J.; Thomopoulos, S.; Genin, G.M.; et al. Protein-free Formation of bone-like apatite; New insights into the key role of carbonation. Biomaterials 2017, 127, 75–88. [Google Scholar] [CrossRef] [PubMed] [Green Version]
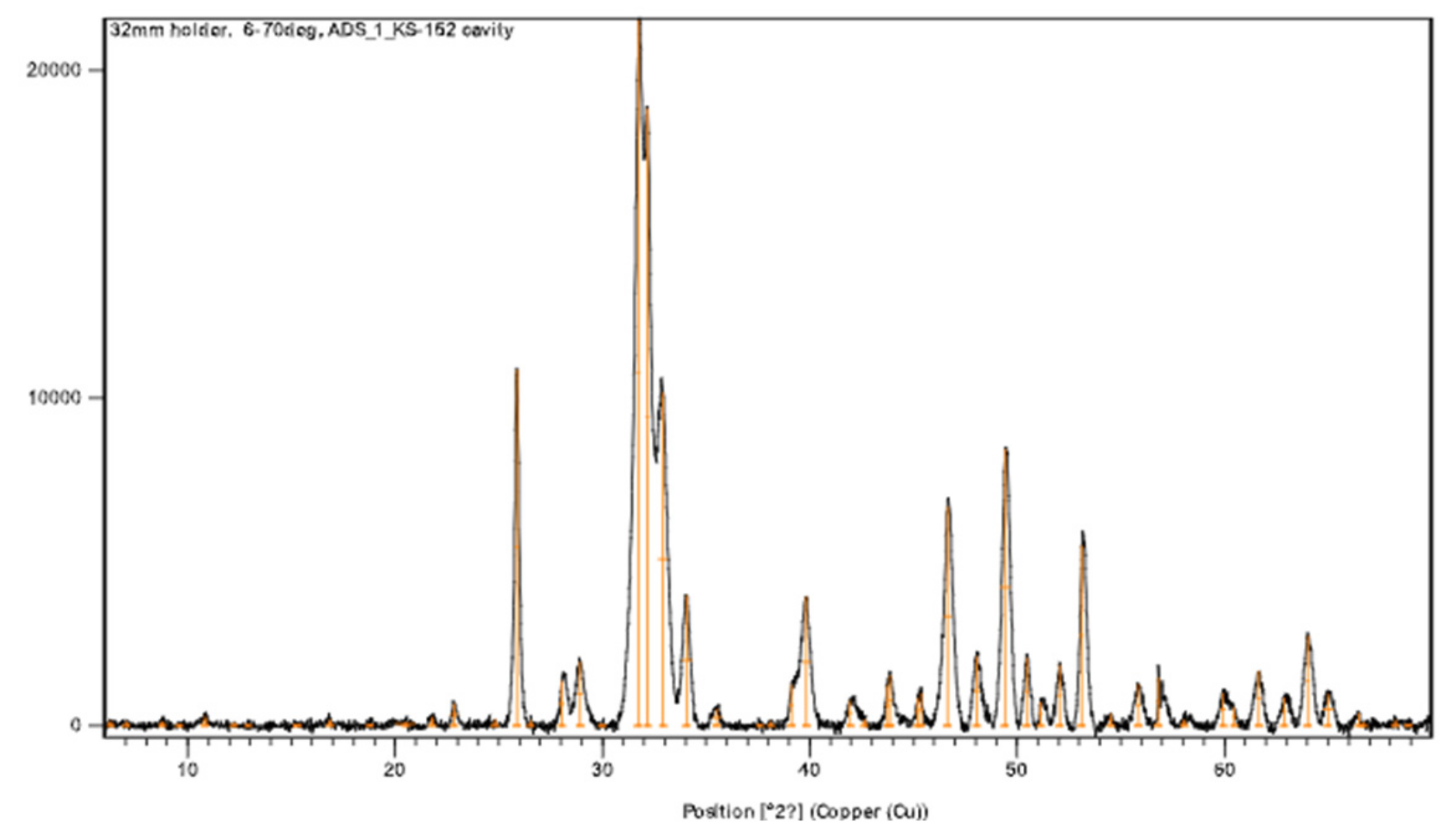



| ID | CO3:PO4 Ratio | Eu:Ca Ratio | PO4 Reagent * | CO3 Reagent * | Synthesis Method |
|---|---|---|---|---|---|
| KS-87 | 1 | 1:9 | T | 13NaH | One-step |
| KS-90 | 2 | 1:9 | T | 13NaH | One-step |
| KS-92 | 1 | 2:8 | T | 13NaH | One-step |
| KS-114 | 1 | 1:9 | Na2HPO4 | 13NaH | One-step |
| KS-116 | 1 | 1:9 | T | 13NaH | One-step + 0.5 g NaNO3 |
| KS-148 | 1 | 0.3:9.7 | T | 13NaH | Direct |
| KS-152 | 0.2 | 1:9 | T | 13NaH | Direct |
| ID | % CO3 | % Na | % Eu | Ca/Eu | Ca/P | (Ca + Eu)/P |
|---|---|---|---|---|---|---|
| KS-87 | 4.8 | 0.47 | 15.17 | 7.35 | 1.31 | 1.49 |
| KS-90 | 10.9 | 1.35 | 16.49 | 6.91 | 1.44 | 1.65 |
| KS-92 | 9.9 | 0.64 | 27.18 | 3.11 | 1.12 | 1.56 |
| KS-114 | 8.25 | 1.48 | 16.81 | 6.80 | 1.50 | 1.73 |
| KS-116 | 3.9 | 1.4 | 15.97 | 6.95 | 1.37 | 1.56 |
| KS-117 * | 2.5 | 0.66 | 0 | - | 1.59 | - |
| KS-148 | 4.6 | 0.82 | 4.8 | 28.7 | 1.55 | 1.61 |
| KS-152 | 2 | 0.36 | 16.03 | 7.03 | 1.36 | 1.55 |
| Starting Mole Ratios | Moles in ca. 1029 g | ||||
|---|---|---|---|---|---|
| ID | Ca: Eu, X: 9 | CO3:PO4 | Ca | PO4 | Eu |
| KS-148 | 0.3 | 1 | 9.3 | 6 | 0.33 |
| KS-87 | 1 | 1 | 7.5 | 5.7 | 1 |
| KS-114 | 1 | 1 | 7.5 | 5 | 1.1 |
| KS-116 | 1 | 1 | 7.3 | 5 | 1.1 |
| KS-152 | 1 | 0.2 | 7.6 | 5.6 | 1.1 |
| KS-90 | 1 | 2 | 7.6 | 5.3 | 1.1 |
| KS-92 | 2 | 1 | 5.7 | 5.1 | 1.8 |
| KS-117 * | - | 1 | 10 | 6.3 | - |
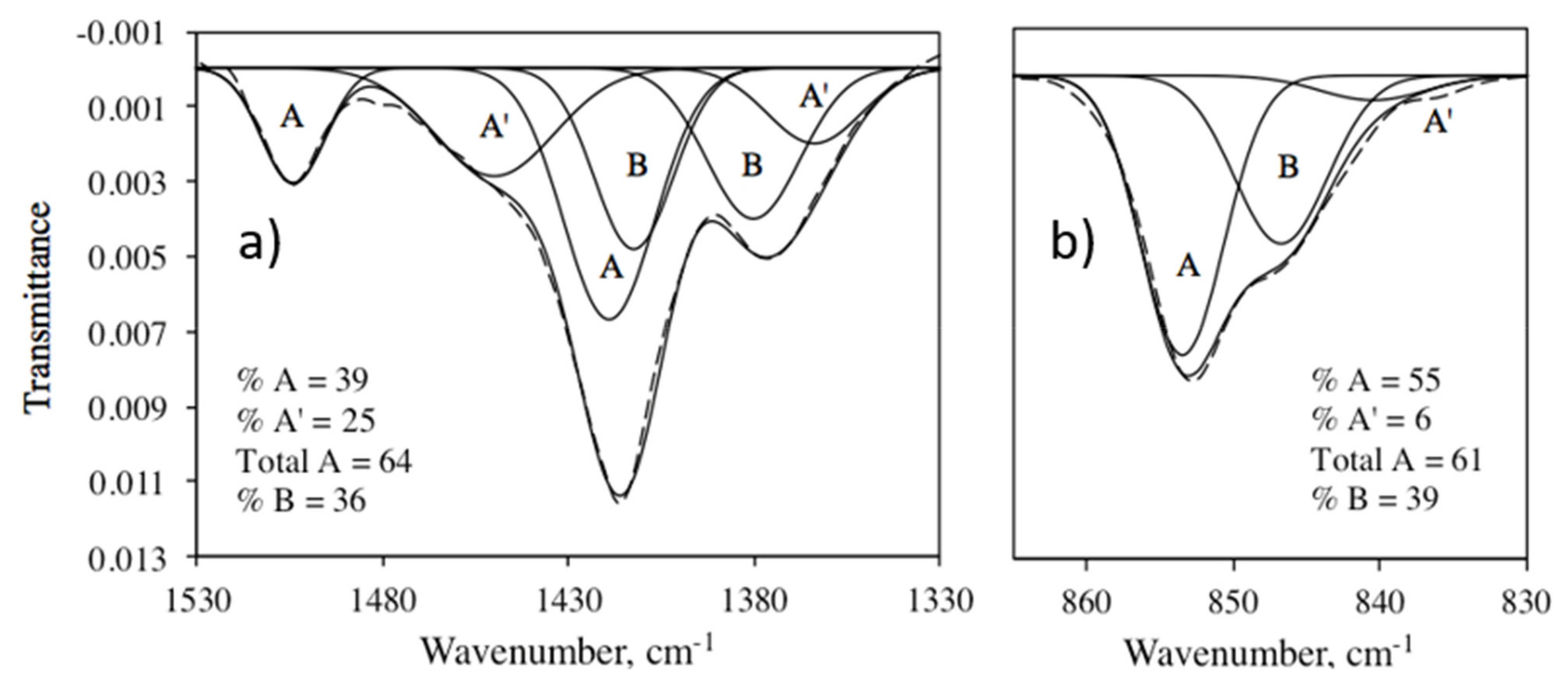
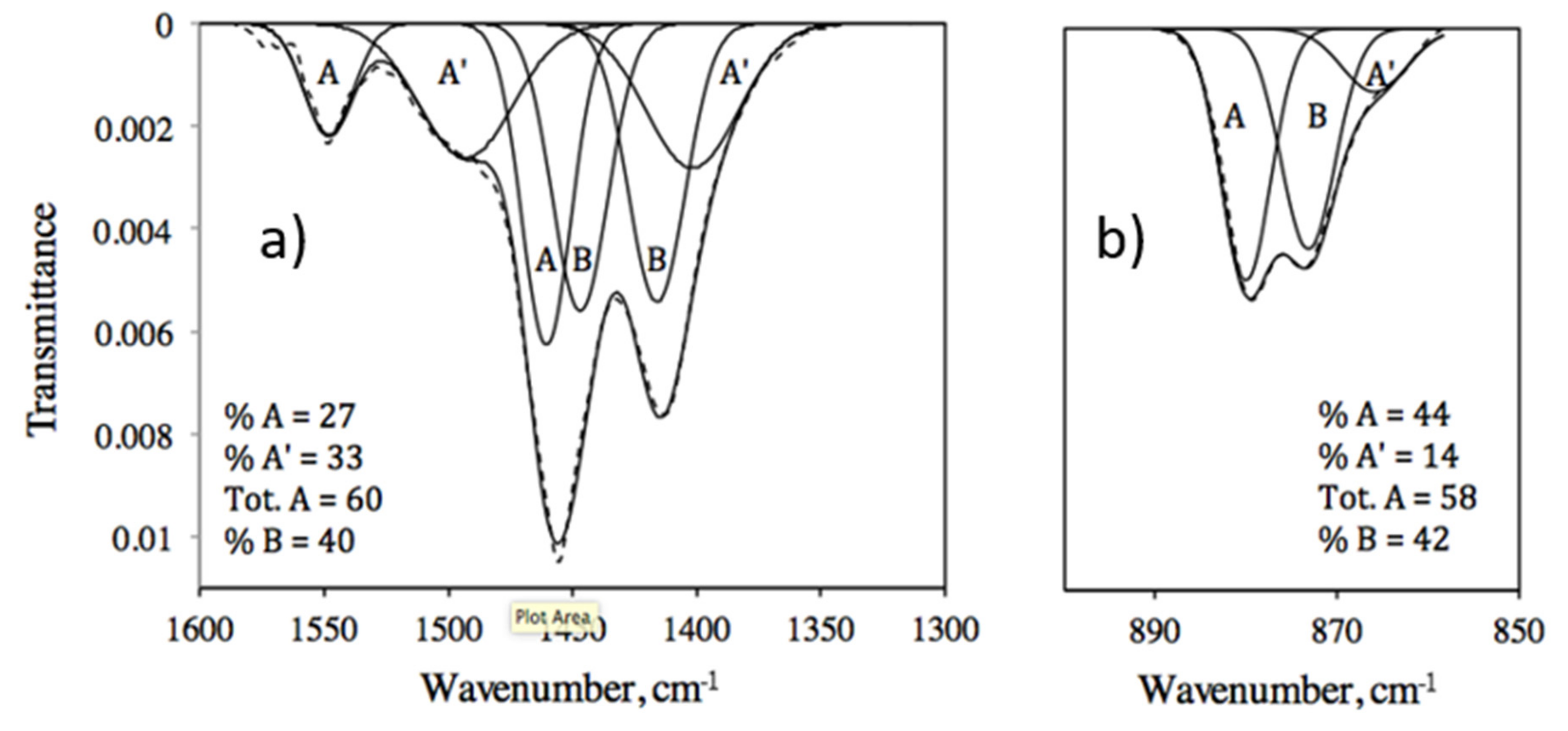
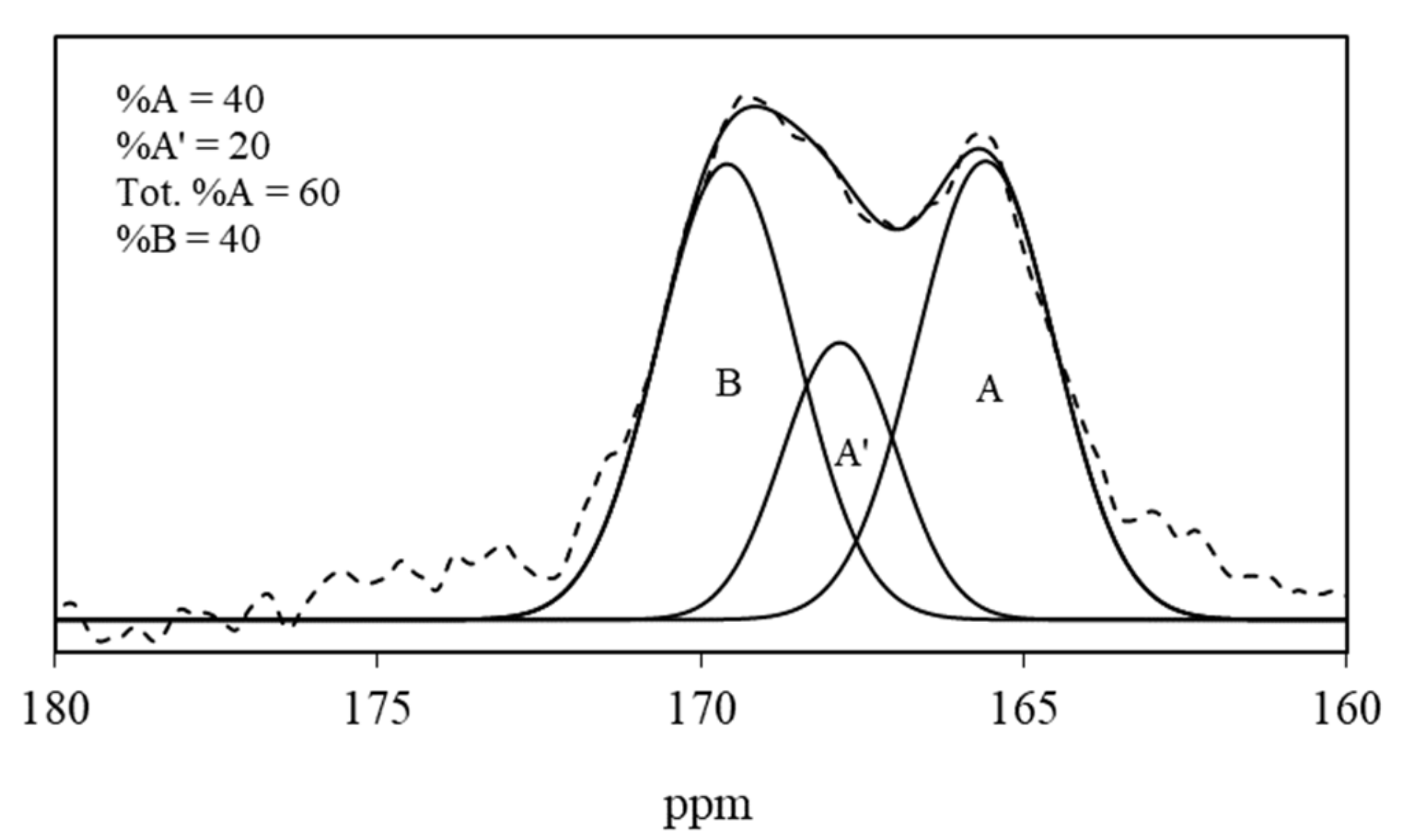
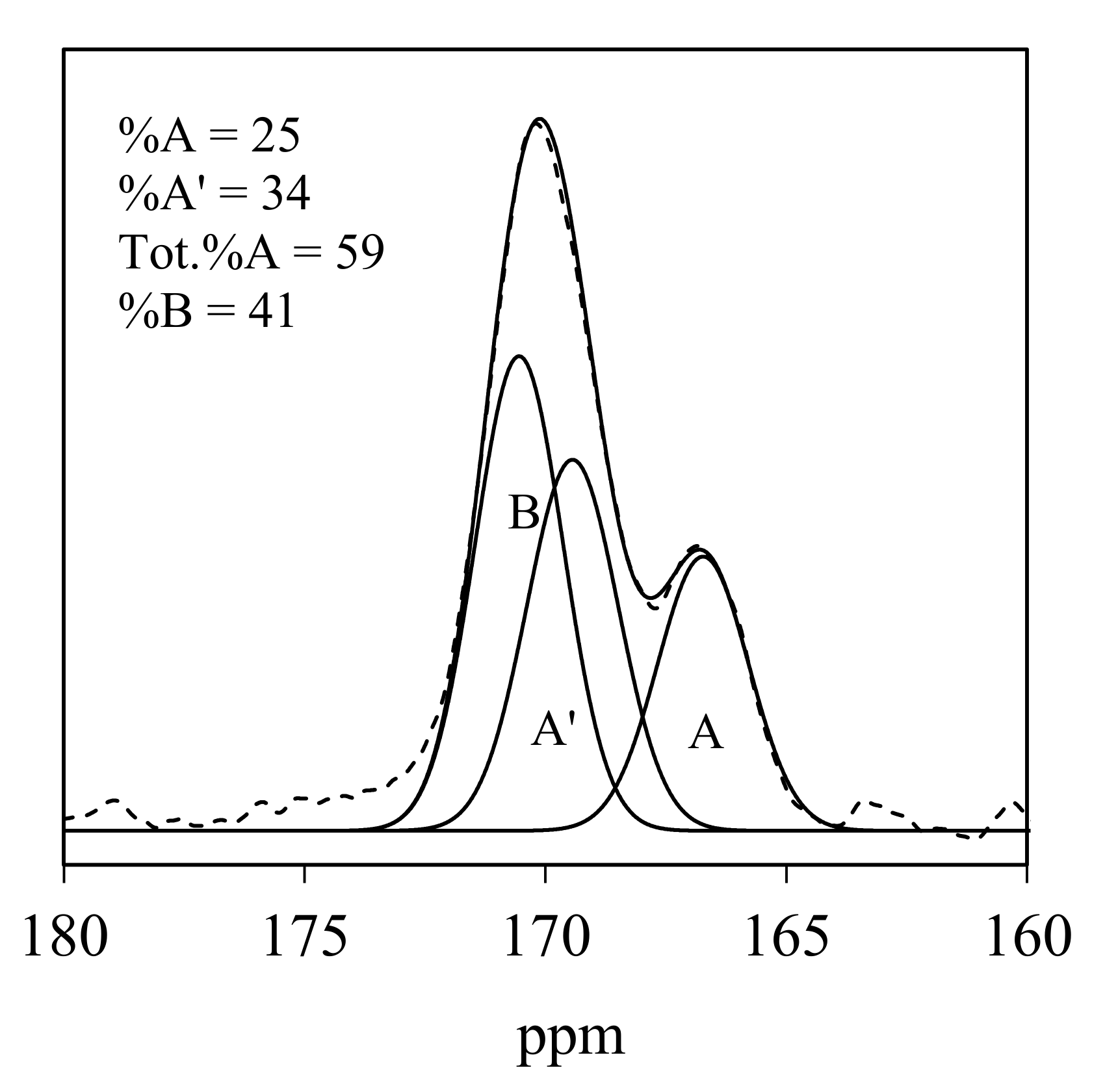
| ID | CO32− Environment | IR ν3 | IR ν2 | NMR | Average (IR ν3 + NMR) | |||
|---|---|---|---|---|---|---|---|---|
| Position (cm−1) | % | Position (cm−1) | % | Position (ppm) | % | |||
| 87 | A | 1419, 1504 | 39 | 853 | 55 | 165.6 | 40 | 39.5 |
| A’ | 1366, 1451 | 25 | 840 | 39 | 167.9 | 20 | 22.5 | |
| B | 1380, 1413 | 36 | 847 | 6 | 169.6 | 40 | 38 | |
| 90 | A | 1431, 1506 | 13 | 854 | 22 | 165.8 | 20 | 16.5 |
| A’ | 1363, 1454 | 46 | 840 | 33 | 168.4 | 39 | 42.5 | |
| B | 1377, 1409 | 41 | 847 | 45 | 170.2 | 41 | 41 | |
| 92 | A | 1422, 1504 | 36 | 853 | 44 | 166.5 | 25 | 30.5 |
| A’ | 1362, 1455 | 34 | 841 | 20 | 169.2 | 38 | 36 | |
| B | 1380, 1409 | 30 | 846 | 36 | 170.4 | 37 | 33.5 | |
| 114 | A | 1435, 1503 | 21 | 853 | 25 | 165.3 | 23 | 22 |
| A’ | 1358, 1460 | 37 | 839 | 30 | 167.7 | 38 | 37.5 | |
| B | 1379, 1410 | 42 | 846 | 45 | 169.3 | 39 | 40.5 | |
| 116 | A | 1431, 1500 | 23 | 853 | 32 | 166.5 | 20 | 21.5 |
| A’ | 1353, 1457 | 32 | 840 | 19 | 168.8 | 36 | 34 | |
| B | 1376, 1410 | 45 | 846 | 48 | 170.3 | 43 | 44 | |
| 117 * | A | 1422, 1505 | 29 | 853 | 46 | 166.7 | 25 | 27 |
| A’ | 1360, 1450 | 32 | 842 | 14 | 169.4 | 34 | 33 | |
| B | 1377, 1414 | 39 | 847 | 39 | 170.5 | 41 | 40 | |
| 148 | A | 1426, 1507 | 20 | 853 | 34 | 166.4 | 18 | 19 |
| A’ | 1365, 1454 | 45 | 841 | 24 | 168.9 | 44 | 44.5 | |
| B | 1378, 1411 | 35 | 847 | 42 | 170.1 | 38 | 36.5 | |
| 150 * | A | 1429, 1499 | 19 | 853 | 35 | 166.1 | 13 | 16 |
| A’ | 1363, 1454 | 39 | 841 | 28 | 168.3 | 41 | 40 | |
| B | 1379, 1409 | 42 | 847 | 38 | 169.6 | 46 | 44 | |
| 152 | A | 1421, 1505 | 30 | 853 | 41 | 165.1 | 14 | 22 |
| A’ | 1365, 1451 | 33 | 842 | 16 | 167.3 | 38 | 35.5 | |
| B | 1380, 1411 | 37 | 847 | 44 | 169.1 | 47 | 42 | |
| ID | % CO3 | % Na | a-axis (Å) | c-axis (Å) | Unit cell Volume (Å3) |
|---|---|---|---|---|---|
| 87 | 4.8 | 0.47 | 9.453(7) | 6.894(7) | 533.6(7) |
| 90 | 10.9 | 1.35 | 9.42(1) | 6.89(2) | 529.(1) |
| 92 | 9.9 | 0.64 | 9.430(6) | 6.870(6) | 529.0(6) |
| 114 | 8.25 | 1.48 | 9.406(1) | 6.879(4) | 527.1(4) |
| 116 | 3.9 | 1.4 | 9.405(5) | 6.894(4) | 528.1(4) |
| 117 * | 2.5 | 0.66 | 9.424(1) | 6.885(2) | 529.5(2) |
| 148 | 4.6 | 0.82 | 9.411(1) | 6.876(4) | 527.5(4) |
| 150 * | 6.05 | 0.58 | 9.408(8) | 6.888(8) | 527.0(8) |
| 152 | 2 | 0.36 | 9.426(6) | 6.889(6) | 530.0(6) |
| Charge-Balance Mechanism (Equation (3)) | Charge-Balance Mechanism (Equation (4)) | |||||
|---|---|---|---|---|---|---|
| ID | Ca | PO4 | OH | Ca | PO4 | OH |
| KS-148 | 9.38 | 5.71 | 0.67 | 9.21 | 5.71 | 1 |
| KS-87 | 8.69 | 5.69 | -0.02 | 8.19 | 5.69 | 0.89 |
| KS-114 | 8.33 | 5.43 | -0.7 | 7.78 | 5.43 | 0.4 |
| KS-152 | 8.76 | 5.86 | 0.5 | 8.21 | 5.86 | |
| KS-90 | 8.12 | 5.22 | -1.48 | 7.57 | 5.22 | 1.6 |
| KS-92 | 7.63 | 5.43 | -2.38 | 6.73 | 5.43 | -0.38 |
| KS-117 * | 9.83 | 5.83 | 1.48 | -0.58 | ||
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Stepien, K.R.; Yoder, C.H. Europium-Doped Carbonated Apatites. Minerals 2022, 12, 503. https://doi.org/10.3390/min12050503
Stepien KR, Yoder CH. Europium-Doped Carbonated Apatites. Minerals. 2022; 12(5):503. https://doi.org/10.3390/min12050503
Chicago/Turabian StyleStepien, Kathleen R., and Claude H. Yoder. 2022. "Europium-Doped Carbonated Apatites" Minerals 12, no. 5: 503. https://doi.org/10.3390/min12050503
APA StyleStepien, K. R., & Yoder, C. H. (2022). Europium-Doped Carbonated Apatites. Minerals, 12(5), 503. https://doi.org/10.3390/min12050503





