Porosity Distribution Law of Overlying Strata in the Goaf of the Adjacent Working Face: From the Perspective of Section Coal Pillar Types
Abstract
:1. Introduction
2. Engineering Geological Background and 3DEC Model Construction
2.1. Overview of Production Geological Conditions in the Longshan Coal Mine
2.2. Adjacent Working Face Model Construction
2.2.1. Model Geometry Size Setting
2.2.2. Model Mechanical Parameter Setting
2.2.3. Constitutive Model Selection and Calculation Conditions Setting
3. Subsidence Law of Overlying Strata in GAWF
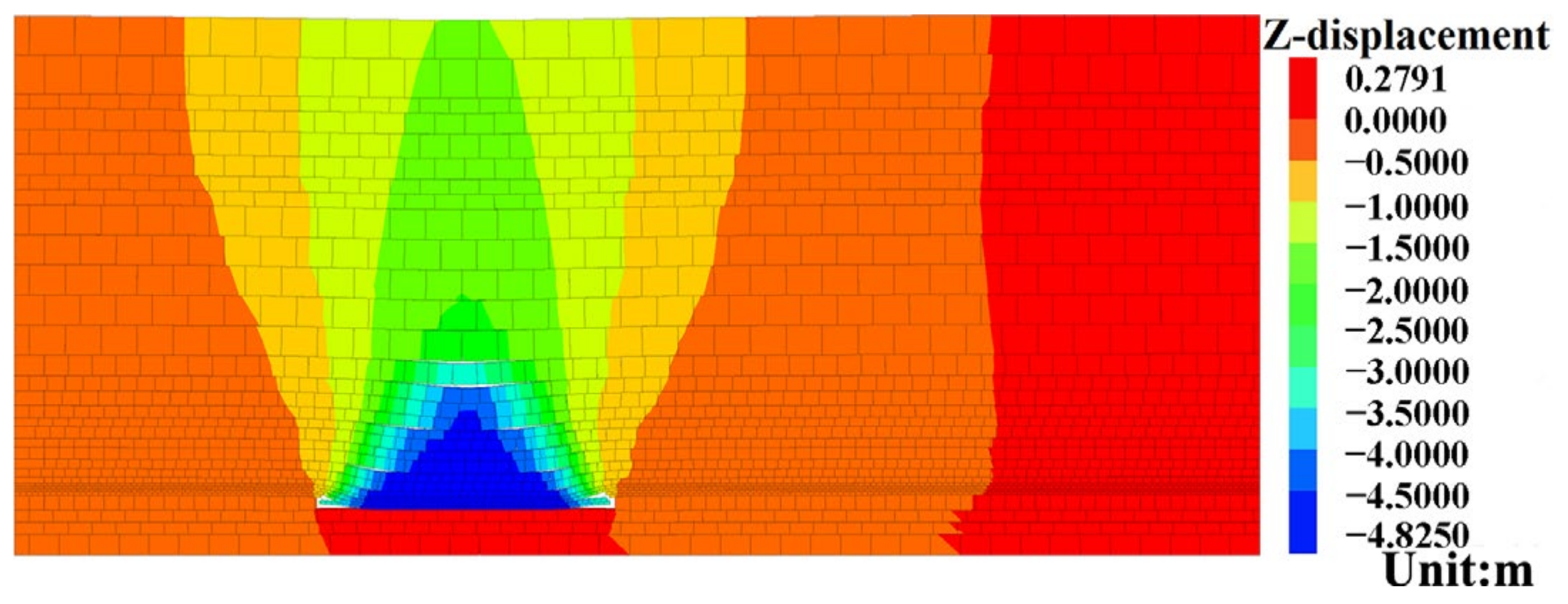
3.1. Numerical Calculation Results of Overlying Strata, Two-Dimensional (2D) Subsidence
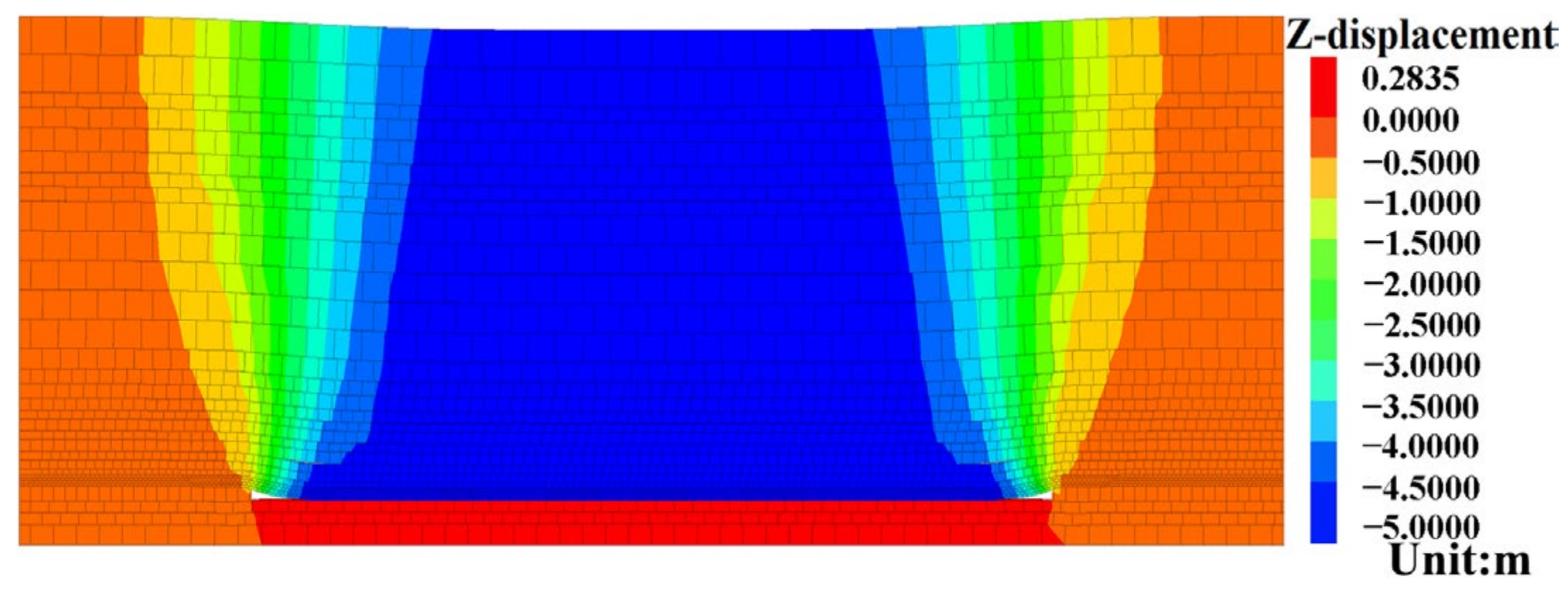
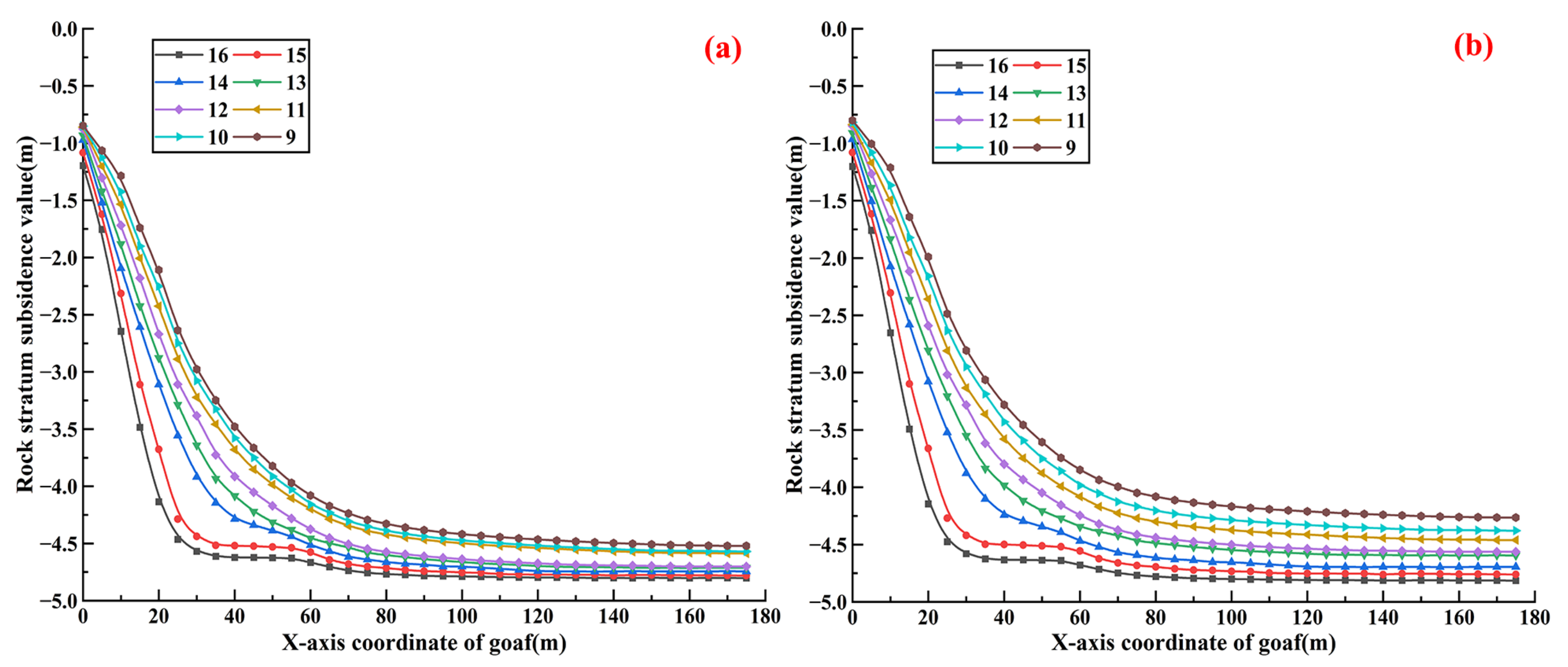
3.1.1. Calculation Results of Overlying Strata Subsidence Data of Single Working Face Strike and Dip Model
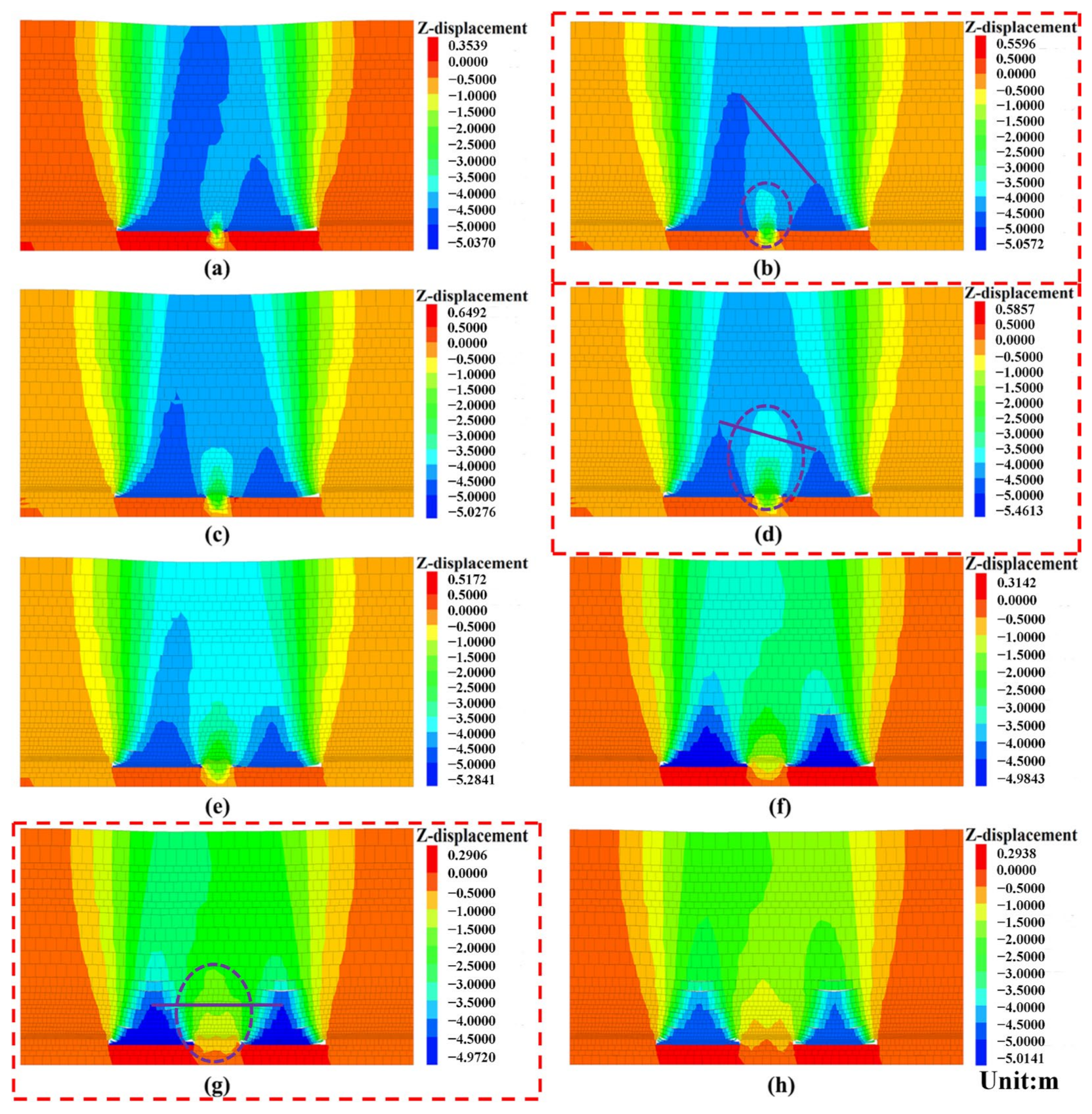
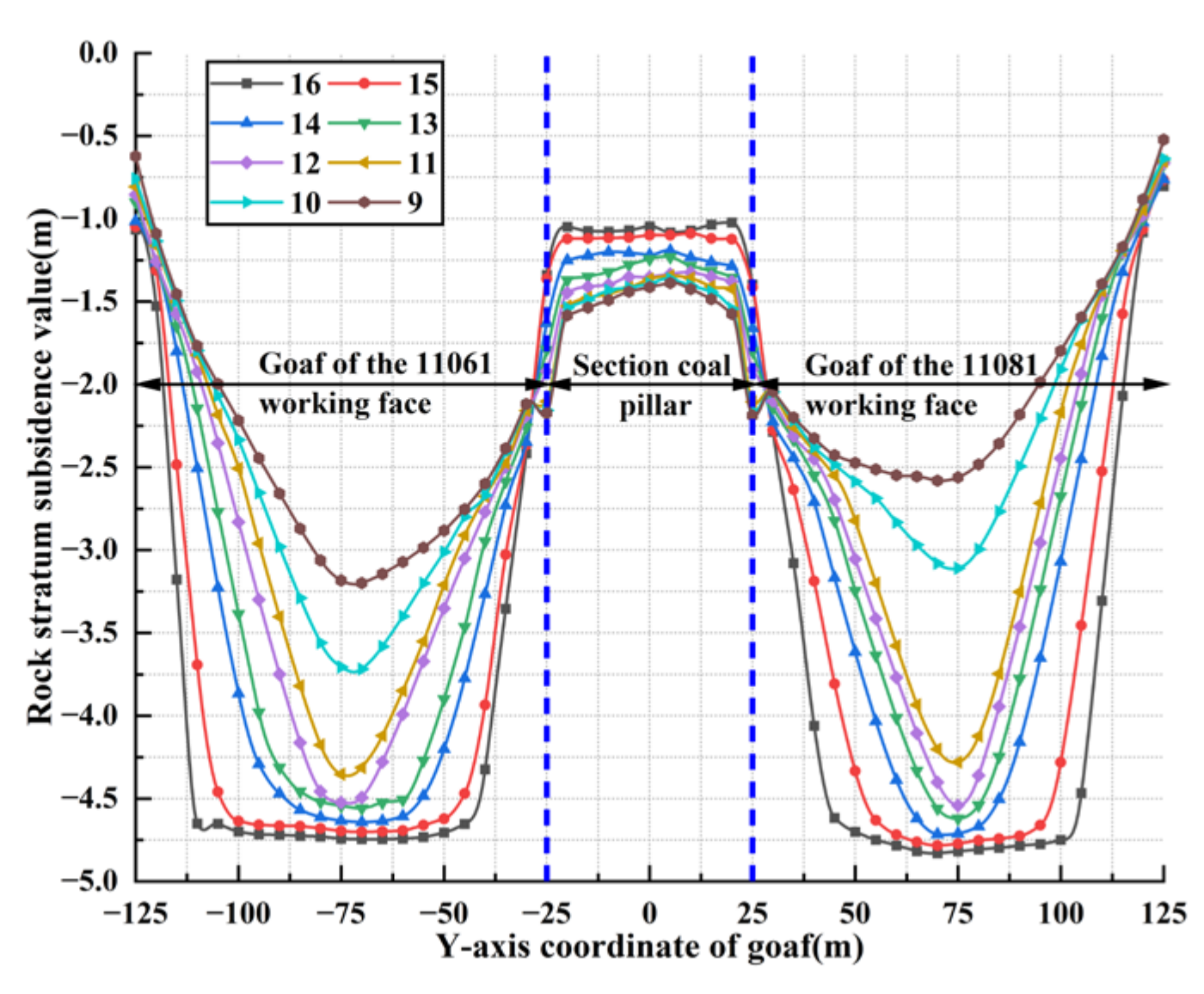
3.1.2. Calculation Results of Overlying Strata Subsidence Data of Adjacent Working Face Dip Model
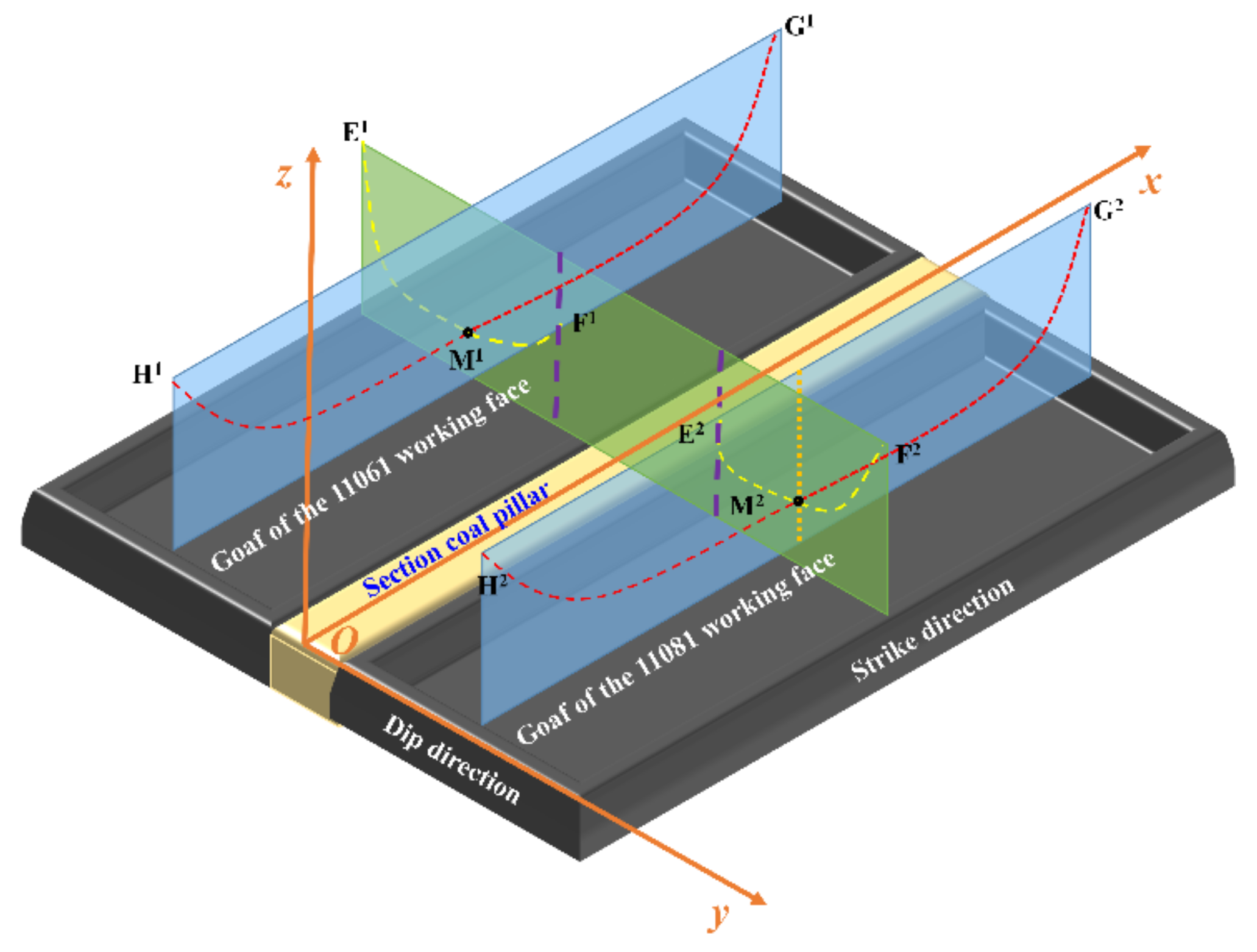
3.2. D Subsidence Value of the Overlying Strata Is Transformed into a 3D Subsidence Value
3.2.1. Principle of 2D Subsidence Value Conversion to 3D Subsidence Value
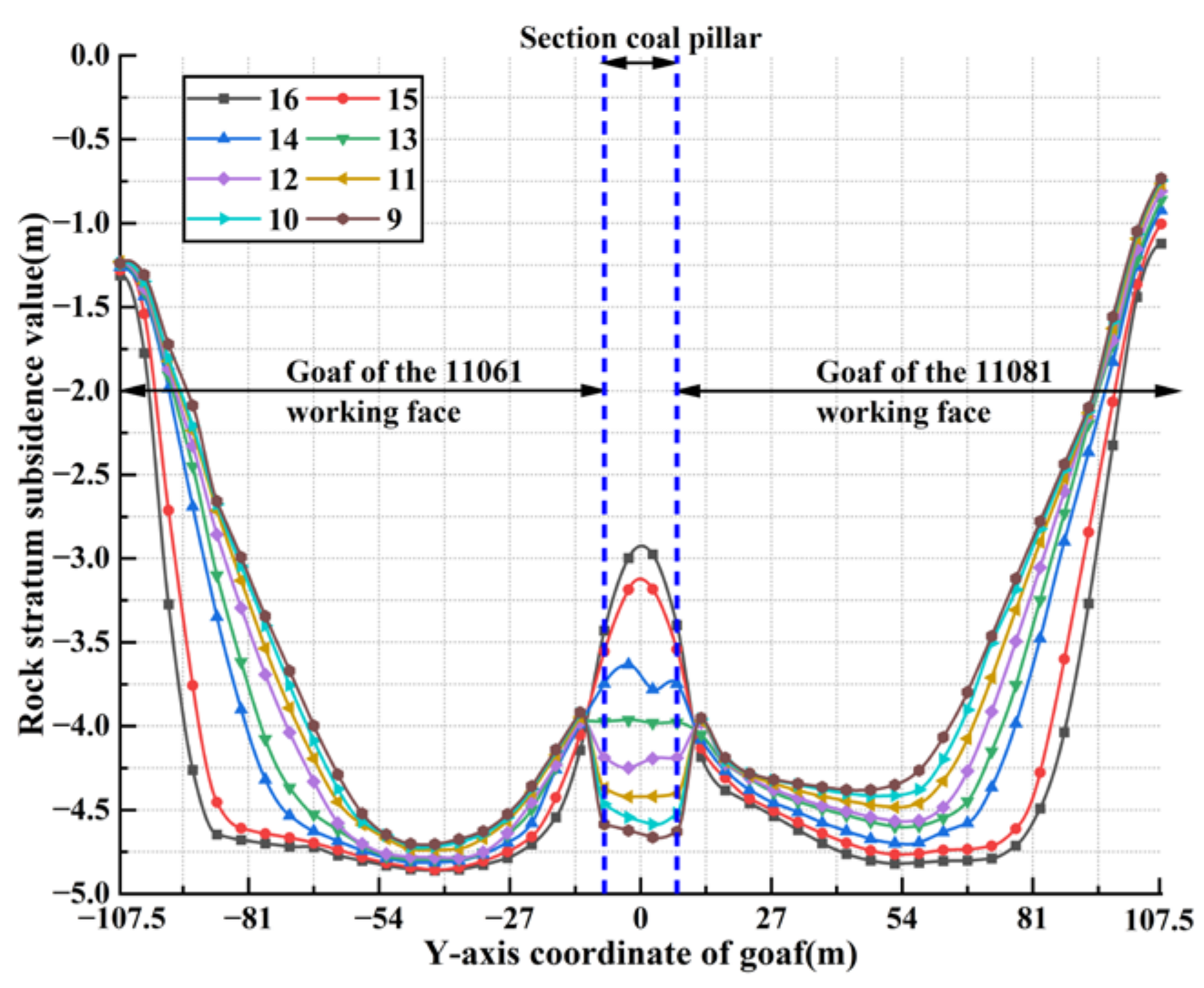
3.2.2. Conversion Results
4. Porosity Distribution Law of Overlying Strata in GAWF
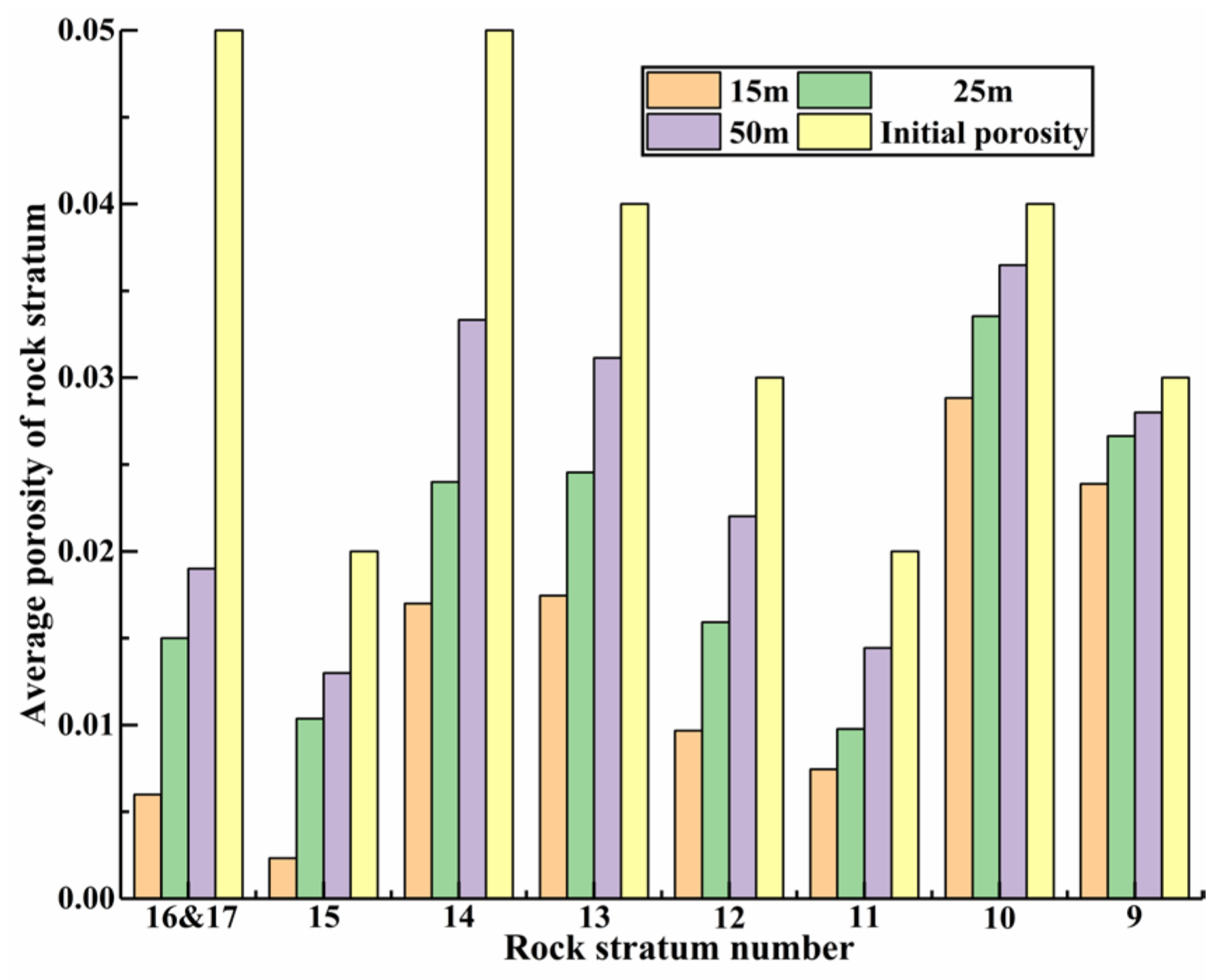
4.1. Porosity Calculation Method
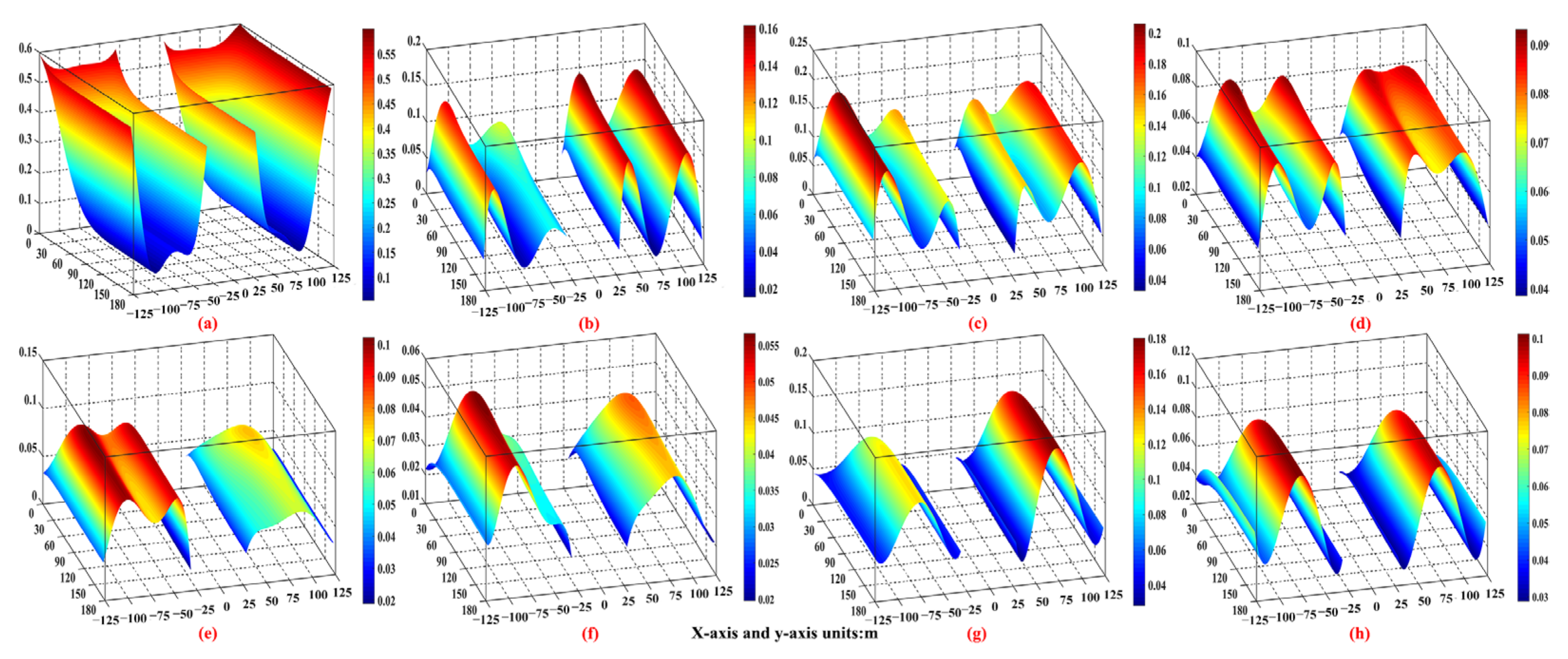
4.2. Porosity Distribution Law
4.2.1. Porosity Distribution Law of Overlying Strata in the GFST
4.2.2. Porosity Distribution Law of Overlying Strata in the GFMT
4.2.3. Porosity Distribution Law of Overlying Strata in the GFLT
4.2.4. Porosity Distribution Law of Overlying Strata in the ASCP
4.2.5. Discussion
5. Conclusions
- (1)
- The offset distance of the maximum subsidence value position of each overlying rock stratum in the goaf of the two adjacent working faces to the goaf direction of the other working face, and the heights of rock strata with large subsidence values, are negatively correlated with the width of the section coal pillar. Using the limit equilibrium theory and combined with the width-to-height ratio of the section coal pillar, the supporting effect of the section coal pillar in GAWF can be accurately predicted. Through the comparative analysis of overlying strata subsidence conditions in the GAWF under different section coal pillar widths, the GAWF can be divided into three types: GFST, GFMT, and GFLT.
- (2)
- In terms of the porosity value distribution law, the offset distance of the maximum porosity value area of each overlying rock stratum in the goaf of the working face to the middle of the rock strata is positively correlated with the height of each overlying stratum. In ASCP, porosity values from the bottom of the section coal pillar to each overlying rock stratum increase with an increase in the section coal pillar width, but are still less than its own initial porosity value, and the increase rate is continuously decreasing. When the section coal pillar width is smaller than (greater than or equal to) the width required to provide stable support, the porosity distribution condition of the overlying strata is significantly affected (not affected) by the mining sequence.
- (3)
- In terms of the porosity spatial form distribution law, from the coal seam floor upward, the spatial form distribution condition of overlying strata in the GFST and GFMT is expressed as follows: partial “dustpan” shape–unilateral “concave–convex peak” combined shape. The spatial form distribution condition of overlying strata in the GFLT is described as follows: “dustpan” shape–“concave–convex peak” combined shape-“Λ” shape.
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Qian, M.; Xu, J. Behaviors of strata movement in coal mining. J. China Coal Soc. 2019, 44, 973–984. [Google Scholar]
- Zhang, Y.; Niu, K.; Du, W.; Zhang, J.; Wang, H.; Zhang, J. A method to identify coal spontaneous combustion-prone regions based on goaf flow field under dynamic porosity. Fuel 2021, 288, 119690. [Google Scholar] [CrossRef]
- Qu, Q.; Xu, J.; Wu, R.; Qin, W.; Hu, G. Three-zone characterisation of coupled strata and gas behaviour in multi-seam mining. Int. J. Rock Mech. Min. 2015, 78, 91–98. [Google Scholar] [CrossRef]
- Ma, L.; Guo, R.; Wu, M.; Wang, W.; Ren, L.; Wei, G. Determination on the hazard zone of spontaneous coal combustion in the adjacent gob of different mining stages. Process Saf. Environ. Prot. 2020, 142, 370–379. [Google Scholar] [CrossRef]
- Brodny, J.; Tutak, M.; John, A. Analysis of Influence of Types of Rocks Forming the Goaf with Caving on the Physical Parameters of Air Stream Flowing Through These Gob and Adjacent Headings. Mechanics 2018, 24, 43–49. [Google Scholar] [CrossRef] [Green Version]
- Tutak, M.; Brodny, J. Determination of Particular Endogenous Fires Hazard Zones in Goaf with Caving of Longwall. IOP Conf. Ser. Earth Environ. Sci. 2017, 95, 042026. [Google Scholar] [CrossRef]
- Brodny, J.; Tutak, M. Applying computational fluid dynamics in research on ventilation safety during underground hard coal mining: A systematic literature review. Process Saf. Environ. Prot. 2021, 151, 373–400. [Google Scholar] [CrossRef]
- Wang, W.; Sui, W.; Faybishenko, B.; Stringfellow, W. Permeability variations within mining-induced fractured rock mass and its influence on groundwater inrush. Environ. Earth Sci. 2016, 75, 326. [Google Scholar] [CrossRef]
- Li, P.; Wang, X.; Cao, W.; Zhang, D.; Qin, D.; Wang, H. Influence of Spatial Relationships between Key Strata on the Height of Mining-Induced Fracture Zone: A Case Study of Thick Coal Seam Mining. Energies 2018, 11, 102. [Google Scholar] [CrossRef] [Green Version]
- Tian, S.; Yang, P.; Tang, K.; Shen, X.; Shi, F. Safety performance of coal mine survey technology using nano-fiber material in coal mining process. Arabian J. Geosci. 2020, 13, 841. [Google Scholar] [CrossRef]
- Kuyucak, N. Water in Mining and Environment for Sustainability. Mine Water Environ. 2021, 40, 815–817. [Google Scholar] [CrossRef]
- Wang, W.; Li, Z.; Yu, H. Goaf Gas Control Improvement by Optimizing the Adjacent Roadway Large-Diameter Boreholes. Adv. Civ. Eng. 2021, 2021, 1933010. [Google Scholar] [CrossRef]
- Liang, Y.; Wang, S. Prediction of coal mine goaf self-heating with fluid dynamics in porous media. Fire Saf. J. 2017, 87, 49–56. [Google Scholar] [CrossRef]
- Wang, G.; Xu, H.; Wu, M.; Wang, Y.; Wang, R.; Zhang, X. Porosity model and air leakage flow field simulation of goaf based on DEM-CFD. Arabian J. Geosci. 2018, 11, 148. [Google Scholar] [CrossRef]
- Jia, N.; Li, Z. Porosity Estimation of a Porous Goaf Area Based on Seismic Wave Attenuation. Pure Appl. Geophys. 2021, 178, 1845–1858. [Google Scholar] [CrossRef]
- Zhu, H.; Fang, S.; Huo, Y.; Liao, Q.; Hu, L.; Zhang, Y.; Li, F. Study on the optimal position of the roof low roadway based on the response surface methodology. Sci. Rep. 2021, 11, 14508. [Google Scholar] [CrossRef]
- Wang, S.; Wang, D.; Cao, K.; Wang, S.; Pi, Z. Distribution law of 3D fracture field of goaf and overlying strata. J. Cent. South Univ. (Sci. Technol.) 2014, 45, 833–839. [Google Scholar]
- Wang, S.; Li, X.; Wang, D. Void fraction distribution in overburden disturbed by longwall mining of coal. Environ. Earth Sci. 2016, 75, 151. [Google Scholar] [CrossRef]
- Li, Z.; Yi, G.; Wu, J.; Guo, D.; Zhao, C.; Zhao, D. Study on spontaneous combustion distribution of goaf based on the “O” type risked falling and non-uniform oxygen. J. China Coal Soc. 2012, 37, 484–489. [Google Scholar]
- Li, L.; Li, G.; Gong, W.; Deng, H. Energy Evolution Pattern and Roof Control Strategy in Non-Pillar Mining Method of Goaf-Side Entry Retaining by Roof Cutting—A Case Study. Sustainability 2019, 11, 7029. [Google Scholar] [CrossRef] [Green Version]
- Yang, H.; Han, C.; Zhang, N.; Sun, C.; Pan, D.; Dong, M. Stability Control of a Goaf-Side Roadway under the Mining Disturbance of an Adjacent Coal Working Face in an Underground Mine. Sustainability 2019, 11, 6398. [Google Scholar] [CrossRef] [Green Version]
- Gao, Y.; Liu, D.; Zhang, X.; He, M. Analysis and Optimization of Entry Stability in Underground Longwall Mining. Sustainability 2017, 9, 2079. [Google Scholar] [CrossRef] [Green Version]
- Najafi, M.; Shishebori, A.; Gholamnejad, J. Numerical Estimation of Suitable Distance between Two Adjacent Panels’ Working Faces in Shortwall Mining. Int. J. Geomech. 2017, 17, 04016090. [Google Scholar] [CrossRef]
- Shen, W.; Bai, J.; Li, W.; Wang, X. Prediction of relative displacement for entry roof with weak plane under the effect of mining abutment stress. Tunn. Undergr. Sp. Tech. 2018, 71, 309–317. [Google Scholar] [CrossRef]
- Yu, B.; Zhang, Z.; Kuang, T.; Liu, J. Stress Changes and Deformation Monitoring of Longwall Coal Pillars Located in Weak Ground. Rock Mech. 2016, 49, 3293–3305. [Google Scholar] [CrossRef]
- Xia, Z.; Yao, Q.; Meng, G.; Xu, Q.; Tang, C.; Zhu, L.; Wang, W.; Shen, Q. Numerical study of stability of mining roadways with 6.0-m section coal pillars under influence of repeated mining. Int. J. Rock Mech. Min. 2021, 138, 104641. [Google Scholar] [CrossRef]
- Chen, Y.; Li, D.; Jiang, F.; Zhang, L.; Wang, C.; Zhu, S. Use of the Equivalent Mining Height Method for Understanding Overlying Strata Movement and Stress Distribution in an Isolated Coal Pillar. Shock Vib. 2020, 2020, 8820886. [Google Scholar] [CrossRef]
- Yu, B.; Tai, Y.; Gao, R.; Yao, Q.; Li, Z.; Xia, H. The sustainable development of coal mines by new cutting roof technology. R. Soc. Open Sci. 2020, 7, 191913. [Google Scholar] [CrossRef]
- Chinese Specifications. National Coal Mine Safety Administration. Regulations of Buildings, Water, Railway and Main Well Lane Leaving Coal Pillar and Press Coal Mining; Coal Industry Press: Beijing, China, 2017; pp. 55–56. Available online: https://www.chinamine-safety.gov.cn/zfxxgk/fdzdgknr/tzgg/201707/t20170703_349044.shtml (accessed on 23 May 2022).
- Wilson, A.; Ashwin, D. Research into the determination of pillar size. Min. Eng. 1972, 31, 409–430. [Google Scholar]
- Lu, S. Roadways in Pillarless Districts: Occurrence of Mine Pressure and Adaptability. J. China Univ. Min. Technol. 1980, 4, 1–22. [Google Scholar]
- Liu, Y. Comprehensive Evaluation of Stability of Residual Coal-pillar after Room-and-pillar Mining. Coal Min. Technol. 2013, 18, 78–80. [Google Scholar]
- Pan, J.; Qi, Q.; Mao, D.; Ren, Y. Study on Movement and Stress Evolutionary Process of Impacted Roof with 3DEC. Chin. J. Rock Mech. Eng. 2007, 26, 3546–3552. [Google Scholar]
- Mohammad, N.; Reddish, D.; Stace, L. The relation between in situ and laboratory rock properties used in numerical modelling. Int. J. Rock Mech. Min. 1997, 34, 289–297. [Google Scholar] [CrossRef]
- Feng, G.; Zhang, A.; Hu, S.; Cheng, J.; Miu, X.; Hao, G.; Han, D.; Guan, S.; Zhao, G. A Methodology for Determining the Methane Flow Space in Abandoned Mine Gobs and Its Application in Methane Drainage. Fuel 2018, 227, 208–217. [Google Scholar] [CrossRef]
- Feng, G.; Li, Z.; Hu, S.; Zhang, Y.; Zhang, A.; Gao, Q.; Jiang, H.; Guo, X.; Li, C.; Cui, J. Distribution of Gob Empty Space for Methane Drainage during the Longwall Mining: A Case Study. J. Nat. Gas Sci. Eng. 2018, 60, 112–124. [Google Scholar] [CrossRef]
- Lai, X.; Li, J.; Cui, F.; Shan, P. Study on Overlying Strata Movement in Mining Adjacent Working Face of Extra Thick Seam. Saf. Coal Mines 2020, 51, 70–75. [Google Scholar]
- Wang, S.; Li, X.; Wang, S. Separation and fracturing in overlying strata disturbed by longwall mining in a mineral deposit seam. Eng. Geol. 2017, 226, 257–266. [Google Scholar] [CrossRef]
- Li, S.; Qian, M.; Shi, P. Study on Bed-Separated Fissures of Overlying Stratum and Interstice Permeability in Fully-Mechanized Top Coal Caving. Chin. J. Rock Mech. Eng. 2000, 19, 604–607. [Google Scholar]
- Liu, Q.; Lin, B.; Zhou, Y.; Li, Y. Porosity model of the goaf based on overlying strata movement and deformation. Environ. Earth. Sci. 2022, 81, 214. [Google Scholar] [CrossRef]
- Zheng, G.; Han, J.; Sang, F.; Gao, T.; Chen, D.; Zhang, Z. Case Study of Gas Drainage Well Location Optimization in Abandoned Coal Mine Based on Reservoir Simulation Model. Energy Explor. Exploit. 2021, 39, 1993–2005. [Google Scholar] [CrossRef]
- Qin, W.; Xu, J.; Hu, G. Optimization of Abandoned Gob Methane Drainage through Well Placement Selection. J. Nat. Gas Sci. Eng. 2015, 25, 148–158. [Google Scholar] [CrossRef]

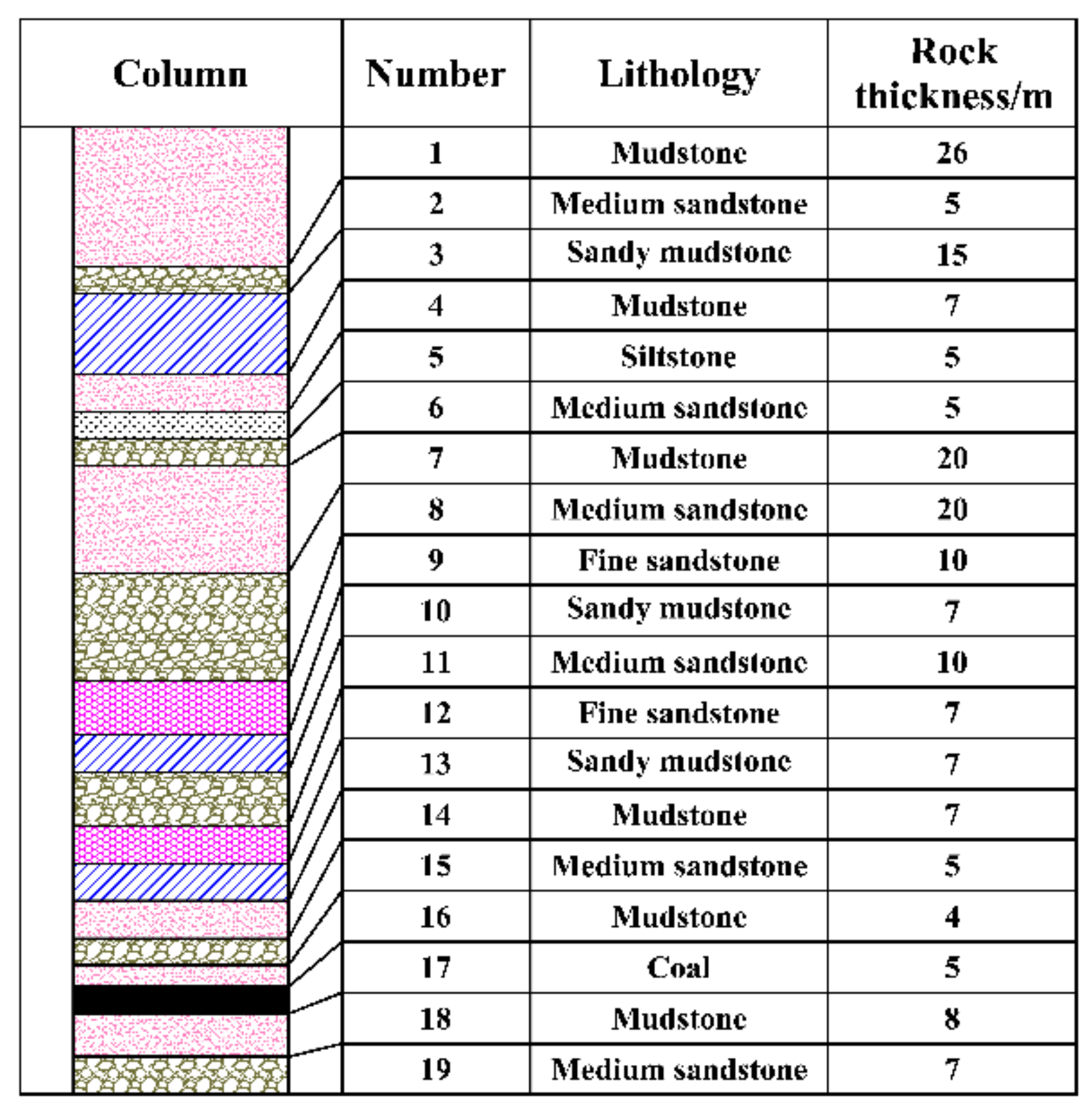
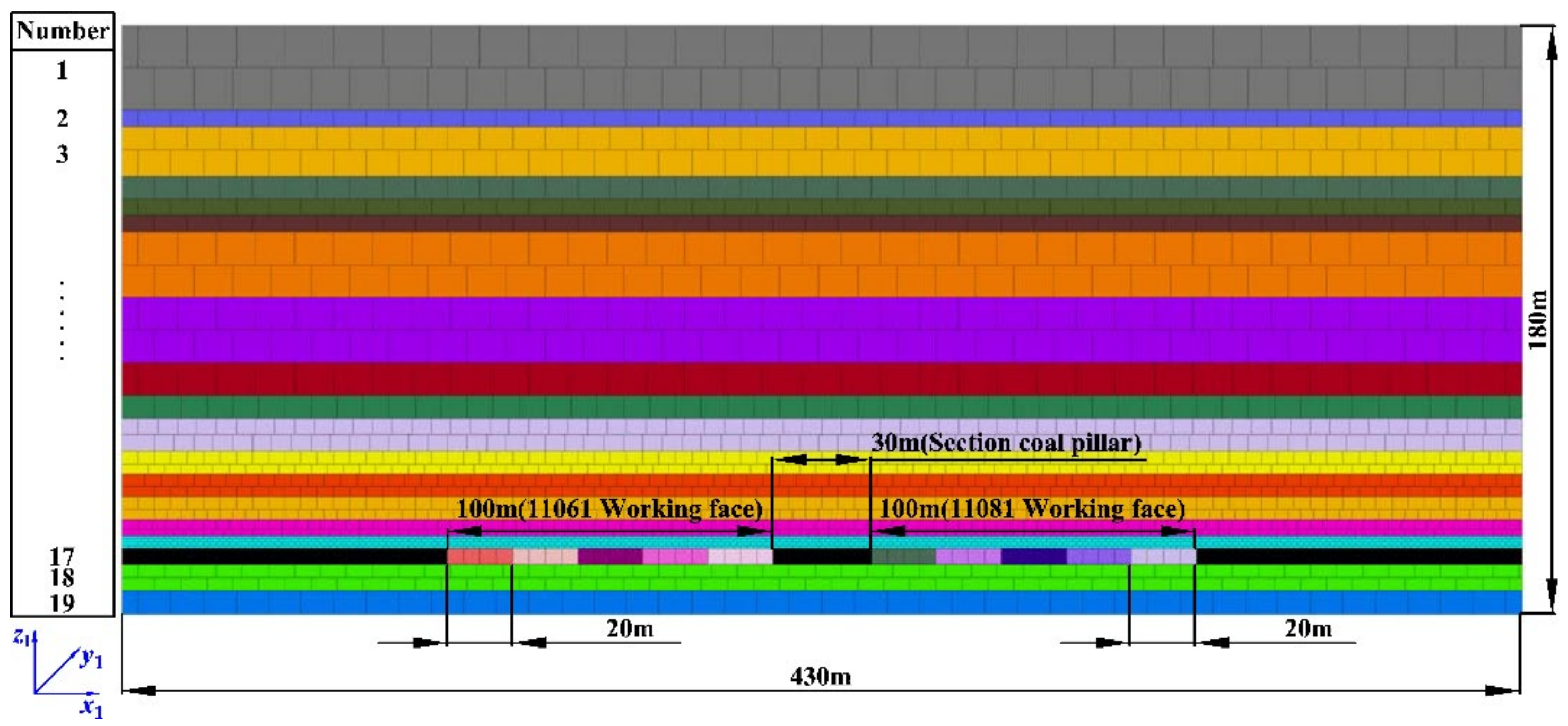
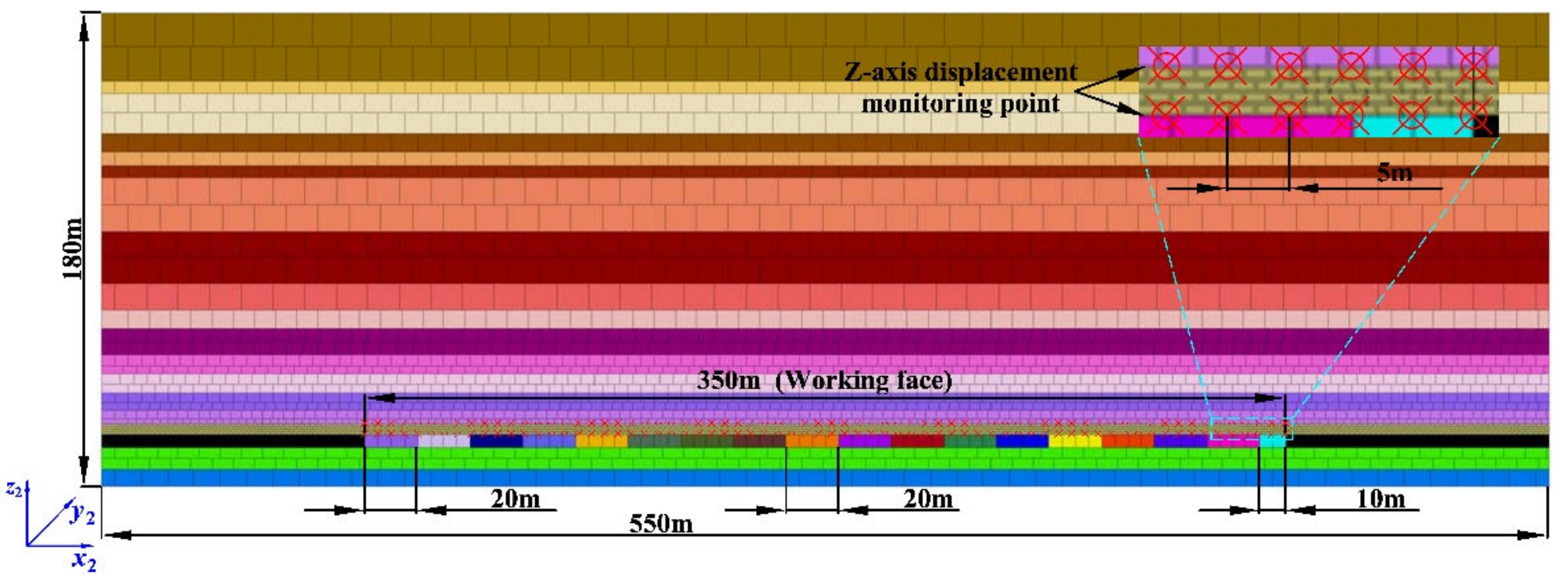




| Parameter Name | f | λ | K | γ | H | c | R | |
|---|---|---|---|---|---|---|---|---|
| Value | 0.12 | 25 | 2.46 | 2.5 | 24,500 | 652 | 1.9 | 10 |
| Lithology | Density /(kg·m−3) | Bulk Modulus /(GPa) | Shear Modulus /(GPa) | Tensile Strength /(MPa) | Cohesion /(MPa) | Internal Friction Angle /(°) |
|---|---|---|---|---|---|---|
| Mudstone | 2690 | 2.41 | 1.05 | 0.42 | 8.50 | 23.0 |
| Medium sandstone | 2680 | 1.89 | 1.03 | 2.50 | 1.90 | 32.0 |
| Sandy mudstone | 2590 | 1.33 | 1.00 | 2.00 | 10.55 | 25.0 |
| Siltstone | 2700 | 0.90 | 0.73 | 1.80 | 1.70 | 21.0 |
| Fine sandstone | 2750 | 4.81 | 3.31 | 3.70 | 4.30 | 27.0 |
| Coal | 1460 | 1.23 | 0.67 | 1.00 | 1.90 | 32.0 |
| Joint Type | Normal Stiffness /(GPa) | Shear Stiffness /(GPa) | Tensile Strength /(MPa) | Cohesion /(MPa) | Internal Friction Angle /(°) |
|---|---|---|---|---|---|
| Mudstone | 0.73 | 0.28 | 0.30 | 4.00 | 22.0 |
| Medium sandstone | 1.63 | 0.64 | 0.70 | 1.00 | 25.0 |
| Sandy mudstone | 0.64 | 0.27 | 1.20 | 5.50 | 21.0 |
| Siltstone | 0.78 | 0.33 | 0.60 | 0.90 | 15.0 |
| Fine sandstone | 3.67 | 1.51 | 0.60 | 2.10 | 23.0 |
| Coal | 0.37 | 0.15 | 0.15 | 1.00 | 25.0 |
| Lithology | Mudstone | Medium Sandstone | Sandy Mudstone | Fine Sandstone | Coal |
|---|---|---|---|---|---|
| Porosity | 0.05 | 0.02 | 0.04 | 0.03 | 0.05 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Tian, S.; Mao, J.; Li, H. Porosity Distribution Law of Overlying Strata in the Goaf of the Adjacent Working Face: From the Perspective of Section Coal Pillar Types. Minerals 2022, 12, 782. https://doi.org/10.3390/min12060782
Tian S, Mao J, Li H. Porosity Distribution Law of Overlying Strata in the Goaf of the Adjacent Working Face: From the Perspective of Section Coal Pillar Types. Minerals. 2022; 12(6):782. https://doi.org/10.3390/min12060782
Chicago/Turabian StyleTian, Shuicheng, Junrui Mao, and Hongxia Li. 2022. "Porosity Distribution Law of Overlying Strata in the Goaf of the Adjacent Working Face: From the Perspective of Section Coal Pillar Types" Minerals 12, no. 6: 782. https://doi.org/10.3390/min12060782
APA StyleTian, S., Mao, J., & Li, H. (2022). Porosity Distribution Law of Overlying Strata in the Goaf of the Adjacent Working Face: From the Perspective of Section Coal Pillar Types. Minerals, 12(6), 782. https://doi.org/10.3390/min12060782






