Effect of Al₂O₃ on Sound Velocity of MgSiO₃ Glass at High Pressure

Abstract

Silicate glass has been used as an analog for silicate melts to understand the nature of dense magmas in the Earth’s mantle. To understand the effect of Al₂O₃ on the sound velocity and structure of MgSiO₃ glass, in this study, combined with Brillouin scattering and diamond anvil cells (DACs), the acoustic velocity of MgSiO₃∙5 mol%Al₂O₃ (MA1) and MgSiO₃∙24.5 mol%Al₂O₃ (MA2) glass were measured up to 20 and 42 GPa, respectively. Our studies show that the incorporation of Al₂O₃ could increase the sound velocity of MgSiO₃ glass. Using the obtained velocities, the bulk and shear moduli (K_S, G), density ($\rho$) and Poisson’s ratio ($\nu$) are calculated at high pressures, and the results indicate that Al₂O₃ could induce the stiffness of MgSiO₃ glass. However, the effect of Al₂O₃ content on the stiffness of MgSiO₃ glass is non-linear, and MA1 and MA2 exhibit similar K_S and G at high pressures. With the increase of pressure, the transverse acoustic mode (V_S) of MA1 and MA2 shows abnormal changes at 17.8 GPa and 31.8 GPa, which are related to the transition of coordination number (CN) for Si-O in Al-bearing MgSiO₃ glass. Compared with previous studies on sound velocity of MgSiO₃ glass, the incorporation of Al₂O₃ delays the transition pressure of Si-O coordination to a higher pressure. Our study has profound implications for understanding the density and sound velocity of Al-bearing MgSiO₃ melt in the Earth’s interior.

1. Introduction

Seismic data have observed many low velocity zones at the Earth’s upper mantle [1], transition zone [2] and core mantle boundary [3], which might be related to the melting or partial melting of silicates. However, due to the limitation of techniques, static experimental studies on physicochemical properties of silicate melts are only investigated at relatively low pressure by large volume presses [4,5,6,7]. To overcome the limitations, theoretical calculations can be used to determine the properties of silicate melts at the whole conditions of the Earth’s interior [8,9,10]. In addition, silicate glass is commonly used as an analog to study the physicochemical properties of silicate melts at high pressure and temperature [11,12]. Thus, investigation on the high-pressure behaviors of silicate glass, such as sound velocity, structural transition and equation of state helps increase understanding of the properties of melt at high pressures [11,13,14].

MgSiO₃, as the most abundant component in the Earth’s mantle, is present as pyroxene and bridgmanite in the Earth’s upper and lower mantle, respectively. The structural and elastic properties of MgSiO₃ glass were investigated by X-ray absorbance, optical laser Raman, X-ray Raman scattering and Brillouin scattering at high pressures [12,14,15,16,17]. These studies indicate that MgSiO₃ glass undergoes a local structural change at the Mg site below 10 GPa and a Si-O coordination number (CN) change above 15.9 GPa [13,14,15,16,17]. Al₂O₃ is one of the most important components in the Earth’s mantle, and the Al₂O₃ contents in pyrolite bulk mantle and in the basalt layer of subducted oceanic lithosphere range between 4 and 16 wt.% [18,19]. The Al₂O₃ solubility in bridgmanite is up to 29 mol.% at 52 GPa [20]. However, to date, only one study investigated the structure and sound velocity of pyrope (Mg₃Al₂Si₃O₁₂) glass below 12.9 GPa [21], and thus the effect of Al₂O₃ on the physical properties of MgSiO₃ glass needs further investigation at higher pressures. In this study, considering the Al₂O₃ content in the Earth’s interior, we investigated the sound velocity of MgSiO₃ glass with two different Al₂O₃ contents up to 20 and 42 GPa. The results indicate that the incorporation of Al₂O₃ makes MgSiO₃ glass stiffer. The substitution of Al in the Si site could delay the transition of Si-O CN to higher pressure. The results have profound implications for understanding the behavior of silicate melts in the Earth’s lower mantle.

2. Experiments

MgSiO₃∙5 mol%Al₂O₃ (MA1) and MgSiO₃∙24.5 mol%Al₂O₃ (MA2) were prepared from oxide mixtures of reagent-grade MgO, SiO₂, and Al₂O₃ in 19:19:1 (MA1) and 3:3:1 (MA2) mole ratio. The starting materials were fused at 2000 K for 1 h, and then quenched into cold water. All the glasses were confirmed to have the intended chemical composition within the analytical uncertainties by using electron microprobe analysis (EMPA, JEOL Superprobe JXA-8230, JEOL Ltd., Jilin University (JLU), Changchun, China) and transmission electron microscopy (TEM, JEOL JEM-2200FS instrument, 200 kV, JEOL Ltd., JLU, Changchun, China) (

Table 1. Element compositions of MgSiO₃∙5 mol%Al₂O₃ and MgSiO₃∙24.5 mol%Al₂O₃ by electron microprobe analysis.

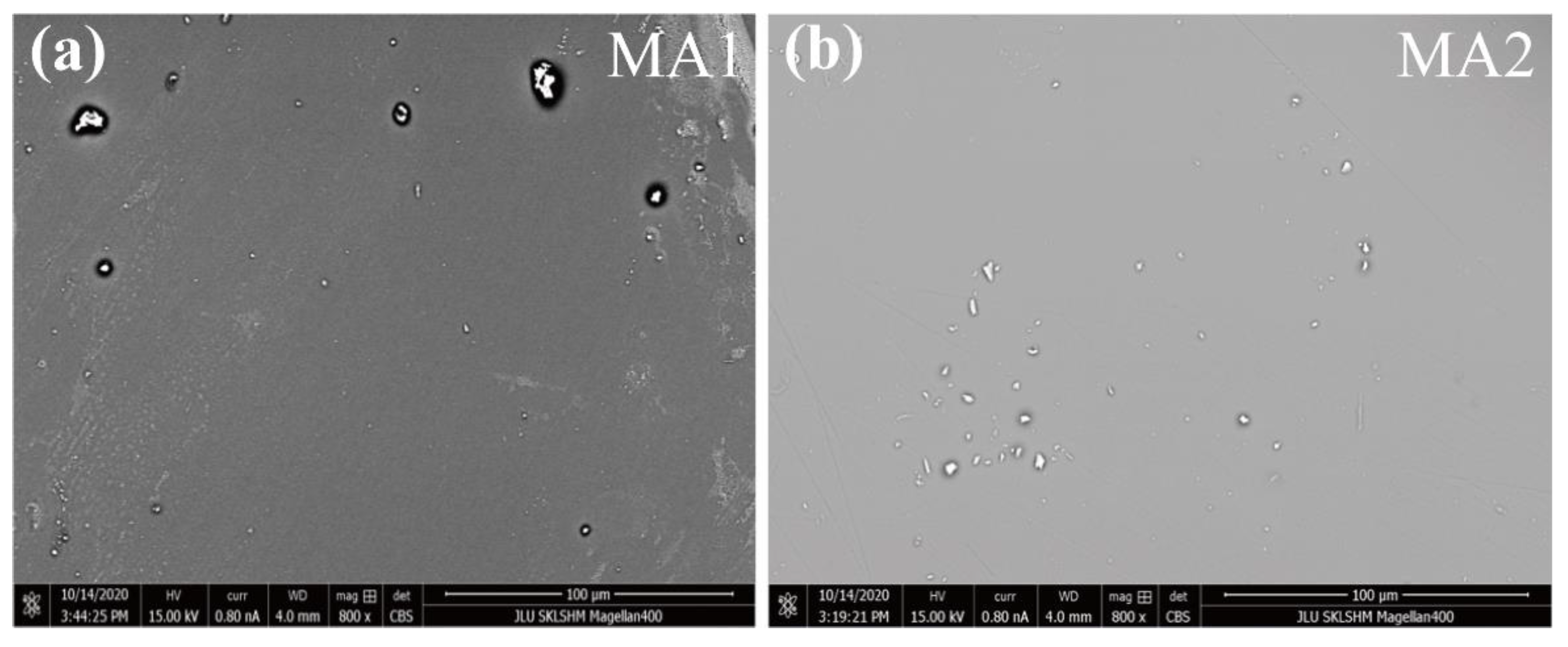
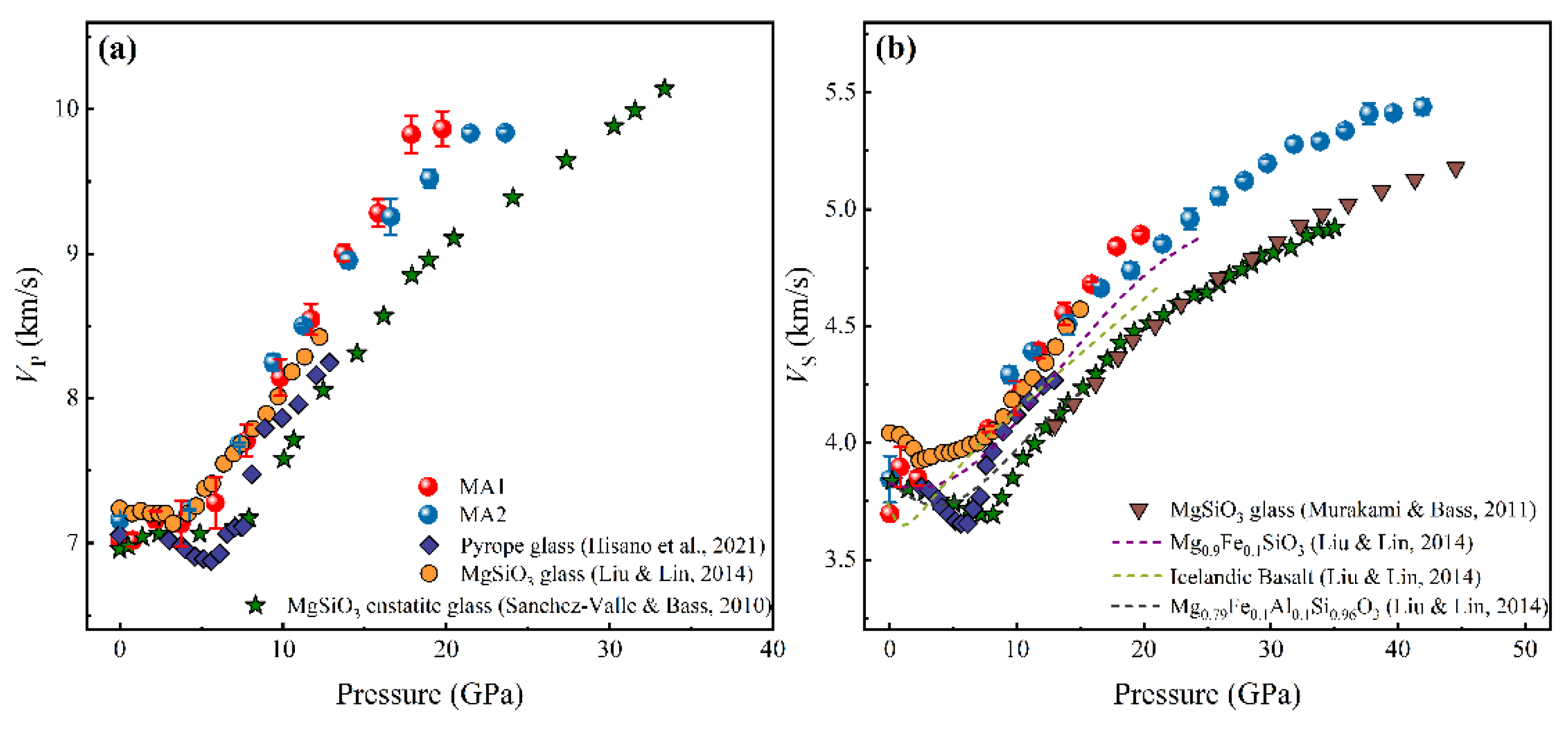
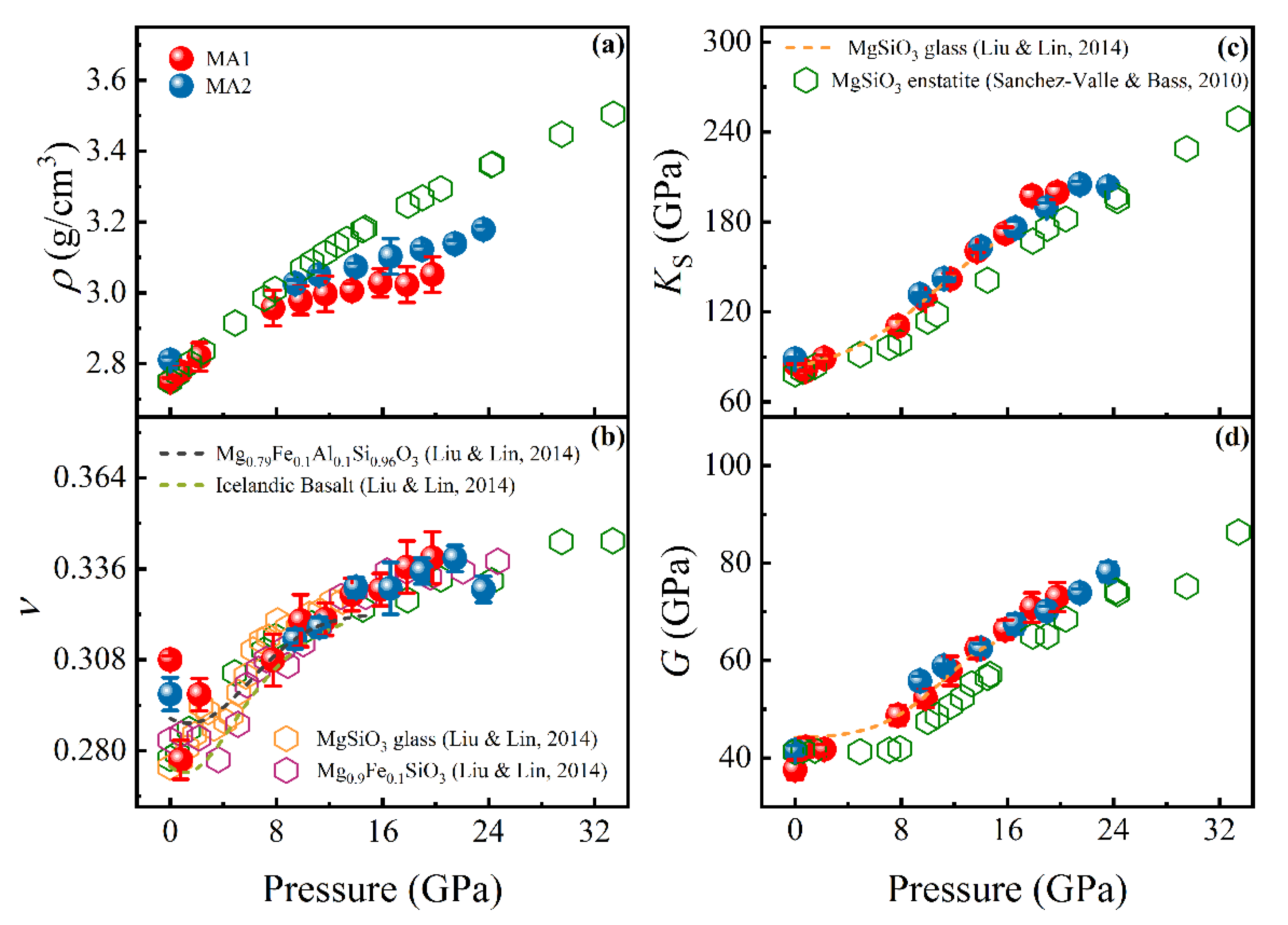
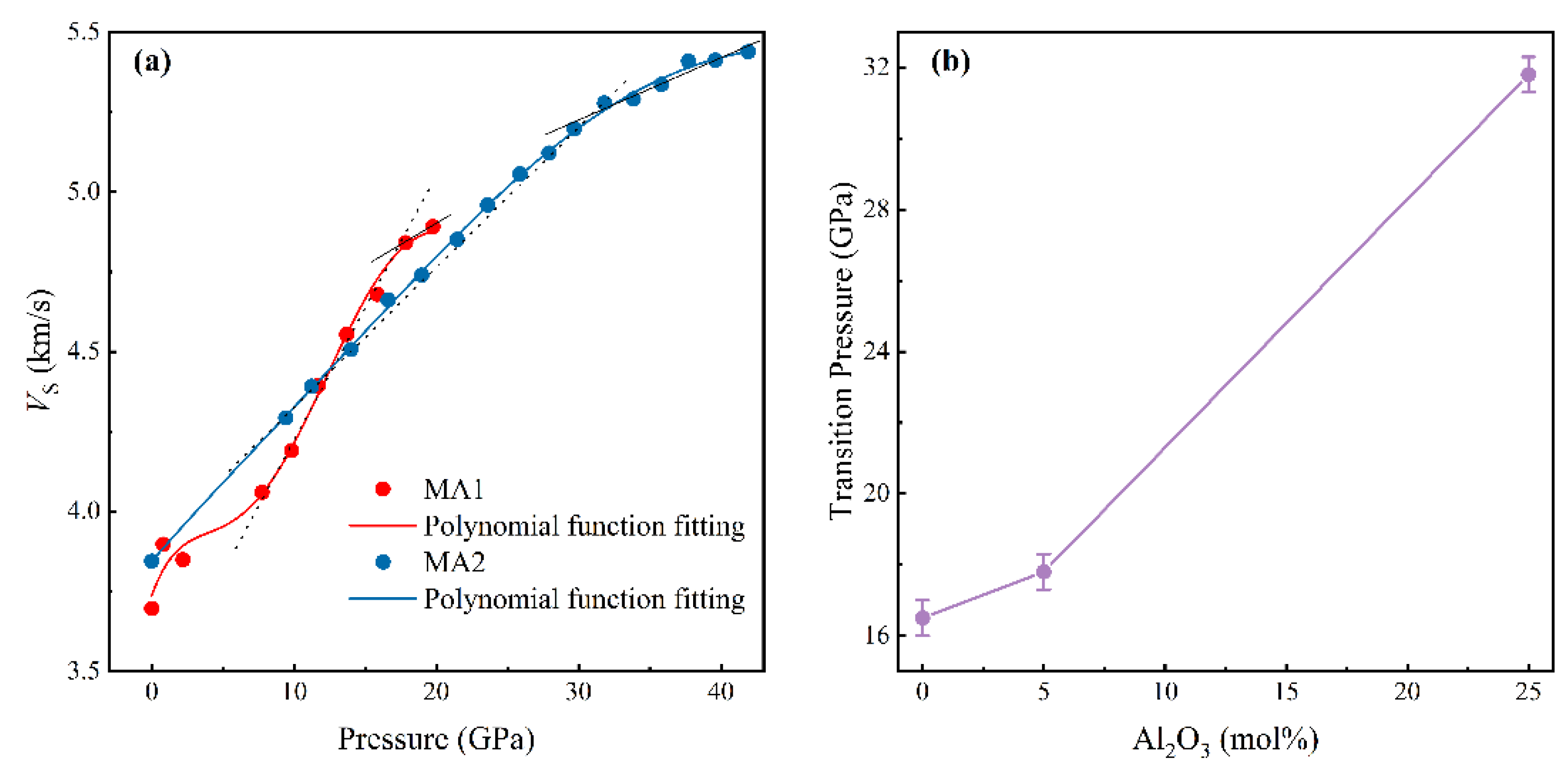
	Sample	MgSiO3∙5 mol%Al2O3 (Mg0.95Al0.1Si0.95O3)	MgSiO3∙24.5 mol%Al2O3 (Mg0.76Al0.49Si0.76O3)
Element
MgO		38.1(1)	30.3(1)
Al2O3		5.1(1)	24.7(1)
SiO2		56.8(1)	45.2(1)
Mg		0.95(2)	0.76(2)
Al		0.101(3)	0.49(1)
Si		0.95(2)	0.76(1)
O		3.00(6)	3.00(6)
Mg/Si		1	1

). The morphology and homogeneity of those glasses were confirmed by scanning electron microscope (SEM, Philips FEI Quanta Magellan 400, Philips, JLU, Changchun, China), which was equipped with backscattered electron (BSE, the resolution is ~10 nm) (Figure 1). The density of the glass was measured by the Archimedes method [22], and the density was 2.75 and 2.81 g/cm³ for MA1 and MA2 at ambient condition, respectively.

In situ high-pressure Brillouin scattering measurements of acoustic velocity were performed at room temperature in a BX-90 DAC [23] by using a solid-state laser as an incident light (532 nm) and a Sandercock-type six-pass tandem Fabry–Perot interferometer to analyze the scattered light. The incident laser was focused on the sample to a spot size of ~50 μm in diameter. The laser power was maintained at 0.3 and 0.5 W at room condition and high pressure, respectively. All measurements were made in the symmetric scattering geometry, and the velocities were calculated by the following equation without knowing the refractive index of the sample [24]:

$$\upsilon =\frac{\Delta \omega \lambda }{2\sin (\theta /2)}$$

(1)

where $\upsilon$ is the acoustic velocity, λ is the laser wavelength of the incident laser beam and θ is the external scattering angle; $\Delta \omega$ indicates the Brillouin frequency shifts of longitudinal or transverse acoustic modes. The external scattering angle is 60°.

The glass was polished to a platelet with parallel sides and a thickness of 20 μm and the samples were cut into 90 × 90 μm² and loaded into the DACs. A Rhenium gasket was pre-indented to 40 μm in thickness, and with a hole of 190 μm in diameter at the center of indentation that was used as the sample chamber. Because the noble gases with small molecular size, for example, He and Ne, could be incorporated into the structure of glass [25,26], we thus used argon as the pressure-transmitting medium. The sample was compressed with a 300 μm diamond culet. In each experiment, two ruby spheres were loaded next to the sample and were used as a pressure calibrant [27]. To stabilize the pressure, the DACs were kept for one night after increasing the pressure. Pressure was measured before and after Brillouin scattering measurements, and the pressure difference was within 0.2 GPa. We collected Brillouin spectra from at least two rotation angles at each pressure and confirmed the uniformity and isotropy of the samples. The difference of sound velocity was lower than 1% at different rotation angles at the same pressure. MA1 and MA2 were measured up to 20 and 42 GPa, respectively.

3. Results and Discussion

Both V_P and V_S for MA1 and MA2 can be observed in our Brillouin scattering measurements (Figure 2). However, the V_P are masked by the V_S of above 20 GPa, while the V_S of MA1 and MA2 between 4–8 GPa are also blocked by the V_P of Ar. The measured V_P and V_S of MA1 and MA2 as a function of pressure are shown in Figure 3 and

Table 2. Density and elastic properties of MA1 and MA2 determined up to 20 GPa and 42 GPa using Brillouin scattering in the diamond anvil cell, respectively.

MA1
Pressure (GPa)	$\rho$ (g/cm3)	VP (km/s)	VS (km/s)	KS (GPa)	G (GPa)	$\nu$
0	2.75(1)	7.02(1)	3.70(1)	85.4(3)	37.5(3)	0.308(1)
0.80	2.78(2)	7.02(5)	3.90(8)	80.6(2)	42(2)	0.277(6)
2.20	2.82(4)	7.17(5)	3.85(3)	89(2)	42(1)	0.298(5)
3.75	-	7.1(2)	-	-	-	-
5.85	-	7.3(2)	-	-	-	-
7.75	2.95(5)	7.7(1)	4.06(1)	111(5)	49(2)	0.308(8)
9.83	2.98(4)	8.2(1)	4.19(7)	128(3)	52(3)	0.320(8)
11.70	3.00(5)	8.6(1)	4.39(3)	142(4)	58(3)	0.321(5)
13.70	3.01(2)	9.01(5)	4.56(5)	160.6(9)	62(2)	0.328(5)
15.85	3.03(4)	9.28(9)	4.68(1)	173(4)	66(2)	0.330(5)
17.85	3.02(5)	9.8(1)	4.84(3)	197(5)	71(3)	0.337(8)
19.76	3.05(5)	9.8(1)	4.89(2)	200(5)	73(3)	0.340(8)
MA2
Pressure (GPa)	$\rho$ (g/cm3)	VP (km/s)	VS (km/s)	KS (GPa)	G (GPa)	$\nu$
0	2.81(1)	7.16(3)	3.8(1)	89(1)	42(1)	0.298(5)
4.25	-	7.23(1)	-	-	-	-
7.30	-	7.68(1)	-	-	-	-
9.41	3.03(1)	8.25(5)	4.29(4)	131(1)	56(1)	0.314(3)
11.23	3.05(1)	8.50(1)	4.391(4)	142(1)	58.9(4)	0.318(3)
14	3.07(1)	8.95(5)	4.51(4)	163(1)	63(1)	0.330(3)
16.60	3.10(5)	9.3(1)	4.662(5)	176(5)	68(2)	0.330(8)
18.97	3.12(1)	9.52(6)	4.74(4)	189(3)	70(1)	0.335(4)
21.47	3.14(1)	9.83(3)	4.85(2)	205(2)	73.9(8)	0.339(4)
23.61	3.18(1)	9.84(3)	4.96(5)	203(1)	78(2)	0.330(4)
25.89	-	-	5.06(3)	-	-	-
27.92	-	-	5.12(2)	-	-	-
29.70	-	-	5.20(2)	-	-	-
31.83	-	-	5.28(3)	-	-	-
33.96	-	-	5.29(2)	-	-	-
35.84	-	-	5.34(2)	-	-	-
37.72	-	-	5.41(4)	-	-	-
39.62	-	-	5.411(1)	-	-	-
41.92	-	-	5.44(3)	-	-	-

. MA1 and MA2 have basically the same V_P and V_S within the uncertainties (Figure 3). Using the obtained sound velocities, the density (ρ), bulk modulus (K_S), shear modulus (G) and Poisson’s ratio ($\mathsf{\nu }$) of MA1 and MA2 were calculated at high pressures (Figure 4; Table 2).

The densities are calculated by the following equation [14]:

$$\frac{\partial \rho }{\partial P}=\frac{1}{V_{\mathrm{B}}^2}.$$

(2)

where $V_{\mathrm{B}}^2{=V}_{\mathrm{P}}^2-\frac{4}{3}{V}_{\mathrm{S}}^2$. Integrating Equation (2), we obtain:

$${\rho - \rho }_0={{\displaystyle \int }}_{P_0}^{P }\frac{\mathit{1}}{V_{\mathrm{B}}^2}\mathrm{d}\mathit{P}.$$

(3)

where $\rho$ and ${\rho }_0$ are densities at high pressures (P) and ambient pressure (P₀), respectively. $V_{\mathrm{B}}$ is the bulk sound velocity.

The K_S and G are calculated by the following equation:

$$V_{\mathrm{P}}=\sqrt{\frac{K_{\mathrm{S}}+\frac{4G}{3}}{\rho }}.$$

(4)

$$V_{\mathrm{S}}=\sqrt{\frac{G}{\rho }}.$$

(5)

where V_P is compressional wave velocity, V_S is shear wave velocity, K_S is the adiabatic bulk modulus, G is the shear modulus and $\rho$ is density.

The Poisson’s ratio ($\nu$) is calculated from the acoustic velocities using the following relationship:

$$\nu =\frac{1}{2}\frac{{\left(V_{\mathrm{P}}/V_{\mathrm{S}}\right)}^2-2}{{\left(V_{\mathrm{P}}/V_{\mathrm{S}}\right)}^2-1}.$$

(6)

To compare the sound velocities of different compositional silicate glasses at high pressures, V_P and V_S from different groups are summarized in Figure 3 [12,13,14]. The results show large discrepancies from different studies (Figure 3) [12,13,14,21]. Studies indicate that the incorporation of noble gas in the structure of glass could make it stiffer [25,26]. Thus, the pressure mediums need to be considered when comparing the results from different groups. For pure MgSiO₃ glass, the velocity of Sanchez-Valle and Bass [14] is in good agreement with Murakami and Bass [13], but lower than the results of Liu and Lin [12]. The discrepancies in the three groups may be due to the different pressure medium used in the experiments, where Ar was used in Sanchez-Valle and Bass [14], Ne was used in Liu and Lin [12], and no pressure medium was used in Murakami and Bass [13]. Because Ar was also used in our experiment, the results of Sanchez-Valle and Bass [14] can be a reference for understanding the effect of Al₂O₃ on the sound velocity of MgSiO₃ glass. In comparison with the results of Sanchez-Valle and Bass [14], the incorporation of 5 mol% Al₂O₃ into MgSiO₃ glass increases its V_P and V_S by 6.5–8.3% and 7.4–10% at 10–20 GPa, respectively. In particular, the MA2 exhibits similar sound velocity with MA1, indicating that the additional Al₂O₃ has a small effect on the sound velocity of MgSiO₃ glass. In addition, MA2 with a similar composition as used in Hisano et al. [21] shows ~4% and ~5% higher V_P and V_S at high pressures. The large volume press combined with ultrasonic measurements were used in Hisano et al. [21], and the discrepancy should be caused by the uncertainty in different techniques. In particular, compared with different compositional MgSiO₃ glass, despite the effect of pressure medium on the sound velocity, the incorporation of Al₂O₃ could substantially enhance the stiffness of MgSiO₃ glass (Figure 3).

At the pressure below 10 GPa, Al-free and Al-bearing MgSiO₃ glasses have similar density (Figure 4a). However, the pure MgSiO₃ glass shows the greatest density above 10 GPa because it has the largest compressibility among these three types of MgSiO₃ glass. In addition, the incorporation of Al₂O₃ in the MgSiO₃ glass could increase the K_S and G, indicating that Al₂O₃ could induce the stiffness of MgSiO₃ glass (Figure 4c,d). However, the effect of Al₂O₃ content on the stiffness should be non-linear at high pressures (Figure 5). The non-linear effect of incorporated element on the stiffness and hardness was also observed in the superhard materials [28,29,30]. The incorporation of Al₂O₃ in different content would have effects on the valence-electron volumetric density (EVD) and structure of MgSiO₃ glass. Thus, MA1 and MA2 exhibit similar K_S and G at high pressures. Compared with the Poisson’s ratios with different compositional silicate glass (Figure 4b), MA1 and MA2 show a similar value, indicating that the composition has a limited effect on Poisson’s ratios [12].

Overall, the velocities of MgSiO₃-type glass have an abnormal change between 2 and 10 GPa (Figure 3). Using magnesium L-edge spectra from XRS, one study indicated that the Mg site in MgSiO₃ glass has a distortion and local structural change below 10 GPa, which is consistent with the pressure range of the abnormal change [15]. Another change of gradient in V_S is also reported between 15–17.9 GPa [14] (Figure 3). Both silicon L-edge and oxygen K-edge indicate a change in the CN for Si-O above 10 GPa [15,17]. Thus, the change of gradient in V_S should be caused by the transition of CN of Si-O or the decreasing of Si-O-Si angle [15,16,17].

To understand the transition of the Si-O polyhedral, the V_S is fitted with a polynomial function to assess the trend change of MgSiO₃-Al₂O₃ glass as a function of pressure (Figure 6a). The change of dV_S/dP occurs at 17.8 GPa and 31.8 GPa in MA1 and MA2, respectively, indicating the transition of CN of Si-O from 4 to 5- or 6-fold coordination in the glass [14]. The transition pressure of MA1 is 2 GPa higher than that of MgSiO₃ glass [14], and the transition pressure of MA2 is 15 GPa higher than that of MgSiO₃ glass (Figure 6b). The results suggested that the incorporation of Al cations delays the transition pressure for the CN of Si-O in MgSiO₃ glass.

4. Implications

Our sound velocity studies on Al₂O₃-MgSiO₃ to lower mantle pressures indicate the effect of Al₂O₃ on the elastic properties and structural changes of MgSiO₃ glass. If the change also occurs in the analog melt, it would strongly affect the dynamics of the magma ocean during the Earth’s evolution. Density is an important factor for understanding the coexistence between melt and solid phase in the Earth’s interior. Previous study indicates that the density of bridgmanite intersected with that of magnesium silicate glass in the deep mantle [14]. However, Al₂O₃ is a critical component in the Earth’s interior, and 4–16 wt.% (4.1–16.3 mol.%) Al₂O₃ can be present in the Earth’s mantle [18,19]. Our study shows that the density of Al-bearing MgSiO₃ glass is much lower than that of Al-bearing bridgmanite [31] (Figure 7). Because of the lower density of Al-bearing MgSiO₃ than Al-bearing bridgmanite (Figure 7), Al-bearing MgSiO₃ melt tends to upwell and cannot coexist with bridgmanite in the deep Earth’s interior. In addition, the incorporation of Al₂O₃ could make the glass stiffer; if this also happens in the analog melt, it will increase the V_P of melt and help to understand the seismic signature of Al-bearing MgSiO₃ melt in the Earth’s interior.

5. Conclusions

In summary, the acoustic velocity of MgSiO₃ 5 mol%Al₂O₃ (MA1) and MgSiO₃∙24.5 mol%Al₂O₃ (MA2) glass were measured up to 20 and 42 GPa, respectively. In comparison with pure MgSiO₃ glass, Al-bearing MgSiO₃ glass exhibited higher velocity, indicating that the incorporation of Al₂O₃ could increase the sound velocity of MgSiO₃ glass. Using obtained velocities, the bulk and shear moduli (K_S, G), density ($\rho$) and Poisson’s ratio ($\nu$) of MA1 and MA2 are calculated at high pressures, and the results indicate that Al₂O₃ could induce the stiffness of MgSiO₃ glass. However, the effect of Al₂O₃ content on the stiffness of MgSiO₃ glass is non-linear, and MA1 and MA2 exhibit similar K and G at high pressures. With the increase of pressure, transverse acoustic modes (V_S) of MA1 and MA2 show abnormal changes at the pressure of 17.8 GPa and 31.8 GPa, which are related to the transition of coordination number (CN) for Si-O in Al-bearing MgSiO₃ glass. Compared with previous studies on sound velocity of MgSiO₃ glass, the incorporation of Al₂O₃ delays the transition pressure of Si-O coordination to higher pressures. Our study also indicates that the density of Al-bearing MgSiO₃ glass is much lower than that of Al-bearing bridgmanite, and the Al-bearing MgSiO₃ is unlikely to co-exist with Al-bearing bridgmanite in the Earth’s lower mantle.