Molecular Simulation of Methane Adsorption in Deep Shale Nanopores: Effect of Rock Constituents and Water
Abstract
:1. Introduction
2. Methodology of Molecular Simulation
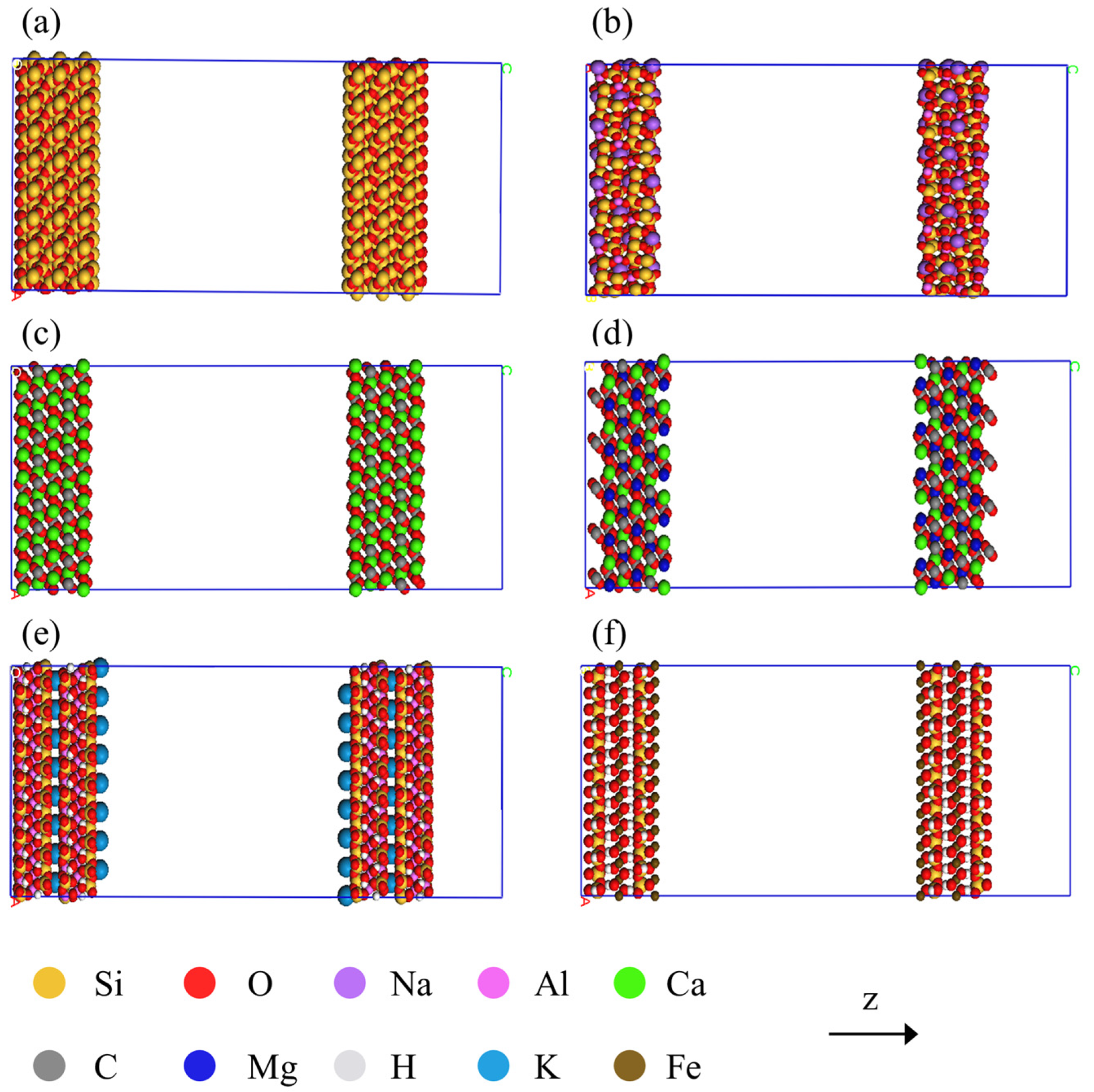
2.1. Molecular Models of Various Shale Constituents
2.2. Simulation Detail of Gas–Water Adsorption
3. Effects of Shale Constituents
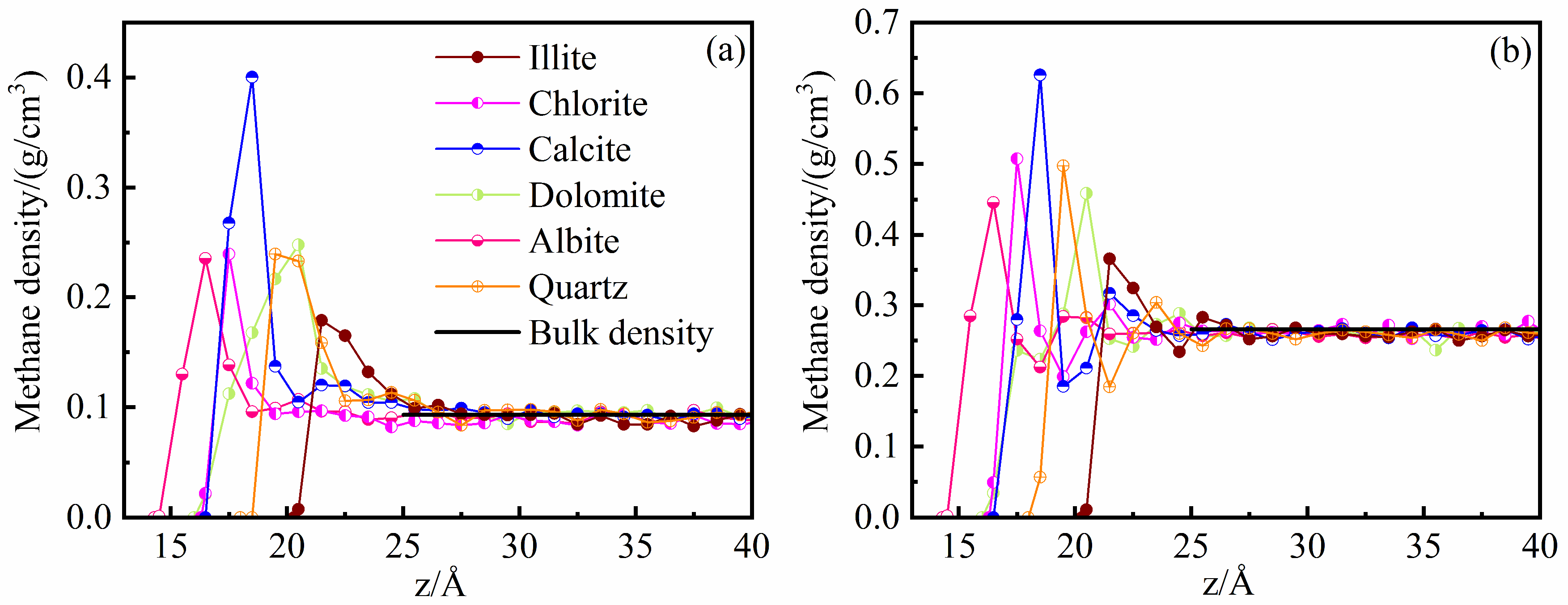
3.1. Differences between Inorganic Mineral Compositions
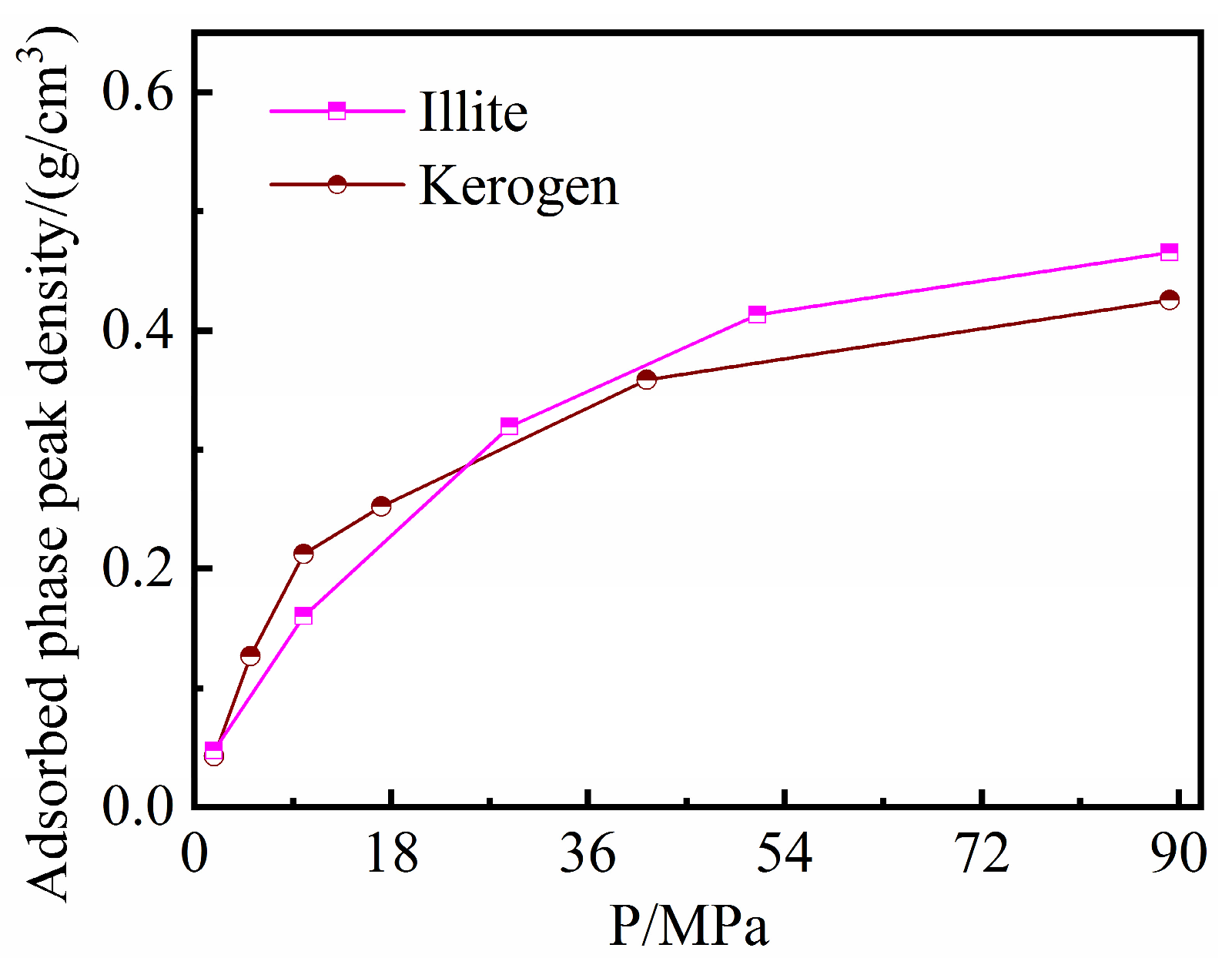
3.2. Discrepancies between Illite and Kerogen
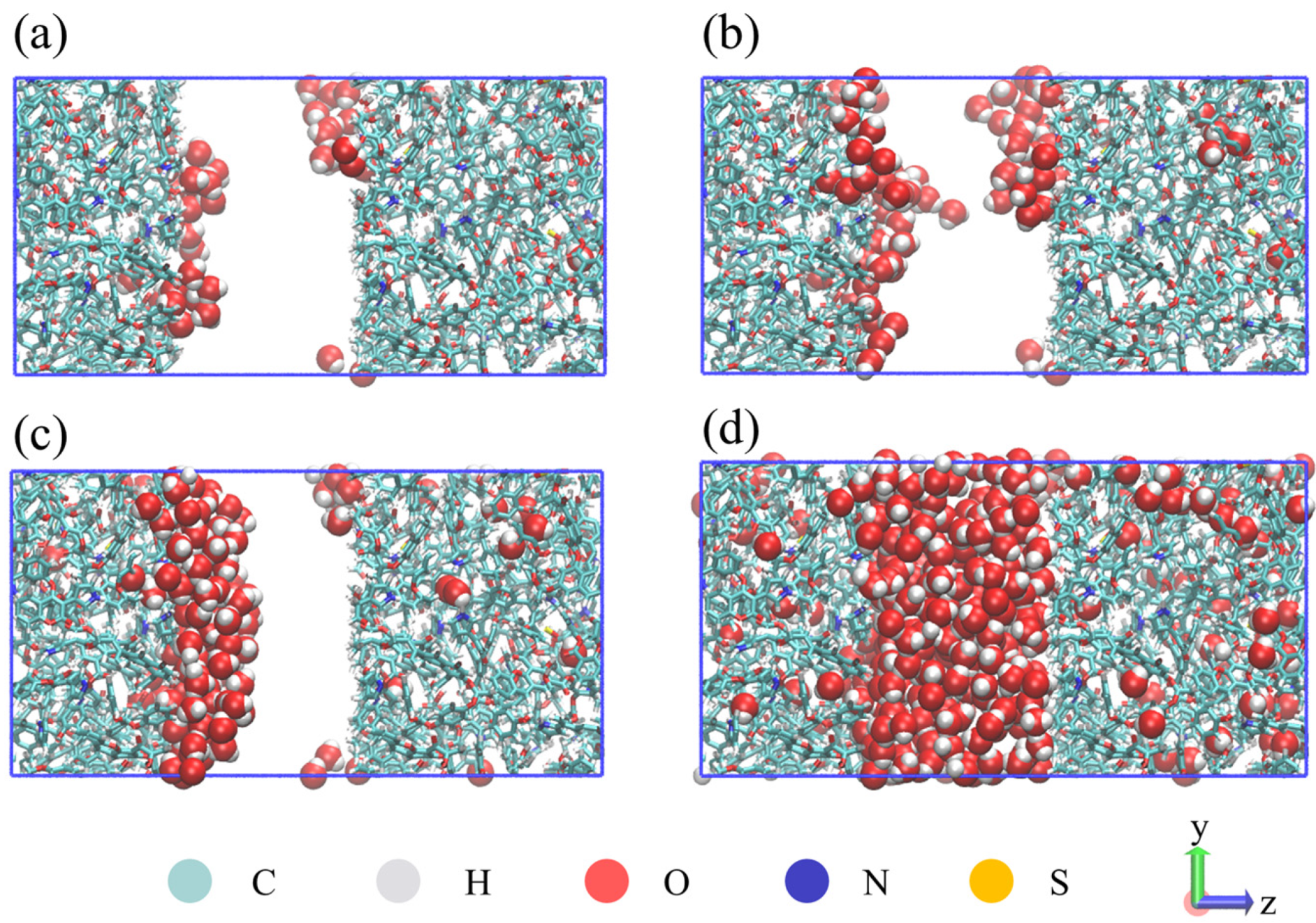
4. Effects of Water in Shale Nanopores
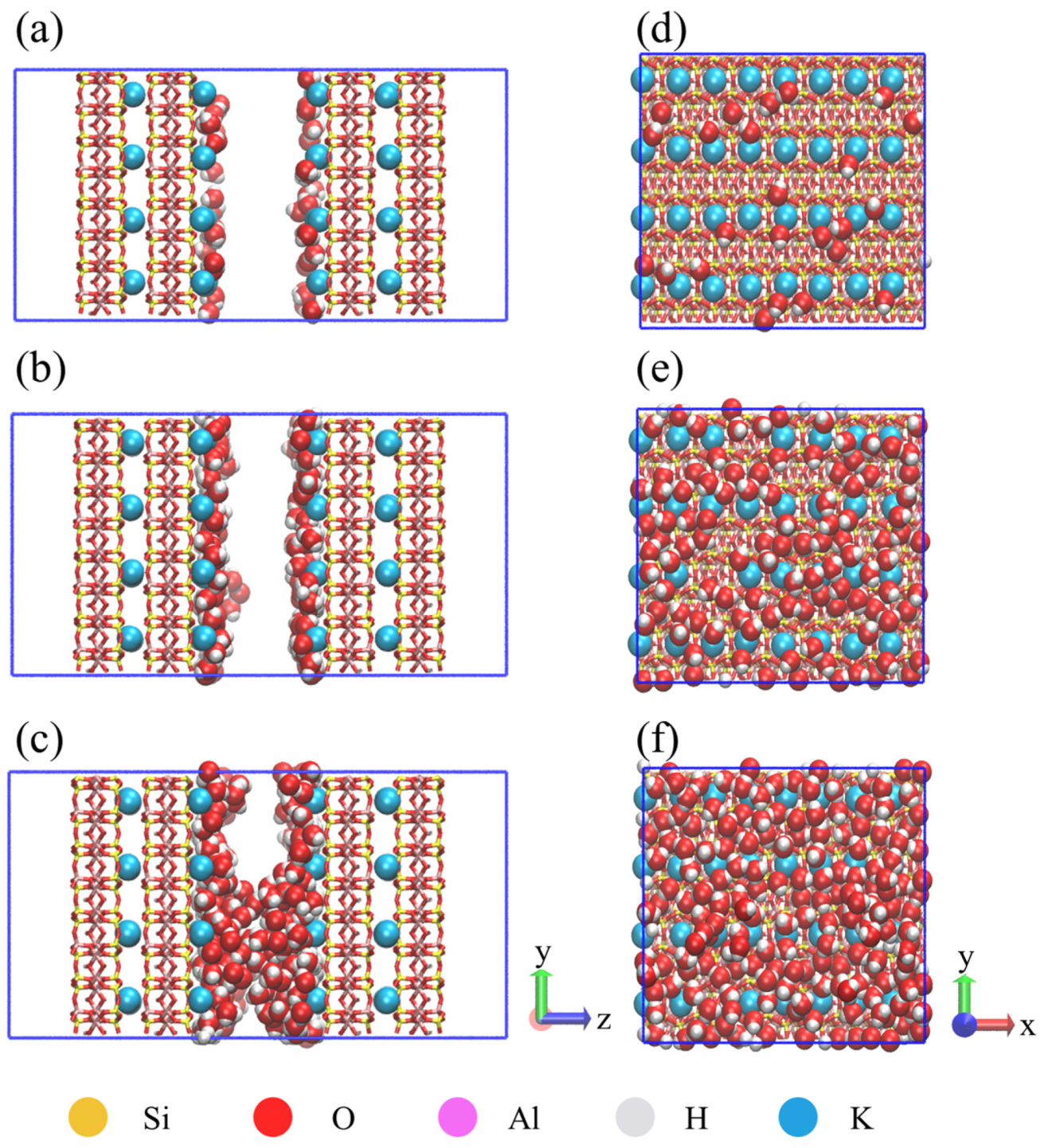
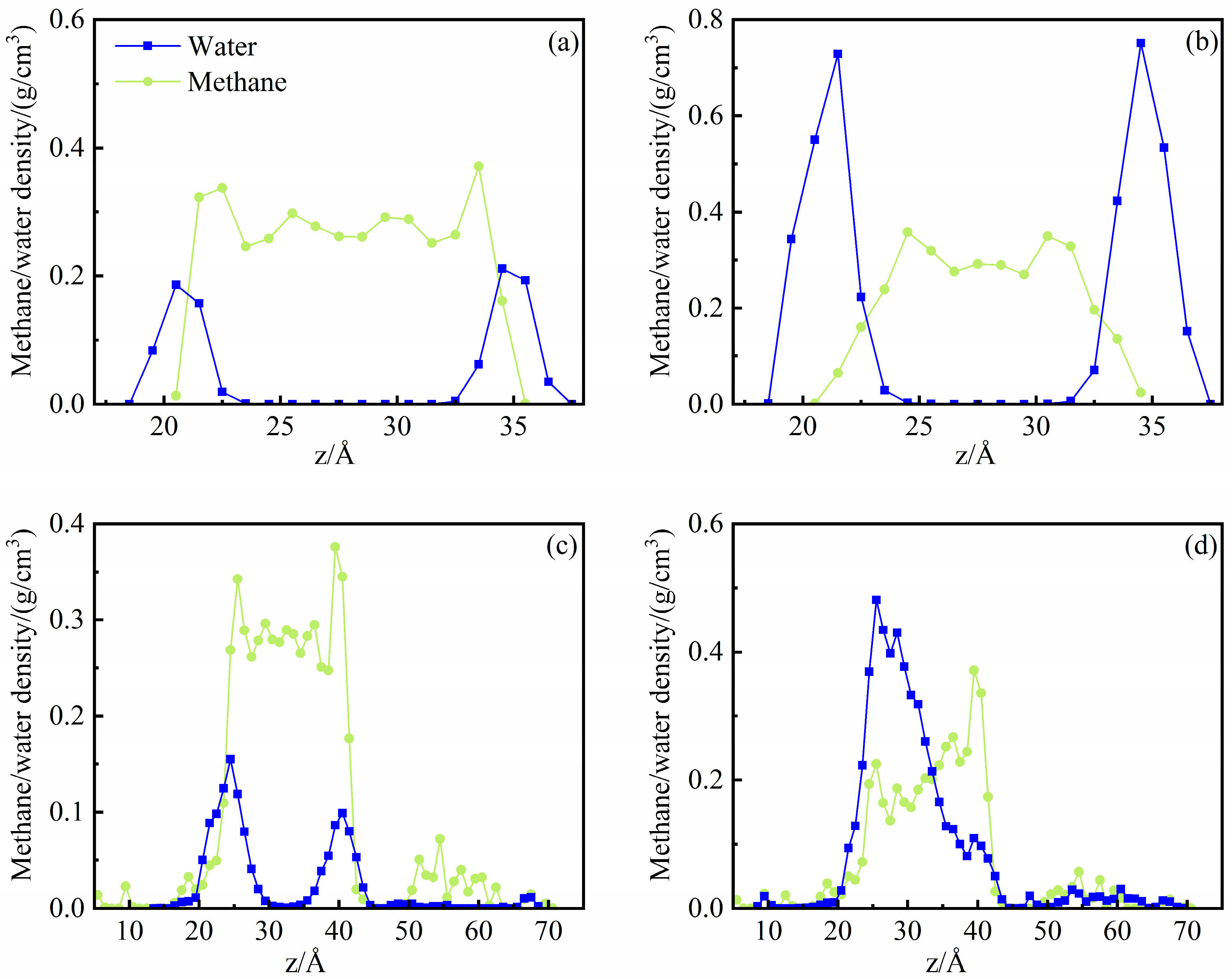
4.1. Microscopic Distribution of Water
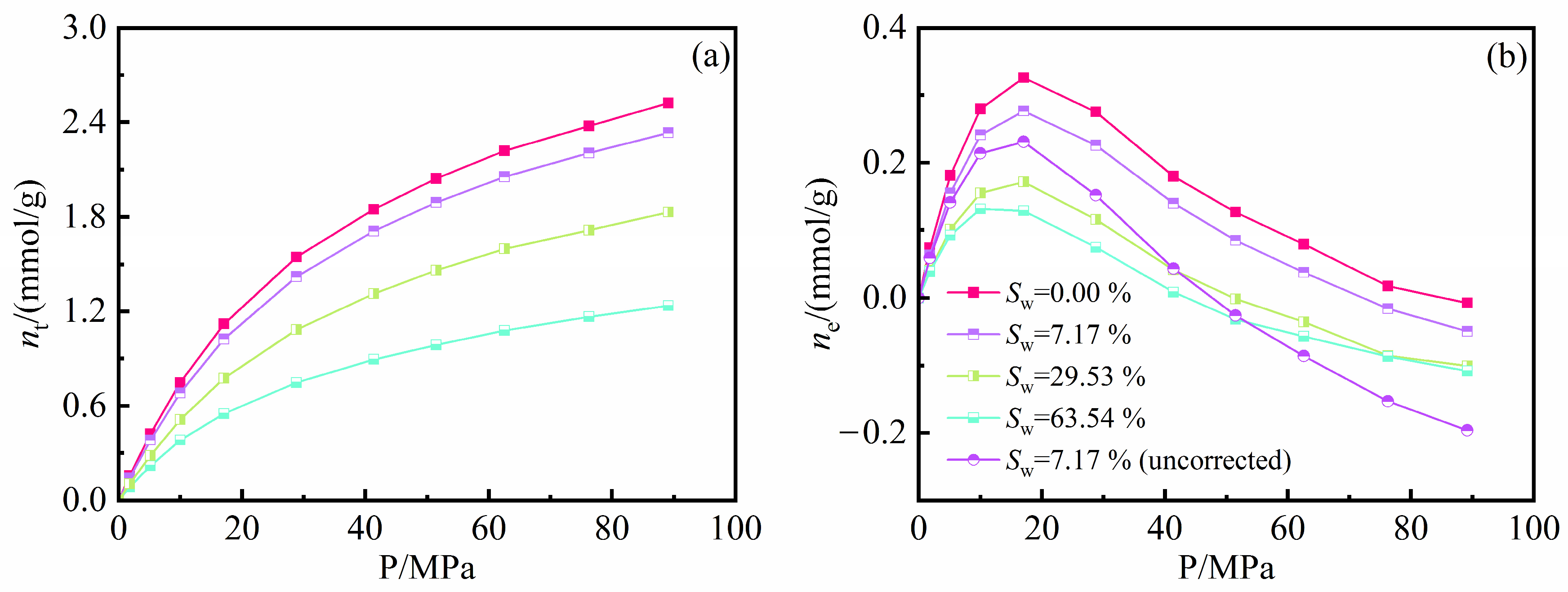
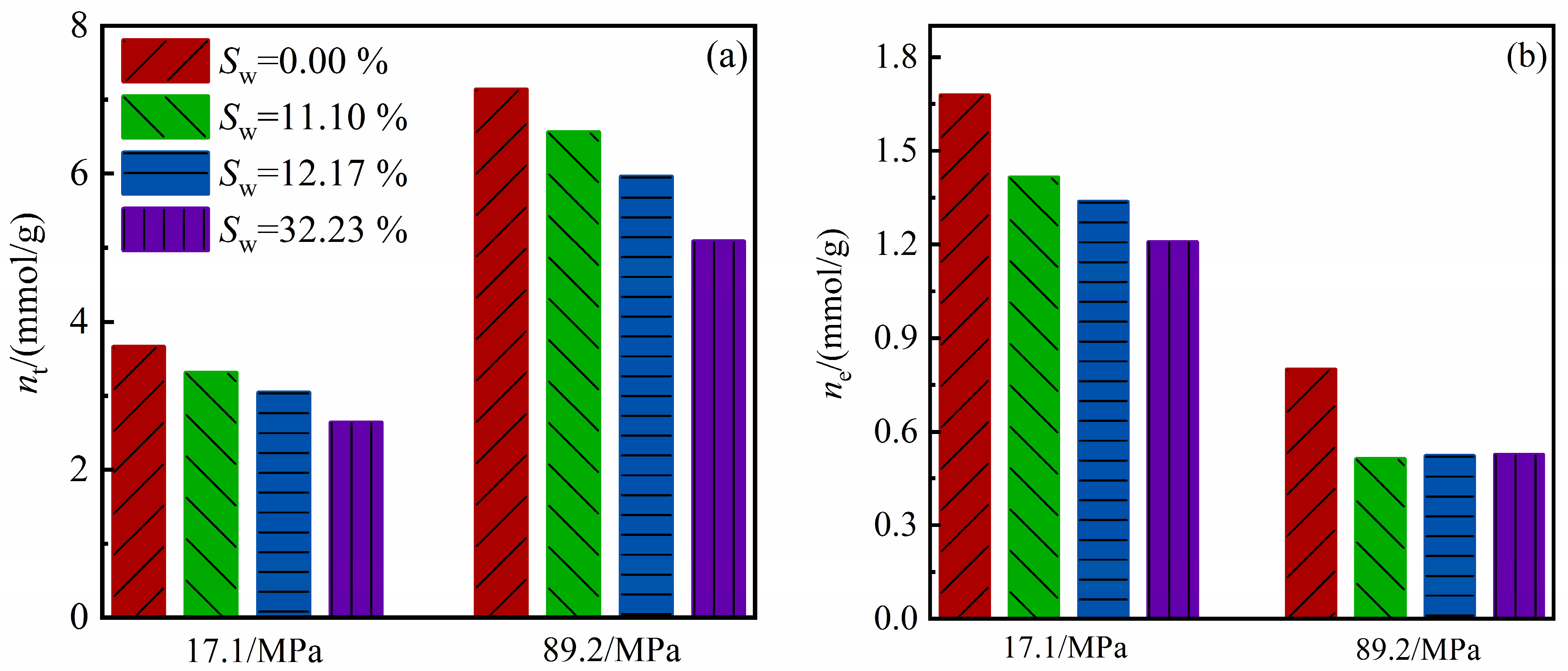
4.2. Water Effects on Methane Amount
4.3. Competitive Adsorption between Water and Methane
5. Conclusions
- (1)
- Despite the discrepancies in methane adsorption capacity, the microscopic adsorption characteristics of methane in different mineral nanopores are basically consistent. At low pressure, methane is adsorbed in a monolayer form on the pore wall of each mineral. At high pressure, a concavo-convex transition zone forms close to the methane adsorption layer, complicating the correlation of the adsorption phase density with the excess adsorption amounts between minerals.
- (2)
- Kerogen and illite slit pores do not differ significantly in terms of their intrinsic adsorption capacity for methane. The adsorption capacity per unit mass of kerogen is greater than that of illite due to the smaller molar mass of the kerogen skeleton and its large intermolecular porosity. The intermolecular pores of the kerogen matrix can accommodate part of the methane molecules in a dissolved state.
- (3)
- In the illite pores, water molecules gradually occupy the high-energy adsorption sites of the pore wall and spread along the wall to form an adsorption layer. The affinity of the illite wall towards water is greater than that towards methane, and the methane adsorption layers are between the water adsorption layers on the pore walls. Methane adsorption has little effect on water adsorption characteristics.
- (4)
- In the kerogen pores, water molecules are preferentially aggregated on the polar functional groups to form clusters. At a high water content, methane adsorption can promote the fusion of the water clusters and inhibit the spread of the water clusters along the wall. Part of the water molecules can be dissolved in the kerogen matrix.
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Huang, S.; Ma, X.; Yang, H.; Wu, J.; Zhang, J.; Zhao, S.; Zhang, D.; Ren, C.; Huang, L. Experimental characterization and molecular modeling of kerogen in Silurian deep gas shale from southern Sichuan Basin, China. Energy Rep. 2022, 8, 1497–1507. [Google Scholar] [CrossRef]
- Loucks, R.G.; Ruppel, S.C. Mississippian Barnett Shale: Lithofacies and depositional setting of a deep-water shale-gas succession in the Fort Worth Basin, Texas. AAPG Bull. 2007, 91, 579–601. [Google Scholar] [CrossRef]
- Yasin, Q.; Sohail, G.M.; Liu, K.-Y.; Du, Q.-Z.; Boateng, C.D. Study on brittleness templates for shale gas reservoirs-A case study of Longmaxi shale in Sichuan Basin, southern China. Pet. Sci. 2021, 18, 1370–1389. [Google Scholar] [CrossRef]
- Karamov, T.; White, V.; Idrisova, E.; Kozlova, E.; Burukhin, A.; Morkovkin, A.; Spasennykh, M. Alterations of carbonate mineral matrix and kerogen micro-structure in Domanik organic-rich shale during anhydrous pyrolysis. Minerals 2022, 12, 870. [Google Scholar] [CrossRef]
- Godinho, J.R.A.; Ma, L.; Chai, Y.; Storm, M.; Burnett, T.L. Mineral precipitation in fractures and nanopores within shale imaged using time-lapse x-ray tomography. Minerals 2019, 9, 480. [Google Scholar] [CrossRef]
- Chalmers, G.R.; Bustin, R.M.; Power, I.M. Characterization of gas shale pore systems by porosimetry, pycnometry, surface area, and field emission scanning electron microscopy/transmission electron microscopy image analyses: Examples from the Barnett, Woodford, Haynesville, Marcellus, and Doig units. AAPG Bull. 2012, 96, 1099–1119. [Google Scholar]
- Kar, N.R.; Mani, D.; Mukherjee, S.; Dasgupta, S.; Puniya, M.K.; Kaushik, A.K.; Biswas, M.; Babu, E.V.S.S.K. Source rock properties and kerogen decomposition kinetics of Eocene shales from petroliferous Barmer basin, western Rajasthan, India. J. Nat. Gas Sci. Eng. 2022, 100, 104497. [Google Scholar] [CrossRef]
- Curtis, J.B. Fractured shale-gas systems. AAPG Bull. 2002, 86, 1921–1938. [Google Scholar]
- Mohd Aji, A.Q.M.; Mohshim, D.F.; Maulianda, B.; El-Raeis, K.A. Molecular simulation study on methane adsorption in amorphous shale structure. Minerals 2023, 13, 214. [Google Scholar] [CrossRef]
- Turlapati, V.Y.; Prusty, B.K.; Pal, S.K.; Raja, E. Examining supercritical methane adsorption on nanoporous shales using constant and varying adsorbed phase density approaches. Energy Fuels 2023, 37, 2078–2090. [Google Scholar] [CrossRef]
- Heller, R.; Zoback, M. Adsorption of methane and carbon dioxide on gas shale and pure mineral samples. J. Unconv. Oil Gas Resour. 2014, 8, 14–24. [Google Scholar] [CrossRef]
- Tian, Y.; Yan, C.; Jin, Z. Characterization of methane excess and absolute adsorption in various clay nanopores from molecular simulation. Sci. Rep. 2017, 7, 12040. [Google Scholar] [CrossRef] [PubMed]
- Onawole, A.T.; Nasser, M.S.; Hussein, I.A.; Al-Marri, M.J.; Aparicio, S. Theoretical studies of methane adsorption on Silica-Kaolinite interface for shale reservoir application. Appl. Surf. Sci. 2021, 546, 149164. [Google Scholar] [CrossRef]
- Bakshi, T.; Vishal, V. A review on the role of organic matter in gas adsorption in shale. Energy Fuels 2021, 35, 15249–15264. [Google Scholar] [CrossRef]
- Huang, L.; Ning, Z.; Wang, Q.; Zhang, W.; Cheng, Z.; Wu, X.; Qin, H. Effect of organic type and moisture on CO2/CH4 competitive adsorption in kerogen with implications for CO2 sequestration and enhanced CH4 recovery. Appl. Energy 2018, 210, 28–43. [Google Scholar] [CrossRef]
- Josh, M.; Esteban, L.; Piane, C.D.; Sarout, J.; Dewhurst, D.; Clennell, M. Laboratory characterisation of shale properties. J. Pet. Sci. Eng. 2012, 88, 107–124. [Google Scholar] [CrossRef]
- Diaz-Perez, A.; Cortes-Monroy, I.; Roegiers, J.C. The role of water/clay interaction in the shale characterization. J. Pet. Sci. Eng. 2007, 58, 83–98. [Google Scholar] [CrossRef]
- Kadoura, A.; Nair, A.K.N.; Sun, S. Adsorption of carbon dioxide, methane, and their mixture by montmorillonite in the presence of water. Microporous Mesoporous Mater. 2016, 225, 331–341. [Google Scholar] [CrossRef]
- Huang, L.; Zhou, W.; Xu, H.; Wang, L.; Zou, J.; Zhou, Q. Dynamic fluid states in organic-inorganic nanocomposite: Implications for shale gas recovery and CO2 sequestration. Chem. Eng. J. 2021, 411, 128423. [Google Scholar] [CrossRef]
- Ruppert, L.F.; Sakurovs, R.; Blach, T.P.; He, L.; Melnichenko, Y.B.; Mildner, D.F.R.; Alcantar-Lopez, L. A USANS/SANS study of the accessibility of pores in the Barnett Shale to methane and water. Energy Fuels 2013, 27, 772–779. [Google Scholar] [CrossRef]
- Abdulelah, H.; Negash, B.M.; Keshavarz, A.; Hoteit, H.; Iglauer, S. Interfacial and wetting properties in shale/methane/water and shale/methane/surfactant systems at geological conditions. Energy Fuels 2022, 36, 10155–10166. [Google Scholar] [CrossRef]
- Zhang, B.; Kang, J.; Kang, T. Effect of water on methane adsorption on the kaolinite (0 0 1) surface based on molecular simulations. Appl. Surf. Sci. 2018, 439, 792–800. [Google Scholar] [CrossRef]
- Merkel, A.; Fink, R.; Littke, R. The role of pre-adsorbed water on methane sorption capacity of Bossier and Haynesville shales. Int. J. Coal Geol. 2015, 147, 1–8. [Google Scholar] [CrossRef]
- Geatches, D.L.; Wilcox, J. Ab initio investigations of dioctahedral interlayer-deficient mica: Modelling 1 M polymorphs of illite found within gas shale. Eur. J. Mineral. 2014, 26, 127–144. [Google Scholar] [CrossRef]
- Plimpton, S. Fast parallel algorithms for short-range molecular dynamics. J. Comput. Phys. 1995, 117, 1–19. [Google Scholar] [CrossRef]
- Cygan, R.T.; Liang, J.J.; Kalinichev, A.G. Molecular models of hydroxide, oxyhydroxide, and clay phases and the development of a general force field. J. Phys. Chem. B 2004, 108, 1255–1266. [Google Scholar] [CrossRef]
- Xiao, S.; Edwards, S.A.; Gräter, F. A new transferable forcefield for simulating the mechanics of CaCO3 crystals. J. Phys. Chem. C 2011, 115, 20067–20075. [Google Scholar] [CrossRef]
- Hagler, A.T.; Lifson, S.; Dauber, P. Consistent force field studies of intermolecular forces in hydrogen-bonded crystals. 2. A benchmark for the objective comparison of alternative force fields. J. Am. Chem. Soc. 1979, 101, 5122–5130. [Google Scholar] [CrossRef]
- Martin, M.G.; Siepmann, J.I. Transferable potentials for phase equilibria. 1. United-atom description of n-alkanes. J. Phys. Chem. B 1998, 102, 2569–2577. [Google Scholar] [CrossRef]
- Berendsen, H.J.C.; Grigera, J.R.; Straatsma, T.P. The missing term in effective pair potentials. J. Phys. Chem. 1987, 91, 6269–6271. [Google Scholar] [CrossRef]
- Shelley, J.C.; Patey, G.N. Boundary condition effects in simulations of water confined between planar walls. Mol. Phys. 1996, 88, 385–398. [Google Scholar] [CrossRef]
- Lorentz, H.A. Ueber die anwendung des satzes vom virial in der kinetischen theorie der gase. Ann. Der Phys. 1881, 248, 127–136. [Google Scholar] [CrossRef]
- Nosé, S. A unified formulation of the constant temperature molecular dynamics methods. J. Chem. Phys. 1984, 81, 511–519. [Google Scholar] [CrossRef]
- Gelb, L.D.; Gubbins, K.E. Pore size distributions in porous glasses: A computer simulation study. Langmuir 1999, 15, 305–308. [Google Scholar] [CrossRef]
- Huang, L.; Chen, Q.; Wu, J.; Yang, Q.; Zhang, J.; Huang, S.; Zhou, W.; Zou, J. Molecular simulation of methane adsorption characteristics in illite nanopores of deep shale reservoirs. J. Cent. South Univ. (Sci. Technol.) 2022, 53, 3522–3531. [Google Scholar]



| Sample | Mineral Composition/w.t.% | ||||||
|---|---|---|---|---|---|---|---|
| Quartz | Feldspar | Calcite | Dolomite | Illite | Chlorite | Others | |
| S1 | 81.27 | 4.57 | 1.03 | 3.24 | 3.54 | 2.51 | 3.83 |
| S2 | 63.27 | 8.41 | 6.64 | 6.19 | 7.67 | 2.65 | 5.16 |
| S3 | 56.19 | 4.87 | 12.68 | 9.88 | 6.49 | 4.42 | 5.46 |
| S4 | 74.48 | 6.05 | 3.39 | 3.83 | 4.13 | 1.92 | 6.19 |
| S5 | 47.35 | 13.42 | 2.21 | 1.77 | 23.89 | 6.19 | 5.16 |
| S6 | 29.20 | 9.88 | 6.19 | 7.08 | 36.58 | 6.64 | 4.42 |
| S7 | 26.70 | 12.39 | 3.24 | 6.49 | 38.94 | 7.08 | 5.16 |
| S8 | 25.07 | 10.03 | 9.73 | 3.98 | 40.27 | 5.60 | 5.31 |
| Mineral Model | Unit Cell Parameter | |||||
|---|---|---|---|---|---|---|
| a/Å | b/Å | c/Å | α/° | β/° | γ/° | |
| Quartz | 4.91 | 4.91 | 5.40 | 90.00 | 90.00 | 120.00 |
| Albite | 8.12 | 12.76 | 7.16 | 94.22 | 116.80 | 87.71 |
| Calcite | 4.99 | 4.99 | 17.06 | 90.00 | 90.00 | 120.00 |
| Dolomite | 4.81 | 4.81 | 16.01 | 90.00 | 90.00 | 120.00 |
| Illite | 5.22 | 9.00 | 10.32 | 90.26 | 103.05 | 89.97 |
| Chlorite | 5.22 | 9.06 | 28.38 | 90.00 | 93.67 | 90.00 |
| Mineral Model | Skeleton Molecular Weight/ (g/mol) | Accessible Surface Area/ (10−20 m2) | Accessible Pore Volume/ (10−30 m3) | Specific Surface Area/ (m2/g) | Specific Pore Volume/ (10−10 m) |
|---|---|---|---|---|---|
| Illite | 97,024 | 4155 | 87,370 | 257.8 | 21.0 |
| Chlorite | 89,208 | 2018 | 70,033 | 136.2 | 34.7 |
| Quartz | 70,464 | 4030 | 73,099 | 344.3 | 18.1 |
| Albite | 100,608 | 7902 | 136,880 | 472.8 | 17.3 |
| Calcite | 85,520 | 4867 | 88,119 | 342.7 | 18.1 |
| Dolomite | 79,240 | 5550 | 84,738 | 421.7 | 15.3 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Wu, J.; Yang, X.; Huang, S.; Zhao, S.; Zhang, D.; Zhang, J.; Ren, C.; Zhang, C.; Jiang, R.; Liu, D.; et al. Molecular Simulation of Methane Adsorption in Deep Shale Nanopores: Effect of Rock Constituents and Water. Minerals 2023, 13, 756. https://doi.org/10.3390/min13060756
Wu J, Yang X, Huang S, Zhao S, Zhang D, Zhang J, Ren C, Zhang C, Jiang R, Liu D, et al. Molecular Simulation of Methane Adsorption in Deep Shale Nanopores: Effect of Rock Constituents and Water. Minerals. 2023; 13(6):756. https://doi.org/10.3390/min13060756
Chicago/Turabian StyleWu, Jianfa, Xuefeng Yang, Shan Huang, Shengxian Zhao, Deliang Zhang, Jian Zhang, Chunyu Ren, Chenglin Zhang, Rui Jiang, Dongchen Liu, and et al. 2023. "Molecular Simulation of Methane Adsorption in Deep Shale Nanopores: Effect of Rock Constituents and Water" Minerals 13, no. 6: 756. https://doi.org/10.3390/min13060756
APA StyleWu, J., Yang, X., Huang, S., Zhao, S., Zhang, D., Zhang, J., Ren, C., Zhang, C., Jiang, R., Liu, D., Yang, Q., & Huang, L. (2023). Molecular Simulation of Methane Adsorption in Deep Shale Nanopores: Effect of Rock Constituents and Water. Minerals, 13(6), 756. https://doi.org/10.3390/min13060756







