Optimal Investment Strategy for DC Pension Plan with Deposit Loan Spread under the CEV Model
Abstract
:1. Introduction
2. Model Hypothesis
3. Model Formulation
3.1. Wealth Process
3.2. The HJB Equation
4. Verification Theorem
- for any initial value and control process , where
- If, for any initial value , there exists satisfyingthen . Here, the operator is defined as follows:
5. Model Solution
5.1. Legendre Transform
5.2. The Solution under the Logarithmic Utility Function
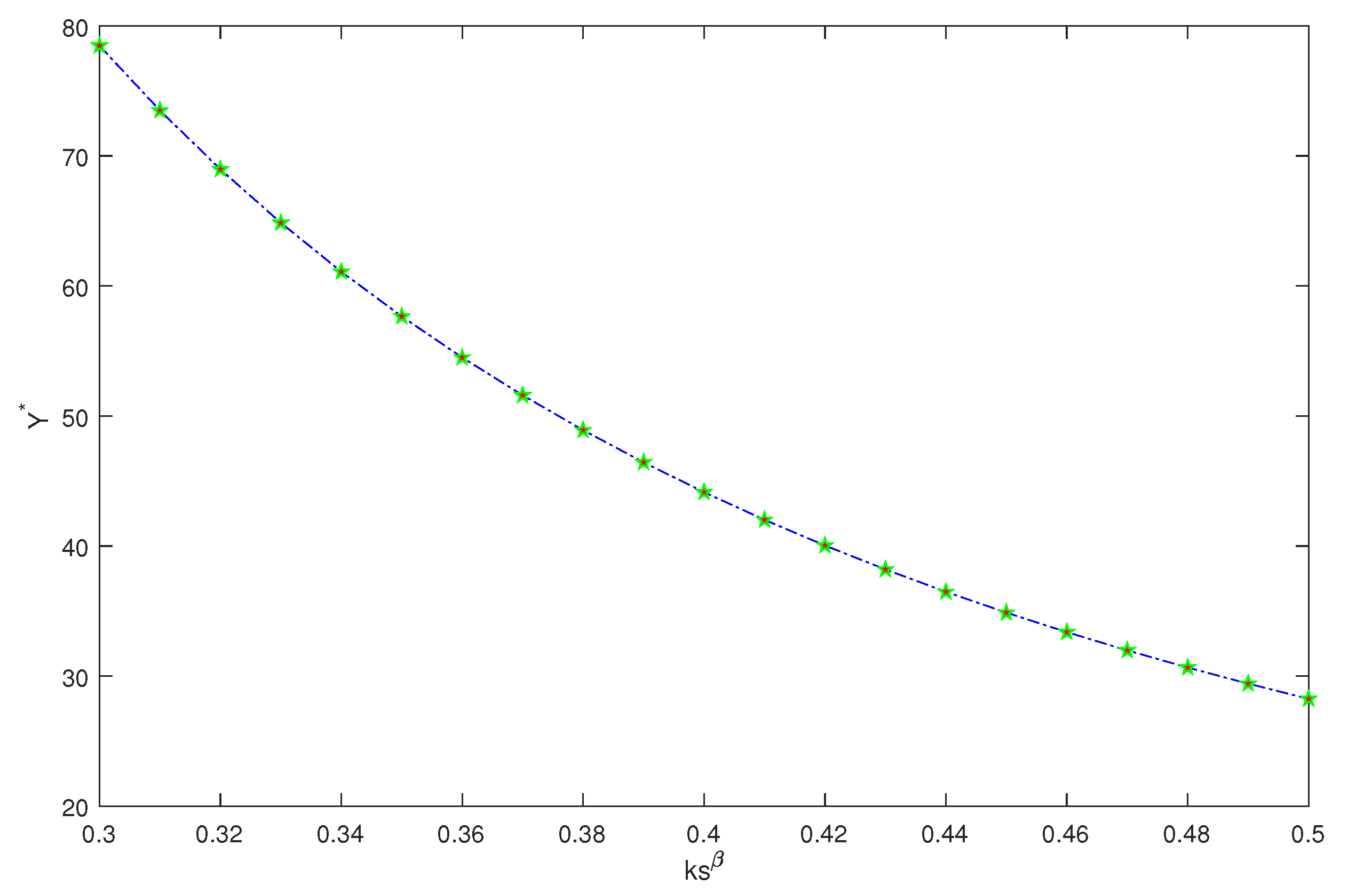
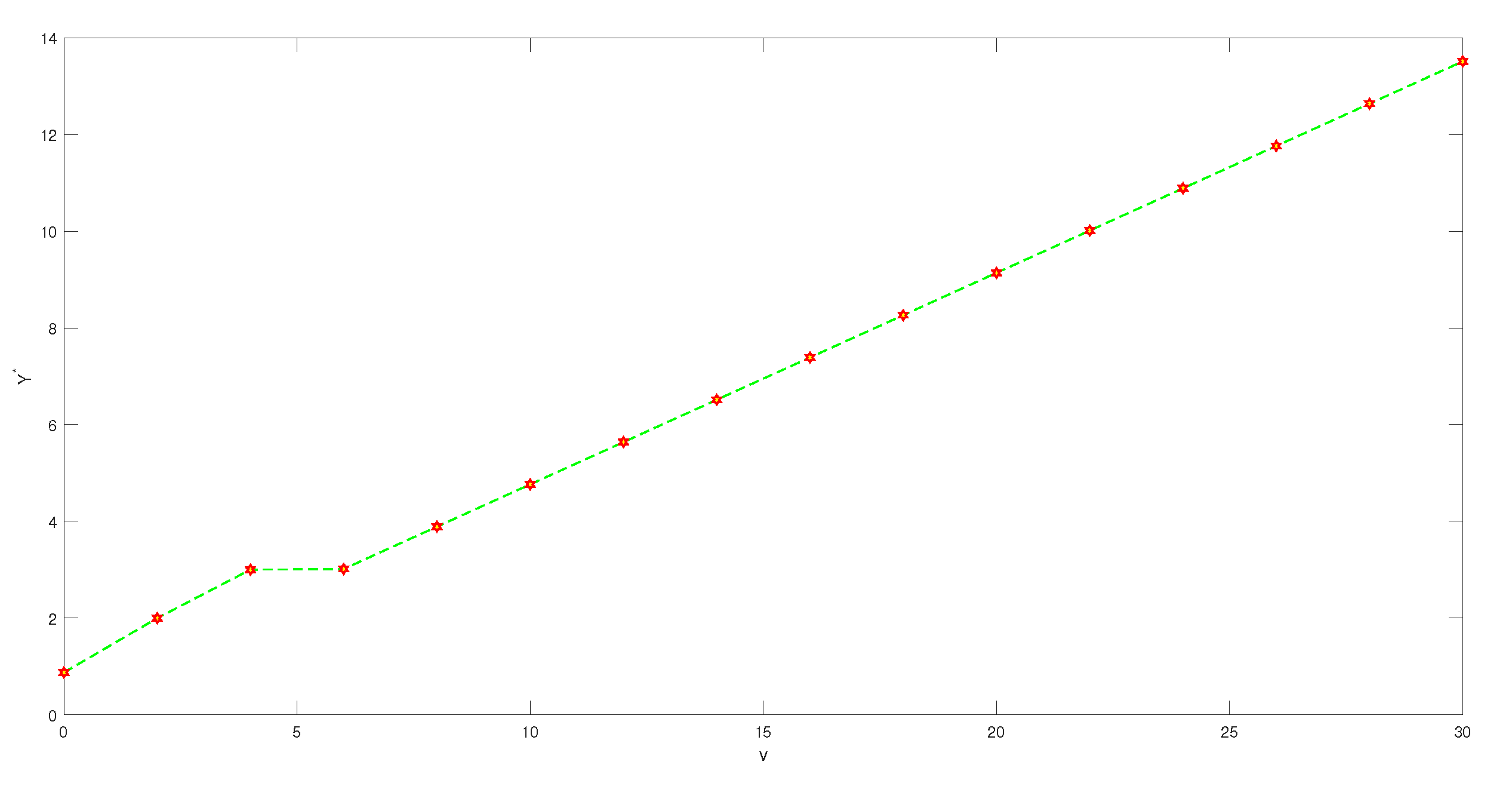
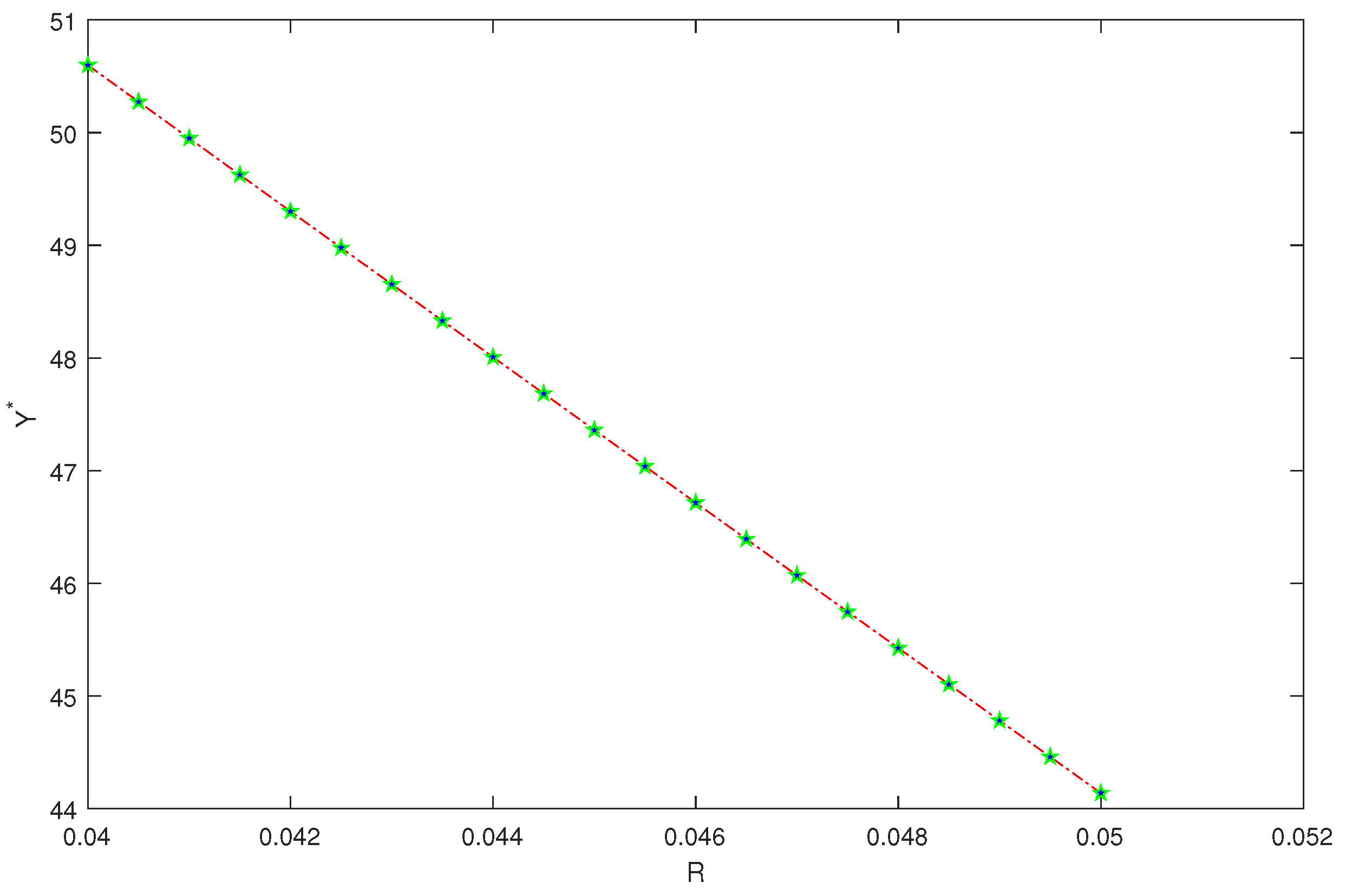
6. Numerical Analysis
7. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Markowitz, H. Portfolio selection. J. Financ. 1952, 7, 77–91. [Google Scholar]
- Boulier, J.F.; Huang, S.; Taillard, G. Optimal management under stochastic interest rates: The case of a protected defined contribution pension fund. Insur. Math. Econ. 2001, 28, 173–189. [Google Scholar] [CrossRef]
- Haberman, S.; Vigna, E. Optimal investment strategies and risk measures in defined contribution pension schemes. Insur. Math. Econ. 2002, 31, 35–69. [Google Scholar] [CrossRef]
- Gerrard, R.; Haberman, S.; Vigna, E. Optimal investment choices post-retirement in a defined contribution pension scheme. Insur. Math. Econ. 2004, 35, 321–342. [Google Scholar] [CrossRef]
- Baev, A.V.; Bondarev, B.V. On the ruin probability of an insurance company dealing in a BS-market. Theory Probab. Math. Stat. 2007, 74, 11–23. [Google Scholar] [CrossRef]
- MacDonald, B.J.; Cairns, A.J.G. The Impact of DC Pension Systems on Population Dynamics. North Am. Actuar. J. 2007, 11, 17–48. [Google Scholar] [CrossRef]
- Byrne, A.; Blake, D.; Mannion, G. Pension plan decisions. Rev. Behav. Financ. 2010, 2, 19–36. [Google Scholar] [CrossRef]
- Gao, J. Stochastic optimal control of dc pension funds. Insur. Math. Econ. 2008, 42, 1159–1164. [Google Scholar] [CrossRef]
- He, L.; Liang, Z. Optimal investment strategy for the DC plan with the return of premiums clauses in a mean-variance framework. Insur. Math. Econ. 2013, 53, 643–649. [Google Scholar] [CrossRef]
- Wu, H.; Zhang, L.; Chen, H. Nash equilibrium strategies for a defined contribution pension management. Insur. Math. Econ. 2015, 62, 202–214. [Google Scholar] [CrossRef]
- Wang, L.; Chen, Z. Nash equilibrium strategy for a DC pension plan with state-dependent risk aversion: A multiperiod mean-variance framework. Discret. Dyn. Nat. Soc. 2018, 2018, 7581231. [Google Scholar] [CrossRef]
- Teng, L.; Ehrhardt, M.; Gnther, M. On the heston model with stochastic correlation. Int. J. Theor. Appl. Financ. 2016, 19, 1–42. [Google Scholar] [CrossRef]
- Guan, G.; Liang, Z. Optimal management of dc pension plan under loss aversion and value-at-risk constraints. Insur. Math. Econ. 2016, 69, 224–237. [Google Scholar] [CrossRef]
- Sun, J.; Li, Z.; Li, Y. The optimal investment strategy for DC pension plan with a dynamic investment target. Syst. Eng. Theory Pract. 2017, 37, 2209–2221. [Google Scholar]
- Bian, L.; Li, Z.; Yao, H. Pre-commitment and equilibrium investment strategies for the DC pension plan with regime switching and a return of premiums clause. Insur. Math. Econ. 2018, 8, 78–94. [Google Scholar] [CrossRef]
- Chen, Z.P.; Wang, L.Y.; Chen, P. Continuous-time mean-variance optimization for defined contribution pension funds with regime-switching. Int. J. Theor. Applied Financ. (IJTAF) 2019, 22, 1–33. [Google Scholar]
- Blake, C.; Sass, J. Finite-horizon optimal investment with transaction costs: Construction of the optimal strategies. Financ. Stochastics Vol. 2019, 23, 861–888. [Google Scholar] [CrossRef]
- Dong, Y.; Zheng, H. Optimal investment of DC pension plan under short-selling constraints and portfolio insurance. Insur. Math. Econ. 2019, 85, 47–59. [Google Scholar] [CrossRef]
- Dong, Y.; Zheng, H. Optimal investment with s-shaped utility and trading and value at risk constraints: An application to defined contribution pension plan. Eur. J. Oper. Res. 2020, 281, 341–356. [Google Scholar] [CrossRef]
- Wang, P.; Shen, Y.; Zhang, L.; Kang, Y. Equilibrium investment strategy for a DC pension plan with learning about stock return predictability. Insur. Math. Econ. 2021, 100, 384–407. [Google Scholar] [CrossRef]
- Guan, G.; Liang, Z. Optimal management of DC pension plan in a stochastic interest rate and stochastic volatility framework. Insur. Math. Econ. 2014, 57, 58–66. [Google Scholar] [CrossRef]
- Wang, P.; Li, Z.F. Robust optimal investment strategy for an aam of DC pension plans with stochastic interest rate and stochastic volatility. Insur. Math. Econ. 2018, 80, 67–83. [Google Scholar] [CrossRef]
- Zhang, L.; Li, D.; Lai, Y. Equilibrium investment strategy for a defined contribution pension plan under stochastic interest rate and stochastic volatility. J. Comput. Appl. Math. 2020, 368, 1–21. [Google Scholar] [CrossRef]
- Battocchio, P.; Menoncin, F. Optimal pension management in a stochastic framework. Insur. Math. Econ. 2004, 34, 79–95. [Google Scholar] [CrossRef]
- Han, N.W.; Hung, M.W. Optimal asset allocation for dc pension plans under inflation. Insur. Math. Econ. 2012, 51, 172–181. [Google Scholar] [CrossRef]
- Nkeki, C.I. Mean-variance portfolio selection problem with stochastic salary for a defined contribution pension scheme: A stochastic linear-quadratic framework. Stat. Optim. Inf. Comput. 2013, 1, 62–81. [Google Scholar] [CrossRef]
- Tang, M.L.; Chen, S.N.; Lai, G.C.; Wu, T.P. Asset allocation for a DC pension fund under stochastic interest rates and inflation-protected guarantee. Insur. Math. Econ. 2018, 78, 87–104. [Google Scholar] [CrossRef]
- Guambe, C.; Kufakunesu, R.; Zyl, G.; Beyers, C. Optimal asset allocation for a DC plan with partial information under inflation and mortality risks. Commun. Stat. Theory Methods 2019, 50, 2048–2061. [Google Scholar] [CrossRef]
- Wang, P.; Li, Z.; Sun, J. Robust portfolio choice for a DC pension plan with inflation risk and mean-reverting risk premium under ambiguity. Optimization 2021, 70, 191–224. [Google Scholar] [CrossRef]
- Peng, X.; Chen, F. Deterministic investment strategy in a DC pension plan with inflation risk under mean-variance criterion. Probab. Eng. Informational Sci. 2022, 36, 201–216. [Google Scholar] [CrossRef]
- Mudzimbabwe, W. A simple numerical solution for an optimal investment strategy for a DC pension plan in a jump diffusion model. J. Comput. Appl. Math. 2019, 360, 55–61. [Google Scholar] [CrossRef]
- Xu, W.; Gao, J. An Optimal Portfolio Problem of DC Pension with Input-Delay and Jump-Diffusion Process. Math. Probl. Eng. 2020, 2020, 4343629. [Google Scholar] [CrossRef]
- Xiao, J.W.; Hong, Z.; Qin, C.L. The constant elasticity of variance (cev) model and the legendre transform-dual solution for annuity contracts. Insur. Math. Econ. 2007, 40, 302–310. [Google Scholar] [CrossRef]
- Gu, M.; Yang, Y.; Li, S.; Zhang, J. Constant elasticity of variance model for proportional reinsurance and investment strategies. Insur. Math. Econ. 2010, 46, 580–587. [Google Scholar] [CrossRef]
- Zhang, C.B.; Rong, X.M.; Zhao, H. Optimal investment for dc pension with stochastic salary under a cev model. Discret. Dyn. Nat. Soc. 2013, 28, 187–203. [Google Scholar]
- Li, D.; Rong, X.; Zhao, H.; Yi, B. Equilibrium investment strategy for DC pension plan with default risk and return of premiums clauses under CEV model. Insur. Math. Econ. 2017, 72, 6–20. [Google Scholar] [CrossRef]
- Chen, H.J.; Yin, Z.; Xie, T.H. Determining equivalent administrative charges for defined contribution pension plans under CEV model. Math. Probl. Eng. 2018, 2018, 6278353. [Google Scholar] [CrossRef]
- Ma, J.; Lu, Z.; Li, W. Least-squares monte-carlo methods for optimal stopping investment under CEV models. quantitative finance. Quant. Financ. 2020, 20, 1199–1211. [Google Scholar] [CrossRef]
- He, Y.; Chen, P. Optimal investment strategy under the cev model with stochastic interest rate. Math. Probl. Eng. 2020, 2020, 7489174. [Google Scholar] [CrossRef]
- He, Y.; Xiang, K.; Chen, P. Optimal investment strategy with constant absolute risk aversion utility under an extended CEV model. Optimazation 2021, 2020, 1–31. [Google Scholar] [CrossRef]
- Yong, X.; Sun, X.; Gao, J. Symmetry-based optimal portfolio for a DC pension plan under a CEV model with power utility. Nonlinear Dyn. 2021, 103, 1775–1783. [Google Scholar] [CrossRef]
- Soner, H.M. Controlled Markov processes, viscosity solutions and applications to mathematical finance. In Viscosity Solutions and Applications; Dolcetta, I.C., Lions, P.L., Eds.; Springer: Berlin, Germany, 1997; Volume 1660, pp. 134–185. [Google Scholar]
- Jonsson, M.; Sircar, R. Optimal investment problems and volatility homogenization approximations. In Modern Methods in Scientific Computing and Applications; Bourlioux, A., Gander, M.l., Eds.; Springer: Dordrecht, The Netherlands, 2002; pp. 255–281. [Google Scholar]

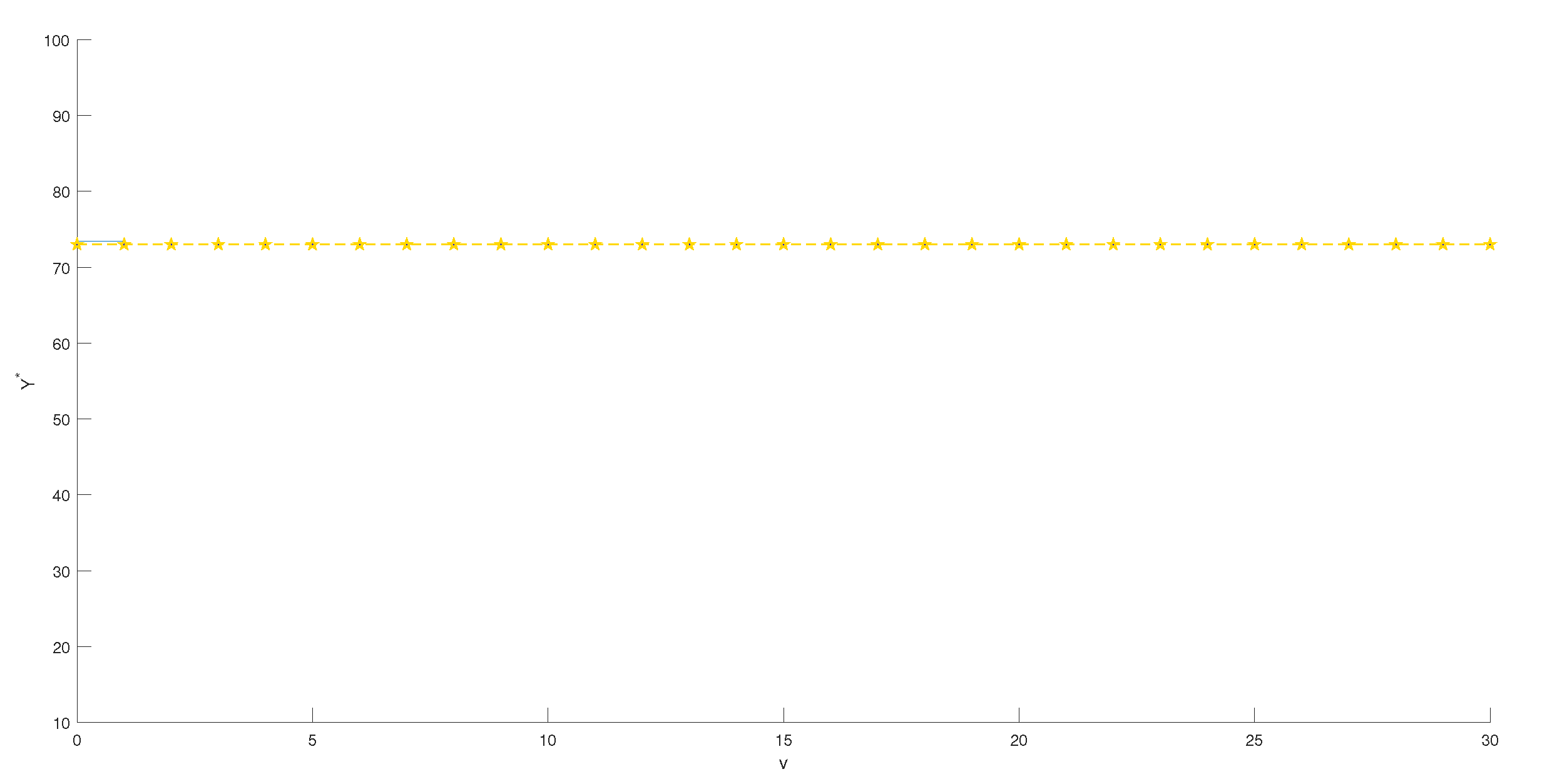

Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Wang, Y.; Xu, X.; Zhang, J. Optimal Investment Strategy for DC Pension Plan with Deposit Loan Spread under the CEV Model. Axioms 2022, 11, 382. https://doi.org/10.3390/axioms11080382
Wang Y, Xu X, Zhang J. Optimal Investment Strategy for DC Pension Plan with Deposit Loan Spread under the CEV Model. Axioms. 2022; 11(8):382. https://doi.org/10.3390/axioms11080382
Chicago/Turabian StyleWang, Yang, Xiao Xu, and Jizhou Zhang. 2022. "Optimal Investment Strategy for DC Pension Plan with Deposit Loan Spread under the CEV Model" Axioms 11, no. 8: 382. https://doi.org/10.3390/axioms11080382





