Some Generalized Results on Grey Number Operations Based on Liu-Lin Axioms of Greyness Degree and Information Content
Abstract
:1. Introduction
2. Liu–Lin Axioms on Greyness Degree
3. Greyness Degree Results of Mathematical Operations
4. Liu–Lin Axioms on Information Content
5. Information Content Results of Mathematical Operations
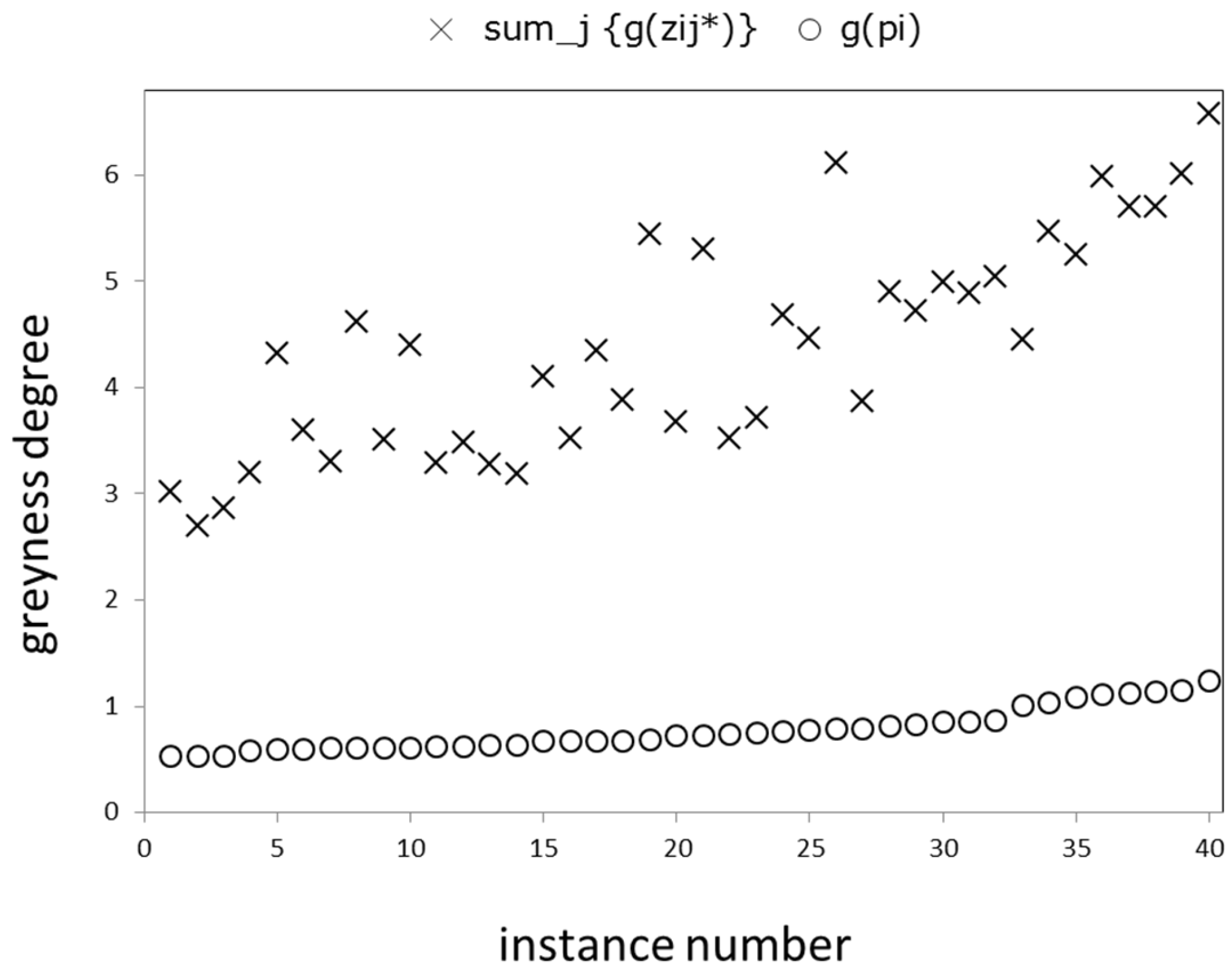
6. Illustration of Greyness Degree Results: A Simple Monte Carlo Simulation
6.1. A Multiple-Attribute Decision-Making Case as a Test Bed
6.2. A Monte Carlo Simulation on the Decision Matrix
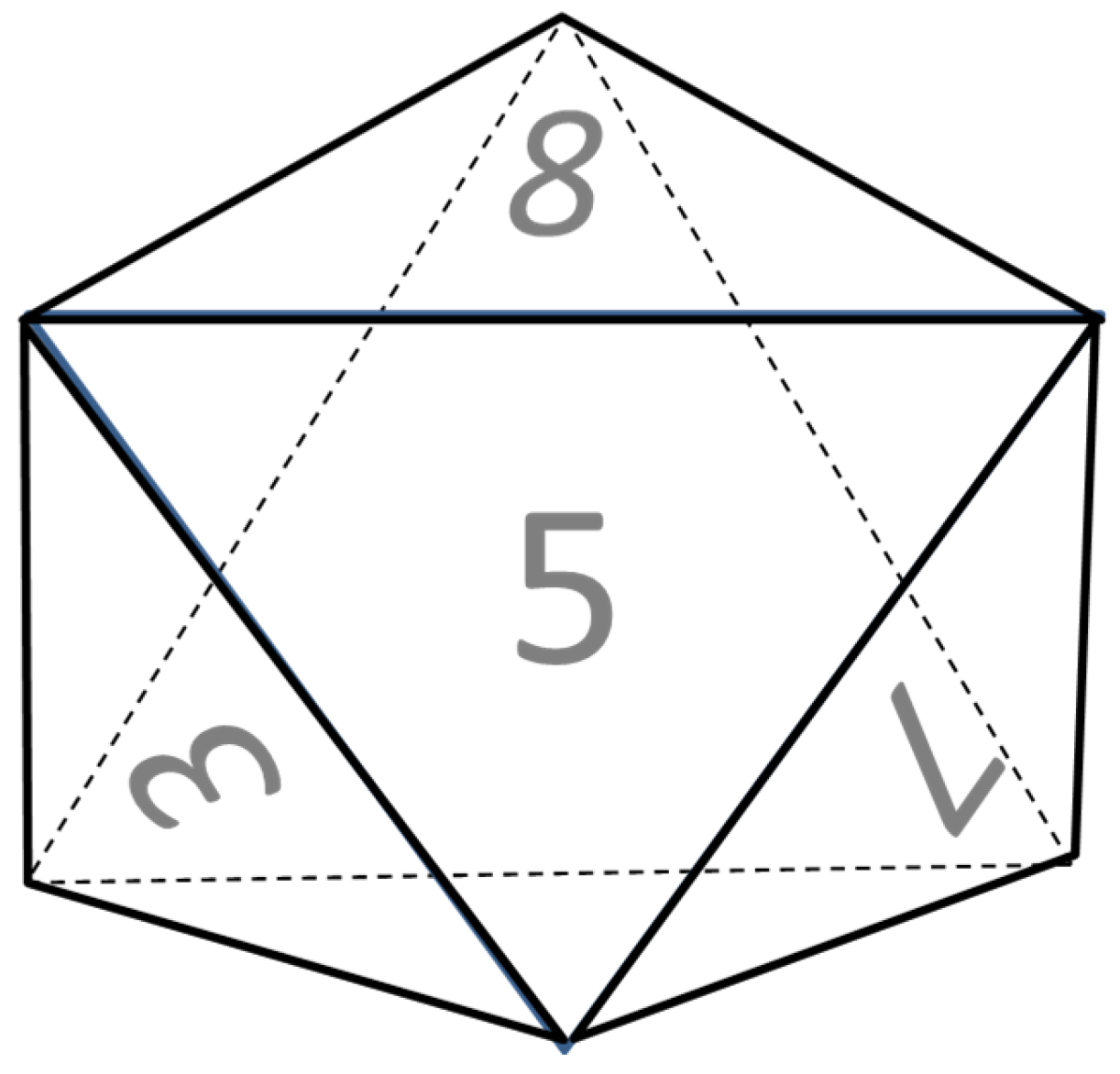
7. Illustration of Information Content Results: A Dice-Rolling Experiment
8. Conclusions and Future Research Directions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Deng, J. Control problems of grey systems. Syst. Control Lett. 1982, 1, 288–294. [Google Scholar]
- Deng, J. Grey systems control. J. Huazhong Univ. Sci. Technol. 1982, 3, 9–18. [Google Scholar]
- Deng, J. The theory and method of socioeconomic grey systems. Soc. Sci. China 1984, 6, 47–60. [Google Scholar]
- Deng, J. Grey Systems: Society, Economy; National Defence Press: Beijing, China, 1985. [Google Scholar]
- Deng, J. The Essential Methods of Grey Systems; Huazhong University of Science and Technology Press: Wuhan, China, 1987. [Google Scholar]
- Liu, S.; Forrest, J. The mysteries for continual growth of grey system theory. In Proceedings of the 2007 IEEE International Conference on Systems, Man and Cybernetics, Montréal, QC, Canada, 7–10 October 2007; pp. 2155–2159. [Google Scholar]
- Li, Q.; Liu, S.; Forrest, J.Y.-L. Fundamental definitions and calculation rules of grey mathematics: A review work. J. Syst. Eng. Electron. 2015, 26, 1254–1267. [Google Scholar] [CrossRef]
- Javanmardi, E.; Liu, S.; Xie, N. Exploring grey systems theory-based methods and applications in sustainability studies: A systematic review approach. Sustainability 2020, 12, 4437. [Google Scholar] [CrossRef]
- Camelia, D. Grey systems theory in economics–a historical applications review. Grey Syst. Theory Appl. 2015, 5, 263–276. [Google Scholar] [CrossRef]
- Islam, R.; Biswal, M.P.; Alam, S.S. Preference programming and inconsistent interval judgments. Eur. J. Oper. Res. 1997, 97, 53–62. [Google Scholar] [CrossRef]
- Lee, Y.-M. Development of the grey analytic hierarchy process: A preliminary study. J. Chin. Inst. Environ. Eng. 2002, 12, 175–183. [Google Scholar]
- Liu, S. A new definition on degree of greyness of grey numbers with several theorems. In Proceedings of the 2005 IEEE International Conference on Networking, Sensing and Control, Tucson, AZ, USA, 19–22 March 2005; pp. 90–93. [Google Scholar]
- Yang, Y. Extended grey numbers and their operations. In Proceedings of the 2007 IEEE International Conference on Systems, Man and Cybernetics, Montréal, QC, Canada, 7–10 October 2007; p. 2181. [Google Scholar]
- Despotis, D.K.; Derpanis, D. A min-max goal programming approach to priority derivation in AHP with interval judgments. Int. J. Inf. Technol. Decis. Mak. 2007, 10, 21. [Google Scholar]
- Jiang, C.; Han, X.; Guan, F.J.; Li, Y.H. An uncertain structural optimization method based on nonlinear interval number programming and interval analysis method. Eng. Struct. 2007, 29, 3168–3177. [Google Scholar] [CrossRef]
- Çakır, O. Post-optimality analysis of priority vectors derived from interval comparison matrices by lexicographic goal programming. Appl. Math. Comput. 2008, 204, 261–268. [Google Scholar]
- Yang, Y.; Liu, S. Kernels of grey numbers and their operations. In Proceedings of the 2008 IEEE International Conference on Fuzzy Systems, Hong Kong, China, 1–6 June 2008; 826-831. [Google Scholar]
- Jiang, C.; Han, X.; Liu, G.P. Uncertain optimization of composite laminated plates using a nonlinear interval number programming method. Comput. Struct. 2008, 86, 1696–1703. [Google Scholar] [CrossRef]
- Çakır, O. The grey extent analysis. Kybernetes 2008, 37, 997–1015. [Google Scholar] [CrossRef]
- Zhang, H.; Zhang, W.; Mei, C. Entropy of interval-valued fuzzy sets based on distance and its relationship with similarity measure. Knowl.-Based Syst. 2009, 22, 449–454. [Google Scholar] [CrossRef]
- Sun, Y.; Huang, G.H.; Li, Y.P.; Xu, Y.; Cao, M.F. An interval fuzzy robust nonlinear program for the planning of municipal solid waste management systems under uncertainty. Eng. Optim. 2009, 41, 989–1016. [Google Scholar] [CrossRef]
- Wu, C.; Huang, G.; Yan, X.; Cai, Y.; Li, Y.; Lv, N. An inexact optimization model for evacuation planning. Kybernetes 2009, 38, 1676–1683. [Google Scholar] [CrossRef]
- Salmeron, J.L. Modelling grey uncertainty with fuzzy grey cognitive maps. Expert Syst. Appl. 2010, 37, 7581–7588. [Google Scholar] [CrossRef]
- Suprajitno, H.; Mohd, I.B. Linear programming with interval arithmetic. Int. J. Contemp. Math. Sci. 2010, 5, 323–332. [Google Scholar]
- Xie, N.-M.; Liu, S. Novel methods on comparing grey numbers. Appl. Math. Model. 2010, 34, 415–423. [Google Scholar] [CrossRef]
- Guo, R.; Guo, D.; Cui, Y.H. An uncertain regression model. Grey Syst. Theory Appl. 2011, 1, 202–215. [Google Scholar] [CrossRef]
- Liu, S.; Xie, N.-M.; Forrest, J. Novel models of grey relational analysis based on visual angle of similarity and nearness. Grey Syst. Theory Appl. 2011, 1, 8–18. [Google Scholar] [CrossRef]
- Yang, Y.; Liu, S. Reliability of operations of grey numbers using kernels. Grey Syst. Theory Appl. 2011, 1, 57–71. [Google Scholar] [CrossRef]
- Çakır, O. A compensatory model for computing with words under discrete labels and incomplete information. Knowl.-Based Syst. 2012, 27, 29–37. [Google Scholar] [CrossRef]
- Wang, Z. Evaluation of the teachers’ occupation ability based on grey system theory. In Proceedings of the IEEE 9th International Conference on Fuzzy Systems and Knowledge Discovery, Sichuan, China, 29–31 May 2012; pp. 565–568. [Google Scholar]
- Wang, J.; Liu, S. Efficiency measures in DEA with grey interval data under the hypotheses of data consistency. Grey Syst. Theory Appl. 2012, 2, 63–69. [Google Scholar] [CrossRef]
- Ruan, A.; Fang, Z. Grey potential degrees and its application in grey game. J. Grey Syst. 2013, 25, 19–35. [Google Scholar]
- Çakır, O. On visualizing the number comparison scheme in grey extent analysis. Kybernetes 2013, 42, 94–105. [Google Scholar] [CrossRef]
- Allahdadi, M. New comparison of interval numbers. Int. J. Appl. Math. 2014, 27, 567–571. [Google Scholar]
- Li, Q.X.; Lin, Y. A briefing to grey systems theory. J. Syst. Sci. Inf. 2014, 2, 178–192. [Google Scholar]
- Liu, Y.; Forrest, J.; Xie, N. Ranking grey numbers based on dominance grey degrees. J. Syst. Eng. Electron. 2014, 25, 618–626. [Google Scholar] [CrossRef]
- Bhattacharyya, R. A grey theory based multiple attribute approach for R&D project portfolio selection. Fuzzy Inf. Eng. 2015, 7, 211–225. [Google Scholar]
- Zakeri, S.; Keramati, M.A. Systematic combination of fuzzy and grey numbers for supplier selection problem. Grey Syst. Theory Appl. 2015, 5, 313–343. [Google Scholar] [CrossRef]
- Yan, S.; Liu, S.; Liu, J.; Wu, L. Dynamic grey target decision making method with grey numbers based on existing state and future development trend of alternatives. J. Intell. Fuzzy Syst. 2015, 28, 2159–2168. [Google Scholar] [CrossRef]
- Nasseri, S.H.; Yazdani, A.; Darvishi, D. A primal simplex algorithm for solving linear programming problem with grey cost coefficients. J. New Res. Math. 2016, 1, 121–141. [Google Scholar]
- Ye, Y.; Yao, N.; Wang, Q.; Wang, Q. A method of ranking interval numbers based on degrees for multiple attribute decision making. J. Intell. Fuzzy Syst. 2016, 30, 211–221. [Google Scholar] [CrossRef]
- Yan, S.; Liu, S.; Liu, X. Dynamic grey target decision making method with three-parameter grey numbers. Grey Syst. Theory Appl. 2016, 6, 169–179. [Google Scholar] [CrossRef]
- Guo, S.; Liu, S.; Fang, Z. Algorithm rules of interval grey numbers based on different kernel and the degree of greyness of grey numbers. Grey Syst. Theory Appl. 2017, 7, 168–178. [Google Scholar] [CrossRef]
- Nasseri, S.H.; Darvishi, D.; Yazdani, A. A new approach for solving grey assignment problems. Control Optim. Appl. Math. 2017, 2, 15–28. [Google Scholar]
- Stanujkic, D.; Zavadskas, E.K.; Liu, S.; Karabasevic, D.; Popovic, G. Improved OCRA method based on the use of interval grey numbers. J. Grey Syst. 2017, 29, 49–60. [Google Scholar]
- Darvishi, D.; Liu, S.; Nasseri, S.H. A new approach in animal diet by grey system theory. Grey Syst. Theory Appl. 2018, 8, 167–180. [Google Scholar]
- Li, P.; Wei, C. A new two-stage grey evaluation decision-making method for interval grey numbers. Kybernetes 2018, 47, 801–815. [Google Scholar] [CrossRef]
- Mahmoudi, A.; Feylizadeh, M.R. A grey mathematical model for crashing of projects by considering time, cost, quality, risk and law of diminishing returns. Grey Syst. Theory Appl. 2018, 8, 272–294. [Google Scholar] [CrossRef]
- Darvishi, D.; Forrest, J.; Liu, S. A comparative analysis of grey ranking approaches. Grey Syst. Theory Appl. 2019, 9, 472–487. [Google Scholar] [CrossRef]
- Javed, S.A.; Liu, S.; Mahmoudi, A.; Nawaz, M. Patients’ satisfaction and public and private sectors’ health care service quality in Pakistan: Application of grey decision analysis approaches. Int. J. Health Plan. Manag. 2019, 34, 168–182. [Google Scholar] [CrossRef] [PubMed]
- Mahmoudi, A.; Liu, S.; Javed, S.A.; Abbasi, M. A novel method for solving linear programming with grey parameters. J. Intell. Fuzzy Syst. 2019, 36, 161–172. [Google Scholar] [CrossRef]
- Chen, N.; Xie, N. Uncertainty representation and information measurement of grey numbers. Grey Syst. Theory Appl. 2020, 10, 495–512. [Google Scholar] [CrossRef]
- Ye, J.; Dang, Y.; Yang, Y. Forecasting the multifactorial interval grey number sequences using grey relational model and GM (1, N) model based on effective information transformation. Soft Comput. 2020, 24, 5255–5269. [Google Scholar] [CrossRef]
- Ulutaş, A.; Popovic, G.; Stanujkic, D.; Karabasevic, D.; Zavadskas, E.K.; Turskis, Z. A new hybrid MCDM model for personnel selection based on a novel grey PIPRECIA and grey OCRA methods. Mathematics 2020, 8, 1698. [Google Scholar] [CrossRef]
- Esangbedo, M.O.; Bai, S.; Mirjalili, S.; Wang, Z. Evaluation of human resource information systems using grey ordinal pairwise comparison MCDM methods. Expert Syst. Appl. 2021, 182, 115151. [Google Scholar] [CrossRef]
- Mahmoudi, A.; Deng, X.; Javed, S.A.; Zhang, N. Sustainable supplier selection in megaprojects: Grey ordinal priority approach. Bus. Strateg. Environ. 2021, 30, 318–339. [Google Scholar] [CrossRef]
- Stanujkić, D.; Karabašević, D.; Popović, G.; Stanimirović, P.S.; Saračević, M.; Smarandache, F.; Katsikis, V.N.; Ulutaş, A. A new grey approach for using SWARA and PIPRECIA methods in a group decision-making environment. Mathematics 2021, 9, 1554. [Google Scholar] [CrossRef]
- Toan, P.N.; Dang, T.-T.; Hong, L.T.T. E-learning platform assessment and selection using two-stage multi-criteria decision-making approach with grey theory: A case study in Vietnam. Mathematics 2021, 9, 3136. [Google Scholar] [CrossRef]
- Zanon, L.G.; Marcelloni, F.; Gerolamo, M.C.; Carpinetti, L.C.R. Exploring the relations between supply chain performance and organizational culture: A fuzzy grey group decision model. Int. J. Prod. Econ. 2021, 233, 108023. [Google Scholar] [CrossRef]
- Wu, W. A revised grey relational analysis method for multicriteria group decision-making with expected utility theory for oil spill emergency management. Math. Probl. Eng. 2021, 2021, 6682332. [Google Scholar] [CrossRef]
- Xiong, P.; Chen, S.; Yan, S. Time-delay nonlinear model based on interval grey number and its application. J. Syst. Eng. Electron. 2022, 33, 370–380. [Google Scholar] [CrossRef]
- Wang, L.; Liu, J. Dynamic uncertainty quantification and risk prediction based on the grey mathematics and outcrossing theory. Appl. Sci. 2022, 12, 5389. [Google Scholar] [CrossRef]
- Gürler, İ.; Çakır, O.; Gündüzyeli, B. Distance-based decision making, consensus building, and preference aggregation systems: A note on the scale constraints. Systems 2022, 10, 112. [Google Scholar] [CrossRef]
- Liu, S.; Fang, Z.; Forrest, J. On algorithm rules of interval grey numbers based on the ‘kernel’ and the degree of greyness of grey numbers. In Proceedings of the 2010 IEEE International Conference on Systems, Man and Cybernetics, İstanbul, Turkey, 10–13 October 2010; pp. 756–760. [Google Scholar]
- Liu, S.; Lin, Y. Grey Information. Theory and Practical Applications; Springer: London, UK, 2006. [Google Scholar]


| Source | Features | Methods and Theories | Cases and Illustrations |
|---|---|---|---|
| [10] | Inconsistent grey judgments | Lexicographic goal programming | Numerical examples |
| [11] | Grey decision making | Preference programming | Project selection |
| [12] | Greyness degree | Theorem proving | Proofs |
| [13] | Extended grey numbers | Grey number operations | Numerical examples |
| [14] | Grey decision making | Goal programming | Numerical example |
| [15] | Uncertain structural optimization | Nonlinear programming | Optimization of an automobile frame |
| [16] | Post-optimality analysis | Lexicographic goal programming | Numerical examples |
| [17] | Kernels of grey numbers | Grey number operations | Numerical examples |
| [18] | Nonlinear grey programming | Hybrid algorithms | Optimization of composite laminated plate |
| [19] | Grey extent analysis | Probability theory | Supplier selection |
| [20] | Entropy of grey numbers | Similarity measure | Numerical example |
| [21] | Grey robust program | Nonlinear programming | Municipal solid waste management |
| [22] | Grey linear program | Model decomposition | Evacuation planning |
| [23] | Grey cognitive map | Cognitive mapping | Analyzing IT project risk |
| [24] | Grey linear programming | Modified simplex method | Numerical example |
| [25] | Grey number comparison | Probability theory | Numerical examples |
| [26] | Uncertain regression | Multivariate analysis | Prediction model examples |
| [27] | Similarity and nearness | Grey relational analysis | Numerical example |
| [28] | Kernels of grey numbers | Grey number operations | Numerical examples |
| [29] | Discrete linguistic labels | Compensatory programming | Budget allocation |
| [30] | Grey target decision making | Grey relational analysis | Evaluation of occupational ability |
| [31] | Data consistency | Data envelopment analysis | Numerical example |
| [32] | Grey potential degree | Game theory | Numerical examples |
| [33] | Visualization | Probability theory | Numerical examples |
| [34] | Grey number comparison | Partial orders | Proofs |
| [35] | Grey information axioms | Grey number operations | Proofs |
| [36] | Dominance grey degree | Ranking | Numerical example |
| [37] | Linguistic labels | Grey possibility degree | R&D project evaluation |
| [38] | Linguistic labels | Grey relational analysis | Supplier selection |
| [39] | Dynamic grey target | Grey relational analysis | Numerical example |
| [40] | Grey linear program | Primal simplex algorithm | Numerical example |
| [41] | Visualization | Ranking | Numerical example |
| [42] | Grey target decision making | Dynamic decision making | Numerical example |
| [43] | Greyness degree | Ranking | Numerical example |
| [44] | Grey linear assignment | Hungarian algorithm | Numerical example |
| [45] | Operational competitiveness rating | Ranking | Numerical examples |
| [46] | Diet problem | Grey linear programming | Animal nutrition case |
| [47] | Clustering of grey numbers | Possibility definition | Numerical example |
| [48] | Project management | Sensitivity analysis | Numerical example |
| [49] | Comparative analysis | Order relations | Numerical example |
| [50] | Empirical data | Grey relational analysis | Healthcare sector case |
| [51] | Staged solution procedure | Grey linear programming | Comparative analysis |
| [52] | Probability function of a grey number | Information representation | Numerical example |
| [53] | Information transformation | Grey prediction | Traffic congestion analysis |
| [54] | Multiple-criteria decision making | Grey arithmetic | Production manager selection |
| [55] | Multiple-criteria decision making | Grey arithmetic | Comparative analysis |
| [56] | Ordinal priority | Membership functions | Supplier selection |
| [57] | Group decision making | Grey arithmetic | Evaluating travel websites |
| [58] | Multiple-criteria decision making | Hybrid grey decision model | E-learning platform assessment |
| [59] | Group decision making | Grey clustering | Two case studies |
| [60] | Expected utilities | Grey relational analysis | Emergency management |
| [61] | Time-delay grey model | Possibility theory | Simulation |
| [62] | Uncertainty quantification | Possibility index | Safety prediction of laminated plates |
| [63] | Consensus building | Distance-metric optimization | Case study, computational experiment |
| Linguistic Label | Underlying Grey Number | Test Number Interval |
|---|---|---|
| Unsatisfactory performance | 0.0000–0.1666 | |
| Poor performance | 0.1667–0.3333 | |
| Mediocre performance | 0.3334–0.5000 | |
| Acceptable performance | 0.5001–0.6667 | |
| Good performance | 0.6668–0.8334 | |
| Exceptional performance | 0.8335–1.0000 |
| Instance | Underlying SAW Step | Numbers of Instances |
|---|---|---|
| Summation | Step 1 | 50 |
| Normalization | Step 2 | 200 |
| Convex combination | Step 3 | 40 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Gürler, İ.; Çakır, O.; Gündüzyeli, B. Some Generalized Results on Grey Number Operations Based on Liu-Lin Axioms of Greyness Degree and Information Content. Axioms 2022, 11, 424. https://doi.org/10.3390/axioms11090424
Gürler İ, Çakır O, Gündüzyeli B. Some Generalized Results on Grey Number Operations Based on Liu-Lin Axioms of Greyness Degree and Information Content. Axioms. 2022; 11(9):424. https://doi.org/10.3390/axioms11090424
Chicago/Turabian StyleGürler, İbrahim, Ozan Çakır, and Bora Gündüzyeli. 2022. "Some Generalized Results on Grey Number Operations Based on Liu-Lin Axioms of Greyness Degree and Information Content" Axioms 11, no. 9: 424. https://doi.org/10.3390/axioms11090424
APA StyleGürler, İ., Çakır, O., & Gündüzyeli, B. (2022). Some Generalized Results on Grey Number Operations Based on Liu-Lin Axioms of Greyness Degree and Information Content. Axioms, 11(9), 424. https://doi.org/10.3390/axioms11090424






