A Nonlinear System of Differential Equations in Supercritical Flow Spread Problem and Its Solution Technique
Abstract
:1. Introduction
- the development of up-to-date application software packages making it easier to use mathematical methods when solving practical tasks in hydraulics of open water flows;
- new approaches in finding the solution of hydraulic structure calculation problems;
- the introduction of new normative indices, requiring detailing of practical HS calculations at large Froude numbers.
2. Research Methods
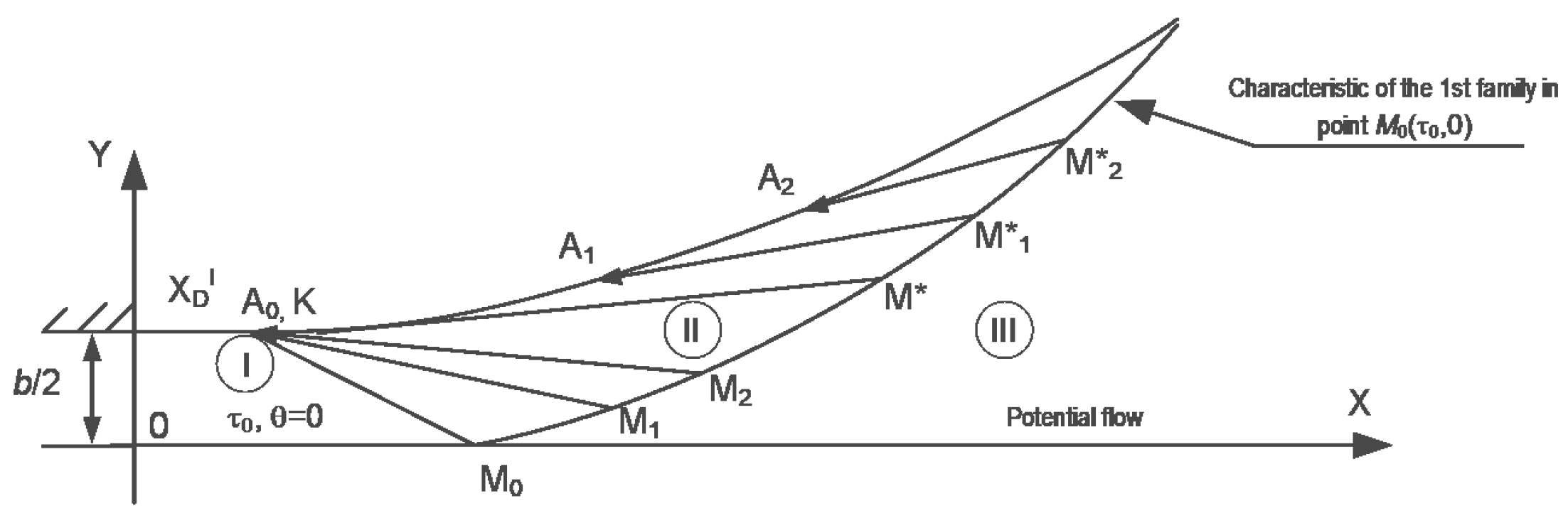
2.1. System of Equations of Motion for Two-Dimensional in Plan Supercriticals Potential, Stationary, Streams Open Water
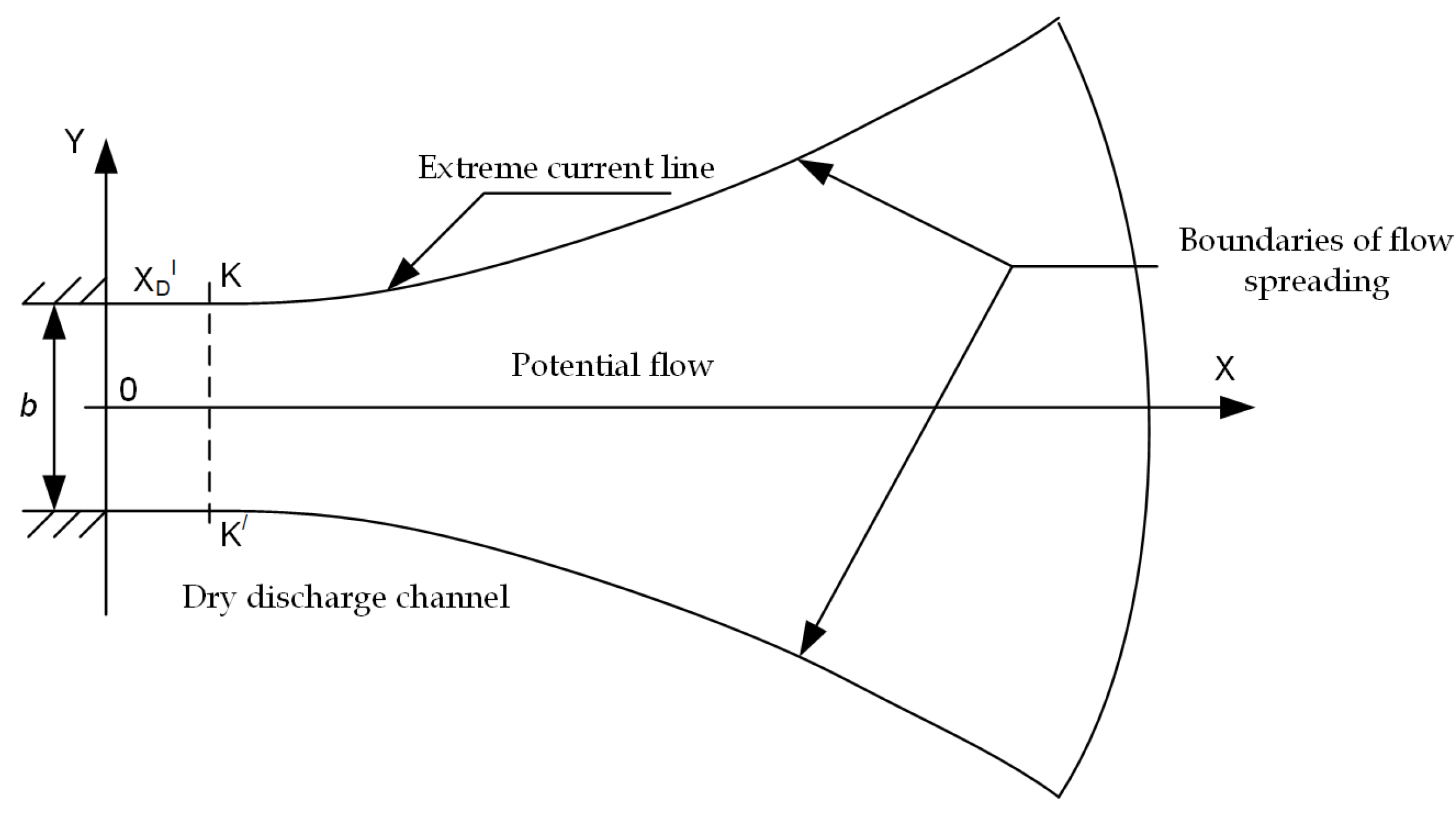
2.2. On the Boundary Problem of the Free Supercritical Flow behind an Unpressurised Culvert When It Spreads into a Wide Discharge Channel
2.3. Description of the Method for Solving the Problem in the Velocity Hodograph Plane
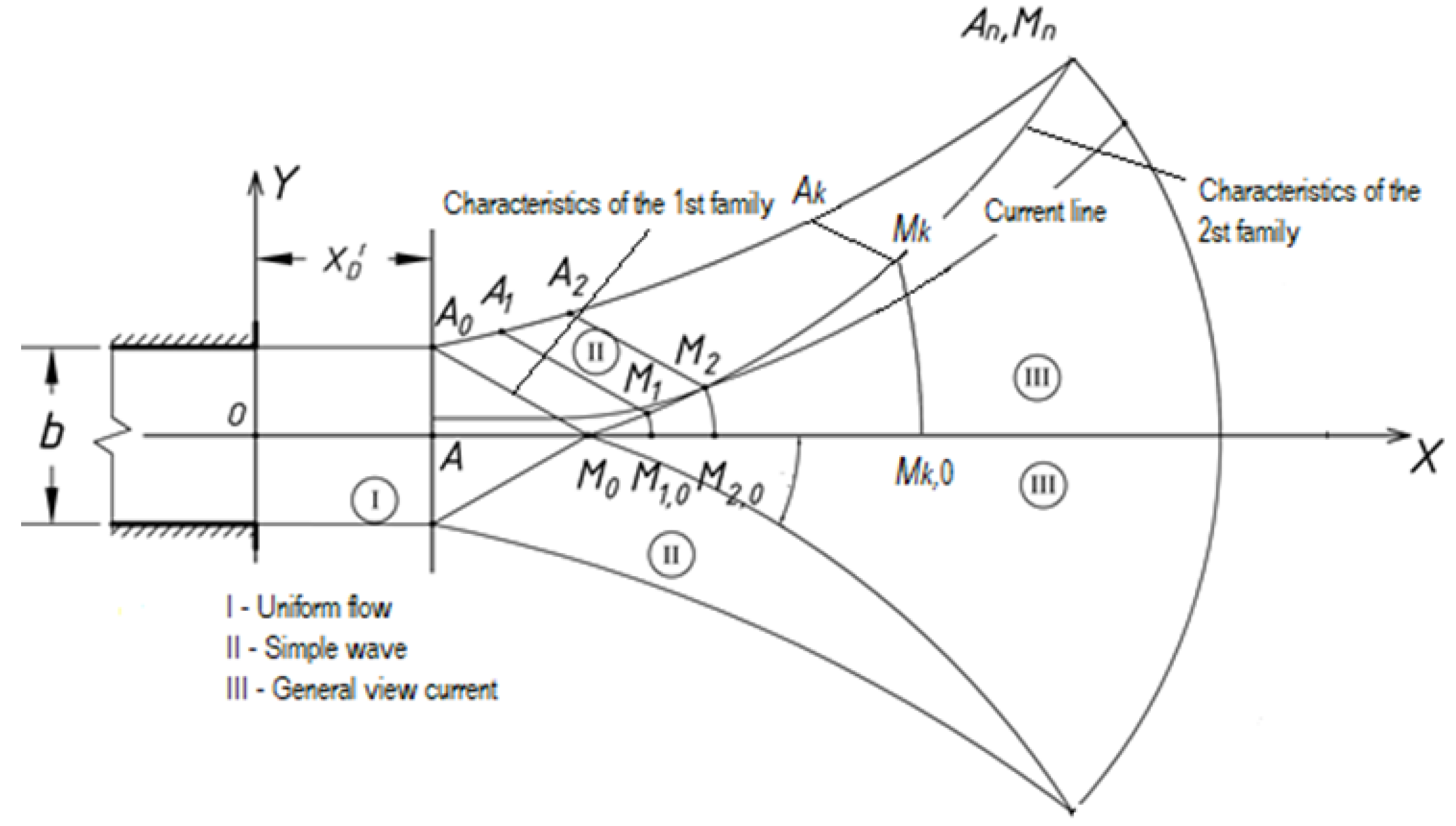
2.3.1. Solving the Problem in the Uniform Flow Area (Section I)
- Since at the flow velocity and the parameter , then the lines angle is also determined at this point:and consequently, the direction of the rectilinear characteristic of the 2nd family—.
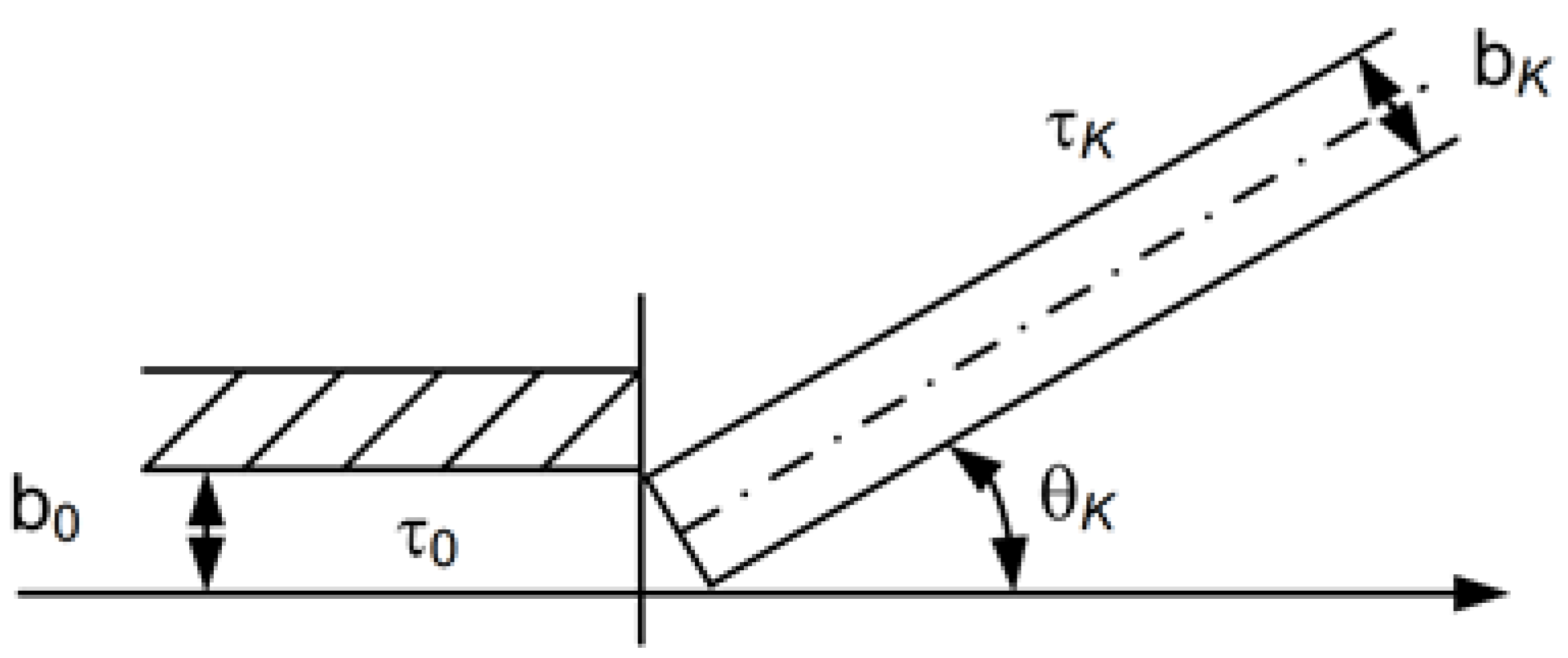
- Knowing the length of the inertial front [33,34,35,36,37], the geometry of the uniform flow section can be determined.—front length of the inertial section along the flow symmetry axis .The geometry of section I is determined by the parameters , , and pipe width b.Since the flow in area I is uniform, then , , , .
- Let us determine parameter values , on the characteristic of the 1st family. From the characteristic equation of the 1st family [20,38], the angle can be determined at the knownwhereSetting the spacing , we get:is determined from (12) at a fixed N.The angle is also determined from the system of Equation (12).
- Let us determine the flow coefficients at the intersection points i of the current line with the characteristic of the 1st family. From the equation equitable along the current linesubject to certain parameters , from (12) the flow coefficient between this current line and the longitudinal axis of flux symmetry OX is determined.
2.3.2. Problem Solving in the General Flow Area (Section III)
- Let us define the parameters , in the flow area of section III. The base flow is given by the equations in the velocity hodograph plane (Figure 5):This section is bounded by the 1st family characteristic and the flow symmetry axis . The characteristic runs through a point with parameters This is the main flow characteristic that runs through the entire flow. It has the form [20]:where
- Setting the parameters , at the points of intersection of the characteristics of the 1st family and the corresponding current line, it is possible to determine the parameters of the intersection points i of the current line and j of the equipotentiality from the systemHerewith
- Coordinates of the points , in region III of the flow is determined from the differential relation (5).If moving along the corresponding current line, Equation (5) by virtue of the condition is recomposed after separating the variables in the form of:
- Determination of parameters at points , . Let us draw the equipotential through the point C. Then the equation of the equipotential passing through the point C:At point we assume Consequently,Alternatively,Similarly, we determine the parameters at the point .
- Determining the coordinates of the points , . Parameters at a point are .The equation of the current line passing through the point :
2.3.3. Determination of Flow Parameters in the Simple Wave Region (Section II)
- We determine as an angular coefficient of slope of the tangent to the 1st family characteristic and a section of its uniformity. Since is a monotonically increasing function of the argument , then we determine the monotonicity areas of the function:whereTo do this, we solve the equationRoot of the Equation (14) defines areas of uniformity of the function , and consequently the functions :At the site the function monotonically decreases, and in the area monotonically increases.
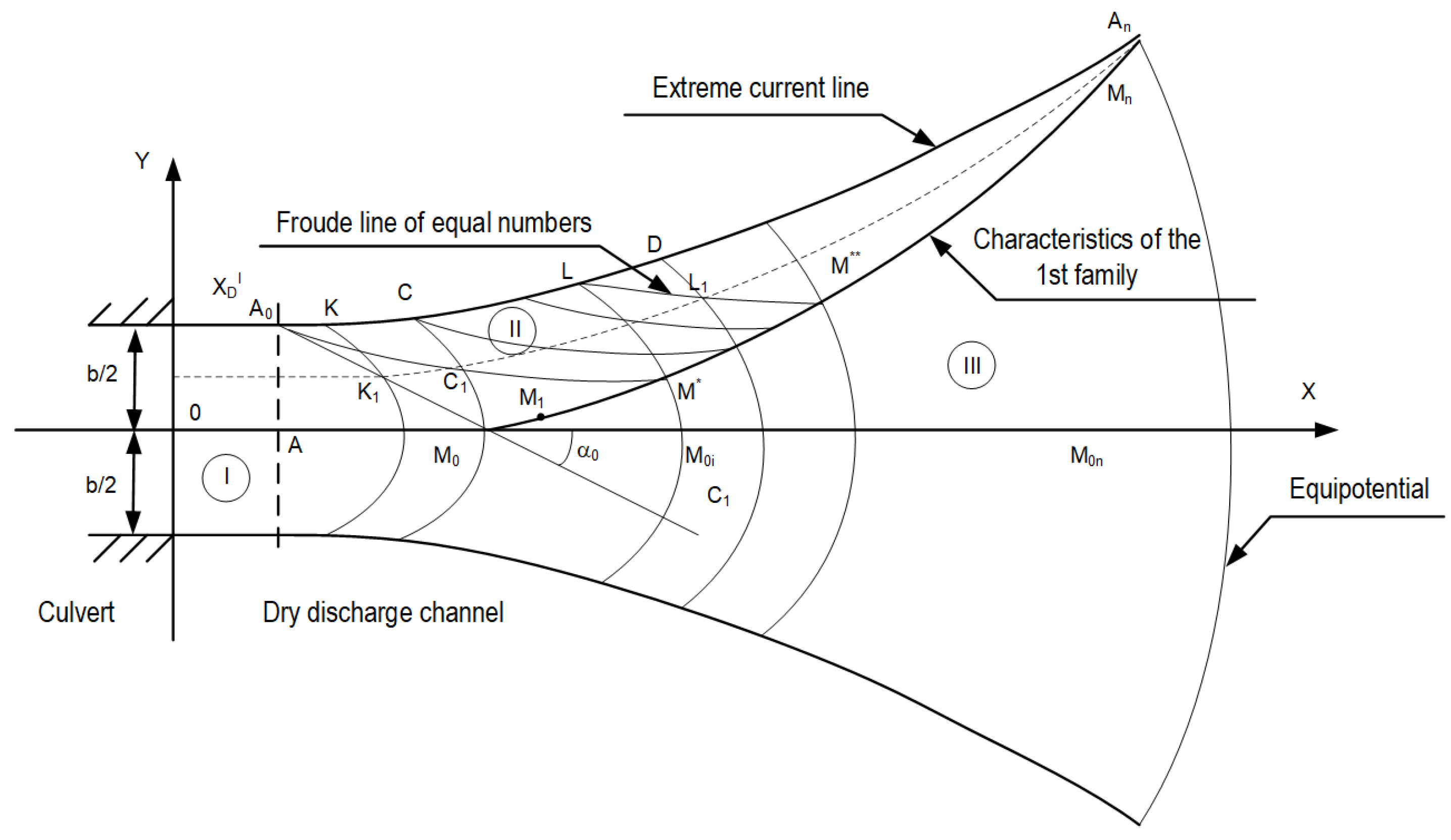
- Similarly, to section II with simple waves, we connect the Froude line points and A of equal numbers , the line on which is , and the angle is determined from the solution to the problem.Equal Froude number lines convey perturbations in the presence of discontinuities in the flow parameters.
- From the equation of the extreme current line determine the angleAs increases ultimately along the extreme current line , the points A and can be connected by Froude’s equal number perturbation waves.Perturbations by equal Froude number lines are more generic disturbances than a simple wave. In a simple waveIn a wave of Froude equal numbers linePoint should necessarily be connected to point A, as the minimum possible value at point A must be and further increase downstream.
- Further conducting an equipotential , let us determine the flow parameters at point C by solving the system:Similarly, we determine the flow parameters at the point L:assuming (15) , where is a parameter at a characteristic point , for which the following equation is true:
- Choice of steps in sections: Step selection on a characteristic between the points and :Then,Selecting the sampling step between the points and :Then,Selecting the sampling step between the points and :Then,
- The right lines , in a simple wave are determined from the condition that the characteristic of the 2nd family passes through the point and has an angular coefficient [5]. Extreme current line points are determined by the distance on the corresponding line :the flow rate between the outermost current line and the longitudinal axis of flow symmetry is maintained. It is equal to half of the total flow rate.
2.3.4. Improvement of the Proposed Algorithm
3. The Discussion of the Results
- initial flow velocity [cm/s];
- initial depth of the flow relative to the bottom [cm];
- gravity acceleration [cm/s];
- pipe width b [cm].
3.1. Solving the Problem in the Uniform Flow Area (Section I)
- Froude number ;
- initial flow velocity cm/s;
- hydrodynamic head cm;
- initial flow kinetics (block 1, item 4) ;
- wave angle at the point where the flow exits the pipe or ;
- angle of the velocity vector of the liquid flow to the OX axis at infinity or ;
- length of inertial front cm;
- distance from the end of the inertial section to the point along the flow symmetry axis cm;
- the length of the straight-line segment of the 2nd family characteristic between the points andcm.
3.2. Problem Solution in the General Flow Area (Section III) and in the Simple Wave Area (Section II)
4. Conclusions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Bolshakov, V.A. Hydraulics, 2nd ed.; Higher School: Kiev, Ukraine, 1984. [Google Scholar]
- Shterenlicht, D.V. Hydraulics, 3rd ed.; Kolos: Moscow, Russia, 2005. [Google Scholar]
- Volchenkova, G.Y. Manual for Hydraulic Calculations of Small Culverts; All-Russian Research Institute of Transport Construction, Main Directorate for Design and Capital Construction of the USSR Ministry of Transport: Moscow, Russia, 1992. [Google Scholar]
- Bernadsky, N.M. The theory of supercritical flow and its application to the construction of the plan of currents in open water bodies. In Materials on Hydrology, Hydrography and Water Forces of the USSR; Gosenergoizdat: Moscow-Leningrad, Russia, 1993; p. 20. [Google Scholar]
- Yemtsev, B.T. Two-Dimensional Stormy Flow; Energy: Moscow, Russia, 1967. [Google Scholar]
- Vysotsky, L.I. Management of Stormy Flows at Spillways; Energy: Moscow, Russia, 1990. [Google Scholar]
- Loytsyansky, L.G. Mechanics of Liquid and Gas, 5th ed.; Nauka: Moscow, Russia, 1978. [Google Scholar]
- Orlov, V.; Gasanov, M. Exact Criteria for the Existence of a Moving Singular Point in a Complex Domain for a Nonlinear Differential Third-Degree Equation with a Polynomial Seventh-Degree Right-Hand Side. Axioms 2022, 11, 222. [Google Scholar] [CrossRef]
- Orlov, V.; Gasanov, M. Existence and Uniqueness Theorem for a Solution to a Class of a Third-Order Nonlinear Differential Equation in the Domain of Analyticity. Axioms 2022, 11, 203. [Google Scholar] [CrossRef]
- Orlov, V.; Gasanov, M. Analytic Approximate Solution in the Neighborhood of a Moving Singular Point of a Class of Nonlinear Equations. Axioms 2022, 11, 637. [Google Scholar] [CrossRef]
- Orlov, V.; Gasanov, M. Technology for Obtaining the Approximate Value of Moving Singular Points for a Class of Nonlinear Differential Equations in a Complex Domain. Mathematics 2022, 10, 3984. [Google Scholar] [CrossRef]
- Korn, G. Mathematics for Scientists and Engineers; Nauka: Moscow, Russia, 1970. [Google Scholar]
- Aleksandrova, M.S. Method of Analogies between Hydraulics of Two-Dimensional Water Flows and Gas Dynamics. Constr. Archit. 2020, 2, 49–52. [Google Scholar] [CrossRef]
- Popov, D.N.; Panaiotti, S.S.; Ryabinin, M.V. Hydromechanics; Bauman Moscow State Technical University: Moscow, Russia, 2002. [Google Scholar]
- Esin, A.I. Problems of Technical Fluid Mechanics in Natural Coordinates; Saratov SAU: Saratov, Russia, 2003. [Google Scholar]
- Bolshakov, V.A.; Galetskiy, V.I.; Denisenko, I.D. Water accounting for automated regulation of water supply to canals. Melior. Water Manag. 1983, 3, 18–24. [Google Scholar]
- Kokhanenko, V.N. Two-Dimensional in Plan Stormy Stationary Flows behind the Culverts in Conditions of Free Spreading, Autor’s Abstract. Ph.D. Thesis, Diss. Doct. Tech. Science.. Moscow State University of Civil Engineering, Moscow, Russia, 17 June 1997. [Google Scholar]
- Kokhanenko, V.N. Modeling of One-Dimensional and Two-Dimensional Open Water Flows; Publishing House of the Southern Federal University: Rostov-on-Don, Russia, 2007. [Google Scholar]
- Chaplygin, S.A. Mechanics of liquid and gas. In Maths. General Mechanics: Selected Works; Nauka: Moscow, Russia, 1976. [Google Scholar]
- Kohanenko, V.N.; Volosukhin, Y.V. Modeling of Stormy Two-Dimensional in Terms of Water Flows; Publishing House of the Southern Federal University: Rostov on Don, Russia, 2013. [Google Scholar]
- Kohanenko, V. Derivation of the main system of motion equations for a two-dimensional flow in the velocity hodograph plane and the search for its particular solutions. The manuscript was deposited with the All-Russian Institute of Scientific and Technical Information of the Russian Academy of Sciences (VINITI). Russia, 1996; Volume 96, 98. [Google Scholar]
- Kajishima, T.; Taira, K. Computational Fluid Dynamics—Incompressible Turbulent Flows; Springer: Berlin/Heidelberg, Germany, 2016. [Google Scholar]
- William, E.B.; Richard, C.D.; Douglas, B.M. Elementary Differential Equations and Boundary Value Problems; John Wiley & Sons: Hoboken, NJ, USA, 2021. [Google Scholar]
- Sherenkov, I.A. The spreading of a stormy flow behind the outlet heads of culverts under railway embankments. Proc. Kharkov Inst. Railw. Eng. Named After S. M. Kirov 1957, 30. [Google Scholar]
- Sherenkov, I.A. On the planned problem of spreading a jet of a turbulent flow of an incompressible liquid. News Acad. Sci. USSR 1958, 1, 72–78. [Google Scholar]
- Sherenkov, I. Experimental study of the supercritical flow spreading behind the outlet heads of culverts. In Proceedings of the Joint Seminar on Hydraulic Engineering and Water Management Construction, Kharkov, Ukraine; 1958; Volume 1. [Google Scholar]
- Sherenkov, I.A. On the Role of Normal Supercritical Stresses in the Currents Plan Formation Hydraulics and Hydraulic Engineering; Technics: Kiev, Ukraine, 1973; Volume 17. [Google Scholar]
- Sherenkov, I.A. Applied Planning Tasks of Gravity Flows Hydraulics; Energy: Moscow, Russia, 1978. [Google Scholar]
- Kokhanenko, V.N. On the planned problem of spreading a supercritical flow of incompressible liquid. Sci. J. Bull. High. Educ. Institutions North Cauc. Reg. Tech. Sci. 2012, 6, 82–88. [Google Scholar]
- Duvanskaya, E.V. Calculation of the Potential Movement of Two-Dimensional Stationary, Quiet Flows. Ph.D. Thesis, Diss. Cand. Tech. Sciences.. Novocherkassk State Reclamation Academy, Novocherkassk, Russia, 25 September 2003. [Google Scholar]
- Kokhanenko, V.N. Determination of Parameters of a Freely Spreading Flow. Certificate of Registration of Computer Programs, Federal Institute of Industrial Property (FIPS) No. RU 2022618552. 2022. Available online: https://www1.fips.ru/ofpstorage/Doc/PrEVM/RUNWPR/000/002/022/618/552/2022618552-00001/ (accessed on 1 July 2022).
- Kokhanenko, V.N.; Kelekhsaev, D.B. The problem solution of determining the extreme streamline equation and parameters along it, taking into account the XD section. In Research Results—2019: Materials of the IV National Conference for Teaching Staff and Scientific Workers; SRSPU (NPI): Novocherkassk, Russia, 2019; pp. 113–117. [Google Scholar]
- Kokhanenko, V.N.; Kelekhsaev, D.B.; Kondratenko, A.I.; Evtushenko, S.I. Two-dimensional motion equations in water flow zone. IOP Conf. Ser. Mater. Sci. Eng. 2019, 698, 066026. [Google Scholar] [CrossRef]
- Kokhanenko, V.N.; Kelekhsaev, D.; Kondratenko, A.; Evtushenko, S. A System of Equations for Potential Two-Dimensional In-Plane Water Courses and Widening the Spectrum of Its Analytical Solutions. AIP Conf. Proc. 2019, 2188, 050017. [Google Scholar] [CrossRef]
- Kokhanenko, V.N.; Kelekhsaev, D.B.; Kondratenko, A.I.; Evtushenko, S.I. Solution of equation of extreme streamline with free flowing of a torrential flow behind rectangular pipe. IOP Conf. Ser. Mater. Sci. Eng. 2020, 775, 012134. [Google Scholar] [CrossRef]
- Kokhanenko, V.N.; Kelekhsaev, D.B.; Kondratenko, A.I.; Evtushenko, S.I. Solution of Equations of Motion of Two-Dimensional Water Flow. Constr. Archit. 2019, 7, 5–12. [Google Scholar] [CrossRef]
- Kokhanenko, V.N.; Burtseva, O.A.; Evtushenko, S.I.; Kondratenko, A.I.; Kelekhsaev, D.B. Two-Dimensional in Plan Radial Flow (NonPressure Potential Source). Constr. Archit. 2019, 7, 25. [Google Scholar] [CrossRef]
- Vysotsky, L.I. Hydraulic Calculation of Scattering Trampolines by the Method of Longitudinal Approximations; MCEU Named after V. V. Kuibyshev: Moscow, Russia, 1960. [Google Scholar]

| 0.545 | 0.596 | 0.646 | 0.697 | 0.747 | 0.798 | 0.848 | 0.899 | 0.949 | 1 | |
| 6.299 | 7.269 | 8.547 | 10.259 | 12.642 | 16.169 | 21.942 | 33.244 | 66.336 | 3233 | |
| 147.654 | 154.345 | 160.759 | 166.926 | 172.873 | 178.622 | 184.192 | 189.599 | 194.855 | 199.963 | |
| 9.274 | 8.234 | 7.215 | 6.176 | 5.157 | 4.117 | 3.098 | 2.059 | 1.039 | 0 |
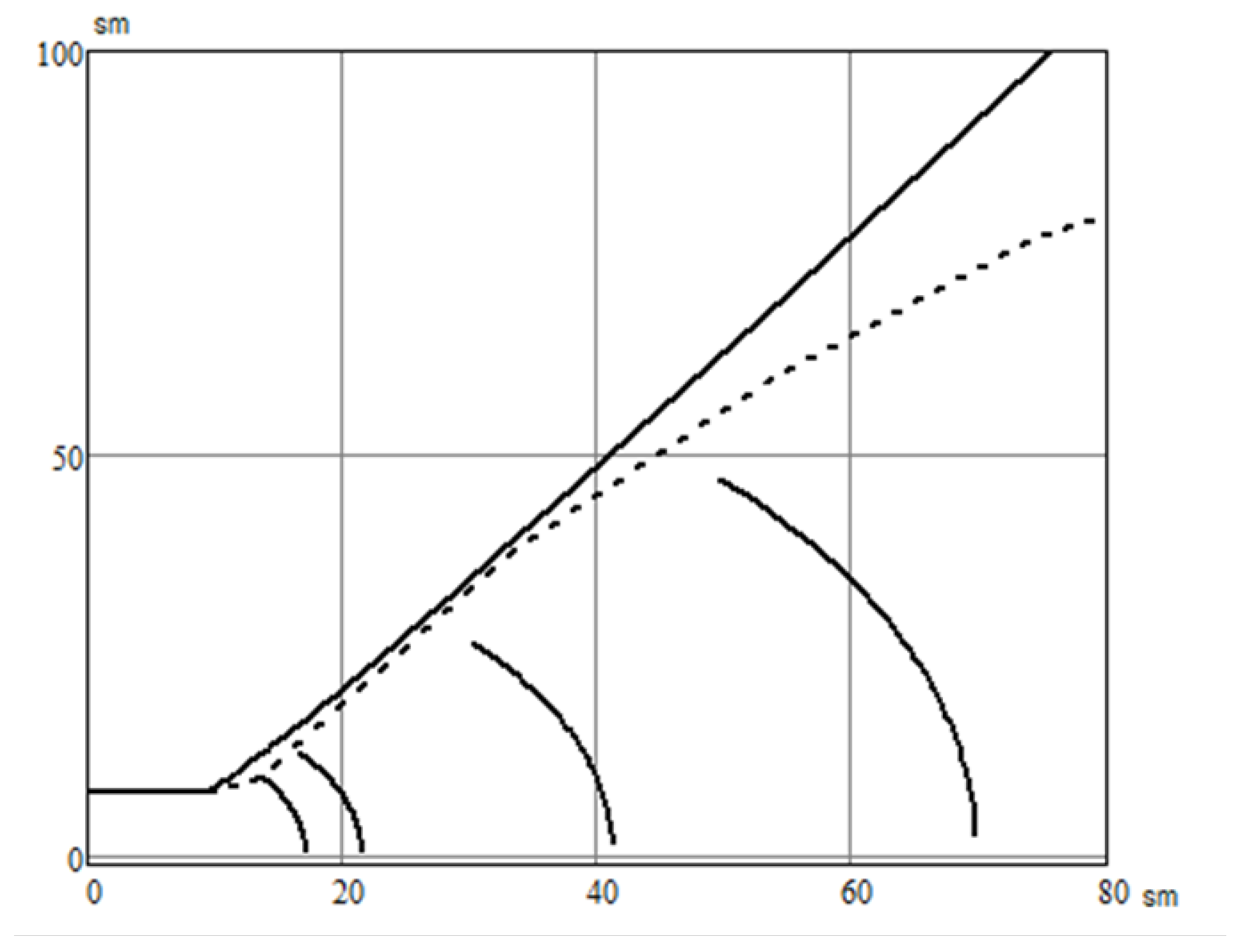
| Point No. | Abscissa on the Symmetry Axis at | Parameter of Kinetics, | Angle of Inclination of the Flow Velocity Vector to the Axis of Symmetry | Flow Ordinates on the Extreme Line | Experimental Data at Some Points | Relative Algorithm Error, % |
|---|---|---|---|---|---|---|
| 1 | 0 | 0.545 | 0.661 | 8 | 8 | 0 |
| 2 | 4 | 0.767 | 0.815 | 10.442 | 11 | 5.073 |
| 3 | 8 | 0.866 | 0.884 | 13.637 | ||
| 4 | 12 | 0.908 | 0.914 | 18.687 | ||
| 5 | 16 | 0.931 | 0.93 | 23.973 | ||
| 6 | 20 | 0.945 | 0.94 | 29.402 | ||
| 7 | 24 | 0.954 | 0.947 | 34.926 | 38 | 8.090 |
| 8 | 28 | 0.961 | 0.952 | 40.519 | ||
| 9 | 32 | 0.966 | 0.956 | 46.162 | ||
| 10 | 36 | 0.97 | 0.959 | 51.846 | ||
| 11 | 40 | 0.973 | 0.961 | 57.56 | ||
| 12 | 44 | 0.975 | 0.963 | 63.3 | 59 | 7.288 |
| 13 | 48 | 0.978 | 0.965 | 69.06 | ||
| 14 | 52 | 0.979 | 0.966 | 74.84 | 73 | 2.458 |
| Step No. | Kinetics | Angle of Inclination of Velocity Vector | Fluid Flow Coefficient |
|---|---|---|---|
| 1 | 0.5452 | 0.145 | 0.212 |
| 2 | 0.5756 | 0.157 | 0.229 |
| 3 | 0.6059 | 0.17 | 0.246 |
| 4 | 0.6363 | 0.184 | 0.263 |
| 5 | 0.6667 | 0.197 | 0.28 |
| 6 | 0.6765 | 0.211 | 0.297 |
| 7 | 0.6862 | 0.224 | 0.314 |
| ⋮ | ⋮ | ⋮ | ⋮ |
| 31 | 0.9208 | 0.697 | 0.788 |
| 32 | 0.9306 | 0.734 | 0.819 |
| 33 | 0.9404 | 0.778 | 0.853 |
| 34 | 0.9501 | 0.834 | 0.896 |
| 35 | 0.9599 | 0.937 | 0.97 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2022 by the author. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Evtushenko, S. A Nonlinear System of Differential Equations in Supercritical Flow Spread Problem and Its Solution Technique. Axioms 2023, 12, 11. https://doi.org/10.3390/axioms12010011
Evtushenko S. A Nonlinear System of Differential Equations in Supercritical Flow Spread Problem and Its Solution Technique. Axioms. 2023; 12(1):11. https://doi.org/10.3390/axioms12010011
Chicago/Turabian StyleEvtushenko, Sergej. 2023. "A Nonlinear System of Differential Equations in Supercritical Flow Spread Problem and Its Solution Technique" Axioms 12, no. 1: 11. https://doi.org/10.3390/axioms12010011
APA StyleEvtushenko, S. (2023). A Nonlinear System of Differential Equations in Supercritical Flow Spread Problem and Its Solution Technique. Axioms, 12(1), 11. https://doi.org/10.3390/axioms12010011







