Three-Way Fuzzy Sets and Their Applications (III)
Abstract
:1. Introduction
2. Preliminaries
3. Three-Way t-Norm and Its Residual Implication
- (1)
- adjoint pairs
- (2)
- Gdel adjoint pairs
4. The Triple I Algorithm of Three-Way Fuzzy Inference
4.1. The Triple I Algorithm Based on Three-Way Residual Implication
4.2. The Triple I Algorithm Based on Three-Way Fuzzy Implication Operator
4.3. Three-Way Fuzzy Inference Based on Triple I Algorithm
- (1)
- is calculated using the TCRI method.
- (2)
- is calculated using the triple I algorithm. From and , we have
- (1)
- is calculated using the TCRI method.
- (2)
- is calculated using the triple I algorithm. From , we have
- (1)
- For the same sets of given: A, B and , the results are different because of the different R, which are generated by different three-way fuzzy implication operators.
- (2)
- For the same sets of given: A, B and , and the same three-way fuzzy implication operator, the results are different due to different inference methods, and the triple I algorithm is better.
5. Application of Three-Way Fuzzy Inference
6. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Ross, S. A First Course in Probability/Ninth Edition; Pearson: London, UK, 2014. [Google Scholar]
- Shafer, G. A Mathematical Theory of Evidence; Princeton University Press: Princeton, NJ, USA, 1976. [Google Scholar]
- Zadeh, L. Fuzzy sets. Inf. Control 1965, 8, 338–353. [Google Scholar] [CrossRef] [Green Version]
- Pawlak, Z. Rough Sets: Theoretical Aspects of Reasoning about Data; Kluwer Academic Publishers: Boston, MA, USA, 1991. [Google Scholar]
- Atanassov, K. Intuitionistic fuzzy sets. Fuzzy Sets Syst. 1986, 20, 87–96. [Google Scholar] [CrossRef]
- Vassilev, P.; Atanassov, K. Extensions and Modifications of Intuitionistic Fuzzy Sets; “Prof. Marin Drinov” Academic Publishing House: Dover, DE, USA, 2019. [Google Scholar]
- Hinde, C.; Patching, R. Inconsistent intuitionistic fuzzy sets. Dev. Fuzzy Sets, Intuitionistic Fuzzy Sets, Gen. Nets Relat. Top. 2008, 1, 133–153. [Google Scholar]
- Behounek, L.; Dankova, M. Towards fuzzy partial set theory. In Proceedings of the International Conference on Information Processing and Management of Uncertainty in Knowledge-Based Systems, Eindhoven, The Netherlands, 12 June 2016. [Google Scholar]
- Daňková, M. Fuzzy relations and fuzzy functions in partial fuzzy set theory. Adv. Intell. Syst. Comput. 2018, 641, 563–573. [Google Scholar]
- Smarandache, F. Neutrosophy, Neutrosophic Probability, Set, and Logic; American Research Press: Rehoboth, DE, USA, 1998. [Google Scholar]
- Sheng, N.; Zhang, X. Regular Partial Residuated Lattices and Their Filters. Mathematics 2022, 10, 2429. [Google Scholar] [CrossRef]
- Hu, Q.; Zhang, X. Neutrosophic Triangular Norms and Their Derived Residuated Lattices. Symmetry 2019, 11, 817. [Google Scholar] [CrossRef] [Green Version]
- Zhang, X.; Liang, R.; Bedregal, B. Weak Inflationary BL-Algebras and Filters of Inflationary (Pseudo) General Residuated Lattices. Mathematics 2022, 10, 3394. [Google Scholar] [CrossRef]
- Cuong, B.; Kreinovich, V. Picture fuzzy sets-A new concept for computational intelligence problems. In Proceedings of the Third World Congress on Information and Communication Technologies WICT’2013, Hanoi, Vietnam, 15–18 December 2013; pp. 1–6. [Google Scholar]
- Zhang, X.; Bo, C.; Smarandache, F.; Park, C. New Operations of Totally Dependent-Neutrosophic Sets and Totally Dependent-Neutrosophic Soft Sets. Symmetry 2018, 10, 187. [Google Scholar] [CrossRef] [Green Version]
- Yao, Y. Three-way decisions with probabilistic rough sets. Inf. Sci. 2010, 180, 341–353. [Google Scholar] [CrossRef] [Green Version]
- Li, X.; Qi, J.; Sun, B.; Yao, Y. Three-Way Decision Theory and Methods; Science Press: Beijing, China, 2019. [Google Scholar]
- Yao, Y. Set-theoretic models of three-way decision. Granul. Comput. 2021, 6, 133–148. [Google Scholar] [CrossRef]
- Yao, Y. The geometry of three-way decision. Appl. Intell. 2021, 51, 6298–6325. [Google Scholar] [CrossRef]
- Dubois, D.; Prade, H. Rough fuzzy sets and fuzzy rough sets. Int. J. Gen. Syst. 1990, 17, 191–209. [Google Scholar] [CrossRef]
- Yao, Y. A comparative study of fuzzy sets and rough sets. J. Inf. Sci. 1998, 109, 227–242. [Google Scholar] [CrossRef]
- Radzikowska, A.; Kerre, E. A comparative study of fuzzy rough sets. Fuzzy Sets Syst. 2002, 126, 137–155. [Google Scholar] [CrossRef]
- Wu, W. Attribute reduction based on evidence theory in incomplete decision systems. Inf. Sci. 2007, 178, 1355–1371. [Google Scholar] [CrossRef]
- Xu, W.; Liu, S.; Zhang, W. Lattice-valued information systems based on dominance relation. Int. J. Mach. Learn. Cybern. 2013, 4, 245–257. [Google Scholar] [CrossRef]
- Chen, D.; Li, W.; Zhang, X.; Kwong, S. Evidence-theory-based numerical algorithms of attribute reduction with neighborhood-covering rough sets. Int. J. Approx. Reason. 2014, 55, 908–923. [Google Scholar] [CrossRef]
- Zhang, X.; Hu, Q.; Wang, J.; Li, X. Three-way fuzzy sets and their applications (I). J. Northwest Univ. Nat. Sci. Ed. 2022, 52, 539–551. [Google Scholar]
- Wang, J.; Zhang, X.; Hu, Q. Three-way fuzzy sets and their applications (II). Axioms 2022, 11, 532. [Google Scholar] [CrossRef]
- Zadeh, L. Outline of a new approach to the analysis of complex systems and decision processed. Trans. Syst. 1973, 3, 28–44. [Google Scholar]
- Jenei, S. Continuity in Zadeh’s compositional rule of inference. Fuzzy Sets Syst. 1999, 104, 333–339. [Google Scholar] [CrossRef]
- Rotshtein, A.; Rakytyanska, H. Diagnosis problem solving using fuzzy relations. IEEE Trans. Fuzzy Syst. 2008, 16, 664–675. [Google Scholar] [CrossRef]
- Li, H.; You, F.; Peng, J.; Zeng, W. A fuzzy controller based on some fuzzy implication operators and its response function. Prog. Nat. Sci. 2003, 13, 5. [Google Scholar]
- Zheng, Z.; Wu, S.; Liu, W.; Cai, K.Y. A feedback based CRI approach to fuzzy reasoning. Appl. Soft Comput. 2011, 11, 1241–1255. [Google Scholar] [CrossRef]
- Zhang, C.; Yuan, X.; Wang, Y. The Reductivity of fuzzy inference. Fuzzy Eng. Oper. Res. 2012, 147, 155–163. [Google Scholar]
- Li, H. Interpolation mechanism of fuzzy control. Sci. China Ser. E 1998, 28, 259–267. [Google Scholar] [CrossRef]
- Wang, G. The full implication triple I method for fuzzy reasoning. Sci. China Ser. E 1999, 29, 43–53. [Google Scholar]
- Pei, D. On the strict logic foundation of fuzzy reasoning. Soft Comput. 2004, 8, 539–545. [Google Scholar] [CrossRef]
- Fu, L.; He, H. Research on the incompatible factor of fuzzy reasoning. Comput. Sci. 2004, 31, 98–100, 116. [Google Scholar]
- Qin, K.; Pei, Z. Opposite direction triple I method under Lukasiewicz implication operator. Fuzzy Syst. Math. 2005, 19, 1–5. [Google Scholar]
- Zhao, Z.; Li, Y. Reverse triple I method of fuzzy reasoning for the implication operator RL. Comput. Math. Appl. 2007, 53, 1020–1028. [Google Scholar] [CrossRef] [Green Version]
- Peng, J. Full implication method of interval-valued intuitionistic fuzzy reasoning. Fuzzy Syst. Math. 2019, 33, 35–45. [Google Scholar]
- Wang, L.; Pei, D. General full implication method of fuzzy reasoning. Fuzzy Syst. Math. 2019, 33, 46–55. [Google Scholar]
- Zheng, M.; Liu, Y. Multiple-rules reasoning based on Triple I method on Atanassov’s intuitionistic fuzzy sets. Int. J. Approx. Reason. 2019, 113, 196–206. [Google Scholar] [CrossRef]
- Song, S.; Feng, C.; Wu, C. On the constraint degree theory of total implication triple I algorithm for fuzzy reasoning. Prog. Nat. Sci. 2000, 10, 22–27. [Google Scholar]
- Pei, D. Two triple I methods for FMT problem and their reductivity. Fuzzy Syst. Math. 2001, 15, 1–7. [Google Scholar]
- Wang, Q.; He, Y.; Song, Z. Sustaining degree of fuzzy triple I method based on residual implication. J. Southwest Jiaotong Univ. 2004, 39, 550–553. [Google Scholar]
- Peng, J. A total implication triple I constraint algorithm for fuzzy reasoning based on some common implication operators. Prog. Nat. Sci. 2005, 15, 539–546. [Google Scholar]
- Hou, J.; Li, H. Reductivity of some fuzzy inference methods. Fuzzy Syst. Math. 2005, 19, 90–95. [Google Scholar]
- Wang, G. Nonclassical Mathematical Logic and Approximate Reasoning; Science Press: Beijing, China, 2008. [Google Scholar]
- Liu, H.; Wang, G. Triple I method based on pointwise sustaining degrees. Comput. Math. Appl. 2008, 55, 2680–2688. [Google Scholar] [CrossRef] [Green Version]
- Luo, M.; Kai, Z. Robustness of full implication algorithms based on interval-valued fuzzy inference. Int. J. Approx. Reason. 2015, 62, 61–72. [Google Scholar] [CrossRef]
- Luo, M.; Wu, L.; Fu, L. Robustness Analysis of the Interval-valued Fuzzy Inference Algorithms. J. Intell. Fuzzy Syst. 2020, 38, 685–696. [Google Scholar] [CrossRef]
- Li, H.; Peng, J.; Wang, J.; Jian, H.; Zhang, Y. Fuzzy systems based on triple I algorithm and their response ability. J. Syst. Sci. Math. Sci. 2006, 26, 578–590. [Google Scholar]
- Tang, Y.; Liu, X. Fuzzy systems constructed by triple I method or CRI method and their response functions. J. Hefei Univ. Technol. (Nat. Sci. Ed.) 2010, 33, 182–187. [Google Scholar]
- Li, L.; Pei, D. Response ability of fuzzy systems based on the universal triple I methods. J. Zhejiang Inst. Sci. Technol. 2013, 30, 59–64. [Google Scholar]
- Deschrijver, G. Implication functions in interval-valued fuzzy set theory. Stud. Fuzziness Soft Comput. 2013, 300, 73–99. [Google Scholar]
- Baczýnski, M.; Jayaram, B. Fuzzy Implications; Springer: Berlin, Heidelberg, 2008. [Google Scholar]
- Zhang, X. Fuzzy Mathematics and Rough Set Theory; Tsinghua University Press: Beijing, China, 2013. [Google Scholar]
- Pei, D. Full implication algorithm of fuzzy inference and its reducibility. Math. Res. Appl. 2004, 24, 359–368. [Google Scholar]
- Liang, R.; Zhang, X. Pseudo General Overlap Functions and Weak Inflationary Pseudo BL-Algebras. Mathematics 2022, 10, 3007. [Google Scholar] [CrossRef]
- Zhang, X.; Liang, R.; Bustince, H.; Bedregal, B.; Fernandez, J.; Li, M.; Ou, Q. Pseudo Overlap Functions, Fuzzy Implications and Pseudo Grouping Functions with Applications. Axioms 2022, 11, 593. [Google Scholar] [CrossRef]
- Zhang, X.; Sheng, N.; Borzooei, R.A. Partial residuated implications induced by partial triangular norms and partial residuated lattices. Axioms 2022, accepted. [Google Scholar]
- Zhang, X.; Du, Y. Left (right) regular and transposition regular semigroups and their structures. Mathematics 2022, 10, 1021. [Google Scholar] [CrossRef]
- Hu, M.; Zhang, X. On cyclic associative semihypergroups and neutrosophic extended triplet cyclic associative semihypergroups. Mathematics 2022, 10, 535. [Google Scholar] [CrossRef]



| NB | NM | NS | ZE | PS | PM | PB | |||
|---|---|---|---|---|---|---|---|---|---|
| NB | PB | PB | PM | PM | PS | PS | ZE | ||
| NM | PB | PM | PM | PS | PS | ZE | NS | ||
| NS | PM | PM | PS | PS | ZE | NS | NS | ||
| ZE | PM | PS | PS | ZE | NS | NS | NM | ||
| PS | PS | PS | ZE | NS | NS | NM | NM | ||
| PM | PS | ZE | NS | NS | NM | NM | NB | ||
| PB | ZE | NS | NS | NM | NM | NB | NB | ||
| u | IP | Mamdani | Gdel | Zadeh | ||
|---|---|---|---|---|---|---|
| DF | ||||||
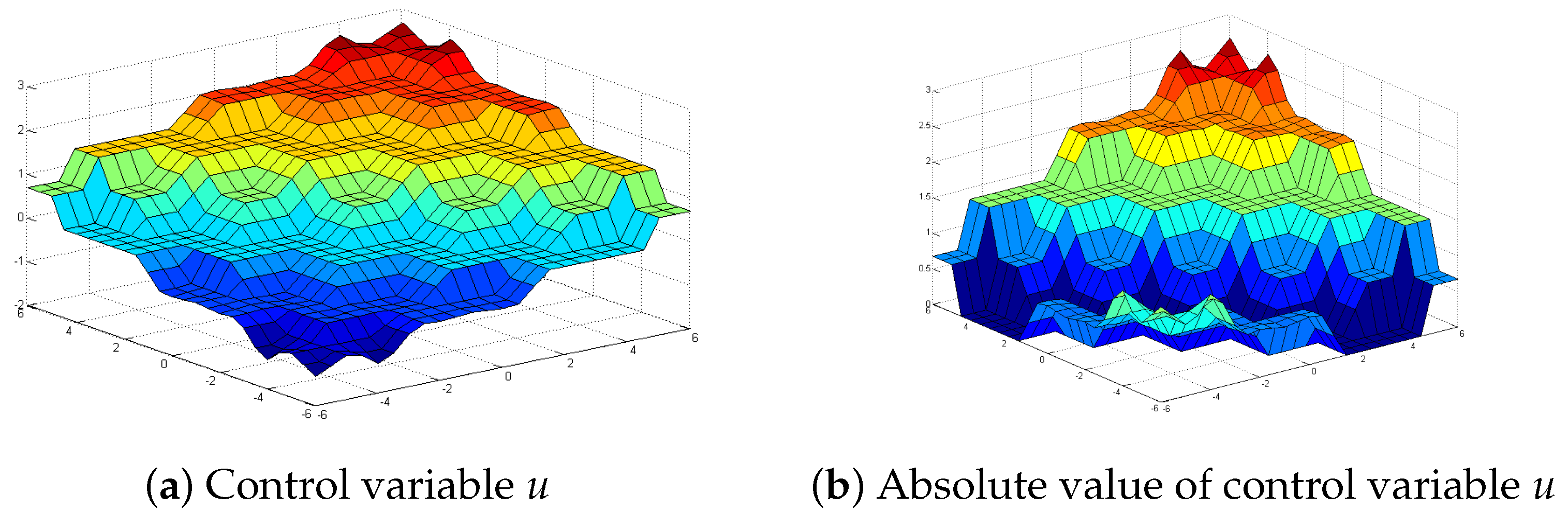
| WA | Figure 4A [26] | Figure 5b | Figure 5b | Figure 5b | ||
| MOM | Figure 5a | Figure 4a | Figure 4a | Figure 4a | ||
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Hu, Q.; Zhang, X. Three-Way Fuzzy Sets and Their Applications (III). Axioms 2023, 12, 57. https://doi.org/10.3390/axioms12010057
Hu Q, Zhang X. Three-Way Fuzzy Sets and Their Applications (III). Axioms. 2023; 12(1):57. https://doi.org/10.3390/axioms12010057
Chicago/Turabian StyleHu, Qingqing, and Xiaohong Zhang. 2023. "Three-Way Fuzzy Sets and Their Applications (III)" Axioms 12, no. 1: 57. https://doi.org/10.3390/axioms12010057
APA StyleHu, Q., & Zhang, X. (2023). Three-Way Fuzzy Sets and Their Applications (III). Axioms, 12(1), 57. https://doi.org/10.3390/axioms12010057








