Sharp Power Mean Bounds for Two Seiffert-like Means †
Abstract
:1. Introduction
2. Preliminaries
2.1. Tools
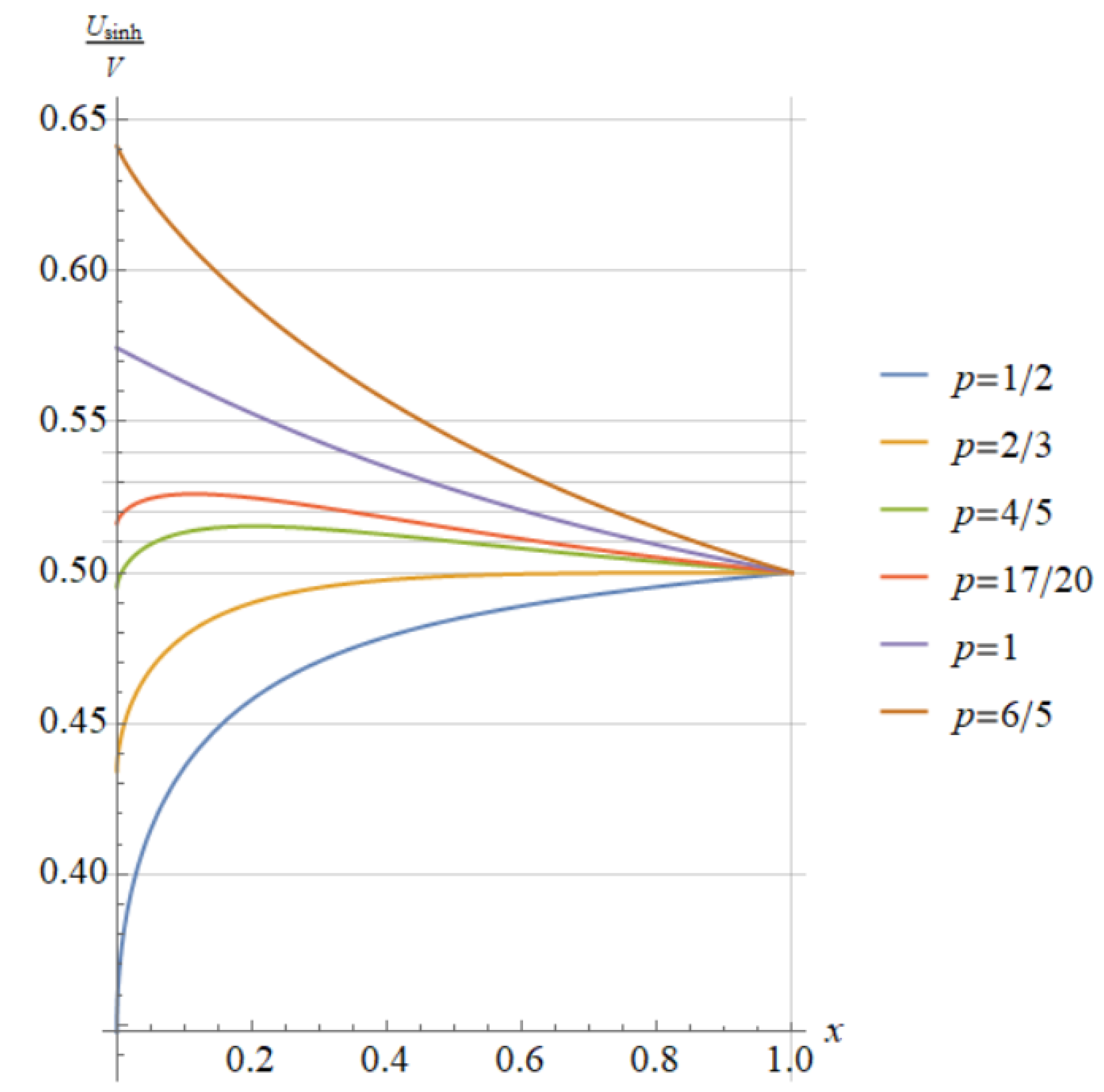
2.2. Three Monotonicity Results
3. Proofs of Main Results
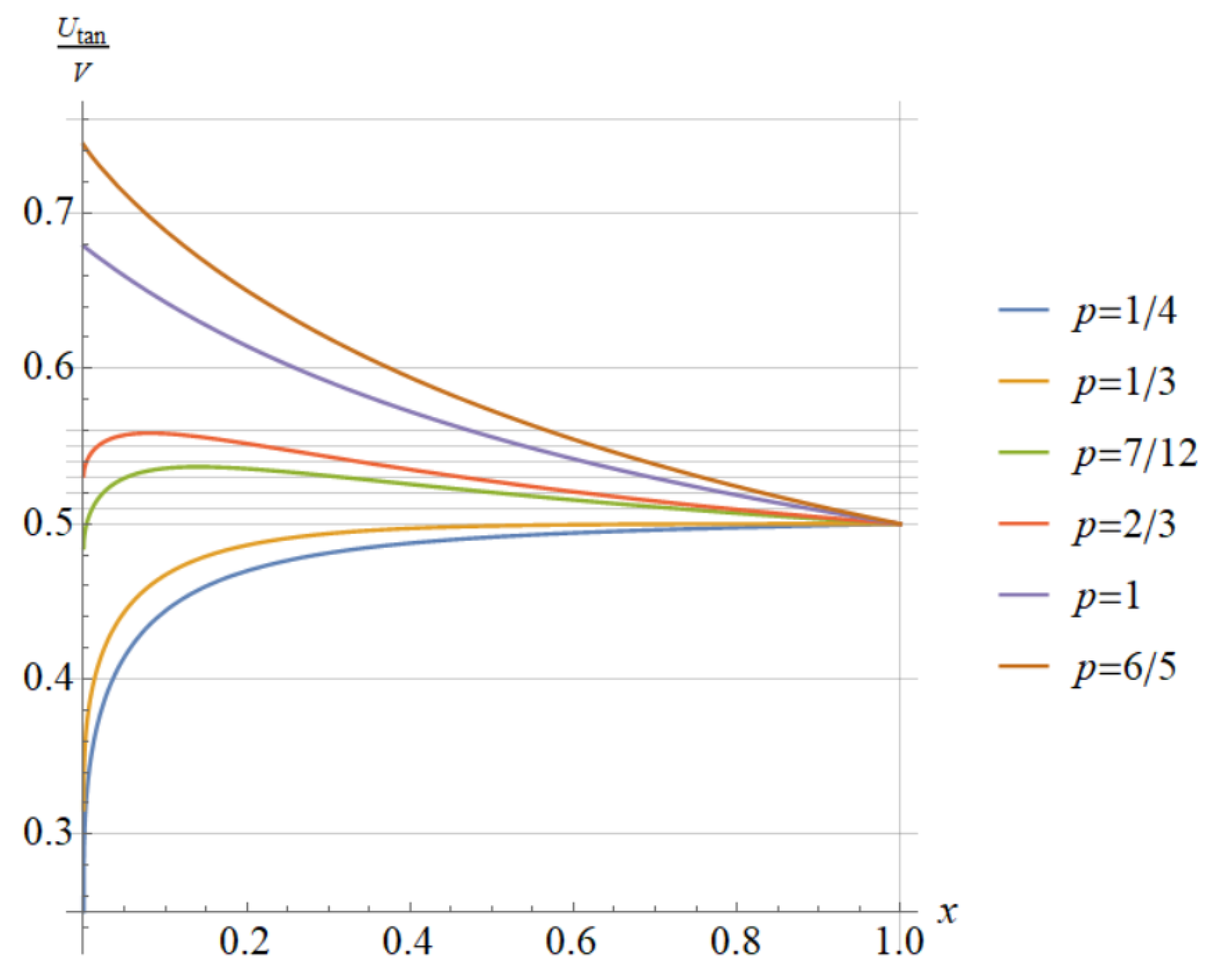
3.1. Proofs of Theorems 1 and 2
3.2. Proofs of Theorems 3 and 4
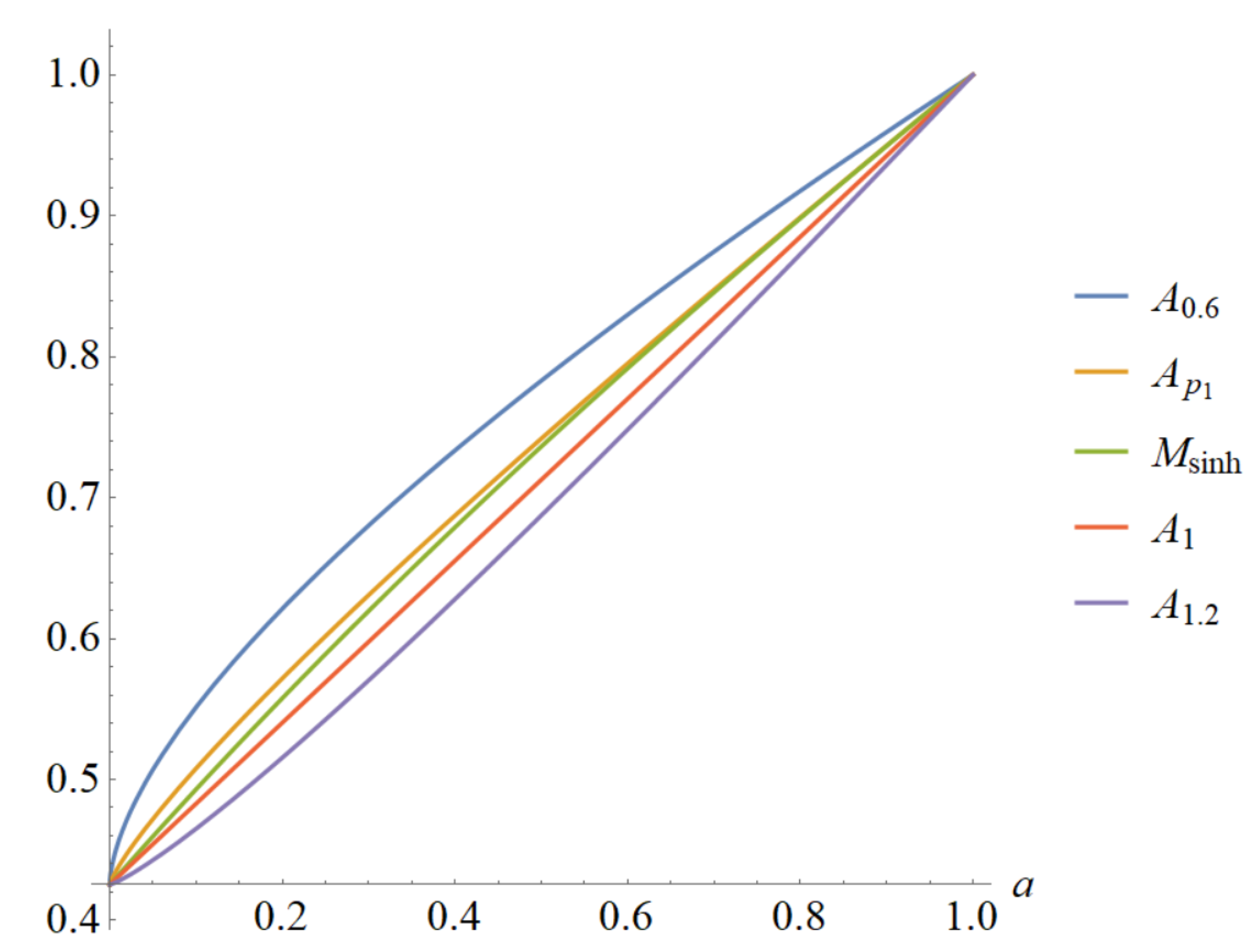
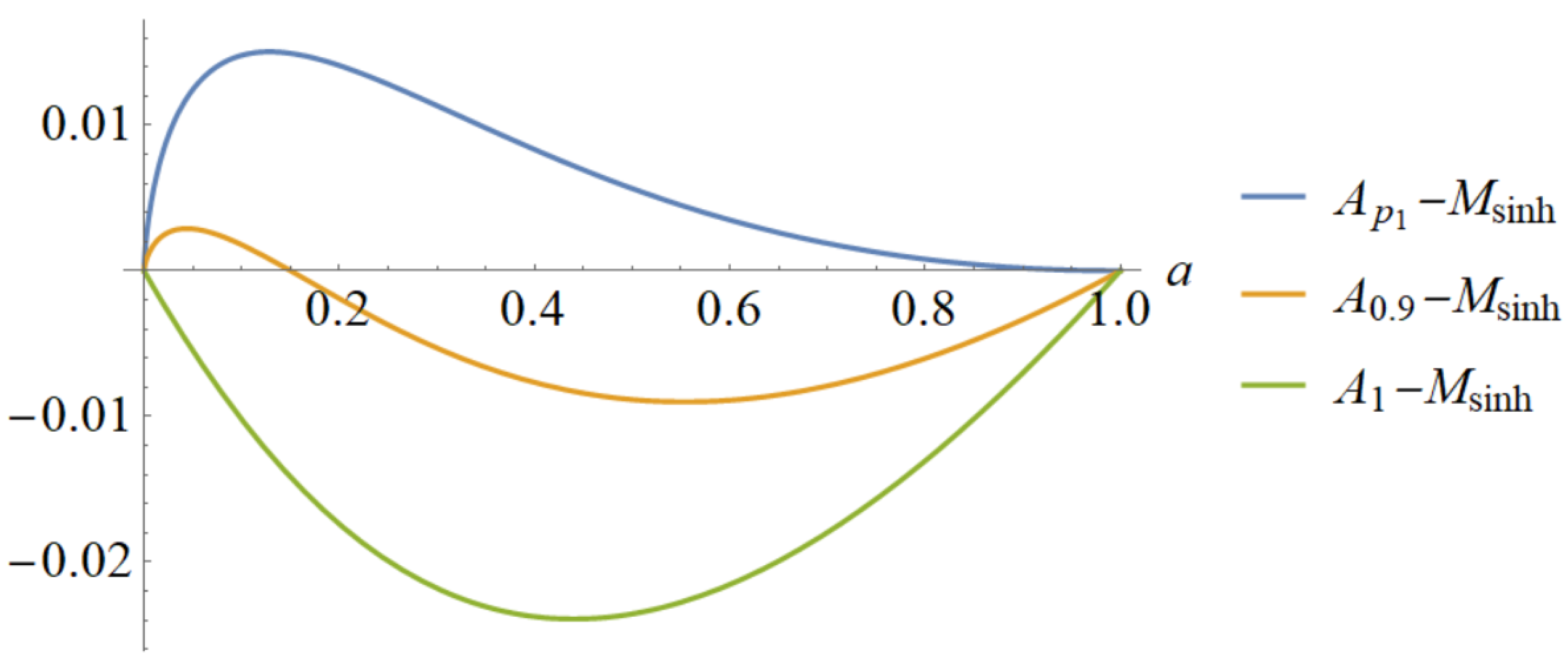
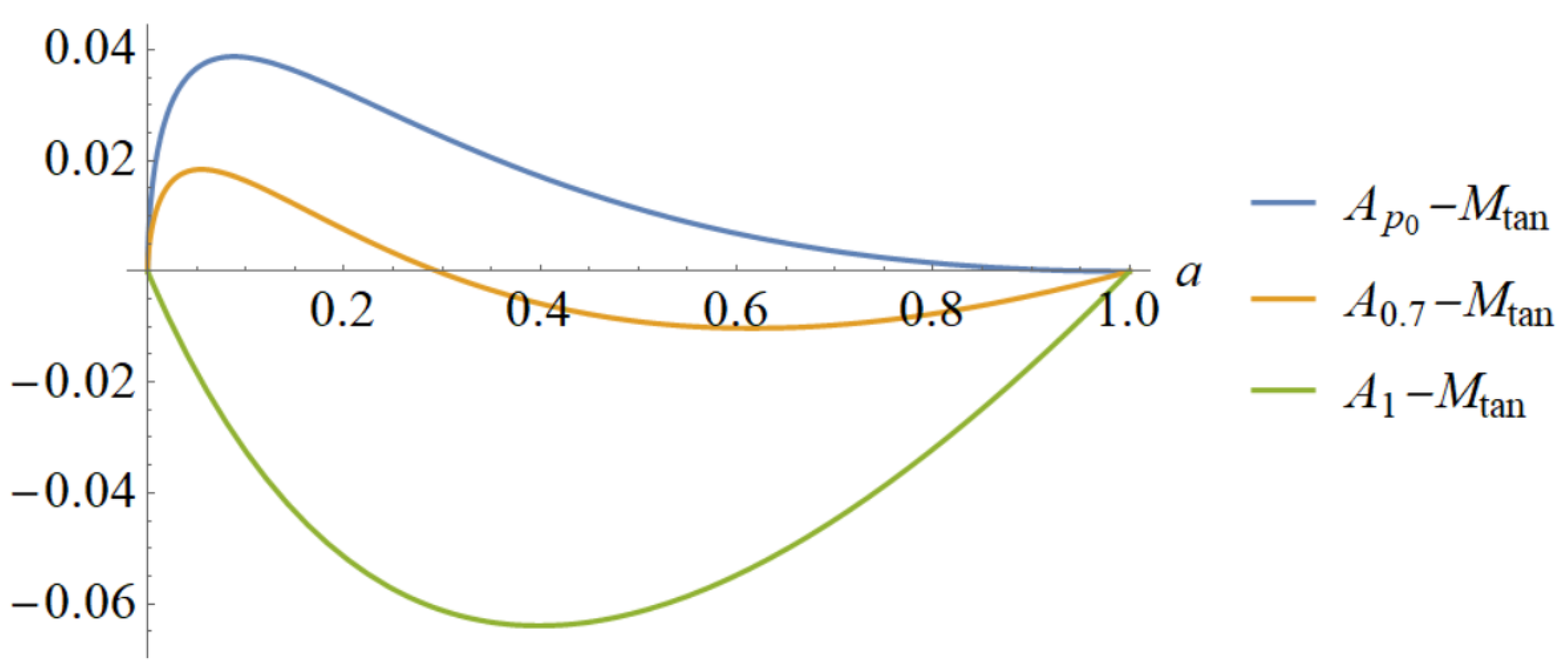
4. Chains of Inequalities for Means
5. Concluding Remarks
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Bullen, P.S.; Mitrinović, D.S.; Vasić, P.M. Means and Their Inequalities; D. Reidel: Dordrecht, The Netherlands, 1988. [Google Scholar]
- Yang, Z.-H. On the log-convexity of two-parameter homogeneous functions. Math. Inequal. Appl. 2007, 10, 499–516. [Google Scholar] [CrossRef]
- Yang, Z.-H. Estimates for Neuman-Sándor mean by power means and their relative errors. J. Math. Inequal. 2013, 7, 711–726. [Google Scholar] [CrossRef]
- Alzer, H.; Janous, W. Solution of problem 8*. Crux Math. 1987, 13, 173–178. [Google Scholar]
- Lin, T.P. The power mean and the logarithmic mean. Am. Math. Mon. 1974, 81, 879–883. [Google Scholar] [CrossRef]
- Stolarsky, K.B. The power and generalized logarithmic means. Am. Math. Mon. 1980, 87, 545–548. [Google Scholar] [CrossRef]
- Pittenger, A.O. Inequalities between arithmetic and logarithmic means. Univ. Beograd. Publ. Elektrotehn. Fak. Ser. Mat. Fiz. 1980, No.678–No.715, 15–18. Available online: https://www.jstor.org/stable/43667864 (accessed on 3 September 2023).
- Seiffert, H.-J. Werte zwischen dem geometrischen und dem arithmetischen Mittel zweier Zahlen. El. Math. 1987, 42, 105–107. (In German) [Google Scholar]
- Seiffert, H.-J. Aufgabe β16. Die Wurzel 1995, 29, 221–222. (In German) [Google Scholar]
- Neuman, E.; Sándor, J. On the Schwab-Borchardt mean II. Math. Pannon. 2006, 17, 49–59. Available online: http://eudml.org/doc/222546 (accessed on 3 September 2023).
- Jagers, A.A. Solution of problem 887. Nieuw Arch. Wiskd. (4) 1994, 12, 230–231. [Google Scholar]
- Hästö, P.A. A monotonicity property of ratios of symmetric homogeneous means. J. Inequal. Pure Appl. Math. 2002, 3, 71. Available online: http://eudml.org/doc/123054 (accessed on 3 September 2023).
- Hästö, P.A. Optimal inequalities between Seiffert’s mean and power mean. Math. Inequal. Appl. 2004, 7, 47–53. [Google Scholar] [CrossRef]
- Li, Y.-M.; Wang, M.-K.; Chu, Y.-M. Sharp power mean bounds for Seiffert mean. Appl. Math. J. Chin. Univ. 2014, 29, 101–107. [Google Scholar] [CrossRef]
- Costin, I.; Toader, G. Optimal evaluations of some Seiffert-type means by power means. Appl. Math. Comput. 2013, 219, 4745–4754. [Google Scholar] [CrossRef]
- Chu, Y.-M.; Long, B.-Y. Bounds of the Neuman-Sándor mean using power and identric means. Abstr. Appl. Anal. 2013, 2013, 832591. [Google Scholar] [CrossRef]
- Yang, Z.-H. Three families of two-parameter means constructed by trigonometric functions. J. Inequal. Appl. 2013, 2013, 541. [Google Scholar] [CrossRef]
- Yang, Z.-H.; Wu, L.-M.; Chu, Y.-M. Optimal power mean bounds for Yang mean. J. Inequal. Appl. 2014, 2014, 401. [Google Scholar] [CrossRef]
- Li, J.-F.; Yang, Z.-H.; Chu, Y.-M. Optimal power mean bounds for the second Yang mean. J. Inequal. Appl. 2016, 2016, 31. [Google Scholar] [CrossRef]
- Borwein, J.M.; Borwein, P.B. Pi and the AGM—A Study in Analytic Number Theory and Computational Complexity; John Wiley & Sons: New York, NY, USA, 1987. [Google Scholar]
- Toader, G. Some mean values related to the arithmetic-geometric mean. J. Math. Anal. Appl. 1998, 218, 358–368. [Google Scholar] [CrossRef]
- Qiu, S.-L.; Shen, J.-M. On two problems concerning means. J. Hangzhou Inst. Electr. Eng. 1997, 17, 1–7. (In Chinese) [Google Scholar] [CrossRef]
- Qiu, S.-L. The Muir mean and the complete elliptic integral of the second kind. J. Hangzhou Inst. Electr. Eng. 2000, 20, 28–33. (In Chinese) [Google Scholar] [CrossRef]
- Alzer, H.; Qiu, S.-L. Monotonicity theorems and inequalities for the complete elliptic integrals. J. Comput. Appl. Math. 2004, 172, 289–312. [Google Scholar] [CrossRef]
- Yang, Z.-H.; Chu, Y.-M. On approximating the modified Bessel function of the first kind and Toader-Qi mean. J. Inequal. Appl. 2016, 2016, 40. [Google Scholar] [CrossRef]
- Sándor, J. Two sharp inequalities for trigonometric and hyperbolic functions. Math. Inequal. Appl. 2012, 15, 409–413. [Google Scholar] [CrossRef]
- Zhao, T.-H.; Qian, W.-M.; Song, Y.-Q. Optimal bounds for two Sándor-type means in terms of power means. J. Inequal. Appl. 2016, 2016, 64. [Google Scholar] [CrossRef]
- He, X.-H.; Qian, W.-M.; Xu, H.-Z.; Chu, Y.-M. Sharp power mean bounds for two Sándor–Yang means. RACSAM 2019, 113, 2627–2638. [Google Scholar] [CrossRef]
- Witkowski, A. On Seiffert-like means. J. Math. Inequal. 2015, 9, 1071–1092. [Google Scholar] [CrossRef]
- Nowicka, M.; Witkowski, A. Optimal bounds for the tangent and hyperbolic sine means. Aequat. Math. 2020, 94, 817–827. [Google Scholar] [CrossRef]
- Nowicka, M.; Witkowski, A. Optimal bounds for the tangent and hyperbolic sine means II. J. Math. Inequal. 2020, 14, 23–33. [Google Scholar] [CrossRef]
- Zhu, L. Optimal bounds for two Seiffert-like means in exponential type. J. Math. Anal. Appl. 2022, 505, 125475. [Google Scholar] [CrossRef]
- Zhu, L.; Malešević, B. Optimal bounds for two Seiffert-like means by arithmetic mean and harmonic mean. RACSAM 2023, 117, 59. [Google Scholar] [CrossRef]
- Nowicka, M.; Witkowski, A. Optimal bounds for the sine and hyperbolic tangent means IV. RACSAM 2021, 115, 79. [Google Scholar] [CrossRef]
- Nowicka, M.; Witkowski, A. Optimal bounds of classical and non-classical means in terms of Q means. RACSAM 2022, 116, 11. [Google Scholar] [CrossRef]
- Zhu, L. Optimal bounds of exponential type for arithmetic mean by Seiffert-like mean and centroidal mean. RACSAM 2022, 116, 1. [Google Scholar] [CrossRef]
- Anderson, G.D.; Vamanamurthy, M.; Vuorinen, M. Monotonicity rules in calculus. Am. Math. Mon. 2006, 113, 805–816. [Google Scholar] [CrossRef]
- Yang, Z.-H. A new way to prove L’Hospital Monotone Rules with applications. arXiv 2014, arXiv:1409.6408. [Google Scholar]
- Tian, J.-F.; Ha, M.-H.; Xing, H.-J. Properties of the power-mean and their applications. AIMS Math. 2020, 5, 7285–7300. [Google Scholar] [CrossRef]
- Biernacki, M.; Krzyż, J. On the monotonicity of certain functionals in the theory of analytic functions. Ann. Univ. Mariae Curie-Skłodowska Sect. A. 1955, 9, 135–147. [Google Scholar]
- Yang, Z.-H.; Chu, Y.-M.; Wang, M.-K. Monotonicity criterion for the quotient of power series with applications. J. Math. Anal. Appl. 2015, 428, 587–604. [Google Scholar] [CrossRef]
- Yang, Z.-H.; Tian, J.-F. Sharp inequalities for the generalized elliptic integrals of the first kind. Ramanujan J. 2019, 48, 91–116. [Google Scholar] [CrossRef]
- Wang, M.-K.; Chu, Y.-M.; Song, Y.-Q. Asymptotical formulas for Gaussian and generalized hypergeometric functions. Appl. Math. Comput. 2016, 276, 44–60. [Google Scholar] [CrossRef]
- Wang, M.-K.; Chu, Y.-M. Refinements of transformation inequalities for zero-balanced hypergeometric functions. Acta Math. Sci. 2017, 37, 607–622. [Google Scholar] [CrossRef]
- Wang, M.-K.; Chu, Y.-M. Landen inequalities for a class of hypergeometric functions with applications. Math. Inequal. Appl. 2018, 21, 521–537. [Google Scholar] [CrossRef]
- Zhu, L. On Frame’s inequalities. J. Inequal. Appl. 2018, 2018, 94. [Google Scholar] [CrossRef]
- Wang, M.-K.; Chu, Y.-M.; Zhang, W. Monotonicity and inequalities involving zero-balanced hypergeometric function. Math. Inequal. Appl. 2019, 22, 601–617. [Google Scholar] [CrossRef]
- Qiu, S.-L.; Ma, X.-Y.; Chu, Y.-M. Sharp Landen transformation inequalities for hypergeometric functions, with applications. J. Math. Anal. Appl. 2019, 474, 1306–1337. [Google Scholar] [CrossRef]
- Zhao, T. Sharp approximations for complete p-elliptic integral of the second kind by weighted power means. Bull. Malays. Math. Sci. Soc. 2023, 46, 126. [Google Scholar] [CrossRef]
- Zhao, T.-H.; He, Z.-Y.; Chu, Y.-M. Sharp bounds for the weighted Hölder mean of the zero-balanced generalized complete elliptic integrals. Comput. Methods Funct. Theory 2021, 21, 413–426. [Google Scholar] [CrossRef]
- Gaunt, R.E. Functional inequalities and monotonicity results for modified Lommel functions of the first kind. Results Math. 2022, 77, 1. [Google Scholar] [CrossRef]
- Zhao, T.-H.; Wang, M.-K. Sharp bounds for the lemniscatic mean by the weighted Hölder mean. RACSAM 2023, 117, 96. [Google Scholar] [CrossRef]
- Abramowitz, M.; Stegun, I.A. (Eds.) Handbook of Mathematical Functions with Formulas, Graphs, and Mathematical Tables; National Bureau of Standards; Applied Mathematics Series 55; 10th Printing; U.S. Government Printing Office: Washington, DC, USA, 1972.
- Qi, F. A double inequality for the ratio of two non-zero neighbouring Bernoulli numbers. J. Comput. Appl. Math. 2019, 351, 1–5. [Google Scholar] [CrossRef]
- Zhu, L. New bounds for the ratio of two adjacent even-indexed Bernoulli numbers. RACSAM 2020, 114, 83. [Google Scholar] [CrossRef]
- Yang, Z.-H.; Chu, Y.-M. An optimal inequalities chain for bivariate means. J. Math. Inequal. 2015, 9, 331–343. [Google Scholar] [CrossRef]
- Yang, Z.-H.; Chu, Y.-M. Inequalities for certain means in two arguments. J. Inequal. Appl. 2015, 2015, 299. [Google Scholar] [CrossRef]

Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Yang, Z.; Zhang, J. Sharp Power Mean Bounds for Two Seiffert-like Means. Axioms 2023, 12, 910. https://doi.org/10.3390/axioms12100910
Yang Z, Zhang J. Sharp Power Mean Bounds for Two Seiffert-like Means. Axioms. 2023; 12(10):910. https://doi.org/10.3390/axioms12100910
Chicago/Turabian StyleYang, Zhenhang, and Jing Zhang. 2023. "Sharp Power Mean Bounds for Two Seiffert-like Means" Axioms 12, no. 10: 910. https://doi.org/10.3390/axioms12100910
APA StyleYang, Z., & Zhang, J. (2023). Sharp Power Mean Bounds for Two Seiffert-like Means. Axioms, 12(10), 910. https://doi.org/10.3390/axioms12100910







