Head Waves in Modified Weiskopf Sandy Medium
Abstract
:1. Introduction
1.1. Weiskopf Sandy Model
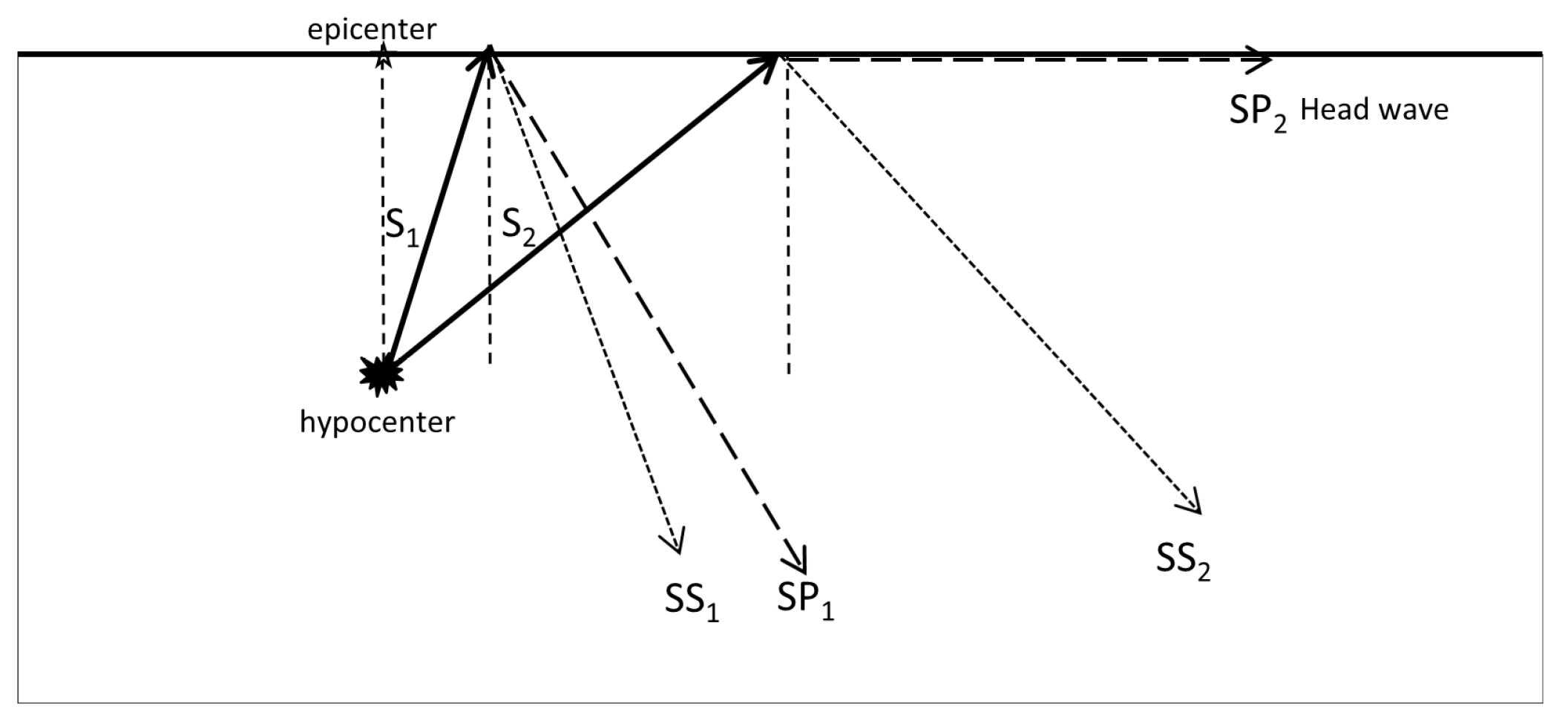
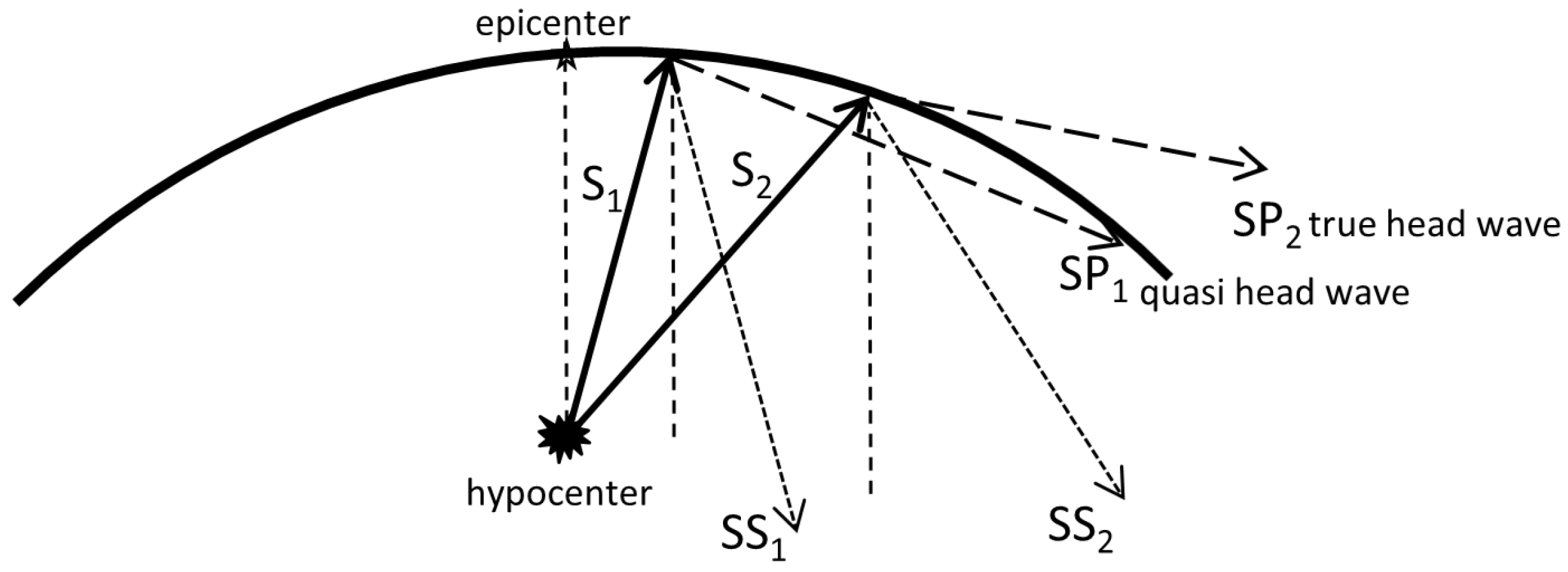
1.2. Head SP Waves
1.3. Current Research
2. The Modified Weiskopf Model
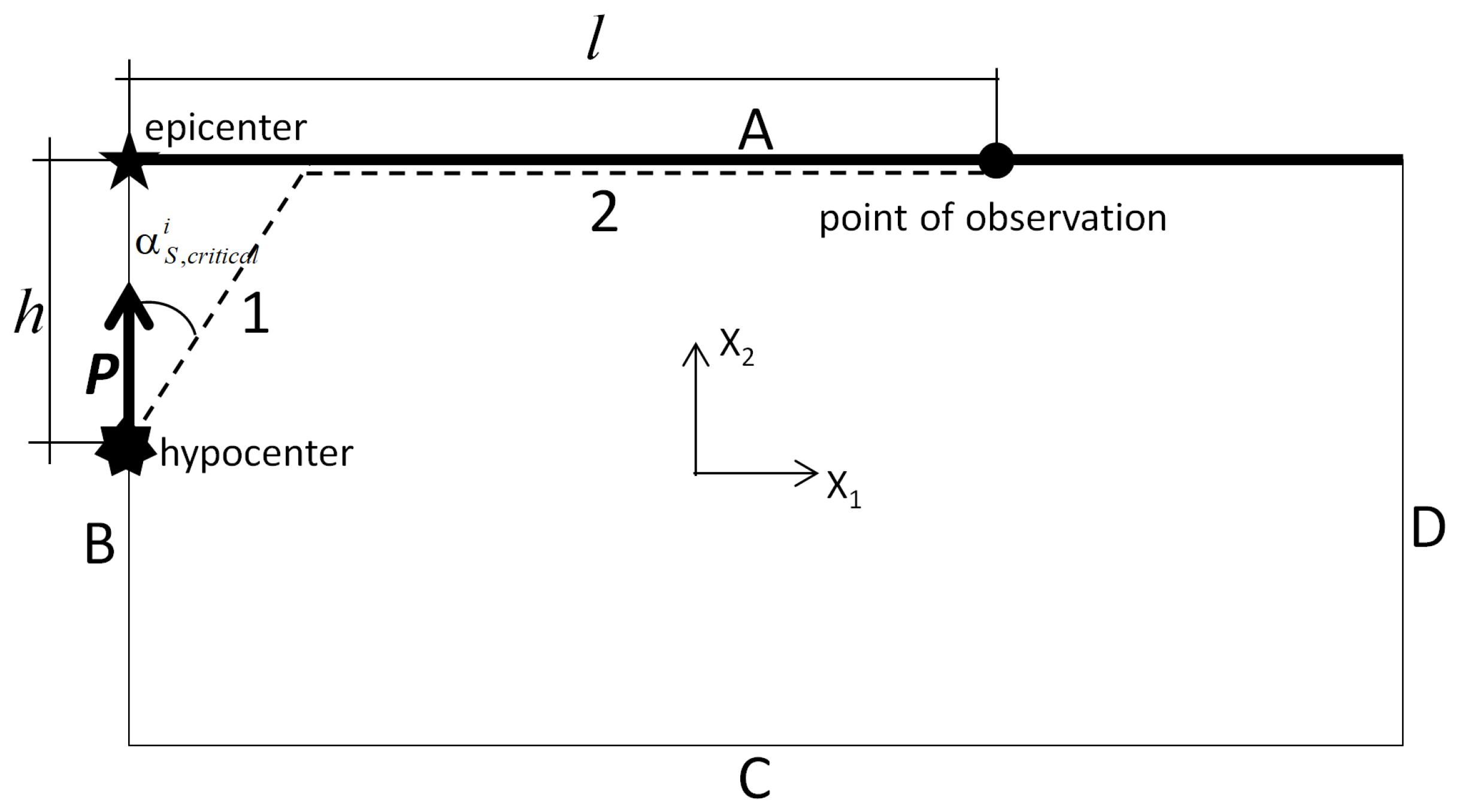
3. Mathematical Model
3.1. Inner Plain Lamb Problem
3.2. Boundary Conditions
3.3. Initial Conditions 22
3.4. Force Loading
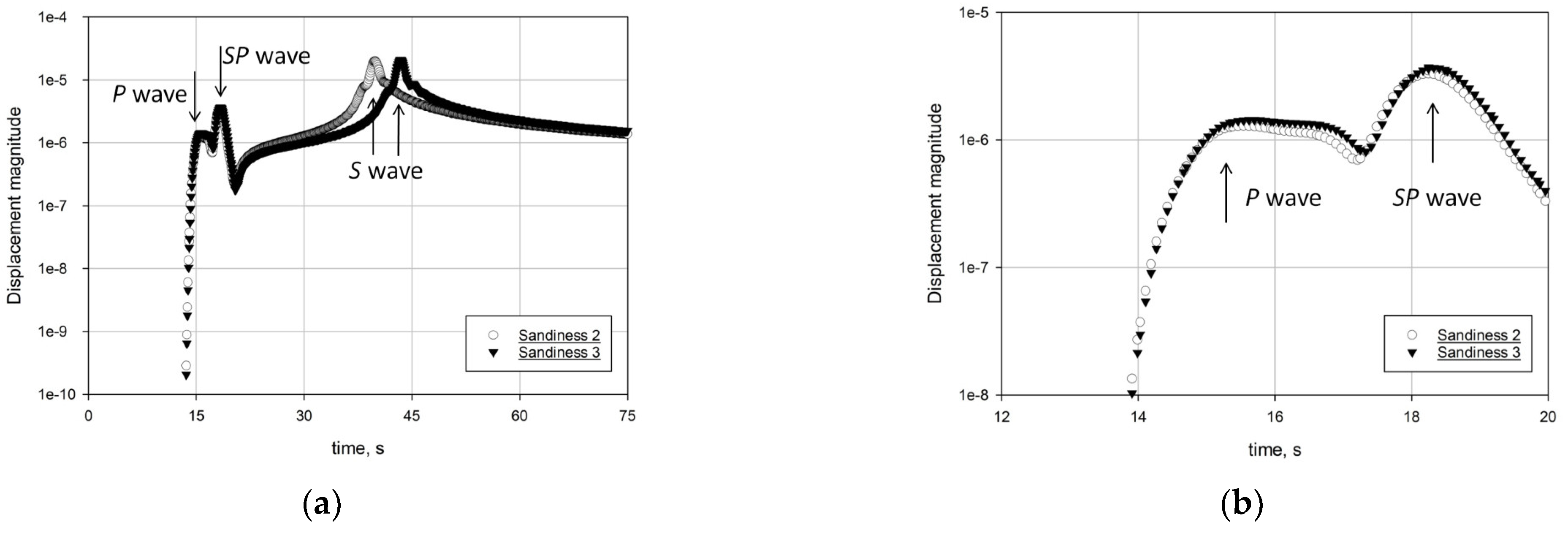
3.5. Numerical Implementation
4. Concluding Remarks
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Weiskopf, W.H. Stresses in soils under a foundation. J. Franklin Inst. 1945, 239, 445–465. [Google Scholar] [CrossRef]
- Pentis, E.A.; White, L. Underpinning, Its Practice and Applications; Columbia University Press: New York, NY, USA, 1931. [Google Scholar]
- Cernica, J.N. Geotechnical Engineering: Foundation Design; John Wiley & Sons, Inc.: New York, NY, USA, 1995. [Google Scholar]
- Georgiadis, M.; Anagnostopoulos, C. Lateral pressure on sheet pile walls due to strip load. J. Geotech. Geoenviron. Eng. 1998, 124, 95–98. [Google Scholar] [CrossRef]
- Gotoh, K.; Yamanouchi, T. Stress and displacement characteristics of soil mass as Weiskopf’s body due to surface load. Soils Found. 1979, 19, 97–107. (In Japanese) [Google Scholar] [CrossRef]
- Misra, B. Lateral pressures on retaining walls due to loads on surfaces of granular backfills. Soils Found. 1981, 20, 33–44. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Motta, E. Generalized Coulomb active-earth pressure for distanced surcharge. J. Geotech. Eng. 1994, 120, 1072–1079. [Google Scholar] [CrossRef]
- Tsvankin, I. Properties of evanescent waves in anisotropic media. J. Seism. Explor. 2008, 17, 67–74. [Google Scholar]
- Goldshtein, M.N. Some problems in soil mechanics and foundation engineering today. Soil Mech. Found. Eng. 1971, 8, 86–90. [Google Scholar] [CrossRef]
- Deep, S.; Sharma, V. Love type waves in a dry sandy layer lying over an isotropic elastic halfspace with imperfect interface. J. Phys. Conf. Ser. 2020, 1531, 012069. [Google Scholar] [CrossRef]
- Dey, S.; Gupta, A.K.; Gupta, S. Propagation of torsional surface waves in dry sandy medium under gravity. Math. Mech. Solids 1998, 3, 229–235. [Google Scholar] [CrossRef]
- Gupta, S.; Ahmed, M. On propagation of Love waves in dry sandy medium sandwiched between fiber-reinforced layer and prestressed porous half-space. Earthq. Struct. 2017, 12, 619–628. [Google Scholar]
- Pal, P.C.; Kumar, S.; Mandal, D. Surface wave propagation in sandy layer overlying a liquid saturated porous half-space and lying under a uniform liquid layer. Mech. Adv. Mater. Struct. 2014, 23, 59–65. [Google Scholar] [CrossRef]
- Pandit, D.K.; Kundu, S. Propagation of Love wave in viscoelastic sandy medium lying over pre-stressed orthotropic half-space. Procedia Eng. 2017, 173, 996–1002. [Google Scholar] [CrossRef]
- Dey, S.; Chandra, A. Surface waves in a dry sandy medium under gravity. Acta Geophys. Pol. 1983, 31, 395–404. [Google Scholar]
- Kakar, R.; Kakar, S. Rayleigh wave in an anisotropic heterogeneous crustal layer lying over a gravitational sandy substratum. Geomech. Eng. 2016, 10, 137–154. [Google Scholar] [CrossRef]
- Pal, P.C.; Kumar, S.; Bose, S. Propagation of Rayleigh waves in anisotropic layer overlying a semi-infinite sandy medium. Ain Shams Eng. J. 2015, 6, 621–627. [Google Scholar] [CrossRef] [Green Version]
- Kumar, P.; Chattopadhyay, A.; Singh, A.K. Propagation of edge wave in homogeneous viscoelastic sandy media. In Advances in Structural Vibration. Lecture Notes in Mechanical Engineering; Dutta, S., Inan, E., Dwivedy, S.K., Eds.; Springer: Singapore, 2021. [Google Scholar] [CrossRef]
- Aki, K.; Richards, P.G. Quantitative Seismology Theory and Methods; W.H. Freeman & Co.: New York, NY, USA, 1980. [Google Scholar]
- Cerveny, V.; Ravindra, R. Theory of Seismic Head Waves; University of Toronto Press: Toronto, ON, Canada, 1971. [Google Scholar]
- Doruelo, J.; Hilterman, F.; Goloshubin, G. Head Waves as Mechanism for Azimuthal PP AVO Magnitude Anomalies; SEG Technical Program Expanded Abstracts; Society of Exploration Geophysicists: Houston, TX, USA, 2006; pp. 199–203. [Google Scholar]
- Angelsky, O.V.; Zenkova, C.Y.; Hanson, S.G.; Zheng, J. Extraordinary manifestation of evanescent wave in biomedical application. Front. Phys. 2020, 8, 159. [Google Scholar] [CrossRef]
- Somekh, M.G.; Pechprasarn, S. Surface Plasmon, Surface Wave, and Enhanced Evanescent Wave Microscopy. In Handbook of Photonics for Biomedical Engineering; Ho, A.P., Kim, D., Somekh, M., Eds.; Springer: Dordrecht, The Netherlands, 2016. [Google Scholar]
- Djeran-Maigre, I.; Kuznetsov, S.V. Velocities, dispersion, and energy of SH-waves in anisotropic laminated plates. Acoust. Phys. 2014, 60, 200–207. [Google Scholar] [CrossRef]
- Dudchenko, A.V.; Dias, D.; Kuznetsov, S.V. Vertical wave barriers for vibration reduction. Arch. Appl. Mech. 2021, 91, 257–276. [Google Scholar] [CrossRef]
- Goldshtein, R.V.; Dudchenko, A.V.; Kuznetsov, S.V. The modified Cam-Clay (MCC) model: Cyclic kinematic deviatoric loading. Arch. Appl. Mech. 2016, 86, 2021–2031. [Google Scholar] [CrossRef]
- Goldshtein, R.V.; Kuznetsov, S.V. Long-wave asymptotics of Lamb waves. Mech. Solids 2017, 52, 700–707. [Google Scholar] [CrossRef]
- Ilyashenko, A.V.; Kuznetsov, S.V. Theoretical aspects of applying Lamb waves in nondestructive testing of anisotropic media. Russ. J. Nondestruct. Test. 2017, 53, 243–259. [Google Scholar] [CrossRef]
- Pekeris, C.L. The seismic buried pulse. Proc. Natl. Acad. Sci. USA 1955, 41, 629–639. [Google Scholar] [CrossRef] [PubMed]
- Pekeris, C.L.; Lifson, H. Motion of the surface of a uniform elastic half-space produced by a burried pulse. J. Acoust. Soc. Am. 1957, 29, 1233–1238. [Google Scholar] [CrossRef]
- Garvin, W.W. Exact transient solution of the buried line source problem. Proc. R. Soc. Lond. Ser. A 1956, 234, 528–541. [Google Scholar]
- Kausel, E.; Manolis, G. Wave Motion in Earthquake Engineering; WIT Press: Southampton, UK, 1999. [Google Scholar]
- Kausel, E. Lamb’s problem at its simplest. Proc. R. Soc. A Math. Phys. Eng. Sci. 2012, 469, 20120462. [Google Scholar] [CrossRef]
- Sánchez-Sesma, F.; Iturrarán-Viveros, U. The classic Garvin’s problem revisited. Bull. Seism. Soc. Am. 2006, 96, 1344–1351. [Google Scholar] [CrossRef]
- Kuznetsov, S.V. Fundamental and singular solutions of Lamé equations for media with arbitrary elastic anisotropy. Q. Appl. Math. 2005, 63, 455–467. [Google Scholar] [CrossRef] [Green Version]
- Kuznetsov, S.V.; Terentjeva, E.O. Planar internal Lamb problem: Waves in the epicentral zone of a vertical power source. Acoust. Phys. 2015, 61, 356–367. [Google Scholar] [CrossRef]
- Moczo, P.; Kristek, J.; Vavrycuk, V.; Archuleta, R.J.; Halada, L. 3D heterogeneous staggered-grid finite-difference modeling of seismic motion with volume harmonic and arithmetic averaging of elastic moduli and densities. Bull. Seism. Soc. Am. 2002, 92, 3042–3066. [Google Scholar] [CrossRef]
- Shuo, M.; Archuleta, R.J.; Liu, P. Hybrid modeling of elastic P-SV wave motion: A combined finite-element and staggered-grid finite-difference approach. Bull. Seism. Soc. Am. 2004, 94, 1557–1563. [Google Scholar]
- Kuznetsov, S.V. Detection of a hidden sandy layer in a stratified substrate by dispersion analysis. In Mechanics of High-Contrast Elastic Solids; Contributions from Euromech Colloquium 626; Springer International Publishing: Cham, Switzerland, 2023; pp. 119–132. [Google Scholar]
- Gurtin, M.E. The Linear Theory of Elasticity. In Linear Theories of Elasticity and Thermoelasticity; Truesdell, C., Ed.; Springer: Berlin/Heidelberg, Germany, 1973. [Google Scholar]
- Li, S.; Brun, M.; Djeran-Maigre, I.; Kuznetsov, S. Explicit/implicit multi-time step co-simulation in unbounded medium with Rayleigh damping and application for wave barrier. Eur. J. Environ. Civ. Eng. 2018, 24, 2400–2421. [Google Scholar] [CrossRef]
- Li, S.; Brun, M.; Djeran-Maigre, I.; Kuznetsov, S. Hybrid asynchronous absorbing layers based on Kosloff damping for seismic wave propagation in unbounded domains. Comp. Geotech. 2019, 109, 69–81. [Google Scholar] [CrossRef]
- Fathi, A.; Poursartip, B.; Kallivokas, L. Time-domain hybrid formulations for wave simulations in three-dimensional PML-truncated heterogeneous media. Int. J. Numer. Meth. Eng. 2015, 101, 165–198. [Google Scholar] [CrossRef]
- Pettit, J.; Walker, A.; Cawley, P.; Lowe, M. A stiffness reduction method for efficient absorption of waves at boundaries for use in commercial finite element codes. Ultrasonics 2014, 54, 1868–1879. [Google Scholar] [CrossRef] [PubMed]
- Lax, P.D. Hyperbolic Systems of Conservation Laws and the Mathematical Theory of Shock Waves; SIAM: Philadelphia, PL, USA, 1972. [Google Scholar]
- Lax, P.D.; Wendroff, B. Difference schemes for hyperbolic equations with high order of accuracy. Commun. Pure Appl. Math. 1964, 17, 381–398. [Google Scholar] [CrossRef]
- Winnicki, I.; Jasinski, J.; Pietrek, S. New approach to the Lax-Wendroff modified differential equation for linear and nonlinear advection. Numer. Methods Partial. Differ. Equ. 2019, 35, 2275–2304. [Google Scholar] [CrossRef] [Green Version]
- Zubeldia, M. Energy concentration and explicit Sommerfeld radiation condition for the electromagnetic Helmholtz equation. J. Funct. Anal. 2012, 263, 2832–2862. [Google Scholar] [CrossRef] [Green Version]
- Rathod, H.D. Some analytical integration formulae for a four node isoparametric element. Comput. Struct. 1988, 30, 1101–1109. [Google Scholar] [CrossRef]
- Finlayson, B.A. Numerical Methods for Problems with Moving Fronts; Ravenna Park Pub: Seattle, WT, USA, 1992. [Google Scholar]
- Craster, R.V.; Joseph, L.M.; Kaplunov, J. Long-wave asymptotic theories: The connection between functionally graded waveguides and periodic media. Wave Motion 2014, 51, 581–588. [Google Scholar] [CrossRef] [Green Version]
- Djeran-Maigre, I.; Kuznetsov, S. Solitary SH waves in two-layered traction-free plates. Comptes Rendus Mécanique 2008, 336, 102–107. [Google Scholar] [CrossRef]
- Kuznetsov, S.V. Low frequency limits for Lamb waves in homogeneous, stratified and functionally graded anisotropic plates. Mech. Adv. Mater. Struct. 2022, 29, 4252–4258. [Google Scholar] [CrossRef]
- Aloisio, A.; Totani, F.; Alaggio, R.; Totani, G. Dispersion curves of transverse waves propagating in multi-layered soils from experimental tests in a 100 m deep borehole. Geosciences 2021, 11, 207. [Google Scholar] [CrossRef]
- Palmer, D. An introduction to the generalized reciprocal method of seismic refraction interpretation. Geophysics 1981, 46, 1508–1518. [Google Scholar] [CrossRef]
- Briickl, E. The interpretation of traveltime fields in refraction seismology. Geophys. Prospect. 1987, 35, 973–992. [Google Scholar] [CrossRef]
- Merzer, A.M. Head waves, normal waves, and the shapes of transition layers. Geophys. J. Int. 1974, 37, 1–7. [Google Scholar] [CrossRef]
- Coulombel, J.-F.; Williams, M. Amplification of pulses in nonlinear geometric optics. J. Hyperbolic Differ. Equ. 2014, 11, 749–793. [Google Scholar] [CrossRef]
- Berryman, J.G. Seismic velocity decrement ratios for regions of partial melt in the lower mantle. Geophys. Res. Lett. 2000, 27, 421–424. [Google Scholar] [CrossRef]
- Braile, L.W. Crustal structure of the continental interior, in Geophysical Framework of the Continental United States. Mem. Geol. Soc. Am. 1989, 172, 285–315. [Google Scholar]
- Buffett, B.A.; Garnero, E.J.; Jeanloz, R. Sediments and the top of Earth’s core. Science 2000, 288, 2007–2012. [Google Scholar] [CrossRef]
- Cagniard, L. Reflection and Refraction of Progressive Seismic Waves; Flinn, E.A., Dix, C.H., Eds.; McGraw-Hill: New York, NY, USA, 1962. [Google Scholar]
- Choy, G.L. Theoretical seismograms of core phases calculated by frequency-dependent full wave theory, and their interpretation. Geophys. J. R. Astron. Soc. 1977, 51, 275–312. [Google Scholar] [CrossRef] [Green Version]
- Davies, D.; Kelly, E.J.; Filson, J.R. Vespa process for analysis of seismic signals. Nat. Phys. Sci. 1971, 232, 1971. [Google Scholar] [CrossRef]
- de Hoop, A.T. A modification of Cagniard’s method for solving seismic pulse problems. Appl. Sci. Res. Ser. B 1960, 8, 349–356. [Google Scholar] [CrossRef]
- Dziewonski, A.M.; Anderson, D.L. Preliminary reference Earth model (PREM). Phys. Earth Planet. Inter. 1981, 25, 297–356. [Google Scholar] [CrossRef]
- Frederiksen, A.W.; Bostock, M.G. Modelling teleseismic waves in dipping anisotropic structures. Geophys. J. Int. 2000, 141, 401–412. [Google Scholar] [CrossRef] [Green Version]
- Fuchs, K.; Muller, G. Computation of synthetic seismograms with the reflectivity method and comparison with observations. Geophys. J. R. Astron. Soc. 1971, 23, 417–433. [Google Scholar] [CrossRef] [Green Version]
- Garnero, E.J.; Helmberger, D.V. A very slow basal layer underlying large-scale low-velocity anomalies in the lower mantle beneath the Pacific—Evidence from core phases. Phys. Earth Planet. Inter. 1995, 91, 161–176. [Google Scholar] [CrossRef]
- Wang, L.; Zhou, Y.; Zhow, S.; Zhang, H. Detection of fault zone head waves and the fault interface imaging in the Xianshuihe–Anninghe Fault zone (Eastern Tibetan Plateau). Geophysi. J. Int. 2023, 234, 1157–1167. [Google Scholar] [CrossRef]
- Li, Z.; Peng, Z. Automatic identification of fault zone head waves and direct P waves and its application in the Parkfield section of the San Andreas Fault, California. Geophys. J. Int. 2016, 205, 1326–1341. [Google Scholar] [CrossRef] [Green Version]
- Nardoni, C.; De Siena, L.; Magrini, F.; Cammarano, F.; Maeda, T.; Mattei, E. Earthquake characteristics and structural properties of the Southern Tyrrhenian Basin from full seismic wave simulations. Surv. Geophys. 2023, 44, 925–945. [Google Scholar] [CrossRef]
- Zhao, P.; Peng, Z.; Shi, Z.; Lewis, M.; Ben-Zion, Y. Variations of the velocity contrast and rupture properties of M6 earthquakes along the Parkfield section of the San Andreas fault. Geophys. J. Int. 2010, 180, 765–780. [Google Scholar] [CrossRef] [Green Version]
- Ben-Zion, Y.; Malin, P. San Andreas fault zone head waves near Parkfield, California. Science 1991, 251, 1592–1594. [Google Scholar] [CrossRef] [PubMed]
- Bennington, N.; Thurber, C.; Feigl, K.; Murray-Moraleda, J. Aftershock distribution as a constraint on the geodetic model of coseismic slip for the 2004 Parkfield earthquake. Pure Appl. Geophys. 2011, 168, 1553–1565. [Google Scholar] [CrossRef]
- Kurzweil, L.G. Ground-borne noise and vibration from underground rail systems. J. Sound Vib. 1979, 66, 363–370. [Google Scholar] [CrossRef]
- Di, H.; Zhou, S.; Luo, Z.; He, C.; Xiao, J.; Li, X. A vehicle-track-tunnel-soil model for evaluating the dynamic response of a double-line metro tunnel in a poroelastic half-space. Comp. Geotech. 2017, 101, 245–263. [Google Scholar] [CrossRef]
- Benech, N.; Negreira, C.A. Longitudinal and lateral low frequency head wave analysis in soft media. J. Acoust. Soc. Am. 2005, 117, 3424–3431. [Google Scholar] [CrossRef]
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Karakozova, A.; Kuznetsov, S. Head Waves in Modified Weiskopf Sandy Medium. Axioms 2023, 12, 679. https://doi.org/10.3390/axioms12070679
Karakozova A, Kuznetsov S. Head Waves in Modified Weiskopf Sandy Medium. Axioms. 2023; 12(7):679. https://doi.org/10.3390/axioms12070679
Chicago/Turabian StyleKarakozova, Anastasia, and Sergey Kuznetsov. 2023. "Head Waves in Modified Weiskopf Sandy Medium" Axioms 12, no. 7: 679. https://doi.org/10.3390/axioms12070679
APA StyleKarakozova, A., & Kuznetsov, S. (2023). Head Waves in Modified Weiskopf Sandy Medium. Axioms, 12(7), 679. https://doi.org/10.3390/axioms12070679







