Existence and Asymptotic Stability of the Solution for the Timoshenko Transmission System with Distributed Delay
Abstract
:1. Introduction and Position of Problem
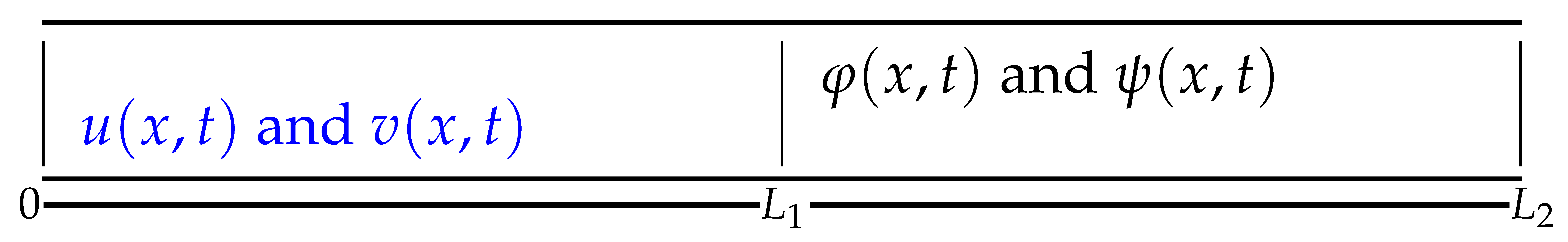
2. Preliminaries and the Main Results
3. Well-Posedness of the Problem
- for all vectors and in ,
4. Asymptotic Behavior
5. Conclusions
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Elhindi, M.; Zennir, K.; Ouchenane, D.; Choucha, A.; El Arwadi, T. Bresse-Timoshenko type systems with thermodiffusion effects: Well-possedness, stability and numerical results. Rend. Circ. Mat. Palermo II. Ser 2023, 72, 169–194. [Google Scholar] [CrossRef]
- Choucha, A.; Ouchenane, D.; Zennir, Kh.; Feng, B. Global well-posedness and exponential stability results of a class of Bresse-Timoshenko-type systems with distributed delay term. Math. Meth. Appl. Sci. 2020. [Google Scholar] [CrossRef]
- Alves, M.S.; Raposo, C.A.; Muñoz Rivera, J.E.; Sepúlveda, M.; Vera Villagrán, O. Uniform stabilization for the transmission problem of the Timoshenko system with memory. J. Math. Anal. Appl. 2010, 369, 323–345. [Google Scholar] [CrossRef]
- Kilbas, A.A.; Srivastava, H.M.; Trujillo, J.J. Theory and Applications of Fractional Differential Equations; Elsevier: Amsterdam, The Netherlands, 2006; Volume 204. [Google Scholar]
- Srivastava, H.M.; Waleed, A.; Izadi, M.; El-Sayed, A.A. Solving Some Physics Problems Involving Fractional-Order Differential Equations with the Morgan-Voyce Polynomials. Fractal Fract. 2023, 7, 301. [Google Scholar] [CrossRef]
- Datko, R. Not all feedback stabilized hyperbolic systems are robust with respect to small time delays in their feedbacks. SIAM J. Control Optim. 1988, 26, 697–713. [Google Scholar] [CrossRef]
- Datko, R.; Lagnese, J.; Polis, M.P. An example on the effect of time delays in boundary feedback stabilization of wave equations. SIAM J. Control Optim. 1986, 24, 152–156. [Google Scholar] [CrossRef]
- Nicaise, A.S.; Pignotti, C. Stabilization of the wave equation with boundary or internal distributed delay. Diff. Int. Equ. 2008, 21, 935–958. [Google Scholar] [CrossRef]
- Xu, G.Q.; Yung, S.P.; Li, L.K. Stabilization of wave systems with input delay in the boundary control. ESAIM Control Optim. Calc. Var. 2006, 12, 770–785. [Google Scholar] [CrossRef]
- Nicaise, S.; Pignotti, C. Stability and instability results of the wave equation with a delay term in the boundary or internal feedbacks. SIAM J. Control Optim. 2006, 45, 1561–1585. [Google Scholar] [CrossRef]
- Muñoz Rivera, J.E.; Oquendo, H.P. The transmission problem of viscoelastic waves. Acta Appl. Math. 2000, 62, 1–21. [Google Scholar] [CrossRef]
- Green, A.E.; Naghdi, P.M. Thermoelasticity without energy dissipation. J. Elast. 1993, 31, 189–208. [Google Scholar] [CrossRef]
- Green, A.E.; Naghdi, P.M. A re-examination of the basic postulates of thermomechanics. Proc. R. Soc. Lond. Ser. A 1991, 432, 171–194. [Google Scholar]
- Ma, T.F.; Oquendo, H.P. A transmission problem for beams on nonlinear supports. Bound. Value Probl. 2006, 14, 75107. [Google Scholar] [CrossRef]
- Marzocchi, A.; Rivera, J.E.M.; Naso, M.G. Asymptotic behavior and exponential stability for a transmission problem in thermoelasticity. Math. Meth. Appl. Sci. 2002, 25, 955–980. [Google Scholar] [CrossRef]
- Marzocchi, A.; Rivera, J.E.M.; Naso, M.G. Transmission problem in thermoelasticity with symmetry. IMA J. Appl. Math. 2002, 63, 23–46. [Google Scholar] [CrossRef]
- Benaissa, A.; Miloudi, M.; Mokhtari, M. Global existence and energy decay of solutions to a Bresse system with delay terms. Comment. Math. Univ. Carolin 2015, 56, 169–186. [Google Scholar] [CrossRef]
- Feng, B. Well-posedness and exponential stability for a plate equation with time-varying delay and past history. Z. Angew. Math. Phys. 2017, 68, 6. [Google Scholar] [CrossRef]
- Liu, G.W.; Yue, H.Y.; Zhang, H.W. Long time Behavior for a wave equation with time delay. Taiwan. J. Math. 2017, 27, 107–129. [Google Scholar] [CrossRef]
- Liu, G.W.; Zhang, H.W. Well-posedness for a class of wave equation with past history and a delay. Z. Angew. Math. Phys. 2016, 67, 1–14. [Google Scholar] [CrossRef]
- Nicaise, S.; Pignotti, C. Exponential stability of abstract evolutions with time delay. J. Evol. Equ. 2015, 15, 107–129. [Google Scholar] [CrossRef]
- Mustafa, M.I. A uniform stability result for thermoelasticity of type III with boundary distributed delay. J. Abstr. Diff. Equ. Appl. 2014, 415, 148–158. [Google Scholar] [CrossRef]
- Bouzettouta, L.; Zitouni, S.; Zennir, K.; Guesmia, A. Stability of bresse system with internal distributed delay. J. Math. Comput. Sci. 2017, 7, 92–118. [Google Scholar]
- Bastos, W.D.; Raposo, C.A. Transmission problem for waves with frictional damping. Electron. J. Differ. Equ. 2007, 2007, 1–10. [Google Scholar]
- Raposo, C.A.; Bastos, W.D.; Santos, M.L. A transmission problem for Timoshenko system. Comput. Appl. Math. 2007, 26, 215–234. [Google Scholar] [CrossRef]
- Raposo, C.A. The transmission problem for Timoshenko’s system of memory type. Int. J. Mod. Math. 2008, 2008, 371–393. [Google Scholar]
- Haraux, A. Two remarks on dissipative hyperbolic problems. In Research Notes in Mathematics no122; Pitman: Boston, MA, USA, 1985; pp. 161–179. [Google Scholar]
- Muñoz Rivera, J.E.; Racke, R. Global stability for damped Timoshenko systems. Discrete Contin. Dyn. Syst. Ser. B 2003, 9, 1625–1639. [Google Scholar] [CrossRef]
- Kamenskii, M.; Fitte, P.R.D.; Wong, N.C.; Zvereva, M. A model of deformations of a discontinuous Stieltjes string with a nonlinear boundary condition. J. Nonlinear Var. Anal. 2021, 5, 737–759. [Google Scholar]
- Kasim, E. The exponential stability of a two-unit system with non-preemptive priority. J. Nonlinear Funct. Anal. 2022, 2022, 28. [Google Scholar]
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Braik, A.; Zennir, K.; Hassan, E.I.; Alfedeel, A.H.A.; M. Mirgani, S. Existence and Asymptotic Stability of the Solution for the Timoshenko Transmission System with Distributed Delay. Axioms 2023, 12, 833. https://doi.org/10.3390/axioms12090833
Braik A, Zennir K, Hassan EI, Alfedeel AHA, M. Mirgani S. Existence and Asymptotic Stability of the Solution for the Timoshenko Transmission System with Distributed Delay. Axioms. 2023; 12(9):833. https://doi.org/10.3390/axioms12090833
Chicago/Turabian StyleBraik, A., Kh. Zennir, E. I. Hassan, A. H. A. Alfedeel, and Safa M. Mirgani. 2023. "Existence and Asymptotic Stability of the Solution for the Timoshenko Transmission System with Distributed Delay" Axioms 12, no. 9: 833. https://doi.org/10.3390/axioms12090833
APA StyleBraik, A., Zennir, K., Hassan, E. I., Alfedeel, A. H. A., & M. Mirgani, S. (2023). Existence and Asymptotic Stability of the Solution for the Timoshenko Transmission System with Distributed Delay. Axioms, 12(9), 833. https://doi.org/10.3390/axioms12090833






